Measurement of the Induced Magnetic Polarisation of Rotated-Domain Graphene Grown on Co Film with Polarised Neutron Reflectivity
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. X-ray Diffraction and X-ray Reflectivity
3.2. Scanning Electron Microscopy
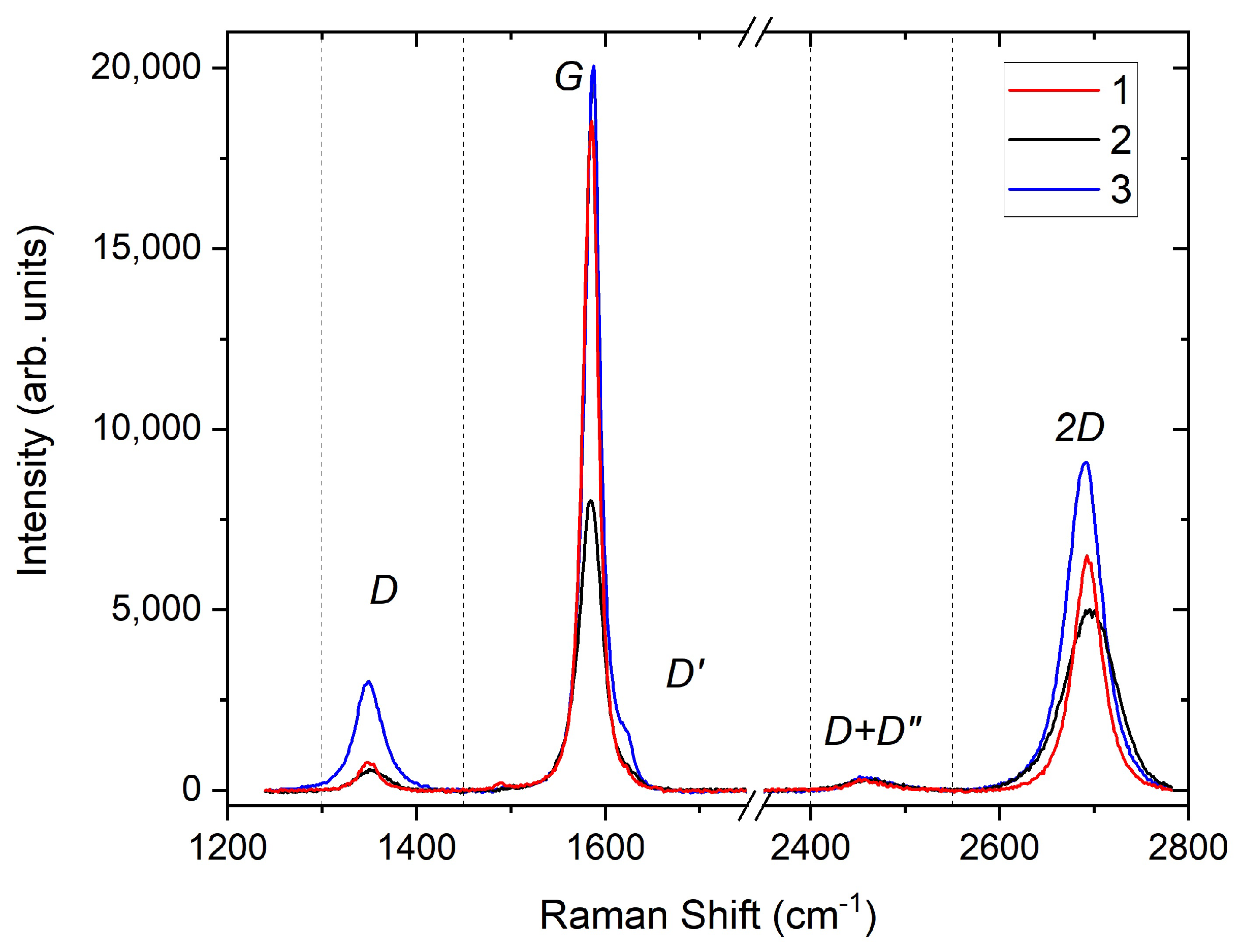
3.3. Raman Spectroscopy
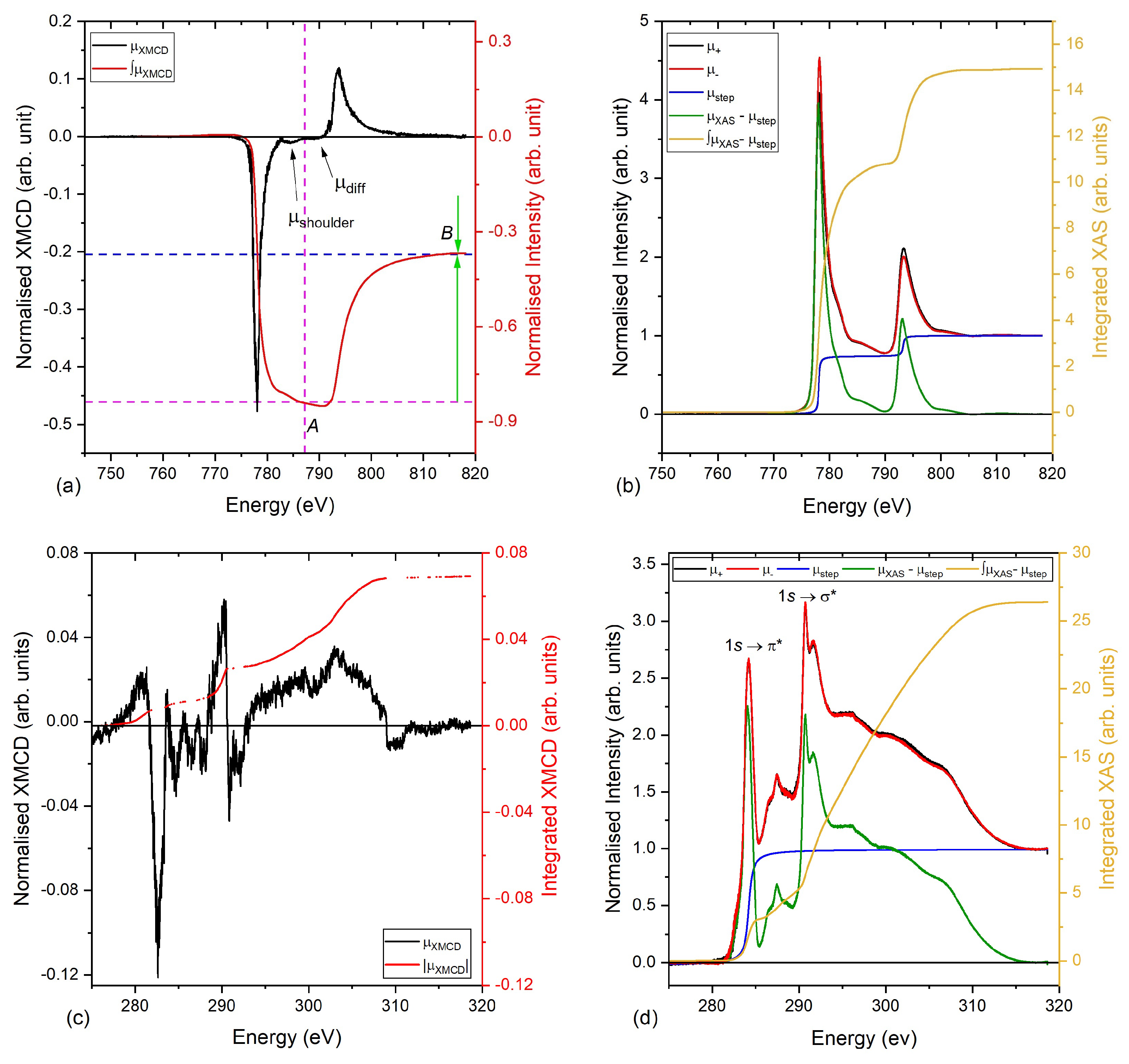
3.4. X-ray Magnetic Circular Dichroism
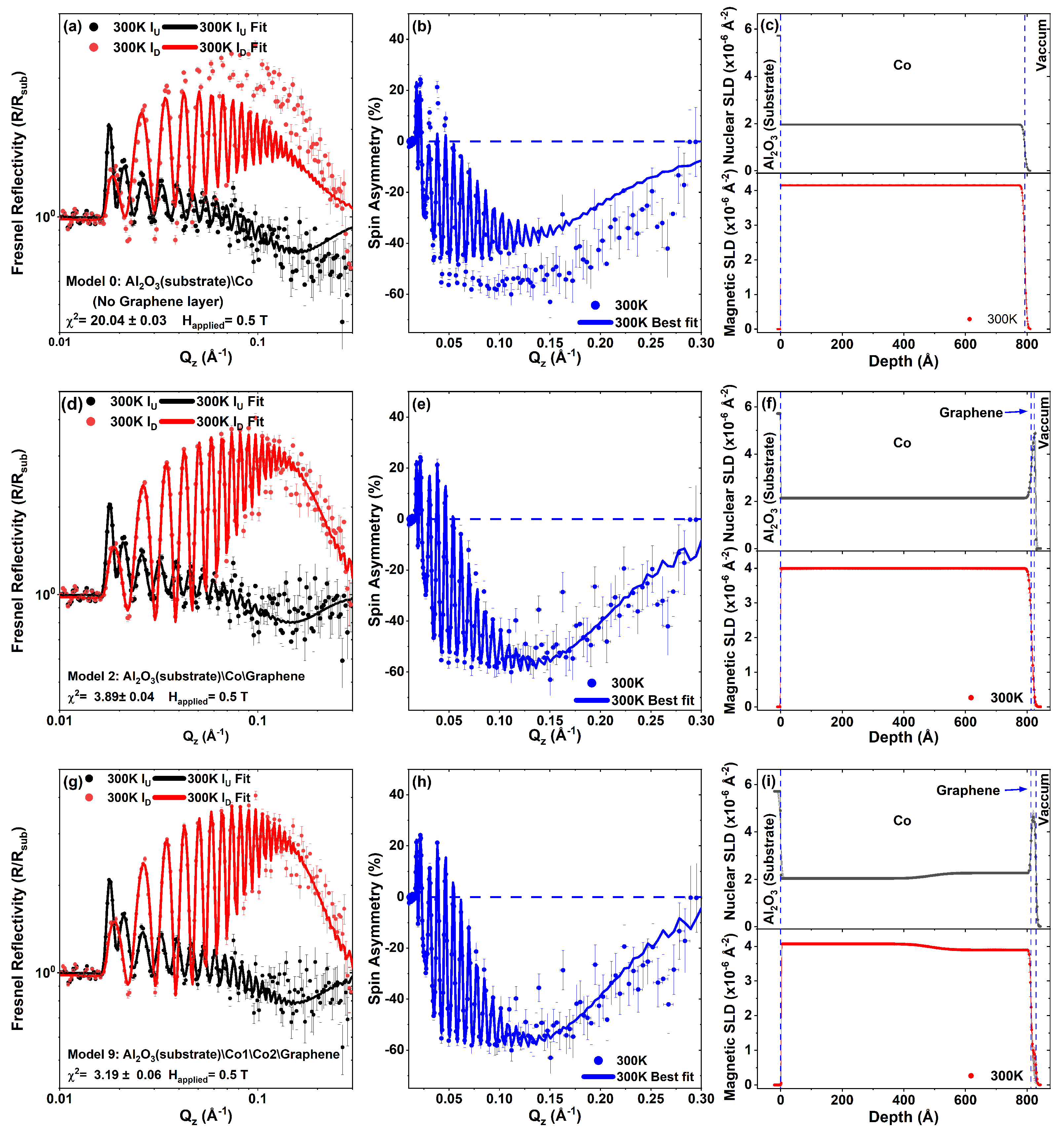
3.5. Polarised Neutron Reflectivity
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hirohata, A.; Yamada, K.; Nakatani, Y.; Prejbeanu, I.L.; Diény, B.; Pirro, P.; Hillebrands, B. Review on spintronics: Principles and device applications. J. Magn. Magn. Mater. 2020, 509, 166711. [Google Scholar] [CrossRef]
- Lin, X.; Yang, W.; Wang, K.L.; Zhao, W. Two-dimensional spintronics for low-power electronics. Nat. Electron. 2019, 2, 274–283. [Google Scholar] [CrossRef]
- Feng, Y.P.; Shen, L.; Yang, M.; Wang, A.; Zeng, M.; Wu, Q.; Chintalapati, S.; Chang, C. Prospects of spintronics based on 2D materials. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2017, 7, e1313. [Google Scholar] [CrossRef]
- Liu, Y.; Zeng, C.; Zhong, J.; Ding, J.; Wang, Z.M.; Liu, Z. Spintronics in Two-Dimensional Materials. Nano-Micro Lett. 2020, 12, 93. [Google Scholar] [CrossRef] [PubMed]
- Yager, T.; Lartsev, A.; Cedergren, K.; Panchal, R.Y.V.; Kazakova, O.; Tzalenchuk, A.; Kim, K.H.; Park, Y.W.; Lara-Avila, S.; Kubatkin, S. Low contact resistance in epitaxial graphene devices for quantum metrology. AIP Adv. 2015, 5, 087134. [Google Scholar] [CrossRef]
- Gheorghiu, M.; Vasilescu, A. Chapter 7—Surface Plasmon Resonance-Modified Graphene Oxide Surfaces for Whole-Cell-Based Sensing. In Advanced Nanomaterials; Elsevier Inc.: Amsterdam, The Netherlands, 2018; p. 151. [Google Scholar] [CrossRef]
- Ye, Z.; Wu, P.; Wang, H.; Jiang, S.; Huang, M.; Lei, D.; Wu, F. Multimode tunable terahertz absorber based on a quarter graphene disk structure. Results Phys. 2023, 48, 106420. [Google Scholar] [CrossRef]
- Chen, Z.; Cai, P.; Wen, Q.; Chen, H.; Tang, Y.; Yi, Z.; Wei, K.; Li, G.; Tang, B.; Yi, Y. Graphene Multi-Frequency Broadband and Ultra-Broadband Terahertz Absorber Based on Surface Plasmon Resonance. Electronics 2023, 12, 2655. [Google Scholar] [CrossRef]
- Weser, M.; Voloshina, E.N.; Horn, K.; Dedkov, Y.S. Electronic Structure and Magnetic Properties of the Graphene/Fe/Ni(111) Intercalation-like System. Phys. Chem. Chem. Phys. 2011, 13, 7534. [Google Scholar] [CrossRef]
- Pi, K.; Han, W.; McCreary, K.M.; Swartz, A.G.; Li, Y.; Kawakami, R.K. Manipulation of Spin Transport in Graphene by Surface Chemical Doping. Phys. Rev. Lett. 2010, 104, 187201. [Google Scholar] [CrossRef]
- Weser, M.; Rehder, Y.; Horn, K.; Sicot, M.; Fonin, M.; Preobrajenski, A.B.; Voloshina, E.N.; Goering, E.; Dedkov, Y.S. Induced Magnetism Of Carbon Atoms at the Graphene/Ni(111) Interface. Appl. Phys. Lett. 2010, 96, 012504. [Google Scholar] [CrossRef]
- Leutenantsmeyer, J.C.; Kaverzin, A.A.; Wojtaszek, M.; van Wees, B.J. Proximity Induced Room Temperature Ferromagnetism in Graphene Probed with Spin Currents. 2D Mater. 2016, 4, 014001. [Google Scholar] [CrossRef]
- Wang, Z.; Tang, C.; Sachs, R.; Barlas, Y.; Shi, J. Proximity-Induced Ferromagnetism in Graphene Revealed by the Anomalous Hall Effect. Phys. Rev. Lett. 2015, 114, 016603. [Google Scholar] [CrossRef] [PubMed]
- Haugen, H.; Huertas-Hernando, D.; Brataas, A. Spin Transport in Proximity-induced Ferromagnetic Graphene. Phys. Rev. B 2008, 77, 115406. [Google Scholar] [CrossRef]
- Voloshina, E.N.; Dedkov, Y.S. General Approach to the Understanding the Electronic Structure of Graphene on Metals. Mater. Res. Express 2014, 1, 035603. [Google Scholar] [CrossRef]
- Dedkov, Y.; Voloshina, E. Graphene Growth and Properties on Metal Substrates. J. Phys. Condens. Matter 2015, 27, 303002. [Google Scholar] [CrossRef]
- Voloshina, E.; Dedkov, Y. Electronic and Magnetic Properties of the Graphene-Ferromagnet Interfaces: Theory vs. Experiment. In Physics and Applications of Graphene—Experiments; InTech: London, UK, 2011; Volume 499, pp. 75–78. [Google Scholar] [CrossRef]
- Dahal, A.; Batzill, M. Graphene–nickel interfaces: A review. Nanoscale 2014, 6, 2548. [Google Scholar] [CrossRef]
- Dedkov, Y.S.; Fonin, M. Electronic and Magnetic Properties of the Graphene–ferromagnet Interface. New J. Phys. 2010, 12, 125004. [Google Scholar] [CrossRef]
- Vita, H.; Böttcher, S.; Leicht, P.; Horn, K.; Shick, A.B.; Máca, F. Electronic structure and magnetic properties of cobalt intercalated in graphene on Ir(111). Phys. Rev. B Condens. Matter Mater. Phys. 2014, 90, 165432. [Google Scholar] [CrossRef]
- Marchenko, D.; Varykhalov, A.; Sánchez-Barriga, J.; Rader, O.; Carbone, C.; Bihlmayer, G. Highly spin-polarized Dirac fermions at the graphene/Co interface. Phys. Rev. B Condens. Matter Mater. Phys. 2015, 91, 235431. [Google Scholar] [CrossRef]
- Aboljadayel, R.O.M.; Kinane, C.J.; Vaz, C.A.F.; Love, D.M.; Weatherup, R.S.; Braeuninger-Weimer, P.; Martin, M.B.; Ionescu, A.; Caruana, A.J.; Charlton, T.R.; et al. Determining the Proximity Effect-Induced Magnetic Moment in Graphene by Polarized Neutron Reflectivity and X-ray Magnetic Circular Dichroism. Appl. Mater. Interfaces 2023, 15, 22367. [Google Scholar] [CrossRef]
- Karpan, V.M.; Khomyakov, P.A.; Starikov, A.A.; Giovannetti, G.; Zwierzycki, M.; Talanana, M.; Brocks, G.; van den Brink, J.; Kelly, P.J. Theoretical Prediction of Perfect Spin Filtering at Interfaces Between Close-packed Surfaces of Ni or Co and Graphite or Graphene. Phys. Rev. B 2008, 78, 195419. [Google Scholar] [CrossRef]
- Cabrero-Vilatela, A.; Weatherup, R.S.; Braeuninger-Weimer, P.; Caneva, S.; Hofmann, S. Towards a general growth model for graphene CVD on transition metal catalysts. Nanoscale 2016, 8, 2149–2158. [Google Scholar] [CrossRef] [PubMed]
- Ago, H.; Ito, Y.; Mizuta, N.; Yoshida, K.; Hu, B.; Orofeo, C.M.; Tsuji, M.; ichi Ikeda, K.; Mizuno, S. Epitaxial Chemical Vapor Deposition Growth of Single-Layer Graphene over Cobalt Film Crystallized on Sapphire. ACS Nano 2010, 4, 7407. [Google Scholar] [CrossRef] [PubMed]
- Machac, P.; Hejna, O.; Slepicka, P. Graphene growth by transfer-free chemicalvapour deposition on a cobalt layer. J. Electr. Eng. 2017, 68, 79. [Google Scholar] [CrossRef]
- Available online: http://www.isis.stfc.ac.uk/instruments/polref/technical/polref-technical-information7280.html (accessed on 28 September 2021).
- Kienzle, P.; Maranville, B.; O’Donovan, K.; Ankner, J.; Berk, N.; Majkrzak, C. 2017. Available online: https://www.nist.gov/ncnr/reflectometry-software (accessed on 28 September 2021).
- Kienzle, P.; Krycka, J.; Patel, N.; Sahin, I. 2011. Available online: https://bumps.readthedocs.io/en/latest/ (accessed on 28 September 2021).
- Shlimak, I.; Haran, A.; Zion, E.; Havdala, T.; Kaganovskii, Y.; Butenko, A.V.; Wolfson, L.; Richter, V.; Naveh, D.; Sharoni, A.; et al. Raman Scattering and Electrical Resistance of Highly Disordered Graphene. Phys. Rev. B 2015, 91, 045414. [Google Scholar] [CrossRef]
- Allard, A.; Wirtz, L. Graphene on Metallic Substrates: Suppression of the Kohn Anomalies in the Phonon Dispersion. Nano Lett. 2010, 10, 4335–4340. [Google Scholar] [CrossRef]
- Malard, L.M.; Pimenta, M.A.; Dresselhaus, G.; Dresselhaus, M.S. Raman Spectroscopy in Graphene. Phys. Rep. 2009, 473, 51–87. [Google Scholar] [CrossRef]
- Ferrari, A.C.; Basko, D.M. Raman Spectroscopy as a Versatile Tool for Studying the Properties of Graphene. Nat. Nanotechnol. 2013, 8, 235–246. [Google Scholar] [CrossRef]
- Reina, A.; Son, H.; Jiao, L.; Fan, B.; Dresselhaus, M.S.; Liu, Z.; Kong, J. Transferring and Identification of Single- and Few-Layer Graphene on Arbitrary Substrates. J. Phys. Chem. C 2008, 112, 17741–17744. [Google Scholar] [CrossRef]
- Ferrari, A.C.; Meyer, J.C.; Scardaci, V.; Casiraghi, C.; Lazzeri, M.; Mauri, F.; Piscanec, S.; Jiang, D.; Novoselov, K.S.; Roth, S.; et al. Raman Spectrum of Graphene and Graphene Layers. Phys. Rev. Lett. 2006, 97, 187401. [Google Scholar] [CrossRef]
- Moon, R.M. Distribution of Magnetic Moment in Hexagonal Cobalt. Phys. Rev. 1964, 136, A195–A202. [Google Scholar] [CrossRef]
- Chen, C.T.; Idzerda, Y.U.; Lin, H.J.; Smith, N.V.; Meigs, G.; Chaban, E.; Ho, G.H.; Pellegrin, E.; Sette, F. Experimental Confirmation of the X-ray Magnetic Circular Dichroism Sum Rules for Iron and Cobalt. Phys. Rev. Lett. 1995, 75, 152–155. [Google Scholar] [CrossRef] [PubMed]
- Eriksson, O.; Johansson, B.; Albers, R.C.; Boring, A.M.; Brooks, M.S.S. Orbital Magnetism in Fe, Co, and Ni. Phys. Rev. B 1990, 42, 2707–2710. [Google Scholar] [CrossRef] [PubMed]
- Pelzl, J.; Meckenstock, R.; Spoddig, D.; Schreiber, F.; Pflaum, J.; Frait, Z. Spin–orbit-coupling effects on g-value and damping factor of the ferromagnetic resonance in Co and Fe films. J. Phys. Condens. Matter 2003, 15, S451. [Google Scholar] [CrossRef]
- Menezes, N.; Alves, V.S.; Marino, E.C.; Nascimento, L.; Nascimento, L.O.; Morais Smith, C. Spin g-factor due to electronic interactions in graphene. Phys. Rev. B 2017, 95, 245138. [Google Scholar] [CrossRef]
- Swart, J.C.W.; van Steen, E.; Ciobica, I.M.; van Santen, R.A. Interaction of Graphene with FCC-Co(111). Phys. Chem. Chem. Phys. 2009, 11, 803–807. [Google Scholar] [CrossRef]
- McCluskey, A.R.; Cooper, J.F.K.; Arnold, T.; Snow, T. A general approach to maximise information density in neutron reflectometry analysis. Mach. Learn. Sci. Technol. 2020, 1, 035002. [Google Scholar] [CrossRef]
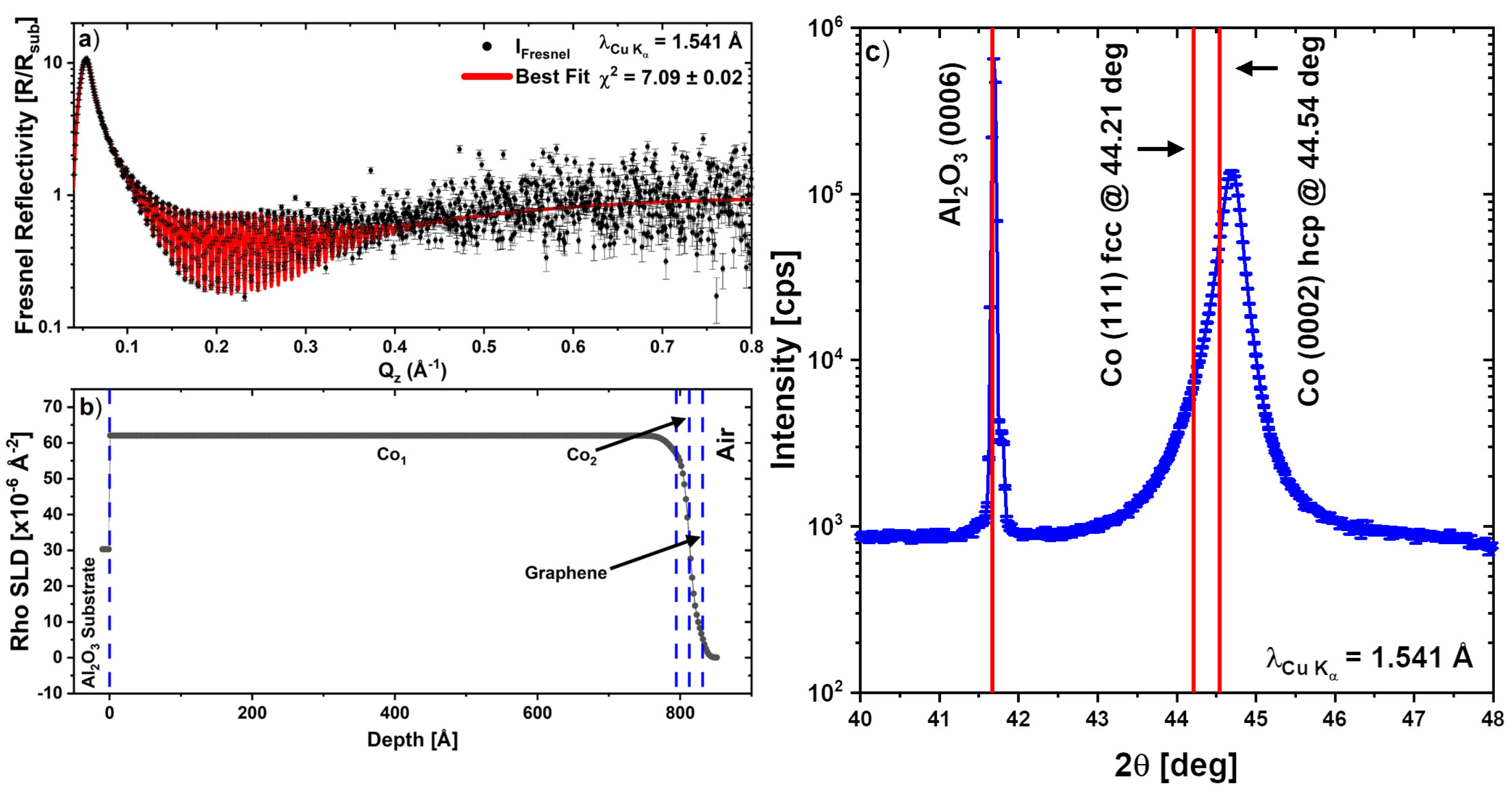


| Layer | Thickness (z) | Roughness () | SLD () |
|---|---|---|---|
| [nm] | [nm] | [×10Å] | |
| Graphene | 1.86 [1.85, 1.88] | 0.64 [0.63, 0.65] | 10.5 [10.4, 10.8] |
| Cobalt 2 | 1.83 [1.78, 1.89] | 0.64 [0.63, 0.65] | 52.0 [52.0, 52.1] |
| Cobalt 1 | 79.4 [79.4, 79.5] | 1.55 [1.51, 1.60] | 62.0 [61.8, 62.3] |
| Sapphire | Substrate | 0.02 [0.02, 0.023] | 30.3 [30.3, 30.32] |
| FM Layer1 + FM Layer2 | Graphene | ||||
|---|---|---|---|---|---|
| Sample | Temperature | Total Thickness | Magnetic Moment | Thickness | Magnetic Moment |
| [K] | [nm] | [/atom] | [nm] | [/atom] | |
| Gr/Co | 300 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aboljadayel, R.O.M.; Kinane, C.J.; Vaz, C.A.F.; Love, D.M.; Martin, M.-B.; Cabrero-Vilatela, A.; Braeuninger-Weimer, P.; Ionescu, A.; Caruana, A.J.; Charlton, T.R.; et al. Measurement of the Induced Magnetic Polarisation of Rotated-Domain Graphene Grown on Co Film with Polarised Neutron Reflectivity. Nanomaterials 2023, 13, 2620. https://doi.org/10.3390/nano13192620
Aboljadayel ROM, Kinane CJ, Vaz CAF, Love DM, Martin M-B, Cabrero-Vilatela A, Braeuninger-Weimer P, Ionescu A, Caruana AJ, Charlton TR, et al. Measurement of the Induced Magnetic Polarisation of Rotated-Domain Graphene Grown on Co Film with Polarised Neutron Reflectivity. Nanomaterials. 2023; 13(19):2620. https://doi.org/10.3390/nano13192620
Chicago/Turabian StyleAboljadayel, Razan Omar M., Christy John Kinane, Carlos Antonio Fernandes Vaz, David Michael Love, Marie-Blandine Martin, Andrea Cabrero-Vilatela, Philipp Braeuninger-Weimer, Adrian Ionescu, Andrew John Caruana, Timothy Randall Charlton, and et al. 2023. "Measurement of the Induced Magnetic Polarisation of Rotated-Domain Graphene Grown on Co Film with Polarised Neutron Reflectivity" Nanomaterials 13, no. 19: 2620. https://doi.org/10.3390/nano13192620
APA StyleAboljadayel, R. O. M., Kinane, C. J., Vaz, C. A. F., Love, D. M., Martin, M.-B., Cabrero-Vilatela, A., Braeuninger-Weimer, P., Ionescu, A., Caruana, A. J., Charlton, T. R., Llandro, J., Monteiro, P. M. d. S., Barnes, C. H. W., Hofmann, S., & Langridge, S. (2023). Measurement of the Induced Magnetic Polarisation of Rotated-Domain Graphene Grown on Co Film with Polarised Neutron Reflectivity. Nanomaterials, 13(19), 2620. https://doi.org/10.3390/nano13192620





