Possibilities of Controlling the Quantum States of Hole Qubits in an Ultrathin Germanium Layer Using a Magnetic Substrate: Results from ab Initio Calculations
Abstract
:1. Introduction
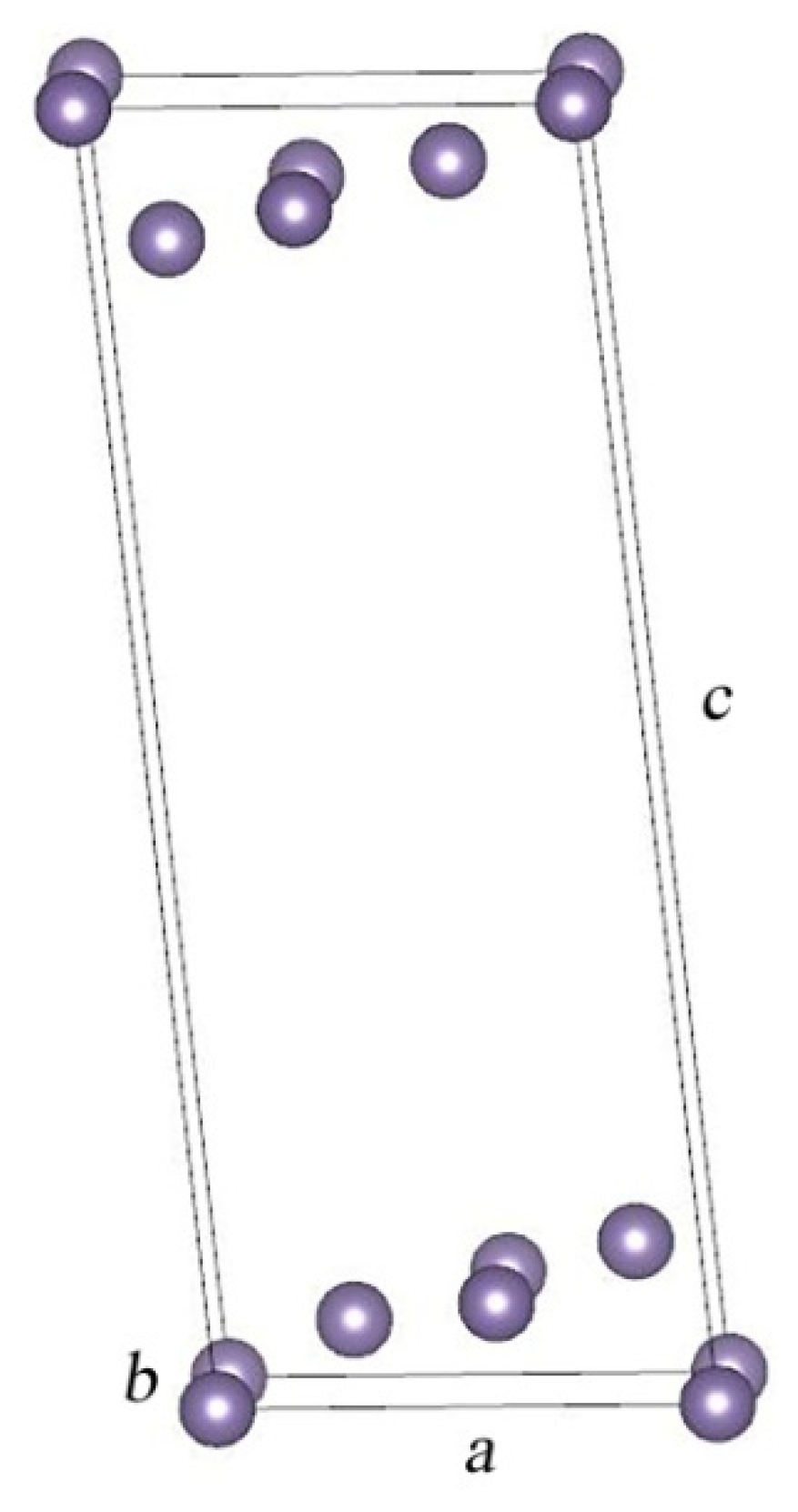
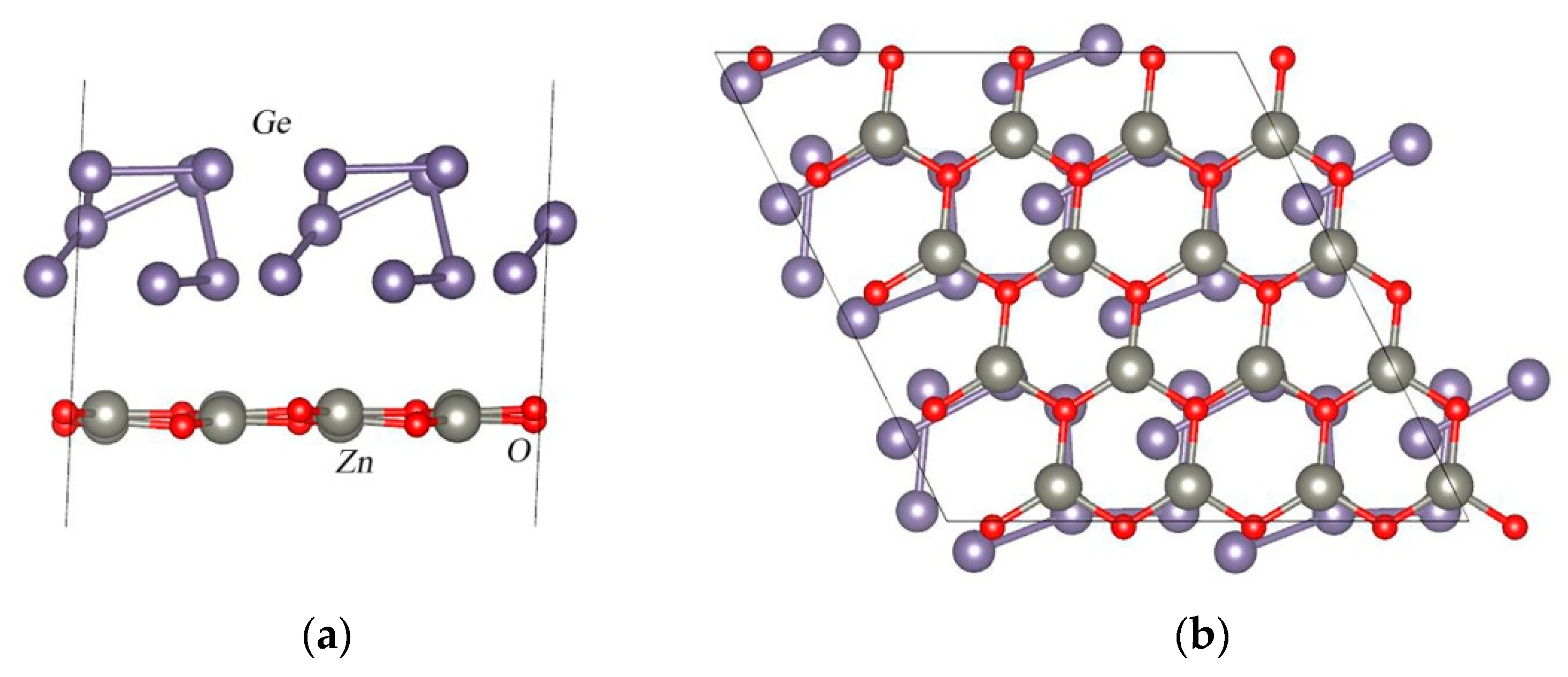
2. Methods and Details of Calculations
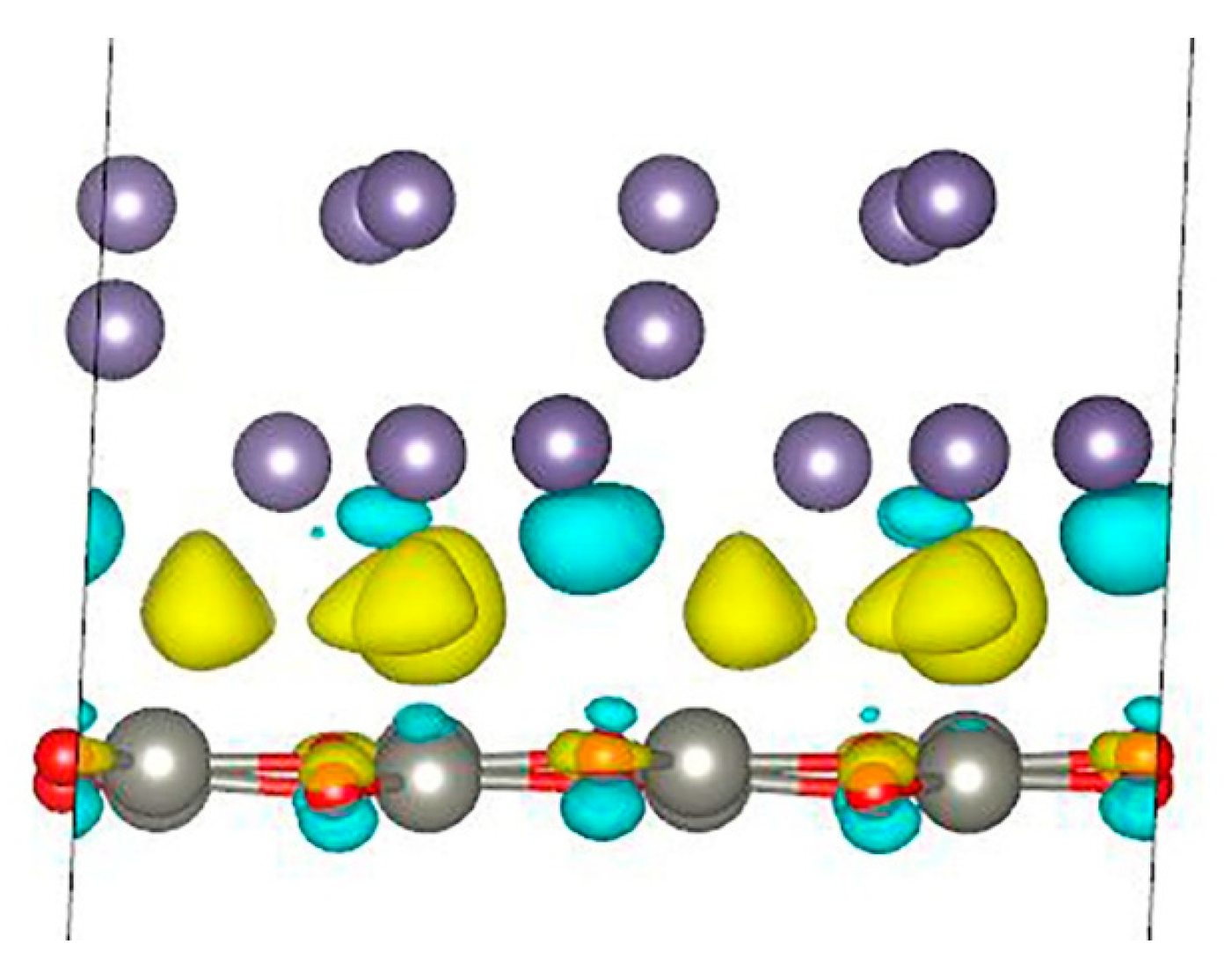
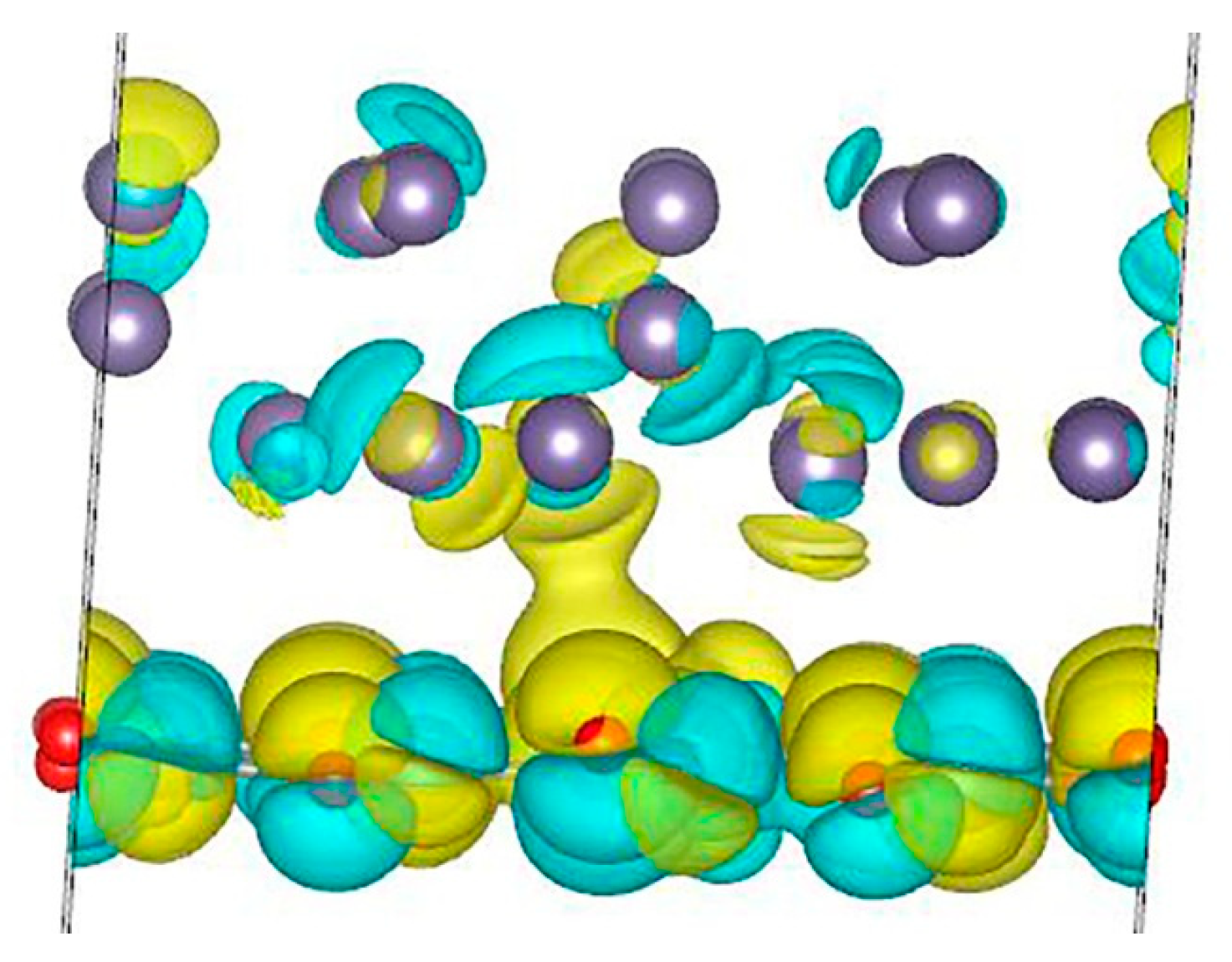
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Fang, Y.; Philippopoulos, P.; Culcer, D.; Coish, W.A.; Chesi, S. Recent advances in hole-spin qubits. Mater. Quantum Technol. 2023, 3, 012003. [Google Scholar] [CrossRef]
- Valentini, M.; Borovkov, M.; Prada, E.; Martí-Sánchez, S.; Botifoll, M.; Hofmann, A.C.; Arbiol, J.; Aguado, R.; San-Jose, P.; Katsaros, G. Majorana-like Coulomb spectroscopy in the absence of zero-bias peaks. Nature 2022, 612, 442–447. [Google Scholar] [CrossRef] [PubMed]
- Watzinger, H.; Kukučka, J.; Vukušić, L.; Gao, F.; Wang, T.; Schäffler, F.; Zhang, J.-J.; Katsaros, G. A germanium hole spin qubit. Nat. Commun. 2018, 9, 1. [Google Scholar] [CrossRef] [PubMed]
- Chibisov, A.N.; Aleshin, M.S.; Chibisova, M.A. DFT Analysis of Hole Qubits Spin State in Germanium thin layer. Nanomaterials 2022, 12, 2244. [Google Scholar] [CrossRef] [PubMed]
- Goncharov, A.V.; Chibisov, A.N. Effect of External Pressure and Quantum State on the Local Magnetization of Germanium Layers: Ab Initio Calculation. Adv. Theory Simul. 2023, 12, 2200816. [Google Scholar] [CrossRef]
- Watzinger, H.; Kloeffel, C.; Vukušić, L.; Rossell, M.D.; Sessi, V.; Kukučka, J.; Kirchschlager, R.; Lausecker, E.; Truhlar, A.; Glaser, M.; et al. Heavy-Hole States in Germanium Hut Wires. Nano Lett. 2016, 16, 6879–6885. [Google Scholar] [CrossRef]
- Terrazos, L.A.; Marcellina, E.; Wang, Z.; Coppersmith, S.N.; Friesen, M.; Hamilton, A.R.; Hu, X.; Koiller, B.; Saraiva, A.L.; Culcer, D.; et al. Theory of hole-spin qubits in strained germanium quantum dots. Phys. Rev. B 2021, 103, 125201. [Google Scholar] [CrossRef]
- Lodari, M.; Tosato, A.; Sabbagh, D.; Schubert, M.A.; Capellini, G.; Sammak, A.; Veldhorst, M.; Scappucci, G. Light effective hole mass in undoped Ge/SiGe quantum wells. Phys. Rev. B 2019, 100, 041304. [Google Scholar] [CrossRef]
- Liu, H.; Wang, K.; Gao, F.; Leng, J.; Liu, Y.; Zhou, Y.-C.; Cao, G.; Wang, T.; Zhang, J.; Huang, P.; et al. Ultrafast and Electrically Tunable Rabi Frequency in a Germanium Hut Wire Hole Spin Qubit. Nano Lett. 2023, 23, 3810–3817. [Google Scholar] [CrossRef]
- Jirovec, D.; Hofmann, A.; Ballabio, A.; Mutter, P.M.; Tavani, G.; Botifoll, M.; Crippa, A.; Kukucka, J.; Sagi, O.; Martins, F.; et al. A singlet-triplet hole spin qubit in planar Ge. Nat. Mater. 2021, 20, 1106–1112. [Google Scholar] [CrossRef]
- Wang, C.-A.; Scappucci, G.; Veldhorst, M.; Russ, M. Modelling of planar germanium hole qubits in electric and magnetic fields. arXiv 2022, arXiv:2208.04795. [Google Scholar]
- Yu, C.X.; Zihlmann, S.; Abadillo-Uriel, J.C.; Michal, V.P.; Rambal, N.; Niebojewski, H.; Bedecarrats, T.; Vinet, M.; Dumur, E.; Filippone, M.; et al. Strong coupling between a photon and a hole spin in silicon. Nat. Nanotechnol. 2023, 18, 741–746. [Google Scholar] [CrossRef] [PubMed]
- Chen, R.; Luo, F.; Liu, Y.; Song, Y.; Dong, Y.; Wu, S.; Cao, J.; Yang, F.; N’Diaye, A.; Shafer, P.; et al. Tunable room-temperature ferromagnetism in Co-doped two-dimensional van der Waals ZnO. Nat. Commun. 2021, 12, 3952. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Yang, X.; Khamis, K.M. Influence of Co doping concentrations and strains on the electronic structure and absorption spectrum of graphene-like ZnO monolayer. Results Phys. 2023, 44, 106161. [Google Scholar] [CrossRef]
- Khan, M.C.; Zou, B.; Yao, S.; Zheng, B.; Cao, J.; Huang, W.; Zhou, Z.; Abdalla, A.S. Carriers induced ferromagnetism in Co(II)-doped ZnO monolayers and their optical properties: First principles calculations. Chin. J. Phys. 2023. [Google Scholar] [CrossRef]
- Kresse, G.; Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 1993, 47, 558. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comput. Mater. Sci. 1996, 6, 15–50. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953. [Google Scholar] [CrossRef]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758. [Google Scholar] [CrossRef]
- Hobbs, D.; Kresse, G.; Hafner, J. Fully unconstrained noncollinear magnetism within the projector augmented-wave method. Phys. Rev. B 2000, 62, 11556. [Google Scholar] [CrossRef]
- Steiner, S.; Khmelevskyi, S.; Marsmann, M.; Kresse, G. Calculation of the magnetic anisotropy with projected-augmented-wave methodology and the case study of disordered Fe1−xCox alloys. Phys. Rev. B 2016, 93, 224425. [Google Scholar] [CrossRef]
- Dudarev, S.L.; Botton, G.A.; Savrasov, S.Y.; Humphreys, C.J.; Sutton, A.P. Electron-energy-loss spectra and the structural stability of nickel oxide: An LSDA+U study. Phys. Rev. B 1998, 57, 1505. [Google Scholar] [CrossRef]
- Grimme, S.; Ehrlich, S.; Goerigk, L. Effect of the damping function in dispersion corrected density functional theory. J. Comput. Chem. 2011, 32, 1456. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188. [Google Scholar] [CrossRef]
- Jafarova, V.N.; Orudzhev, G.S. Structural and electronic properties of ZnO: A first-principles density-functional theory study within LDA(GGA) and LDA(GGA)+U methods. Solid State Commun. 2021, 325, 114166. [Google Scholar] [CrossRef]
- Ma, X.G.; Wu, Y.; Lv, Y.H.; Zhu, Y.F. Correlation Effects on Lattice Relaxation and Electronic Structure of ZnO within the GGA+U Formalism. J. Phys. Chem. C 2013, 117, 26029–26039. [Google Scholar] [CrossRef]
- Dong, C.L.; Persson, C.; Vayssieres, L.; Augustsson, A.; Schmitt, T.; Mattesini, M.; Ahuja, R.; Chang, C.L.; Guo, J.-H. Electronic structure of nanostructured ZnO from X-ray absorption and emission spectroscopy and the local density approximation. Phys. Rev. B 2004, 70, 195325. [Google Scholar] [CrossRef]
- Guana, Y.; Yaoa, H.; Zhan, H.; Wangab, H.; Zhoua, Y.H.; Kang, J. Optoelectronic properties and strain regulation of the 2D WS 2/ZnO van der Waals heterostructure. RSC Adv. 2021, 11, 14085–14092. [Google Scholar] [CrossRef]
- Yu, M.; Trinkle, D.R. Accurate and efficient algorithm for Bader charge integration. J. Chem. Phys. 2011, 134, 064111. [Google Scholar] [CrossRef]
- Philippopoulos, P.; Chesi, S.; Culcer, D.; Coish, W.A. Pseudospin-electric coupling for holes beyond the envelope-function approximation. Phys. Rev. B 2020, 102, 075310. [Google Scholar] [CrossRef]
- Marcellina, E.; Srinivasan, A.; Miserev, D.S.; Croxall, A.F.; Ritchie, D.A.; Farrer, I.; Sushkov, O.P.; Culcer, D.; Hamilton, A.R. Electrical control of the Zeeman spin splitting in two-dimensional hole systems. Phys. Rev. Lett. 2018, 121, 077701. [Google Scholar] [CrossRef] [PubMed]
- Park, S.; Chen, H.; Hiura, S.; Takayama, J.; Sueoka, K.; Murayama, A. Electric-Field-Effect Spin Switching with an Enhanced Number of Highly Polarized Electron and Photon Spins Using p-Doped Semiconductor Quantum Dots. ACS Omega 2021, 6, 8561–8569. [Google Scholar] [CrossRef] [PubMed]
- Dargys, A. Ultrafast control of hole spin by electric field in semiconductors. IEEE J. Sel. Top. Quantum Electron. 2004, 10, 155–158. [Google Scholar] [CrossRef]
- Martinez, B.; Abadillo-Uriel, J.C.; Rodríguez-Mena, E.A.; Niquet, Y.-M. Hole spin manipulation in inhomogeneous and nonseparable electric fields. Phys. Rev. B 2022, 106, 235426. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chibisov, A.N.; Chibisova, M.A.; Prokhorenko, A.V.; Obrazcov, K.V.; Fedorov, A.S.; Yu, Y.-X. Possibilities of Controlling the Quantum States of Hole Qubits in an Ultrathin Germanium Layer Using a Magnetic Substrate: Results from ab Initio Calculations. Nanomaterials 2023, 13, 3070. https://doi.org/10.3390/nano13233070
Chibisov AN, Chibisova MA, Prokhorenko AV, Obrazcov KV, Fedorov AS, Yu Y-X. Possibilities of Controlling the Quantum States of Hole Qubits in an Ultrathin Germanium Layer Using a Magnetic Substrate: Results from ab Initio Calculations. Nanomaterials. 2023; 13(23):3070. https://doi.org/10.3390/nano13233070
Chicago/Turabian StyleChibisov, Andrey N., Mary A. Chibisova, Anastasiia V. Prokhorenko, Kirill V. Obrazcov, Aleksandr S. Fedorov, and Yang-Xin Yu. 2023. "Possibilities of Controlling the Quantum States of Hole Qubits in an Ultrathin Germanium Layer Using a Magnetic Substrate: Results from ab Initio Calculations" Nanomaterials 13, no. 23: 3070. https://doi.org/10.3390/nano13233070
APA StyleChibisov, A. N., Chibisova, M. A., Prokhorenko, A. V., Obrazcov, K. V., Fedorov, A. S., & Yu, Y.-X. (2023). Possibilities of Controlling the Quantum States of Hole Qubits in an Ultrathin Germanium Layer Using a Magnetic Substrate: Results from ab Initio Calculations. Nanomaterials, 13(23), 3070. https://doi.org/10.3390/nano13233070






