Entropy Generation and Thermal Radiation Analysis of EMHD Jeffrey Nanofluid Flow: Applications in Solar Energy
Abstract
:1. Introduction
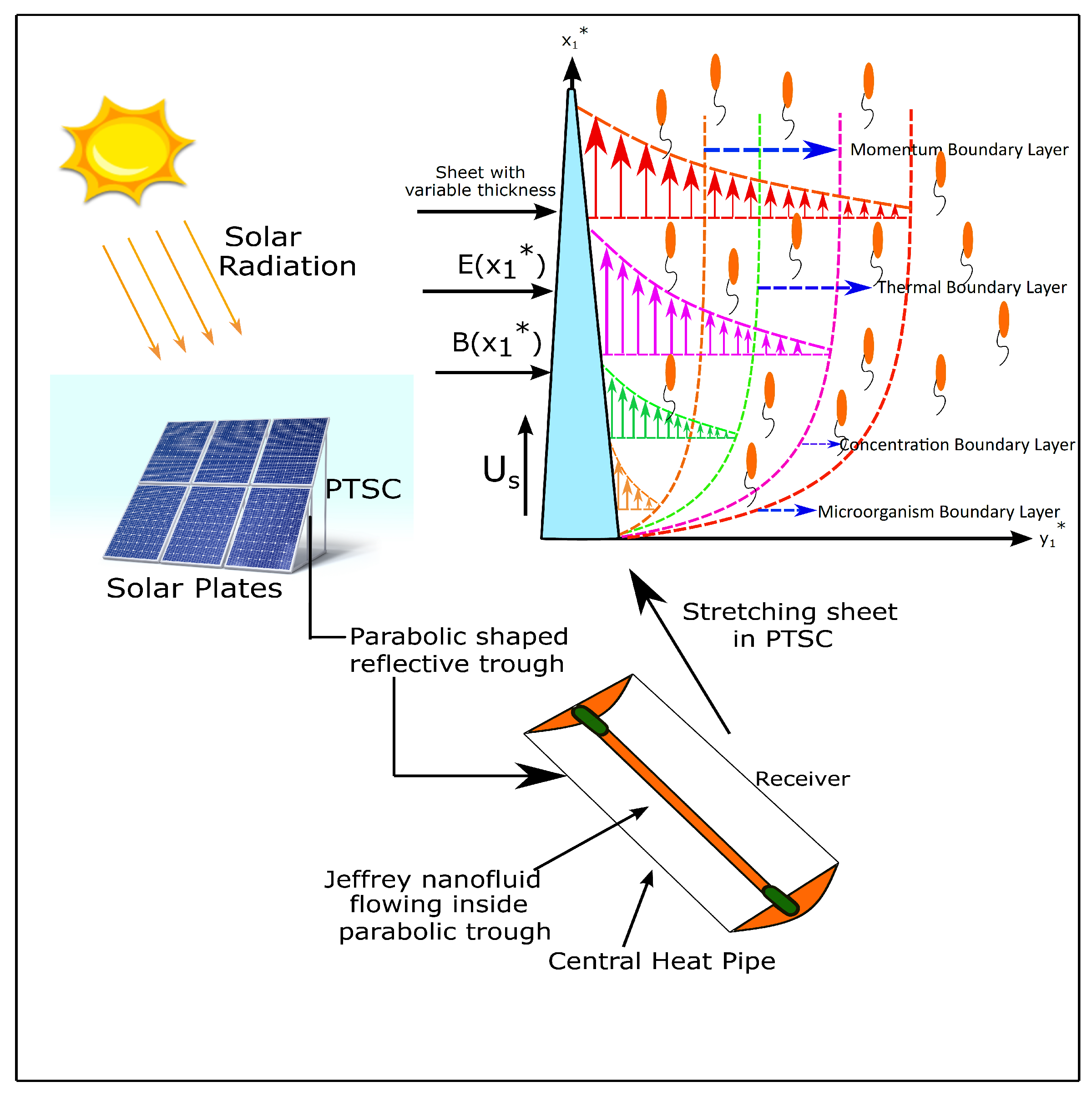
2. Formulation of Model
2.1. Physical Assumptions
2.2. Governing Equations of the Physical Model
2.3. Similarity Transformations
2.4. Boundary Conditions
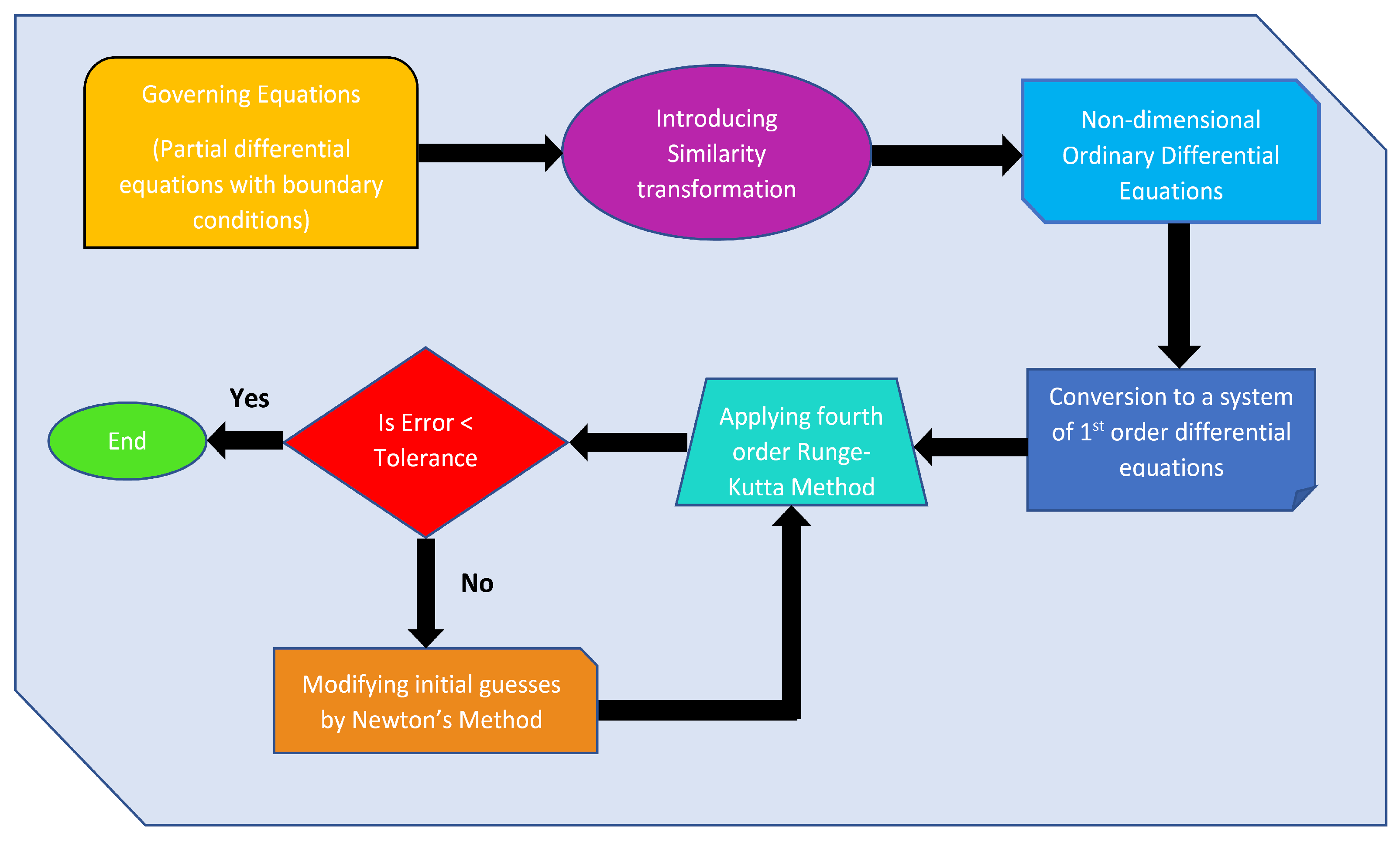
2.5. Numerical Methodology
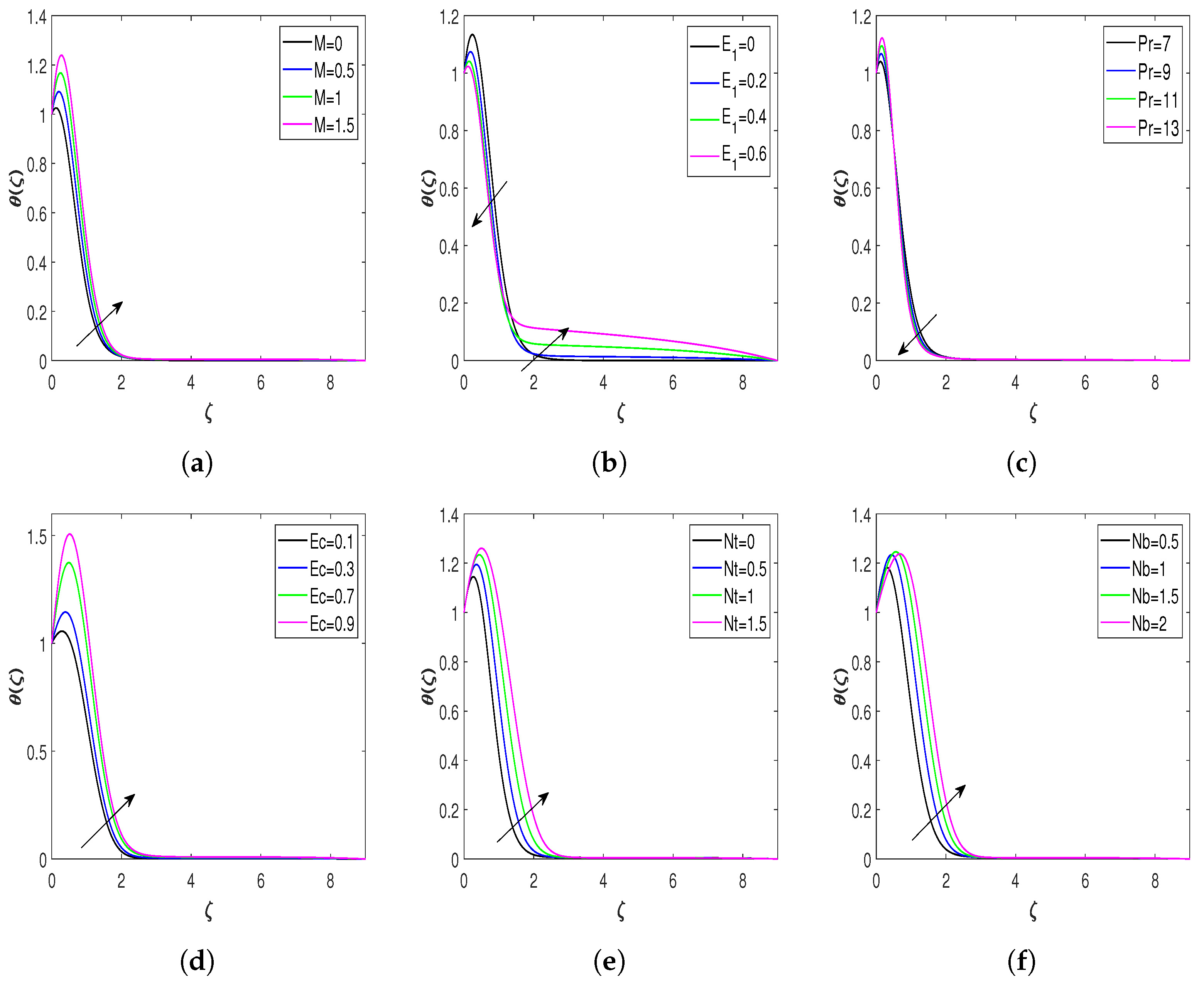
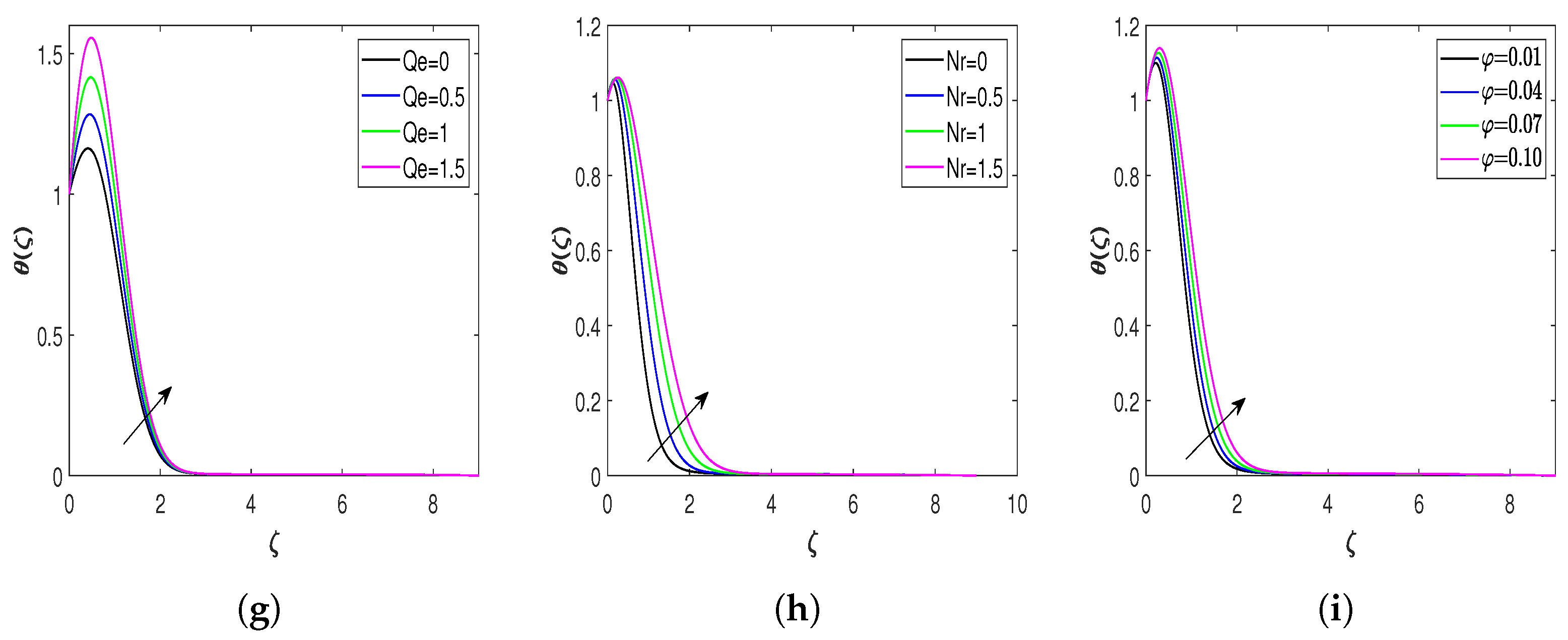
3. Result and Discussion
3.1. Entropy Generation Model
3.2. Physical Quantities of Engineering Interest
4. Conclusions
- Deborah number diminishes the velocity profile, while Deborah number enhances the velocity profile.
- Fluid velocity shows enhancement for rising values of , , and , while fluid velocity decays for augmenting values of M, , and .
- The increment in M, , , , , , and enhances the temperature profile, whereas the temperature profile decays for the magnifying values of Pr.
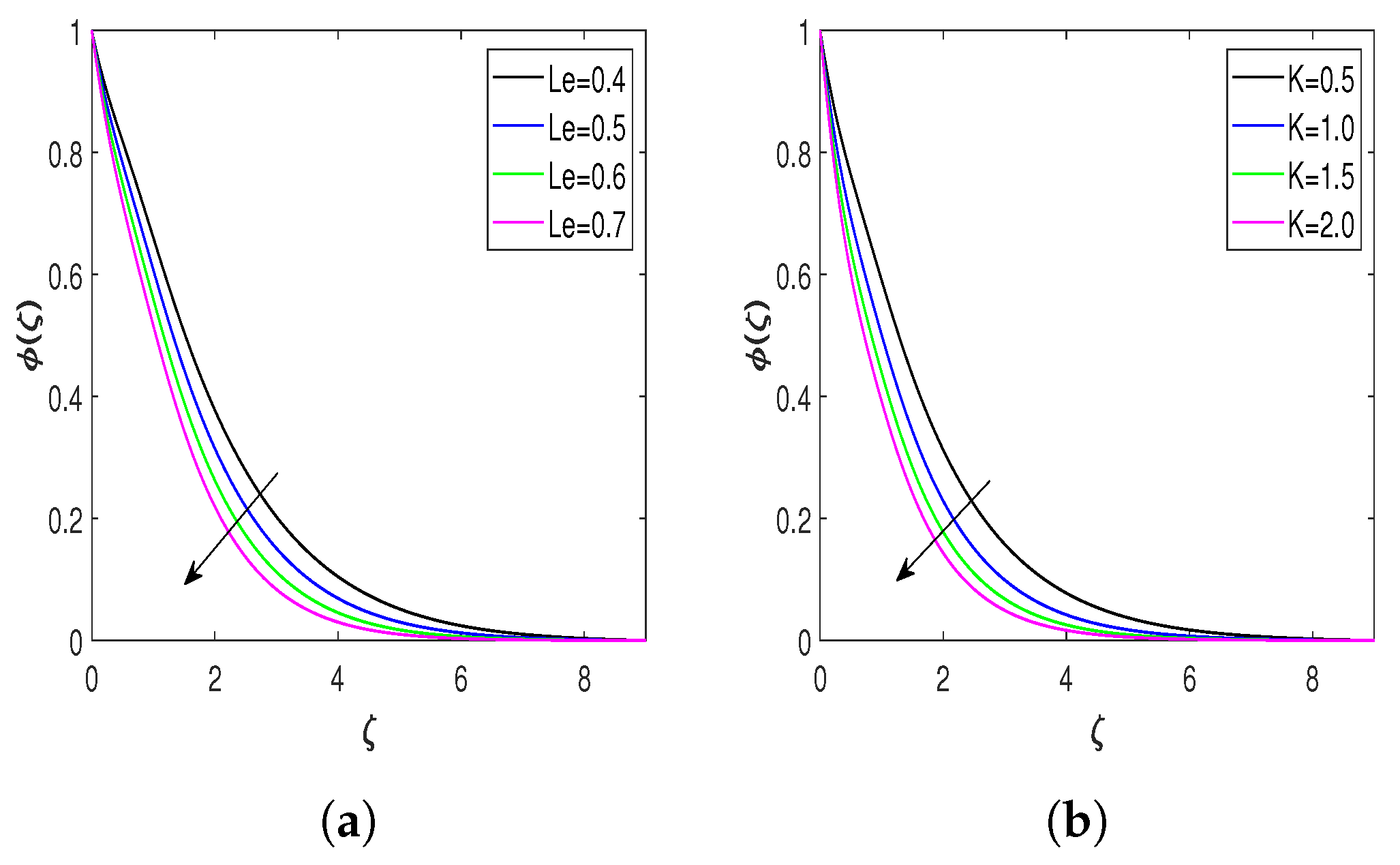
- The concentration profile decays for increasing values of and K.
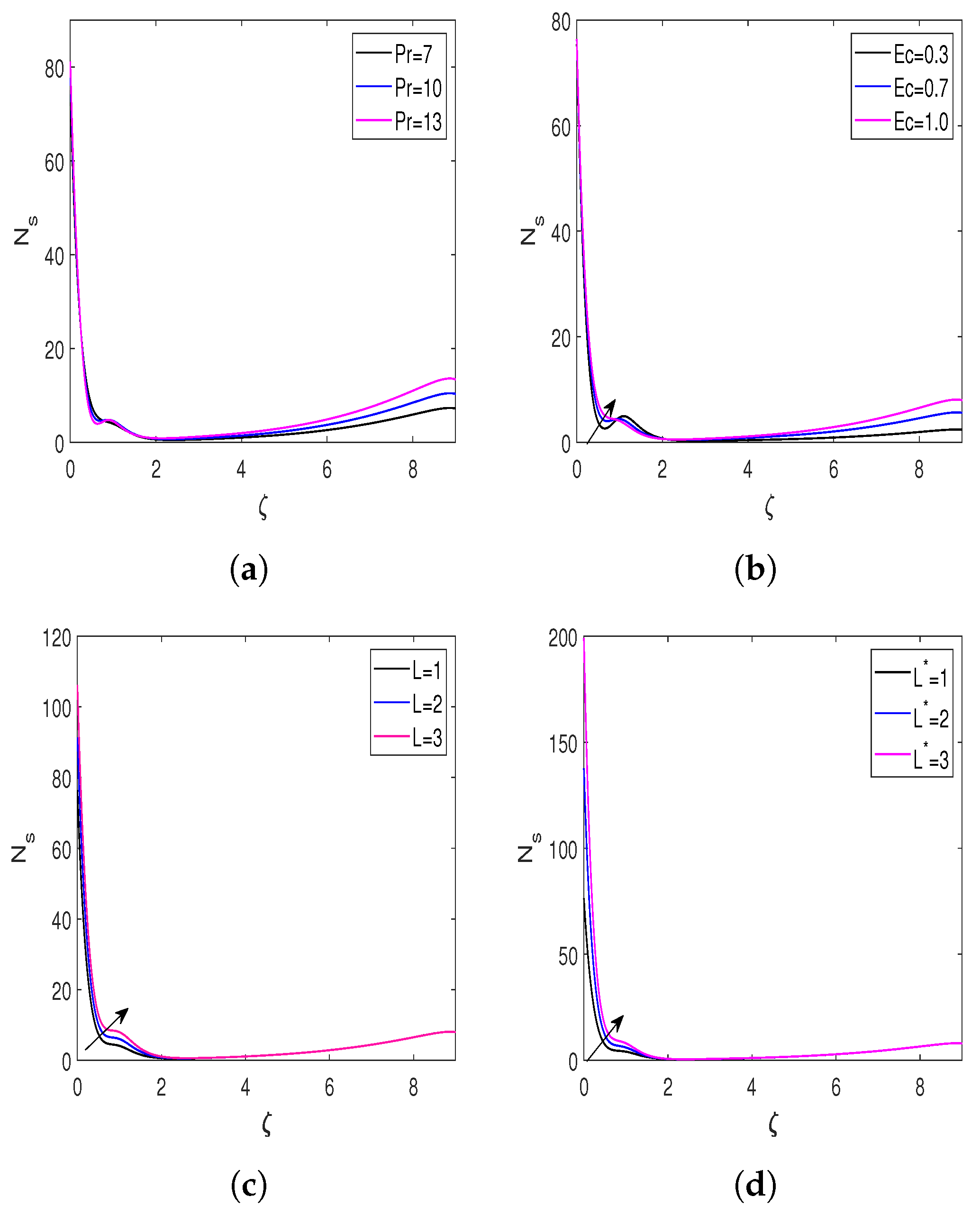
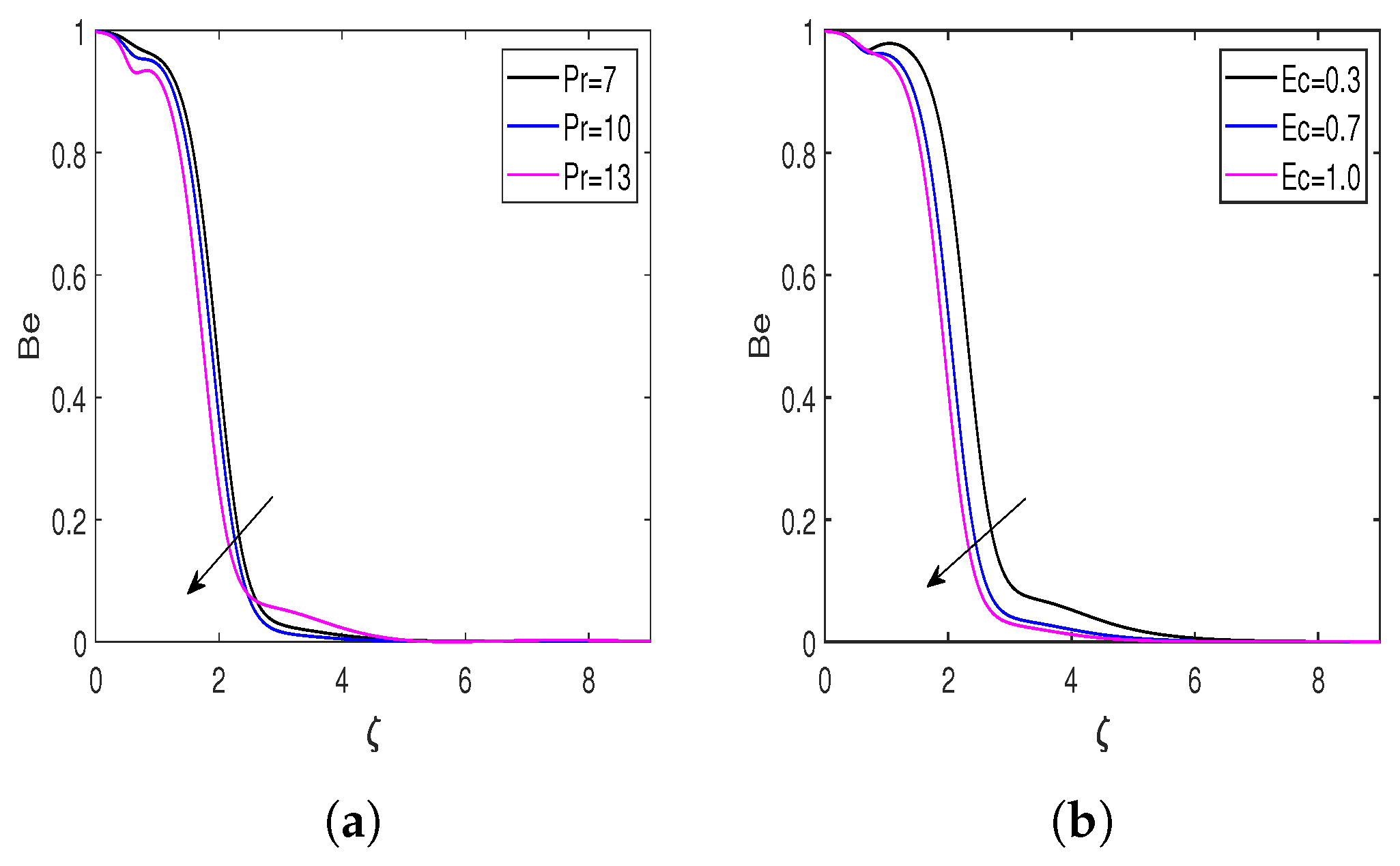
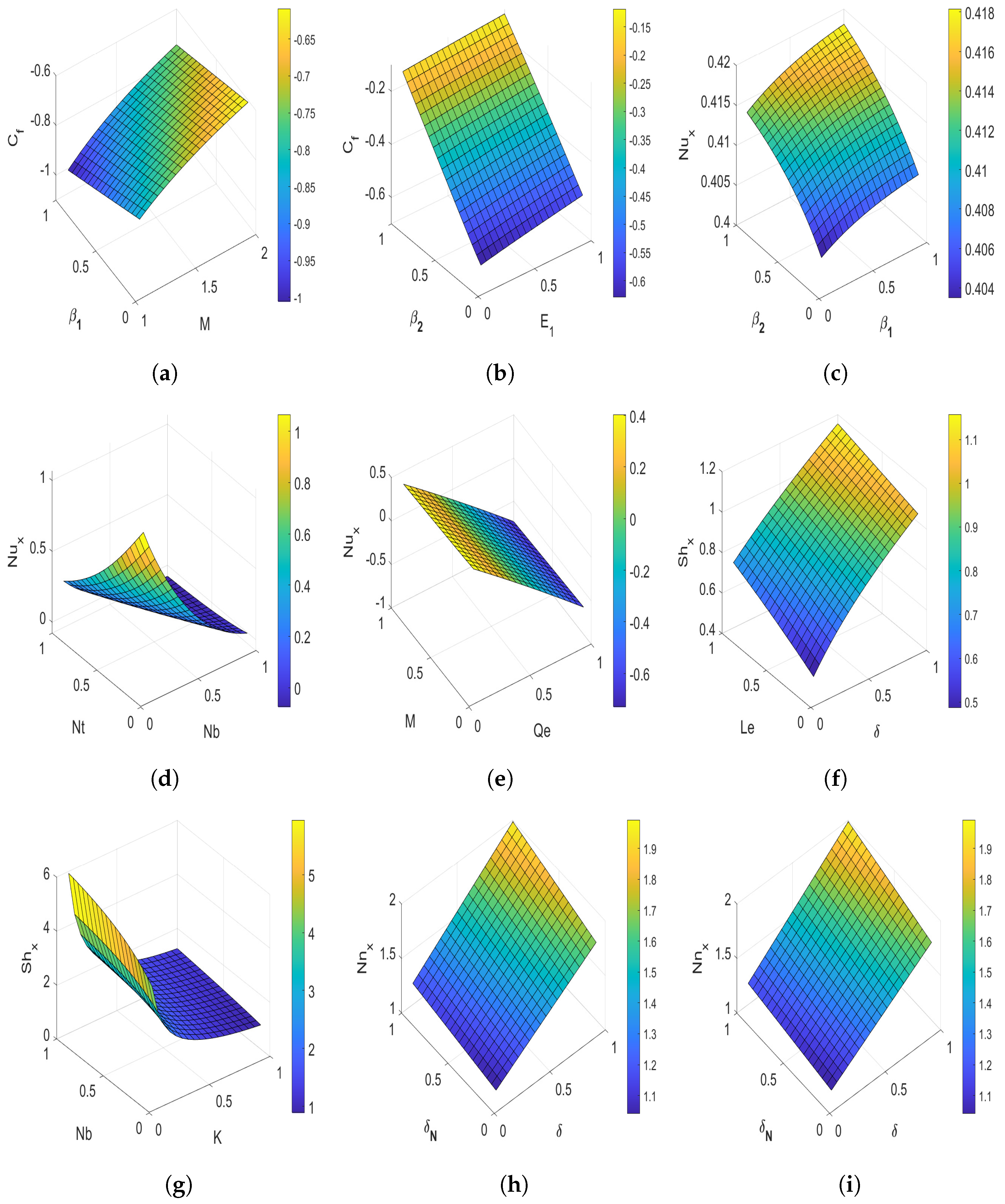
- The rate of entropy increases with an increment in and , while the Bejan number shows declination.
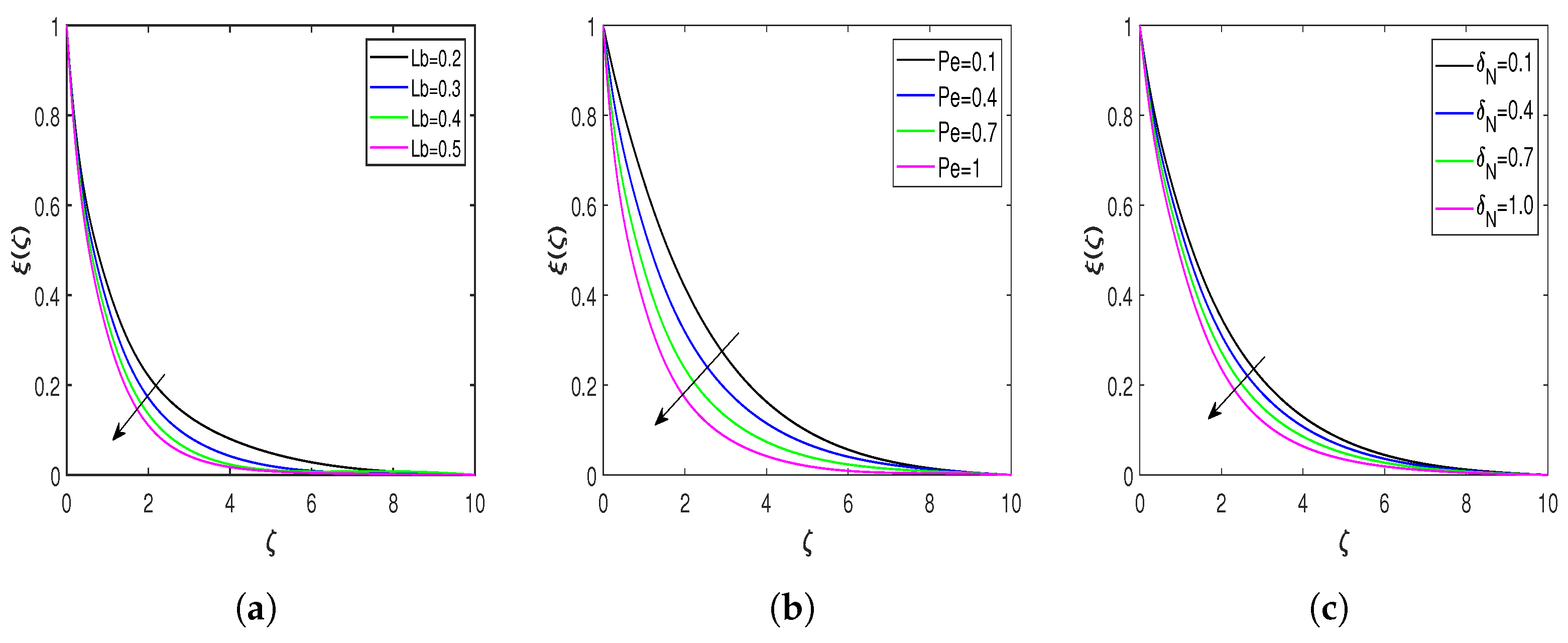
- Both the entropy formation rate and Bejan number enhance with the increment diffusion parameter L and bioconvection diffusion parameter .
- Drag coefficient improves with the growing values of M, , and , while the drag coefficient reduces with an increase in .
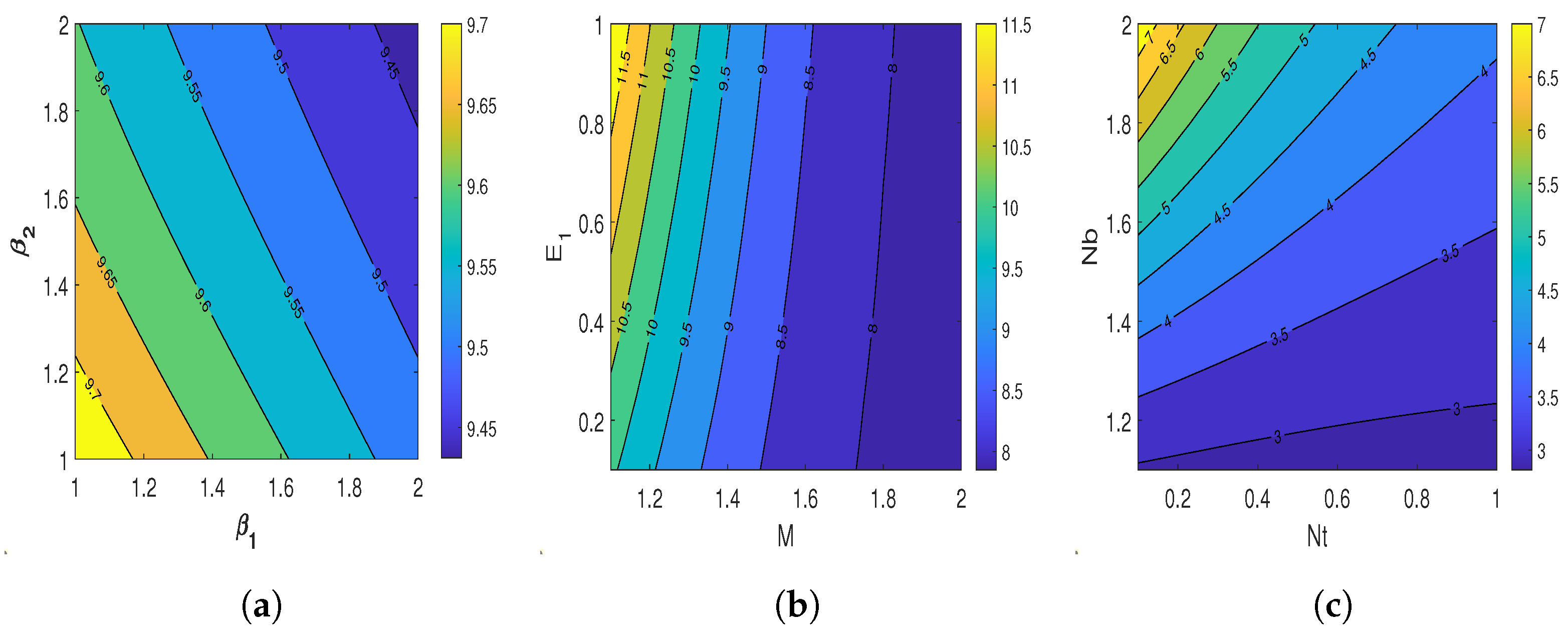
- Nusselt number enlarges with the enhancement in , , and . Moreover, it diminishes with a higher , , , , and M.
- Sherwood number escalates with the augmenting values of and . Moreover, it will reduce with augmenting values of K.
- improves with the growth in , , and , while it decreases with .
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Brinkmann number | Exponential heat source parameter | ||
| Ambient temperature (unit: K) | Sherwood number | ||
| Ambient concentration (unit: mol/m ) | Temperature at the surface of the wall (unit: K) | ||
| Skin friction coefficient | Stretching sheet velocity (unit: m/s) | ||
| Concentration at the surface of the wall (unit: mol/m ) | Velocity component in x-direction (unit: m/s) | ||
| Specific heat capacity (unit: mol Jkg K ) | Velocity component in y-direction (unit: m/s) | ||
| Brownian diffusion coefficient (unit: kg m s) | Greek Letters | ||
| Thermophoresis diffusion coefficient (unit: kg m s K) | Thickness parameter | ||
| Electric field parameter | Thermal diffusivity (unit: s/m) | ||
| Dimensional activation energy (unit: KJ/mol ) | Buoyancy force due to concentration (unit: K) | ||
| Nondimensional activation energy | Buoyancy force due to temperature (unit: K ) | ||
| Eckert number | Relaxation and retardation time ratio parameter | ||
| Dimensionless velocity | Retardation time parameter | ||
| Grashof number | Similarity variable | ||
| Solutal Grashof number | Thermal conductivity (unit: W/(m.K)) | ||
| Chemical reaction rate (unit: mol/s) | Dynamic viscosity (unit: Pa.s) | ||
| K | Chemical reaction parameter | Fluids’ kinematic viscosity (unit: m · s) | |
| Lewis number | Relaxation and retardation time ratio | ||
| Bioconvection Lewis number | Microorganism diffusion parameter | ||
| m | Fitted rate constant | Retardation time (unit: s) | |
| M | Magnetic field parameter | Dimensionless concentration (unit: mol/m ) | |
| Thermophoresis diffusion parameter | Density of fluid (unit: Kg/m) | ||
| Nusselt number | Stream function (unit: Kg/(m.s)) | ||
| Brownian diffusion parameter | Electrical conductivity (unit: S/m ) | ||
References
- Mushtaq, A.; Mustafa, M.; Hayat, T.; Alsaedi, A. Nonlinear radiative heat transfer in the flow of nanofluid due to solar energy: A numerical study. J. Taiwan Inst. Chem. Eng. 2014, 45, 1176–1183. [Google Scholar] [CrossRef]
- Shehzad, S.A.; Hayat, T.; Alsaedi, A.; Obid, M.A. Nonlinear thermal radiation in three-dimensional flow of Jeffrey nanofluid: A model for solar energy. Appl. Math. Comput. 2014, 248, 273–286. [Google Scholar] [CrossRef]
- Khan, J.A.; Mustafa, M.; Hayat, T.; Farooq, M.A.; Alsaedi, A.; Liao, S. On model for three-dimensional flow of nanofluid: An application to solar energy. J. Mol. Liq. 2014, 194, 41–47. [Google Scholar] [CrossRef]
- Khan, J.A.; Mustafa, M.; Hayat, T.; Alsaedi, A. Three-dimensional flow of nanofluid over a non-linearly stretching sheet: An application to solar energy. Int. J. Heat Mass Transf. 2015, 86, 158–164. [Google Scholar] [CrossRef]
- Zin, N.A.M.; Khan, I.; Shafie, S. The impact silver nanoparticles on MHD free convection flow of Jeffrey fluid over an oscillating vertical plate embedded in a porous medium. J. Mol. Liq. 2016, 222, 138–150. [Google Scholar]
- Reddy, K.; Kamnapure, N.R.; Srivastava, S. Nanofluid and nanocomposite applications in solar energy conversion systems for performance enhancement: A review. Int. J. Low-Carbon Technol. 2017, 12, 1–23. [Google Scholar] [CrossRef] [Green Version]
- Daniel, Y.S.; Aziz, Z.A.; Ismail, Z.; Salah, F. Impact of thermal radiation on electrical MHD flow of nanofluid over nonlinear stretching sheet with variable thickness. Alex. Eng. J. 2018, 57, 2187–2197. [Google Scholar] [CrossRef]
- Wahab, A.; Hassan, A.; Qasim, M.A.; Ali, H.M.; Babar, H.; Sajid, M.U. Solar energy systems–potential of nanofluids. J. Mol. Liq. 2019, 289, 111049. [Google Scholar] [CrossRef]
- Azam, M.; Shakoor, A.; Rasool, H.; Khan, M. Numerical simulation for solar energy aspects on unsteady convective flow of MHD Cross nanofluid: A revised approach. Int. J. Heat Mass Transf. 2019, 131, 495–505. [Google Scholar] [CrossRef]
- Sunitha, G. Influence of thermal radiation on peristaltic blood flow of a Jeffrey fluid with double diffusion in the presence of gold nanoparticles. Informatics Med. Unlocked 2019, 17, 100272. [Google Scholar]
- Acharya, N. On the flow patterns and thermal behaviour of hybrid nanofluid flow inside a microchannel in presence of radiative solar energy. J. Therm. Anal. Calorim. 2020, 141, 1425–1442. [Google Scholar] [CrossRef]
- Song, Y.Q.; Obideyi, B.; Shah, N.A.; Animasaun, I.; Mahrous, Y.; Chung, J.D. Significance of haphazard motion and thermal migration of alumina and copper nanoparticles across the dynamics of water and ethylene glycol on a convectively heated surface. Case Stud. Therm. Eng. 2021, 26, 101050. [Google Scholar] [CrossRef]
- Jamshed, W.; Eid, M.R.; Safdar, R.; Pasha, A.A.; Mohamed Isa, S.S.P.; Adil, M.; Rehman, Z.; Weera, W. Solar energy optimization in solar-HVAC using Sutterby hybrid nanofluid with Smoluchowski temperature conditions: A solar thermal application. Sci. Rep. 2022, 12, 11484. [Google Scholar] [CrossRef] [PubMed]
- Sharma, B.K.; Poonam; Chamkha, A.J. Effects of heat transfer, body acceleration and hybrid nanoparticles (Au-Al2O3) on MHD blood flow through a curved artery with stenosis and aneurysm using hematocrit-dependent viscosity. Waves Random Complex Media 2022, 1–31. [Google Scholar] [CrossRef]
- Gandhi, R.; Sharma, B.; Kumawat, C.; Bég, O.A. Modeling and analysis of magnetic hybrid nanoparticle (Au-Al2O3/blood) based drug delivery through a bell-shaped occluded artery with Joule heating, viscous dissipation and variable viscosity effects. Proc. Inst. Mech. Eng. Part E J. Process. Mech. Eng. 2022, 236, 09544089221080273. [Google Scholar] [CrossRef]
- Sharma, B.; Kumawat, C.; Vafai, K. Computational biomedical simulations of hybrid nanoparticles (Au-Al2O3/blood-mediated) transport in a stenosed and aneurysmal curved artery with heat and mass transfer: Hematocrit dependent viscosity approach. Chem. Phys. Lett. 2022, 800, 139666. [Google Scholar]
- Bhatti, M.; Ellahi, R.; Doranehgard, M.H. Numerical study on the hybrid nanofluid (Co3O4-Go/H2O) flow over a circular elastic surface with non-Darcy medium: Application in solar energy. J. Mol. Liq. 2022, 361, 119655. [Google Scholar] [CrossRef]
- Bouslimi, J.; Alkathiri, A.A.; Althagafi, T.M.; Jamshed, W.; Eid, M.R. Thermal properties, flow and comparison between Cu and Ag nanoparticles suspended in sodium alginate as Sutterby nanofluids in solar collector. Case Stud. Therm. Eng. 2022, 39, 102358. [Google Scholar] [CrossRef]
- Shahzad, F.; Jamshed, W.; Eid, M.R.; Safdar, R.; Putri Mohamed Isa, S.S.; El Din, S.M.; Mohd Nasir, N.A.A.; Iqbal, A. Thermal cooling efficacy of a solar water pump using Oldroyd-B (aluminum alloy-titanium alloy/engine oil) hybrid nanofluid by applying new version for the model of Buongiorno. Sci. Rep. 2022, 12, 19817. [Google Scholar] [CrossRef]
- Pakravan, H.A.; Yaghoubi, M. Combined thermophoresis, Brownian motion and Dufour effects on natural convection of nanofluids. Int. J. Therm. Sci. 2011, 50, 394–402. [Google Scholar] [CrossRef]
- Anbuchezhian, N.; Srinivasan, K.; Chandrasekaran, K.; Kandasamy, R. Thermophoresis and Brownian motion effects on boundary layer flow of nanofluid in presence of thermal stratification due to solar energy. Appl. Math. Mech. 2012, 33, 765–780. [Google Scholar] [CrossRef]
- Kandasamy, R.; Muhaimin, I.; Mohamad, R. Thermophoresis and Brownian motion effects on MHD boundary-layer flow of a nanofluid in the presence of thermal stratification due to solar radiation. Int. J. Mech. Sci. 2013, 70, 146–154. [Google Scholar] [CrossRef] [Green Version]
- Hayat, T.; Asad, S.; Alsaedi, A. Analysis for flow of Jeffrey fluid with nanoparticles. Chin. Phys. B 2015, 24, 044702. [Google Scholar] [CrossRef]
- Mabood, F.; Ibrahim, S.; Khan, W. Framing the features of Brownian motion and thermophoresis on radiative nanofluid flow past a rotating stretching sheet with magnetohydrodynamics. Results Phys. 2016, 6, 1015–1023. [Google Scholar] [CrossRef] [Green Version]
- Sulochana, C.; Ashwinkumar, G.; Sandeep, N. Transpiration effect on stagnation-point flow of a Carreau nanofluid in the presence of thermophoresis and Brownian motion. Alex. Eng. J. 2016, 55, 1151–1157. [Google Scholar] [CrossRef] [Green Version]
- Astanina, M.; Abu-Nada, E.; Sheremet, M. Combined effects of thermophoresis, brownian motion, and nanofluid variable properties on CuO-water nanofluid natural convection in a partially heated square cavity. J. Heat Transf. 2018, 140, 082401. [Google Scholar] [CrossRef]
- Awan, S.E.; Raja, M.A.Z.; Mehmood, A.; Niazi, S.A.; Siddiqa, S. Numerical treatments to analyze the nonlinear radiative heat transfer in MHD nanofluid flow with solar energy. Arab. J. Sci. Eng. 2020, 45, 4975–4994. [Google Scholar] [CrossRef]
- Rekha, M.; Sarris, I.E.; Madhukesh, J.; Raghunatha, K.; Prasannakumara, B. Impact of thermophoretic particle deposition on heat transfer and nanofluid flow through different geometries: An application to solar energy. Chin. J. Phys. 2022, 80, 190–205. [Google Scholar] [CrossRef]
- Sharma, B.; Kumar, A.; Gandhi, R.; Bhatti, M. Exponential space and thermal-dependent heat source effects on electro-magneto-hydrodynamic Jeffrey fluid flow over a vertical stretching surface. Int. J. Mod. Phys. B 2022, 36, 2250220. [Google Scholar] [CrossRef]
- Sharma, B.; Khanduri, U.; Mishra, N.K.; Mekheimer, K.S. Combined effect of thermophoresis and Brownian motion on MHD mixed convective flow over an inclined stretching surface with radiation and chemical reaction. Int. J. Mod. Phys. B 2022, 2350095. [Google Scholar] [CrossRef]
- Sharma, B.; Gandhi, R.; Mishra, N.K.; Al-Mdallal, Q.M. Entropy generation minimization of higher-order endothermic/exothermic chemical reaction with activation energy on MHD mixed convective flow over a stretching surface. Sci. Rep. 2022, 12, 17688. [Google Scholar] [CrossRef] [PubMed]
- Parvin, S.; Nasrin, R.; Alim, M. Heat transfer and entropy generation through nanofluid filled direct absorption solar collector. Int. J. Heat Mass Transf. 2014, 71, 386–395. [Google Scholar] [CrossRef]
- Khan, M.I.; Hayat, T.; Khan, M.I.; Waqas, M.; Alsaedi, A. Numerical simulation of hydromagnetic mixed convective radiative slip flow with variable fluid properties: A mathematical model for entropy generation. J. Phys. Chem. Solids 2019, 125, 153–164. [Google Scholar] [CrossRef]
- Wang, W.W.; Cai, Y.; Wang, L.; Liu, C.W.; Zhao, F.Y.; Sheremet, M.A.; Liu, D. A two-phase closed thermosyphon operated with nanofluids for solar energy collectors: Thermodynamic modeling and entropy generation analysis. Sol. Energy 2020, 211, 192–209. [Google Scholar] [CrossRef]
- Naz, R.; Noor, M.; Shah, Z.; Sohail, M.; Kumam, P.; Thounthong, P. Entropy generation optimization in MHD pseudoplastic fluid comprising motile microorganisms with stratification effect. Alex. Eng. J. 2020, 59, 485–496. [Google Scholar] [CrossRef]
- Farooq, U.; Munir, S.; Malik, F.; Ahmad, B.; Lu, D. Aspects of entropy generation for the non-similar three-dimensional bioconvection flow of nanofluids. AIP Adv. 2020, 10, 075110. [Google Scholar] [CrossRef]
- Yusuf, T.A.; Mabood, F.; Prasannakumara, B.; Sarris, I.E. Magneto-bioconvection flow of Williamson nanofluid over an inclined plate with gyrotactic microorganisms and entropy generation. Fluids 2021, 6, 109. [Google Scholar] [CrossRef]
- Li, Y.X.; Khan, M.I.; Gowda, R.P.; Ali, A.; Farooq, S.; Chu, Y.M.; Khan, S.U. Dynamics of aluminum oxide and copper hybrid nanofluid in nonlinear mixed Marangoni convective flow with entropy generation: Applications to renewable energy. Chin. J. Phys. 2021, 73, 275–287. [Google Scholar] [CrossRef]
- Sharma, B.; Gandhi, R.; Bhatti, M. Entropy analysis of thermally radiating MHD slip flow of hybrid nanoparticles (Au-Al2O3/Blood) through a tapered multi-stenosed artery. Chem. Phys. Lett. 2022, 790, 139348. [Google Scholar] [CrossRef]
- Khanduri, U.; Sharma, B.K. Entropy Analysis for MHD Flow Subject to Temperature-Dependent Viscosity and Thermal Conductivity. In Nonlinear Dynamics and Applications; Springer: Berlin/Heidelberg, Germany, 2022; pp. 457–471. [Google Scholar]
- Salawu, S.; Obalalu, A.; Shamshuddin, M. Nonlinear solar thermal radiation efficiency and energy optimization for magnetized hybrid Prandtl–Eyring nanoliquid in aircraft. Arab. J. Sci. Eng. 2022, 1–12. [Google Scholar] [CrossRef]
- Avramenko, A.; Kuznetsov, A. Stability of a suspension of gyrotactic microorganisms in superimposed fluid and porous layers. Int. Commun. Heat Mass Transf. 2004, 31, 1057–1066. [Google Scholar] [CrossRef]
- Avramenko, A.; Kuznetsov, A. The onset of bio-thermal convection in a suspension of gyrotactic microorganisms in a fluid layer with an inclined temperature gradient. Int. J. Numer. Methods Heat Fluid Flow 2010, 20, 111–129. [Google Scholar] [CrossRef]
- Mutuku, W.N.; Makinde, O.D. Hydromagnetic bioconvection of nanofluid over a permeable vertical plate due to gyrotactic microorganisms. Comput. Fluids 2014, 95, 88–97. [Google Scholar] [CrossRef]
- Acharya, N.; Das, K.; Kundu, P.K. Framing the effects of solar radiation on magneto-hydrodynamics bioconvection nanofluid flow in presence of gyrotactic microorganisms. J. Mol. Liq. 2016, 222, 28–37. [Google Scholar] [CrossRef]
- Saleem, S.; Rafiq, H.; Al-Qahtani, A.; El-Aziz, M.A.; Malik, M.; Animasaun, I. Magneto Jeffrey nanofluid bioconvection over a rotating vertical cone due to gyrotactic microorganism. Math. Probl. Eng. 2019, 2019, 3478037. [Google Scholar] [CrossRef]
- Sohail, M.; Naz, R.; Abdelsalam, S.I. On the onset of entropy generation for a nanofluid with thermal radiation and gyrotactic microorganisms through 3D flows. Phys. Scr. 2020, 95, 045206. [Google Scholar] [CrossRef]
- Song, Y.Q.; Hamid, A.; Khan, M.I.; Gowda, R.P.; Kumar, R.N.; Prasannakumara, B.; Khan, S.U.; Khan, M.I.; Malik, M. Solar energy aspects of gyrotactic mixed bioconvection flow of nanofluid past a vertical thin moving needle influenced by variable Prandtl number. Chaos Solitons Fractals 2021, 151, 111244. [Google Scholar] [CrossRef]
- Naidu, K.K.; Babu, D.H.; Reddy, S.H.; Narayana, P.S. Radiation and partial slip effects on magnetohydrodynamic Jeffrey nanofluid containing gyrotactic microorganisms over a stretching surface. J. Therm. Sci. Eng. Appl. 2021, 13, 031011. [Google Scholar] [CrossRef]
- Sharma, B.K.; Khanduri, U.; Mishra, N.K.; Chamkha, A.J. Analysis of Arrhenius activation energy on magnetohydrodynamic gyrotactic microorganism flow through porous medium over an inclined stretching sheet with thermophoresis and Brownian motion. Proc. Inst. Mech. Eng. Part E J. Process. Mech. Eng. 2022, 09544089221128768. [Google Scholar] [CrossRef]
- Bhatti, M.; Arain, M.; Zeeshan, A.; Ellahi, R.; Doranehgard, M. Swimming of Gyrotactic Microorganism in MHD Williamson nanofluid flow between rotating circular plates embedded in porous medium: Application of thermal energy storage. J. Energy Storage 2022, 45, 103511. [Google Scholar] [CrossRef]
- Hussain, A.; Farooq, N. Gyrotactic micro-organisms swimming under the Hyperbolic Tangent Blood Nano Material and Solar biomimetic system over the Esophagus. Int. Commun. Heat Mass Transf. 2023, 141, 106579. [Google Scholar] [CrossRef]
- Shahzad, F.; Jamshed, W.; Nisar, K.S.; Khashan, M.M.; Abdel-Aty, A.H. Computational analysis of Ohmic and viscous dissipation effects on MHD heat transfer flow of Cu-PVA Jeffrey nanofluid through a stretchable surface. Case Stud. Therm. Eng. 2021, 26, 101148. [Google Scholar] [CrossRef]
- Ali, A.; Maqsood, M.; Anjum, H.; Awais, M.; Sulaiman, M. Analysis of heat transfer on MHD Jeffrey nanofluid flow over nonlinear elongating surface of variable thickness. ZAMM-J. Appl. Math. Mech. Angew. Math. Mech. 2022, 102, e202100250. [Google Scholar] [CrossRef]
- Muhammad, T.; Waqas, H.; Manzoor, U.; Farooq, U.; Rizvi, Z.F. On doubly stratified bioconvective transport of Jeffrey nanofluid with gyrotactic motile microorganisms. Alex. Eng. J. 2022, 61, 1571–1583. [Google Scholar] [CrossRef]




| Magnetic field parameter, | Electric field parameter, |
| Grashof number, | Solutal Grashof number, |
| Bioconvection Rayleigh parameter, | Radiation parameter, |
| Eckert number, | Prandtl number, |
| Temperature difference parameter, | Exponential heat source parameter, |
| Brownian diffusion parameter, | Thermophoresis diffusion parameter, |
| Chemical reaction parameter, | Lewis number, |
| Bioconvection Lewis number, | Peclet number, |
| Diffusion parameter, | Bioconvection diffusion parameter, |
| Concentration difference parameter, | Microorganism concentration difference parameter, |
| Properties | Mathematical Expression for Nanofluid |
|---|---|
| Viscosity | |
| Density | |
| Heat Capacity | |
| Thermal Conductivity | |
| Electrical Conductivity | |
| Thermal Expansion Coefficient | |
| Concentration Thermal Expansion Coefficient | |
| Microorganism Thermal Expansion Coefficient |
| Physical Properties | Copper | PVA | Water |
|---|---|---|---|
| Density [ (kg/m)] | 8933 | 1020 | 997 |
| Thermal Conductivity [ (W/mK)] | 400 | 0.2 | 0.613 |
| Electrical Conductivity [ (S/m)] | 5.96 × 107 | 11.7 × 10−6 | 0.05 |
| Thermal Expansion Coefficient [ 10 (K] | 1.67 | 2.5 | 21 |
| Specific Heat Capacity [ (J/kgK)] | 385 | 2000 | 4179 |
| Parameters | Values | Parameters | Values | Parameters | Values | Parameters | Values |
|---|---|---|---|---|---|---|---|
| 0.7 | 7.743 | 1 | 1 | ||||
| 0.5 | 1 | 0.3 | M | 0.1 | |||
| 0.3 | 0.1 | n | 0.5 | 0.1 | |||
| m | 0.9 | 1 | K | 0.5 | 0.01 | ||
| 1 | 0.5 | 1 | 0.3 | ||||
| L | 1 | 1 | 3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sharma, B.K.; Kumar, A.; Gandhi, R.; Bhatti, M.M.; Mishra, N.K. Entropy Generation and Thermal Radiation Analysis of EMHD Jeffrey Nanofluid Flow: Applications in Solar Energy. Nanomaterials 2023, 13, 544. https://doi.org/10.3390/nano13030544
Sharma BK, Kumar A, Gandhi R, Bhatti MM, Mishra NK. Entropy Generation and Thermal Radiation Analysis of EMHD Jeffrey Nanofluid Flow: Applications in Solar Energy. Nanomaterials. 2023; 13(3):544. https://doi.org/10.3390/nano13030544
Chicago/Turabian StyleSharma, Bhupendra Kumar, Anup Kumar, Rishu Gandhi, Muhammad Mubashir Bhatti, and Nidhish Kumar Mishra. 2023. "Entropy Generation and Thermal Radiation Analysis of EMHD Jeffrey Nanofluid Flow: Applications in Solar Energy" Nanomaterials 13, no. 3: 544. https://doi.org/10.3390/nano13030544
APA StyleSharma, B. K., Kumar, A., Gandhi, R., Bhatti, M. M., & Mishra, N. K. (2023). Entropy Generation and Thermal Radiation Analysis of EMHD Jeffrey Nanofluid Flow: Applications in Solar Energy. Nanomaterials, 13(3), 544. https://doi.org/10.3390/nano13030544







