Influence of Stress Jump Condition at the Interface Region of a Two-Layer Nanofluid Flow in a Microchannel with EDL Effects
Abstract
:1. Introduction
2. Problem Formulation
- Region I:
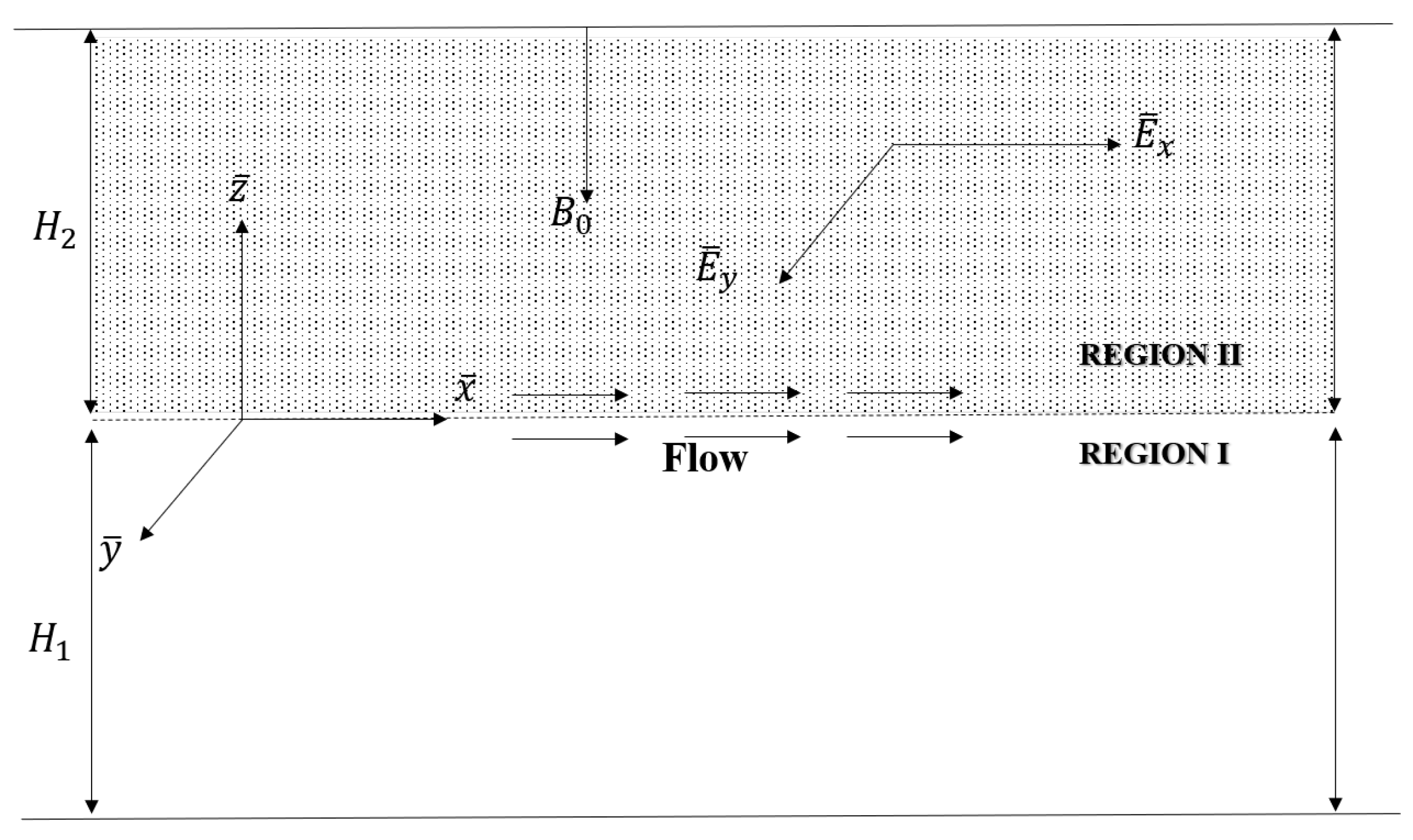
2.1. Problem Statement and Assumptions
- The direction of the flow is assumed to be along the x-axis.
- The flow velocity in the -direction is negligible, since the length of microchannel L is much larger than its height H. Hence ,
- The velocity component in the -direction is considered to be zero, i.e., ,
- The flow is assumed to uni-directional along the -axis but its properties changes with respect to the -axis, hence ,
- The body force, , represents the sum of electro-osmosis and the electromagnetic forces, where is the electric field, is the applied magnetic field, and is the current density of the ion.
- The inertial effects in the porous region of the microchannel (Region II) are negligible.
- Region I of the channel is filled with nanofluid, while the channel’s Region II is filled with the porous medium saturated with nanofluid, having uniform permeability only.
- Proceeding from the analysis presented in [26], the stress jump condition is utilized at the interface. Simultaneously, the electric potential, temperature, nanoparticle concentration, and flux at the interface are presumed to be continuous. Finally, the no-slip condition is applied to the velocity boundaries, while the temperature and nanoparticle concentration are assumed to have a constant distribution on the boundaries.
- Region I: ()
- when :
2.2. Problem Non-Dimensionalization
- Region I :
- when :
2.3. Skin Friction Coefficient and Nusselt Number
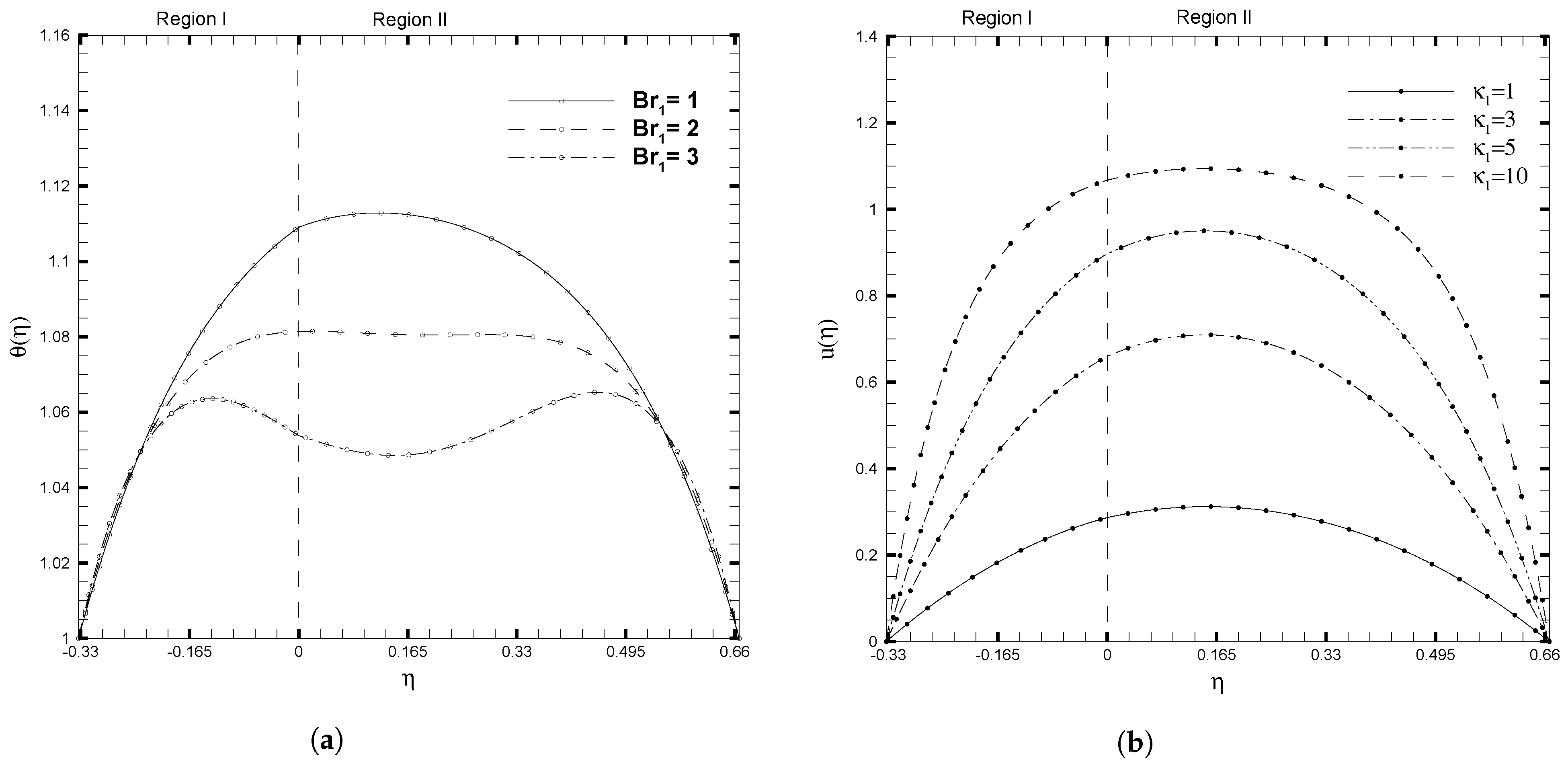
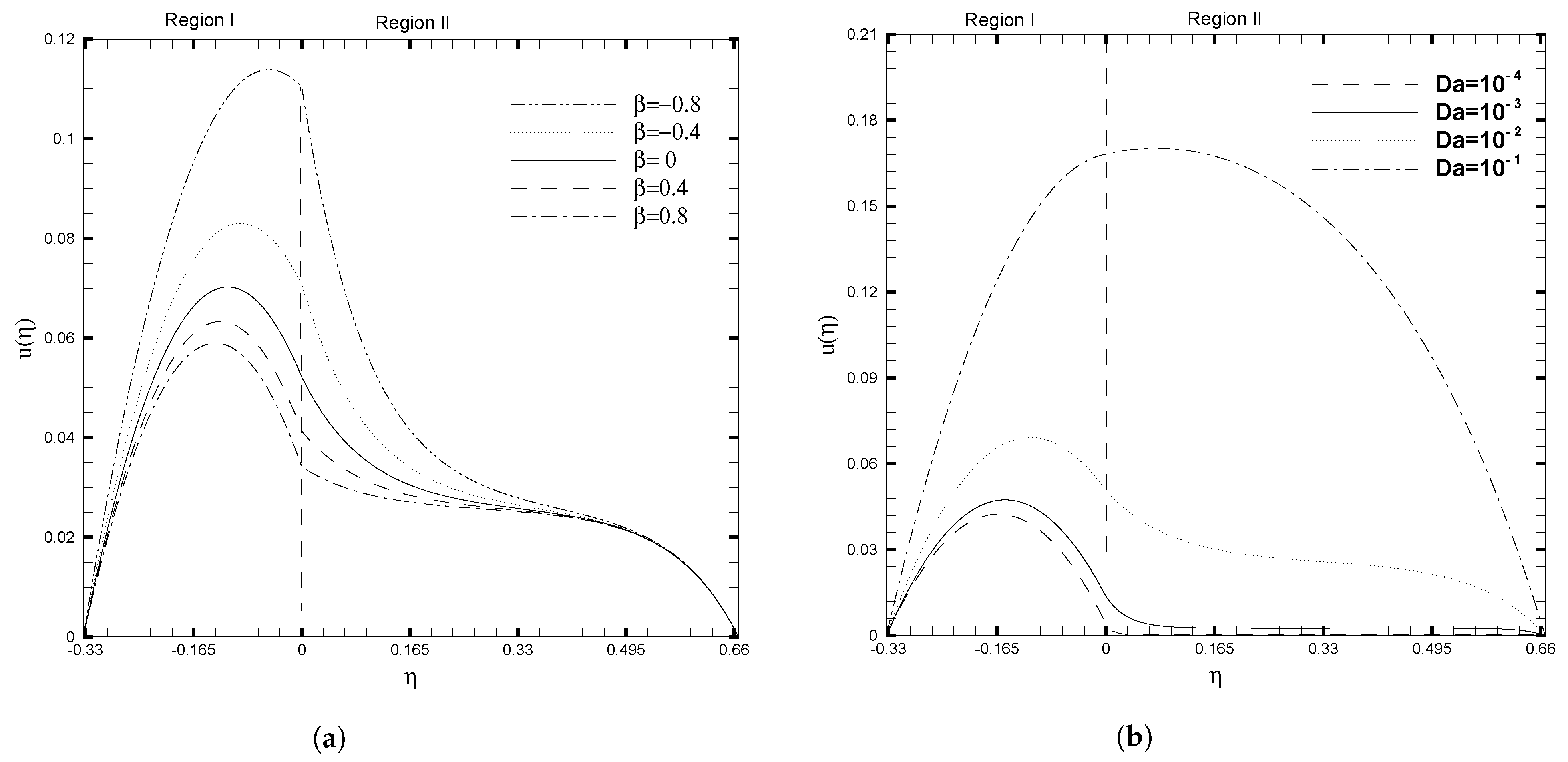
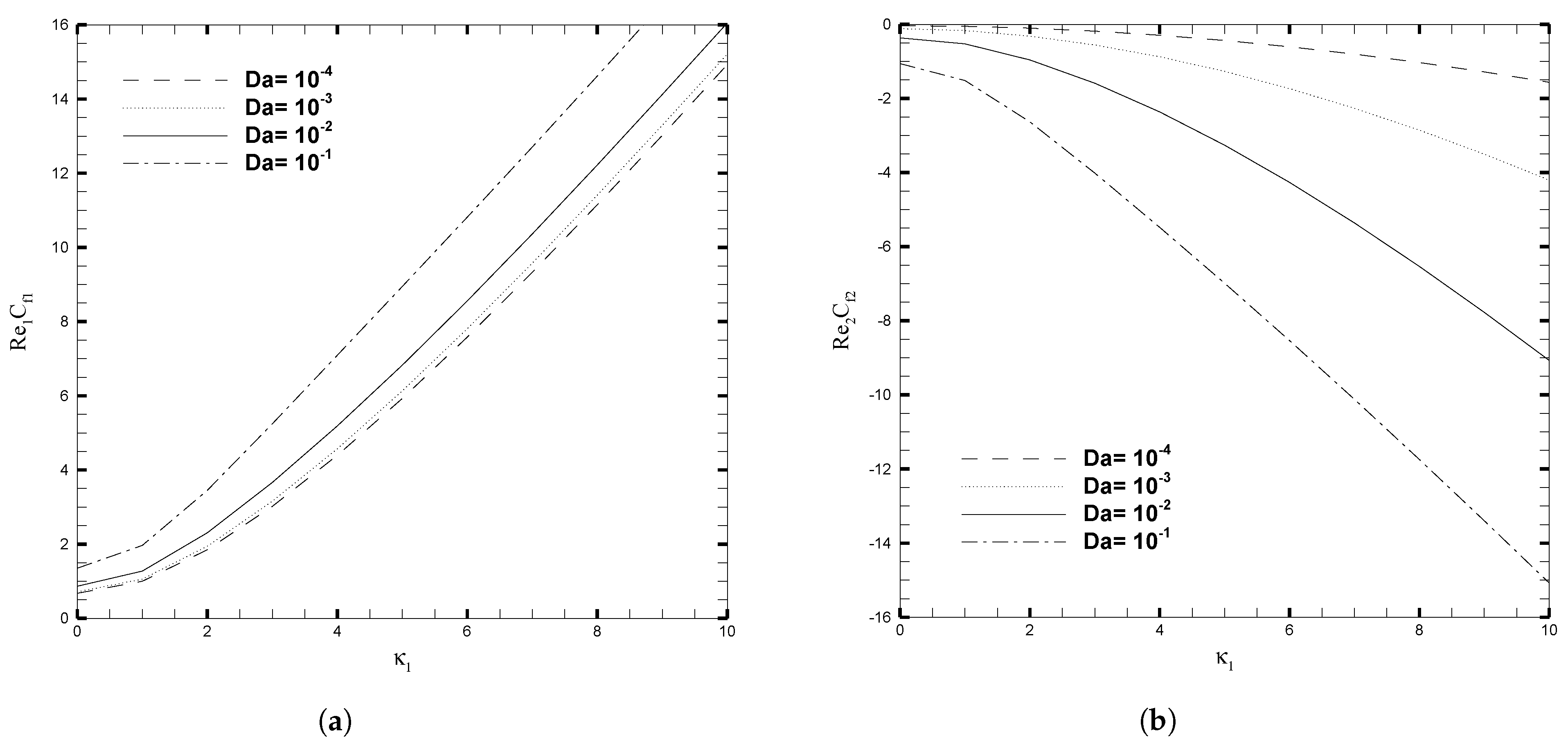
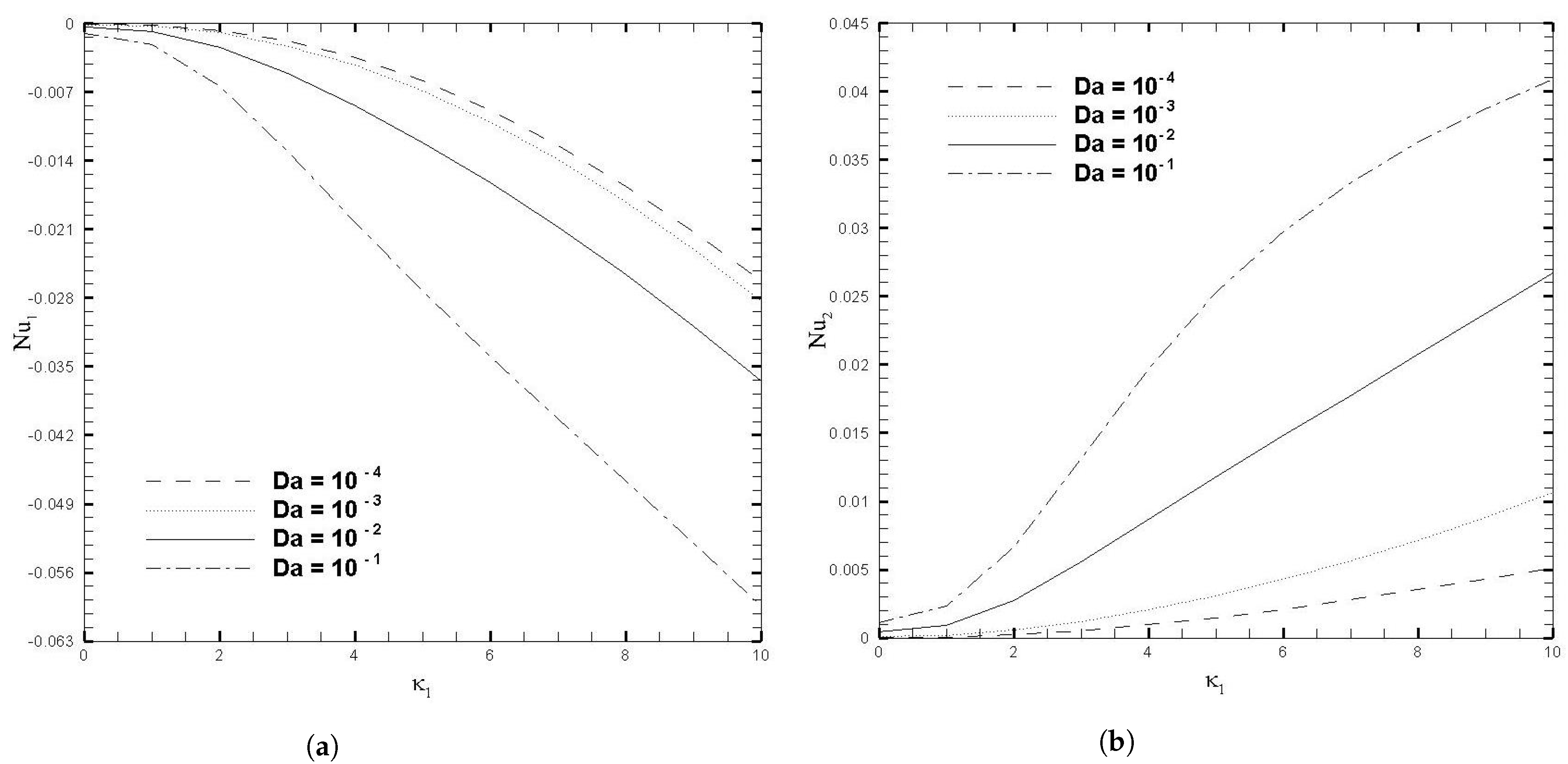
3. Problem Solution
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| volumetric fractions of nanoparticles; | |
| local skin friction coefficients; | |
| Brownian diffusion coefficients; | |
| thermophoretic diffusion coefficients; | |
| pressure, Pa; | |
| non-dimensional nanofluid temperatures in two regions, K; | |
| non-dimensional velocities of the fluid, ; | |
| Brinkman numbers; | |
| magnetic field in z-direction; | |
| Darcy number; | |
| fluid and nanoparticle specific heats; | |
| e | charge of a proton; |
| reference volume fraction for nanoparticles; | |
| volume fraction for nanoparticles on the microchannel walls; | |
| non-dimensional external electric field parameter; | |
| electric field in x− and y−directions, respectively; | |
| body forces caused by uniform electromagnetic field; | |
| H | channel height; |
| channel height of two regions; | |
| non-dimensional heights of two regions; | |
| Hartman numbers; | |
| Boltzmann constant; | |
| fluid’s thermal conductivity in two regions; | |
| the ratio of the fluid’s thermal conductivities; | |
| L | microchannel length; |
| bulk ionic concentration; | |
| Brownian motion parameters; | |
| thermophoresis parameters; | |
| local Nusselt numbers; | |
| P | non-dimensional pressure gradient; |
| heat flux on the channel walls; | |
| Reynolds numbers; | |
| lateral direction electric field strengths; | |
| average velocities of the fluid; | |
| velocity of fluid in two regions; | |
| W | microchannel’s width; |
| Cartesian coordinates; | |
| ion valency. | |
| thermal diffusivity of the nanofluid, ; | |
| porosity of the porous region; | |
| permittivity of vacuum, ; | |
| medium’s dielectric constants; | |
| , | medium’s dielectric constant ratio; |
| permeability of the porous region; | |
| constant coefficient; | |
| the adjustable stress jump coefficient; | |
| dimensional electrostatic potential, V; | |
| nanoparticles density, ; | |
| nanofluid’s density, ; | |
| densities of charges, ; | |
| non-dimensional spatial variable; | |
| non-dimensional pressure gradient parameters; | |
| electro-osmotic parameters; | |
| the ratio of the any physical quantity N, where ; | |
| fluid’s dynamic viscosity in two regions; | |
| the ratio of viscosity to porosity in Region II; | |
| dimensionless reference temperature; | |
| temperature distributions (non-dimensional); | |
| non-dimensional nano-particle volume fractions; | |
| densities of the charges; | |
| shear stresses on channel’s opposite walls; | |
| non-dimensional nano-particle volume fractions; | |
| zeta potentials (dimensional). | |
| zeta potentials (non-dimensional); | |
| viscous dissipation factors; | |
| indices for regions I and II; | |
| subscript notations for solids and fluids; | |
| w | indicate the quantities on walls of the channel. |
| electric double layer; | |
| finite difference method. |
References
- Manikandan, S.; Rajan, K.S. MgO-therminol 55 nanofluids for efficient energy management: Analysis of transient heat transfer performance. Energy 2015, 88, 408–416. [Google Scholar] [CrossRef]
- Karimipour, A.; Nezhad, A.H.; Dorazio, A.; Esfe, M.H.; Safaei, M.R.; Shirani, E. Simulation of copper-water nanofluid in a microchannel in slip flow regime using the lattice Boltzmann method. Eur. J. -Mech.-B/Fluids 2015, 49, 89–99. [Google Scholar] [CrossRef]
- Hunter, R.J. Zeta Potential in Colloid Science: Principles and Applications; Harcour Brace Jovanovich: San Diego, CA, USA, 2013; Volume 2. [Google Scholar]
- Mala, G.M.; Li, D.; Dale, J.D. Heat transfer and fluid flow in microchannels. Int. J. Heat Mass Transf. 1997, 40, 3079–3088. [Google Scholar] [CrossRef]
- Mala, G.M.; Li, D. Flow characteristics of water in microtubes. Int. J. Heat Fluid Flow 1999, 20, 142–148. [Google Scholar] [CrossRef]
- Ren, L.Q.; Qu, W.L.; Li, D.Q. Interfacial electrokinetic effects on liquid flow in microchannels. Int. J. Heat Mass Transf. 2001, 44, 3125–3134. [Google Scholar] [CrossRef]
- Darabi, J.; Ekula, K. Development of a chip-integrated micro cooling device. Microelectron. J. 2003, 34, 1067–1074. [Google Scholar] [CrossRef]
- Donaldson, L. Small and powerful nuclear battery developed. Mater. Today 2009, 12, 10. [Google Scholar] [CrossRef]
- Wang, B.X.; Peng, X.F. Experimental investigation on liquid forced-convection heat transfer through microchannels. Int. J. Heat Mass Transf. 1994, 37, 73–82. [Google Scholar] [CrossRef]
- Guo, Z.Y.; Li, Z.X. Size effect on single-phase channel flow and heat transfer at microscale. Int. J. Heat Fluid Flow 2003, 24, 284–298. [Google Scholar] [CrossRef]
- Ren, C.L.; Li, D. Improved understanding of the effect of electrical double layer on pressure driven flow in microchannels. Anal. Chim. Acta 2005, 531, 15–23. [Google Scholar] [CrossRef]
- Gao, Y.; Wong, T.N.; Yang, C.; Ooi, K.T. Two-fluid electroosmotic flow in microchannels. J. Colloid Interface Sci. 2005, 284, 306–314. [Google Scholar] [CrossRef] [PubMed]
- Gao, Y.; Wang, C.; Wong, T.N.; Yang, C.; Nguyen, N.T.; Ooi, K.T. Electroosmotic control of the interface position of two-liquid flow through a microchannel. J. Micromech. Microeng. 2007, 17, 358–366. [Google Scholar] [CrossRef]
- Gaikwad, H.; Basu, D.N.; Mondal, P.K. Electroosmotic transport of immiscible binary system with a layer of non-conducting fluid under interfacial slip: The role applied pressure gradient. Electrophoresis 2016, 37, 1998–2009. [Google Scholar] [CrossRef] [PubMed]
- Gaikwad, H.S.; Basu, D.N.; Mondal, P.K. Slip driven micro-pumping of binary system with a layer of non-conducting fluid under electrical double layer phenomenon. Colloids Surf. Physicochem. Eng. Asp. 2017, 518, 166–172. [Google Scholar] [CrossRef]
- Xie, Z.Y.; Jian, Y.J. Entropy generation of two-layer magnetohydrodynamic electroosmotic flow through microparallel channels. Energy 2017, 139, 1080–1093. [Google Scholar] [CrossRef]
- Niazi, M.D.K.; Xu, H. Modelling two-layer nanofluid flow in a micro-channel with electro-osmotic effects by means of Buongiorno model. Appl. Math. Mech. 2020, 41, 83–104. [Google Scholar] [CrossRef]
- Buongiorno, J. Convective transport in nanofluids. J. Heat Transf. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Tahir, H.; Khan, U.; Anwarud, D.; Chu, Y.; Muhammad, N.; Li, X. Hybridized two phase ferromagnetic nanofluid with NiZnFe2O4 and MnZnFe2O4. Ain Shams Eng. J. 2021, 12, 3063–3070. [Google Scholar] [CrossRef]
- Younes, H.; Mao, M.; Murshed, S.; Lou, D.; Hong, H.; Peterson, G.P. Nanofluids: Key parameters to enhance thermal conductivity and its applications. Appl. Therm. Eng. 2022, 207, 118202. [Google Scholar] [CrossRef]
- Mohamad, A.A. High efficiency solar air heater. Sol. Energy 1997, 60, 1–76. [Google Scholar] [CrossRef]
- Beavers, G.S.; Joseph, D.D. Boundary conditions at a naturally permeable wall. J. Fluid Mech. 1967, 30, 197–207. [Google Scholar] [CrossRef]
- Vafai, K.; Thiyagaraja, R. Analysis of flow and heat transfer at the interface region of a porous medium. Int. J. Heat Mass Transf. 1987, 30, 1391–1405. [Google Scholar] [CrossRef]
- Vafai, K.; Kim, S.J. Fluid mechanics of the interface region between a porous medium and a fluid layer- An exact solution. Int. J. Heat Fluid Flow 1990, 11, 254–256. [Google Scholar] [CrossRef]
- Nield, D.A. The limitations of the Brinkman-Forchheimer equation in modeling flow in a saturated porous medium and at an interface. Int. J. Heat Fluid Flow 1991, 12, 269–272. [Google Scholar] [CrossRef]
- Kuznetsov, A.V. Analytical investigation of the fluid flow in the interface region between a porous medium and a clear fluid in channels partially filled with a porous medium. Appl. Sci. Res. 1996, 56, 53–67. [Google Scholar] [CrossRef]
- Ochoa-Tapia, J.A.; Whitaker, S. Momentum transfer at the boundary between a porous medium and a homogeneous fluid—I. Theoretical development. Int. J. Heat Mass Transf. 1995, 38, 2635–2646. [Google Scholar] [CrossRef]
- Ochoa-Tapia, J.A.; Whitaker, S. Momentum transfer at the boundary between a porous medium and a homogeneous fluid—II. Comparison with experiment. Int. J. Heat Mass Transf. 1995, 38, 2647–2655. [Google Scholar] [CrossRef]
- Dauenhauer, E.C.; Majdalani, J. Exact self-similarity solution of the Navier-Stokes equations for a porous channel with orthogonally moving walls. Phys. Fluids 2003, 151, 1485–1495. [Google Scholar] [CrossRef]
- Yang, W.Y.; Cao, W.; Kim, J.; Park, K.W.; Park, H.H.; Joung, J.; Ro, R.; Lee, H.L.; Hong, C.; Im, T. Applied Numerical Methods Using MATLAB; John Wiley & Sons: Hoboken, NJ, USA, 2005. [Google Scholar]





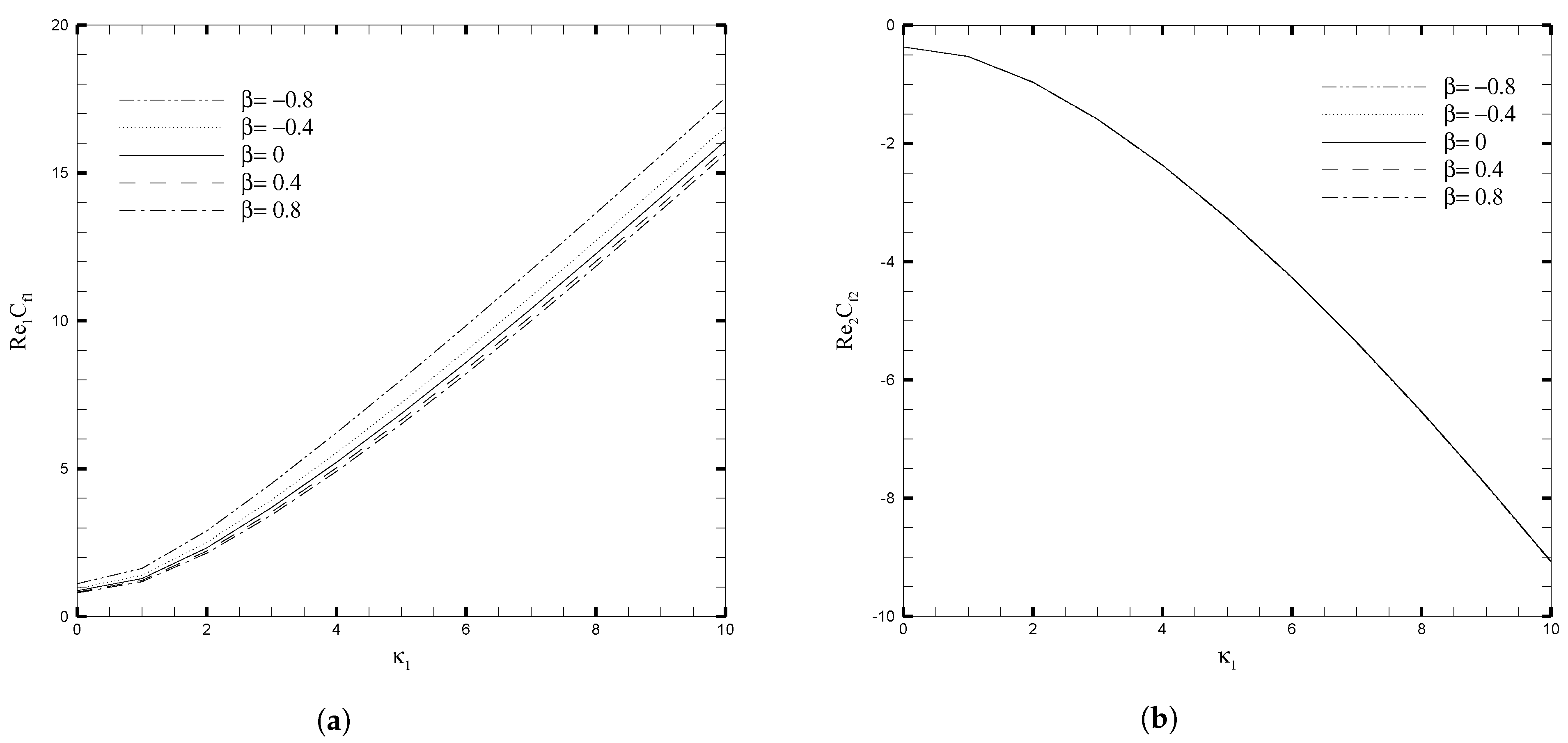

| Physical Characteristic | |||
|---|---|---|---|
| 4179.0 | 765.0 | 686.2 | |
| 997.1 | 3970.0 | 4250.0 | |
| 0.6130 | 40.0 | 8.9538 | |
| 1.47 | 131.70 | 30.70 | |
| 21.00 | 0.85 | 0.90 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Raees ul Haq, M.; Raees, A.; Xu, H.; Xiao, S. Influence of Stress Jump Condition at the Interface Region of a Two-Layer Nanofluid Flow in a Microchannel with EDL Effects. Nanomaterials 2023, 13, 1198. https://doi.org/10.3390/nano13071198
Raees ul Haq M, Raees A, Xu H, Xiao S. Influence of Stress Jump Condition at the Interface Region of a Two-Layer Nanofluid Flow in a Microchannel with EDL Effects. Nanomaterials. 2023; 13(7):1198. https://doi.org/10.3390/nano13071198
Chicago/Turabian StyleRaees ul Haq, Muhammad, Ammarah Raees, Hang Xu, and Shaozhang Xiao. 2023. "Influence of Stress Jump Condition at the Interface Region of a Two-Layer Nanofluid Flow in a Microchannel with EDL Effects" Nanomaterials 13, no. 7: 1198. https://doi.org/10.3390/nano13071198
APA StyleRaees ul Haq, M., Raees, A., Xu, H., & Xiao, S. (2023). Influence of Stress Jump Condition at the Interface Region of a Two-Layer Nanofluid Flow in a Microchannel with EDL Effects. Nanomaterials, 13(7), 1198. https://doi.org/10.3390/nano13071198






