Potentiometric Studies on Ion-Transport Selectivity in Charged Gold Nanotubes
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Electroless Plating and Measurement of Nanotube Inside Diameter
2.3. Potentiometric Measurements and Methods
2.4. Surface Contact Angle
2.5. X-ray Photoelectron Spectroscopy (XPS)
3. Results and Discussion
3.1. Dimensions of the Nanotubes and Surface Films
3.2. Surface Contact Angle and XPS Measurements
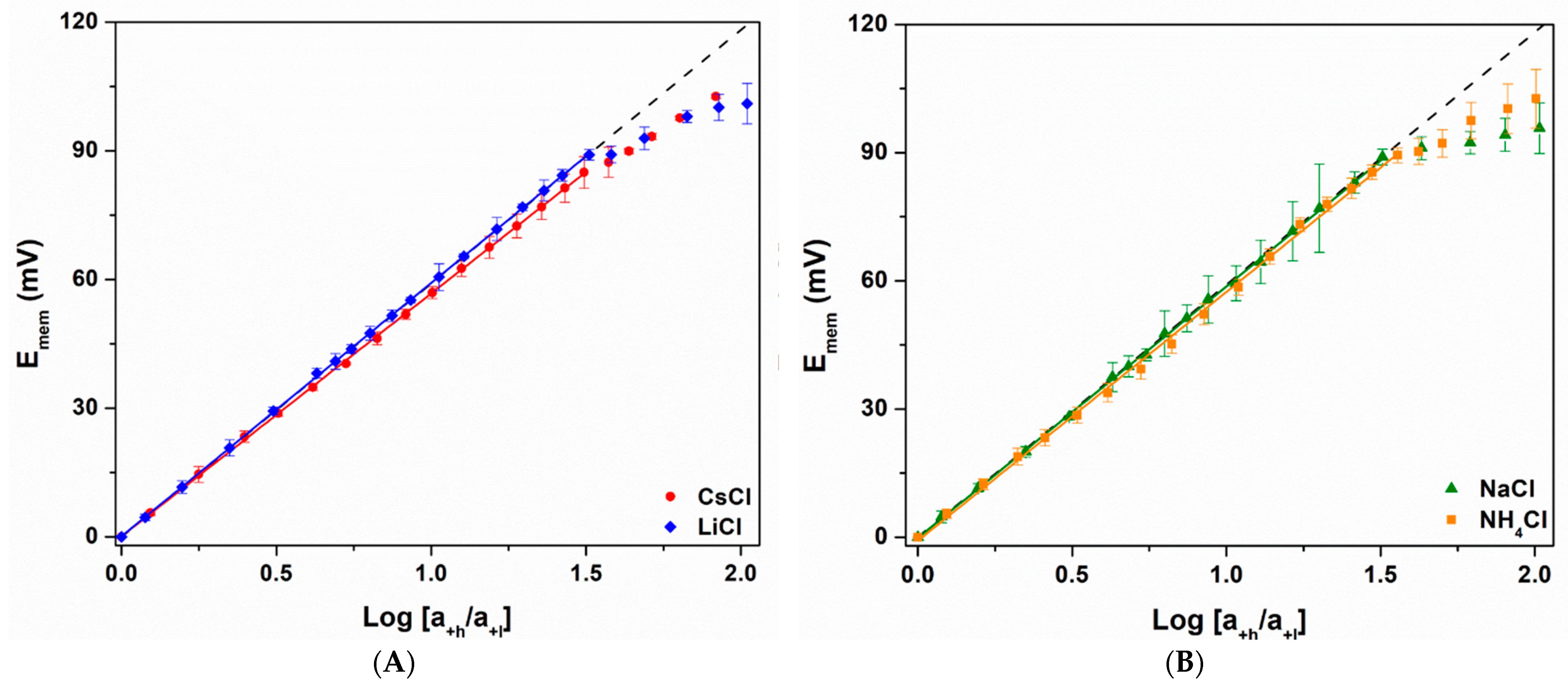
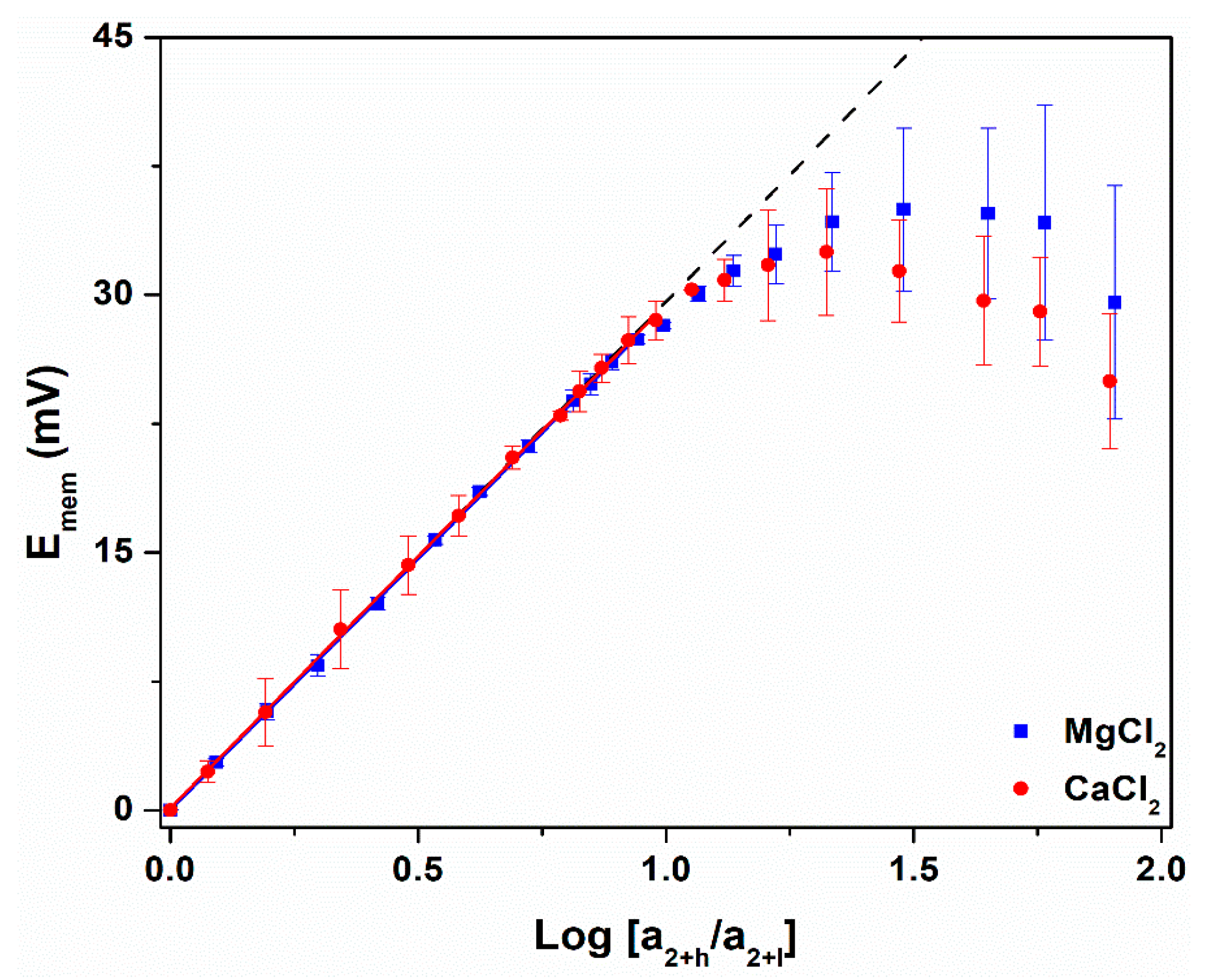
3.3. Nernst Plots and Ccrit
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Faucher, S.; Aluru, N.; Bazant, M.Z.; Blankschtein, D.; Brozena, A.H.; Cumings, J.; Pedro de Souza, J.; Elimelech, M.; Epsztein, R.; Fourkas, J.T.; et al. Critical Knowledge Gaps in Mass Transport through Single-Digit Nanopores: A Review and Perspective. J. Phys. Chem. C 2019, 123, 21309–21326. [Google Scholar] [CrossRef]
- Aluru, N.R.; Aydin, F.; Bazant, M.Z.; Blankschtein, D.; Brozena, A.H.; de Souza, J.P.; Elimelech, M.; Faucher, S.; Fourkas, J.T.; Koman, V.B.; et al. Fluids and Electrolytes under Confinement in Single-Digit Nanopores. Chem. Rev. 2023, 123, 2737–2831. [Google Scholar] [CrossRef] [PubMed]
- Siria, A.; Bocquet, M.-L.; Bocquet, L. New avenues for the large-scale harvesting of blue energy. Nat. Rev. Chem. 2017, 1, 0091. [Google Scholar] [CrossRef]
- Wang, L.; Wang, Z.; Patel, S.K.; Lin, S.; Elimelech, M. Nanopore-Based Power Generation from Salinity Gradient: Why It Is Not Viable. ACS Nano 2021, 15, 4093–4107. [Google Scholar] [CrossRef] [PubMed]
- Siria, A.; Poncharal, P.; Biance, A.-L.; Fulcrand, R.; Blase, X.; Purcell, S.T.; Bocquet, L. Giant osmotic energy conversion measured in a single transmembrane boron nitride nanotube. Nature 2013, 494, 455–458. [Google Scholar] [CrossRef] [PubMed]
- Hou, S.; Ji, W.; Chen, J.; Teng, Y.; Wen, L.; Jiang, L. Free-Standing Covalent Organic Framework Membrane for High-Efficiency Salinity Gradient Energy Conversion. Angew. Chem. Int. Ed. 2021, 60, 9925–9930. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Cao, T.; Dykstra, J.E.; Porada, S.; Biesheuvel, P.M.; Elimelech, M. Salt and Water Transport in Reverse Osmosis Membranes: Beyond the Solution-Diffusion Model. Environ. Sci. Technol. 2021, 55, 16665–16675. [Google Scholar] [CrossRef]
- Liu, T.; Lyv, J.; Xu, Y.; Zheng, C.; Liu, Y.; Fu, R.; Liang, L.; Wu, J.; Zhang, Z. Graphene-based woven filter membrane with excellent strength and efficiency for water desalination. Desalination 2022, 533, 115775. [Google Scholar] [CrossRef]
- Heiranian, M.; Farimani, A.B.; Aluru, N.R. Water desalination with a single-layer MoS2 nanopore. Nat. Commun. 2015, 6, 8616. [Google Scholar] [CrossRef]
- Rabinowitz, J.; Cohen, C.; Shepard, K.L. An Electrically Actuated, Carbon-Nanotube-Based Biomimetic Ion Pump. Nano Lett. 2020, 20, 1148–1153. [Google Scholar] [CrossRef]
- Cervera, J.; Ramirez, P.; Nasir, S.; Ali, M.; Ensinger, W.; Siwy, Z.S.; Mafe, S. Cation pumping against a concentration gradient in conical nanopores characterized by load capacitors. Bioelectrochemistry 2023, 152, 108445. [Google Scholar] [CrossRef]
- Noy, A.; Li, Z.; Darling, S.B. Fluid learning: Mimicking brain computing with neuromorphic nanofluidic devices. Nano Today 2023, 53, 102043. [Google Scholar] [CrossRef]
- Tunuguntla, R.H.; Henley, R.Y.; Yao, Y.-C.; Pham, T.A.; Wanunu, M.; Noy, A. Enhanced water permeability and tunable ion selectivity in subnanometer carbon nanotube porins. Science 2017, 357, 792–796. [Google Scholar] [CrossRef] [PubMed]
- Corry, B. Water and ion transport through functionalised carbon nanotubes: Implications for desalination technology. Energy Environ. Sci. 2011, 4, 751–759. [Google Scholar] [CrossRef]
- Ritt, C.L.; de Souza, J.P.; Barsukov, M.G.; Yosinski, S.; Bazant, M.Z.; Reed, M.A.; Elimelech, M. Thermodynamics of Charge Regulation during Ion Transport through Silica Nanochannels. ACS Nano 2022, 16, 15249–15260. [Google Scholar] [CrossRef] [PubMed]
- Xiong, M.; Song, K.; Leburton, J.-P. Ionic coulomb drag in nanofluidic semiconductor channels for energy harvest. Nano Energy 2023, 117, 108860. [Google Scholar] [CrossRef]
- Daiguji, H.; Yang, P.; Majumdar, A. Ion Transport in Nanofluidic Channels. Nano Lett. 2004, 4, 137–142. [Google Scholar] [CrossRef]
- Nishizawa, M.; Menon, V.P.; Martin, C.R. Metal Nanotubule Membranes with Electrochemically Switchable Ion-Transport Selectivity. Science 1995, 268, 700–702. [Google Scholar] [CrossRef] [PubMed]
- Makra, I.; Jágerszki, G.; Bitter, I.; Gyurcsányi, R.E. Nernst–Planck/Poisson model for the potential response of permselective gold nanopores. Electrochim. Acta 2012, 73, 70–77. [Google Scholar] [CrossRef]
- Bush, S.N.; Ken, J.S.; Martin, C.R. The Ionic Composition and Chemistry of Nanopore-Confined Solutions. ACS Nano 2022, 16, 8338–8346. [Google Scholar] [CrossRef]
- Martin, C.R.; Nishizawa, M.; Jirage, K.; Kang, M. Investigations of the Transport Properties of Gold Nanotubule Membranes. J. Phys. Chem. B 2001, 105, 1925–1934. [Google Scholar] [CrossRef]
- Huang, X.; Xie, L.; Lin, X.; Su, B. Permselective Ion Transport Across the Nanoscopic Liquid/Liquid Interface Array. Anal. Chem. 2016, 88, 6563–6569. [Google Scholar] [CrossRef] [PubMed]
- Amatore, C.; Oleinick, A.I.; Svir, I. Theory of Ion Transport in Electrochemically Switchable Nanoporous Metallized Membranes. ChemPhysChem 2009, 10, 211–221. [Google Scholar] [CrossRef] [PubMed]
- Baldessari, F. Electrokinetics in nanochannels: Part I. Electric double layer overlap and channel-to-well equilibrium. J. Colloid Interface Sci. 2008, 325, 526–538. [Google Scholar] [CrossRef] [PubMed]
- Bard, A.J.; Faulkner, L.R. Double Layer Structure and Adsorption. In Electrochemical Methods: Fundamentals and Applications, 2nd ed.; John Wiley & Sons: Nashville, TN, USA, 2001; pp. 534–579. [Google Scholar]
- Collins, K.D. Why continuum electrostatics theories cannot explain biological structure, polyelectrolytes or ionic strength effects in ion–protein interactions. Biophys. Chem. 2012, 167, 43–59. [Google Scholar] [CrossRef] [PubMed]
- Menon, V.P.; Martin, C.R. Fabrication and Evaluation of Nanoelectrode Ensembles. Anal. Chem. 1995, 67, 1920–1928. [Google Scholar] [CrossRef]
- Shi, Z.; Lipkowski, J. Chloride adsorption at the Au(111) electrode surface. J. Electroanal. Chem. 1996, 403, 225–239. [Google Scholar] [CrossRef]
- Kolics, A.; Thomas, A.E.; Wieckowski, A. 36Cl labelling and electrochemical study of chloride adsorption on a gold electrode from perchloric acid media. J. Chem. Soc. Faraday Trans. 1996, 92, 3727–3736. [Google Scholar] [CrossRef]
- Ávila, M.; Juárez, M.F.; Santos, E. Role of the Partial Charge Transfer on the Chloride Adlayers on Au(100). ChemElectroChem 2020, 7, 4269–4282. [Google Scholar] [CrossRef]
- Lipkowski, J.; Shi, Z.; Chen, A.; Pettinger, B.; Bilger, C. Ionic adsorption at the Au(111) electrode. Electrochim. Acta 1998, 43, 2875–2888. [Google Scholar] [CrossRef]
- Apel, P. Track etching technique in membrane technology. Radiat. Meas. 2001, 34, 559–566. [Google Scholar] [CrossRef]
- Harrell, C.C.; Lee, S.B.; Martin, C.R. Synthetic Single-Nanopore and Nanotube Membranes. Anal. Chem. 2003, 75, 6861–6867. [Google Scholar] [CrossRef] [PubMed]
- Liu, B.; Kelly, E.Y.; Liu, J. Cation-Size-Dependent DNA Adsorption Kinetics and Packing Density on Gold Nanoparticles: An Opposite Trend. Langmuir 2014, 30, 13228–13234. [Google Scholar] [CrossRef] [PubMed]
- Ramírez, P.; Mafé, S.; Alcaraz, A.; Cervera, J. Modeling of pH-Switchable Ion Transport and Selectivity in Nanopore Membranes with Fixed Charges. J. Phys. Chem. B 2003, 107, 13178–13187. [Google Scholar] [CrossRef]
- Bard, A.J.; Faulkner, L.R. Potentials and Thermodynamics of Cells. In Electrochemical Methods: Fundamentals and Applications, 2nd ed.; John Wiley & Sons: Nashville, TN, USA, 2001; pp. 44–86. [Google Scholar]
- Smith, L.A.; Glasscott, M.W.; Vannoy, K.J.; Dick, J.E. Enzyme Kinetics via Open Circuit Potentiometry. Anal. Chem. 2020, 92, 2266–2273. [Google Scholar] [CrossRef] [PubMed]
- Shahi, V.K.; Makawana, B.S.; Thampy, S.K.; Rangarajan, R. Electrochemical characterization of cation exchange membrane with immobilized anionic and cationic surfactants. Indian J. Chem. 1999, 38, 124–129. [Google Scholar]
- Lakshminarayanaiah, N. Theories of Membrane Electrode Potentials. In Membrane Electrodes; Lakshminarayanaiah, N., Ed.; Academic Press: New York, NY, USA, 1976; pp. 50–94. [Google Scholar]
- Jacquemin, M.; Genet, M.J.; Gaigneaux, E.M.; Debecker, D.P. Calibration of the X-ray Photoelectron Spectroscopy Binding Energy Scale for the Characterization of Heterogeneous Catalysts: Is Everything Really under Control? ChemPhysChem 2013, 14, 3618–3626. [Google Scholar] [CrossRef]
- Bush, S.N.; Experton, J.; de La Serve, A.T.; Johnson, E.P.; Martin, C.R. Imaging Cycle-Induced Damage of MnO2 Microparticles. J. Electrochem. Soc. 2020, 167, 132501. [Google Scholar] [CrossRef]
- Jirage, K.B.; Hulteen, J.C.; Martin, C.R. Nanotubule-Based Molecular-Filtration Membranes. Science 1997, 278, 655–658. [Google Scholar] [CrossRef]
- De Leo, M.; Pereira, F.C.; Moretto, L.M.; Scopece, P.; Polizzi, S.; Ugo, P. Towards a Better Understanding of Gold Electroless Deposition in Track-Etched Templates. Chem. Mater. 2007, 19, 5955–5964. [Google Scholar] [CrossRef]
- Erb, R.A. Wettability of gold. J. Phys. Chem. 1968, 72, 2412–2417. [Google Scholar] [CrossRef]
- Smith, T. The hydrophilic nature of a clean gold surface. J. Colloid Interface Sci. 1980, 75, 51–55. [Google Scholar] [CrossRef]
- Li, C.; Zhang, J.; Han, J.; Yao, B. A numerical solution to the effects of surface roughness on water–coal contact angle. Sci. Rep. 2021, 11, 459. [Google Scholar] [CrossRef] [PubMed]
- Brown, P.S.; Bhushan, B. Mechanically durable liquid-impregnated honeycomb surfaces. Sci. Rep. 2017, 7, 6083. [Google Scholar] [CrossRef] [PubMed]
- Haag, A.-L.; Nagai, Y.; Lennox, R.B.; Grütter, P. Characterization of a gold coated cantilever surface for biosensing applications. EPJ Tech. Instrum. 2015, 2, 1. [Google Scholar] [CrossRef]
- Mateos, H.; Picca, R.A.; Mallardi, A.; Dell’Aglio, M.; De Giacomo, A.; Cioffi, N.; Palazzo, G. Effect of the Surface Chemical Composition and of Added Metal Cation Concentration on the Stability of Metal Nanoparticles Synthesized by Pulsed Laser Ablation in Water. Appl. Sci. 2020, 10, 4169. [Google Scholar] [CrossRef]
- Steinle, E.D.; Mitchell, D.T.; Wirtz, M.; Lee, S.B.; Young, V.Y.; Martin, C.R. Ion Channel Mimetic Micropore and Nanotube Membrane Sensors. Anal. Chem. 2002, 74, 2416–2422. [Google Scholar] [CrossRef]
- Jellander, R.; Marčelja, S.; Quirk, J.P. Attractive double-layer interactions between calcium clay particles. J. Colloid Interface Sci. 1988, 126, 194–211. [Google Scholar] [CrossRef]
- Amadu, M.; Miadonye, A. Applicability of the linearized Poisson–Boltzmann theory to contact angle problems and application to the carbon dioxide–brine–solid systems. Sci. Rep. 2022, 12, 5710. [Google Scholar] [CrossRef]
- Deserno, M.; Holm, C. Cell Model and Poisson-Boltzmann Theory: A Brief Introduction. In Proceedings of the Electrostatic Effects in Soft Matter and Biophysics, Les Houches, France, 1–13 October 2000; Springer: Dordrecht, The Netherlands, 2001; pp. 27–52. [Google Scholar]
- Volta, T.T.; Walters, S.N.; Martin, C.R. Effect of Organic Cation Adsorption on Ion-Transport Selectivity in a Cation-Permselective Nanopore Membrane. Langmuir 2024, 40, 10825–10833. [Google Scholar] [CrossRef]
- Salis, A.; Ninham, B.W. Models and mechanisms of Hofmeister effects in electrolyte solutions, and colloid and protein systems revisited. Chem. Soc. Rev. 2014, 43, 7358–7377. [Google Scholar] [CrossRef] [PubMed]
- Haynes, W.M. (Ed.) CRC Handbook of Chemistry and Physics, 94th ed.; CRC Press: Boca Raton, FL, USA, 2013; p. 2668. [Google Scholar]
- Geada, I.L.; Ramezani-Dakhel, H.; Jamil, T.; Sulpizi, M.; Heinz, H. Insight into induced charges at metal surfaces and biointerfaces using a polarizable Lennard–Jones potential. Nat. Commun. 2018, 9, 716. [Google Scholar] [CrossRef] [PubMed]
- Zhou, J.; Hao, J.; Wu, R.; Su, L.; Wang, J.; Qiu, M.; Bao, B.; Ning, C.; Teng, C.; Zhou, Y.; et al. Maximizing Ion Permselectivity in MXene/MOF Nanofluidic Membranes for High-Efficient Blue Energy Generation. Adv. Funct. Mater. 2022, 32, 2209767. [Google Scholar] [CrossRef]
- Hong, J.G.; Zhang, B.; Glabman, S.; Uzal, N.; Dou, X.; Zhang, H.; Wei, X.; Chen, Y. Potential ion exchange membranes and system performance in reverse electrodialysis for power generation: A review. J. Membr. Sci. 2015, 486, 71–88. [Google Scholar] [CrossRef]
- Jönsson, A.-S.; Jönsson, B. The influence of nonionic and ionic surfactants on hydrophobic and hydrophilic ultrafiltration membranes. J. Membr. Sci. 1991, 56, 49–76. [Google Scholar] [CrossRef]
- Gaarenstroom, S.W.; Winograd, N. Initial and final state effects in the ESCA spectra of cadmium and silver oxides. J. Chem. Phys. 1977, 67, 3500–3506. [Google Scholar] [CrossRef]
- Moulder, J.F.; Stickle, W.F.; Sobol, P.E.; Bomben, K.D. Handbook of X-ray Photoelectron Spectroscopy; Perkin-Elmer Corporation, Physical Electronics Division: Eden Prairie, MN, USA, 1992. [Google Scholar]
- Liu, C.; Félix, R.; Forberich, K.; Du, X.; Heumüller, T.; Matt, G.J.; Gu, E.; Wortmann, J.; Zhao, Y.; Cao, Y.; et al. Utilizing the unique charge extraction properties of antimony tin oxide nanoparticles for efficient and stable organic photovoltaics. Nano Energy 2021, 89, 106373. [Google Scholar] [CrossRef]


| Element Peak | PC30,10, Control (%) | PC30,10, NaCl 1 (%) |
|---|---|---|
| C1s | 32.4 | 48 ± 3 |
| Au4f | 46.9 | 25 ± 3 |
| O1s | 16.0 | 12 ± 3 |
| Ag3d | 4.0 | 3.1 ± 0.5 |
| Sn3d | 0.8 | 0.9 ± 0.2 |
| Cl2p | - | 5 ± 2 |
| Na1s | - | 5 ± 2 |
| Cation | Slope of Linear Response Region (mV) | t+ of Linear Response Region | Ccrit (mM) |
|---|---|---|---|
| Ideal | 59.2 | 1.000 a | 3.7 b |
| Li+ | 59.1 ± 0.1 | 0.999 ± 0.001 | 3.5 ± 0.1 |
| Na+ | 58.8 ± 0.3 | 0.997 ± 0.003 | 3.7 ± 0.1 |
| K+ | 58.5 ± 0.2 | 0.994 ± 0.002 | 3.5 ± 0.2 |
| NH4+ | 58.2 ± 0.6 | 0.992 ± 0.005 | 3.8 ± 0.1 |
| Cs+ | 56.6 ± 0.2 | 0.978 ± 0.002 | 3.4 ± 0.3 |
| Cation | Slope of Linear Response Region (mV) | t+ of Linear Response Region | Ccrit (mM) |
|---|---|---|---|
| Ideal | 29.6 | 1.000 a | 1.2 b |
| Ca2+ | 29.4 ± 0.2 | 0.997 ± 0.003 | 0.9 ± 0.2 |
| Mg2+ | 29.3 ± 0.1 | 0.995 ± 0.002 | 0.9 ± 0.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Volta, T.T.; Walters, S.N.; Martin, C.R. Potentiometric Studies on Ion-Transport Selectivity in Charged Gold Nanotubes. Nanomaterials 2024, 14, 1209. https://doi.org/10.3390/nano14141209
Volta TT, Walters SN, Martin CR. Potentiometric Studies on Ion-Transport Selectivity in Charged Gold Nanotubes. Nanomaterials. 2024; 14(14):1209. https://doi.org/10.3390/nano14141209
Chicago/Turabian StyleVolta, Thomas T., Stevie N. Walters, and Charles R. Martin. 2024. "Potentiometric Studies on Ion-Transport Selectivity in Charged Gold Nanotubes" Nanomaterials 14, no. 14: 1209. https://doi.org/10.3390/nano14141209
APA StyleVolta, T. T., Walters, S. N., & Martin, C. R. (2024). Potentiometric Studies on Ion-Transport Selectivity in Charged Gold Nanotubes. Nanomaterials, 14(14), 1209. https://doi.org/10.3390/nano14141209







