Reactions of Hydrogen-Passivated Silicon Vacancies in α-Quartz with Electron Holes and Hydrogen
Abstract
1. Introduction
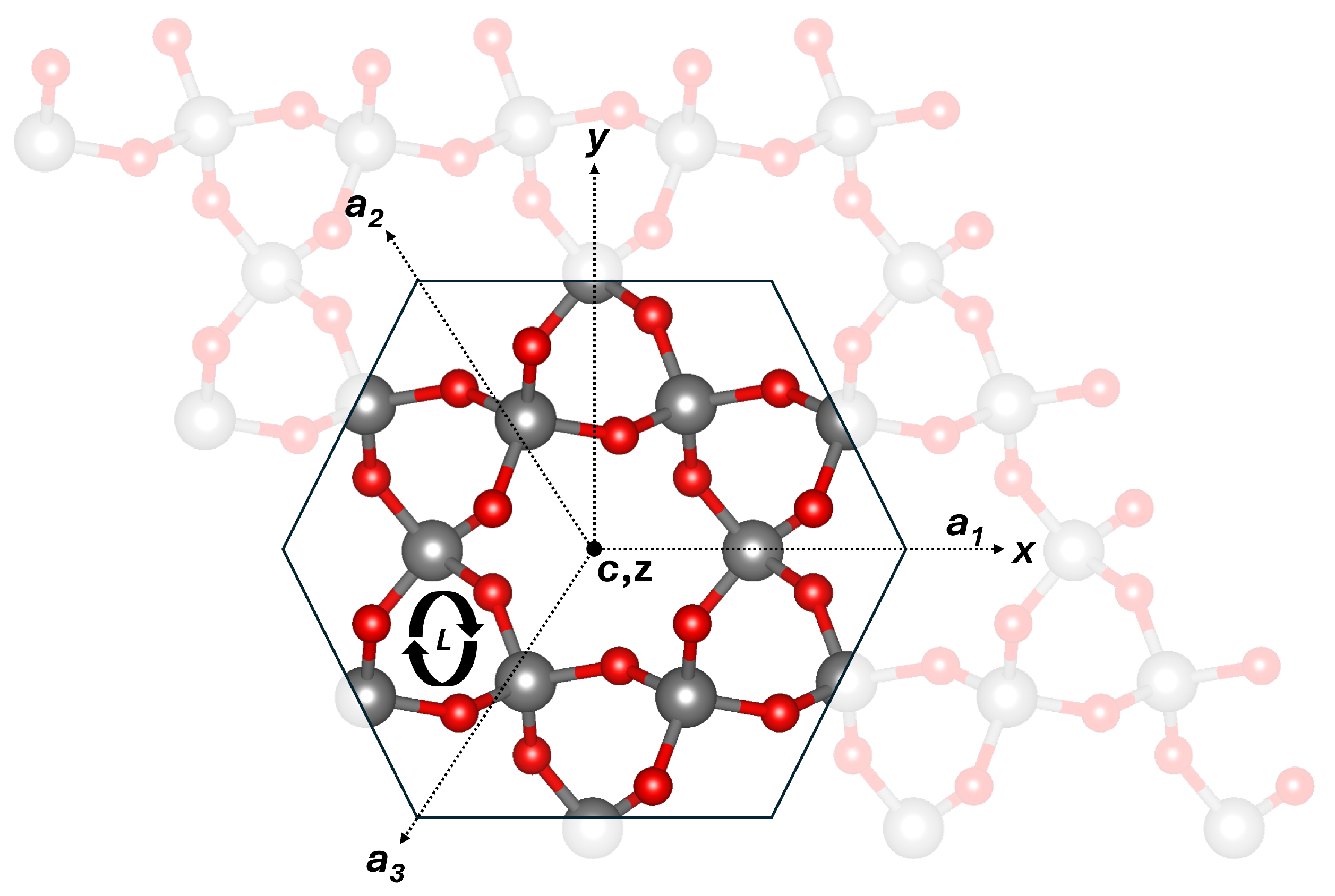
2. Methods of Calculations
3. Results and Discussion
3.1. Barriers to Hydrogen Diffusion in Different Charge States
3.2. Hole Trapping at Si Vacancy Centres
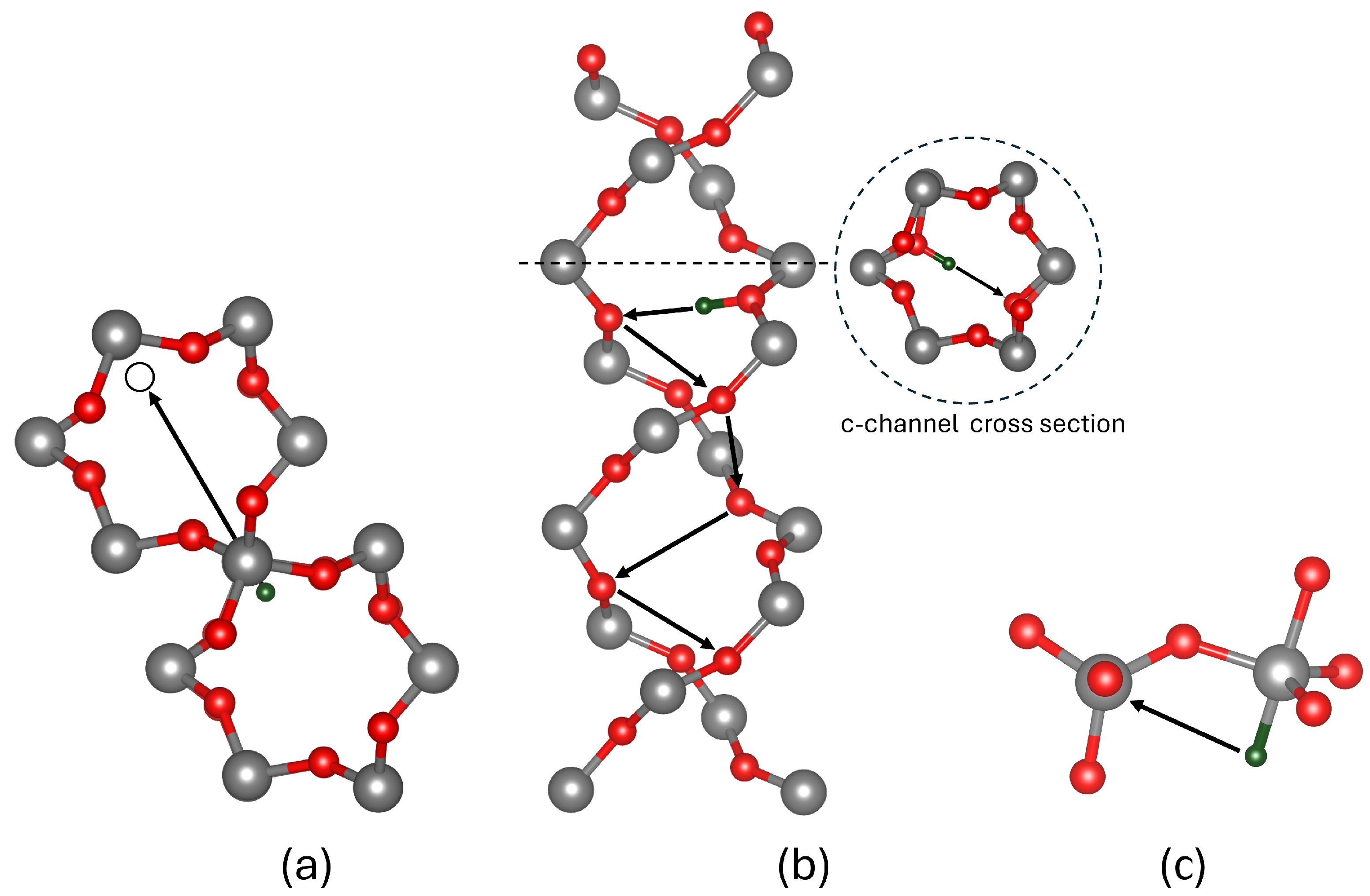
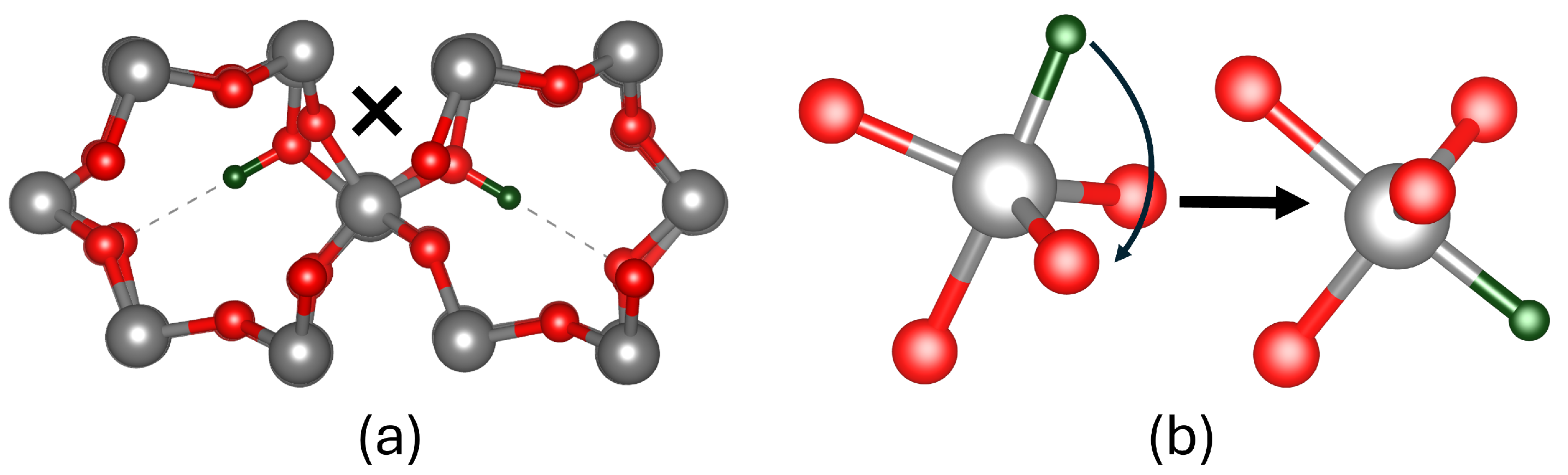
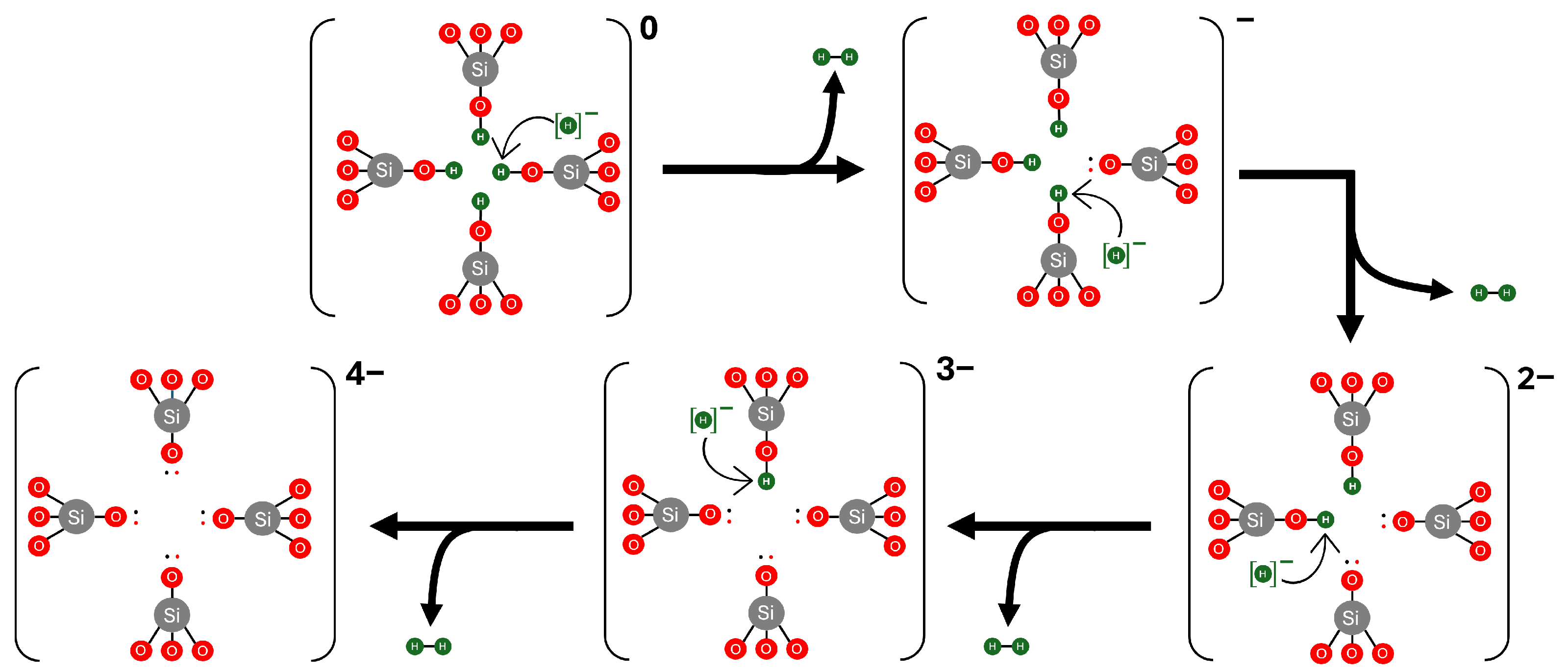
3.3. Hydrogen Reactions with Si Vacancy Centres
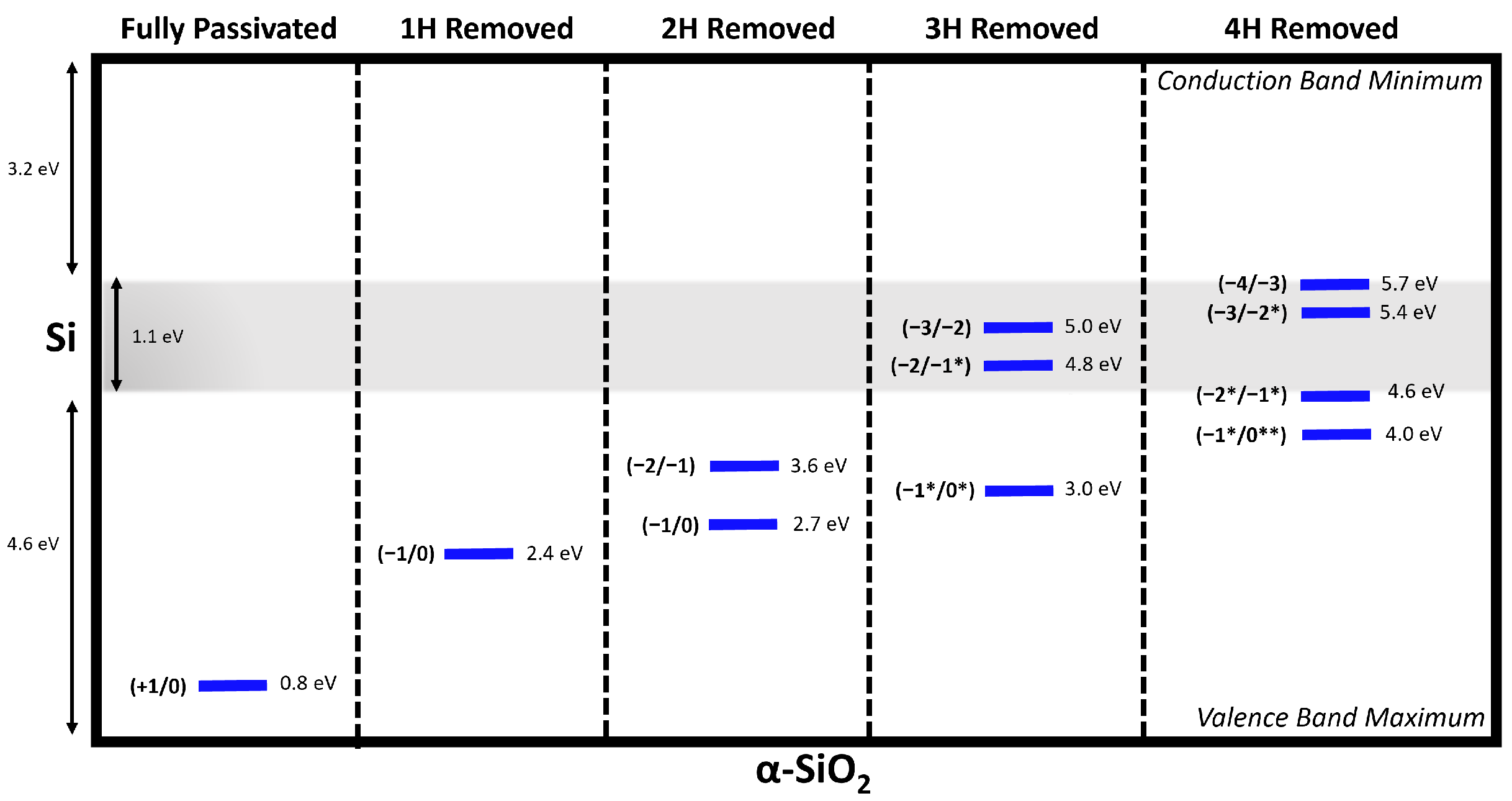
3.4. Charge Transition Levels of [4H]Si Defects
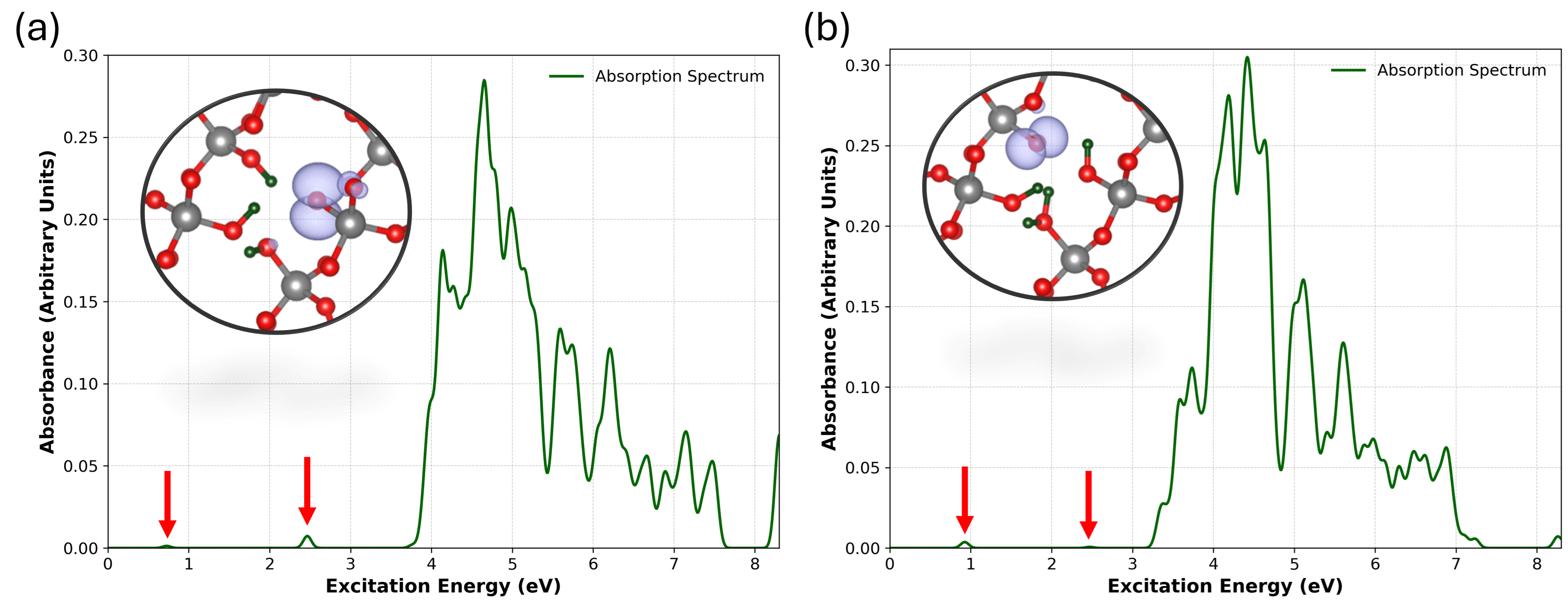
3.5. Optical Absorption of NBOHC in [4H]Si
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| NBOHC | Non-bridging oxygen hole center |
| DFT | Density functional theory |
| EPR | Electron paramagnetic resonance |
| NBTI | Negative bias temperature instability |
| GPW | Gaussian plane wave |
| GTH | Goedecker–Teter–Hutter |
| ADMM | Auxiliary density matrix method |
| OT | Orbital transformation |
| BFGS | Broyden–Fletcher–Goldfarb–Shanno |
| KS | Kohn–Sham |
| CI-NEB | Climbing image-nudged elastic band |
| LR-TDDFT | Linear-response time-dependent density functional theory |
| TDA | Tamm–Dancoff approximation |
| ZPE | Zero-point energy |
| CBM | Conduction band minimum |
| MOSFET | Metal–oxide semiconductor field-effect transistor |
| CTL | Charge transition level |
| VBM | Valence band maximum |
References
- Götze, J.; Pan, Y.; Müller, A. Mineralogy and mineral chemistry of quartz: A review. Mineral. Mag. 2021, 85, 639–664. [Google Scholar] [CrossRef]
- Pan, X.; Li, S.; Li, Y.; Guo, P.; Zhao, X.; Cai, Y. Resource, characteristic, purification and application of quartz: A review. Miner. Eng. 2022, 183, 107600. [Google Scholar] [CrossRef]
- Moon, G.; Lee, N.; Kang, S.; Park, J.; Kim, Y.E.; Lee, S.A.; Chitumalla, R.K.; Jang, J.; Choe, Y.; Oh, Y.K.; et al. Hydrothermal synthesis of novel two-dimensional α-quartz nanoplates and their applications in energy-saving, high-efficiency, microalgal biorefineries. Chem. Eng. J. 2021, 413, 127467. [Google Scholar] [CrossRef]
- Moon, G.; Jang, E.H.; Kim, S.; Choe, Y.; Chung, S. Hydrothermal synthesis and characterization of quartz nanocrystals—Implications from a simple kinetic growth model. Korean J. Chem. Eng. 2022, 39, 440–450. [Google Scholar] [CrossRef]
- Stalder, R. OH point defects in quartz—A review. Eur. J. Mineral. 2021, 33, 145–163. [Google Scholar] [CrossRef]
- Godbeer, W.C.; Wilkins, R.W.T. The water content of a synthetic quartz. Am. Mineral. 1977, 62, 831–832. [Google Scholar]
- McLaren, A.C.; Cook, R.F.; Hyde, S.T.; Tobin, R.C. The mechanisms of the formation and growth of water bubbles and associated dislocation loops in synthetic quartz. Phys. Chem. Miner. 1983, 9, 79–94. [Google Scholar] [CrossRef]
- Dilnesa, B.Z.; Lothenbach, B.; Renaudin, G.; Wichser, A.; Kulik, D. Synthesis and characterization of hydrogarnet Ca3(AlxFe1−x)2(SiO4)y(OH)4(3−y). Cem. Concr. Res. 2014, 59, 96–111. [Google Scholar] [CrossRef]
- Gaweł, B.A.; Ulvensøen, A.; Łukaszuk, K.; Arstad, B.; Muggerud, A.M.F.; Erbe, A. Structural evolution of water and hydroxyl groups during thermal, mechanical and chemical treatment of high purity natural quartz. RSC Adv. 2020, 10, 29018–29030. [Google Scholar] [CrossRef]
- Jollands, M.C.; Blanchard, M.; Balan, E. Structure and theoretical infrared spectra of OH defects in quartz. Eur. J. Mineral. 2020, 32, 311–323. [Google Scholar] [CrossRef]
- Purton, J.; Jones, R.; Heggie, M.; Öberg, S.; Catlow, C.R.A. LDF pseudopotential calculations of the α-quartz structure and hydrogarnet defect. Phys. Chem. Miner. 1992, 18, 389–392. [Google Scholar] [CrossRef]
- Lin, J.S.; Payne, M.C.; Heine, V.; McConnell, J.D.C. Ab initio calculations on (OH)4 defects in α-quartz. Phys. Chem. Miner. 1994, 21, 9150–9155. [Google Scholar] [CrossRef]
- Rosa, A.L.; El-Barbary, A.A.; Heggie, M.; Briddon, P.R. Structural and thermodynamic properties of water related defects in α-quartz. Phys. Chem. Miner. 2005, 32, 323–331. [Google Scholar] [CrossRef]
- Richard, N.; Martin-Samos, L.; Roma, G.; Limoge, Y.; Crocombette, J.P. First principle study of neutral and charged self-defects in amorphous SiO2. J. Non-Cryst. Solids 2005, 351, 1825–1829. [Google Scholar] [CrossRef]
- Sokol, A.A.; Catlow, C.R.A.; Garcés, J.M.; Kuperman, A. Transformation of hydroxyl nests in microporous aluminosilicates upon annealing. J. Phys. Condens. Matter 2004, 16, S2781. [Google Scholar] [CrossRef]
- Pascale, F.; Ugliengo, P.; Civalleri, B.; Orlando, R.; D’Arco, P.; Dovesi, R. Hydrogarnet defect in chabazite and sodalite zeolites: A periodic Hartree–Fock and B3-LYP study. J. Chem. Phys. 2002, 117, 5337–5346. [Google Scholar] [CrossRef]
- Nuttall, R.; Weil, J. Two hydrogenic trapped-hole species in α-quartz. Solid State Commun. 1980, 33, 99–102. [Google Scholar] [CrossRef]
- Bahadur, H. Radiation-induced modifications of point defects in quartz crystals and their application in radiation dosimetry. Radiat. Meas. 2003, 36, 493–497. [Google Scholar] [CrossRef]
- Bahadur, H. Point defects in quartz crystals and their radiation response—A review [quartz resonator applications]. In Proceedings of the 2004 IEEE International Frequency Control Symposium and Exposition 2004, Montreal, QC, Canada, 23–27 August 2004; pp. 651–659. [Google Scholar] [CrossRef]
- Silva, C.M.; Rosseel, T.M.; Kirkegaard, M.C. Radiation-Induced Changes in Quartz, A Mineral Analog of Nuclear Power Plant Concrete Aggregates. Inorg. Chem. 2018, 57, 3329–3338. [Google Scholar] [CrossRef] [PubMed]
- Bunson, P.; Di Ventra, M.; Pantelides, S.; Fleetwood, D.; Schrimpf, R. Hydrogen-related defects in irradiated SiO2. IEEE Trans. Nucl. Sci. 2000, 47, 2289–2296. [Google Scholar] [CrossRef]
- Wimmer, Y.; El-Sayed, A.M.; Gös, W.; Grasser, T.; Shluger, A.L. Role of hydrogen in volatile behaviour of defects in SiO2-based electronic devices. Proc. R. Soc. A Math. Phys. Eng. Sci. 2016, 472, 20160009. [Google Scholar] [CrossRef]
- Stathis, J.; Zafar, S. The negative bias temperature instability in MOS devices: A review. Microelectron. Reliab. 2006, 46, 270–286. [Google Scholar] [CrossRef]
- Mahapatra, S.; Parihar, N. A review of NBTI mechanisms and models. Microelectron. Reliab. 2018, 81, 127–135. [Google Scholar] [CrossRef]
- Skuja, L. The origin of the intrinsic 1.9 eV luminescence band in glassy SiO2. J. Non-Cryst. Solids 1994, 179, 51–69. [Google Scholar] [CrossRef]
- Skuja, L.; Ollier, N.; Kajihara, K.; Smits, K. Creation of glass-characteristic point defects in crystalline SiO2 by 2.5 MeV electrons and by fast neutrons. J. Non-Cryst. Solids 2019, 505, 252–259. [Google Scholar] [CrossRef]
- Crespillo, M.; Graham, J.; Weber, W.; Agulló-López, F. Defect generation mechanisms in silica under intense electronic excitation by ion beams below 100 K: Interplay between radiative emissions. Acta. Mater. 2023, 255, 119097. [Google Scholar] [CrossRef]
- Kühne, T.D.; Iannuzzi, M.; Del Ben, M.; Rybkin, V.V.; Seewald, P.; Stein, F.; Laino, T.; Khaliullin, R.Z.; Schütt, O.; Schiffmann, F.; et al. CP2K: An electronic structure and molecular dynamics software package–Quickstep: Efficient and accurate electronic structure calculations. J. Chem. Phys. 2020, 152, 194103. [Google Scholar] [CrossRef]
- Hartwigsen, C.; Goedecker, S.; Hutter, J. Relativistic separable dual-space Gaussian pseudopotentials from H to Rn. Phys. Rev. B 1998, 58, 3641–3662. [Google Scholar] [CrossRef]
- Guidon, M.; Hutter, J.; VandeVondele, J. Robust Periodic Hartree-Fock Exchange for Large-Scale Simulations Using Gaussian Basis Sets. J. Chem. Theory Comput. 2009, 5, 3010–3021. [Google Scholar] [CrossRef] [PubMed]
- Guidon, M.; Hutter, J.; VandeVondele, J. Auxiliary Density Matrix Methods for Hartree-Fock Exchange Calculations. J. Chem. Theory Comput. 2010, 6, 2348–2364. [Google Scholar] [CrossRef] [PubMed]
- Bart, F.; Gautier, M.; Duraud, J.; Henriot, M. (010) α-quartz surface: A LEED, XANES and ELS study. Surf. Sci. 1992, 274, 317–328. [Google Scholar] [CrossRef]
- Bart, F.; Gautier, M.; Jollet, F.; Duraud, J. Electronic structure of the (0001) and (1010) quartz surfaces and of their defects as observed by reflection electron energy loss spectroscopy (REELS). Surf. Sci. 1994, 306, 342–358. [Google Scholar] [CrossRef]
- Garvie, L.; Rez, P.; Alvarez, J.; Buseck, P. Interband transitions of crystalline and amorphous SiO2: An electron energy-loss spectroscopy (EELS) study of the low-loss region. Solid State Commun. 1998, 106, 303–307. [Google Scholar] [CrossRef]
- Garvie, L.A.; Rez, P.; Alvarez, J.R.; Buseck, P.R.; Craven, A.J.; Brydson, R. Bonding in alpha-quartz (SiO2): A view of the unoccupied states. Am. Mineral. 2000, 85, 732–738. [Google Scholar] [CrossRef]
- Henkelman, G.; Uberuaga, B.P.; Jónsson, H. A climbing image nudged elastic band method for finding saddle points and minimum energy paths. J. Chem. Phys. 2000, 113, 9901–9904. [Google Scholar] [CrossRef]
- Lany, S.; Zunger, A. Assessment of correction methods for the band-gap problem and for finite-size effects in supercell defect calculations: Case studies for ZnO and GaAs. Phys. Rev. B 2008, 78, 235104. [Google Scholar] [CrossRef]
- Li, H.; Robertson, J. Behaviour of hydrogen in wide band gap oxides. J. Appl. Phys. 2014, 115, 203708. [Google Scholar] [CrossRef]
- Shkrob, I.A.; Trifunac, A.D. Time-resolved EPR of spin-polarized mobile H atoms in amorphous silica: The involvement of small polarons. Phys. Rev. B 1996, 54, 15073–15078. [Google Scholar] [CrossRef]
- Griscom, D.L. Diffusion of radiolytic molecular hydrogen as a mechanism for the post-irradiation buildup of interface states in SiO2-on-Si structures. J. Appl. Phys. 1985, 58, 2524–2533. [Google Scholar] [CrossRef]
- Kajihara, K.; Skuja, L.; Hirano, M.; Hosono, H. Diffusion and Reactions of Hydrogen in F2-Laser-Irradiated SiO2 Glass. Phys. Rev. Lett. 2002, 89, 135507. [Google Scholar] [CrossRef] [PubMed]
- Kats, A.; Haven, Y.; Stevels, J.M. Hydroxyl groups in α-quartz. Phys. Chem. Glas. 1962, 3, 69–76. [Google Scholar]
- Bolse, W.; Gustafsson, M.; Harbsmeier, F.; Roccaforte, F. Diffusion of hydrogen implanted in α-quartz during air annealing. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms 2000, 161, 641–645. [Google Scholar] [CrossRef]
- Jollands, M.C.; Ellis, B.; Tollan, P.M.; Müntener, O. An eruption chronometer based on experimentally determined H-Li and H-Na diffusion in quartz applied to the Bishop Tuff. Earth Planet. Sci. Lett. 2020, 551, 116560. [Google Scholar] [CrossRef]
- Bongiorno, A.; Colombo, L.; Trioni, M. Migration of atomic hydrogen in crystalline and amorphous SiO2: A molecular dynamics study. J. Non-Cryst. Solids 1997, 216, 30–35. [Google Scholar] [CrossRef]
- Kronenberg, A.K.; Kirby, S.H.; Aines, R.D.; Rossman, G.R. Solubility and diffusional uptake of hydrogen in quartz at high water pressures: Implications for hydrolytic weakening. J. Geophys. Res. Solid Earth 1986, 91, 12723–12741. [Google Scholar] [CrossRef]
- Bongiorno, A.; Colombo, L.; Cargnoni, F. Hydrogen diffusion in crystalline SiO2. Chem. Phys. Lett. 1997, 264, 435–440. [Google Scholar] [CrossRef]
- Tuttle, B. Energetics and diffusion of hydrogen in SiO2. Phys. Rev. B 2000, 61, 4417–4420. [Google Scholar] [CrossRef]
- Devine, R.A.B.; Herrera, G.V. Electric-field-induced transport of protons in amorphous SiO2. Phys. Rev. B 2001, 63, 233406. [Google Scholar] [CrossRef]
- Vanheusden, K.; Warren, W.; Devine, R. Non-volatile memory device based on mobile protons in SiO2 thin films. Nature 1997, 386, 587–589. [Google Scholar] [CrossRef]
- Godet, J.; Pasquarello, A. Proton Diffusion Mechanism in Amorphous SiO2. Phys. Rev. Lett. 2006, 97, 155901. [Google Scholar] [CrossRef]
- Edwards, A.H.; Shedd, W.; Pugh, R. Theory of H− in SiO2. J. Non-Cryst. Solids 2001, 289, 42–52. [Google Scholar] [CrossRef]
- Vaccaro, L.; Cannas, M.; Boscaino, R. Phonon coupling of non-bridging oxygen hole center with the silica environment: Temperature dependence of the 1.9 eV emission spectra. J. Lumin. 2008, 128, 1132–1136. [Google Scholar] [CrossRef]
- Yokozawa, A.; Miyamoto, Y. First-principles calculations for charged states of hydrogen atoms in SiO2. Phys. Rev. B 1997, 55, 13783–13788. [Google Scholar] [CrossRef]
- Bersch, E.; Di, M.; Consiglio, S.; Clark, R.D.; Leusink, G.J.; Diebold, A.C. Complete band offset characterization of the HfO2/SiO2/Si stack using charge corrected X-ray photoelectron spectroscopy. J. Appl. Phys. 2010, 107, 043702. [Google Scholar] [CrossRef]
- Cook, T.E., J.; Fulton, C.C.; Mecouch, W.J.; Tracy, K.M.; Davis, R.F.; Hurt, E.H.; Lucovsky, G.; Nemanich, R.J. Measurement of the band offsets of SiO2 on clean n- and p-type GaN(0001). J. Appl. Phys. 2003, 93, 3995–4004. [Google Scholar] [CrossRef]
- Bersch, E.; Rangan, S.; Bartynski, R.A.; Garfunkel, E.; Vescovo, E. Band offsets of ultrathin high-κ oxide films with Si. Phys. Rev. B 2008, 78, 085114. [Google Scholar] [CrossRef]
- Suzuki, T.; Skuja, L.; Kajihara, K.; Hirano, M.; Kamiya, T.; Hosono, H. Electronic Structure of Oxygen Dangling Bond in Glassy SiO2: The Role of Hyperconjugation. Phys. Rev. Lett. 2003, 90, 186404. [Google Scholar] [CrossRef] [PubMed]
- Giordano, L.; Sushko, P.V.; Pacchioni, G.; Shluger, A.L. Optical and EPR properties of point defects at a crystalline silica surface: Ab initio embedded-cluster calculations. Phys. Rev. B 2007, 75, 024109. [Google Scholar] [CrossRef]
- Sousa, C.; de Graaf, C.; Pacchioni, G. Optical properties of peroxy radicals in silica: Multiconfigurational perturbation theory calculations. J. Chem. Phys. 2001, 114, 6259–6264. [Google Scholar] [CrossRef][Green Version]



| id | Reaction Mechanism | (eV) |
|---|---|---|
| i | Si(OH)3-OH → Si(OH)3-O· + H0 | +6.0 |
| ii | Si(OH)3-OH + H0 → Si(OH)3-O· + H2 | +1.04 |
| iii | Si(OH)3-OH + H+ → Si(OH)3- | −0.61 |
| iv | Si(OH)3-OH + H− → Si(OH)3-O− + H2 | −0.98 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cobos Freire, T.; Strand, J.; Shluger, A.L. Reactions of Hydrogen-Passivated Silicon Vacancies in α-Quartz with Electron Holes and Hydrogen. Nanomaterials 2025, 15, 142. https://doi.org/10.3390/nano15020142
Cobos Freire T, Strand J, Shluger AL. Reactions of Hydrogen-Passivated Silicon Vacancies in α-Quartz with Electron Holes and Hydrogen. Nanomaterials. 2025; 15(2):142. https://doi.org/10.3390/nano15020142
Chicago/Turabian StyleCobos Freire, Teofilo, Jack Strand, and Alexander L. Shluger. 2025. "Reactions of Hydrogen-Passivated Silicon Vacancies in α-Quartz with Electron Holes and Hydrogen" Nanomaterials 15, no. 2: 142. https://doi.org/10.3390/nano15020142
APA StyleCobos Freire, T., Strand, J., & Shluger, A. L. (2025). Reactions of Hydrogen-Passivated Silicon Vacancies in α-Quartz with Electron Holes and Hydrogen. Nanomaterials, 15(2), 142. https://doi.org/10.3390/nano15020142







