Rebuilding the Strain Hardening at a Large Strain in Twinned Au Nanowires
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Mechanical Behavior of the NT Au Nanowires at Small Strain
3.2. Mechanical Behavior of the NT Au Nanowires with θ = 54.74°
3.3. Yielding Mechanism
3.4. Strain Hardening Mechanism of the NT Nanowires with θ = 90°
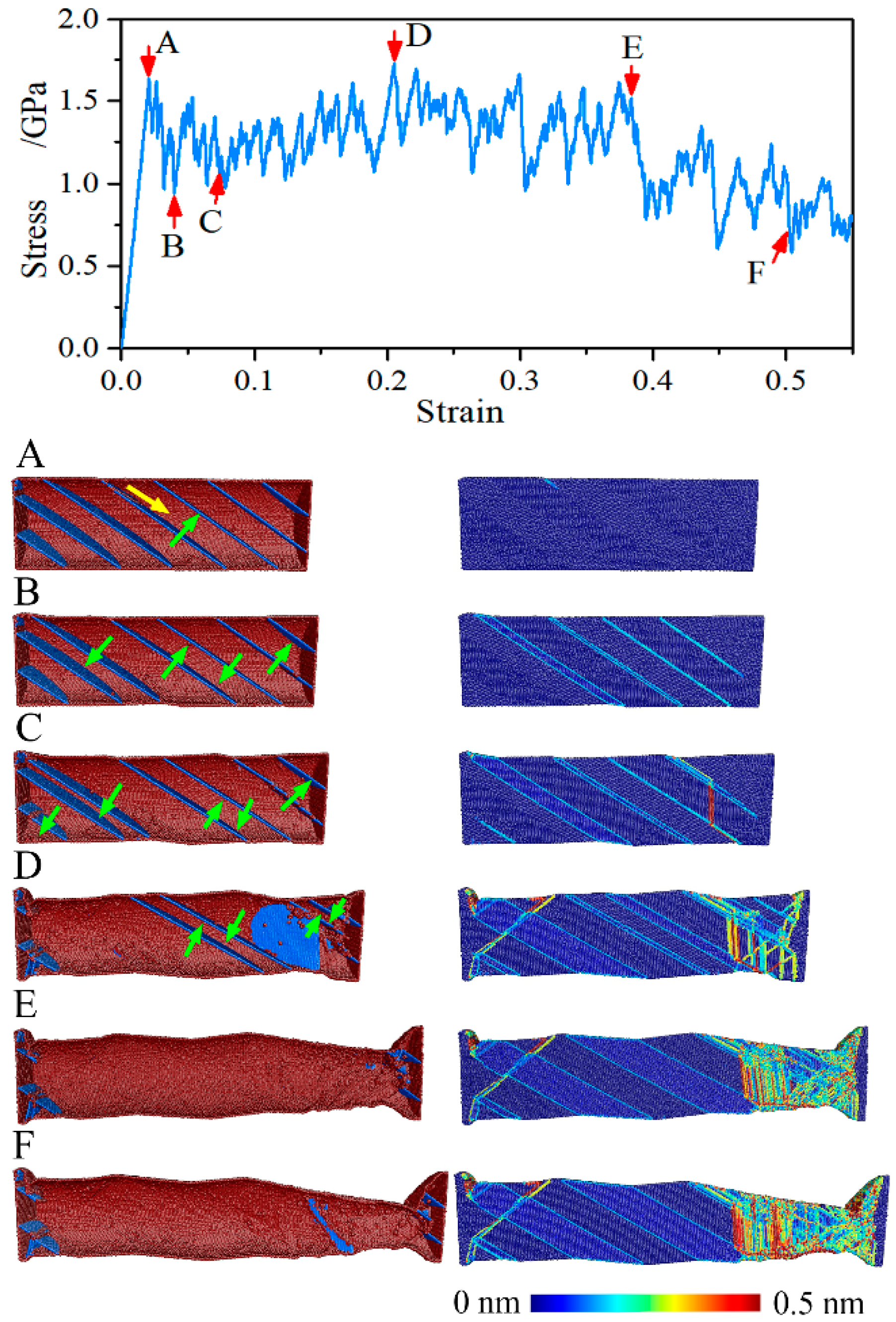
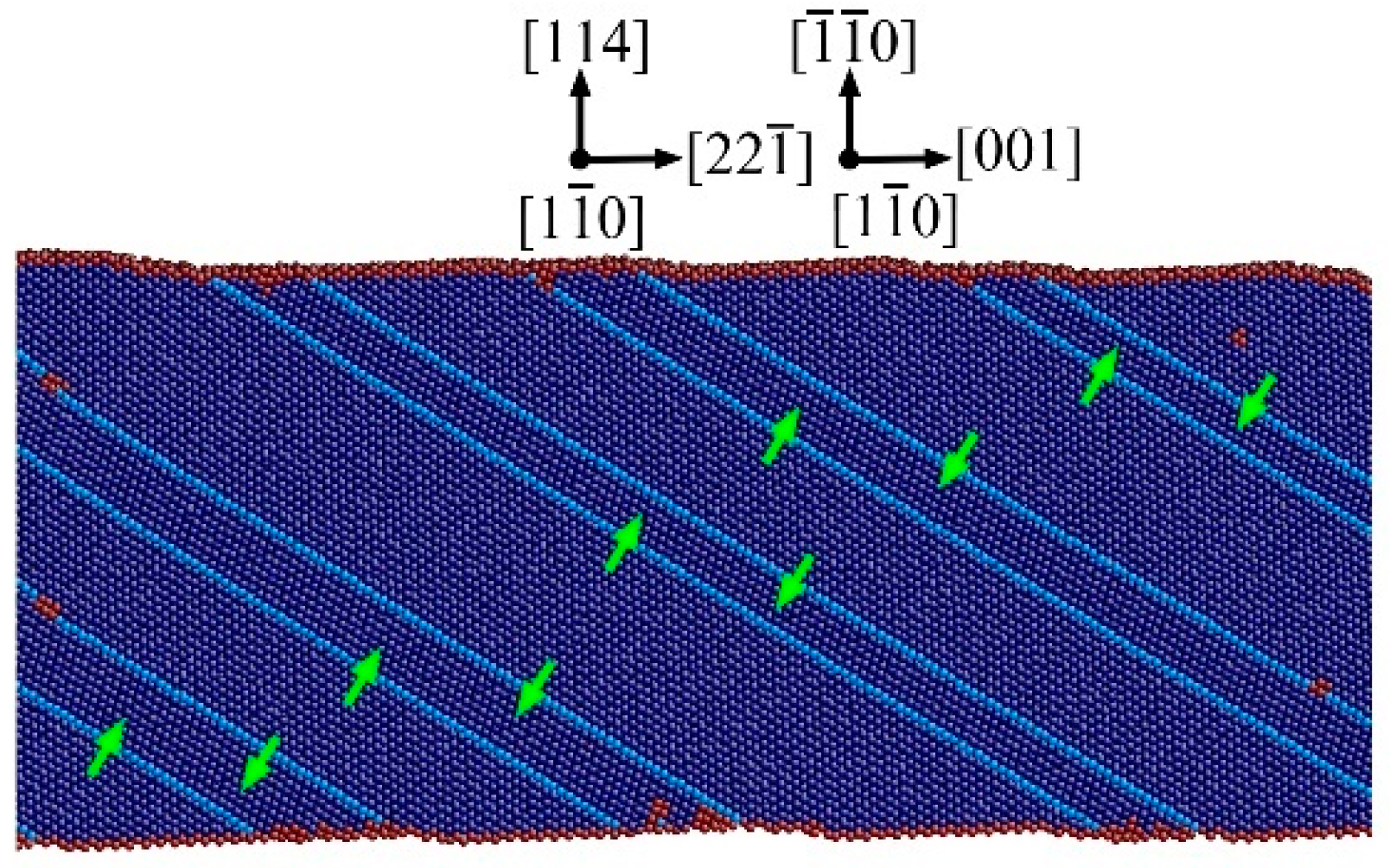
3.5. Unique Strain Hardening Mechanism of the NT Nanowires with θ = 54.74°
3.6. Deformation Mechanism of the NT Au Nanowires with θ = 19.47°
3.7. Twin Orientation, Slip System and Dislocation Process
4. Conclusions
- (1)
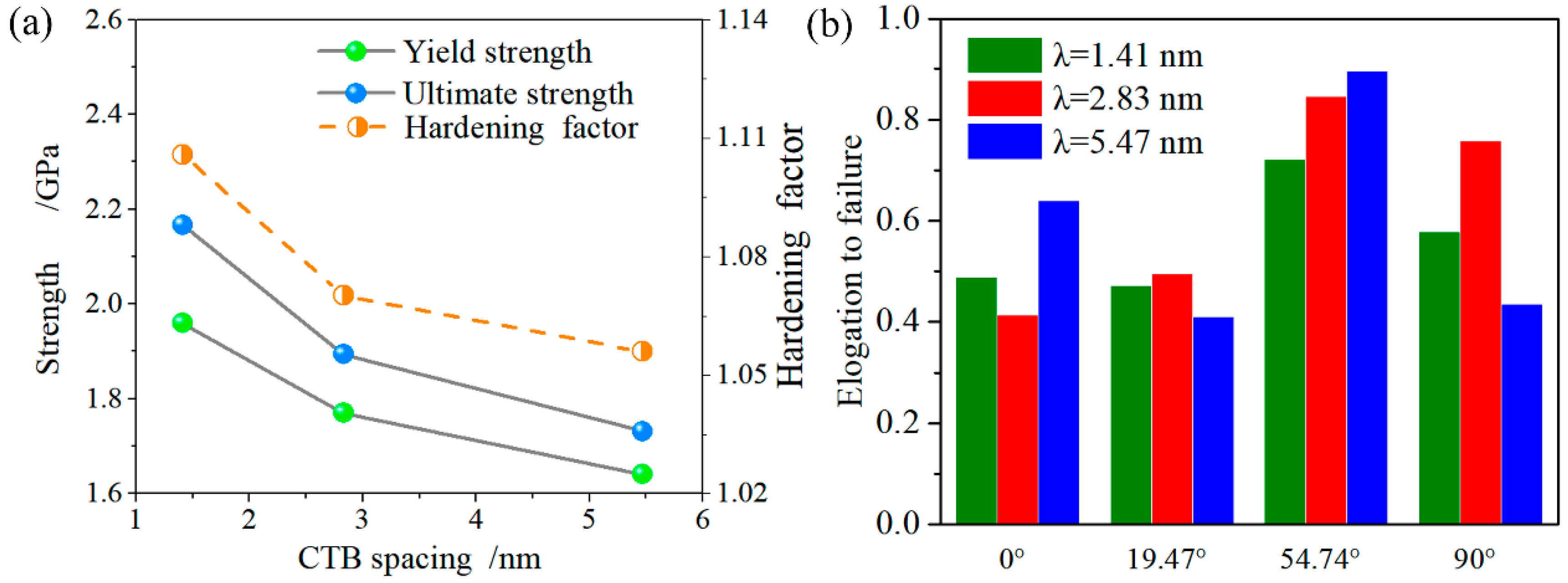
- With the twin orientation varying from 0° to 90°, two types of strain hardening behaviors and a strain softening behavior are found. A general strain hardening behavior in the initial stage of plastic deformation is observed with twin orientation of 0° and 90°, which resulted by the blockage effect of twin boundary on the initial dislocation sliding. A unique strain hardening behavior at a larger strain (0.21 to 0.31) is rebuilt when twin orientation is changed to 54.74°, which is related to twin migration. Because of this unique strain hardening, an improved ductility is achieved.
- (2)
- The twin orientation plays a main role in the mechanical behavior and deformation mechanism of the cylindrical NT Au nanowires. The orthogonally oriented CTBs have the optimal strengthening capability in the NT Au nanowires, followed by the parallel CTBs and the slant CTBs.
- (3)
- As twin orientation increases from 0° to 90°, the dominating deformation mechanism shifts from slip-CTB interaction to dislocation slip, Twin migration, and slip transmission in sequence.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lao, J.J.; Tam, M.N.; Pinisetty, D.; Gupta, N. Molecular dynamics simulation of FCC metallic nanowires: A review. JOM 2013, 65, 175–184. [Google Scholar] [CrossRef]
- Park, H.S.; Cai, W.; Espinosa, H.D.; Huang, H. Mechanics of crystalline nanowires. Mrs. Bull. 2009, 34, 178–183. [Google Scholar] [CrossRef]
- Li, L.L.; Zhang, Z.J.; Zhang, P.; Tan, J.; Yang, J.B.; Zhang, Z.F. Deformation behaviors of Cu bicrystals with an inclined twin boundary at multiple scales. J. Mater. Sci. Technol. 2017, 33, 698–702. [Google Scholar] [CrossRef]
- Zhang, Y.; Yu, D.J.; Wang, K.M. Atomistic Simulation of the Orientation-dependent Plastic Deformation Mechanisms of Iron Nanopillars. J. Mater. Sci. Technol. 2012, 28, 164–168. [Google Scholar] [CrossRef]
- Mukhopadhyay, T.; Mahata, A.; Dey, S.; Adhikari, S. Probabilistic Analysis and Design of HCP Nanowires: An Efficient Surrogate Based Molecular Dynamics Simulation Approach. J. Mater. Sci. Technol. 2016, 32, 1345–1351. [Google Scholar] [CrossRef]
- Wu, Z.X.; Zhang, Y.W.; Jhon, M.H.; Gao, H.J.; Srolovitz, D.J. Nanowire failure: Long = brittle and short = ductile. Nano. Lett. 2012, 12, 910–914. [Google Scholar] [CrossRef] [PubMed]
- Sainath, G.; Rohith, P.; Choudhary, B.K. Size dependent deformation behaviour and dislocation mechanisms in <100> Cu nanowires. Philos. Mag. 2017, 97, 2632–2657. [Google Scholar] [CrossRef]
- Diao, J.K.; Gall, K.; Dunn, M.L.; Zimmerman, J.A. Atomistic simulations of the yielding of gold nanowires. Acta Mater. 2006, 54, 643–653. [Google Scholar] [CrossRef]
- Wang, J.; Sansoz, F.; Huang, J.; Sun, S.; Zhang, Z.; Mao, S.X. Near-ideal theoretical strength in gold nanowires containing angstrom scale twins. Nat. Commun. 2013, 4, 1742. [Google Scholar] [CrossRef] [Green Version]
- Monk, J.; Farkas, D. Tension-compression asymmetry and size effects in nanocrystalline Ni nanowires. Philos. Mag. 2007, 87, 2233–2244. [Google Scholar] [CrossRef]
- Zhang, F.; Zhou, J. Tension-compression asymmetry and twin boundaries spacings effects in polycrystalline Ni nanowires. J. Appl. Phys. 2016, 120, 427. [Google Scholar] [CrossRef]
- Yu, H.H.; Xin, Y.C.; Wang, M.Y.; Liu, Q. Hall-Petch relationship in Mg alloys: A. review. J. Mater. Sci. Technol. 2018, 34, 248–256. [Google Scholar] [CrossRef]
- Jang, D.C.; Li, X.Y.; Gao, H.J.; Greer, J.R. Deformation mechanisms in nanotwinned metal nanopillars. Nat. Nanotechnol. 2012, 7, 594–601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sun, J.; Fang, L.; Ma, A.; Jiang, J.; Han, Y.; Chen, H.; Han, J. The fracture behavior of twinned Cu nanowires: A molecular dynamics simulation. Mat. Sci. Eng. A Struct. 2015, 634, 86–90. [Google Scholar] [CrossRef]
- Sun, J.; Li, C.; Han, J.; Shao, X.; Yang, X.; Liu, H.; Song, D.; Ma, A. Size effect and deformation mechanism in twinned copper nanowires. Metals 2017, 7, 438. [Google Scholar] [CrossRef]
- Wang, L.; Lu, Y.; Kong, D.; Xiao, L.; Sha, X.; Sun, J.; Zhang, Z.; Han, X. Dynamic and atomic-scale understanding of the twin thickness effect on dislocation nucleation and propagation activities by in situ bending of Ni nanowires. Acta Mater. 2015, 90, 194–203. [Google Scholar] [CrossRef]
- Zhu, W.; Wang, H.; Yang, W. Orientation-and microstructure-dependent deformation in metal nanowires under bending. Acta Mater. 2012, 60, 7112–7122. [Google Scholar] [CrossRef]
- Deng, C.; Sansoz, F. Near-Ideal strength in gold nanowires achieved through microstructural design. ACS Nano. 2009, 3, 3001–3008. [Google Scholar] [CrossRef] [PubMed]
- Han, J.; Fang, L.; Sun, J.P.; Han, Y.; Sun, K. Length-dependent mechanical properties of gold nanowires. J. Appl. Phys. 2012, 112, 114314. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cheng, G.; Yin, S.; Chang, T.; Richter, G.; Gao, H.; Zhu, Y. Anomalous tensile detwinning in twinned nanowires. Phys. Rev. Lett. 2017, 119, 256101. [Google Scholar] [CrossRef] [PubMed]
- Deng, C.; Sansoz, F. Fundamental differences in the plasticity of periodically twinned nanowires in Au, Ag, Al, Cu, Pb and Ni. Acta Mater. 2009, 57, 6090–6101. [Google Scholar] [CrossRef]
- Narayanan, S.; Cheng, G.; Zeng, Z.; Zhu, Y.; Zhu, T. Strain hardening and size effect in five-fold twinned ag nanowires. Nano. Lett. 2015, 15, 4037–4044. [Google Scholar] [CrossRef] [PubMed]
- Deng, C.; Sansoz, F. Size-dependent yield stress in twinned gold nanowires mediated by site-specific surface dislocation emission. Appl. Phys. Lett. 2009, 95, 091914. [Google Scholar] [CrossRef]
- Guo, X.; Xia, Y.Z. Repulsive force vs. Source number: Competing mechanisms in the yield of twinned gold nanowires of finite length. Acta Mater. 2011, 59, 2350–2357. [Google Scholar] [CrossRef]
- Deng, C.; Sansoz, F. Repulsive force of twin boundary on curved dislocations and its role on the yielding of twinned nanowires. Scripta. Mater. 2010, 63, 50–53. [Google Scholar] [CrossRef]
- Sun, J.; Fang, L.; Sun, K.; Han, J. Direct observation of dislocations originating from perfect twin boundaries. Scripta. Mater. 2011, 65, 501–504. [Google Scholar] [CrossRef]
- Zhu, Y.T.; Wu, X.L.; Liao, X.Z.; Narayan, J.; Kecskés, L.J.; Mathaudhu, S.N. Dislocation-twin interactions in nanocrystalline fcc metals. Acta Mater. 2011, 59, 812–821. [Google Scholar] [CrossRef]
- Wei, Y. Anisotropic size effect in strength in coherent nanowires with tilted twins. Phys. Rev. B 2011, 84, 014107. [Google Scholar] [CrossRef]
- Yang, Z.; Zheng, L.; Yue, Y.; Lu, Z. Effects of twin orientation and spacing on the mechanical properties of Cu nanowires. Sci. Rep. 2017, 7, 10056. [Google Scholar] [CrossRef] [PubMed]
- Lu, Q.; You, Z.; Huang, X.; Hansen, N.; Lu, L. Dependence of dislocation structure on orientation and slip systems in highly oriented nanotwinned Cu. Acta Mater. 2017, 127, 85–97. [Google Scholar] [CrossRef]
- Zhao, X.; Lu, C.; Tieu, A.K.; Zhan, L.; Huang, M.; Su, L.; Pei, L.; Zhang, L. Deformation twinning and dislocation processes in nanotwinned copper by molecular dynamics simulations. Comp. Mater. Sci. 2018, 142, 59–71. [Google Scholar] [CrossRef]
- Zhao, X.; Lu, C.; Tieu, A.K.; Pei, L.; Zhang, L.; Su, L.; Zhan, L. Deformation mechanisms in nanotwinned copper by molecular dynamics simulation. Mat. Sci. Eng. A Struct. 2017, 687, 343–351. [Google Scholar] [CrossRef]
- Grochola, G.; Russo, S.P.; Snook, I.K. On fitting a gold embedded atom method potential using the force matching method. J. Chem. Phys. 2005, 123, 204719. [Google Scholar] [CrossRef] [PubMed]
- Tsuzuki, H.; Branicio, P.S.; Rino, J.P. Structural characterization of deformed crystals by analysis of common atomic neighborhood. Comput. Phys. Commun. 2007, 177, 518–523. [Google Scholar] [CrossRef]
- Zimmerman, J.A.; Kelchner, C.L.; Klein, P.A.; Hamilton, J.C.; Foiles, S.M. Surface step effects on nanoindentation. Phys. Rev. Lett. 2001, 87, 165507. [Google Scholar] [CrossRef] [PubMed]
- Plimpton, S. Fast parallel algorithms for short-range molecular-dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Zhu, T.; Gao, H. Plastic deformation mechanism in nano-twinned metals: Insight from molecular dynamics and mechanistic modeling. Scripta. Mater. 2012, 66, 843–848. [Google Scholar] [CrossRef]
- Deng, C.; Sansoz, F. Enabling ultrahigh plastic flow and work hardening in twinned gold nanowires. Nano. Lett. 2009, 9, 1517–1522. [Google Scholar] [CrossRef] [PubMed]
- Zhou, H.; Li, X.; Qu, S.; Yang, W.; Gao, H. A jogged dislocation governed strengthening mechanism in nanotwinned metals. Nano. Lett. 2014, 14, 5075–5080. [Google Scholar] [CrossRef] [PubMed]
- Yue, Y.; Zhang, Q.; Zhang, X.; Yang, Z.; Yin, P.; Guo, L. In situ observation of twin boundary sliding in single crystalline cu nanowires. Small 2017, 13, 1604296. [Google Scholar] [CrossRef] [PubMed]
- Park, H.S.; Gall, K.; Zimmerman, J.A. Shape memory and pseudoelasticity in metal nanowires. Phys. Rev. Lett. 2005, 95, 255504. [Google Scholar] [CrossRef] [PubMed]
- Liang, W.W.; Zhou, M. Atomistic simulations reveal shape memory of fcc metal nanowires. Phys. Rev. B 2006, 73, 115409. [Google Scholar] [CrossRef]
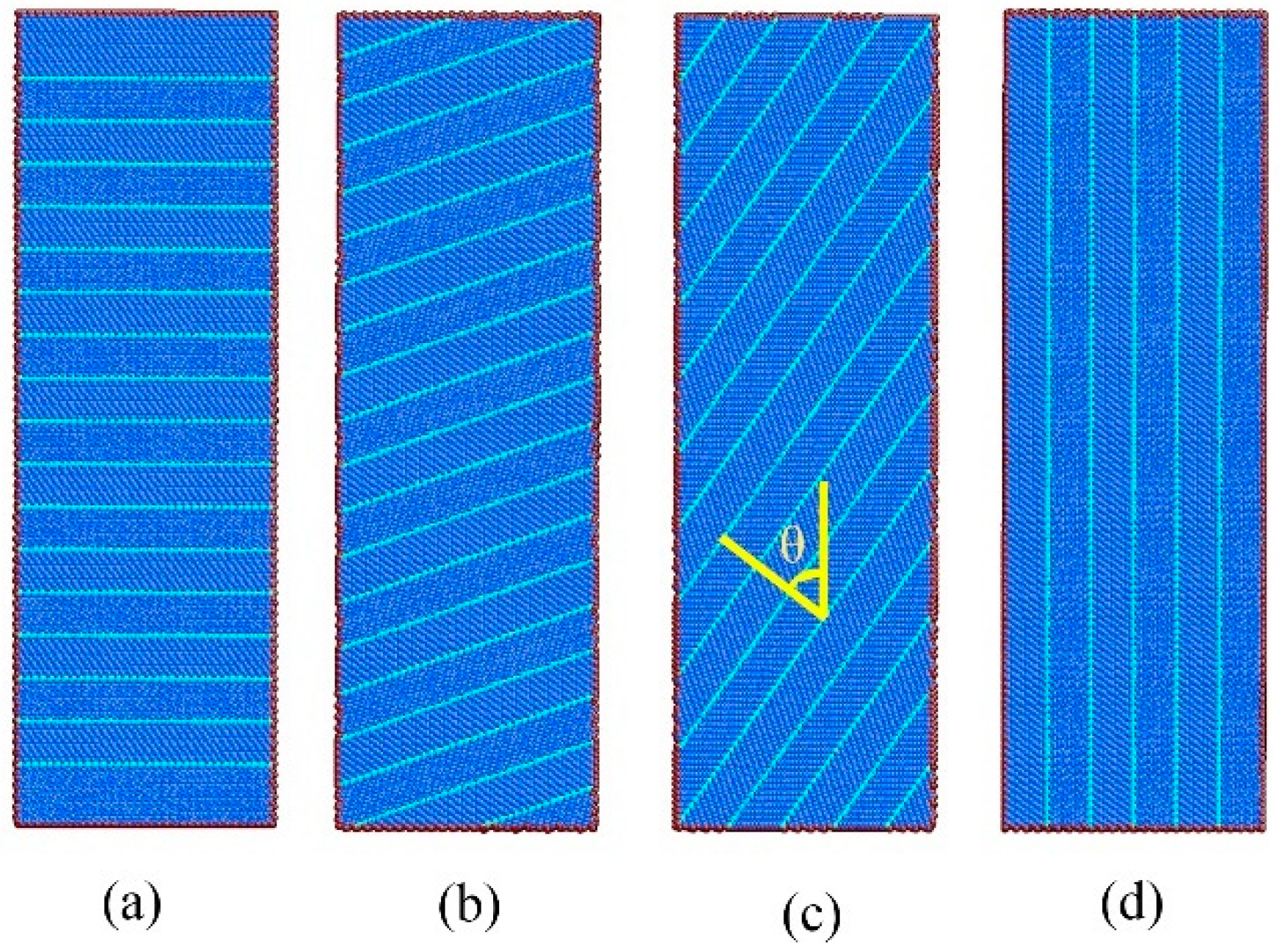







| 0° | 19.47° | 54.74° | 90° | |
|---|---|---|---|---|
| Matrix | [111] | [112] | [001] | [11] |
| Twin | [111] | [552] | [22] | [2] |
| Mechanical Behavior | First Active Dislocation | Nucleation Site of the First Dislocation | Dominated Deformation Mechanism | |
|---|---|---|---|---|
| 0° | General hardening | Partial | CTB-surface intersection site | Slip-twin interaction |
| 19.47° | Softening | Twinning partial | CTB-surface intersection site | Slip |
| 54.74° | Unique hardening | Twinning partial | CTB-surface intersection site | Twin migration |
| 90° | General hardening | Partial | Surface between adjacent CTBs | Slip transmission (zigzag extended dislocation) |
| 0° | 19.47° | 54.74° | 90° | |||||
|---|---|---|---|---|---|---|---|---|
| TP | P | TP | P | TP | P | TP | P | |
| Matrix | 0 | 0.31 | 0.31 | 0.39 | 0.47 | 0.47 | 0 | 0.39 |
| Twin | 0 | 0.31 | 0.31 | 0.49 | 0.47 | 0.26 | 0 | 0.39 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, J.; Han, J.; Yang, Z.; Liu, H.; Song, D.; Ma, A.; Fang, L. Rebuilding the Strain Hardening at a Large Strain in Twinned Au Nanowires. Nanomaterials 2018, 8, 848. https://doi.org/10.3390/nano8100848
Sun J, Han J, Yang Z, Liu H, Song D, Ma A, Fang L. Rebuilding the Strain Hardening at a Large Strain in Twinned Au Nanowires. Nanomaterials. 2018; 8(10):848. https://doi.org/10.3390/nano8100848
Chicago/Turabian StyleSun, Jiapeng, Jing Han, Zhenquan Yang, Huan Liu, Dan Song, Aibin Ma, and Liang Fang. 2018. "Rebuilding the Strain Hardening at a Large Strain in Twinned Au Nanowires" Nanomaterials 8, no. 10: 848. https://doi.org/10.3390/nano8100848
APA StyleSun, J., Han, J., Yang, Z., Liu, H., Song, D., Ma, A., & Fang, L. (2018). Rebuilding the Strain Hardening at a Large Strain in Twinned Au Nanowires. Nanomaterials, 8(10), 848. https://doi.org/10.3390/nano8100848





