The Role of Determinism in the Prediction of Corrosion Damage
Abstract
:1. Introduction
2. Philosophical Basis of Determinism vs. Empiricism
- The definition of determinism versus empiricism;
- Why determinism is so important;
- The structures of deterministic vs. empirical models;
- The concept of the corrosion evolutionary path (CEP).
3. The Structure of a Deterministic Model
- A theory must be based upon experimental observation [23].
- The model based on that theory contains N “constitutive” equations that describe the relationships between various components and M “constraints”, which are statements of the natural laws (typically the conservation conditions) and which constrain the output to that which is “physically real”.
- M + N must be at least equal to the number of unknowns in the model. If it is not, the system is said to be mathematically underdetermined and deterministic prediction is not possible. If N + M is greater than the number of unknowns, the model is said to be mathematically “overdetermined” and deterministic prediction is unimpeded.
- All equations must be mathematically independent.
- Ad hoc relations cannot be added simply to “make the model work” (Einstein’s famous admonishment to the scientific community!) [23].
4. Model Building
- Collate property data—a valid “global” theory must account for all the known properties of the system;
- Formulate hypotheses, postulates, and assumptions. These must agree with our empirical knowledge or theoretical expectation of the system;
- Specify the “mechanism”, and hence the “constitutive equations”;
- Specify the “constraints” (e.g., conservation equations, Faraday’s law of mass-charge equivalency);
- Solve the equations and predict the output;
- Compare the output with the experimental data and adjust the model to make new predictions that are in better agreement with the experiment;
- The last step is repeated until no amount of valid adjustment can make the model “work” by accounting for new observations within experimental uncertainty. The theory/model is then rejected, a new theory/model is developed, and the process starts over again;
- Finally, it is important to recognize that modeling is always a compromise between complexity and mathematical tractability. After a certain threshold, the modeler must make a compromise by either simplifying the model (e.g., reducing the number of species considered, and hence the number of independent variables) or by invoking assumptions to simplify the mathematics, or both. This is particularly important in the development of analytical models that may require numerical solutions of coupled high order differential equations for which analytical solutions do not exist.
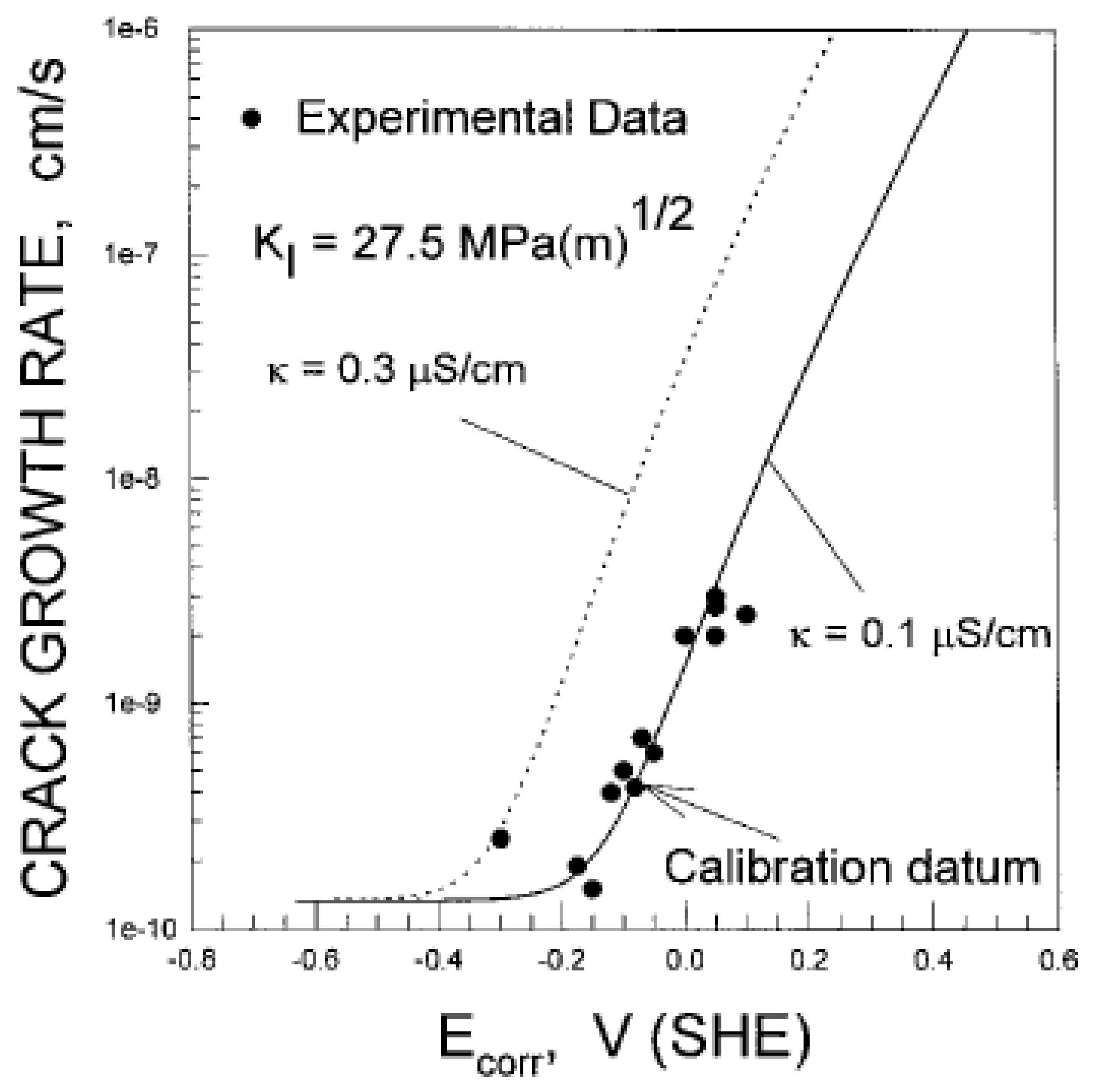
- The crack growth rate (CGR) increases roughly exponentially with the potential of the metal at sufficiently high potentials. At lower potentials, the CGR is potential-independent, corresponding to mechanical creep fracture (Figure 4);
- In the case of IGSCC, the crack propagates intergranularly, giving rise to the intergranular crack pathway (Figure 3). In other cases, the crack propagates across the grain in a process termed transgranular stress corrosion cracking (TGSCC), and in still other cases mixed mode (IDSCC/TGSCC) may be observed [55];
- The CGR increases with the DOS, the yield strength, the hardness, and the extent of cold work [37];
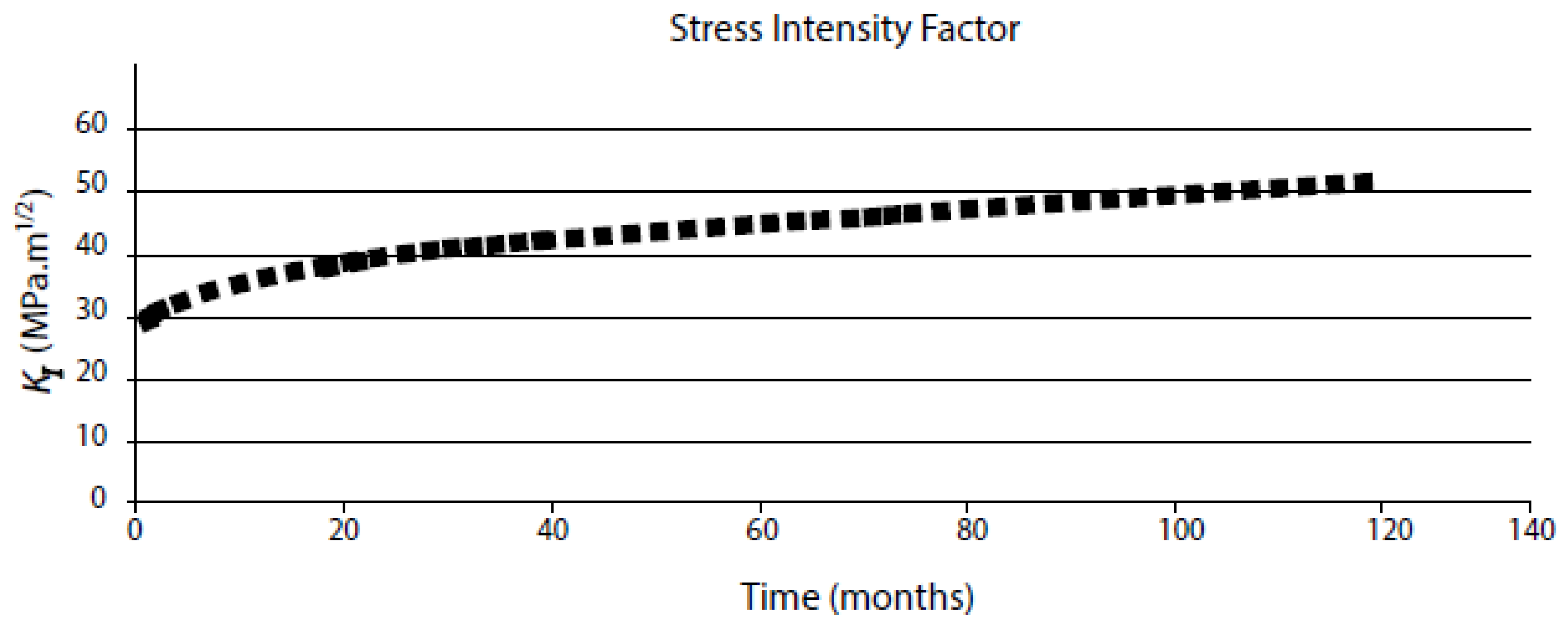
- SCC only occurs if the stress intensity factor (KI) exceeds a lower limit, KISCC. The upper limit of the stress intensity factor, KIc, is defined by unstable, mechanical fracture. Between these limits, cracking occurs via stress corrosion cracking, with the CGR increasing sharply with KI in a Stage I region and then progressing almost independently of KI in a Stage II region [55];
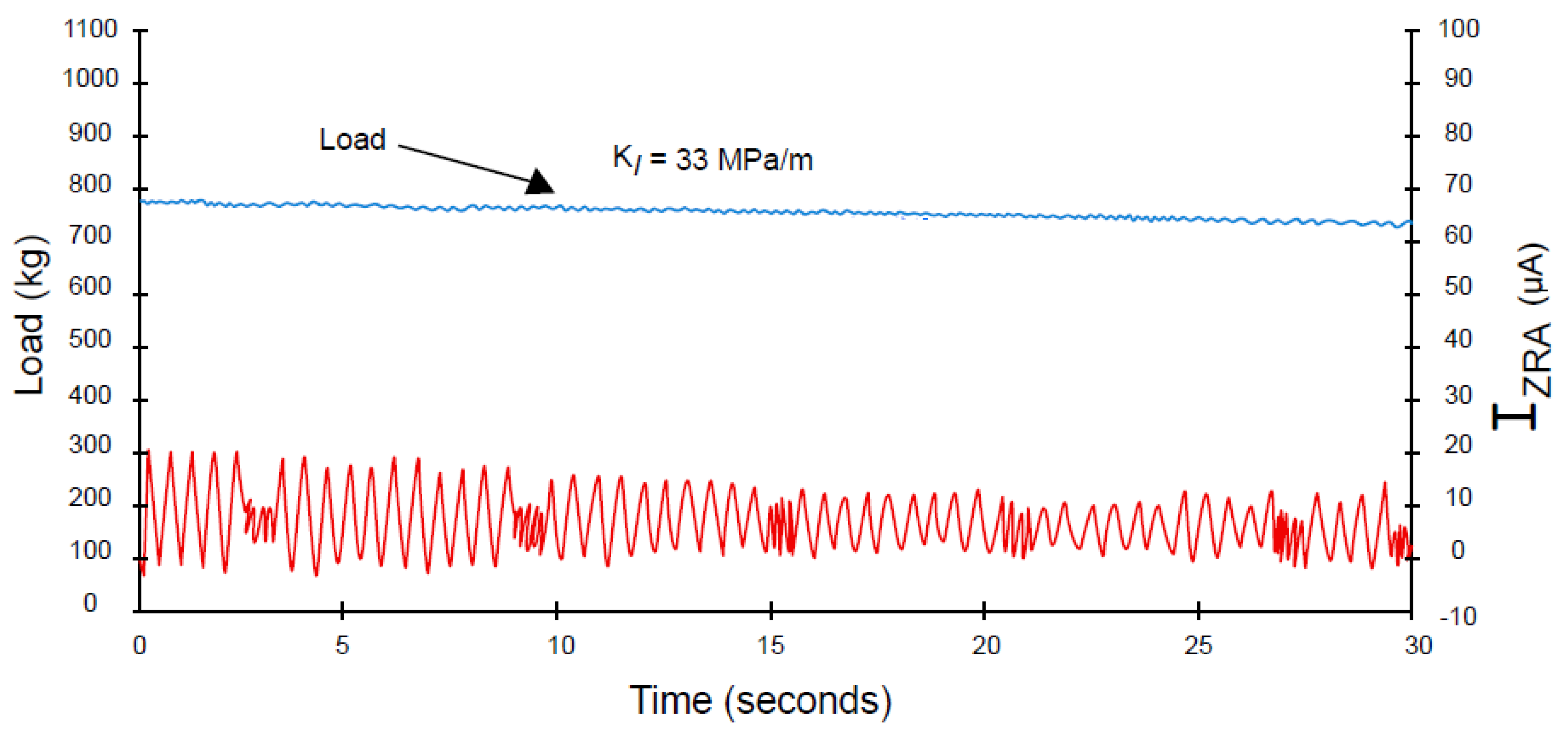
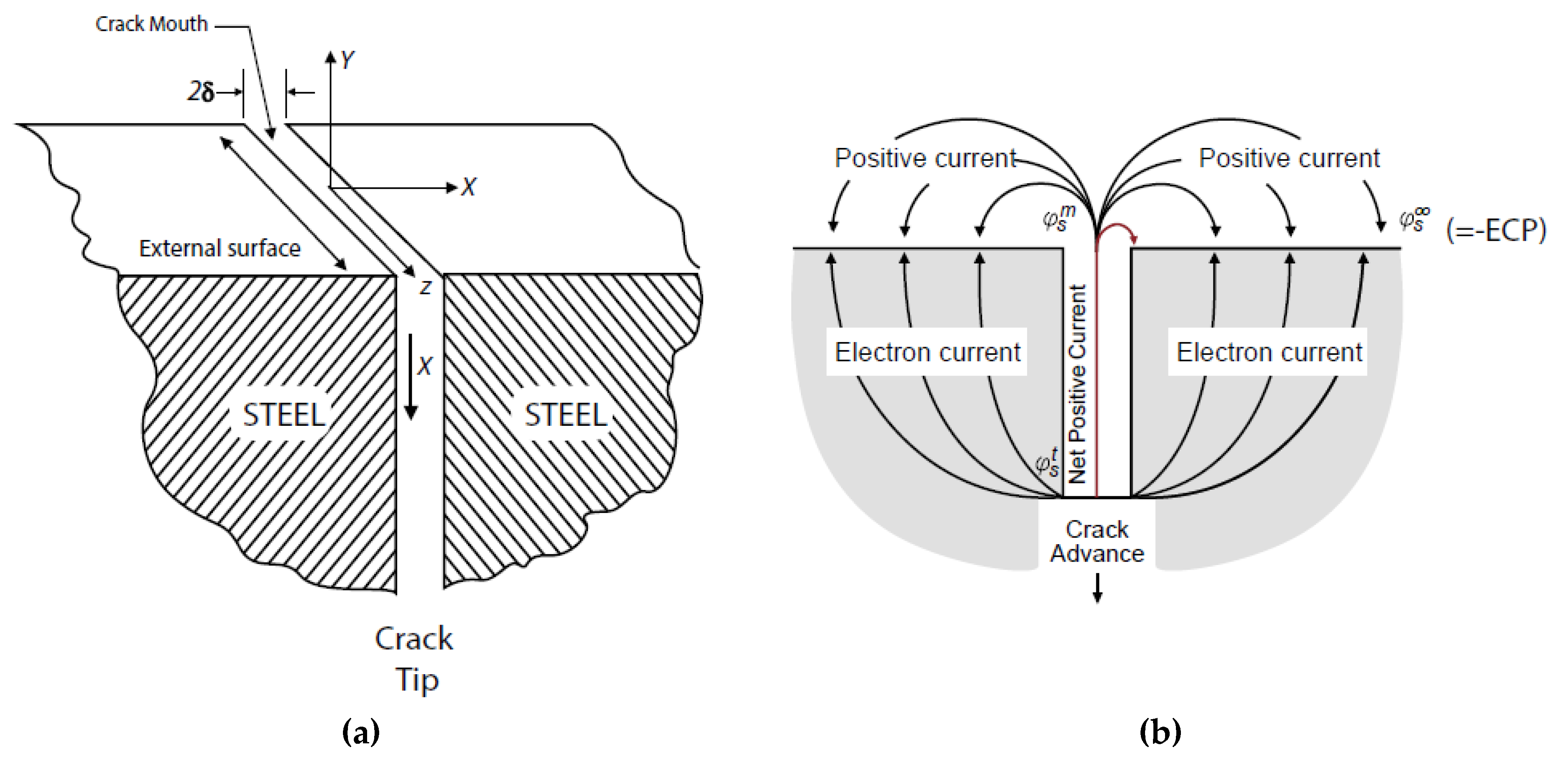
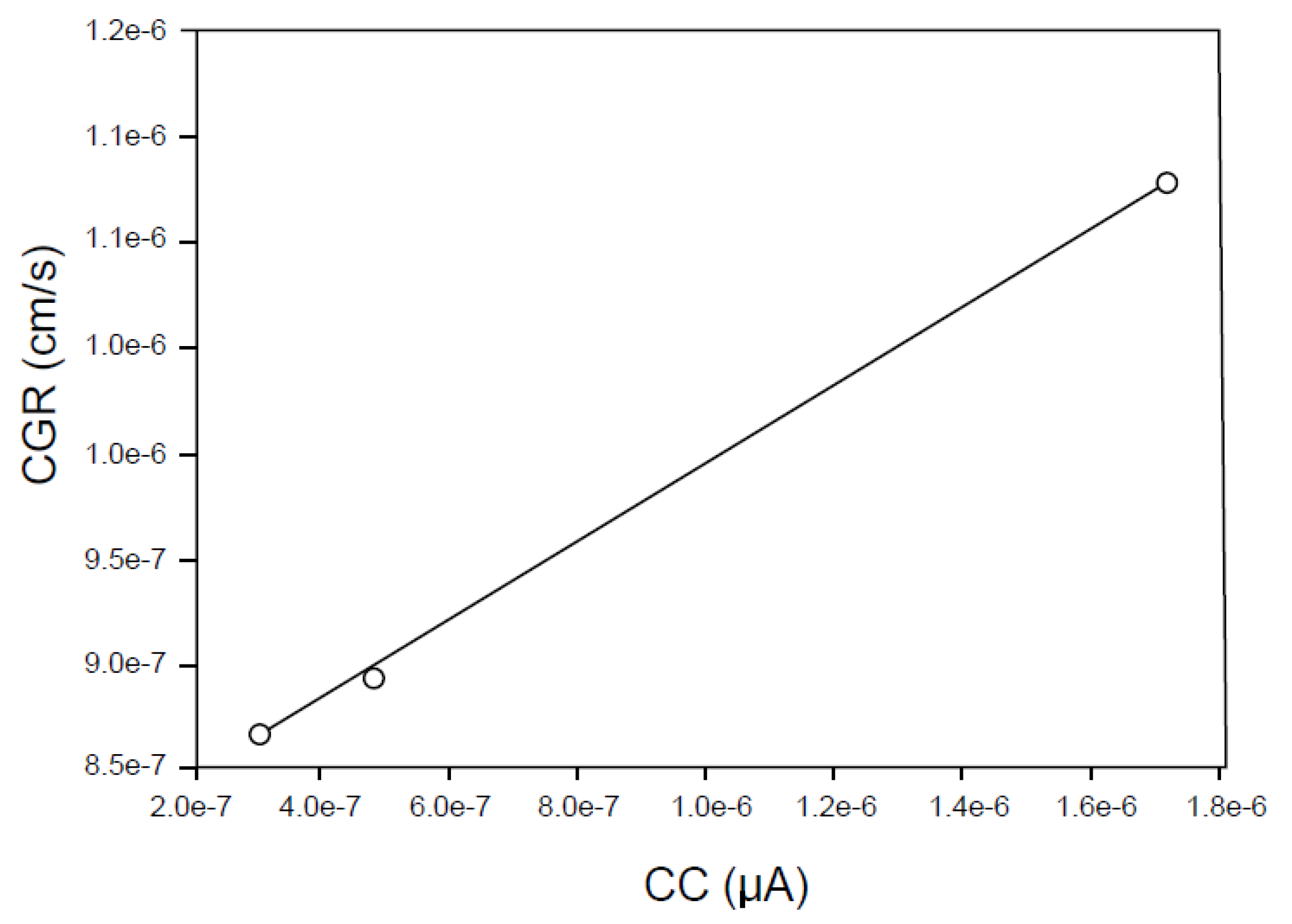
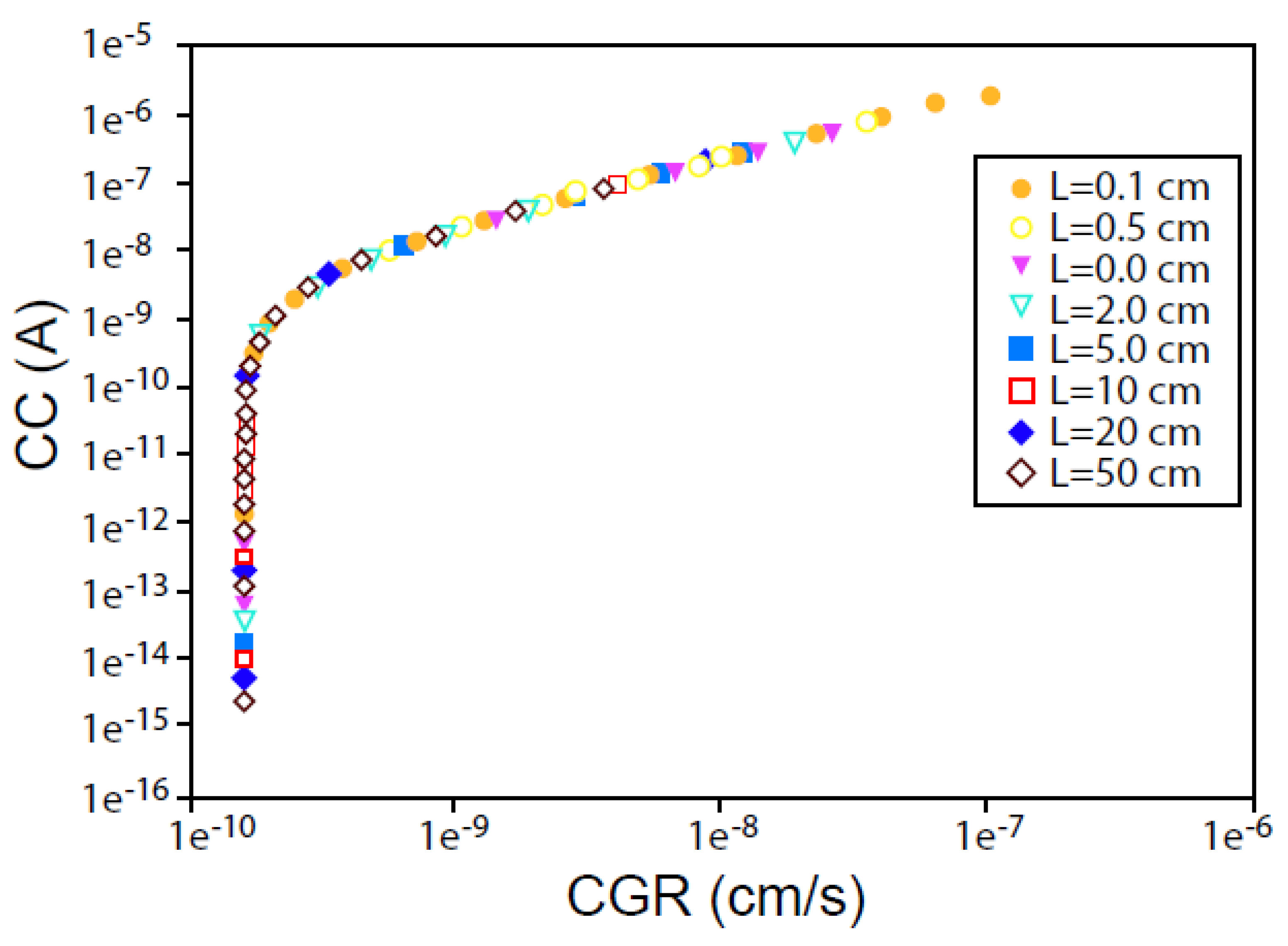
- A coupling current is observed to flow through the solution (including that in the crack) from the crack tip to the external surfaces, where it is annihilated by the corresponding electron current flowing through the metal via a charge transfer reaction (e.g., oxygen reduction) [7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,45,46]. The environmentally mediated CGR is proportional to the magnitude of the coupling current [56];
- Oscillations appear in the coupling current that are attributed to microfracture events at the crack tip. In the case of IGSCC in sensitized Type 304 SS, as described in Ref. [45], the oscillations come in packages that are separated by brief periods of intense activity;
- Coating the external surfaces with an insulator, and hence inhibiting the reduction of oxygen, causes the coupling current to sharply decrease and the crack to be reduced accordingly [57];
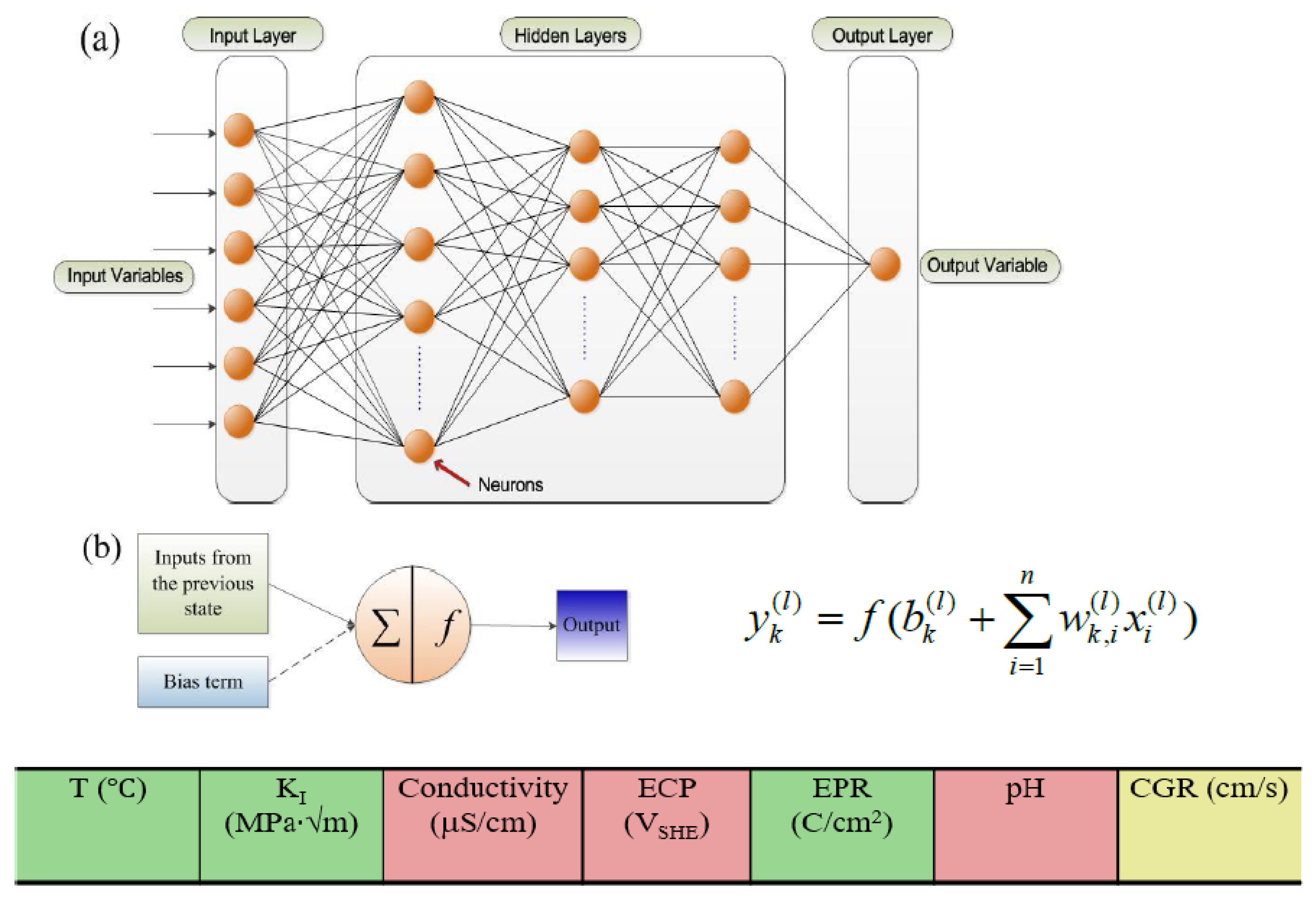
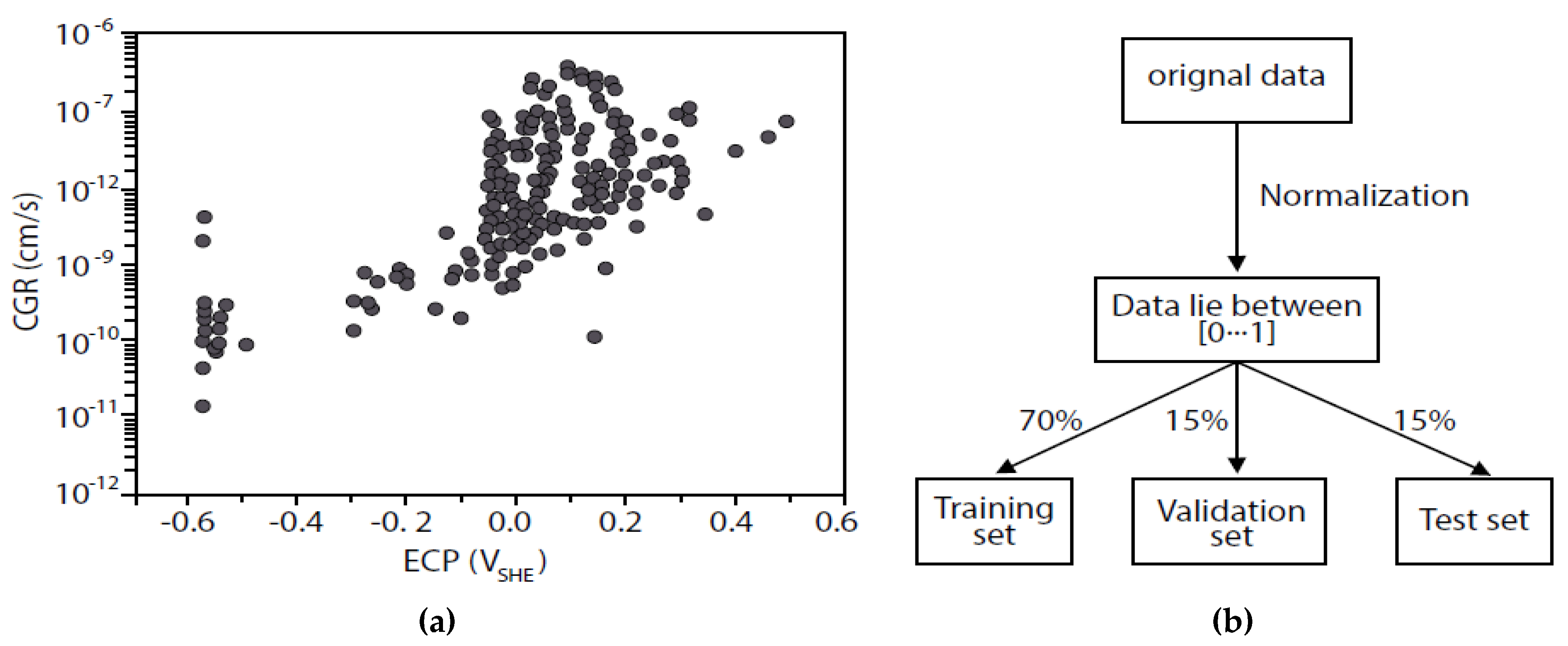
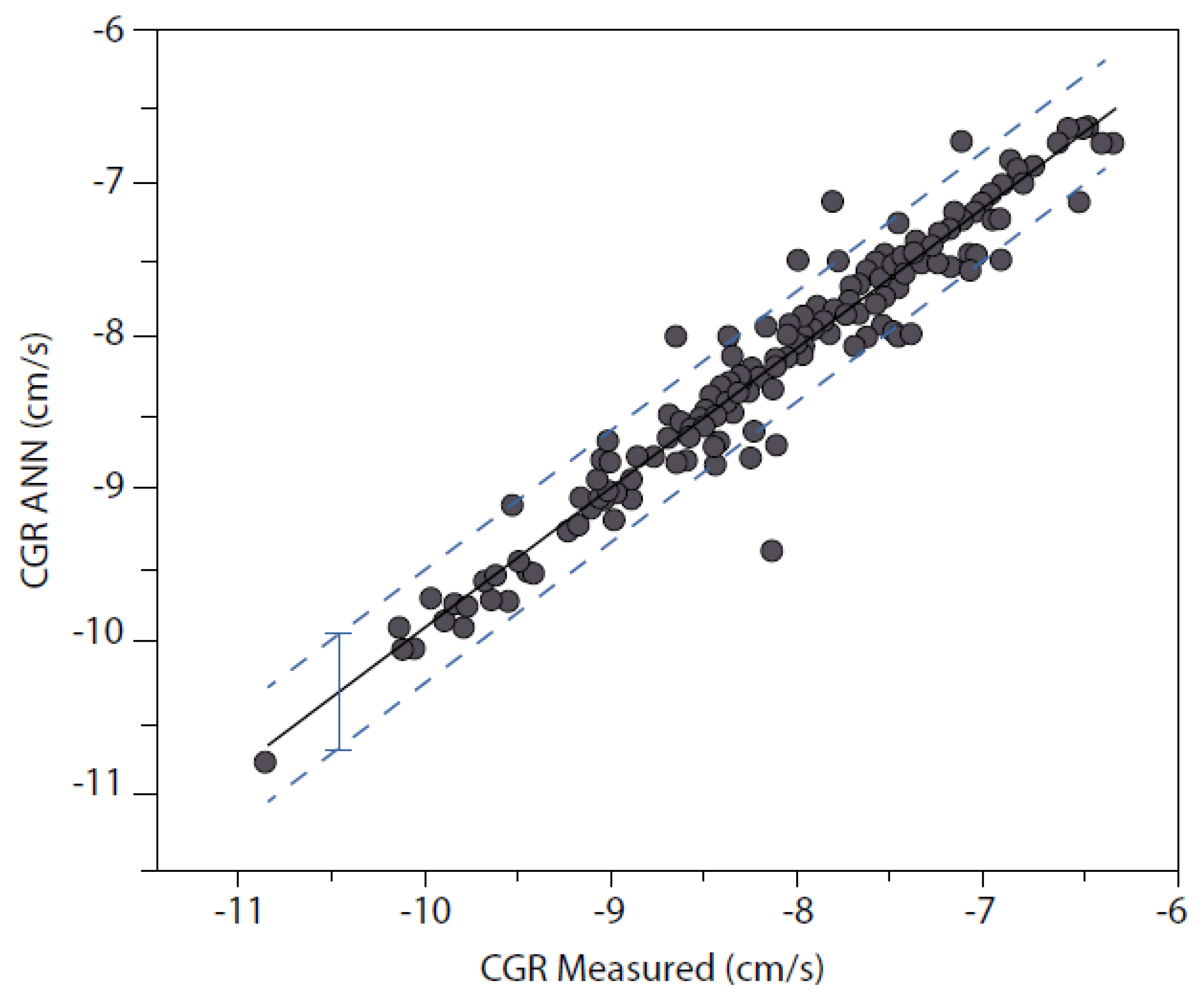
- The character of an SCC model contains contributions from electrochemistry, mechanics and metallurgy, as demonstrated by the ANN analyses reported by Shi et al. [38,39]. The model engine must contain mechanistic concepts and relationships that allow the model to predict the character without the input of any additional information;
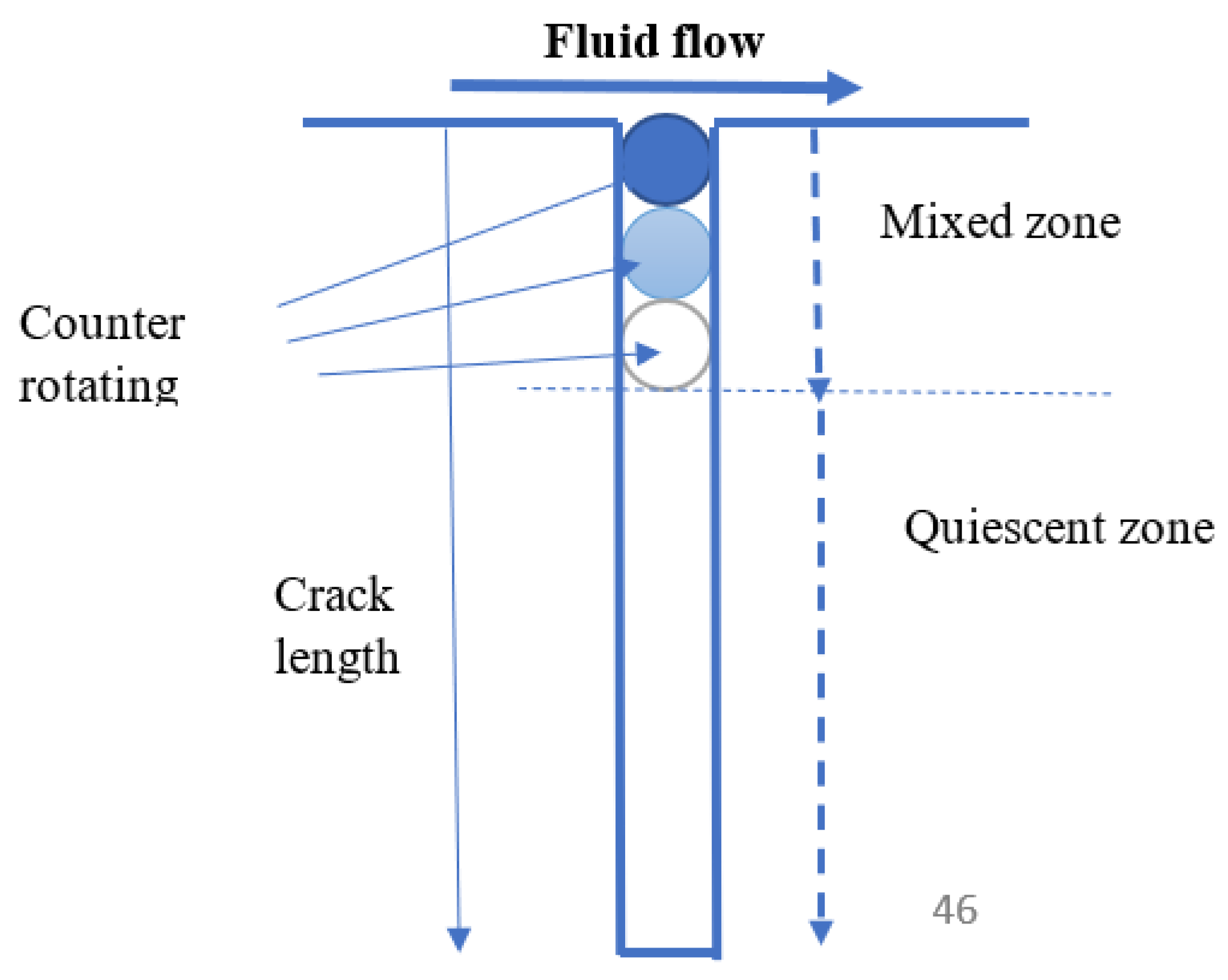
- Enhanced mass transfer of oxygen to the external surfaces increases the CGR [58]. For a sufficiently short, open crack, increasing the flow rate may destroy the aggressive conditions that develop within the cavity and hence inhibit crack growth;
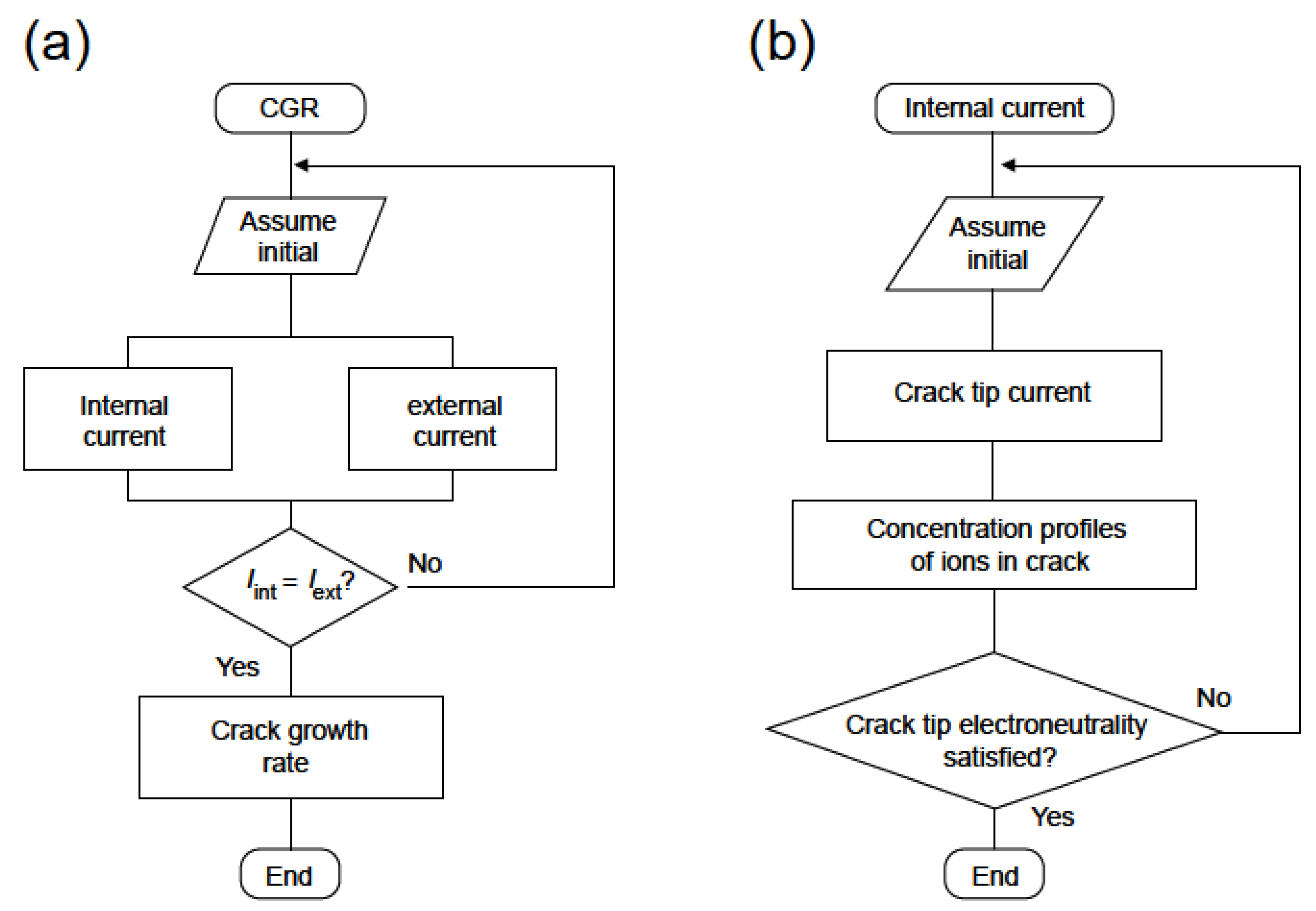
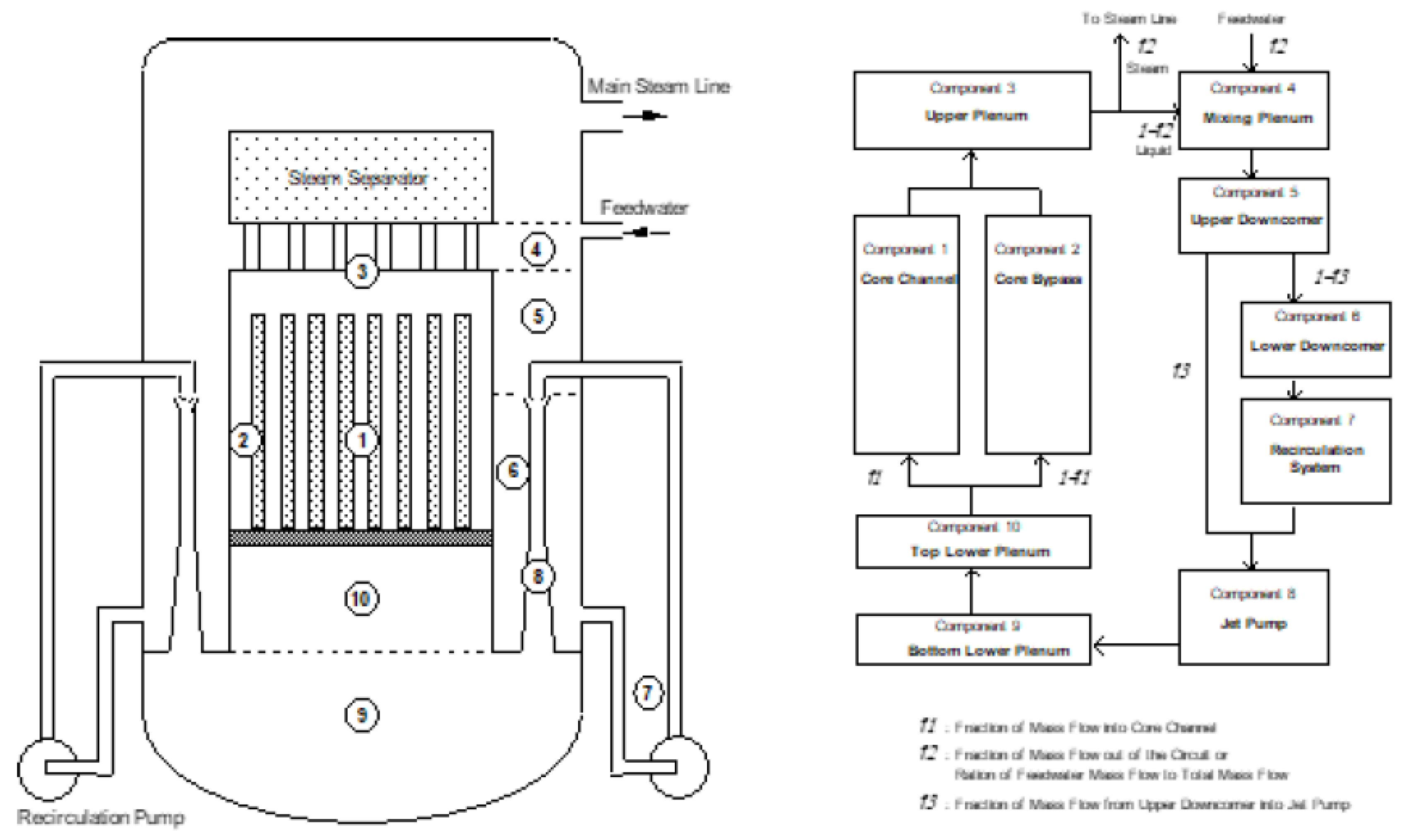
5. The Coupled Environment Fracture Model
5.1. Default Conditions
5.2. Constitutive Equations and Constraints
5.3. Creep Crack Growth Rate
5.4. CEFM Algorithm
6. The Predictions of the CEFM
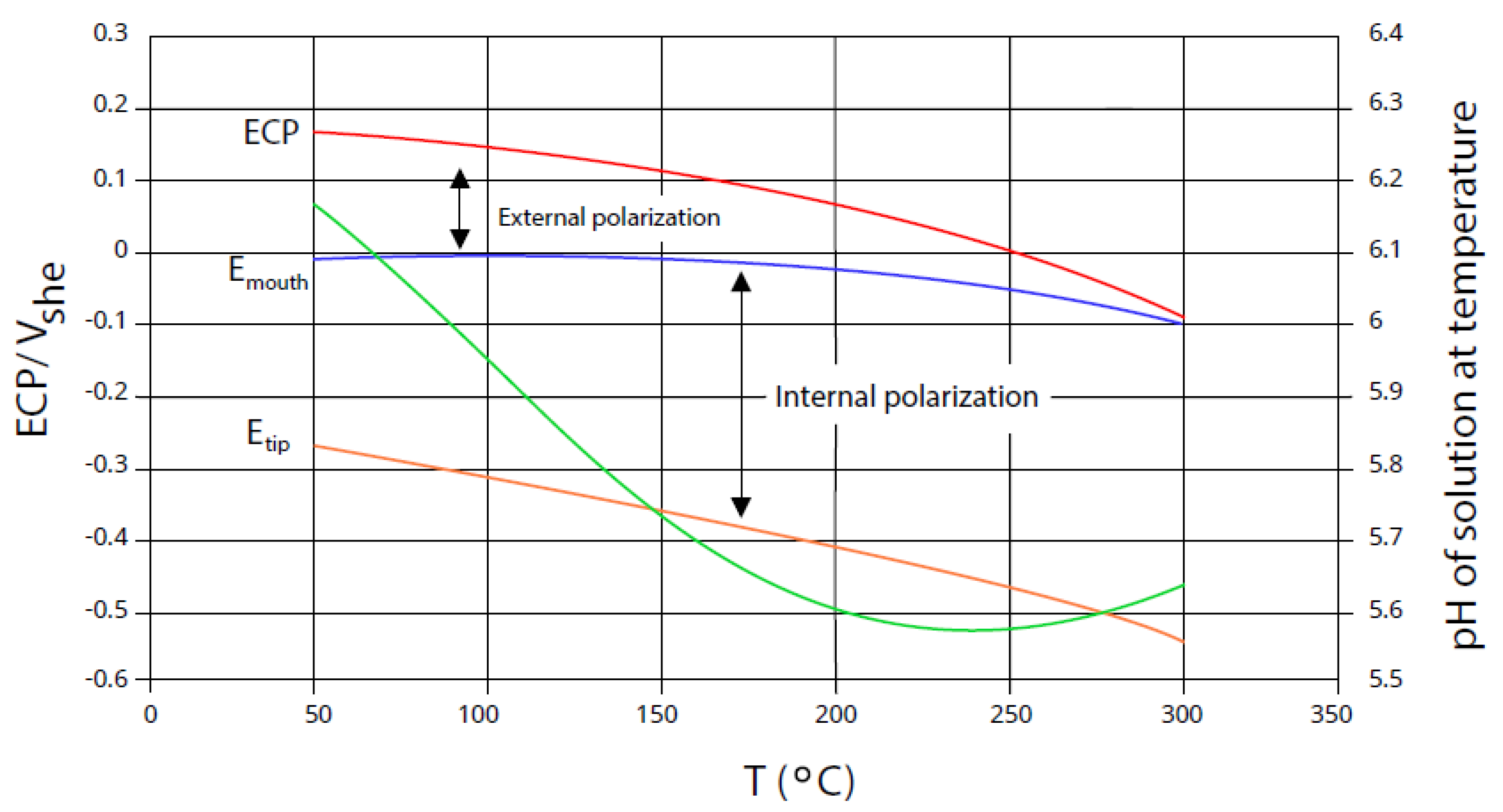
6.1. External Polarization
6.2. Role of the Reactions on the External Surface
6.3. Crack Growth Rate/Coupling Current Relationship
6.4. Crack Tip Conditions
6.5. Effect of Crack Length
6.6. Microfracture Frequency and Dimension
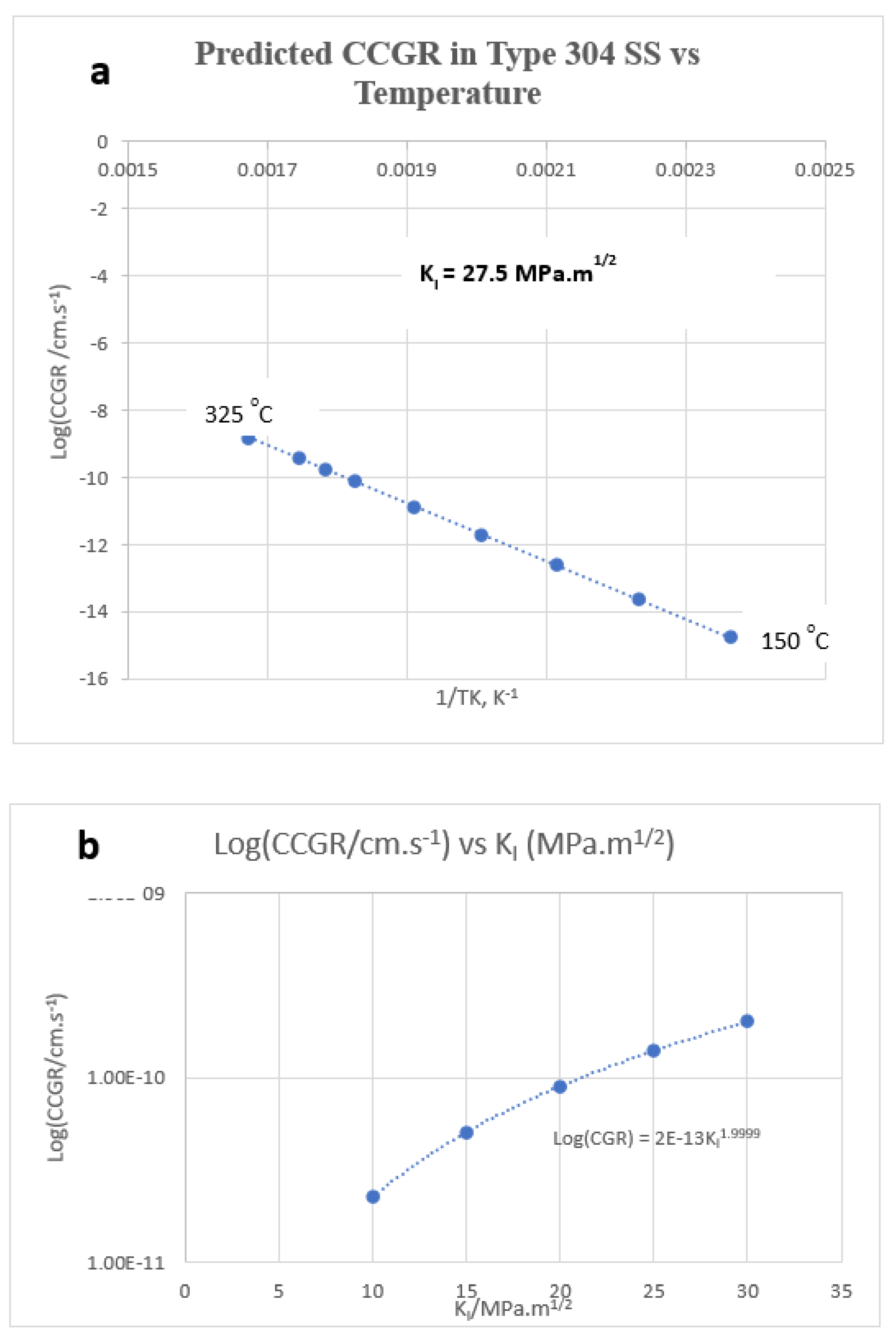
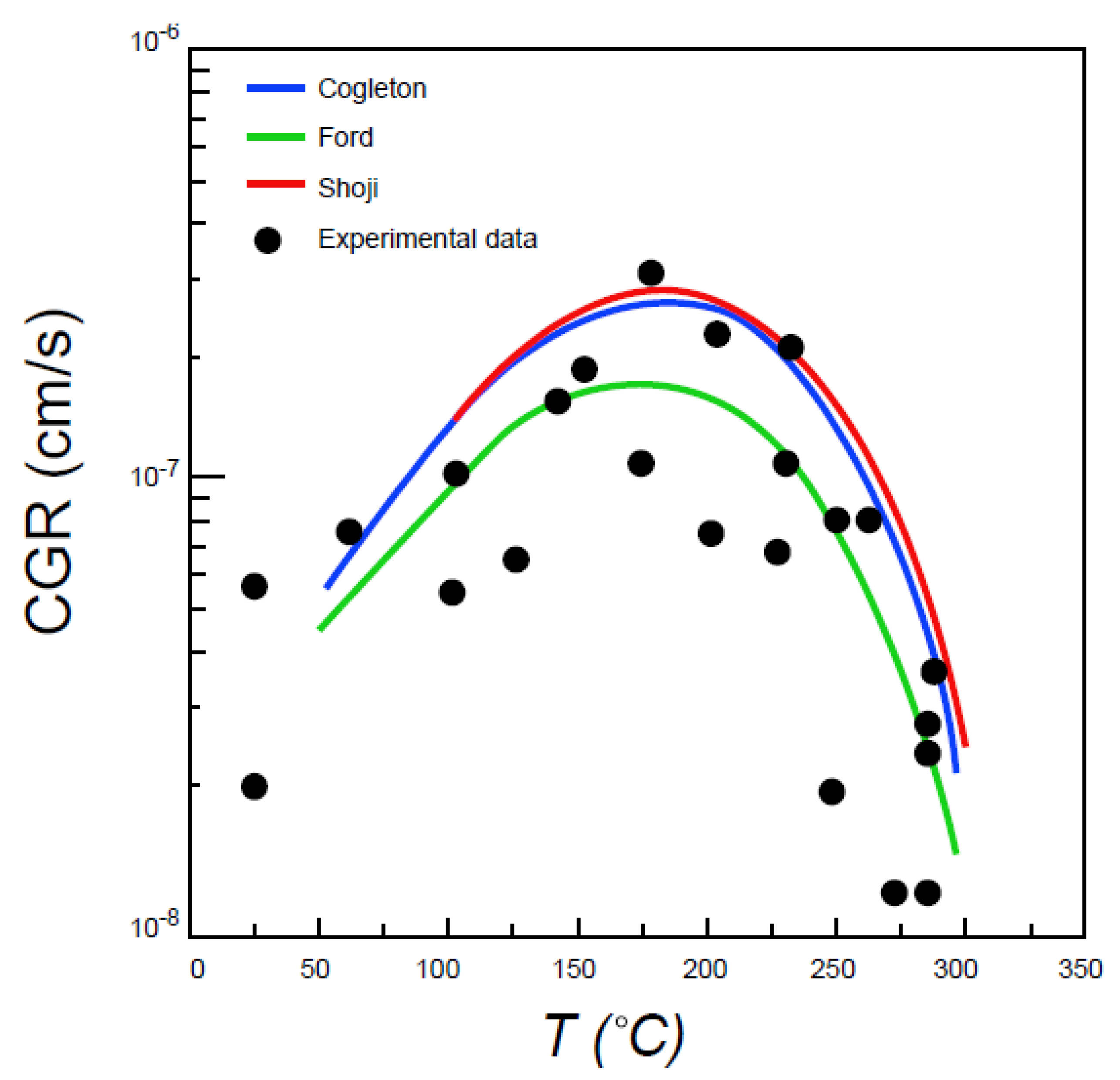
6.7. Effect of Temperature
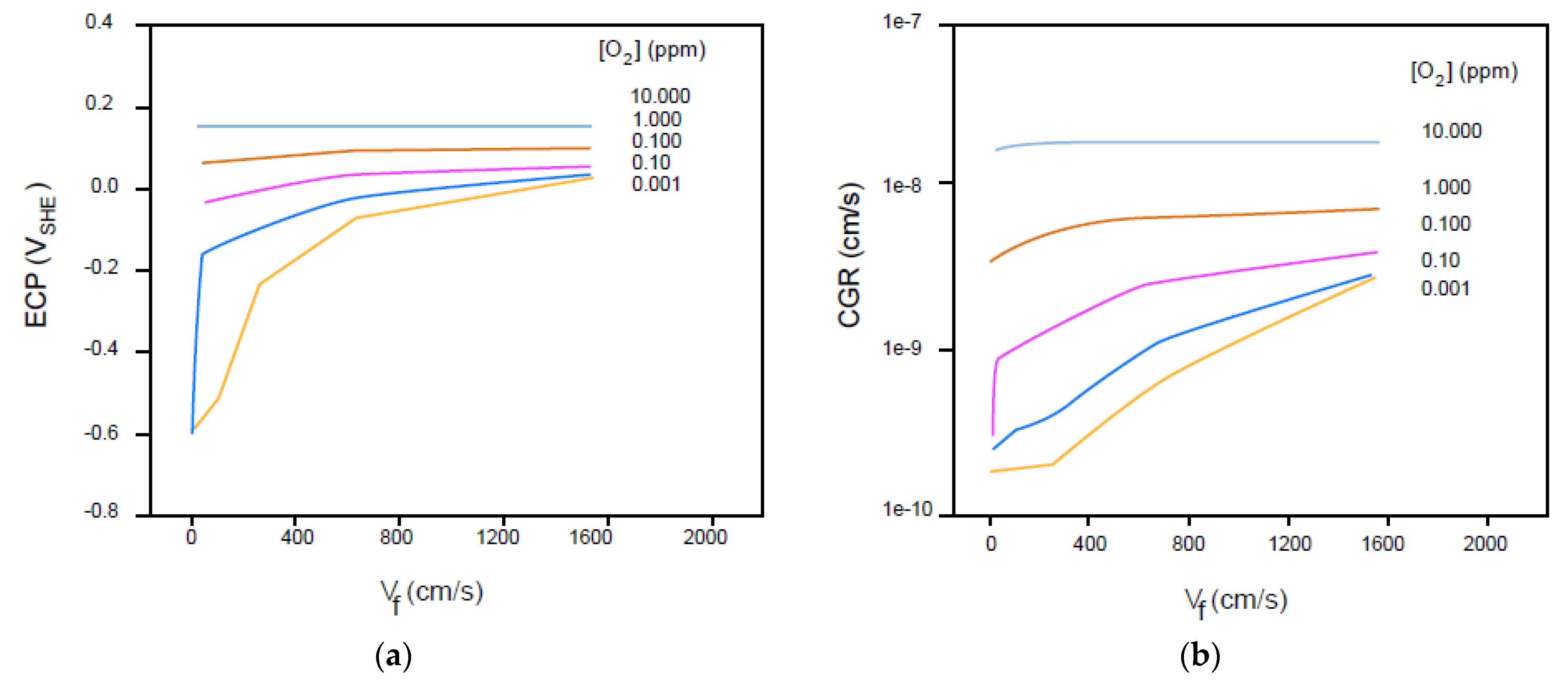
6.8. Effect of Flow Velocity
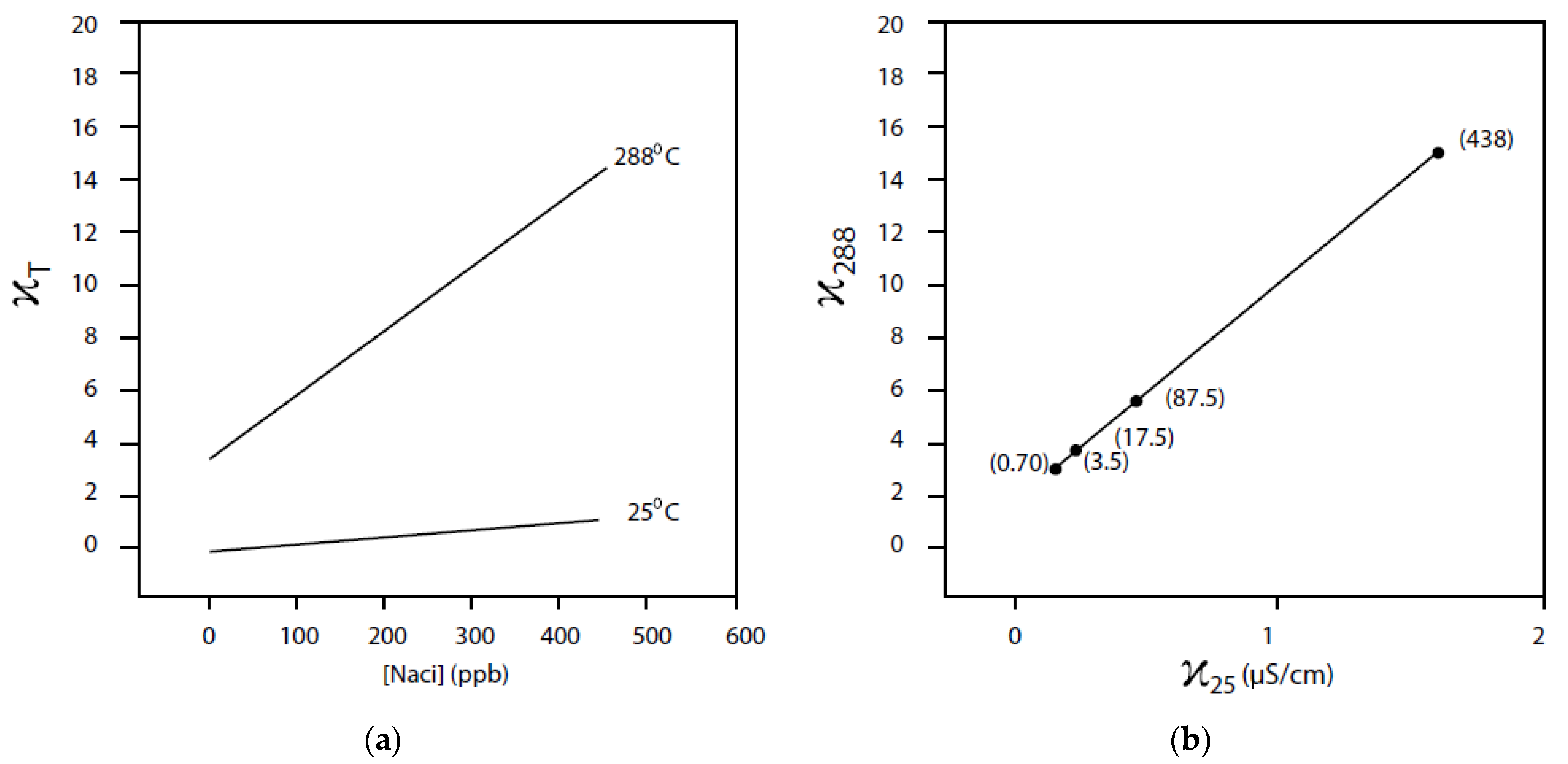
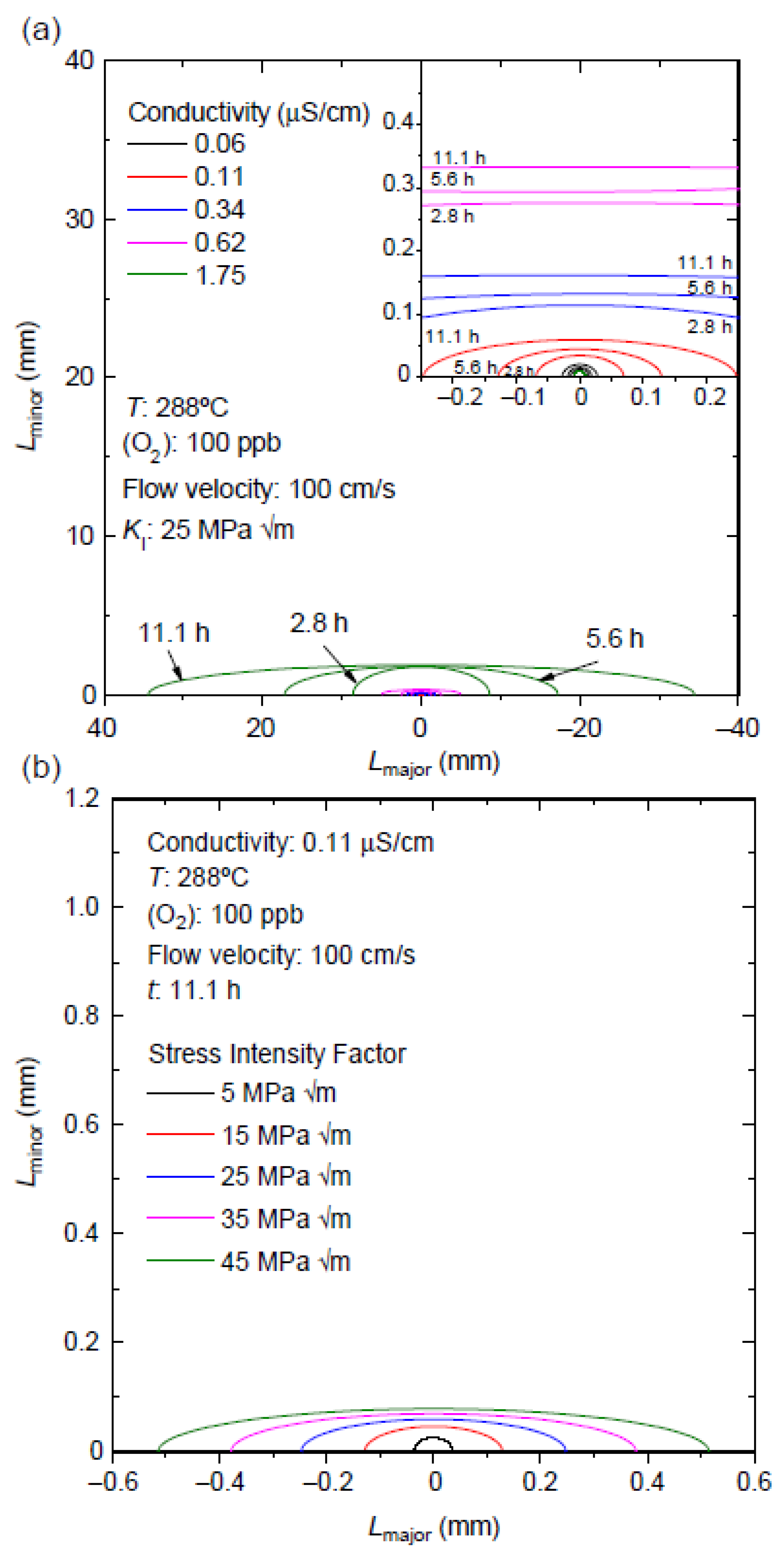
6.9. Effect of Solution Conductivity
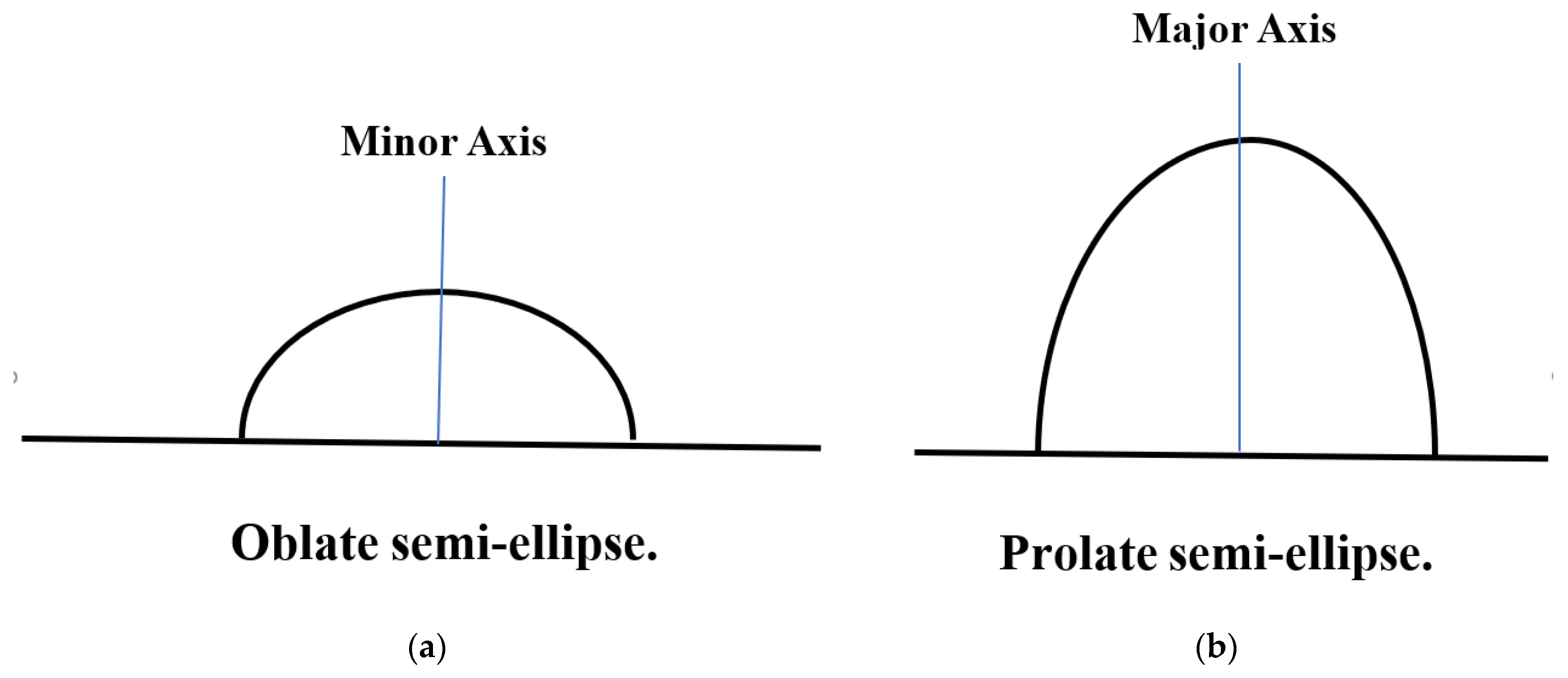
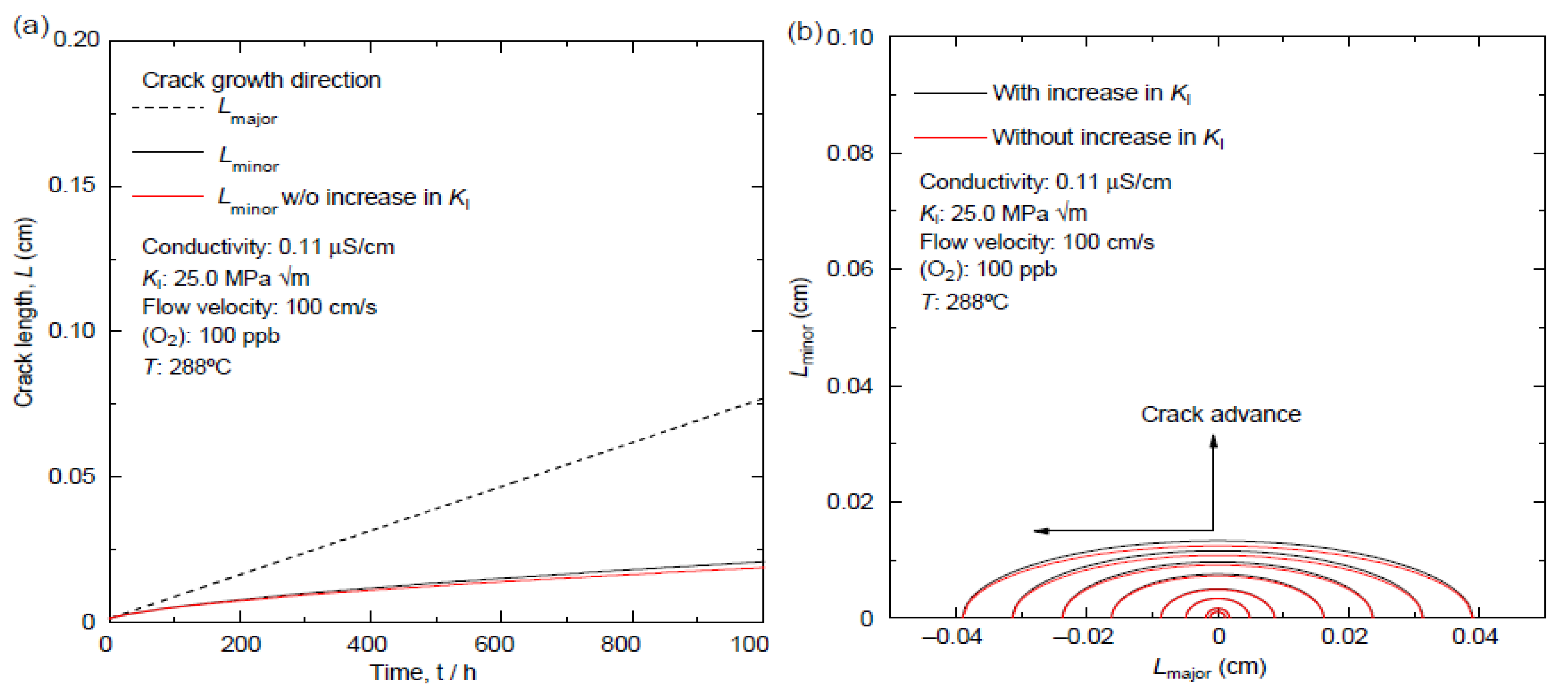
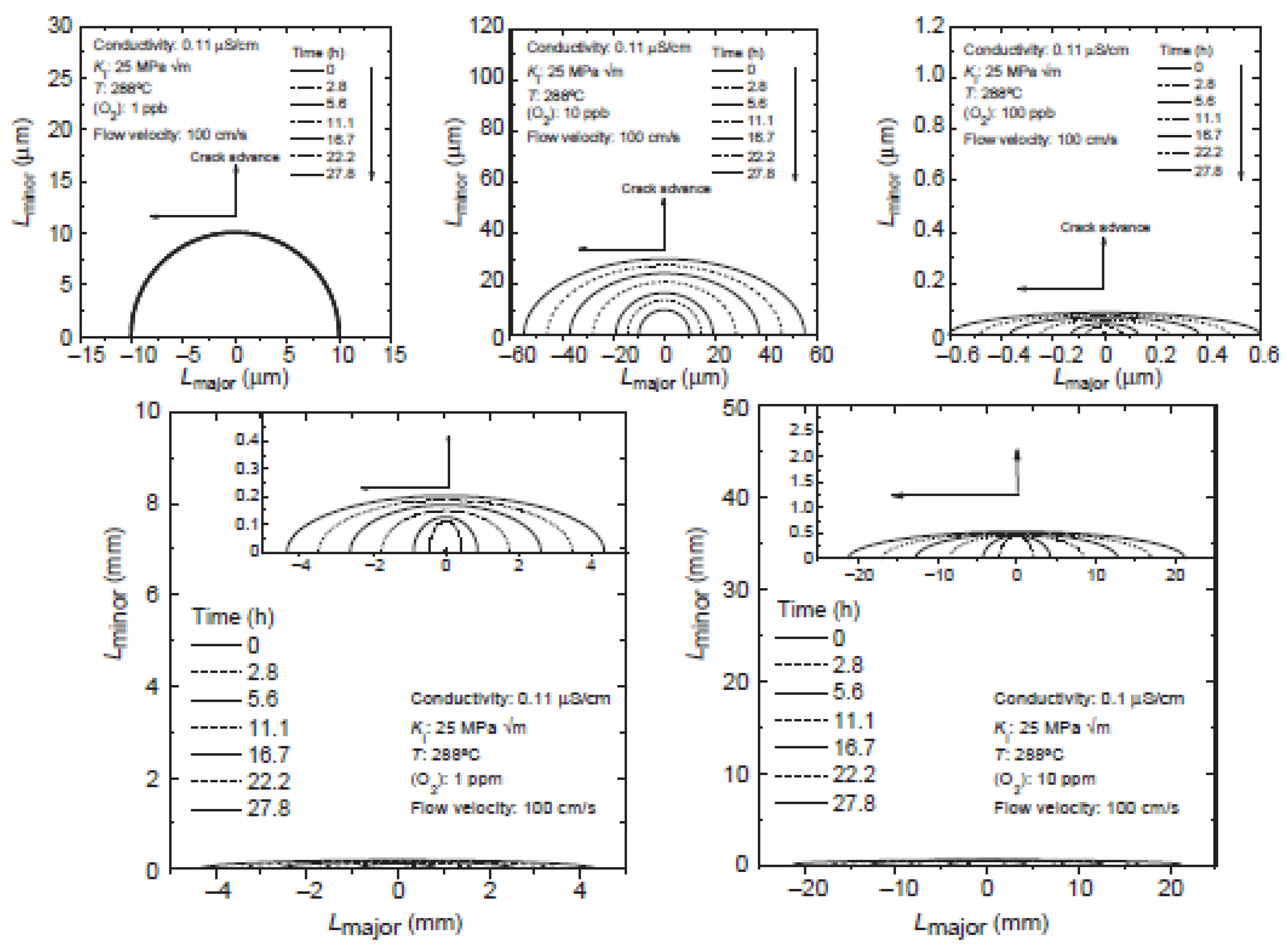
6.10. Development of Semi-Elliptical Cracks
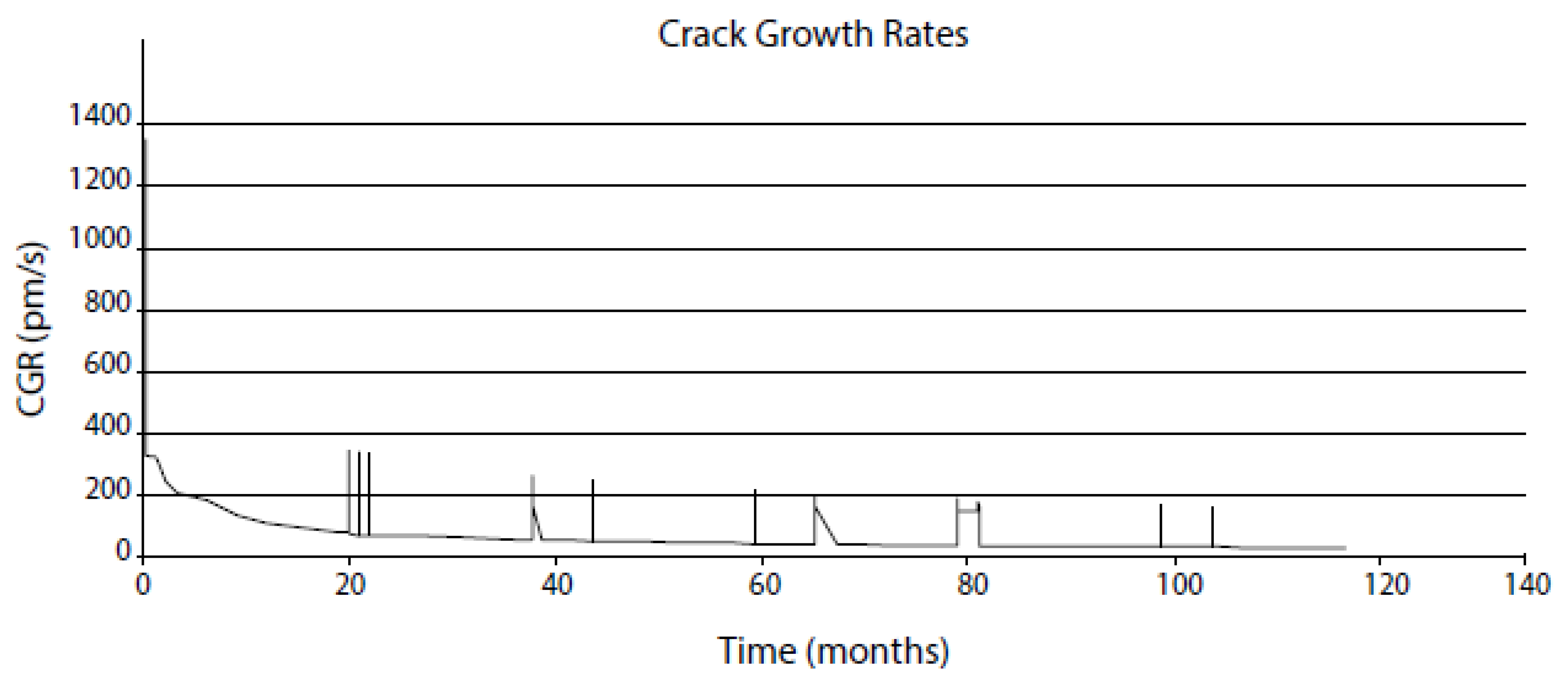
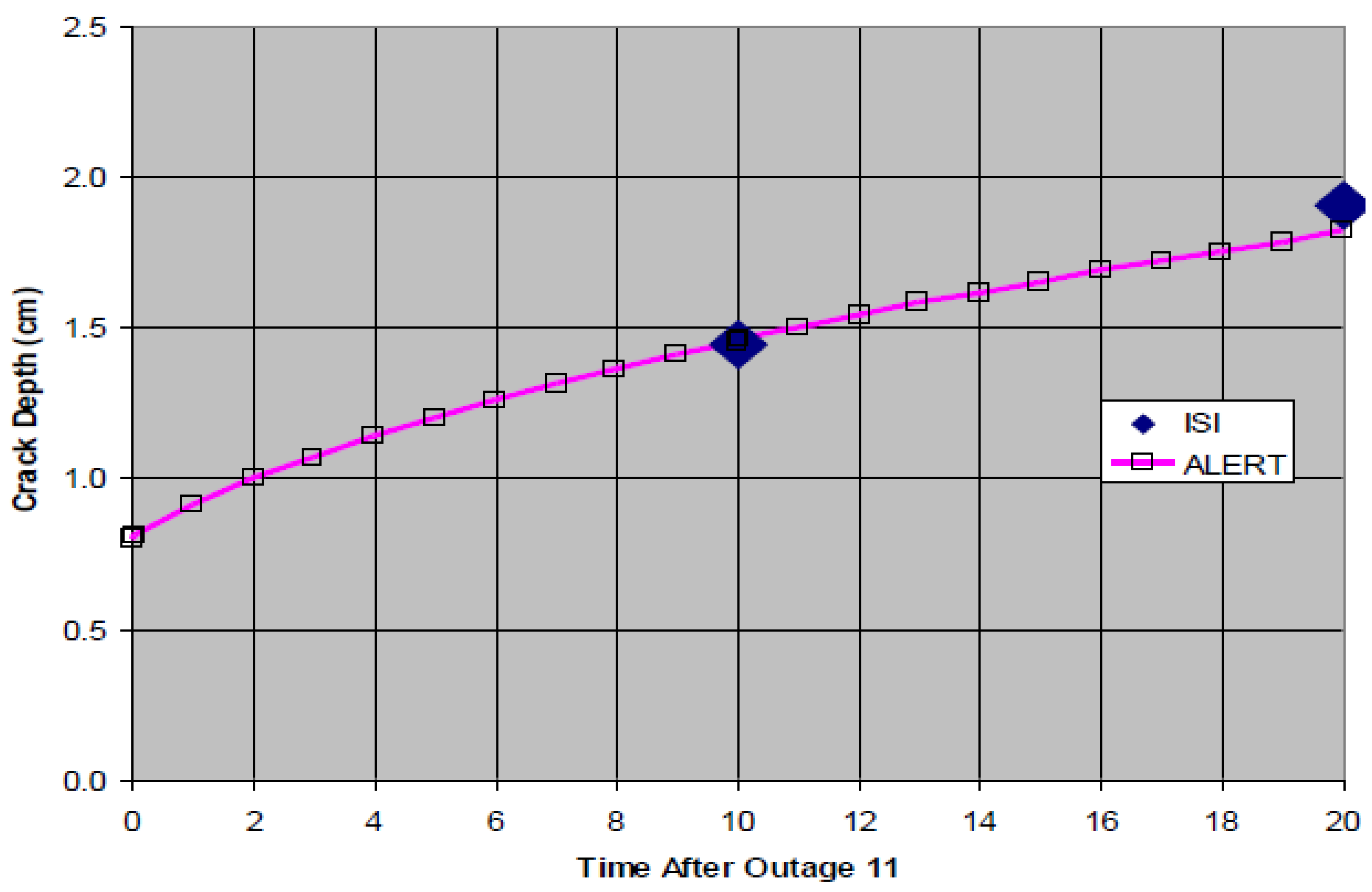
6.11. Global Assessment of the Accuracy of the CEFM
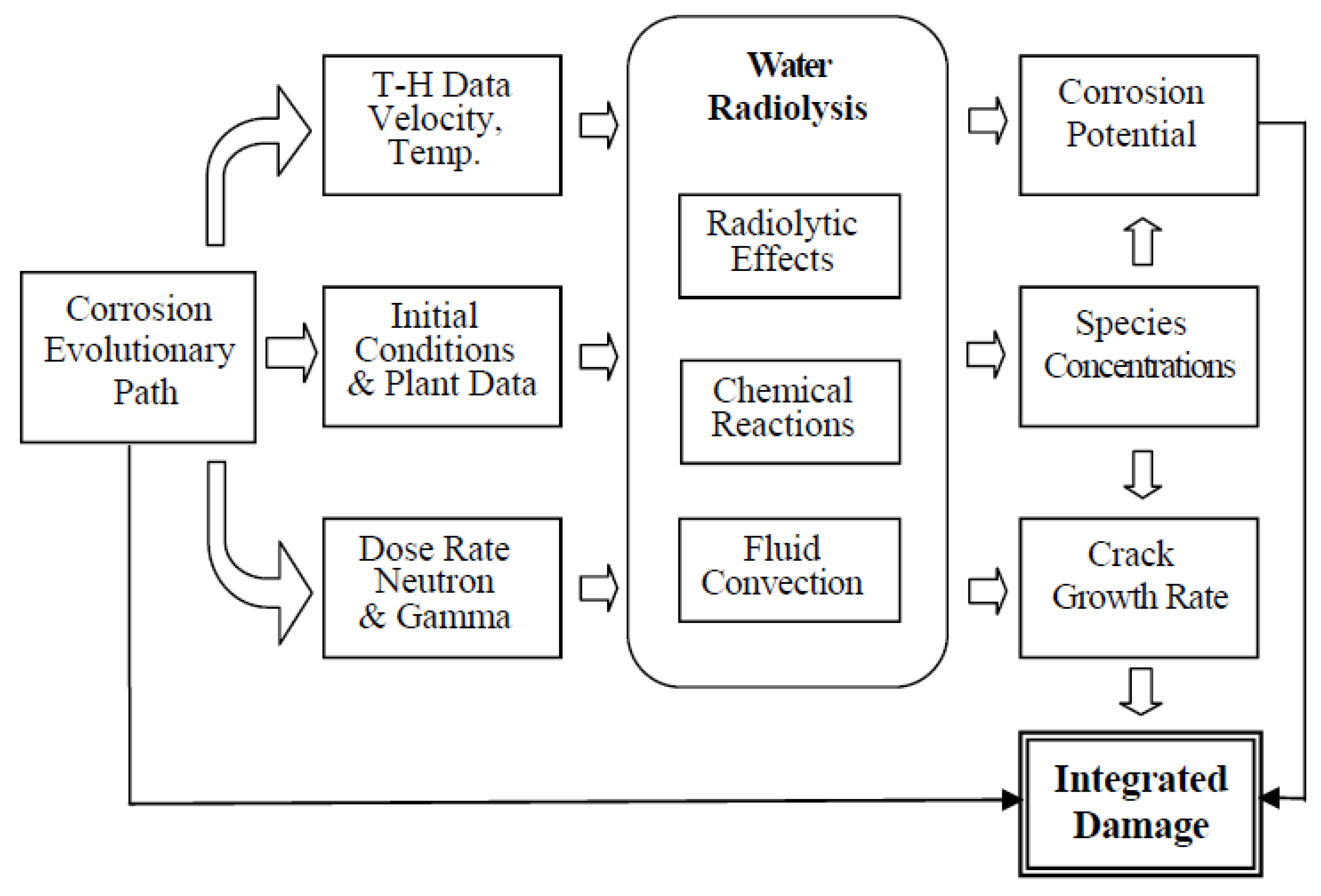
7. Corrosion Evolutionary Path
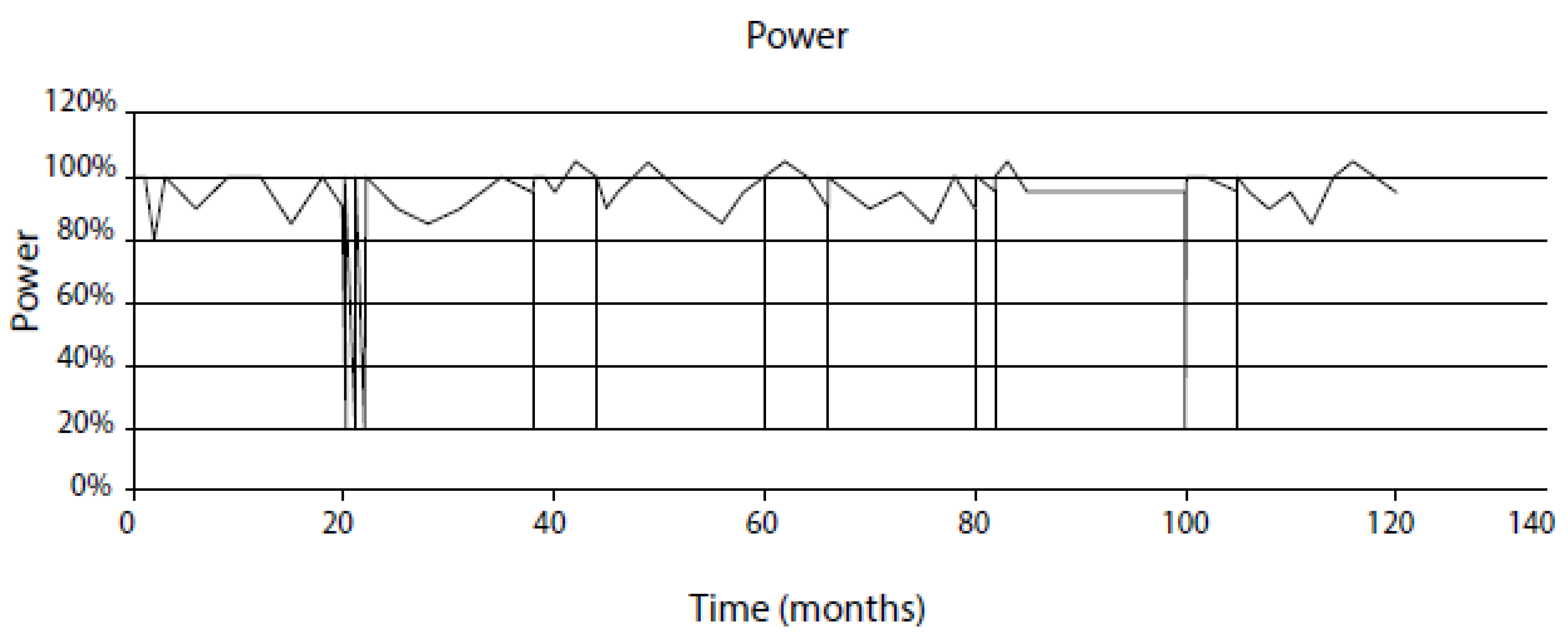
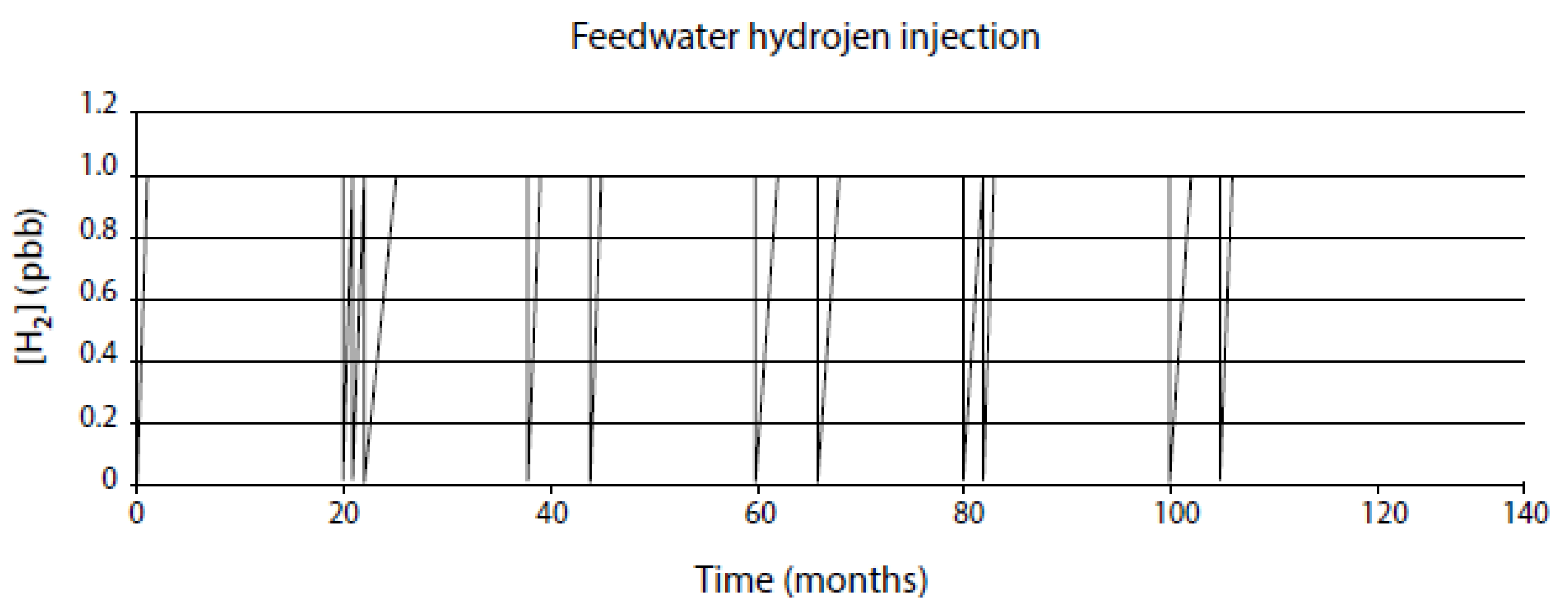
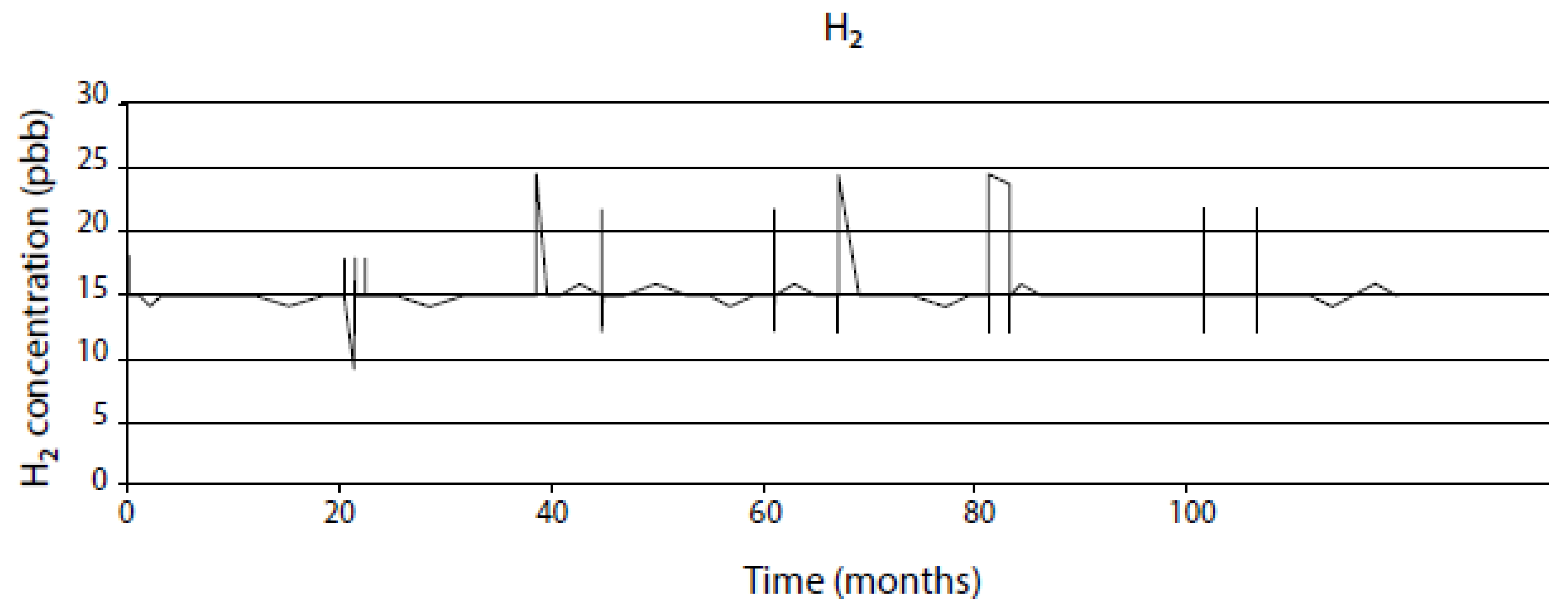
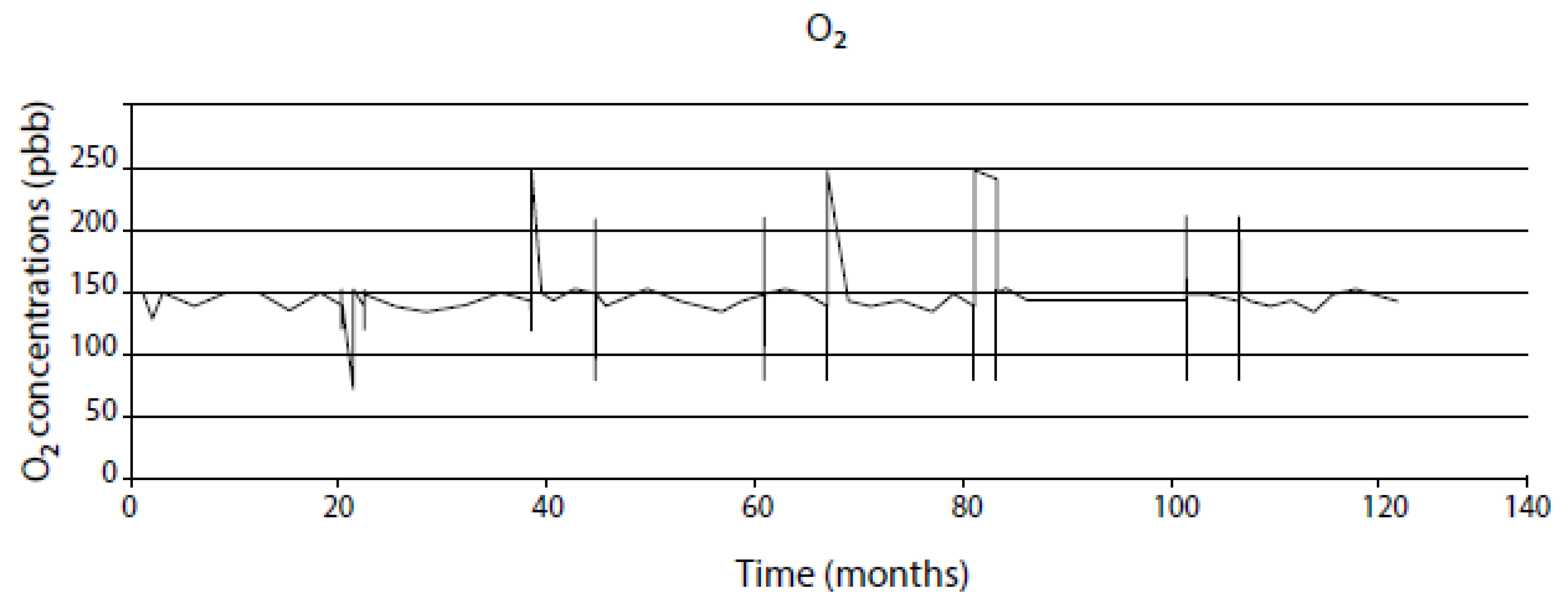
- Assume that the system will behave in the future as it has in the recent past for which a record exists on the evolution of the independent variables. This is a viable approach for modeling nuclear reactors because of the wealth of information that is recorded during operation, although the data are not always of the type or in the form that are readily incorporated into predictive models. For example, power plants record ambient temperature conductivity, not the conductivity at the operating temperature that is employed in the CEFM. Likewise, to the author’s knowledge no nuclear plants regularly monitor the ECP at any point in the coolant circuit. Fortunately, these parameters can be calculated with sufficient accuracy to permit their inclusion in the models.
- Assume a future operating history (CEP) that is designed to probe the impact of specific operating issues, such as HWC, reduced conductivity, stress relief, etc. These “what if” scenarios are one of the great benefits of the modeling described in this paper, because they allow issues to be addressed in a computer that are not practical in an operating reactor. For example, if one sought to define the cost/benefit of operating a reactor with ultralow conductivity, that is more easily done, and at much lower cost, with programs developed by the author and his colleagues, such as REMAIN, ALERT, FOCUS and MASTER_BWR, than by installing the additional ion-exchange columns in the RWCU system that would be required to achieve the desired low conductivity.
8. Summary and Conclusions
- All theories and models are inherently incorrect because they are figments of the modeler’s imagination as conceived via imperfect senses and intellect, so that they can never describe “reality”. However, they are nudged toward that ideal goal by the “scientific method” of cyclical prediction and evaluation. Because of the inherent defects, models ultimately fail (i.e., are “falsified”) and must be replaced by a new model that addresses the shortcomings of the old model.
- The models and theories themselves must be based upon empirical observations and must be employ postulates that are consistent with those observation and upon assumptions that, while not necessarily being demonstratably true, are reasonable expectations of current knowledge.
- In the “scientific method”, the model must not be evaluated against the same data and postulates that were used in formulating the theory and calibrating the model;
- The theory itself should be “global”, in that it accounts for all known observations about the system. “Local” theories are discouraged because they are based upon incomplete information, often being only based upon observations made by a single researcher;
- Importantly, all deterministic models must possess a theoretical basis but not all theories need to calculate;
- All deterministic models must contain a feedback loop that facilitates the enactment of the “scientific method”, in which the model is continually tested against new observations. If discrepancies are observed, the model is modified within the bounds of observation and the prediction is repeated;
- A deterministic model generally comprises constitutive equations that describe the operation of the model and constraints, the latter commonly being the natural conservation laws. The number of constitutive equations and the number of constraints must be at least equal to the number of unknown parameters in the model;
- If no amount of change within the bounds of observation can resolve the problem, the model and the theory must be discarded (“i.e., the model is “falsified”).
- It is important to note that no amount of successful prediction can “prove” a model, and its underlying theory to be correct, but only one instance of disagreement is necessary to prove the model and theory incorrect.
Funding
Data Availability Statement
Conflicts of Interest
References
- Macdonald, D.D.; Engelhardt, G.R. Predictive Modeling of Corrosion. In Shrier’s Corrosion; Cottis, B., Graham, M., Lindsay, R., Lyon, S., Richardson, T., Scantlebury, D., Stott, H., Eds.; Elsevier: Amsterdam, The Netherlands, 2010; Volume 2, pp. 1630–1679. [Google Scholar]
- Koch, G.H.; Brongers, M.P.H.; Thompson, N.G.; Virmani, P.; Payer, J.H. Corrosion Costs and Preventive Strategies in the United States; Publication No. FHWA-RD-01-156; NACE International: Houston, TX, USA, 2002. [Google Scholar]
- GDP Growth & Recessions. Available online: https://www.thebalance.com/hurricane-damage-economic-costs-4150369 (accessed on 23 February 2023).
- Earthquakes Are Estimated to Cost the Nation $6.1 Billion Annually in Building Stock Losses According to an Updated Report Published Today by FEMA. Available online: https://www.usgs.gov/news/usgs-collaborates-fema-national-earthquake-loss-estimate#:~:text=Earthquakes%20are%20estimated%20to%20cost,report%20published%20today%20by%20FEMA (accessed on 23 February 2023).
- Nuclear Plant Outages Costly. Available online: https://richmond.com/business/nuclear-plant-outages-costly/article_da2b7cbe-b2ef-567c-a28d-694459d0b726.html (accessed on 23 February 2023).
- Aristotle. Physiks: 350 BC; Infomotions, Inc.: Champaign, IL, USA, 2001. [Google Scholar]
- Macdonald, D.D.; Urquidi-Macdonald, M. A coupled environment model for stress corrosion cracking in sensitized type 304 stainless steel in LWR environments. Corros. Sci. 1991, 32, 51–81. [Google Scholar] [CrossRef]
- Vankeerberghen, M.; Macdonald, D.D. Calculating the Temperature-Maximum and the Lower Potential Limit for the Crack Growth Rate in Type 304 SS Using the CEFM. In Proceedings of the CORROSION 2003 (NACE), San Diego, CA, USA, 16–20 March 2003. Paper No. 03520. [Google Scholar]
- Engelhardt, G.R.; Macdonald, D.D.; Urquidi-Macdonald, M. Development of Fast Algorithms for Estimating Stress Corrosion Crack Growth Rate in Sensitized Stainless Steels in Boiling Water Reactor Coolant Environments. In Proceedings of the CORROSION/99, San Antonio, TX, USA, 25–30 April 1999; Paper #452. pp. 1–32. [Google Scholar]
- Macdonald, D.D.; Urquidi-Macdonald, M. An Advanced Coupled Environment Fracture Model for Predicting Crack Growth Rates. Chapter 4—Control, Mitigation, and Prediction of Stress Corrosion Cracking. In Proceedings of the TMS Parkins Symposium on Fundamental Aspects of Stress Corrosion Cracking, Cincinnati, OH, USA, 20–24 October 1991; pp. 443–455. [Google Scholar]
- Macdonald, D.D.; Urquidi-Macdonald, M. An Advanced Coupled Environment Fracture Model for Predicting Crack Growth Rates in LWR Heat Transport Circuits. In Proceedings of the Fifth International Symposium on Environmental Degradation of Materials in Nuclear Power Systems—Water Reactors, Monterey, CA, USA, 25–29 August 1991; National Association of Corrosion Engineers, International (ANS/NACE): Houston, TX, USA, 1992; pp. 345–349. [Google Scholar]
- Lu, P.C.; Macdonald, D.D.; Urquidi-Macdonald, M.; Yeh, T.K. Theoretical Estimation of Crack Growth Rates in Type 304 Stainless Steel in BWR Coolant Environments. Corrosion 1996, 52, 768–785. [Google Scholar]
- Vankeerberghen, M.; Macdonald, D.D. Predicting crack growth rate vs. temperature behaviour of Type 304 stainless steel in dilute sulphuric acid solutions. Corros. Sci. 2002, 44, 1425–1441. [Google Scholar] [CrossRef]
- Macdonald, D.D. Chapter 6 in Stress Corrosion Cracking of Nickel-based alloys in Water-cooled Reactors. In The Electrochemical Nature of Stress Corrosion Cracking; The Coriou Effect; Woodhead Publishing: Cambridge, UK, 2016. [Google Scholar]
- Yeh, T.K.; Macdonald, D.D.; Motta, A.T. Modeling Water Chemistry, Electrochemical Corrosion Potential and Crack Growth Rate in the Boiling Water Reactor Heat Transport Circuits—Part I: The DAMAGE-PREDICTOR Algorithm. Nucl. Sci. Eng. 1995, 121, 468–482. [Google Scholar] [CrossRef]
- Yeh, T.K.; Macdonald, D.D.; Motta, A.T. Modeling Water Chemistry, Electrochemical Corrosion Potential and Crack Growth Rate in the Boiling Water Reactor Heat Transport Circuits—Part II: Simulation of Operating Reactors. Nucl. Sci. Eng. 1996, 123, 295–304. [Google Scholar] [CrossRef]
- Yeh, T.K.; Macdonald, D.D.; Motta, A.T. Modeling Water Chemistry, Electrochemical Corrosion Potential, and Crack Growth Rate in the Boiling Water Reactor Heat Transport Circuits—Part III: Effect of Reactor Power Level. Nucl. Sci. Eng. 1996, 123, 305–316. [Google Scholar] [CrossRef]
- Yeh, T.K.; Macdonald, D.D. Predictions of Enhancing Hydrogen Water Chemistry for Boiling Water Reactors by General Catalysis and General Inhibition. In Proceedings of the Corrosion/96, Denver, CO, USA, 26–31 March 1996; NACE International: Houston, TX, USA; pp. 124/1–124/10, Paper #124. [Google Scholar]
- Yeh, T.K.; Macdonald, D.D. Effects of Power Level Change on the Development of Damage in Boiling Water Reactors under Hydrogen Water Chemistry. In Proceedings of the Corrosion/96, Denver, CO, USA, 26–31 March 1996; NACE International: Houston, TX, USA; pp. 126/1–126/12, Paper 126. [Google Scholar]
- Yeh, T.K.; Liang, C.-H.; Yu, M.-S.; Macdonald, D.D. The Effect of Catalytic Coatings on IGSCC Mitigation for Boiling Water Reactors Operated under Hydrogen Water Chemistry. In Proceedings of the 8th International Symposium on Environmental Degradation of Materials in Nuclear Power Systems—Water Reactors, Amelia Island, FL, USA, 10–14 August 1997; NACE International: Houston, TX, USA, 1997; Volume 1, pp. 551–558. [Google Scholar]
- Macdonald, D.D.; Yeh, T.K. The Efficiency of Noble Metals in Reducing the Corrosion Potential in the Primary Coolant Circuits of Boiling Water Reactors Operating under Hydrogen Water Chemistry Operation. J. Nuclear Sci. Tech. 2006, 43, 1228–1236. [Google Scholar]
- Frigg, R.; Hartmann, S. Models in Science. In The Stanford Encyclopedia of Philosophy; Zalta, E.N., Ed.; Stanford University: Stanford, CA, USA, 2019. [Google Scholar]
- Shelton, J. The Role of Observation and Simplicity in Einstein’s Epistemology. Stud. Hist. Philos. Sci. 1988, 19, 103–118. [Google Scholar] [CrossRef]
- Macdonald, D.D. The Anthropogenic Global Warming Hypothesis and the Causality Principle. J. Miner. Mater. Sci 2023, 4, 1052. [Google Scholar]
- Macdonald, D.D.; Sikora, E.; Sikora, J. The Point Defect Model vs. the High Field Model for Describing the Growth of Passive Films. In Proceedings of the 7th International Symposium on Oxide Films on Metals and Alloys VII, Miami Beach, FL, USA, 9–14 October 1994; Herbert, K.R., Thompson, G.E., Eds.; The Electrochemical Society: Pennington, NJ, USA, 1994; Volume 94-25, pp. 139–151. [Google Scholar]
- Gamow, S.G. My Worldline; Viking Press: New York, NY, USA, 1970; p. 44. [Google Scholar]
- Casti, J.L. Complexity. Encyclopedia Britannica. 15 December 2021. Available online: https://www.britannica.com/science/complexity-scientific-theory (accessed on 23 February 2023).
- Evans, U.R.; Bannister, L.C.; Britton, S.C. The Velocity of Corrosion from the Electrochemical Standpoint. Proc. Roy Soc. Lond. Ser. A 1931, 131, 355–375. [Google Scholar]
- US Nuclear Regulatory Commission. Report of the US Nuclear Regulatory Commission Piping Review Committee. Volume 1. Investigation and Evaluation of Stress Corrosion Cracking in Piping of Boiling Water Reactor Plants; US Nuclear Regulatory Commission (NRC): Washington, DC, USA, 1984. [CrossRef] [Green Version]
- Shi, J.; Fekete, B.; Wang, J.; Macdonald, D.D. Customization of the coupled environment fracture model for predicting stress corrosion cracking in Alloy 600 in PWR environment. Corros. Sci. 2018, 139, 58–67. [Google Scholar] [CrossRef]
- Fekete, B.; Ai, J.; Yang, J.; Han, J.S.; Maeng, W.Y.; Macdonald, D.D. An advanced coupled environment fracture model for hydrogen-induced cracking in alloy 600 in PWR primary heat transport environment. Theor. Appl. Fract. Mech. 2018, 95, 233–241. [Google Scholar] [CrossRef]
- Lee, S.-K.; Lv, P.; Macdonald, D.D. Customization of the CEFM for Predicting Stress Corrosion Cracking in Lightly Sensitized Al-Mg alloys in Marine Applications. J. Solid State Electrochem. 2013, 17, 2319–2332. [Google Scholar] [CrossRef]
- Maeng, W.Y.; Macdonald, D.D. The effect of acetic acid on the stress corrosion cracking of 3.5NiCrMoV turbine sheets of steel in high-temperature water. Corros. Sci. 2008, 50, 2239–2250. [Google Scholar] [CrossRef]
- Macdonald, D.D.; Yang, J.; Fekete, B.; Balachov, I.; Spencer, B.W. Development and Integration of Light Water Reactor (LWR) Materials Corrosion Degradation Codes into Grizzly; Final Report, DOE/NEUP, Award DE-NE0008541; Idaho National Laboratory: Idaho Falls, ID, USA, 2019. [Google Scholar]
- Zhang, Z.; Wu, X.; Tan, J. In-situ monitoring of stress corrosion cracking of 304 stainless steel in high-temperature water by analyzing acoustic emission waveform. Corros. Sci. 2019, 146, 90–98. [Google Scholar] [CrossRef]
- Scott, P.M. An Overview of Internal Oxidation as a Possible Explanation of Intergranular Stress Corrosion Cracking of Alloy 600 In PWRs. In Proceedings of the Ninth International Symposium on Environmental Degradation of Materials in Nuclear Power Systems—Water Reactors, Newport Beach, CA, USA, 1–5 August 1999; Ford, P.P., Bruemmer, S.M., Was, G.S., Eds.; The Minerals, Metals & Materials Society (TMS): Pittsburgh, PA, USA, 1999. [Google Scholar]
- Ford, F.P.; Taylor, D.F.; Andresen, P.L.; Ballinger, R.G. Corrosion-Assisted Cracking of Stainless and Low-Alloy Steels in LWR Environments; NP-5064M, Final Report; Electric Power Research Institute: Palo Alto, CA, USA, 1987. [Google Scholar]
- Shi, J.; Wang, J.; Macdonald, D.D. Prediction of crack growth rate in Type 304 stainless steel using artificial neural networks and the coupled environment fracture model. Corros. Sci. 2014, 89, 69–80. [Google Scholar] [CrossRef]
- Shi, J.; Wang, J.; Macdonald, D.D. Prediction of primary water stress corrosion crack growth rates in Alloy 600 using artificial neural networks. Corros. Sci. 2015, 92, 217–227. [Google Scholar] [CrossRef]
- Macdonald, D.D. Viability of Hydrogen Water Chemistry for Protecting In-Vessel Components of Boiling Water Reactors. Corrosion 1992, 48, 194–205. [Google Scholar] [CrossRef]
- Robinson, R.A.; Stokes, R.H. Electrolyte Solutions, 2nd ed.; Dover: Downers Grove, IL, USA, 1980. [Google Scholar]
- Quist, A.S.; Marshall, W.L. Electrical Conductances of Aqueous Sodium Chloride Solutions from 0 to 800° and at Pressures to 4000 bar. J. Phys. Chem. 1968, 72, 684–703. [Google Scholar] [CrossRef]
- Gerberich, W.W.; Jones, R.H.; Friesel, M.A.; Nozue, A. Acoustic Emission Monitoring of Stress Corrosion Cracking. Mat. Sci. Eng. A 1988, 103, 185–191. [Google Scholar] [CrossRef]
- Xu, J.; Han, E.-H.; Wu, X. Acoustic emission response of 304 stainless steel during constant load test in high-temperature aqueous environment. Corros. Sci. 2012, 63, 91–99. [Google Scholar] [CrossRef]
- Manahan, M.P.; Macdonald, D.D.; Peterson, A.J., Jr. Determination of the Fate of the Current in the Stress-Corrosion Cracking of Sensitized Type 304SS in High-Temperature Aqueous Systems. Corros. Sci. 1995, 37, 189–208. [Google Scholar] [CrossRef]
- Stewart, J.; Wells, D.B.; Scott, P.M.; Williams, D.E. Electrochemical noise measurements of stress corrosion cracking of sensitized austenitic stainless steel in high-purity oxygenated water at 288 °C. Corros. Sci. 1992, 33, 73–88. [Google Scholar] [CrossRef]
- Lee, S.-K.; Kuang, W.; Mathews, J.A.; Macdonald, D.D. Coupling Current Monitoring of Crevice Corrosion: Part I, Detecting Crevice Activation, Inversion, and Inhibition. Power Plant Chem. 2013, 15, 240–250. [Google Scholar]
- Lee, S.-K.; Kuang, W.; Mathews, J.A.; Macdonald, D.D. Determining the Coupling Current as a Means of Detecting Crevice Activation and Inhibition. ECS Trans. 2013, 50, 69–78. [Google Scholar] [CrossRef]
- Kovalov, D.; Ghanbari, E.; Mao, F.; Kursten, B.; Macdonald, D.D. Investigation of artificial pit growth in carbon steel in highly alkaline solutions containing 0.5 M NaCl under oxic and anoxic conditions. Electrochim. Acta 2019, 320, 134554. [Google Scholar] [CrossRef] [Green Version]
- Bao, J.; Macdonald, D.D. Hydrogen Oxidation on Oxidized Platinum at Elevated Temperatures, Part I: The Tunneling Current. J. Electroanal. Chem. 2007, 600, 205–216. [Google Scholar] [CrossRef]
- Qiu, J.; Macdonald, D.D.; Xu, Y.; Sun, L. General corrosion of carbon steel in a synthetic concrete pore solution. Mater. Corros. 2020, 72, 107–119. [Google Scholar] [CrossRef]
- Sutanto, F.; Macdonald, D.D. On the Role of Quantum Mechanics in Corrosion Processes. In Proceedings of the Australian Corrosion Conference, Auckland, New Zealand, 13–18 November 2016. Paper 118. [Google Scholar]
- Sun, X.; Xu, J.; Li, Y. Hydrogen Permeation Behaviour in Austenitic Stainless Steels. Mater. Sci. Eng. 1989, 114, 179–187. [Google Scholar] [CrossRef]
- Gomez-Duran, M.; Macdonald, D.D. Stress corrosion cracking of sensitized Type 304 stainless steel in thiosulfate solution: I. Fate of the coupling current. Corros. Sci. 2003, 45, 1455–1471. [Google Scholar] [CrossRef]
- Jones, R.H.; Ricker, R.E. Mechanisms of Stress Corrosion Cracking. In Stress-Corrosion Cracking Materials Performance and Evaluation; Jones, R.H., Ed.; ASTM International: West Conshohocken, PA, USA, 1992; pp. 1–40. [Google Scholar]
- Wuensche, A.; Macdonald, D.D. Relationship between the Crack Growth Rate and the Coupling Current for IGSCC in Sensitized Type 304 SS in High Temperature Water. 2005; unpublished data. [Google Scholar]
- Zhou, X.Y.; Balachov, I.I.; Macdonald, D.D. The Effect of Dielectric Coatings on IGSCC in Sensitized Type 304 SS in High-Temperature Dilute Sodium Sulfate Solution. Corros. Sci. 1998, 40, 1349–1362. [Google Scholar] [CrossRef]
- Kwon, H.-S.; Wuensche, A.; Macdonald, D.D. Effect of Flow Rate on Crack Growth in Sensitized Type 304 Stainless Steel in High-Temperature Solutions. Corrosion 2000, 56, 482–491. [Google Scholar] [CrossRef]
- Andresen, P.L.; Seeman, R.A. Effects of temperature and corrosion potential on SCC. In Proceedings of the 15th International Conference on Environmental Degradation of Materials in Nuclear Power Systems—Water Reactors, Colorado Springs, CO, USA, 7–11 August 2011; Springer: Cham, Switzerland, 2016; pp. 849–882. [Google Scholar]
- Lee, S.K.; Macdonald, D.D. Theoretical aspects of stress corrosion cracking of Alloy 22. J. Nucl. Mater. 2018, 503, 124–139. [Google Scholar] [CrossRef]
- Shoji, T.; Lu, Z.; Murakami, H. Formulating stress corrosion cracking growth rates by combination of crack tip mechanics and crack tip oxidation kinetics. Corros. Sci. 2010, 52, 769–779. [Google Scholar] [CrossRef]
- Yamazaki, S.; Lu, Z.; Ito, Y.; Takeda, Y.; Shoji, T. The effect of prior deformation on stress corrosion cracking growth rates of Alloy 600 materials in a simulated pressurized water reactor primary water. Corros. Sci. 2008, 50, 835–846. [Google Scholar] [CrossRef]
- Hou, J.; Peng, Q.J.; Shoji, T.; Wang, J.Q.; Han, E.-H.; Ke, W. Effects of cold working path on strain concentration, grain boundary microstructure and stress corrosion cracking in Alloy 600. Corros. Sci. 2011, 53, 2956–2962. [Google Scholar] [CrossRef]
- Engelhardt, G.R.; Urquidi-Macdonald, M.; Macdonald, D.D. A Simplified Method for Estimating Corrosion Cavity Growth Rates. Corros. Sci. 1997, 39, 419–441. [Google Scholar] [CrossRef]
- Engelhardt, G.R.; Macdonald, D.D.; Urquidi-Macdonald, M. Development of fast algorithms for estimating stress corrosion crack growth rate. Corros. Sci. 1999, 41, 2267–2302. [Google Scholar] [CrossRef]
- Macdonald, D.D. The Point Defect Model for the Passive State. J. Electrochem. Soc. 1992, 139, 3434–3449. [Google Scholar] [CrossRef]
- Macdonald, D.D. The History of the Point Defect Model for the Passive State: A Brief Review of Film Growth Aspects. Electrochim. Acta 2011, 56, 1761–1772. [Google Scholar] [CrossRef]
- Congleton, J.; Shoji, T.; Parkins, R.N. The stress corrosion cracking of reactor pressure vessel steel in high temperature water. Corros. Sci. 1985, 25, 633–650. [Google Scholar] [CrossRef]
- Hall, M.M. An alternative to the Shoji crack tip strain rate equation. Corros. Sci. 2008, 50, 2902–2905. [Google Scholar] [CrossRef]
- Wilkerson, D.S.; Vitek, V. The propagation of cracks by cavitation: A general theory. Acta Metall. 1982, 30, 1723–1732. [Google Scholar] [CrossRef]
- Sharland, S.M.; Tasker, P.W. A Mathematical Model of Crevice and Pitting Corrosion—I. The Physical Model. Corros. Sci. 1988, 28, 603–620. [Google Scholar] [CrossRef]
- Que, Z.; Saario, T.; Toivonen, A.; Ehrnsten, U. Stress corrosion cracking initiation susceptibility of Alloy 182 with different surface treatments. Corros. Sci. 2022, 196, 110037. [Google Scholar] [CrossRef]
- Macdonald, D.D.; Balachov, I.; Engelhardt, G. Deterministic prediction of localized corrosion damage in power plant coolant circuits. Power Plant Chem. 1999, 1, 9–16. [Google Scholar]
- Balachov, I.; Engelhardt, G.R.; Macdonald, D.D. The Electrochemistry of Stress Corrosion Cracking—From Theory to Damage Prediction in Practical Systems. In Environment-Induced Cracking of Materials; Volume 2: Prediction, Industrial Development and Evaluation; Elsevier: Amsterdam, The Netherlands, 2008; pp. 55–92. [Google Scholar]
- Haugen, R.L.; Dhanak, A.M. Momentum transfer in turbulent separated flow past a rectangular cavity. J. Appl. Mech. 1966, 33, 641–646. [Google Scholar] [CrossRef]
- Li, Z.; Jiang, X.; Hopman, H.; Zhu, L.; Liu, Z. An investigation on the circumferential surface crack growth in steel pipes subjected to fatigue bending. Theor. Appl. Fract. Mech. 2020, 105, 102403. [Google Scholar] [CrossRef]
- Scott, P.M.; Thorpe, T.W. A Critical Review of Crack Tip Stress Intensity Factors for Semi-Elliptic Cracks. Fatigue Fract. Eng. Mater. Struct. 1981, 4, 291–309. [Google Scholar] [CrossRef]
- Parks, D. A Surface Crack Review: Elastic and Elastic-Plastic Behavior. In Surface-Crack Growth: Models, Experiments, and Structures; ASTM International: West Conshohocken, PA, USA, 2009; p. 9. [Google Scholar]
- James, L.A.; Schwenk, E.B., Jr. Fatigue-Crack Propagation Behavior of Type 304 Stainless Steel at Elevated Temperatures. Metall. Trans. 1971, 2, 491. [Google Scholar] [CrossRef]
- Lee, S.-K.; Kramer, D.; Macdonald, D.D. On the shape of stress corrosion cracks in water-cooled nuclear power reactor piping. ESC Trans. 2013, 50, 27. [Google Scholar] [CrossRef]
- Lee, S.-K.; Kramer, D.; Macdonald, D.D. On the Shape of Stress Corrosion Cracks in Sensitized Type 304 SS in Boiling Water Reactor Primary Coolant Piping at 288 °C. J. Nucl. Mater. 2014, 454, 359–372. [Google Scholar] [CrossRef]
- Tang, J.-R.; Kao, L.; Shiau, D.-Y.; Yao, C.-C.; Chung, S.-C. Thermal-hydraulic analysis of core shroud crack for Chinshan BWR/4 unit 2 using RETRAN-02/MOD5. Nucl. Technol. 1998, 121, 324. [Google Scholar] [CrossRef]























| Independent Variable | Range | Type 304 SS in BWR Primary Coolant | Range | Alloy 600 in PWR Primary Coolant |
|---|---|---|---|---|
| Temperature (°C) | 25–292 | 17.8 | 290–360 | 18.6 |
| ECP (Vshe) | −0.575–0.496 | 43.6 | −1.096 to −0.610 | 14.1 |
| Stress Intensity Factor (MPa∙√m) | 10.4–67.78 | 10.8 | 4.6–101 | 15.2 |
| Conductivity (μS/cm) | 0.52–5.72 | 14.0 | 1.7–116 | 14.1 |
| Degree of Sensitization (DoS) (C/cm2) | 0–33.79 | 13.8 | - | - |
| Yield Strength (MPa) | N/I | - | 211–500 | 12.0 |
| [LiOH] ppm | N/A | - | 0–10 | 4.0 |
| [H3BO3] ppm | N/A | - | 0–1800 | 7.6 |
| pH | N/A | - | 5.52–9.19 | 14.5 |
| Parameter | Value | Comments |
|---|---|---|
| T | 288 °C | Operating temperature of a BWR. |
| COD | 0.001 cm | Typical of a tight crack |
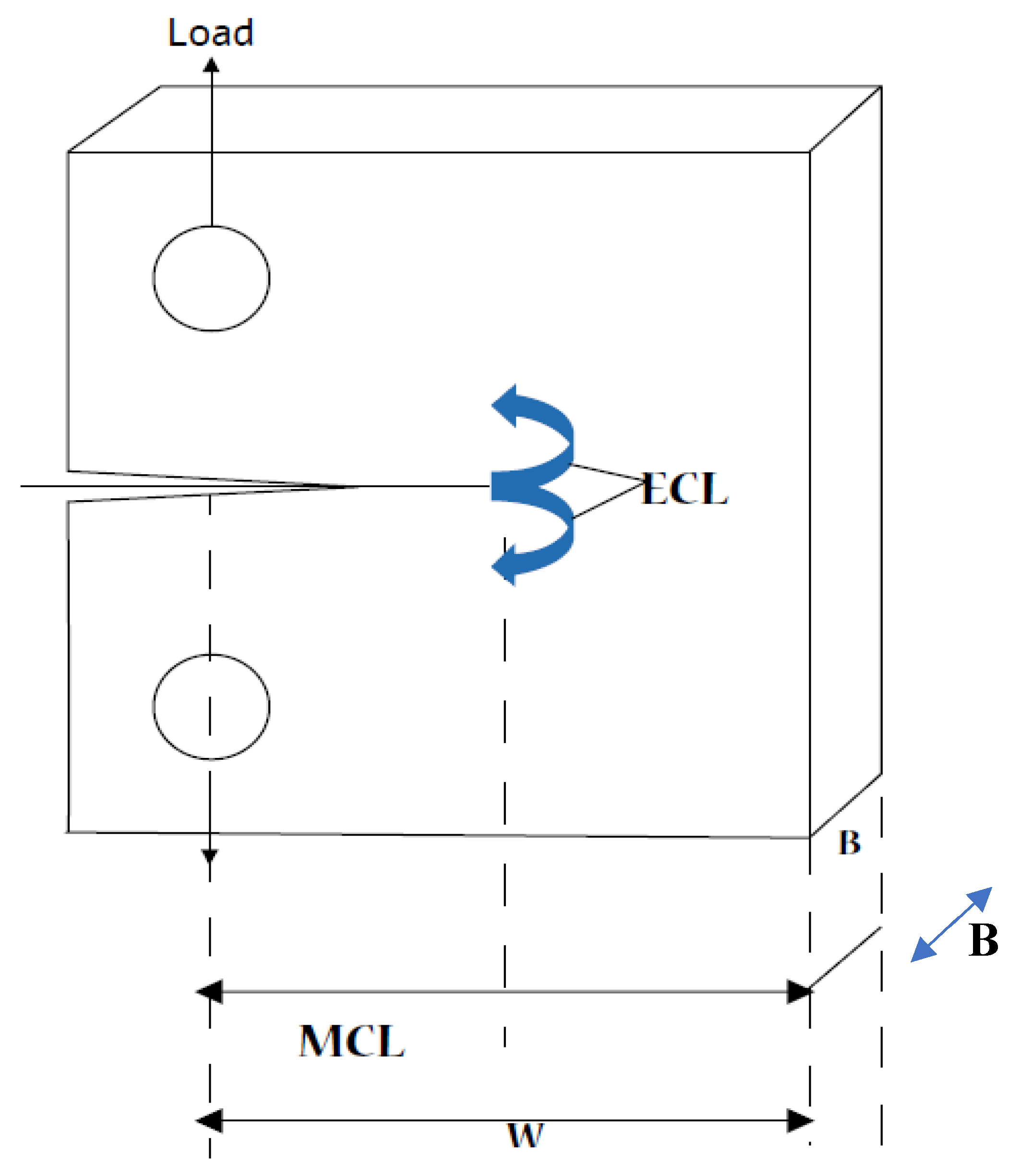
| Crack width | 1.0 cm | Assumed |
| Crack length | 0.5 cm | Assumed for a “standard crack” |
| Pipe hydrodynamic diameter | 50 cm | Typical of BWR recirculation system |
| Flow velocity | 100 cm/s | Assumed |
| Stress intensity factor | 27.5 MPa.m1/2 | Assumed |
| O2 concentration | 100 ppb | Typical of BWR under Normal Water Chemistry (NWC) |
| H2 concentration | 1 ppb | Assumed |
| H2O2 concentration | 1 ppb | Assumed |
| Degree of sensitization (EPR) | 15 C/cm2 | Typical of weld sensitization of Type 304 |
| Atomic volume | 1.18 × 10−23 cm3 | Fundamental |
| Fracture strain at the crack tip | 8 × 10−4 | Assumed |
| Young’s Modulus (E) | 2 × 105 MPa | Typical of Type 304 SS |
| Dimensionless constant (β) | 5.08 | Refs. [61,62,63] |
| Density of the steel (ρ) | 8 g/cm3 | Typical of Type 304 SS |
| Yield strength () | 215 MPa | Typical of Type 304 SS |
| Hwang–Gao strain hardening exponent () | 1.7 | Refs. [61,62,63] |
| Ramberg–Osgood strain hardening exponent () | xxx | Refs. [61,62,63] |
| Dimensionless constant (λ) | 0.11 | Refs. [61,62,63] |
| Shear modulus (G) | 7.31 × 1010 Pa | Typical of Type 304 SS |
| Grain boundary self-diffusion constant (Db0) | 2.5 × 10−4 m2/s | Typical of stainless steels |
| Activation energy for diffusion (Ea,D) | 168 kJ/mol | Refs. [61,62,63] |
| Grain boundary diffusion width | 5 × 10−10 m | Typical of stainless steels |
| Tafel constant for the HER | 0.065/V | Typical of Type 304 SS [40] |
| Exchange current density (i0) for HER | 5 × 10−4 A/cm2 | Typical of Type 304 SS [40] |
| Tafel constant for the OER | 0.071/V | Typical of Type 304 SS [40] |
| Exchange current density (i0) for OER | 5.05 × 10−3 A/cm2 | Typical of Type 304 SS [40] |
| Passive current density for the steel | 2.6 × 10−3 A/cm2 | Typical of Type 304 SS [40] |
| Standard potential (E0) for steel electrodissolution at the crack tip. | −0.47 Vshe | Calculated from thermodynamics for Fe2+/Fe |
| Model | Equation |
|---|---|
| Ford [37] | |
| Congelton [68] | |
| Shoji [61,62,63] | |
| Hall [69] | |
| Temperature Dependence |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Macdonald, D.D. The Role of Determinism in the Prediction of Corrosion Damage. Corros. Mater. Degrad. 2023, 4, 212-273. https://doi.org/10.3390/cmd4020013
Macdonald DD. The Role of Determinism in the Prediction of Corrosion Damage. Corrosion and Materials Degradation. 2023; 4(2):212-273. https://doi.org/10.3390/cmd4020013
Chicago/Turabian StyleMacdonald, Digby D. 2023. "The Role of Determinism in the Prediction of Corrosion Damage" Corrosion and Materials Degradation 4, no. 2: 212-273. https://doi.org/10.3390/cmd4020013
APA StyleMacdonald, D. D. (2023). The Role of Determinism in the Prediction of Corrosion Damage. Corrosion and Materials Degradation, 4(2), 212-273. https://doi.org/10.3390/cmd4020013






