Is Government Spending an Important Factor in Economic Growth? Nonlinear Cubic Quantile Nexus from Eastern Europe and Central Asia (EECA)
Abstract
1. Introduction
2. Theoretical Background and Literature Review
2.1. Brief Theoretical Background
2.2. Literature Review
2.2.1. Nexus between Government Spending and Economic Growth
2.2.2. Nexus between Macro-Governance Indicators and Economic Growth
2.2.3. Nexus between Other Macroeconomic Indicators and Economic Growth
3. Materials and Methods
3.1. Methodology
3.2. Variables Selection
3.2.1. Dependent Variable: Economic Growth
3.2.2. Independent Variable: Government Spending
3.2.3. Independent Variable: Macroeconomic Indicators
3.2.4. Independent Variable: Macro-Governance Indicators
3.3. Data
4. Results
4.1. Relation between Government Spending and Economic Growth
4.1.1. Linear Relation
4.1.2. Nonlinear Quadratic Relation
4.1.3. Nonlinear Cubic Relation
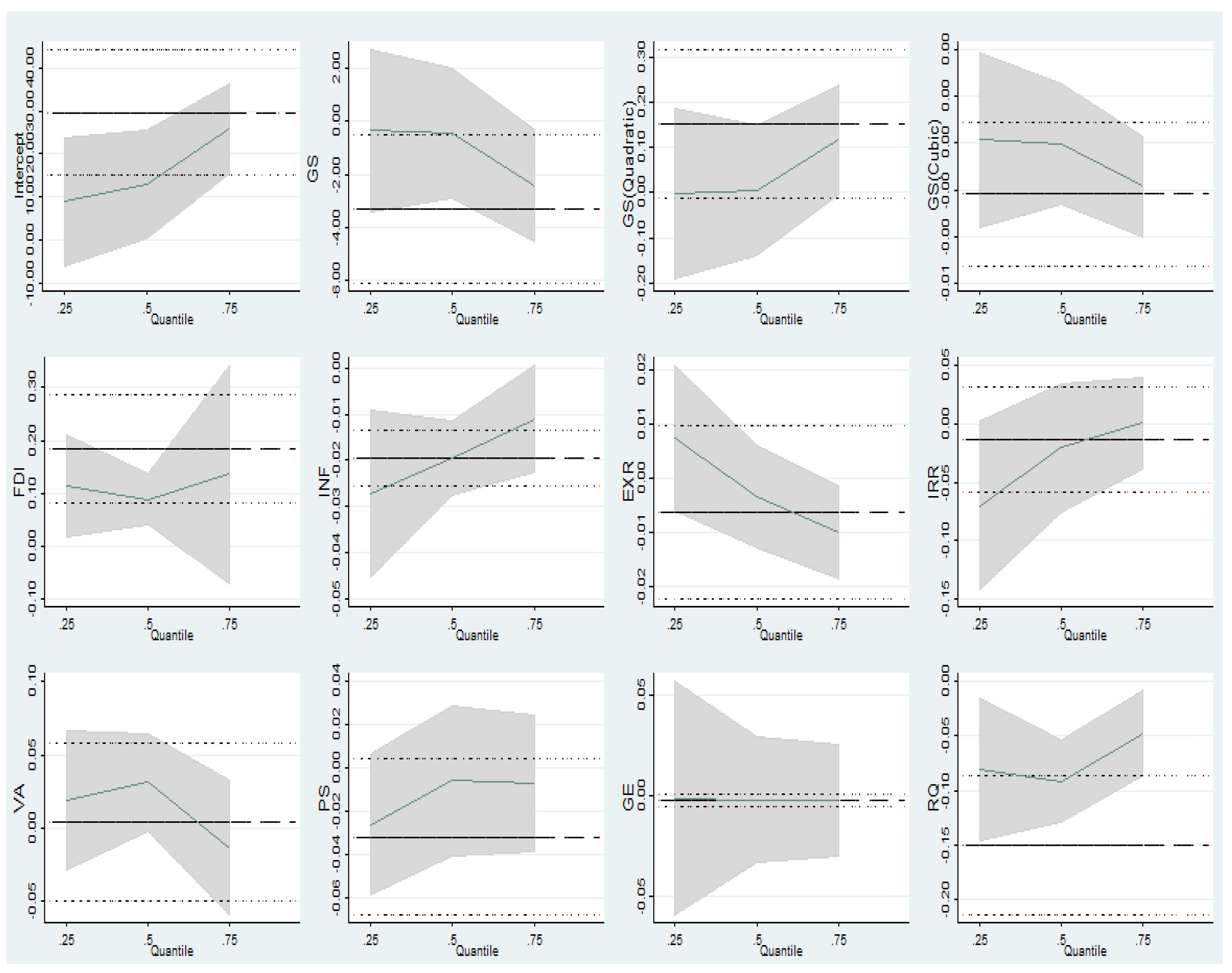
4.2. Relation between Macro-Economic and Macro-Governance Indicators and Economic Growth
4.3. Robustness Checks: The Relation between Government Spending and Economic Growth for Grouped Variables
4.4. Robustness Checks: Generalized Method of Moments, Fixed Effect, and Instrumental Variable (Fitted Values)
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
| 1 | According to an OECD iLibrary report “… this region has long been at a global crossroads—at the intersection of diverse cultures, trade routes and relations, political systems.” Thus, many international organisations have considered EECA countries as one panel that share educational, health, economic and political issues; see World Bank, OECD, World Health Organization. |
| 2 | See World Bank Country and Lending Groups report. |
| 3 | −16.7% in Tajikistan in 1996 during the Tajikistani Civil War; 22.96% in Bosnia in 1996, perhaps because of a boom after the Bosnia War (1992–1995). |
| 4 | Government spending was 6% in Turkmenistan, a desert country, in 1996, before oil and gas exploitation, and it was 32% in Uzbekistan in 2015, associated with high government revenues from oil, gas, and gold. |
| 5 | Nearly all countries in the EECA recorded high inflation levels, particularly in the mid-1990s. |
| 6 | A financial crisis led to Bulgaria’s hyperinflation in 1997 (Charles and Marie 2017). |
| 7 | To assess whether certain high values may influence the average. |
| 8 | Many Eastern European nationals work in EU countries, and a considerable number lost their employment and returned home during the EU debt crisis and other crises (Esposito et al. 2014). |
| 9 | Central Asian countries are the main oil and gas producers in the region. |
| 10 | Most Eastern Europe countries’ average public debt increased from 26% at the end of 2007 to 54% at the end of 2020. However, there is still variation from one country to another (Semik and Zimmermann 2021). |
| 11 | A correlation coefficient exceeding (0.7) indicates a potential problem (Anderson et al. 2016). |
| 12 | The fight against inflation continues throughout Central and Eastern Europe. ING. |
| 13 | The average FDI did not exceed 5%; see Table 2. |
References
- Afonso, António, and João Tovar Jalles. 2014. Causality for the government budget and economic growth. Applied Economics Letters 21: 1198–201. [Google Scholar] [CrossRef]
- Afonso, António, and Ricardo M. Sousa. 2011. The macroeconomic effects of fiscal policy in Portugal: A Bayesian SVAR analysis. Portuguese Economic Journal 10: 61–82. [Google Scholar] [CrossRef]
- Agell, Jonas, Thomas Lindh, and Henry Ohlsson. 1997. Growth and the public sector: A critical review essay. European Journal of Political Economy 13: 33–52. [Google Scholar] [CrossRef]
- Aizenman, Joshua, Yothin Jinjarak, Kim Thi Kim Nguyen, and Donghyun Park. 2019. Fiscal space and government-spending and tax-rate cyclicality patterns: A cross-country comparison, 1960–2016. Journal of Macroeconomics 60: 229–52. [Google Scholar] [CrossRef]
- Akpan, Usenobong F., and Dominic E. Abang. 2013. Does government spending spur economic growth? Evidence from Nigeria. Journal of Economics and Sustainable Development 4: 36–52. [Google Scholar]
- Allard, Alexandra, Johanna Takman, Gazi Salah Uddin, and Ali Ahmed. 2018. The N-shaped environmental Kuznets curve: An empirical evaluation using a panel quantile regression approach. Environmental Science and Pollution Research 25: 5848–5861. [Google Scholar] [CrossRef]
- Alnori, Faisal. 2020. Cash holdings: Do they boost or hurt firms’ performance? Evidence from listed non-financial firms in Saudi Arabia. International Journal of Islamic and Middle Eastern Finance and Management. ahead of print. [Google Scholar] [CrossRef]
- Alshahrani, Saad, and Ali Alsadiq. 2014. Economic Growth and Government Spending in Saudi Arabia: An Empirical Investigation. Washington, DC: International Monetary Fund. [Google Scholar]
- Altunc, O. Faruk, and Celil Aydın. 2013. The relationship between optimal size of government and economic growth: Empirical evidence from Turkey, Romania and Bulgaria. Procedia-Social and Behavioral Sciences 92: 66–75. [Google Scholar] [CrossRef]
- Anderson, David Dennis, Dennis Sweeney, Thomas Williams, Jeffery Camm, and James Cochran. 2016. Statistics for Business & Economics. London: Cengage Learning. [Google Scholar]
- Arpaia, Alfonso, and Alessandro Turrini. 2007. Government Expenditure and Economic Growth in the EU: Long-Run Tendencies and Short-Term Adjustment. Available online: https://ssrn.com/abstract=2004461 (accessed on 24 August 2022).
- Asimakopoulos, Stylianos, and Yiannis Karavias. 2016. The impact of government size on economic growth: A threshold analysis. Economics Letters 139: 65–68. [Google Scholar] [CrossRef]
- Asongu, Simplice, and Nicholas Odhiambo. 2020. Foreign direct investment, information technology and economic growth dynamics in Sub-Saharan Africa. Telecommunications Policy 44: 101838. [Google Scholar] [CrossRef]
- Atems, Bebonchu. 2019. The effects of government spending shocks: Evidence from US states. Regional Science and Urban Economics 74: 65–80. [Google Scholar] [CrossRef]
- Auerbach, Alan, and Yuriy Gorodnichenko. 2012. Measuring the output responses to fiscal policy. American Economic Journal: Economic Policy 4: 1–27. [Google Scholar] [CrossRef]
- Aydin, Celil, Merter Akinci, and Ömer Yilmaz. 2016. The analysis of visible hand of government: The threshold effect of government spending on economic growth. International Journal of Trade, Economics and Finance 7: 170. [Google Scholar] [CrossRef]
- Barro, Robert. 1990. Government spending in a simple model of endogeneous growth. Journal of Political Economy 98: S103–S125. [Google Scholar] [CrossRef]
- Becker, Gary S., Kevin M. Murphy, and Robert Tamura. 1990. Human capital, fertility, and economic growth. Journal of Political Economy 98: S12–S37. [Google Scholar] [CrossRef]
- Beckmann, Joscha, Marek Endrichs, and Rainer Schweickert. 2016. Government activity and economic growth–one size fits all? International Economics and Economic Policy 13: 429–50. [Google Scholar] [CrossRef][Green Version]
- Bökemeier, Bettina, and Andreea Stoian. 2018. Debt sustainability issues in central and East European countries. Eastern European Economics 56: 438–70. [Google Scholar] [CrossRef]
- Cade, Brian, and Barry R. Noon. 2003. A gentle introduction to quantile regression for ecologists. Frontiers in Ecology and the Environment 1: 412–20. [Google Scholar] [CrossRef]
- Cass, David. 1965. Optimum growth in an aggregative model of capital accumulation. The Review of Economic Studies 32: 233–40. [Google Scholar] [CrossRef]
- Charles, Sébastien, and Jonathan Marie. 2017. Bulgaria’s hyperinflation in 1997: Transition, banking fragility and foreign exchange. Post-Communist Economies 29: 313–35. [Google Scholar] [CrossRef]
- Chen, Sheng-Tung, and Chien-Chiang Lee. 2005. Government size and economic growth in Taiwan: A threshold regression approach. Journal of Policy Modeling 27: 1051–66. [Google Scholar] [CrossRef]
- Chen, Shyh-Wei. 2014. Testing for fiscal sustainability: New evidence from the G-7 and some European countries. Economic Modelling 37: 1–15. [Google Scholar] [CrossRef]
- Chen, Yong, and Dingming Liu. 2018. Government spending shocks and the real exchange rate in China: Evidence from a sign-restricted VAR model. Economic Modelling 68: 543–54. [Google Scholar] [CrossRef]
- Chiou-Wei, Song-Zan, Zhen Zhu, and Yung-Hsing Kuo. 2010. Government size and economic growth: An application of the smooth transition regression model. Applied Economics Letters 17: 1405–415. [Google Scholar] [CrossRef]
- Christie, Tamoya. 2014. The effect of government spending on economic growth: Testing the non-linear hypothesis. Bulletin of Economic Research 66: 183–204. [Google Scholar] [CrossRef]
- Combes, Jean-Louis, Alexandru Minea, and Moussé Sow. 2017. Is fiscal policy always counter- (pro-) cyclical? The role of public debt and fiscal rules. Economic Modelling 65: 138–46. [Google Scholar] [CrossRef]
- De Groot, Henri, Gert Jan Linders, Piet Rietveld, and Uma Subramanian. 2004. The institutional determinants of bilateral trade patterns. Kyklos 57: 103–23. [Google Scholar] [CrossRef]
- Di Serio, Mario, Matteo Fragetta, and Emanuel Gasteiger. 2020. The government spending multiplier at the zero lower bound: Evidence from the United States. Oxford Bulletin of Economics and Statistics 82: 1262–294. [Google Scholar] [CrossRef]
- Divino, Jose Angelo, Daniel T. G. N. Maciel, and Wilfredo Sosa. 2020. Government size, composition of public spending and economic growth in Brazil. Economic Modelling 91: 155–66. [Google Scholar] [CrossRef]
- Dollar, David, and Aart Kraay. 2002. Growth is good for the poor. Journal of Economic Growth 7: 195–225. [Google Scholar] [CrossRef]
- Erdoğan, Seyfettin, Durmuş Çağrı Yıldırım, and Ayfer Gedikli. 2020. Natural resource abundance, financial development and economic growth: An investigation on Next-11 countries. Resources Policy 65: 101559. [Google Scholar] [CrossRef]
- Esposito, Mark, Chatzimarkakis Jorgo, Tse Terence, Dimitriou Giorgos, Akiyoshi Rieko, Eswara Balusu, and Arianna Valezano. 2014. The European Financial Crisis: Analysis and a Novel Intervention. Cambridge: Harvard University Press. [Google Scholar]
- Fayissa, Bichaka, and Christian Nsiah. 2013. The impact of governance on economic growth in Africa. The Journal of Developing Areas 47: 91–108. [Google Scholar] [CrossRef]
- Fedderke, Johannes W., Peter Perkins, and John Manuel Luiz. 2006. Infrastructural investment in long-run economic growth: South Africa 1875–2001. World Development 34: 1037–59. [Google Scholar] [CrossRef]
- Ferreira, João J. M., Cristina Fernandes, and Fernando Ferreira. 2020. Technology transfer, climate change mitigation, and environmental patent impact on sustainability and economic growth: A comparison of European countries. Technological Forecasting and Social Change 150: 119770. [Google Scholar] [CrossRef]
- Forte, Francesco, and Cosimo Magazzino. 2016. Government size and economic growth in Italy: A time-series analysis. European Scientific Journal 12. [Google Scholar] [CrossRef]
- Gan, Li, Susan Sunila Sharma, and Zhichao Yin. 2020. Editorial of applied finance, macroeconomic performance and economic growth. Economic Modelling 86: 239–40. [Google Scholar] [CrossRef]
- Gemmell, Norman, and Joey Au. 2013. Do smaller governments raise the level or growth of output? A review of recent evidence. Review of Economics 64: 85–116. [Google Scholar] [CrossRef]
- Ghali, Khalifa H. 1999. Government size and economic growth: Evidence from a multivariate cointegration analysis. Applied Economics 31: 975–87. [Google Scholar] [CrossRef]
- Ghirmay, Teame. 2004. Financial development and economic growth in Sub-Saharan African countries: Evidence from time series analysis. African Development Review 16: 415–32. [Google Scholar] [CrossRef]
- Ghose, Arpita, and Sutapa Das. 2013. Government size and economic growth in emerging market economies: A panel co-integration approach. Macroeconomics and Finance in Emerging Market Economies 6: 14–38. [Google Scholar] [CrossRef]
- Ghosh, Atish, Jun Kim Enrique, Mendoza Jonathan Ostry, and Mahvash Qureshi. 2013. Fiscal fatigue, fiscal space and debt sustainability in advanced economies. The Economic Journal 123: F4–F30. [Google Scholar] [CrossRef]
- Gray, Cheryl, Tracey Lane, and Aristomene Varoudakis. 2007. Fiscal Policy and Economic Growth: Lessons for Eastern Europe and Central Asia. Washington, DC: World Bank Publications. [Google Scholar]
- Grindle, Merilee. 2004. Good enough governance: Poverty reduction and reform in developing countries. Governance 17: 525–48. [Google Scholar] [CrossRef]
- Haberler, Gottfried, and Joseph T. Salerno. 2017. Prosperity and Depression: A Theoretical Analysis of Cyclical Movements. London: Routledge. [Google Scholar]
- Hajamini, Mehdi, and Mohammad Ali Falahi. 2018. Economic growth and government size in developed European countries: A panel threshold approach. Economic Analysis and Policy 58: 1–13. [Google Scholar] [CrossRef]
- Halkos, George, and Epameinondas Α. Paizanos. 2013. The effect of government expenditure on the environment: An empirical investigation. Ecological Economics 91: 48–56. [Google Scholar] [CrossRef]
- Heitger, Bernhard. 2001. The Scope of Government and its Impact on Economic Growth in OECD Countries. Kiel Working Papers 1034. Kiel: Kiel Institute for the World Economy. [Google Scholar]
- Herath, Shanaka. 2012. Size of government and economic growth: A nonlinear analysis. Economic Annals 57: 7–30. [Google Scholar] [CrossRef]
- Huang, Choung-Ju, and Yuan-Hong Ho. 2017. Governance and economic growth in Asia. The North American Journal of Economics and Finance 39: 260–72. [Google Scholar] [CrossRef]
- Hübler, Michael. 2017. The inequality-emissions nexus in the context of trade and development: A quantile regression approach. Ecological Economics 134: 174–85. [Google Scholar] [CrossRef]
- Hung, Fu-Sheng, and Chien-Chiang Lee. 2010. Asymmetric information, government fiscal policies, and financial development. Economic Development Quarterly 24: 60–73. [Google Scholar] [CrossRef]
- Huynh, Kim, and David Jacho-Chávez. 2009. A nonparametric quantile analysis of growth and governance. In Nonparametric Econometric Methods. London: Emerald Group Publishing Limited. [Google Scholar] [CrossRef]
- Iyidogan, Pelin Varol, and Taner Turan. 2017. Government size and economic growth in Turkey: A threshold regression analysis. Prague Economic Papers, 142–54. [Google Scholar]
- Jalilian, Hossein, Colin Kirkpatrick, and David Parker. 2007. The impact of regulation on economic growth in developing countries: A cross-country analysis. World Development 35: 87–103. [Google Scholar] [CrossRef]
- Kaufmann, Daniel, Aart Kraay, and Pablo Zoido. 1999. Governance Matters. Available online: https://ssrn.com/abstract=188568 (accessed on 24 August 2022).
- Kim, Dong-Hyeon, Yi-Chen Wu, and Shu-Cin Lin. 2018. Heterogeneity in the effects of government size and governance on economic growth. Economic Modelling 68: 205–16. [Google Scholar] [CrossRef]
- Kimaro, Edmund Lawrence, Choong Chee Keong, and Lau Lin Sea. 2017. Government expenditure, efficiency and economic growth: A panel analysis of Sub-Saharan African low income countries. African Journal of Economic Review 5: 34–54. [Google Scholar]
- Kronborg, Anders. 2021. Estimating Government Spending Shocks in a VAR Model. Danish Research Institute for Economic Analysis and Modelling. Available online: https://dreamgruppen.dk/media/12338/estimating_government_spending_shocks_in_a_var_model.pdf (accessed on 24 August 2022).
- Larch, Mario, and Wolfgang Lechthaler. 2013. Buy national or buy international? The optimal design of government spending in an open economy. International Review of Economics & Finance 26: 87–108. [Google Scholar]
- Linh, Nguyen Thi My, Phan Thi Hang Nga, and Tam Thanh Phan. 2019. The optimal public expenditure decision: A case of economic growth in Southeast Asian countries. Journal of Management Information and Decision Sciences 22: 25–35. [Google Scholar]
- Linnemann, Ludger, and Roland Winkler. 2016. Estimating nonlinear effects of fiscal policy using quantile regression methods. Oxford Economic Papers 68: 1120–145. [Google Scholar] [CrossRef]
- Lucas, Robert, Jr. 1988. On the mechanics of economic development. Journal of Monetary Economics 22: 3–42. [Google Scholar] [CrossRef]
- Magazzino, Cosimo. 2014. Government size and economic growth in Italy: An empirical analyses based on new data (1861–2008). International Journal of Empirical Finance 3: 38–54. [Google Scholar]
- Mandeya, Shelton Masimba Tafadzwa, and Sin-Yu Ho. 2021. Inflation, inflation uncertainty and the economic growth nexus: An impact study of South Africa. MethodsX 8: 101501. [Google Scholar] [CrossRef]
- Mavrov, Hristo. 2007. The size of government expenditure and the rate of economic growth in Bulgaria. Economic Alternatives 1: 53–63. [Google Scholar]
- Méndez-Picazo, María-Teresa, Miguel-Ángel Galindo-Martín, and Domingo Ribeiro-Soriano. 2012. Governance, entrepreneurship and economic growth. Entrepreneurship & Regional Development 24: 865–77. [Google Scholar]
- Miniesy, Rania, and Mariam AbdelKarim. 2021. Generalized trust and economic growth: The nexus in MENA countries. Economies 9: 39. [Google Scholar] [CrossRef]
- Mishchenko, Volodymyr, Svitlana Naumenkova, Svitlana Mishchenko, and Viktor Ivanov. 2018. Inflation and economic growth: The search for a compromise for the Central Bank’s monetary policy. Banks & Bank Systems 13: 153–63. [Google Scholar]
- Miyamoto, Wataru, Thuy Lan Nguyen, and Viacheslav Sheremirov. 2019. The effects of government spending on real exchange rates: Evidence from military spending panel data. Journal of International Economics 116: 144–57. [Google Scholar] [CrossRef]
- Nelson, Michael, and Ram Singh. 1994. The deficit-growth connection: Some recent evidence from developing countries. Economic Development and Cultural Change 43: 167–91. [Google Scholar] [CrossRef]
- Nuru, Naser Yenus, and Hayelom Yrgaw Gereziher. 2021. The effect of fiscal policy on economic growth in South Africa: A nonlinear ARDL model analysis. Journal of Economic and Administrative Sciences. ahead of print. [Google Scholar] [CrossRef]
- Nurudeen, Abu, and Abdullahi Usman. 2010. Government expenditure and economic growth in Nigeria, 1970–2008: A disaggregated analysis. Business and Economics Journal 2010: 1–11. [Google Scholar]
- Odhiambo, Nicholas. 2008. Financial depth, savings and economic growth in Kenya: A dynamic causal linkage. Economic Modelling 25: 704–13. [Google Scholar] [CrossRef]
- Olaoye, Olumide Olusegun, Monica Orisadare, and Ukafor Ukafor Okorie. 2019. Government expenditure and economic growth nexus in ECOWAS countries: A panel VAR approach. Journal of Economic and Administrative Sciences. ahead of print. [Google Scholar] [CrossRef]
- Olaoye, Olumide Oluwatosin, Eluwole Aziz Ayesha, and Olugbenga Afolabi. 2020. Government spending and economic growth in ECOWAS: An asymmetric analysis. The Journal of Economic Asymmetries 22: e00180. [Google Scholar] [CrossRef]
- Olaoye, Olumide, and Oluwatosin Aderajo. 2020. Institutions and economic growth in ECOWAS: An investigation into the hierarchy of institution hypothesis (HIH). International Journal of Social Economics 47: 1081–108. [Google Scholar] [CrossRef]
- Onifade, Stephen Taiwo, Savas Çevik, Savas Erdoğan, Simplice Asongu, and Festus Bekun. 2020. An empirical retrospect of the impacts of government expenditures on economic growth: New evidence from the Nigerian economy. Journal of Economic Structures 9: 1–13. [Google Scholar] [CrossRef]
- Osei, Michael, and Jaebeom Kim. 2020. Foreign direct investment and economic growth: Is more financial development better? Economic Modelling 93: 154–61. [Google Scholar] [CrossRef]
- Paleologou, Suzanna-Maria. 2013. Asymmetries in the revenue–expenditure nexus: A tale of three countries. Economic Modelling 30: 52–60. [Google Scholar] [CrossRef]
- Pevcin, Primoz. 2004. Does optimal size of government spending exist? University of Ljubljana 10: 101–35. [Google Scholar]
- Poznańska, Joanna, and Kazimierz Poznański. 2015. Comparison of patterns of convergence among “emerging markets” of Central Europe, Eastern Europe and Central Asia. Comparative economic research. Central and Eastern Europe 18: 5–23. [Google Scholar] [CrossRef][Green Version]
- Pradhan, Rudra, Mak Arvin, and Atanu Ghoshray. 2015. The dynamics of economic growth, oil prices, stock market depth, and other macroeconomic variables: Evidence from the G-20 countries. International Review of Financial Analysis 39: 84–95. [Google Scholar] [CrossRef]
- Pradhan, Rudra, Mak Arvin, John Hall, and Sahar Bahmani. 2014. Causal nexus between economic growth, banking sector development, stock market development, and other macroeconomic variables: The case of ASEAN countries. Review of Financial Economics 23: 155–73. [Google Scholar] [CrossRef]
- Pragidis, Ioannis-Chrisostomos, Panagiotis Tsintzos, and Bill Plakandaras. 2018. Asymmetric effects of government spending shocks during the financial cycle. Economic Modelling 68: 372–87. [Google Scholar] [CrossRef]
- Prasetyo, Ahmad Danu, and Ubaidillah Zuhdi. 2013. The government expenditure efficiency towards the human development. Procedia Economics and Finance 5: 615–22. [Google Scholar] [CrossRef]
- Rahn, Richard, and Helen Fox. 1996. What Is the Optimum Size of Government? Boulder: Vernon Krieble Foundation. [Google Scholar]
- Ravn, Morten, Stephanie Schmitt-Grohé, and Martin Uribe. 2012. Consumption, government spending, and the real exchange rate. Journal of Monetary Economics 59: 215–34. [Google Scholar] [CrossRef]
- Rebelo, Sergio. 1992. Long-run policy analysis and long-run growth. Journal of Political Economy 99: 500–21. [Google Scholar] [CrossRef]
- Rigobon, Roberto, and Dani Rodrik. 2005. Rule of law, democracy, openness, and income: Estimating the interrelationships1. Economics of Transition 13: 533–64. [Google Scholar] [CrossRef]
- Romer, Paul. 1986. Increasing returns and long run growth. Journal of Political Economy 94: 1002–37. [Google Scholar] [CrossRef]
- Romp, Ward, and Jakob De Haan. 2007. Public capital and economic growth: A critical survey. Perspektiven der Wirtschaftspolitik 8: 6–52. [Google Scholar] [CrossRef]
- Sawyer, Malcolm. 2012. The tragedy of UK fiscal policy in the aftermath of the financial crisis. Cambridge Journal of Economics 36: 205–21. [Google Scholar] [CrossRef]
- Sekrafi, Hbib, and Asma Sghaier. 2018. Examining the relationship between corruption, economic growth, environmental degradation, and energy consumption: A panel analysis in MENA region. Journal of the Knowledge Economy 9: 963–79. [Google Scholar] [CrossRef]
- Semik, Sofia, and Lilli Zimmermann. 2021. Determinants of substantial public debt reductions in Central and Eastern European Countries. Empirica 49: 53–70. [Google Scholar] [CrossRef]
- Shaddady, Ali, and Moore Tomoe. 2019. Investigation of the effects of financial regulation and supervision on bank stability: The application of CAMELS-DEA to quantile regressions. Journal of International Financial Markets, Institutions and Money 58: 96–116. [Google Scholar] [CrossRef]
- Shahbaz, Muhammad, Bright Gyamfi, Festus Bekun, and Divine Agozie. 2022. Toward the fourth industrial revolution among E7 economies: Assessment of the combined impact of institutional quality, bank funding, and foreign direct investment. Evaluation Review 46: 779–803. [Google Scholar] [CrossRef]
- Shahbaz, Muhammad, Ilham Haouas, and Thi Hong Van Hoang. 2019. Economic growth and environmental degradation in Vietnam: Is the environmental Kuznets curve a complete picture? Emerging Markets Review 38: 197–218. [Google Scholar] [CrossRef]
- Shaukat, Badiea, Qigui Zhu, and M. Ijaz Khan. 2019. Real interest rate and economic growth: A statistical exploration for transitory economies. Physica A: Statistical Mechanics and Its Applications 534: 122193. [Google Scholar] [CrossRef]
- Shonchoy, Abu. 2010. Determinants of Government Consumption Expenditure in Developing Countries: A Panel Data Analysis. Institute of Developing Economies (IDE) Discussion Paper 266. Available online: https://www.researchgate.net/publication/49175193_Determinants_of_government_consumption_expenditure_in_developing_countries_a_panel_data_analysis (accessed on 24 August 2022).
- Sokhanvar, Amin. 2019. Does foreign direct investment accelerate tourism and economic growth within Europe? Tourism Management Perspectives 29: 86–96. [Google Scholar] [CrossRef]
- Solow, Robert. 1956. A contribution to the theory of economic growth. Quarterly Journal of Economics 70: 65–94. [Google Scholar] [CrossRef]
- Stoilova, Desislava. 2017. Tax structure and economic growth: Evidence from the European Union. Contaduría y Administración 62: 1041–57. [Google Scholar] [CrossRef]
- Thomas, Melissa. 2010. What do the worldwide governance indicators measure? European Journal of Development Research 22: 31–54. [Google Scholar] [CrossRef]
- Ulucak, Recep. 2019. The effect of globalization on economic growth: Evidence from emerging economies. In Emerging Economic Models for Global Sustainability and Social Development. Hershey: IGI Global, pp. 1–19. [Google Scholar] [CrossRef]
- Van Dan, Dang, and Vu Duc Binh. 2019. The effect of macroeconomic variables on economic growth: A cross-country study. In Beyond Traditional Probabilistic Methods in Economics. Cham: Springer, vol. 809. [Google Scholar]
- Wu, Shih-Ying, Jenn-Hong Tang, and Eric Lin. 2010. The impact of government expenditure on economic growth: How sensitive to the level of development? Journal of Policy Modeling 32: 804–17. [Google Scholar] [CrossRef]
- Wu, Weijun, Ling Yuan, Xiaoming Wang, Xiaping Cao, and Sili Zhou. 2020. Does FDI drive economic growth? Evidence from city data in China. Emerging Markets Finance and Trade 56: 2594–607. [Google Scholar] [CrossRef]
- Yang, Zhou. 2016. Tax reform, fiscal decentralization, and regional economic growth: New evidence from China. Economic Modelling 59: 520–28. [Google Scholar] [CrossRef]
- Zagler, Martin, and Georg Dürnecker. 2003. Fiscal policy and economic growth. Journal of Economic Surveys 17: 397–418. [Google Scholar] [CrossRef]
- Zungu, Lindokuhle, Lorraine Greyling, and Mashapa Sekome. 2020. Government expenditure and economic growth: Testing for nonlinear effect among SADC countries, 1994–2017. African Journal of Business and Economic Research 15: 37–96. [Google Scholar] [CrossRef]


| Variables | Variable Abbreviation | Definition | Sources |
|---|---|---|---|
| Dependent variable | |||
| Economic growth | EG | Change in real GDP growth as annual percentage growth | WDI |
| Independent variables | |||
| Government spending | GS | Percentage change of real government spending | WDI |
| Other macro-indicators | |||
| FDI | FDI | FDI inflows as percentage of GDP | WDI |
| Inflation | INF | Percentage change in consumer price index | WDI |
| Real interest rate | IRR | Lending interest rate adjusted for inflation | WDI |
| Effective exchange rate | EXR | Currency value against weighted average of several foreign currencies divided by a price deflator or index of costs | IMF |
| Macro-governance indicators (Thomas 2010) | |||
| Voice and accountability | VA | The extent of citizens’ freedoms of selecting their government, expression, association, and media | WGI |
| Political stability | PS | Perceptions of potential government destabilization (including political violence and terrorism) | WGI |
| Government effectiveness | GE | Quality of public services, civil service independence from political pressure, policy formulation and implementation, and government commitment to such policies | WGI |
| Regulatory quality | RQ | Ability to formulate and implement sound policies and regulation promoting competition and private sector development | WGI |
| Variable | Obs | Mean | Std. Dev. | Min | Max |
|---|---|---|---|---|---|
| EG | 475 | 4.668077 | 6.741784 | −16.7 | 22.96 |
| GS | 475 | 15.49627 | 4.258418 | 5.94 | 32.01 |
| FDI | 475 | 5.086079 | 5.58653 | −1.39 | 15.08 |
| INF | 475 | 28.02541 | 99.58989 | −8.5 | 1058.4 |
| EXR | 475 | 98.5145 | 39.69589 | 45.10725 | 476.6331 |
| IRR | 475 | 4.40016 | 13.32158 | −6.13 | 39.81 |
| VA | 475 | 32.58102 | 19.46081 | 0 | 68.26923 |
| PS | 475 | 33.7587 | 16.24975 | 3.01 | 72.51185 |
| GE | 475 | 43.58616 | 17.87952 | 1.470588 | 69.14 |
| RQ | 475 | 39.42311 | 21.3978 | 1.421801 | 83.17308 |
| Year | World-EG | EG | GS | Countries | EG | GS |
|---|---|---|---|---|---|---|
| 1995 | 3 | −0.48053 | 17.41211 | Albania | 4.602889 | 11.01 |
| 1996 | 3.5 | 6.460725 | 16.74474 | Armenia | 6.3276 | 11.14 |
| 1997 | 3.7 | 3.097102 | 17.67895 | Azerbaijan | 7.4548 | 11.7404 |
| 1998 | 2.5 | 3.568621 | 16.78579 | Belarus | 4.2484 | 17.7596 |
| 1999 | 3.25 | 2.954822 | 16.15632 | Bosnia | 9.4984 | 21.4596 |
| 2000 | 4.4 | 5.938119 | 15.86421 | Bulgaria | 2.5108 | 17.3848 |
| 2001 | 1.9 | 5.585872 | 15.94 | Georgia | 5.5324 | 12.7756 |
| 2002 | 2.17 | 5.872199 | 15.86158 | Kazakhstan | 5.012 | 11.2164 |
| 2003 | 2.95 | 6.835975 | 15.56632 | Kyrgyzstan | 4.2592 | 18.0284 |
| 2004 | 4.41 | 8.164641 | 15.29684 | Moldova | 2.9824 | 18.3988 |
| 2005 | 3.9 | 8.381406 | 15.4879 | North Macedonia | 2.649241 | 17.6788 |
| 2006 | 4.37 | 8.954362 | 15.08947 | Romania | 3.3508 | 15.0032 |
| 2007 | 4.32 | 8.881395 | 15.12632 | Russia | 2.745682 | 17.9544 |
| 2008 | 1.86 | 6.791188 | 15.08579 | Serbia | 3.402999 | 19.3352 |
| 2009 | −1.66 | −1.63492 | 16.20579 | Tajikistan | 5.4076 | 12.7832 |
| 2010 | 4.31 | 4.230363 | 15.27211 | Turkey | 4.830746 | 13.2704 |
| 2011 | 3.12 | 5.162437 | 14.63947 | Turkmenistan | 6.924 | 10.7672 |
| 2012 | 2.52 | 3.077098 | 14.77684 | Ukraine | 0.9916 | 19.4572 |
| 2013 | 2.67 | 4.651533 | 14.50947 | Uzbekistan | 5.8292 | 17.2652 |
| 2014 | 2.87 | 3.134505 | 14.51947 | Total | 4.668077 | 15.49627 |
| 2015 | 2.92 | 2.125984 | 14.76842 | |||
| 2016 | 2.61 | 2.813346 | 14.91526 | |||
| 2017 | 3.28 | 4.205301 | 14.59158 | |||
| 2018 | 3.03 | 4.078969 | 14.47158 | |||
| 2019 | 2.33 | 3.851417 | 14.64053 | |||
| Total | 2.9692 | 4.668077 | 15.49627 |
| Variables | (1) | (2) | (3) | (4) | (5) | (6) | (7) | (8) | (9) | (10) |
|---|---|---|---|---|---|---|---|---|---|---|
| EG (1) | 1 | |||||||||
| GS (2) | −0.1533 | 1 | ||||||||
| FDI (3) | 0.1811 | −0.2245 | 1 | |||||||
| INF (4) | −0.2527 | 0.0442 | −0.0523 | 1 | ||||||
| EXR (5) | −0.0216 | 0.2286 | −0.1002 | −0.0039 | 1 | |||||
| IRR (6) | 0.0324 | −0.0639 | 0.097 | −0.3002 | −0.05 | 1 | ||||
| VA (7) | −0.1147 | 0.2519 | 0.0003 | −0.0883 | −0.2473 | 0.0681 | 1 | |||
| PS (8) | −0.0558 | −0.0655 | 0.0708 | 0.041 | −0.082 | −0.0687 | 0.1962 | 1 | ||
| GE (9) | −0.0715 | −0.0049 | −0.0163 | −0.006 | −0.0639 | −0.018 | 0.1043 | 0.0951 | 1 | |
| RQ (10) | −0.1487 | −0.018 | 0.0256 | −0.1818 | −0.2127 | 0.1764 | 0.5627 | 0.1845 | 0.0895 | 1 |
| (1) | (2) | (3) | (4) | (5) | (6) | (7) | (8) | (9) | (10) | (11) | (12) | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| VRs | OLS | Q 25 | Q 50 | Q 75 | OLS | Q 25 | Q 50 | Q 75 | OLS | Q25 | Q50 | Q75 |
| Linear | Quadratic | Cubic | ||||||||||
| GS | −0.252 *** | −0.308 *** | −0.280 *** | −0.237 *** | −1.402 *** | −0.444 ** | −0.425 *** | −1.161 ** | −0.805 ** | −0.422 ** | −0.433 ** | −0.347 *** |
| (0.086) | (0.0001) | (0.0020) | (0.070) | (0.5014) | (0.0017) | (0.0131) | (0.4912) | (0.169) | (0.089) | (0.035) | (0.085) | |
| 0.360 *** | 0.00434 ** | 0.0483 *** | 0.0295 ** | 0.151 ** | 0.266 * | 0.00529 ** | 0.00220 ** | |||||
| (0.0172) | (0.0008) | (0.009) | (0.0166) | (0.077) | (0.117) | (0.0005) | (0.0001) | |||||
| −0.00217 * | −0.00186 ** | −0.008754 *** | −0.00132 ** | |||||||||
| (0.00120) | (0.00032) | (0.0009) | (0.0006) | |||||||||
| FDI | 0.191 *** | 0.111 *** | 0.906 ** | 0.237 * | 0.183 *** | 0.113 *** | 0.0878 ** | 0.144 ** | 0.184 *** | 0.136 *** | 0.879 *** | 0.113 *** |
| (0.0625) | (0.0383) | (0.0386) | (0.142) | (0.0589) | (0.0415) | (0.0375) | (0.0642) | (0.0578) | (0.041) | (0.014) | (0.041) | |
| INF | −0.0193 *** | −0.0266 *** | −0.0200 *** | −0.0408 * | −0.0199 *** | −0.0276 *** | −0.0195 *** | −0.00980 ** | −0.0195 *** | −0.0610 * | −0.0495 ** | −0.0272 *** |
| (0.00487) | (0.00949) | (0.00750) | (0.0208) | (0.00473) | (0.00940) | (0.00723) | (0.00712) | (0.00460) | (0.0110) | (0.0100) | (0.00758) | |
| EXR | −0.00391 | 0.00695 * | −0.00285 | −0.0105 *** | −0.00882 | 0.00719 * | −0.00327 | −0.00332 | −0.00322 | −0.00994 * | −0.00126 | 0.00733 * |
| (0.00548) | (0.00187) | (0.00482) | (0.00395) | (0.00998) | (0.00173) | (0.00484) | (0.00996) | (0.00692) | (0.00290) | (0.00428) | (0.00201) | |
| IRR | −0.0113 ** | −0.0663 * | −0.0476 * | −0.0835 ** | −0.0866 ** | −0.0710 * | −0.0208 ** | −0.0781 ** | −0.0138 | 0.000523 | −0.0206 | −0.0698 ** |
| (0.0017) | (0.0198) | (0.0106) | (0.0143) | (0.0113) | (0.0207) | (0.0100) | (0.0229) | (0.0210) | (0.0220) | (0.0263) | (0.0139) | |
| VA | 0.0148 | 0.0222 | 0.0327 | 0.00517 | 0.00959 | 0.0200 | 0.0313 | −0.0240 | 0.00424 | −0.0133 | 0.0313 | 0.0192 |
| (0.0213) | (0.0359) | (0.0420) | (0.0222) | (0.0213) | (0.0365) | (0.0514) | (0.0342) | (0.0215) | (0.0339) | (0.0502) | (0.0348) | |
| PS | −0.0243 | −0.0266 | −0.00222 | −0.00795 | −0.00297 | −0.00267 | −0.00567 | −0.00351 | −0.00316 | −0.00684 | −0.00563 | −0.00259 |
| (0.0489) | (0.0392) | (0.0168) | (0.0133) | (0.0298) | (0.0199) | (0.0183) | (0.0136) | (0.0201) | (0.0600) | (0.0161) | (0.0236) | |
| GE | −0.0021 *** | −0.00151 | −0.00225 | −0.00261 | −0.0029 *** | −0.00147 | −0.00222 | −0.00245 | −0.00240 *** | −0.00248 | −0.00222 | −0.00148 |
| (0.000568) | (0.0186) | (0.0177) | (0.0276) | (0.000479) | (0.0175) | (0.0165) | (0.0241) | (0.000464) | (0.00353) | (0.00751) | (0.0223) | |
| RQ | −0.172 ** | −0.0842 * | −0.0890 *** | −0.0281 | −0.154 ** | −0.0791 * | −0.0915 *** | −0.0212 | −0.151 ** | −0.0278 | −0.0915 *** | −0.0804 * |
| (0.0739) | (0.0248) | (0.0032) | (0.0654) | (0.0661) | (0.0237) | (0.0028) | (0.0690) | (0.0653) | (0.0545) | (0.0029) | (0.0359) | |
| Constant | 0.040 *** | 0.519 *** | 0.180 *** | 0.345 *** | 0.313 *** | 0.414 *** | 0.193 *** | 0.661 *** | 0.262 *** | 0.592 *** | 0.196 *** | 0.330 |
| (0.006) | (0.064) | (0.007) | (0.016) | (0.018) | (0.047) | (0.086) | (0.028) | (0.035) | (0.057) | (0.042) | (0.715) | |
| Years | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes |
| Country | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes |
| Obs | 475 | 475 | 475 | 475 | 475 | 475 | 475 | 475 | 475 | 475 | 475 | 475 |
| R-sq. | 0.177 | 0.194 | 0.197 | |||||||||
| Min | Max | |
|---|---|---|
| Interval | 5.94 | 32.01 |
| Slope | −0.97 *** | 0.90 *** |
| (−3.24) | −1.47 | |
| SLM test for U shape | 1.47 *** | |
| p-value | 0.07 | |
| Extreme point | 19.40 |
| Variables | Intercept | Coefficients | p-Value |
|---|---|---|---|
| GS | 13.83 | −0.62 | 0.00 |
| 191.27 | 0.22 | 0.01 | |
| 2645.25 | −0.00 | 0.03 | |
| R2 | 0.60 | ||
| Adjusted R2 | 0.54 | ||
| Predicted (inflection point) | 13.32 | ||
| Observations | 474 |
| (1) | (2) | (3) | (4) | (5) | (6) | (7) | (8) | (9) | (10) | (11) | (12) | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Variables | OLS | Q25 | Q50 | Q75 | OLS | Q25 | Q50 | Q75 | OLS | Q25 | Q50 | Q75 |
| Government spending indicators | ||||||||||||
| GS | −0.537 *** | −0.517 *** | −0.253 *** | −0.065 ** | −0.304 *** | −0.971 *** | −0.615 ** | −0.307 *** | −0.572 *** | −0.096 * | −0.629 ** | −0.285 * |
| GS2 | 0.220 *** | 0.196 ** | 0.168 ** | 0.152 * | 0.206 *** | 0.157 ** | 0.126 *** | 0.164 *** | 0.164 ** | 0.111 * | 0.0804 *** | 0.167 ** |
| GS3 | −0.00337 *** | −0.00374 ** | −0.00287 ** | −0.00259 ** | −0.0030 *** | −0.00285 *** | −0.00203 * | −0.00269 ** | −0.00243 ** | −0.00222 ** | −0.0015 ** | −0.00291 * |
| Macro-economic indicators | ||||||||||||
| FDI | 0.166 *** | 0.139 *** | 0.1000 *** | 0.0584 * | ||||||||
| INF | −0.0185 *** | −0.0227 *** | −0.0201 *** | −0.00819 ** | ||||||||
| REX | −0.00108 | 0.0114 ** | 0.00321 | −0.00202 | ||||||||
| IRR | −0.0312 | −0.0649 | −0.0374 | −0.00498 | ||||||||
| Macro-governance indicators | ||||||||||||
| VC | 0.0361 | 0.0312 | 0.0349 | 0.0065 | ||||||||
| PS | −0.0257 | −0.0139 | −0.0165 | −0.00073 | ||||||||
| GE | −0.00195 *** | −0.00147 | −0.00212 | −0.0026 | ||||||||
| RQ | −0.0532 ** | −0.0461 | −0.0653 ** | −0.0452 * | ||||||||
| Constant | 0.056 *** | 0.333 *** | 0.603 *** | 0.732 *** | 0.399 *** | 0.969 ** | 0.255 *** | 0.878 *** | 0.359 *** | 0.170 * | 0.840 *** | 0.014 *** |
| 0.428 | 0.882 | 0.093 | 0.983 | 0.251 | 0.785 | 0.014 | 0.025 | 0.551 | 0.459 | 0.422 | 0.993 | |
| Observations | 475 | 475 | 475 | 475 | 475 | 475 | 475 | 475 | 475 | 475 | 475 | 475 |
| R-squared | 0.059 | 0.147 | 0.078 | |||||||||
| (1) | (2) | (3) | (4) | (5) | (6) | (7) | (8) | (9) | |
|---|---|---|---|---|---|---|---|---|---|
| Variables | GMM | GMM | GMM | FE | FE | FE | IV | IV | IV |
| 0.234 *** | 0.255 *** | 0.268 *** | |||||||
| (0.085) | (0.084) | (0.084) | |||||||
| GS | −0.344 *** | −0.811 * | −0.0721 ** | −0.266** | −1.266 * | −2.473 *** | |||
| (0.012) | (0.178) | (0.017) | (0.013) | (0.629) | (1.993) | ||||
| GS2 | 0.0143 ** | 0.360 *** | 0.0294 *** | 0.604 ** | |||||
| (0.0015) | (0.011) | (0.0026) | (0.111) | ||||||
| GS3 | −0.0990 ** | −0.0140 ** | |||||||
| (0.0022) | (0.0019) | ||||||||
| GS (fitted value) | −0.484 ** | −0.559 *** | −0.897 *** | ||||||
| (0.015) | (0.021) | (0.070) | |||||||
| GS2 (fitted value) | 0.446 *** | 0.920 *** | |||||||
| (0.0766) | (0.047) | ||||||||
| GS3 (fitted value) | −0.0554 *** | ||||||||
| (0.0015) | |||||||||
| FDI | 0.128 ** | 0.127 ** | 0.127 ** | 0.188 *** | 0.198 *** | 0.112 *** | 0.167 ** | 0.0802 * | 0.0670 ** |
| (0.0547) | (0.0540) | (0.0546) | (0.0372) | (0.039) | (0.0393) | (0.0654) | (0.0344) | (0.0334) | |
| INF | −0.0167 ** | −0.0171 *** | −0.0170 *** | −0.0198 *** | −0.0200 *** | −0.0198 *** | −0.0187 *** | −0.0245 *** | −0.0217 *** |
| (0.0017) | (0.0017) | (0.0016) | (0.00655) | (0.00645) | (0.00635) | (0.00519) | (0.00507) | (0.00505) | |
| EXR | −0.0597 * | −0.0585 | −0.0584 | −0.000534 | −0.0041 | −0.00323 | −0.00103 | −0.0029 | −0.00109 |
| (0.0265) | (0.0766) | (0.0672) | (0.00468) | (0.00714) | (0.00711) | (0.00859) | (0.00832) | (0.00826) | |
| RRI | −0.0172 | −0.0201 | −0.0202 | −0.0318 | −0.0400 ** | −0.0287 | −0.0207 | −0.0844 ** | −0.0760 * |
| (0.0552) | (0.0528) | (0.0520) | (0.03440) | (0.0220) | (0.03110) | (0.03970) | (0.0395 | (0.0389) | |
| VA | 0.0615 | 0.082 | 0.0803 | 0.0318 | 0.0275 | 0.0279 | 0.021 | 0.0247 | 0.00613 |
| (0.0959) | (0.0972) | (0.0976) | (0.0469) | (0.0444) | (0.0438) | (0.0358) | (0.0294) | (0.0294) | |
| PS | 0.00473 | 0.00625 | 0.0063 | 0.00146 | 0.00531 | 0.00576 | 0.00131 | 0.00554 | 0.00199 |
| (0.0254) | (0.0258) | (0.0260) | (0.0239) | (0.0241) | (0.0241) | (0.0255) | (0.0244) | (0.0243) | |
| GE | −0.000836 | −0.008304 * | −0.000805 | −0.00295 ** | −0.00296 * | −0.0391 *** | −0.00325 * | −0.00286 * | −0.00225 |
| (0.00594) | (0.00543) | (0.00659) | (0.00175) | (0.00107) | (0.00221) | (0.00177) | (0.0017) | (0.00368) | |
| RQ | −0.121 *** | −0.120 *** | −0.123 *** | −0.738 * | −0.124 *** | −0.420 * | −0.815 *** | −0.0468 * | −0.0637 ** |
| (0.0370) | (0.0380) | (0.0368) | (0.288) | (0.0745) | (0.144) | (0.0257) | (0.017) | (0.0270) | |
| Constant | 0.574 *** | 0.919 *** | 0.481 ** | 0.582 *** | 0.672 *** | 0.376 ** | 0.124 * | 0.221 *** | 0.266 *** |
| Time | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes |
| Countries | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes |
| AB test AR (1) | 0.006 | 0.003 | 0.004 | ||||||
| AB test AR (2) | 0.580 | 0.655 | 0.632 | ||||||
| Hansen test (p-value | 0.321 | 0.291 | 0.355 | ||||||
| Instruments | 28 | 33 | 34 | ||||||
| Groups | 41 | 41 | 41 | ||||||
| R-squared | 0.551 | 0.461 | 0.562 | 0.691 | 0.575 | 0.204 | |||
| Observations | 437 | 437 | 437 | 475 | 475 | 475 | 475 | 475 | 475 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shaddady, A. Is Government Spending an Important Factor in Economic Growth? Nonlinear Cubic Quantile Nexus from Eastern Europe and Central Asia (EECA). Economies 2022, 10, 286. https://doi.org/10.3390/economies10110286
Shaddady A. Is Government Spending an Important Factor in Economic Growth? Nonlinear Cubic Quantile Nexus from Eastern Europe and Central Asia (EECA). Economies. 2022; 10(11):286. https://doi.org/10.3390/economies10110286
Chicago/Turabian StyleShaddady, Ali. 2022. "Is Government Spending an Important Factor in Economic Growth? Nonlinear Cubic Quantile Nexus from Eastern Europe and Central Asia (EECA)" Economies 10, no. 11: 286. https://doi.org/10.3390/economies10110286
APA StyleShaddady, A. (2022). Is Government Spending an Important Factor in Economic Growth? Nonlinear Cubic Quantile Nexus from Eastern Europe and Central Asia (EECA). Economies, 10(11), 286. https://doi.org/10.3390/economies10110286







