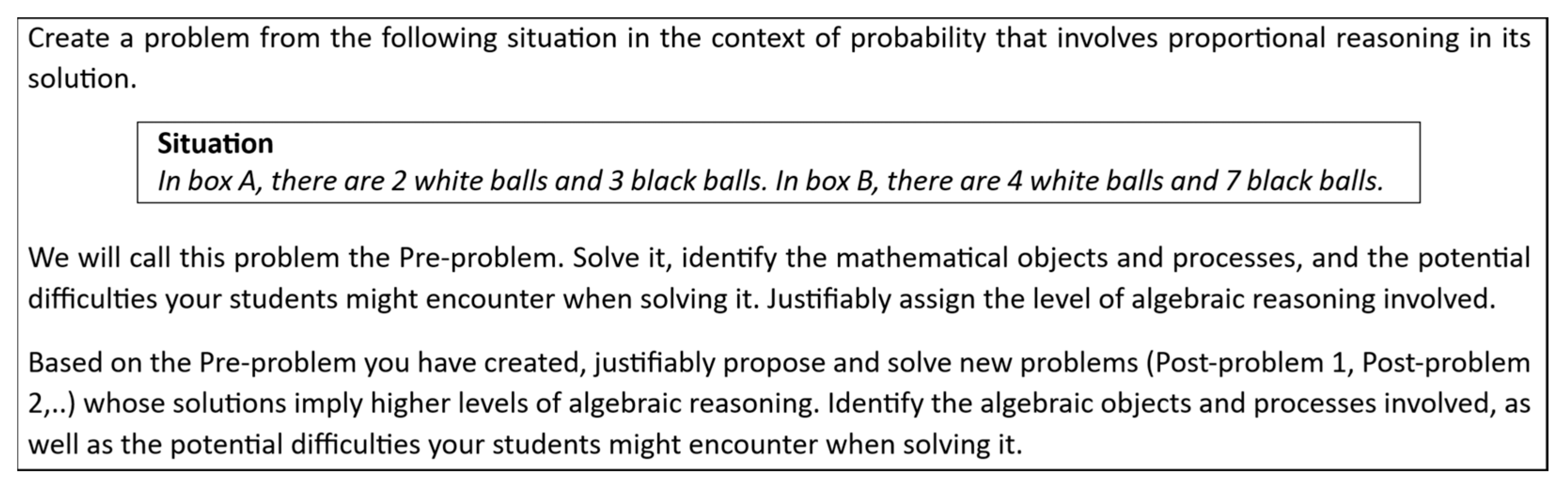
Creation of Problems by Prospective Teachers to Develop Proportional and Algebraic Reasonings in a Probabilistic Context
Abstract
:1. Introduction
- RQ1—How do prospective teachers pose problems in a probabilistic context to promote proportional reasoning?
- RQ2—What objects and processes related to proportional reasoning involved in their solutions do prospective teachers identify?
- RQ3—What potential difficulties do prospective teachers identify after analyzing the posed problems, and how do they relate to the analysis of objects and processes?
- RQ4—How do prospective teachers transform proportionality problems in a probabilistic context to increase the level of algebraic reasoning involved in their solutions?
2. Theoretical Framework
2.1. Pragmatic Meaning and Levels of Algebraization
- Level 0. Operations are carried out with intensive objects of the first degree of generality, using natural, numeric, iconic, or gestural languages.
- Level 1. Intensive objects of the second degree of generality are used, properties of the algebraic structure of natural numbers, and equality as equivalence.
- Level 2. Symbolic–literal representations are used to refer to recognized intensive objects, which are linked to spatial, temporal, and contextual information. Equations of the form Ax + B = C are solved.
- Level 3. Symbols are used analytically, without referring to contextual information. Operations are performed with unknowns. Equations of the form Ax + B = Cx + D are solved.
2.2. Teacher’s Didactic-Mathematical Knowledge and Competence Model
2.3. Problem Posing
- The variation of a given problem, by which a new problem is posed by modifying one or more of the four elements of an initial problem.
- The elaboration of a new problem, which can be performed freely, from a (given or configured by the author) situation, or from a specific requirement, which can have a mathematical or didactic emphasis. Didactic-mathematical knowledge about the content, in our case proportionality, is especially relevant to adequately respond to the requirement.
3. Method
- Partially relevant (PrePR). Although it is significant, one of the following errors is made:
- ○
- The proposed situation is modified (information error, IE).
- ○
- Its solution does not involve proportional reasoning (mathematical environment error, EE).
- Non-relevant (PreNR). The proposed situation is modified, and its solution does not involve proportional reasoning.
- Relevant (PosR). The Post-problem is a variation of the Pre-problem, and its solution involves a higher EAR level than the actual one involved in the solution of the Pre-problem.
- Partially relevant (PosPR). Although it is significant, one of the following situations occurs:
- ○
- It is not a variation of the Pre-problem (NoPre).
- ○
- It does not involve in its solution a higher EAR level than the one involved in the solution of the Pre-problem (NoEAR).
- Non-relevant (PosNR). It is not a variation of the Pre-problem, nor does its solution imply a higher EAR level than the corresponding Pre-problem solution.
- Relevant difficulty (RD). The difficulty is appropriate to the posed problem and refers to knowledge or skills involved in the proposed solution.
- Partially relevant difficulty (PRD). The difficulty is appropriate to the posed problem, but it does not refer to knowledge or skills involved in the proposed solution.
- Non-relevant difficulty (NRD). The difficulty is not appropriate to the posed problem.
4. Results
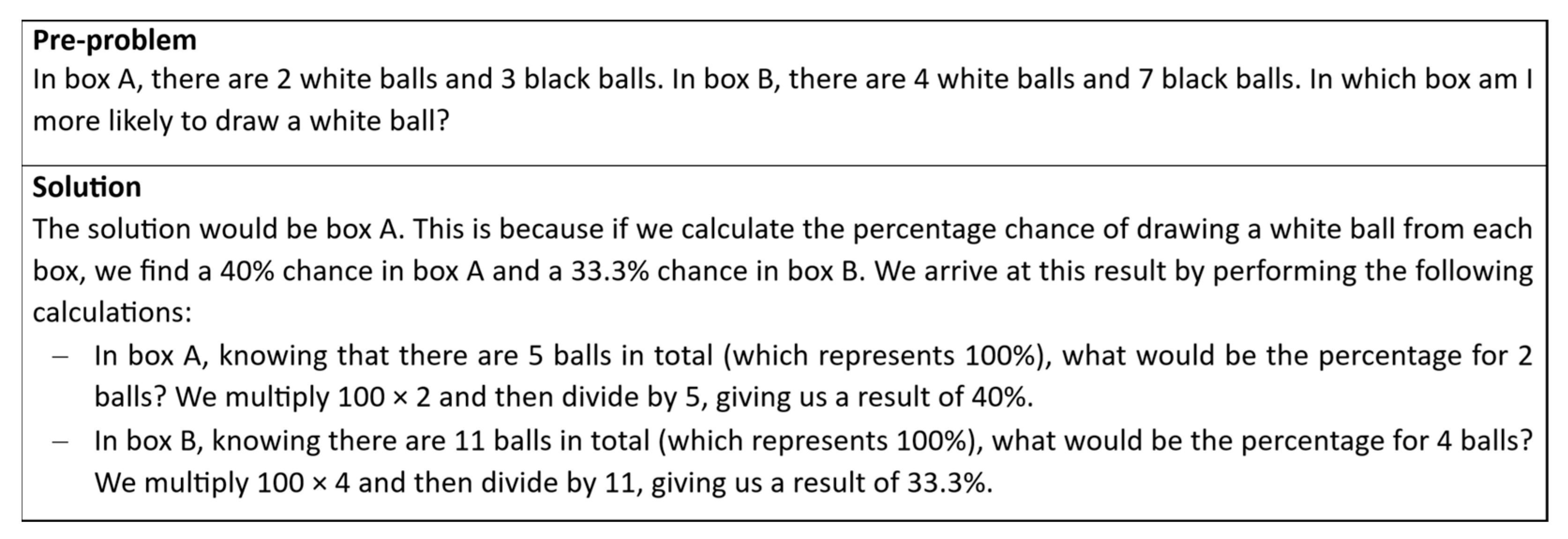
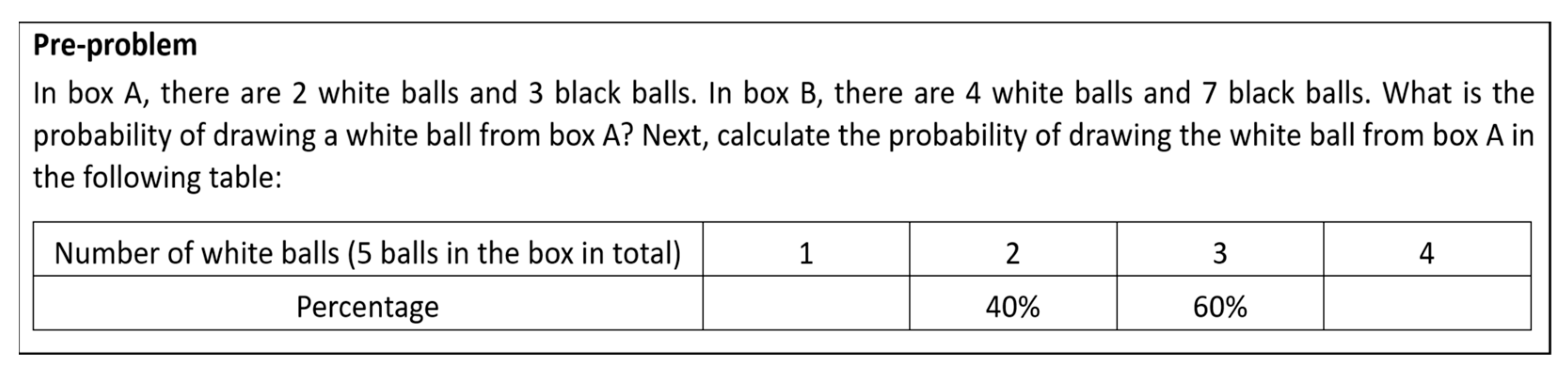
4.1. Pre-Problem
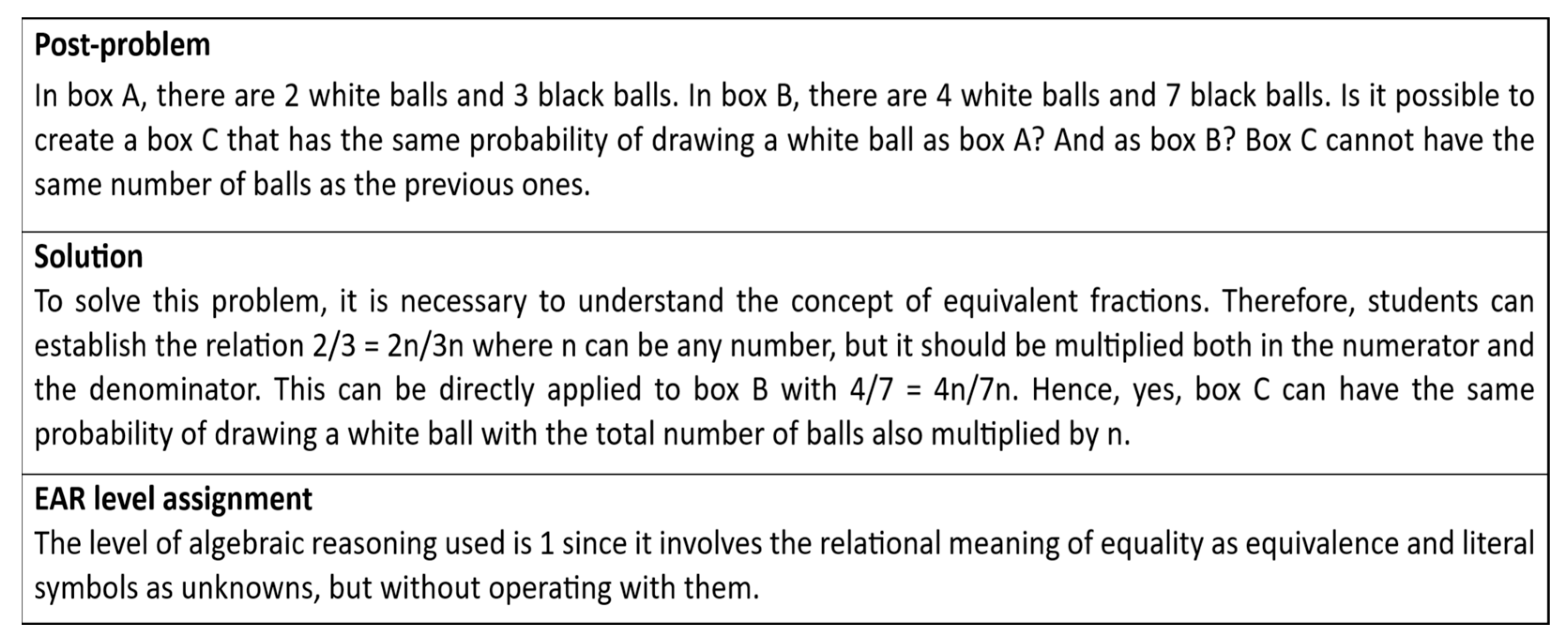
4.2. Post-Problem
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chen, T.; Cai, J. An elementary mathematics teacher learning to teach using problem-posing: A case of the distributive property of multiplication over addition. Int. J. Educ. Res. 2020, 102, 101420. [Google Scholar] [CrossRef]
- Koichu, B. Problem-posing in the context of teaching for advanced problem solving. Int. J. Educ. Res. 2020, 102, 101428. [Google Scholar] [CrossRef]
- Silber, S.; Cai, J. Exploring underprepared undergraduate students’ mathematical problem-posing. ZDM Math. Educ. 2021, 53, 877–889. [Google Scholar] [CrossRef]
- Xu, B.; Cai, J.; Liu, Q.; Hwang, S. Teachers’ predictions of students’ mathematical thinking related to problem-posing. Int. J. Educ. Res. 2020, 102, 101427. [Google Scholar] [CrossRef]
- Cai, J.; Hwang, S. Seeing algebra in arithmetic through mathematical problem-posing. J. Educ. Res. Math. 2022, 32, 309–329. [Google Scholar] [CrossRef]
- Pino-Fan, L.R.; Báez-Huaiquián, D.I.; Molina-Cabero, J.G.; Hernández-Arredondo, E. Criterios utilizados por profesores de matemáticas para el planteamiento de problemas en el aula. Uniciencia 2020, 34, 114–136. [Google Scholar] [CrossRef]
- Silver, E.A. Problem-posing research in mathematics education: Looking back, looking around, and looking ahead. Educ. Stud. Math. 2013, 83, 157–162. [Google Scholar] [CrossRef]
- Christou, C.; Mousoulides, N.; Pittalis, M.; Pitta-Pantazi, D.; Sriraman, B. An empirical taxonomy of problem-posing processes. ZDM Math. Educ. 2005, 37, 149–158. [Google Scholar] [CrossRef]
- Fernández, M.E.; Carrillo, J. Un acercamiento a la forma en que los estudiantes de primaria formulan problemas. REMat 2020, 17, 1–19. [Google Scholar] [CrossRef]
- Milinković, J. Conceptualizing problem posing via transformation. In Mathematical Problem Posing: From Research to Effective Practice, 1st ed.; Cai, J., Ellerton, N., Singer, F.M., Eds.; Springer: New York, NY, USA, 2015; pp. 47–70. [Google Scholar] [CrossRef]
- Mallart, A.; Font, V.; Diez, J. Case Study on Mathematics Pre-service Teachers’ Difficulties in Problem Posing. Eurasia J. Math. Sci. Technol. Educ. 2018, 14, 1465–1481. [Google Scholar] [CrossRef]
- Tichá, M.; Hošpesová, A. Developing teachers’ subject didactic competence through problem-posing. Educ. Stud. Math. 2013, 83, 133–143. [Google Scholar] [CrossRef]
- Breda, A.; Pino-Fan, L.; Font, V. Meta didactic-mathematical knowledge of teachers: Criteria for the reflection and assessment on teaching practice. Eurasia J. Math. Sci. Technol. Educ. 2017, 13, 1893–1918. [Google Scholar] [CrossRef]
- Malaspina, U.; Torres, C.; Rubio, N. How to stimulate in-service teachers’ didactic analysis competence by means of problem posing. In Mathematical Problem Solving, 1st ed.; Liljedahl, P., Santos-Trigo, L., Eds.; Springer: Cham, Switzerland, 2019; pp. 133–151. [Google Scholar]
- Crespo, S.; Sinclair, N. What makes a problem mathematically interesting? Inviting prospective teachers to pose better problems. J. Math. Teach. Educ. 2008, 11, 395–415. [Google Scholar] [CrossRef]
- Lee, Y.; Capraro, R.M.; Capraro, M.M. Mathematics Teachers’ Subject Matter Knowledge and Pedagogical Content Knowledge in Problem-posing. Int. Electron. J. Math. Educ. 2018, 13, 75–90. [Google Scholar] [CrossRef] [PubMed]
- Xie, J.; Masingila, J. Examining interactions between problem-posing and problem solving with prospective primary teachers: A case of using fractions. Educ. Stud. Math. 2017, 96, 101–118. [Google Scholar] [CrossRef]
- Singer, F.; Ellerton, N.; Cai, J. Problem-posing research in mathematics education: New questions and directions. Educ. Stud. Math. 2013, 83, 1–7. [Google Scholar] [CrossRef]
- Lamon, S. Rational number and proportional reasoning: Toward a theoretical framework for research. In Second Handbook of Research on Mathematics Teaching and Learning, 1st ed.; Lester, F.K., Ed.; Information Age Publishing: Charlotte, NC, USA, 2007; pp. 629–667. [Google Scholar]
- Sánchez, E.; Valdez, J. Las ideas fundamentales de probabilidad en el razonamiento de estudiantes de bachillerato. Av. Investig. Educ. Matemática 2017, 11, 127–143. [Google Scholar] [CrossRef]
- Bryant, P.; Nunes, T. Children’s Understanding of Probability: A Literature Review (Full Report), 1st ed.; The Nutffield Foundation: Londres, UK, 2012. [Google Scholar]
- Blanton, M.; Brizuela, B.M.; Murphy, A.; Sawrey, K.; Newman-Owens, A. A learning trajectory in 6-year-olds’s thinking about generalizing functional relationship. J. Res. Math. Educ. 2015, 46, 511–559. [Google Scholar] [CrossRef]
- Burgos, M.; Godino, J.D. Assessing the Epistemic Analysis Competence of Prospective Primary School Teachers on Proportionality Tasks. Int. J. Sci. Math. Educ. 2022, 20, 367–389. [Google Scholar] [CrossRef]
- Hilton, A.; Hilton, G. Primary school teachers implementing structured mathematics interventions to promote their mathematics knowledge for teaching proportional reasoning. J. Math. Teach. Educ. 2019, 22, 545–574. [Google Scholar] [CrossRef]
- Weiland, T.; Orrill, C.; Brown, R.; Nagar, G.G. Mathematics teachers’ ability to identify situations appropriate for proportional reasoning. Res. Math. Educ. 2019, 21, 233–250. [Google Scholar] [CrossRef]
- Batanero, C.; Arteaga, P.; Serrano, L.; Ruiz, B. Prospective primary school teachers’ perception of randomness. In Probabilistic Thinking: Presenting Plural Perspectives, 1st ed.; Chernoff, E., Sriraman, B., Eds.; Springer: New York, NY, USA, 2014; pp. 345–366. [Google Scholar]
- Begolli, K.N.; Dai, T.; McGinn, K.M.; Booth, J.L. Could probability be out of proportion? Self-explanation and example-based practice help students with lower proportional reasoning skills learn probability. Instr. Sci. 2021, 49, 441–473. [Google Scholar] [CrossRef]
- Chernoff, E.J.; Russell, G.L. The fallacy of composition: Prospective mathematics teachers’ use of logical fallacies. Can. J. Sci. Math. Technol. Educ. 2012, 12, 259–271. [Google Scholar] [CrossRef]
- Vásquez, C.; Alsina, A. El conocimiento del profesorado para enseñar probabilidad: Un análisis global desde el modelo del Conocimiento Didáctico-Matemático. Av. Investig. Educ. Mat. 2015, 7, 27–48. [Google Scholar] [CrossRef]
- Stylianou, D.A.; Stroud, R.; Cassidy, M.; Knuth, E.; Stephens, A.; Gardiner, A.; Demers, L. Putting early algebra in the hands of elementary school teachers: Examining fidelity of implementation and its relation to student performance. Infancia y Aprendiz. 2019, 42, 523–569. [Google Scholar] [CrossRef]
- Zapatera, A.; Quevedo, E. The Initial Algebraic Knowledge of Preservice Teachers. Mathematics 2021, 9, 2117. [Google Scholar] [CrossRef]
- Burgos, M.; Chaverri-Hernández, J.J. Knowledge and competencies of prospective teachers for the creation of proportionality problems. Acta Sci. 2022, 24, 270–306. [Google Scholar] [CrossRef]
- Burgos, M.; Chaverri-Hernández, J.J. Creation of proportionality problems for the training of prospective primary school teachers. Uniciencia 2023, 37, 1–24. [Google Scholar] [CrossRef]
- Şengül, S.; Katranci, Y. The analysis of the problems posed by prospective mathematics teachers about ‘ratio and proportion’ subject. Procedia Soc. Behav. Sci. 2015, 174, 1364–1370. [Google Scholar] [CrossRef]
- Şengül, S.; Katranci, Y. Free problem posing cases of prospective mathematics teachers: Difficulties and solutions. Procedia Soc. Behav. Sci. 2015, 174, 1983–1990. [Google Scholar] [CrossRef]
- Godino, J.D.; Batanero, C.; Font, V. The onto-semiotic approach: Implications for the prescriptive character of didactics. Learn. Math. 2019, 39, 37–42. [Google Scholar]
- Godino, J.D.; Neto, T.; Wilhelmi, M.R.; Aké, L.; Etchegaray, S.; Lasa, A. Algebraic reasoning levels in primary and secondary education. In Proceedings of the CERME 9—Ninth Congress of the European Society for Research in Mathematics Education, Prague, Czech Republic, 4–8 February 2015. [Google Scholar]
- Godino, J.D.; Giacomone, B.; Batanero, C.; Font, V. Enfoque ontosemiótico de los conocimientos y competencias del profesor de matemáticas. Bolema 2017, 31, 90–113. [Google Scholar] [CrossRef]
- Burgos, M.; Godino, J.D. Modelo ontosemiótico de referencia de la proporcionalidad. Implicaciones para la planificación curricular en primaria y secundaria. Av. Investig. Educ. Mat. 2020, 18, 1–20. [Google Scholar] [CrossRef]
- Burgos, M.; Batanero, C.; Godino, J.D. Algebraization levels in the study of probability. Mathematics 2022, 10, 91. [Google Scholar] [CrossRef]
- Pino-Fan, L.; Font, V.; Breda, A. Mathematics teachers’ knowledge and competences model based on the onto-semiotic approach. In Proceedings of the 41st Conference of the International Group for the Psychology of Mathematics Education, Singapore, 17–22 July 2017; Kaur, B., Ho, W.K., Toh, T.L., Choy, B.H., Eds.; PME: Singapore, 2017; pp. 33–40. [Google Scholar]
- Font, V.; Sánchez, A.; Sala, G. Prospective Teachers’ Narrative Analysis Using the Didactic-Mathematical Knowledge and Competences Model (DMKC). In Advances in the Anthropological Theory of the Didactic, 1st ed.; Chevallard, Y., Barquero, B., Bosch, M., Florensa, I., Gascón, J., Nicolás, P., Ruiz-Munzón, N., Eds.; Birkhäuser: Cham, Switzerland, 2022; pp. 147–153. [Google Scholar]
- Silver, E.A. On mathematical problem-posing. Learn. Math. 1994, 14, 19–28. [Google Scholar]
- Stoyanova, E.; Ellerton, N.F. A framework for research into students’ problem-posing. In Technology in Mathematics Education, 1st ed.; Clarkson, P., Ed.; Mathematics Education Research Group of Australasia: Melbourne, Australia, 1996; pp. 518–525. [Google Scholar]
- Baumanns, L.; Rott, B. Rethinking problem-posing situations: A review. Investig. Math. Learn. 2021, 13, 59–76. [Google Scholar] [CrossRef]
- Malaspina, U.; Mallart, A.; Font, V. Development of teachers’ mathematical and didactic competencies by means of problem posing. In Proceedings of the CERME 9—Ninth Congress of the European Society for Research in Mathematics Education, Prague, Czech Republic, 4–8 February 2015. [Google Scholar]
- Godino, J.D.; Batanero, C.; Contreras, A.; Estepa, A.; Lacasta, E.; Wilhelmi, M.R. Didactic engineering as design-based research in mathematics education. In Proceedings of the CERME 8—Eighth Congress of the European Society for Research in Mathematics Education, Antalya, Turkye, 6–10 February 2013. [Google Scholar]
- Hart, L.C.; Smith, S.Z.; Swars, S.L.; Smith, M.E. An examination of research methods in mathematics education (1995–2005). J. Mix. Methods Res. 2009, 3, 26–41. [Google Scholar] [CrossRef]
- Burgos, M.; Godino, J.D. Prospective primary school teachers’ competence for analysing the difficulties in solving proportionality problem. Math. Educ. Res. J. 2022, 34, 269–291. [Google Scholar] [CrossRef]
- Lim, W.; Guerra, P. Using a Pedagogical Content Knowledge Assessment to Inform a Middle Grades Mathematics Teacher Preparation Program. Ga. Educ. Res. 2013, 10, 1. [Google Scholar] [CrossRef]
- Pincheria, N.; Vázquez, C.; Giacomone, B. Una aproximación al conocimiento didáctico-matemático de futuros profesores de Educación Básica para enseñar matemáticas elementales. Uniciencia 2021, 35, 1–18. [Google Scholar] [CrossRef]
- Ostermann, A. Factors influencing the accuracy of diagnostic judgments. In Diagnostic Competence of Mathematics Teachers, 1st ed.; Leuders, T., Philipp, K., Leuders, J., Eds.; Springer International Publishing AG: Cham, Switzerland, 2018; pp. 95–108. [Google Scholar]
- Supply, A.S.; Vanluydt, E.; Van Dooren, W.; Onghena, P. Out of proportion or out of context? Comparing 8- to 9-year-olds’ proportional reasoning abilities across fair-sharing, mixtures, and probability contexts. Educ. Stud. Math. 2023, 113, 371–388. [Google Scholar] [CrossRef] [PubMed]


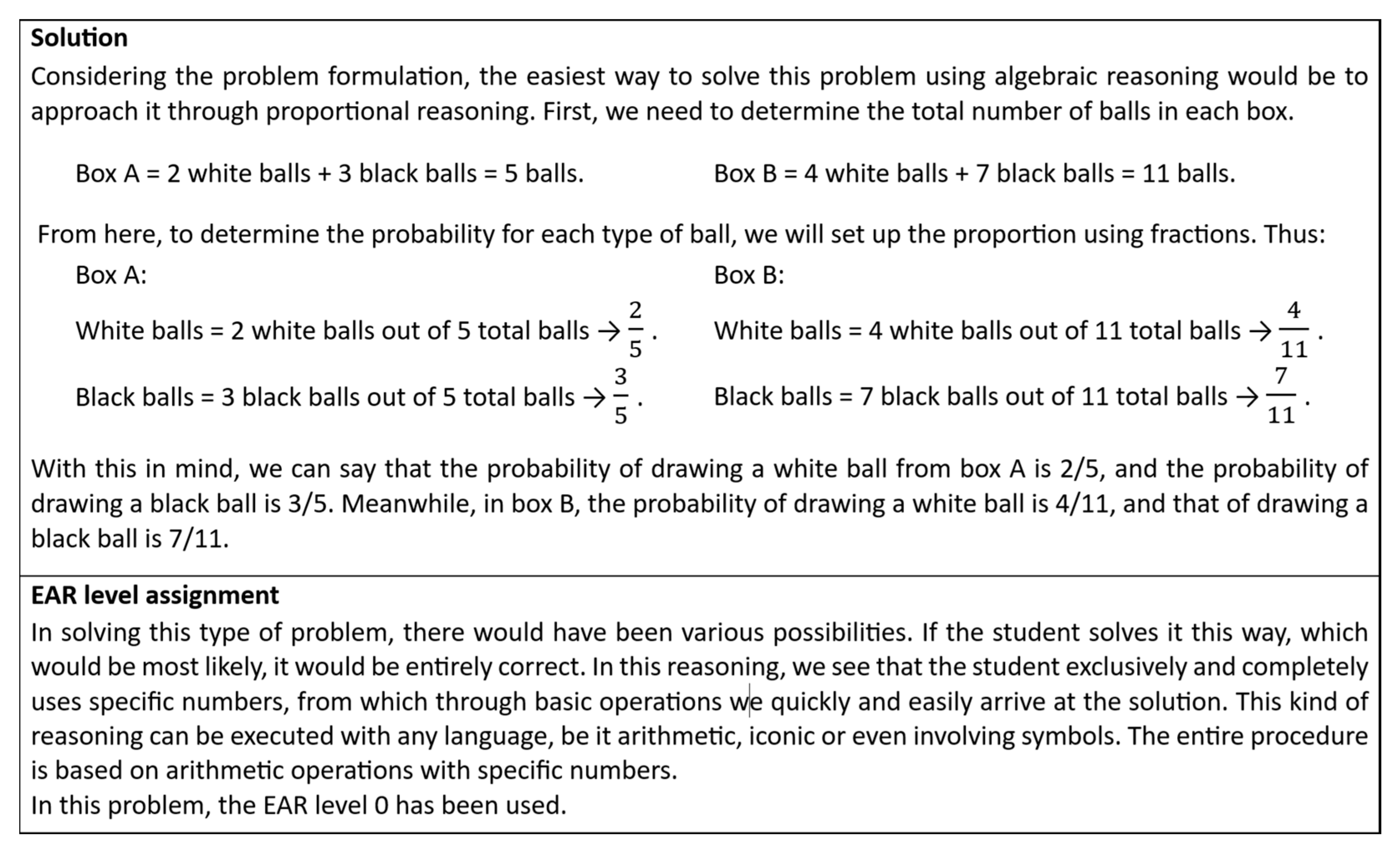


| Category | Fr. | |||
|---|---|---|---|---|
| PreR | PrePR | PreNR | ||
| IE | EE | |||
| CLP. Explicit calculation of the probability of drawing one or more balls in one or two boxes. | 0 | 0 | 6 | 1 |
| CMP. Explicit comparison of the probability of success in both boxes. | 4 | 1 | 0 | 0 |
| SEL. Selection of a box to perform a draw leading to a prize. | 4 | 0 | 0 | 0 |
| CCP. Explicit calculation and comparison of the probability of success in both boxes. | 0 | 1 | 0 | 0 |
| Type of Difficulties | Fr. | |||
|---|---|---|---|---|
| RD | PRD | NRD | Total | |
| D-PBR. Associated to probabilistic reasoning. | 11 | 1 | 1 | 13 |
| D-PPR. Related to proportional reasoning. | 7 | 1 | 2 | 10 |
| D-RN. Associated to the rational number. | 3 | 1 | 2 | 6 |
| D-G. Of a generic nature. | 0 | 6 | 2 | 8 |
| Category | Fr. | |||
|---|---|---|---|---|
| PosR | PosPR | PosNR | ||
| NoPre | NoEAR | |||
| DCP. Determination of the composition of a box from a known probability. | 7 | 0 | 1 | 0 |
| DCR. Determination of the composition of boxes knowing the ratio of white to black (or white to total) balls. | 3 | 0 | 3 | 0 |
| CAP. Explicit calculation of the simple or compound probability. | 5 | 0 | 2 | 0 |
| COP. Comparison of probabilities of drawing a ball from the two boxes. | 1 | 2 | 1 | 1 |
| EAR Level | Fr. | |||
|---|---|---|---|---|
| Increase | Decrease | |||
| Correct Id. | Incorrect Id. | Correct Id. | Incorrect Id. | |
| Level 0. | 0 | 0 | 0 | 4 |
| Level 1. | 4 | 4 | 1 | 3 |
| Level 2. | 4 | 2 | 0 | 0 |
| Level 3. | 0 | 2 | 0 | 0 |
| Higher levels. | 0 | 2 | 0 | 0 |
| Total. | 18 | 8 | ||
| Type of Difficulties | Fr. (%) | |||
| RD | PRD | NRD | Total | |
| D-A. | 14 (20.29) | 0 (0) | 0 (0) | 14 (20.29) |
| D-PBR. | 13 (18.84) | 0 (0) | 2 (2.90) | 15 (21.74) |
| D-PPR. | 13 (18.84) | 0 (0) | 3 (4.35) | 16 (23.19) |
| D-RN. | 10 (14.49) | 2 (2.90) | 3 (4.35) | 15 (21.74) |
| D-G. | 0 (0) | 2 (2.90) | 7 (10.14) | 9 (13.04) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tizón-Escamilla, N.; Burgos, M. Creation of Problems by Prospective Teachers to Develop Proportional and Algebraic Reasonings in a Probabilistic Context. Educ. Sci. 2023, 13, 1186. https://doi.org/10.3390/educsci13121186
Tizón-Escamilla N, Burgos M. Creation of Problems by Prospective Teachers to Develop Proportional and Algebraic Reasonings in a Probabilistic Context. Education Sciences. 2023; 13(12):1186. https://doi.org/10.3390/educsci13121186
Chicago/Turabian StyleTizón-Escamilla, Nicolás, and María Burgos. 2023. "Creation of Problems by Prospective Teachers to Develop Proportional and Algebraic Reasonings in a Probabilistic Context" Education Sciences 13, no. 12: 1186. https://doi.org/10.3390/educsci13121186
APA StyleTizón-Escamilla, N., & Burgos, M. (2023). Creation of Problems by Prospective Teachers to Develop Proportional and Algebraic Reasonings in a Probabilistic Context. Education Sciences, 13(12), 1186. https://doi.org/10.3390/educsci13121186






