Functional Thinking: A Study with 10th-Grade Students
Abstract
:1. Introduction
2. Functional Thinking
2.1. Functional Thinking as Part of Algebraic Reasoning
(1) recursive patterning involves finding variation within a sequence of values; (2) covariational thinking is based on analyzing how two quantities vary simultaneously and keeping that change as an explicit, dynamic part of a function’s description (e.g., “as increases by one, increases by three”); and (3) a correspondence relationship is based on identifying a correlation between variables (e.g., “ is 3 times plus 2”). (p. 8)
2.2. Functional Thinking and Students Learning about Functions
- Mapping: for each element x of the domain, there is a single element y of the range. This is a level of functional thinking that translates functions as a requirement to perform calculations.
- Covariation: the variation in the independent variable x and the resulting covariation of the dependent variable y. This describes the change in y if x changes uniformly.
- Function as object: this includes the recognition that functions offer the possibility of understanding the described process as a whole.
- -
- Recognize the correspondence between related variables independently of the representation used;
- -
- Determine the values of the dependent variable given the value of the independent one and vice versa;
- -
- Recognize the joint variation in the variables involved in a relation independently of the representation used;
- -
- Determine the interval of variation in one variable given the interval of variation in the other one;
- -
- Symbolize a functional relationship based on the analysis of the data of a problem.
3. Method
| Requested abilities | |
| Mapping | Calculate variable values. |
| Correspondence | Establish relationships between domain and range values. |
| Covariation | Relate the variation in the dependent variable as a function of the variation in the independent variable. |
| Function as object | Elaborate definitions.Operate with functions. |
4. Results
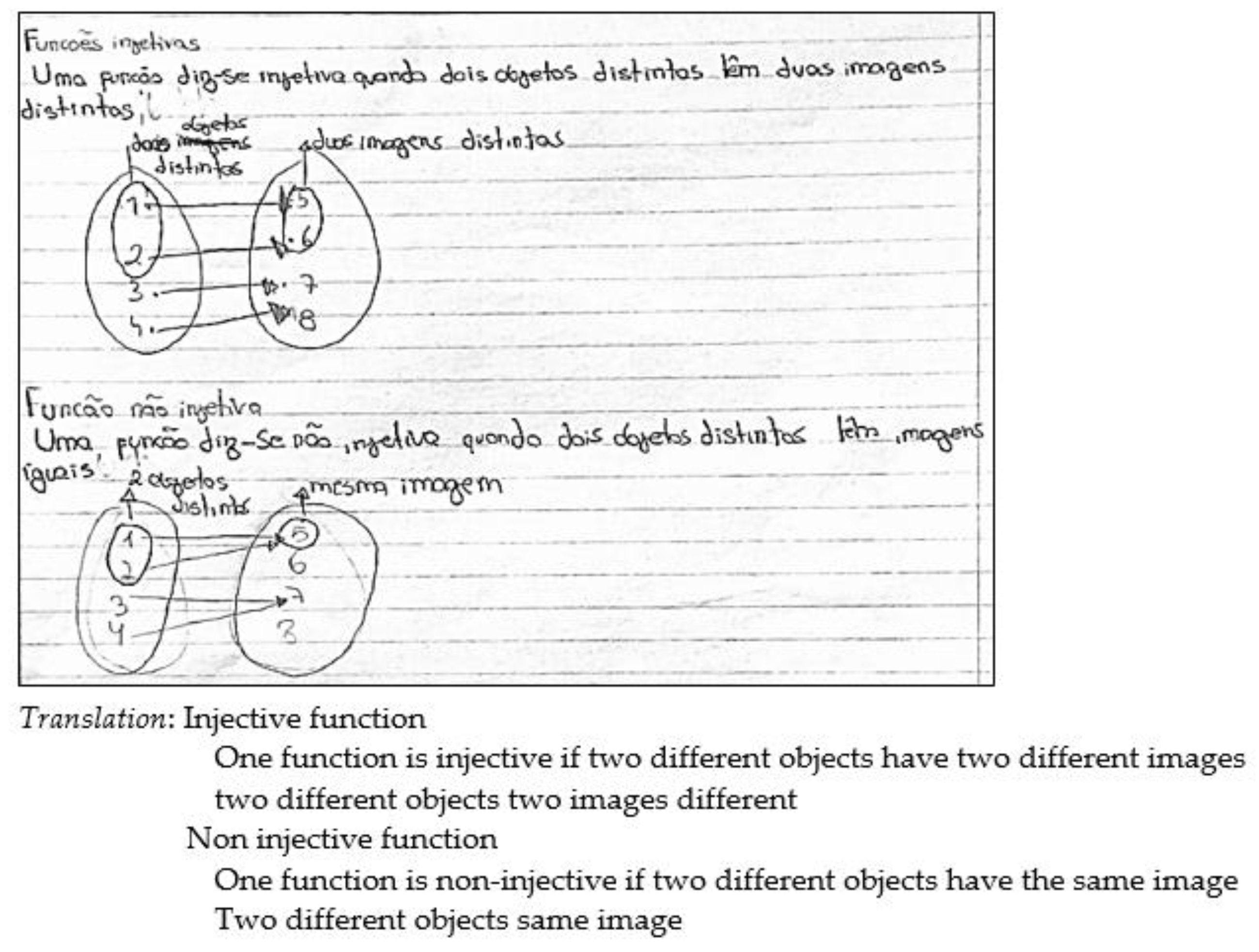
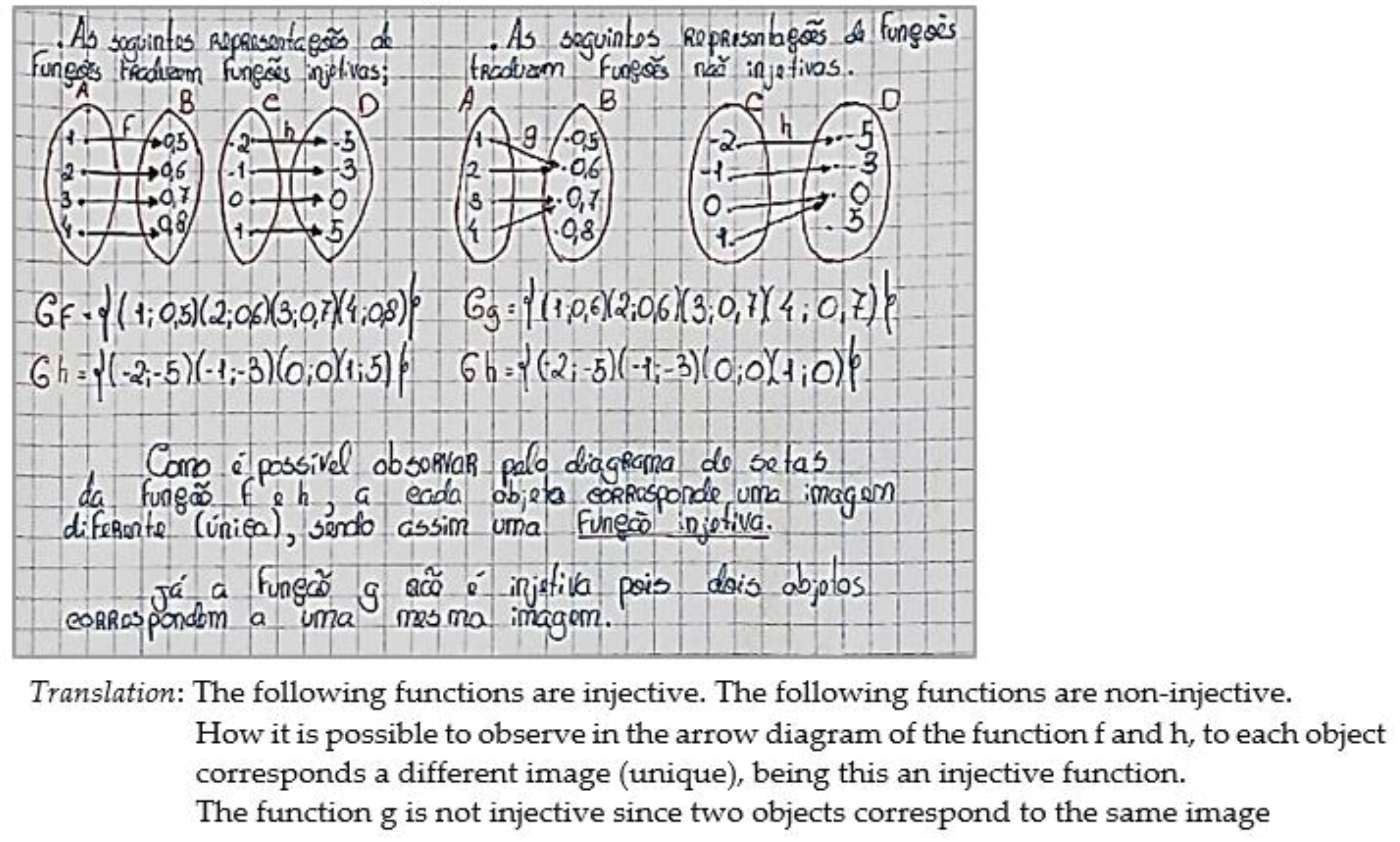
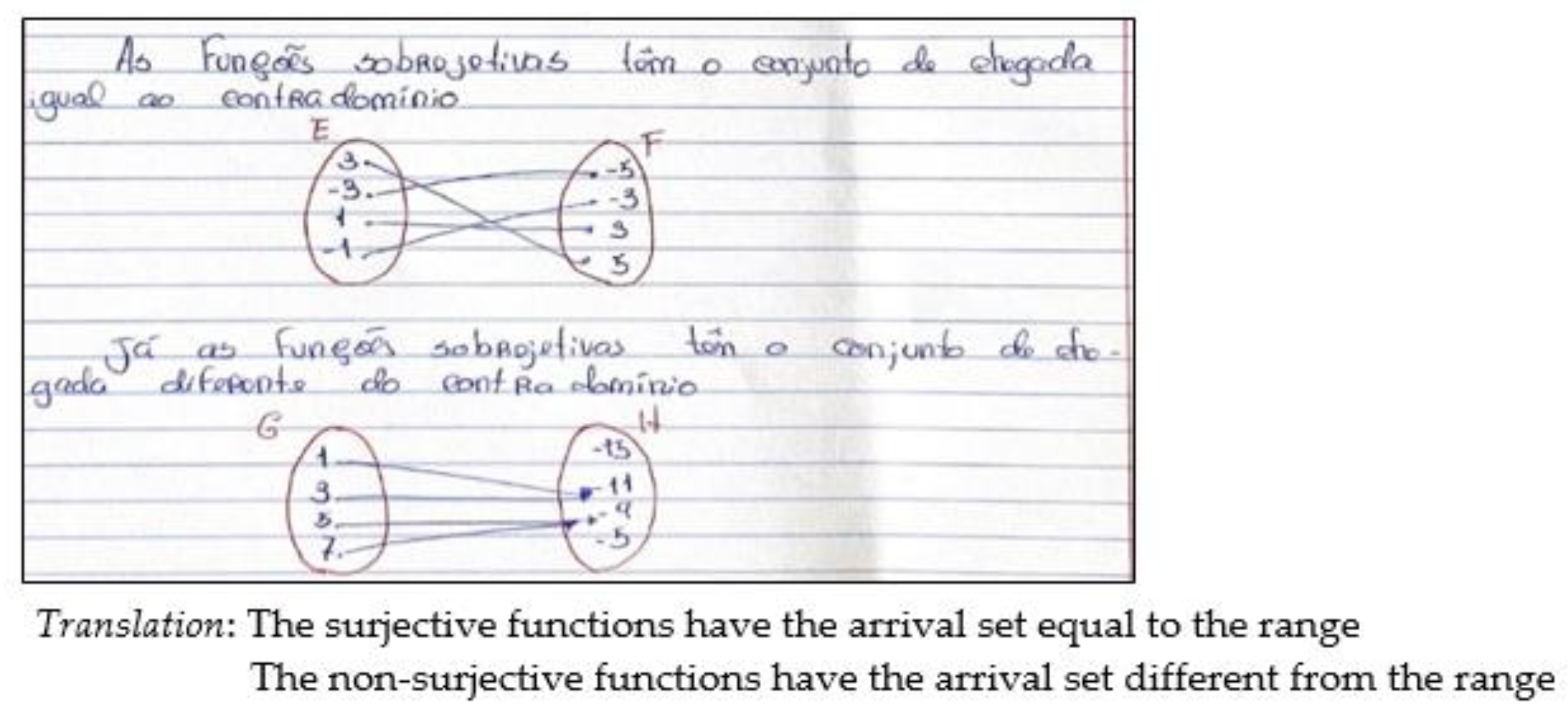
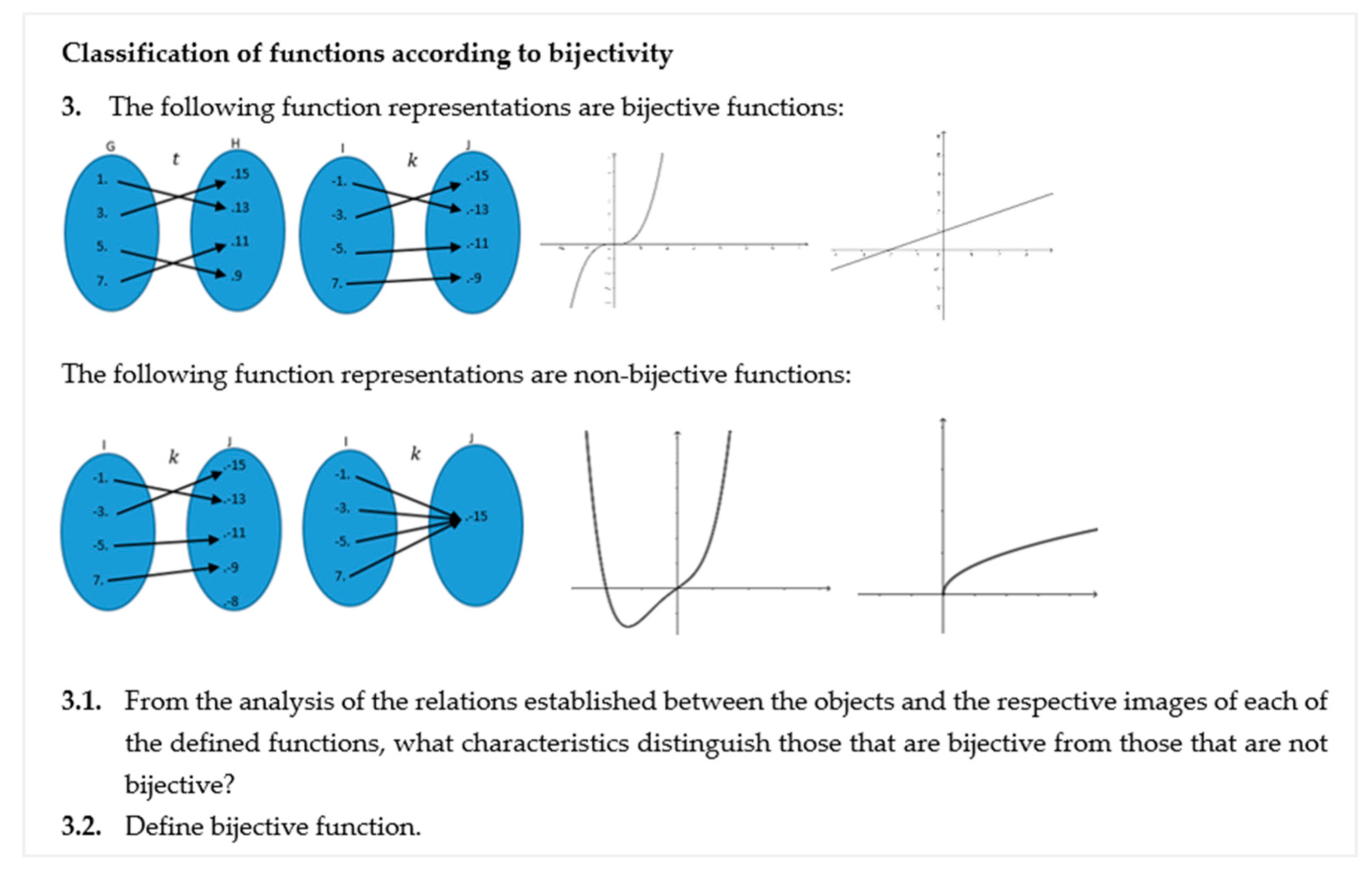
4.1. Task 1: Classification of Functions—Injectivity, Subjectivity, and Bijectivity
4.2. Task 2: Composite Function
- S16: We first find g(5); that is 25.
- S8: And f(25) is .
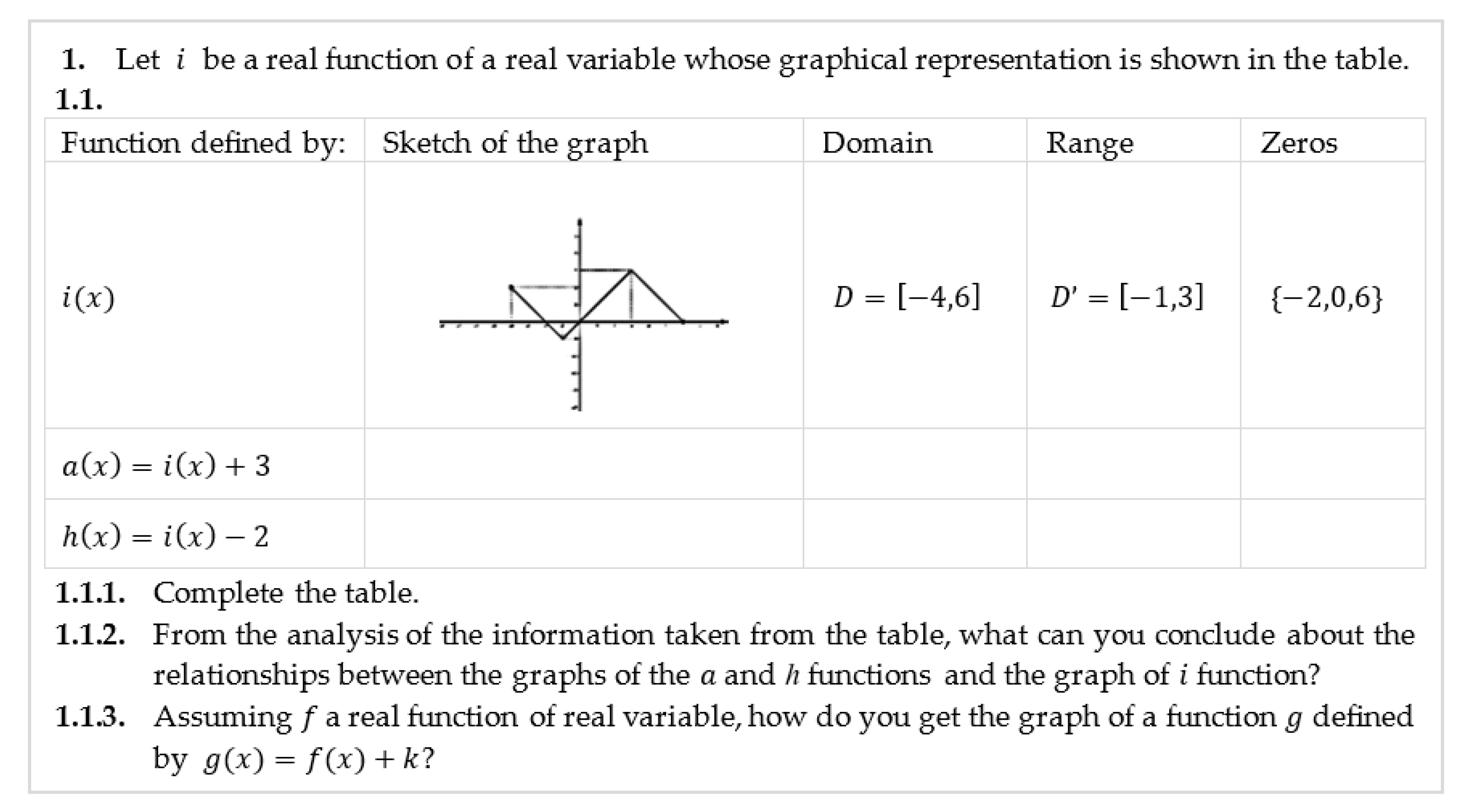
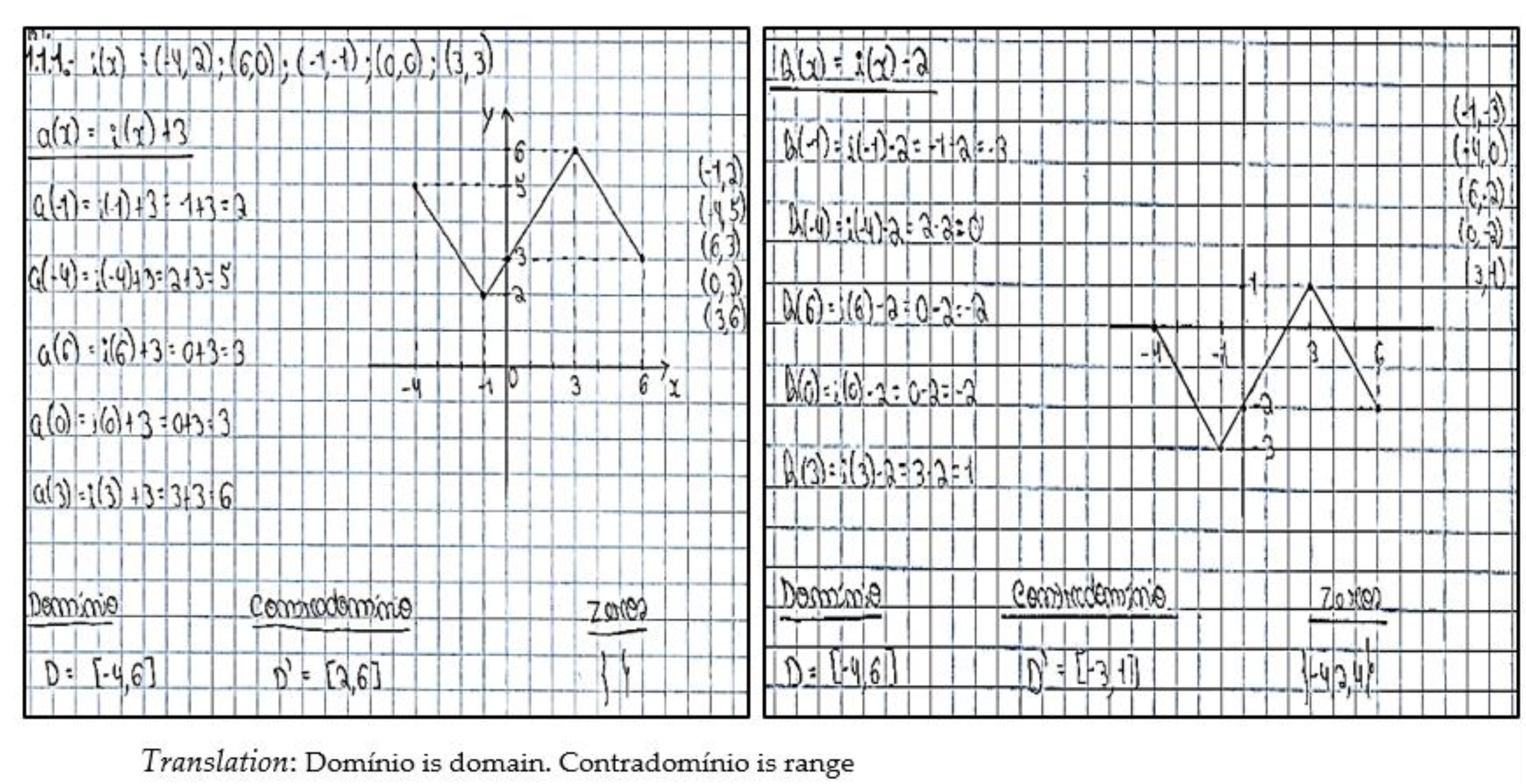
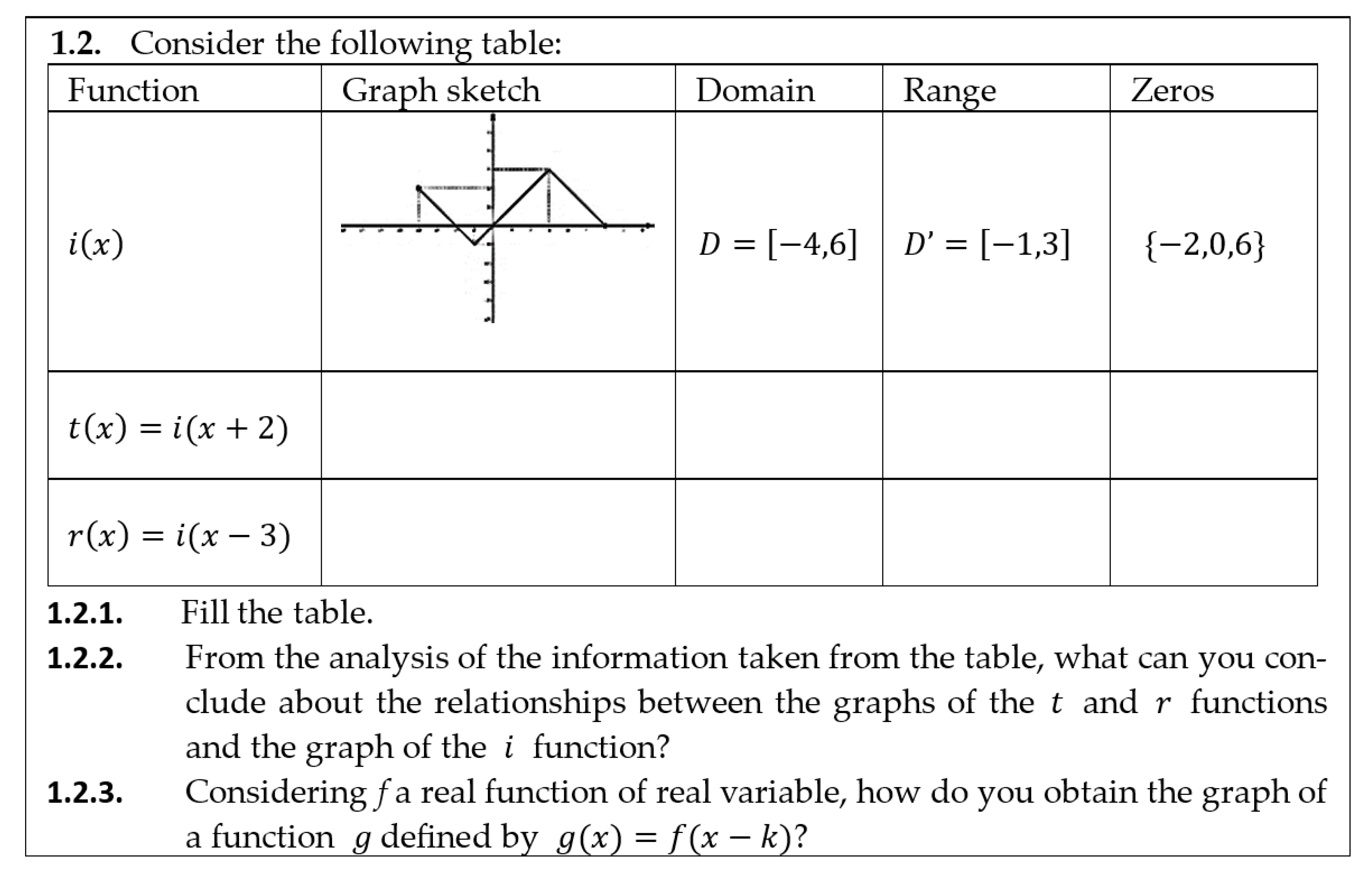
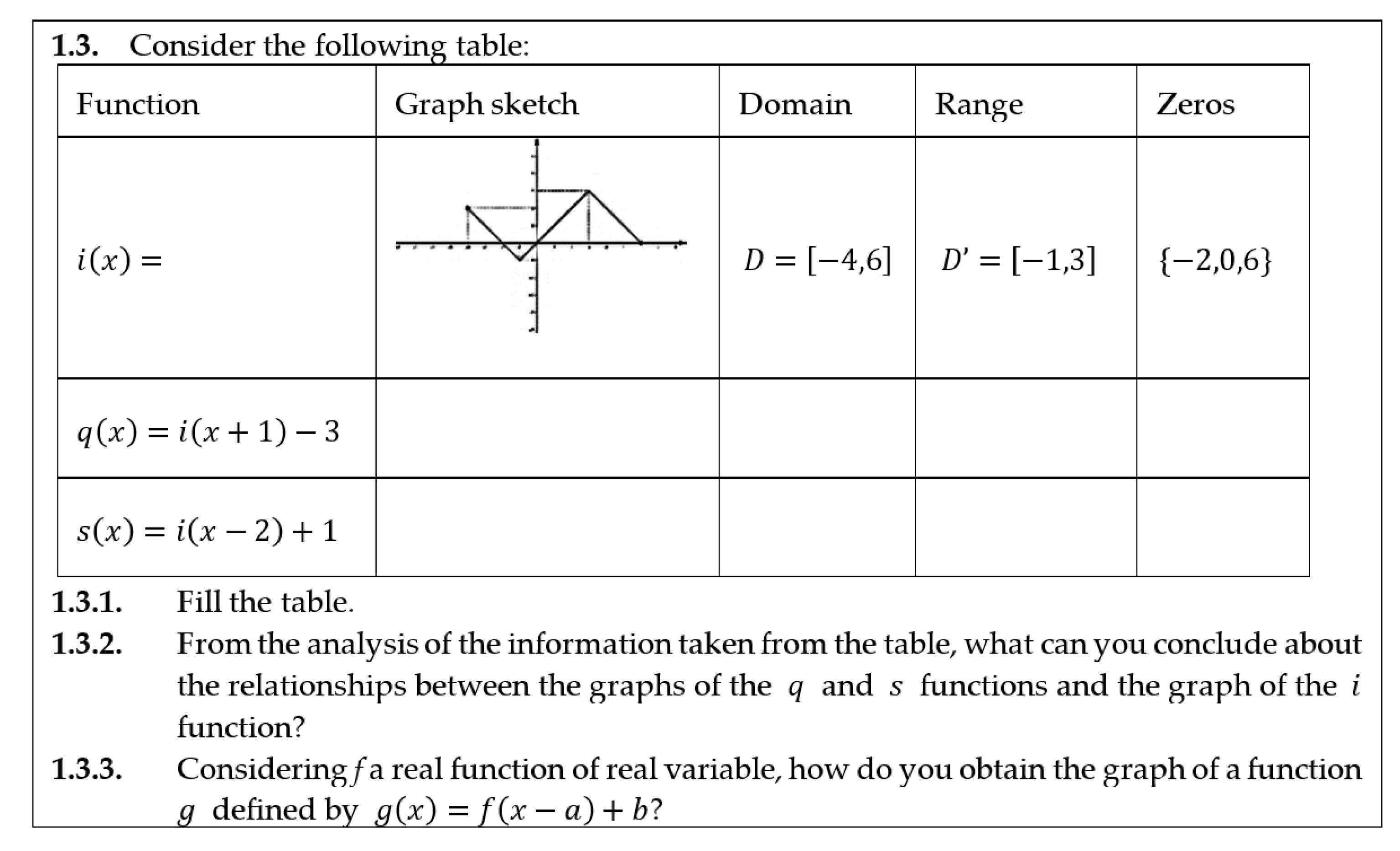
4.3. Task 3: Transformations of Functions’ Graphs
5. Conclusions
5.1. Characteristics of the Students Functional Thinking
5.2. Difficulties Revealed by the Students
5.3. Final Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- NCTM. Principles and Standards for School Mathematics; National Council of Teachers of Mathematics: Reston, VA, USA, 2002; ISBN 0-87353-480-8. [Google Scholar]
- Blanton, M. Algebra and the Elementary Classroom: Transforming Thinking, Transforming Practice; Heinemann: Portsmouth, UK, 2008. [Google Scholar]
- Lichti, M.; Roth, J. Functional Thinking—A Three-Dimensional Construct? J. Für Math. Didakt. 2019, 40, 169–195. [Google Scholar] [CrossRef]
- Mata-Pereira, J.; Ponte, J.P. Enhancing students’ mathematical reasoning in the classroom: Teacher actions facilitating generalization and justification. Educ. Stud. Math. 2017, 96, 169–186. [Google Scholar] [CrossRef]
- Akkus, R.; Hand, B.; Seymour, J. Understanding students’ understanding of functions. Math. Teach. 2008, 207, 10–13. [Google Scholar]
- Elia, I.; Panoura, A.; Eracleous, A.; Gagatsis, A. Relations between secondary pupils’ conceptions about functions and problem solving in different representations. Int. J. Sci. Math. Educ. 2007, 5, 533–556. [Google Scholar] [CrossRef]
- Rocha, H. Graphical representation of functions using technology: A window to teacher knowledge. Teach. Math. Its Appl. 2020, 39, 105–126. [Google Scholar] [CrossRef]
- Vollrath, H.J. Search strategies as indicators of functional thinking. Educ. Stud. Math. 1986, 17, 387–400. [Google Scholar] [CrossRef]
- Lichti, M.; Roth, J. How to foster functional thinking in learning environments using computer based simulations or real materials. J. STEM Educ. Res. 2018, 1, 148–172. [Google Scholar] [CrossRef]
- Doorman, M.; Drijvers, P.; Gravemeijer, K.; Boon, P.; Reed, H. Tool use and the development of the function concept: From repeated calculations to functional thinking. Int. J. Sci. Math. Educ. 2012, 10, 1243–1267. [Google Scholar] [CrossRef] [Green Version]
- Pittalis, M.; Pitta-Pantazi, D.; Christou, C. Young students’ functional thinking modes: The relation between recursive patterning, covariational thinking, and correspondence relations. J. Res. Math. Educ. 2020, 51, 631–674. [Google Scholar] [CrossRef]
- Wilkie, K.J.; Clarke, D.M. Developing students’ functional thinking in algebra through different visualisations of a growing pattern’s structure. Math. Educ. Res. J. 2016, 28, 223–243. [Google Scholar] [CrossRef] [Green Version]
- Smith, E. Representational thinking as a framework for introducing functions in the elementary curriculum. In Algebra in the Early Grades, 1st ed.; Kaput, J.J., Carraher, D.W., Blanton, M., Eds.; Routledge: New York, NY, USA, 2008; pp. 133–160. [Google Scholar] [CrossRef]
- Leinhardt, G.; Zaslavsky, O.; Stein, M.K. Functions, Graphs, and Graphing: Tasks, Learning, and Teaching. Rev. Educ. Res. 1990, 60, 1–64. [Google Scholar] [CrossRef]
- Viseu, F.; Silva, A.; Rocha, H.; Martins, P.M. A representação gráfica na aprendizagem de funções por alunos do 10.º ano de escolaridade. Educ. Mat. 2022, 34, 186–213. [Google Scholar] [CrossRef]
- Steketee, S.; Scher, D. Using multiple representations to teach composition of functions. Math. Teach. 2012, 106, 260–268. [Google Scholar] [CrossRef]
- Markovits, Z.; Eylon, B.; Bruckheimer, M. Difficulties students have with the function concept. In The Ideas of Algebra, K-12; Coxford, A.F., Shulte, A.P., Eds.; National Council of Teachers of Mathematics: Reston, VA, USA, 1988; pp. 43–60. [Google Scholar]
- Jeannotte, D.; Kieran, C. A conceptual model of mathematical reasoning for school mathematics. Educ. Stud. Math. 2017, 96, 1–16. [Google Scholar] [CrossRef] [Green Version]
- Carraher, D.W.; Schliemann, A.D. Early algebraic thinking and the US mathematics standards for grades K to 5. Infanc. Y Aprendiz. 2019, 42, 479–522. [Google Scholar] [CrossRef] [Green Version]
- Kusumaningsih, W.; Darhim, D.; Herman, T.; Turmudi, T. Improvement algebraic thinking ability using multiple representation strategy on realistic mathematics education. J. Math. Educ. 2018, 9, 281–290. [Google Scholar] [CrossRef]
- Stephens, A.C.; Ellis, A.B.; Blanton, M.; Brizuela, B.M. Algebraic thinking in the elementary and middle grades. In Compendium for Research in Mathematics Education; Cai, J., Ed.; National Council of Teachers of Mathematics: Reston, VA, USA, USA, 2017; pp. 386–410. [Google Scholar]
- Kaput, J.J. What is algebra? What is algebraic reasoning. In Algebra in the Early Grades, 1st ed.; Kaput, J.J., Carraher, D.W., Blanton, M., Eds.; Routledge: New York, NY, USA, 2008; pp. 5–17. [Google Scholar] [CrossRef]
- Blanton, M.; Kaput, J.J. Characterizing a classroom practice that promotes algebraic reasoning. J. Res. Math. Educ. 2005, 36, 412–446. [Google Scholar]
- Blanton, M.; Kaput, J.J. Functional thinking as a route into algebra in the elementary grades. In Early Algebraization, Advances in Mathematics Education; Cai, J., Knuth, E., Eds.; Springer: Berlin/Heidelberg, Germany, 2011; pp. 5–23. [Google Scholar] [CrossRef]
- Kieran, C.; Pang, J.; Schifter, D.; Ng, S.F. Early Algebra. Research into Its Nature, Its Learning, Its Teaching; Springer Nature: Cham, Switzerland, 2016. [Google Scholar] [CrossRef] [Green Version]
- Radford, L. Iconicity and contraction: A semiotic investigation of forms of algebraic generalizations of patterns in different contexts. ZDM 2008, 40, 83–96. [Google Scholar] [CrossRef]
- Warren, E. Generalising the pattern rule for visual growth patterns: Actions that support 8 year olds’ thinking. Educ. Stud. Math. 2008, 67, 171–185. [Google Scholar] [CrossRef]
- Ursini, S.; Trigueros, M. A model for the uses of variable in elementary algebra. In Proceedings of the 25th Conference of the International Group for the Psychology of Mathematics Education, Utrecht, The Netherlands, 12–17 July 2001; van den Heuvel-Panhuizen, M., Ed.; Utrecht University: Utrecht, The Netherlands, 2001; Volume 4, pp. 327–334. [Google Scholar]
- Apsari, R.A.; Putri, R.I.I.; Sariyasa, S.; Abels, M.; Prayitno, S. Geometry representation to develop algebraic thinking: A recommendation for a pattern investigation in pre-algebra class. J. Math. Educ. 2019, 11, 45–58. [Google Scholar] [CrossRef] [Green Version]
- Blanton, M.; Levi, L.; Crites, T.; Dougherty, B. Developing Essential Understanding of Algebraic Thinking for Teaching Mathematics in Grades 3–5; Essential Understanding Series; Zbiek, R.M., Ed.; National Council of Teachers of Mathematics: Reston, VA, USA, 2011. [Google Scholar]
- Cyrino, M.C.C.T.; Oliveira, H.M. Pensamento algébrico ao longo do ensino básico em Portugal. Bolema 2011, 24, 97–126. [Google Scholar]
- Kieran, C. The learning and teaching of school algebra. In Handbook of Research on Mathematics Teaching and Learning; Grouws, D.A., Ed.; Macmillan Publishing Co., Inc.: New York, NY, USA, 1992; pp. 390–419. [Google Scholar]
- Kaput, J.J. Teaching and learning a new algebra. In Mathematics Classrooms That Promote Understanding; Fennema, E., Romberg, T., Eds.; Lawrence Erlbaum Associates: Mahwah, NJ, USA, 1999; pp. 133–135. [Google Scholar]
- Smith, E. Stasis and change: Integrating patterns, functions, and algebra throughout the K-12 curriculum. In A Research Companion to Principles and Standards for School Mathematics; Kilpatrick, J., Martin, W.G., Schifter, D., Eds.; National Council of Teachers of Mathematics: Reston, VA, USA, 2003; pp. 136–150. [Google Scholar]
- Heyd-Metzuyanim, E. The co-construction of learning difficulties in mathematics—Teacher–student interactions and their role in the development of a disabled mathematical identity. Educ. Stud. Math. 2013, 83, 341–368. [Google Scholar] [CrossRef]
- Rocha, H. Pre-service teachers’ knowledge and the use of different technologies to teach Mathematics. In Perspectives and Trends in Education and Technology; Mesquita, A., Abreu, A., Carvalho, J., Eds.; Springer: Singapore, 2022; pp. 505–515. [Google Scholar] [CrossRef]
- Stein, M.K.; Smith, M.S. Mathematical tasks as a framework for reflection: From research to practice. Math. Teach. Middle Sch. 1998, 3, 268–275. [Google Scholar] [CrossRef]
- Ponte, J.P. Gestão curricular em Matemática. In O Professor e o Desenvolvimento Curricular; GTI, Ed.; Associação de Professores de Matemática: Lisbon, Portugal, 2005; pp. 11–34. [Google Scholar]
- McMillan, J.H.; Schumacher, S. Research in Education Evidence-Based Inquiry, 7th ed.; Pearson Education Limited: London, UK, 2014. [Google Scholar]
- Rodrigues, A. O Raciocínio Funcional de Alunos de 8.º ano na Resolução de Tarefas. Master’s Thesis, Universidade de Lisboa, Lisbon, Portugal, 2016. [Google Scholar]
- Ponte, J.P. The history of concept of function and some educational implications. Math. Educ. 1992, 3, 3–8. [Google Scholar]
- Panasuk, R.M. Three phase ranking framework for assessing conceptual understanding in algebra using multiple representations. Education 2010, 13, 235–257. [Google Scholar]
- Ponte, J.P.; Branco, N.; Matos, A. Álgebra no Ensino Básico; Ministério da Educação—Direção Geral de Inovação e de Desenvolvimento Curricular: Lisbon, Portugal, 2009.





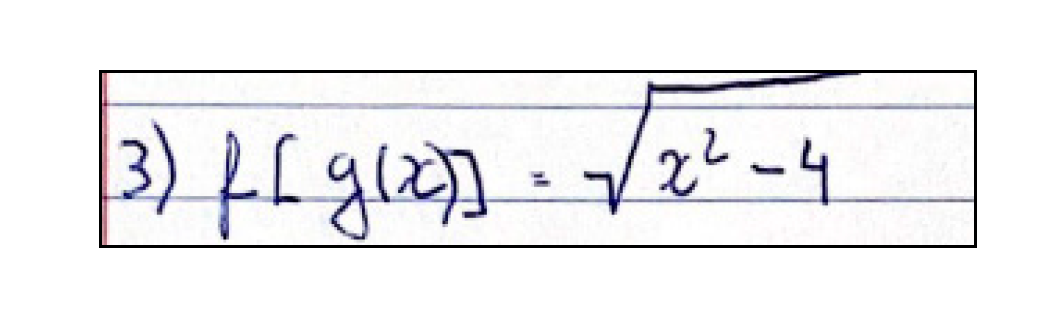






| Lesson | Mathematical Focus of the Tasks | Tasks Addressed |
|---|---|---|
| 1 | Review of concepts about functions | |
| 2 | Generalities related to the concept of function | |
| 3 | Classification of functions—injectivity, subjectivity, and bijectivity | √ |
| 4 | Composite function | √ |
| 5 | Inverse function | |
| 6 | Even and odd functions | |
| 79 | Transformations of function graphs | √ |
| 10 | Monotony and extremes of a function |
| Question | 1 | 2 | 3 | |||
|---|---|---|---|---|---|---|
| 1.1 | 1.2 | 2.1 | 2.2 | 3.1 | 3.2 | |
| (i) Mapping | – | – | – | – | – | – |
| (ii) Correspondence | √ | √ | √ | √ | √ | √ |
| (iii) Covariation | – | – | – | – | – | – |
| (iv) Function as object | √ | √ | √ | √ | √ | √ |
| Question | 1 | 2 | 3 |
|---|---|---|---|
| (i) Mapping | – | √ | √ |
| (ii) Correspondence | – | √ | √ |
| (iii) Covariation | – | – | – |
| (iv) Function as object | √ | √ | √ |
| Question | 1.1 | 1.2 | 1.3 | 1.4 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 1 | 2 | 3 | 1 | 2 | 3 | 1 | 2 | 3 | 4 | |
| (i) Mapping | √ | – | – | √ | – | – | √ | – | – | √ | – | – | – |
| (ii) Correspondence | √ | – | – | √ | – | – | √ | – | – | √ | – | √ | √ |
| (iii) Covariation | √ | √ | √ | √ | √ | √ | √ | √ | √ | √ | √ | √ | √ |
| (iv) Function as object | √ | √ | √ | √ | √ | √ | √ | √ | √ | √ | √ | √ | √ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martins, R.; Viseu, F.; Rocha, H. Functional Thinking: A Study with 10th-Grade Students. Educ. Sci. 2023, 13, 335. https://doi.org/10.3390/educsci13040335
Martins R, Viseu F, Rocha H. Functional Thinking: A Study with 10th-Grade Students. Education Sciences. 2023; 13(4):335. https://doi.org/10.3390/educsci13040335
Chicago/Turabian StyleMartins, Raquel, Floriano Viseu, and Helena Rocha. 2023. "Functional Thinking: A Study with 10th-Grade Students" Education Sciences 13, no. 4: 335. https://doi.org/10.3390/educsci13040335
APA StyleMartins, R., Viseu, F., & Rocha, H. (2023). Functional Thinking: A Study with 10th-Grade Students. Education Sciences, 13(4), 335. https://doi.org/10.3390/educsci13040335






