Development and Experimentation of a New Mathematical Model for Teaching–Learning the Radioactive Decay Law
Abstract
:1. Introduction
2. Problematic
3. Research Paradigm
3.1. Theoretical Reference Concepts
3.2. Didactic Engineering
4. Methodology
4.1. Characteristics of Experiment
4.2. Research Questions
- What causal relationships exist between the Moroccan curricular orientations, and the blockages observed during the teaching–learning of the phenomenon of radioactive decay?
- Where are the degrees of difficulties related to the teaching and learning of radioactive decay situated, and where are those related to the mobilization of mathematics?
- What solutions are we proposing? On which theoretical and organizational pillars are we based to verify that these solutions are good alternatives?
4.3. Research Hypotheses
- We believe that the mobilization of ICT increases greatly the success of teaching and learning of radioactive decay.
- We believe that the mobilization of mathematics, in their frame of intelligibility, facilitates the linking of registers and frames, and considerably attenuates the difficulties of the teaching–learning of radioactive decay.
- We believe that ModLI is an alternative to the blockage observed in the teaching and learning of radioactive decay, under the current Moroccan official instructions.
- We believe that collaborative work greatly attenuates the difficulties of teaching learning of radioactive decay. Even more, in groups with rotating members.
4.4. ModLI Mathematical Elaboration
4.4.1. Approximate expression of the population number N(t)
4.4.2. Approximate Expression of the Time in the Interval
5. Preliminary Study
5.1. Didactic Observartions
- In terms of reality space: Discuss the influence of ICT mobilization on the activity of production and apprehension of different radioactive decay models, knowing that it is impossible to realize real classroom experiments;
- In terms of register: Check the degree of control of the pupils to pass between different registers, by their capacities to look for the numerical regularities and to transpose them in graphic representations, and by their capacities to establish the analytical expression reflecting the radioactive decay law;
- In terms of organization of work: Analyze the influence of the alternation between individual work and collaborative work, on the teaching and learning of mathematical modeling of phenomena in physics.
5.2. Target Level
5.3. Intervention Modalities
5.4. Experimentation
5.4.1. Presentation of Our Created ICT Resource
5.4.2. Test 1
5.4.3. Test 2
5.4.4. Conclusions
6. Validation Study
6.1. Intervention Modalities
6.2. Experimentation Results and Discussion
6.2.1. Existing Models of the Radioactive Decay Law
6.2.2. Test 3 (Part 1)
6.2.3. Test 3 (Part 2)
6.2.4. Pre-Test 4
6.2.5. Post-test 4
7. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Lévy-Leblond, J.-M. Physique et Mathématique, Encyclopædia Universalis, Consulté le 12 août. 2014. Available online: http://www.universalis.fr/encyclopedie/physique-physique-et-mathematique/ (accessed on 12 August 2014).
- Kuhn, T.S. Tradition mathématique et tradition expérimentale dans le développement de la physique. Ann. Hist. Sci. Soc. 1975, 30, 975–998. [Google Scholar]
- Kastler, M.A. Ampère et les lois de l’électrodynamique. Rev. Hist. Sci. Paris 1977, 2, 143–157. [Google Scholar] [CrossRef]
- Bensaude Vincent, B. Paul Paul Langevin: L’histoire des sciences comme remède à tout dogmatisme/Paul Langevin: History of science as a remedy for every dogmatism Langevin: L’histoire des sciences comme remède à tout dogmatisme/Paul Langevin: History of science as a remedy for every dogmatism. Rev. Hist. Sci. Paris 2005, 58, 311–328. [Google Scholar]
- Rosmorduc, J. L’histoire de la physique peut-elle eclairer les obstacles epistemologiques? Aster 1987, 5, 117–142. [Google Scholar] [CrossRef]
- Mayrargue, A.; Savaton, P. Quels liens entre l’histoire des sciences, l’épistémologie et la didactique des disciplines? Tréma 2006, 26. [Google Scholar] [CrossRef]
- Martinand, J. Histoire et didactique de la physique et de la chimie: Quelles relations? Didaskalia 1993, 2, 89–99. [Google Scholar] [CrossRef]
- Duval, R. Quel cognitif retenir en didactique des mathématiques. Rech. Didact. Math. 1996, 16, 349–382. [Google Scholar]
- Malafosse, D.; Lerouge, A.; Dusseau, J. Étude en inter-didactíque des mathématiques et de la physique de l’ acquisition de la loi d’ Ohm au collège: Espace de réalité. Didaskalia 2000, 16, 81–106. [Google Scholar]
- Mastafi, M. Intégration et usages des TIC dans le système éducatif marocain: Attitudes des enseignants de l’enseignement primaire et secondaire. Adjectif. Net Mis en ligne dimanche 28 avril 2013 [En ligne]. Available online: http://www.adjectif.net/spip/spip.php?article228 (accessed on 1 June 2019).
- Mamane, A.; Benjelloun, N. Study of the Dependence between the Teaching- Learning of Physics and the ICT Resources available to Pupils in Moroccan High Schools. IJEIS Int. J. Educ. Inf. Stud. 2018, 8, 1–15. [Google Scholar]
- Conseil Supérieur de l’Éducation de la Formation et de la Recherche Instance Nationale d’Evaluation du Système d’Education de Formation et de la Recherche Scientifique. Royaume du Maroc Résultats des Elèves Marocains en Mathématiques et en Sciences dans un Contexte International; TIMSS 2015. Available online: http://www.csefrs.ma/wp-content/uploads/2018/06/TIMSS-Version-Fr-26-05-2018.pdf (accessed on 1 June 2019).
- Mamane, A.; Benjelloun, N. Mathematical modeling, ICT and collaborative work in Moroccan high schools: From ModLet to ModL2t, experimentation of a semiotic set in the teaching and learning of the radioactive decay law. In Proceedings of the Apel’2018 FST Fès: 3ème édition de la Conférence International sur les Approches Pédagogique et E-Learning, FST Fez, Morocco, 26–27 December 2018. [Google Scholar]
- Klein, L.; Kagan, D. “ Radio-Active ” Learning: Visual Representation of Radioactive Decay Using Dice. Phys. Teach. 2010, 45, 5–6. [Google Scholar] [CrossRef]
- Jesse, K.E. Computer Simulation of Radioactive Decay. Phys. Teach. 2003, 542, 9–11. [Google Scholar] [CrossRef]
- Schultz, E. Dice-Shaking as an Analogy for Radioactive Decay and First-Order Kinetics. J. Chem. Educ. 1997, 74, 505–507. [Google Scholar] [CrossRef]
- Bakaç, M.; TaúR-lu, A.K.; Uyumaz, G. Modeling radioactive decay. Procedia-Soc. Behav. Sci. 2011, 15, 2196–2200. [Google Scholar] [Green Version]
- Edge, R.D. Radioactive Decay-An Analogy. Phys. Teach. 1978, 445–458. Available online: https://aapt.scitation.org/doi/10.1119/1.2340024 (accessed on 1 June 2019). [CrossRef]
- Hughes, E.A.; Zalts, A. Radioactivity in the Classroom. J. Chem. Educ. 2000, 77, 6–7. [Google Scholar] [CrossRef]
- Lapp, D.R. Obtaining and Investigating Unconventional Sources of Radioactivity. Phys. Teach. 2010, 90, 10–13. [Google Scholar] [CrossRef]
- Direction des programmes Ministère de l’éducation nationale et de l’enseignement supérieur et de la formation des cadres et de la recherche scientifique Royaume du Maroc. Instructions Pédagogiques et Programmes Spécifiques de L’enseignement de la Physique et de la Chimie au Cycle Secondaire Qualifiant. 2007. Available online: https://waliye.men.gov.ma/Ar/curriculum1/Documents/curr_progr%20Physique%20et%20Chimie.pdf (accessed on 1 June 2019).
- Direction des programmes Ministère de l’éducation nationale et de l’enseignement supérieur et de la formation des cadres et de la recherche scientifique Royaume du Maroc. Instructions Pédagogiques et Programmes Spécifiques de L’enseignement des Mathématiques au Cycle Secondaire Qualifiant. 2017. Available online: https://waliye.men.gov.ma/Ar/curriculum1/Documents/curr_progr%20Math%C3%A9matiques.pdf (accessed on 1 June 2019).
- Mamane, A.; Benjelloun, N. Mathematical modeling, ICT and collaborative work in Moroccan high schools: From ModLet to ModL2t, experimentation of a semiotic set in the teaching and learning of the radioactive decay law. IOSR J. Res. Method Educ. 2018, 8, 32–40. [Google Scholar]
- Artigue, M. Ingéniérie didactique. Vème École D’été Didact; Mathématiques Rennes; Des Mathématiques L’informatique Publ. L’institut Rech: Rennes, France, 1989; pp. 124–128. [Google Scholar]
- Duval, R. Registres de représentation sémiotique et fonctionnement cognitif de la pensée. Ann. Didact. Sci. Cogn. 1993, 5, 37–65. [Google Scholar]
- Malafosse, D.; Lerouge, A.; Dusseau, J. Étude en inter-didactíque des mathématiques et de la physique de l’acquisition de la loi d’Ohm au collège: Changement de cadre de rationalité. Didaskalia 2001, 18, 61–98. [Google Scholar] [CrossRef]
- Malafosse, D. Pour une formation inter-didactique Mathématiques-Physique des professeurs de collège et de lycée. Tréma 2003, 20–21, 187–209. [Google Scholar] [CrossRef]
- Malonga Moungabio, F.; Beaufils, D. Modélisation et registres sémiotiques: Exemple d’étude de manuels de physique de terminale. RDST 2010, 1, 293–316. [Google Scholar] [CrossRef]
- Martinand, J. Introduction à La Modélisation; INRP: Paris, France, 1987; Available online: http://www.inrp.fr/Tecne/Rencontre/Univete/Tic/Pdf/Modelisa.pdf (accessed on 1 June 2019).
- Douady, R. Ingénierie didactique et évolution du rapport au savoir. Repères IREM 1994, 15, 37–61. [Google Scholar]
- Marty, O. Cours Ingénierie Didactique; 2015 Université de Lille 1. 2015. Available online: https://halshs.archives-ouvertes.fr/cel-01260590/document (accessed on 1 June 2019).
- Droui, M. L’impact d’une Simulation sur des Dispositifs Mobiles et en Situation de Collaboration sur la Comprehension de L’effet Photoelectrique au Niveau Collegial; Université de Montréal: Montreal, QC, Canada, 2012; Volume NR98438. [Google Scholar]
- Charuau, J.; Labed, V.; Robe1, M.C.; Venin, J.C.; Aubert, C.; Fazileabasse, J.; Florence, D.; Gibaud, C.; Heleschewitz, H.; Klein, D.; et al. Manifestation la radioactivité du Radon 222 dans la nature. Radioprotection 1996, 31, 371–388. [Google Scholar] [CrossRef]
- Mermoz, L.J.; Louis, S.; Rhin, H. France Radioactivité et réactions nucléaires-TP activité du granite. Available online: http://physicus.free.fr/premiere_S/1S-CHAP-08-radioact-reac-nuc.php (accessed on 1 June 2019).
- CCNR. EDP-Sciences Famille radioactive de l’uranium 238., Canadian Coalition for Nuclear Responsibility, 53 Dufferin Road, Hampstead QC. Available online: http://www.ccnr.org/decay_U238_f.html (accessed on 1 June 2019).
- Ministère de l’éducation nationale et de la jeunesse. France Baccalauréat des Voies Générale et Technologique Épreuve de Physique-Chimie de Série S Physique, Enseignement Obligatoire: Décroissance Radioactive du Radon 220 Décroissance Radioactive Du Radon 220; Ministry of National Education, 2002; p. 7.
- Asp, A. Scolaire Personnalisée La décroissance Radioactive du Carbone 14. Available online: https://www.assistancescolaire.com/enseignant/elementaire/ressources/base-documentaire-en-sciences/la-decroissance-radioactive-du-carbone-14-t_t103i03 (accessed on 1 June 2019).
- Mamane, A.; Benjelloun, N. Modélisations mathématiques, TICE et travail collaboratif dans l’enseignement-apprentissage de la décroissance radioactive aux lycées du Maroc par le Modèle de Linéarisation par Intervalle ModLI”. In Proceedings of the Workshop LIRDIST Université Sidi Mohamed Ben Abdellah Fés, 6–7 July 2018. [Google Scholar]

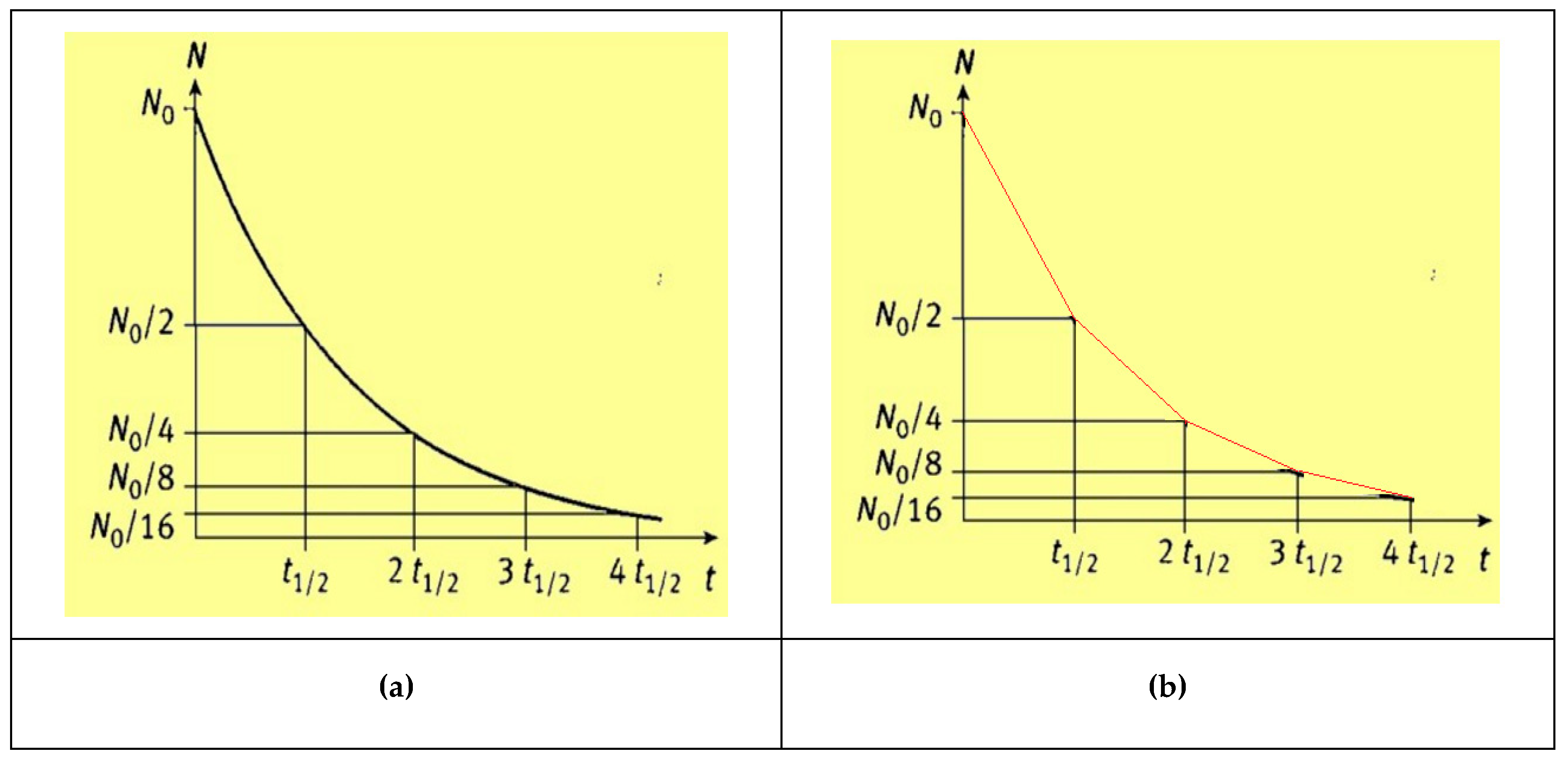
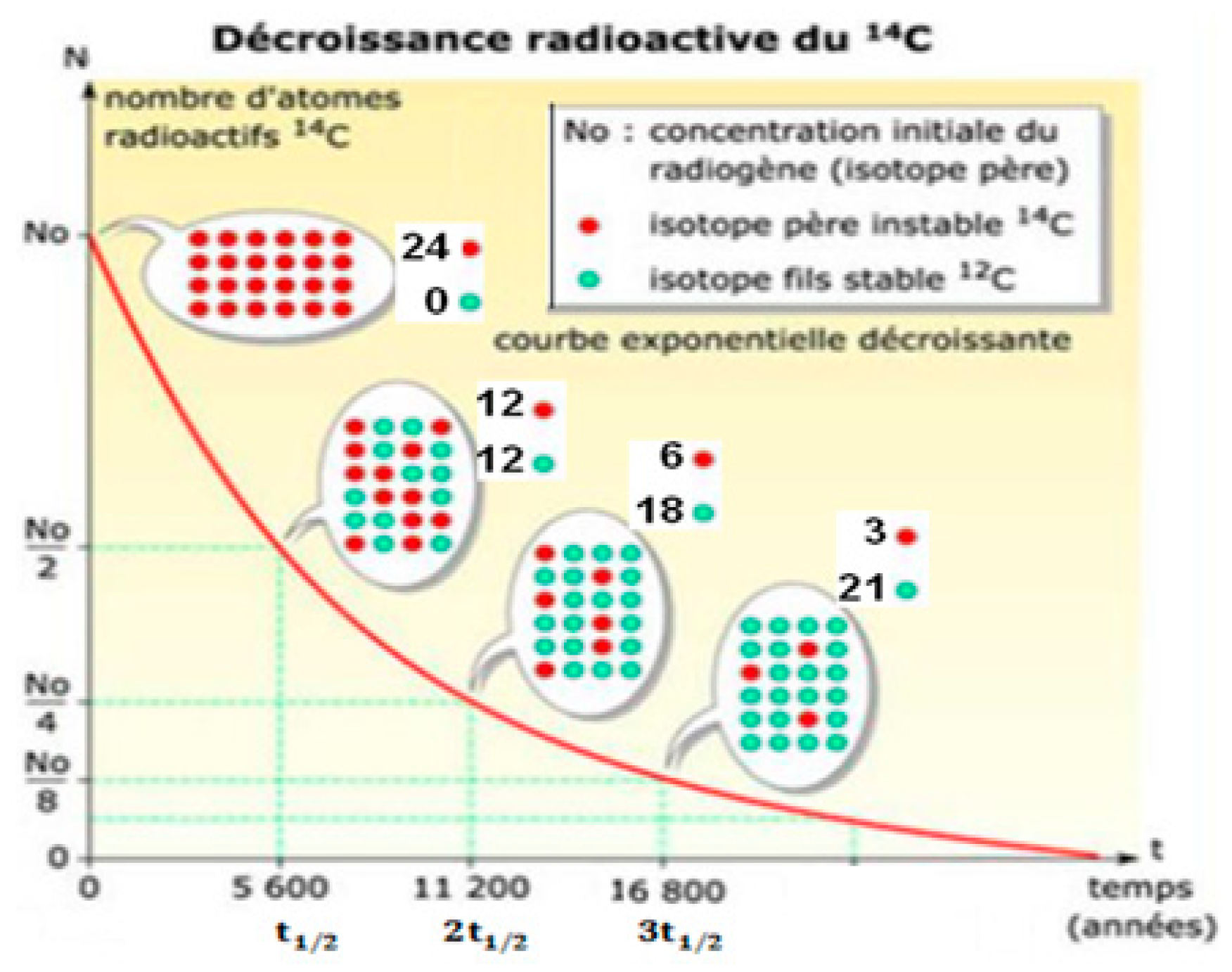

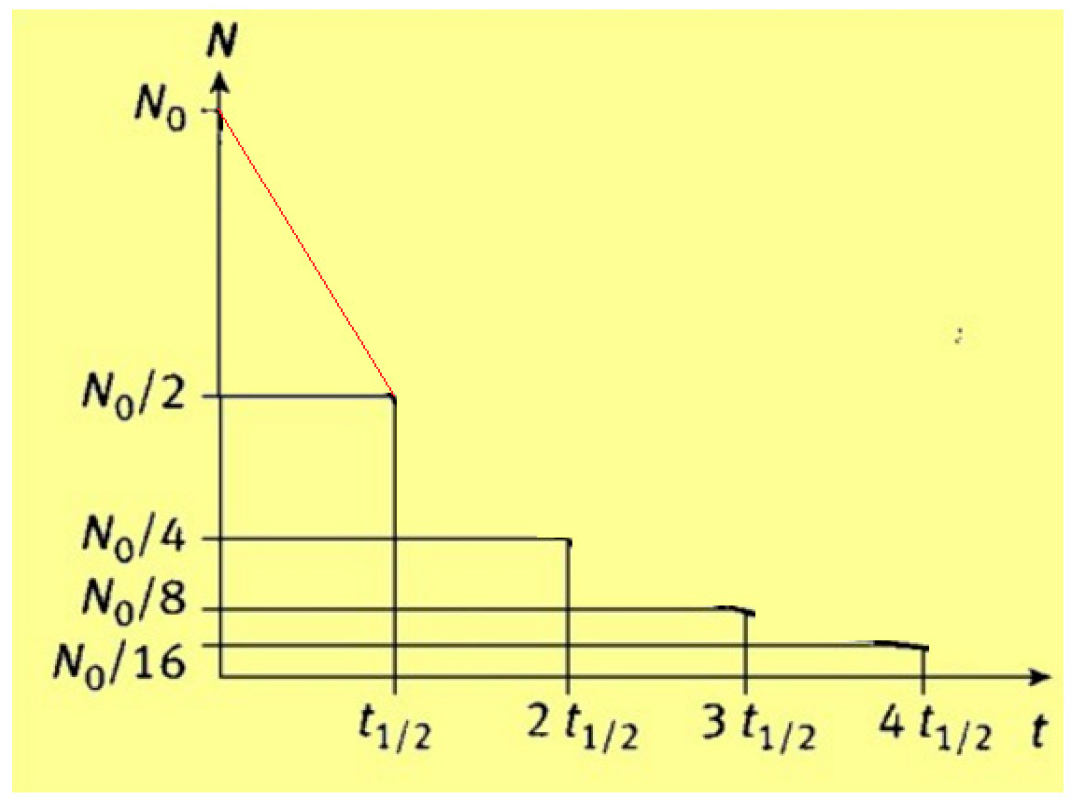



| Answers Analysis | Branch | Acceptable Rate Works | |||
|---|---|---|---|---|---|
| in Individual Configuration | in Intergroups with Rotating Members | ||||
| Plots of a landmark | PC | 100% | 100% | 100% | 100% |
| LES | 100% | 100% | |||
| Productions similar to curve in Chart 1a | PC | 85.7% | 75% | 100% | 95.2% |
| LES | 66.7% | 91.7% | |||
| Productions similar to curve in Chart 1b | PC | 85.7% | 81.2% | 100% | 100% |
| LES | 77.8% | 100% | |||
| Answers Analysis | Branch | Acceptable Rate Works | |||
|---|---|---|---|---|---|
| in Individual Configuration | in Intergroups with Rotating Members | ||||
| establishment of the expression of N(t) relative to the curve in Chart 1a | PC | 0% | 0% | 0% | 0% |
| LES | 0% | 0% | |||
| establishment of the first three expressions of N(t) relative to the curve in Chart 1b | PC | 57.1% | 37.5% | 88.9% | 71.4% |
| LES | 22.2% | 58.3% | |||
| general analytical model deduction for N(t) relative to the curve in Chart 1b | PC | 42.9% | 31.2% | 100% | 95.2% |
| LES | 22.2% | 91.7% | |||
| deduction of a general form of time intervals from the curve in Chart 1b | PC | 71.4% | 50% | 100% | 90.4% |
| LES | 33.3% | 83.3% | |||
| establishment of a general analytical model of time t for the curve in Chart 1b | PC | 85.7% | 75% | 100% | 100% |
| LES | 66.7% | 100% | |||
| (a) | |||||
| Analysis Elements | Branch | Response Frequencies | Frequency of No Response | Total | |
| Admitted | Not Admitted | ||||
| Deduction of the value of N(t) for time | PC | 89.47% | 10.53% | 0% | 100% |
| 100% | |||||
| LES | 67.57% | 24.32% | 8.11% | 100% | |
| 91.89% | |||||
| Total | 78.67% | 17.33% | 4% | 100% | |
| 96% | |||||
| Deduction of the value of N(t) for time | PC | 84.2% | 7.9% | 7.9% | 100% |
| 92.11% | |||||
| LES | 59.46% | 27.03% | 13.51% | 100% | |
| 86.49% | |||||
| Total | 72% | 17.33% | 10.67% | 100% | |
| 89.33% | |||||
| (b) | |||||
| Analysis Elements | Branch | Response Frequencies | Frequency of No Response | Total | |
| Admitted | Not Admitted | ||||
| Establishment of the analytic expression of N(t) for | PC | 68.42% | 23.68% | 7.9% | 100% |
| 92.11% | |||||
| LES | 43.24% | 24.32% | 32.43% | 100% | |
| 67.57% | |||||
| Total | 56% | 21.33% | 20% | 100% | |
| 77.33% | |||||
| Analysis Elements | Branch | Responses Frequency | Frequency of No Responses | Total | |
|---|---|---|---|---|---|
| Admitted | No admitted | ||||
| establishment of the expression of time t for n = 1 | PC | 36.84% | 60.53% | 2.63% | 100% |
| 97.37% | |||||
| SVT | 5.41% | 94.59% | 0% | 100% | |
| 100% | |||||
| Total | 21.33% | 77.33% | 1.33% | 100% | |
| 98.67% | |||||
| establishment of the expression of time t for n = 2 | PC | 44.74% | 50% | 5.26% | 100% |
| 94.74% | |||||
| LES | 5.41% | 81.08% | 13.51% | 100% | |
| 86.49% | |||||
| Total | 25.33% | 65.33% | 9.33% | 100% | |
| 90.67% | |||||
| establishment of the expression of time t for n = 3 | PC | 73.68% | 26.32% | 0% | 100% |
| 100% | |||||
| LES | 40.54% | 54.05% | 5.41% | 100% | |
| 94.59% | |||||
| Total | 57.33% | 40% | 2.67% | 100% | |
| 97.33% | |||||
| deduction of the expression for N(t) and t if n = 4 | PC | 71.05% | 18.42% | 10.63% | 100% |
| 89.47% | |||||
| LES | 67.57% | 29.73% | 2.7% | 100% | |
| 97.3% | |||||
| Total | 69.33% | 24% | 6.67% | 100% | |
| 93.33% | |||||
| deduction of the general expressions for N(t) and t | PC | 73.68% | 26.32% | 0% | 100% |
| 100% | |||||
| LES | 56.76% | 43.24% | 0% | 100% | |
| 100% | |||||
| Total | 65.33% | 34.67% | 0% | 100% | |
| 100% | |||||
| Analysis Elements | Frequency of Unlocking Situations by ModLI | Frequency of Blocking Situations | Total | |||
|---|---|---|---|---|---|---|
| Branch | Successful Use | Unsuccessful Use | Use of ModLet | No Response or Others | ||
| PC | 73.68% | 13.16% | 5.26% | 7.89% | 100% | |
| 86.84% | 13.16% | |||||
| LES | 72.97% | 10.81% | 8.11% | 8.11% | 100% | |
| 83.78% | 16.22% | |||||
| Total | 73.33% | 12% | 6.67% | 8% | 100% | |
| 85.33% | 14.67% | |||||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mamane, A.; Benjelloun, N. Development and Experimentation of a New Mathematical Model for Teaching–Learning the Radioactive Decay Law. Educ. Sci. 2019, 9, 123. https://doi.org/10.3390/educsci9020123
Mamane A, Benjelloun N. Development and Experimentation of a New Mathematical Model for Teaching–Learning the Radioactive Decay Law. Education Sciences. 2019; 9(2):123. https://doi.org/10.3390/educsci9020123
Chicago/Turabian StyleMamane, Adnane, and Nadia Benjelloun. 2019. "Development and Experimentation of a New Mathematical Model for Teaching–Learning the Radioactive Decay Law" Education Sciences 9, no. 2: 123. https://doi.org/10.3390/educsci9020123
APA StyleMamane, A., & Benjelloun, N. (2019). Development and Experimentation of a New Mathematical Model for Teaching–Learning the Radioactive Decay Law. Education Sciences, 9(2), 123. https://doi.org/10.3390/educsci9020123





