Gum Arabic-Magnetite Nanocomposite as an Eco-Friendly Adsorbent for Removal of Lead(II) Ions from Aqueous Solutions: Equilibrium, Kinetic and Thermodynamic Studies
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Preparation of GA/MNPs
2.3. Characterization of the Prepared Composite
2.4. Adsorption Experiments
2.5. Kinetic Studies
2.6. Adsorption Isotherms Models
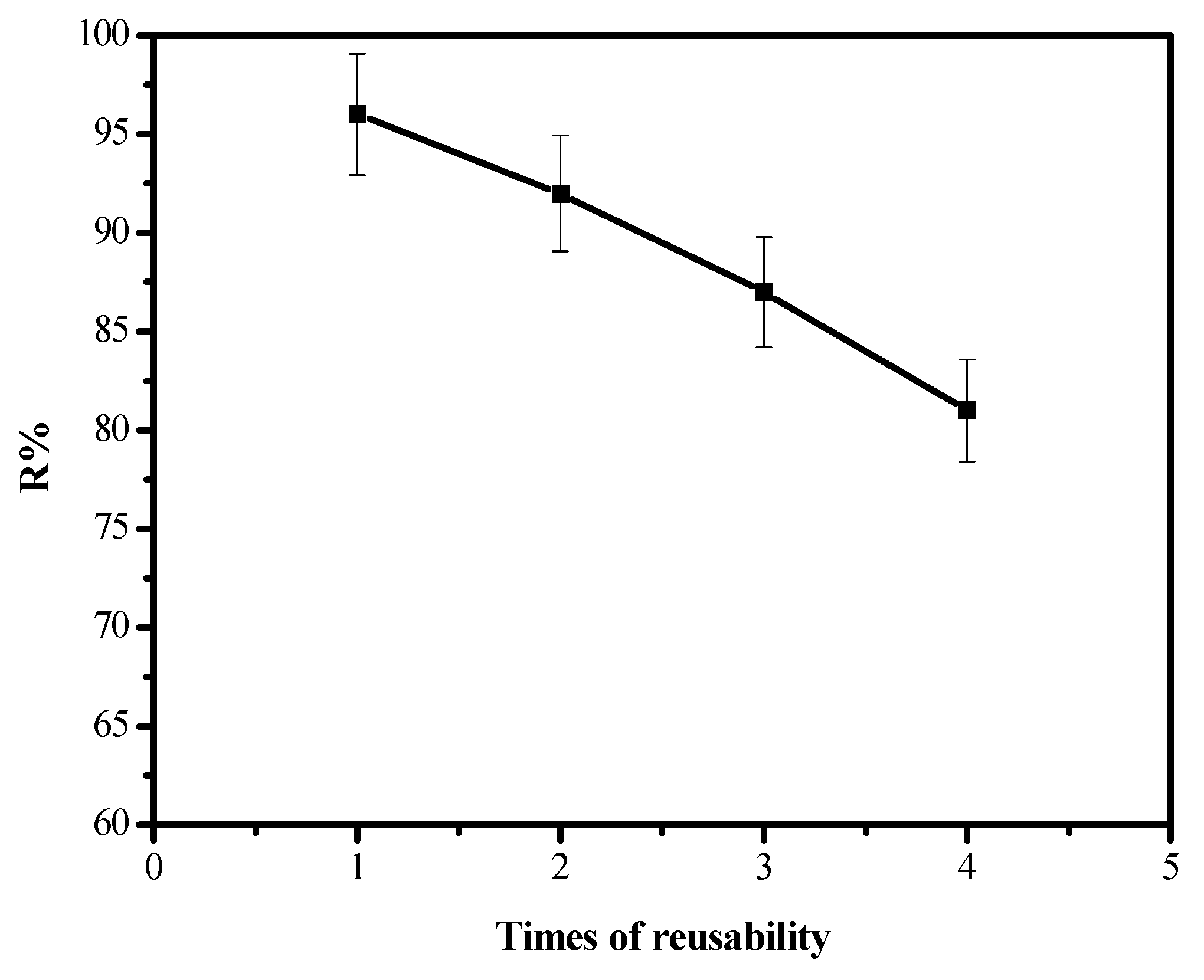
2.7. Reusability of the Composite
3. Results
3.1. Characterization
3.1.1. FTIR Spectroscopy
3.1.2. SEM
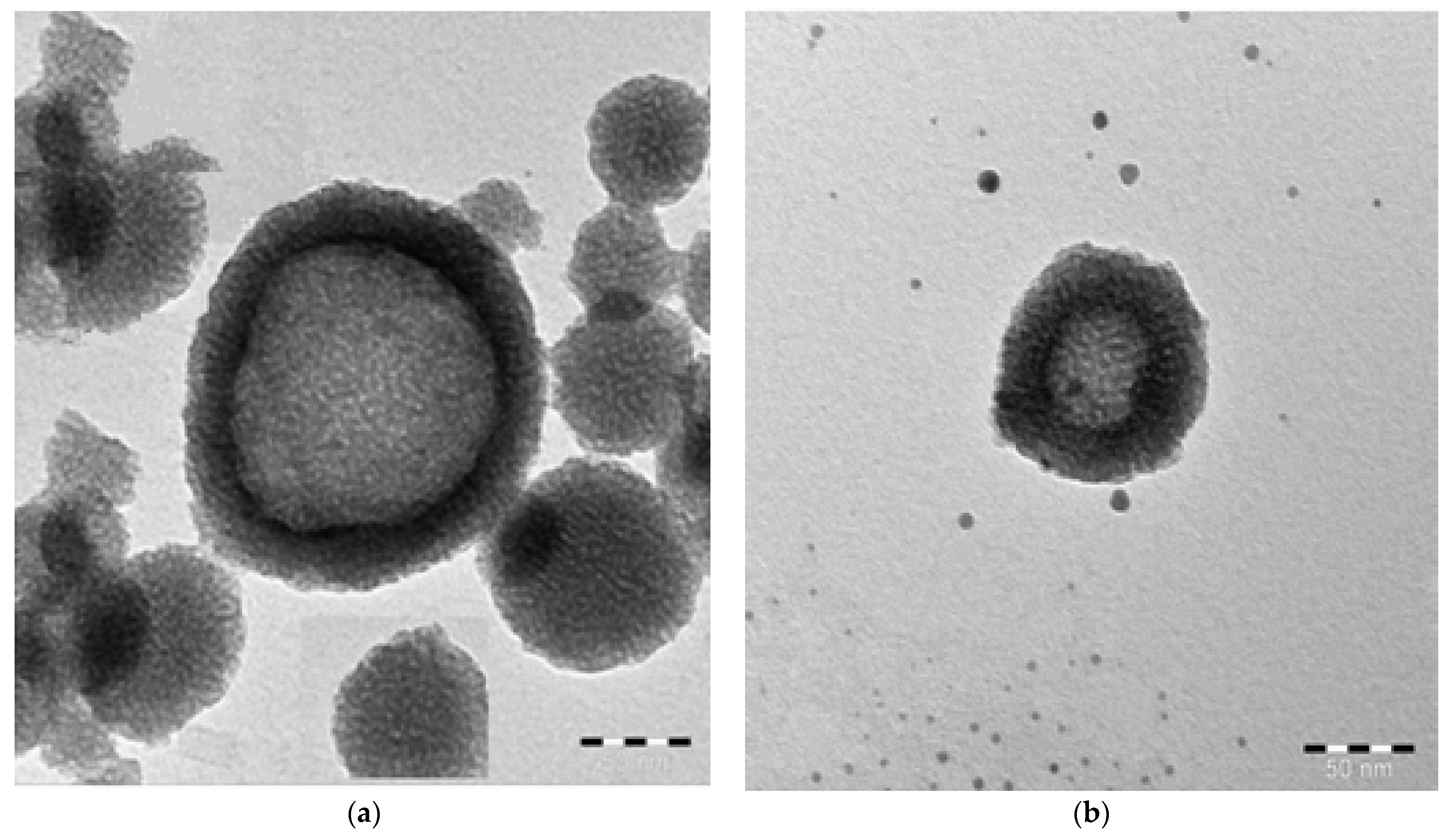
3.1.3. TEM
3.1.4. XRD
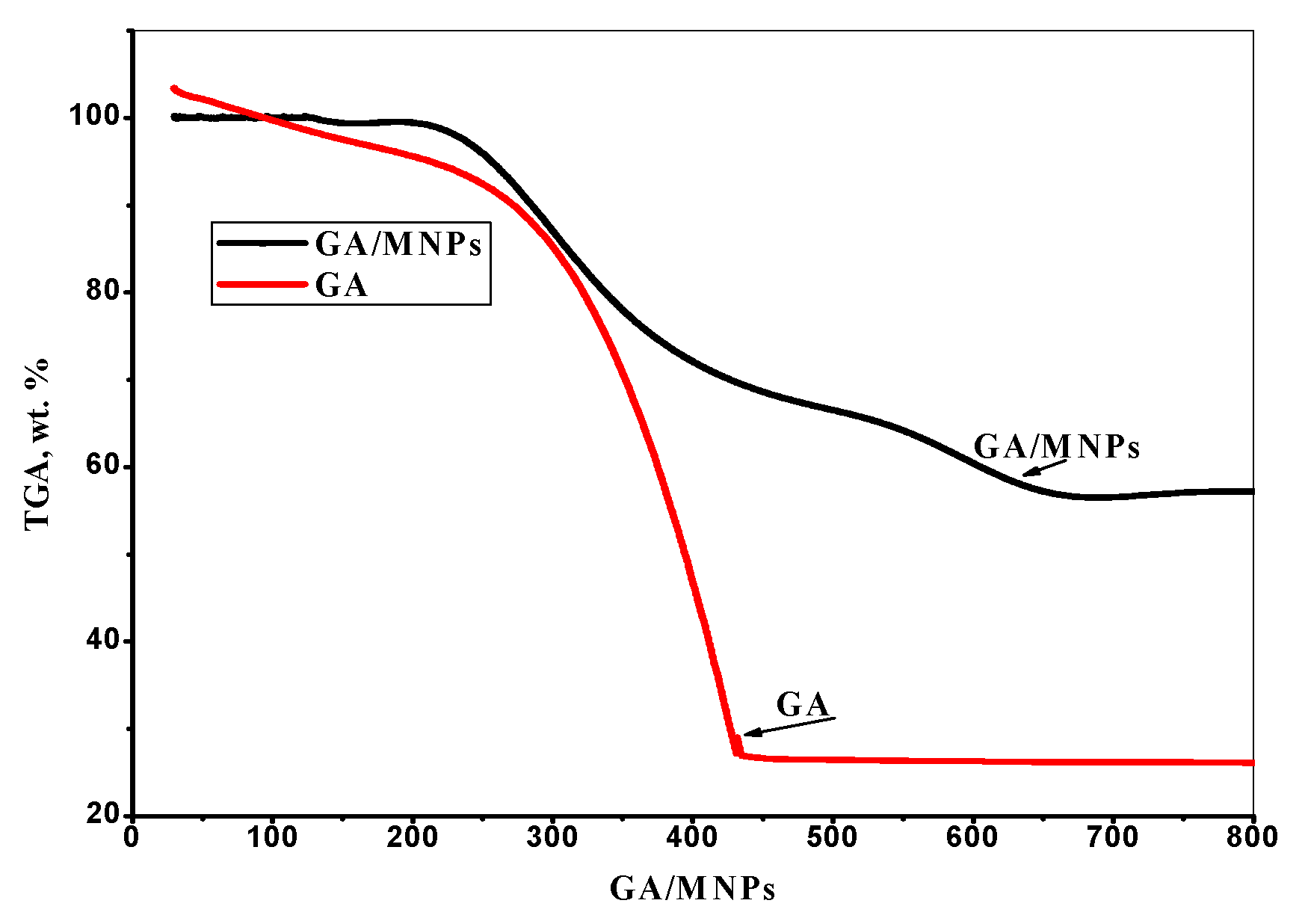
3.1.5. TGA
3.1.6. Magnetic Properties
3.2. Adsorption Studies
3.2.1. GA Capacity to Adsorb Pb(II) Ions
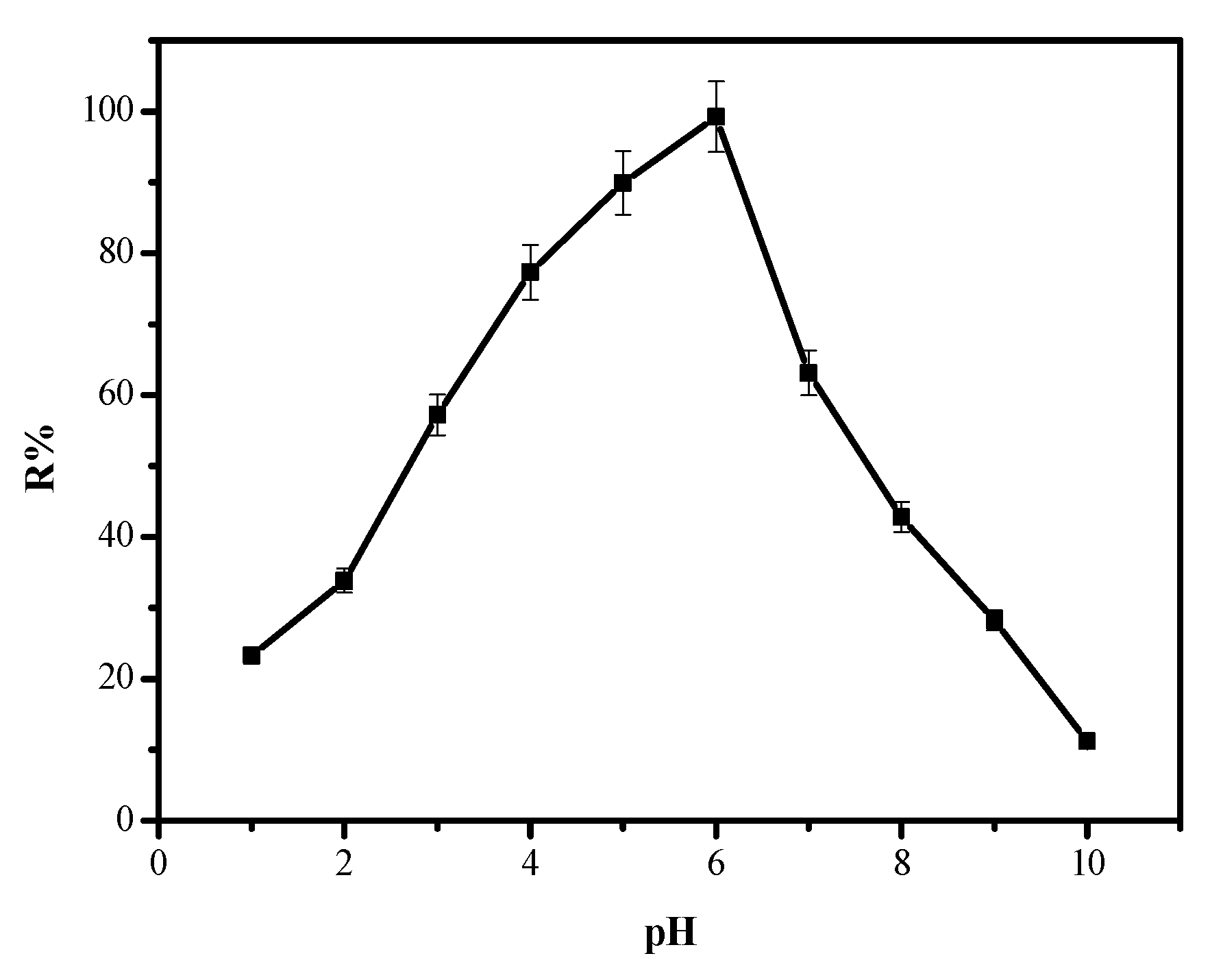
3.2.2. Influence of pH
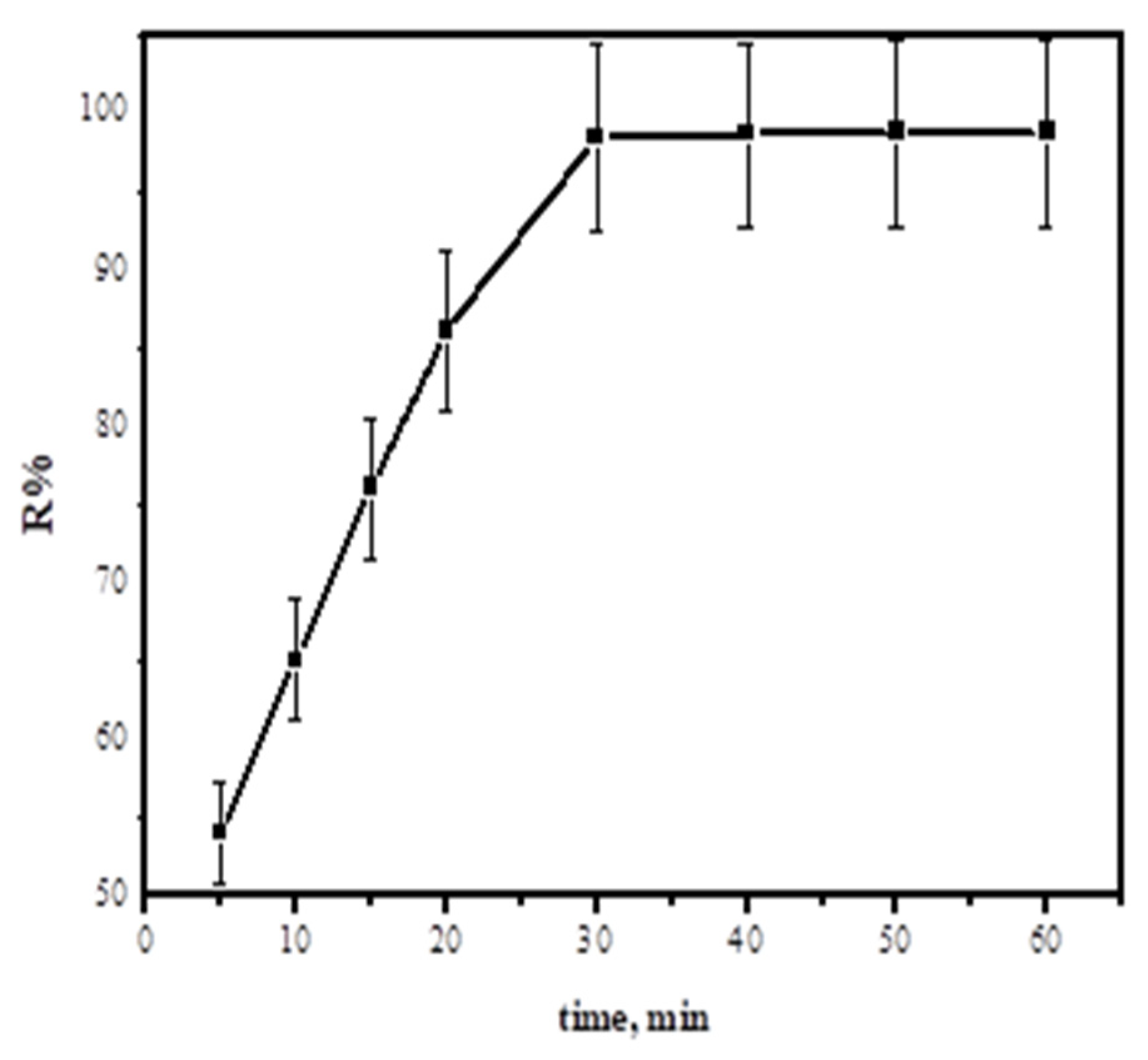
3.2.3. Effect of Contact Time
3.2.4. Kinetic Behavior
Pseudo-First and Second-Order Models
Intra-Particle Diffusion Kinetic Model
Elovich Model
3.2.5. Effect of Initial Pb(II) Concentration
3.3. Isotherm Models
3.3.1. Langmuir Model
3.3.2. Freundlich Model
3.3.3. Temkin Isotherm
3.3.4. Dubinin–Radushkevich Model
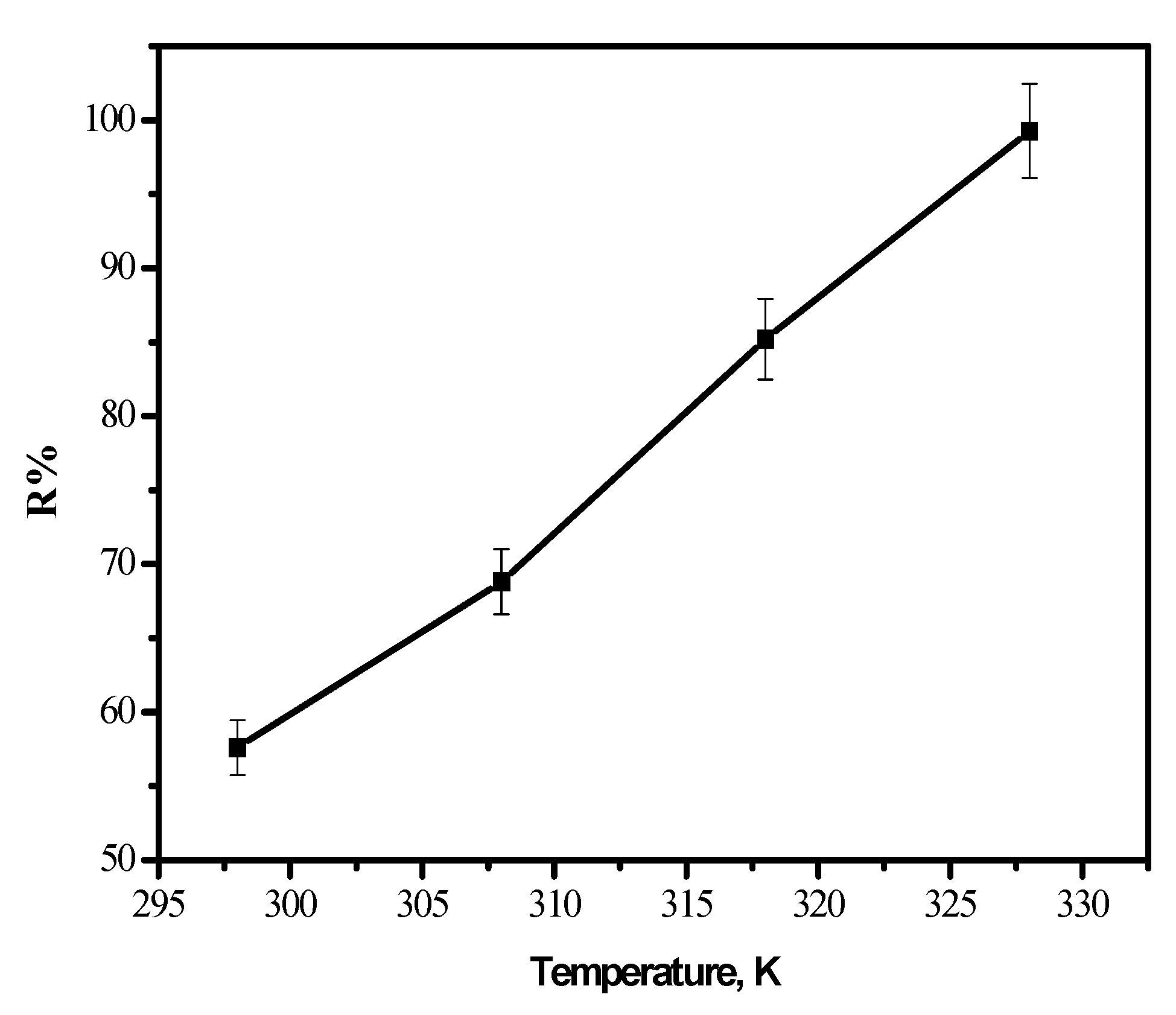
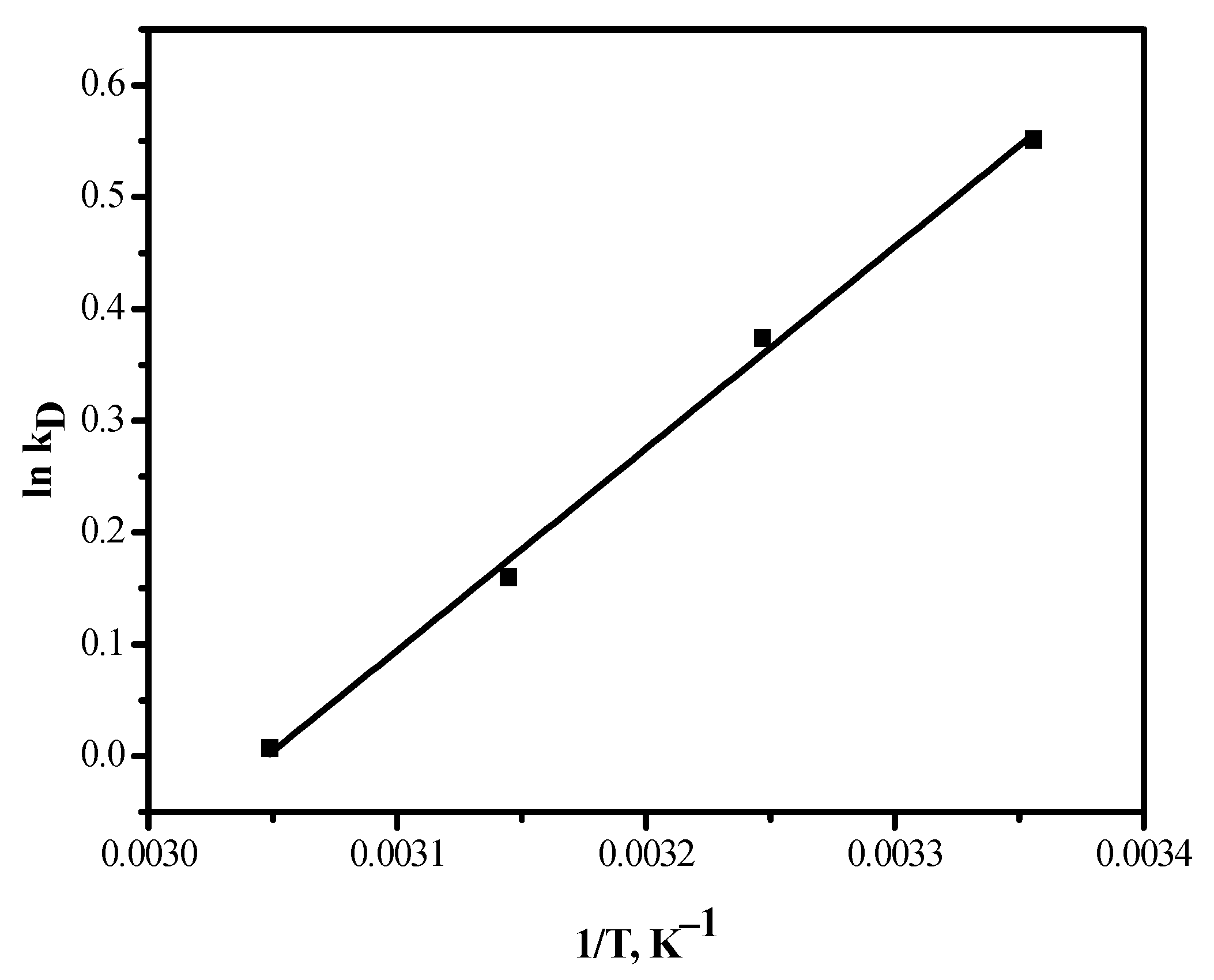
3.4. Effect of Temperature
3.5. Thermodynamic Parameters
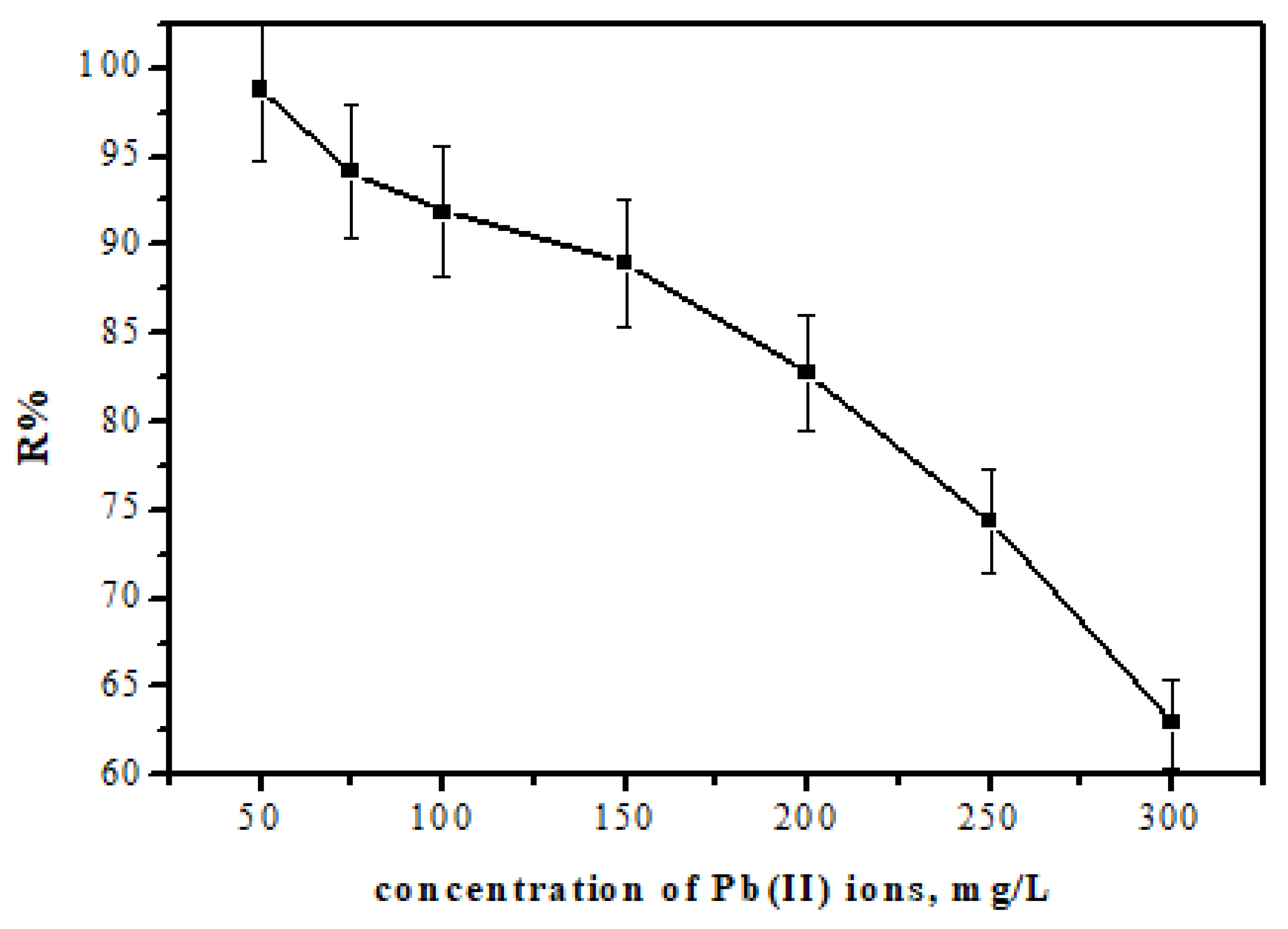
3.6. Selectivity
3.7. Reusability of the Composite
3.8. Comparison of GAMNPs with Other Materials Used for Pb(II) Removal
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Malkoc, E.; Nuhoglu, Y.; Abali, Y. Cr (VI) Adsorption by Waste Acorn of Quercus ithaburensis in Fixed Beds: Prediction of Breakthrough Curves. Chem. Eng. J. 2006, 119, 61–68. [Google Scholar] [CrossRef]
- Gebretsadik, H.; Gebrekidan, A.; Demlie, L.; Suvarapu, N. (Reviewing editor)Removal of heavy metals from aqueous solutions using Eucalyptus Camaldulensis: An alternate low cost adsorbent. Cogent Chem. 2020, 6, 1720892. [Google Scholar] [CrossRef]
- Eltayeb, N.; Khan, A. Design and Preparation of a New and Novel Nanocomposite with CNTs and Its Sensor Applications. J. Mater. Res. Technol. 2019, 8, 2238–2246. [Google Scholar] [CrossRef]
- Khan, A.; Asiri, A.; Khan, A.; Abdul Rub, M.; Azum, N.; Rahman, M.M.; Khan, S.B.; Alamry, K.A.; Ab Ghani, S. Sol-gel synthesis and characterization of conducting polythiophene/tin phosphate nano tetrapod composite cation-exchanger and its application as Hg (II) selective membrane electrode. J. Sol-Gel Sci. Technol. 2013, 65, 160–169. [Google Scholar] [CrossRef]
- Demirbaş, Ö.; Çalımlı, M.H.; Demirkan, B.; Alma, M.H.; Nas, M.S.; Khan, A.; Asiri, A.M.; Sen, F. The Kinetic Parameters of Adsorption of Enzymes Using Carbon-Based Materials Obtained from Different Food Wastes. BioNanoScience 2019, 9, 749–757. [Google Scholar] [CrossRef]
- Al Mesfer, M.K.; Danish, M.; Ali, I.H.; Khan, M.I. Adsorption behavior of molecular sieve 3 Å and silica gel for CO2 separation: Equilibrium, breakthrough and mass transfer zone. Heat Mass Transf. 2020, 56, 3243–3259. [Google Scholar] [CrossRef]
- Ali, I.H.; Al Mesfer, M.K.; Khan, M.I.; Danish, M.; Alghamdi, M.M. Exploring Adsorption Process of Lead (II) and Chromium (VI) Ions from Aqueous Solutions on Acid Activated Carbon Prepared from Juniperus procera Leaves. Processes 2019, 7, 217. [Google Scholar] [CrossRef] [Green Version]
- Jun, B.; Her, N.; Park, C.M.; Yoon, Y. Effective removal of Pb(ii) from synthetic wastewater using Ti3C2Tx MXene. Environ. Sci. Water Res. Technol. 2020, 6, 173–180. [Google Scholar] [CrossRef]
- Tao, Y.; Zhang, C.; Lü, T.; Zhao, H. Removal of Pb (II) Ions from Wastewater by Using Polyethyleneimine-Functionalized Fe3O4 Magnetic Nanoparticles. Appl. Sci. 2020, 10, 948. [Google Scholar] [CrossRef] [Green Version]
- Greenwood, N.N.; Earnshaw, A. Chemistry of the elements. In Applied Organometallic Chemistry, 2nd ed.; Wiley & Sons: Oxford, UK, 1998; Volume 9, p. 3365. [Google Scholar]
- Akartasse, N.; Mejdoubi, E.; Razzouki, B.; Azzaoui, K.; Jodeh, S.; Hamed, O.; Ramdani, M.; Lamhamdi, A.; Berrabah, M.; Lahmass, I.; et al. Natural product based composite for extraction of arsenic (III) from waste water. Chem. Cent. J. 2017, 11, 33–45. [Google Scholar] [CrossRef] [Green Version]
- Montenegro, M.A.; Boiero, M.L.; Valle, L.; Borsarelli, C.D. Gum Arabic: More Than an Edible Emulsifier, 1st ed.; IntechOpen: London, UK, 2012; pp. 1–26. [Google Scholar]
- Abdul Khalil, H.P.S.; Chong, E.W.N.; Owolabi, F.A.T.; Asniza, M.; Tye, Y.Y.; Rizal, S.; Nural Fazita, M.R.; Haafiz, M.K.M.; Nurmiati, Z.; Paridah, M.T. Enhancement of basic properties of polysaccharide-based composites with organic and inorganic fillers: A review. J. Appl. Polym. Sci. 2019, 136, 47251–47270. [Google Scholar] [CrossRef] [Green Version]
- Banerjee, S.S.; Chen, D.-H. Fast removal of copper ions by gum arabic modified magnetic nano-adsorbent. J. Hazard. Mater. 2007, 147, 792–799. [Google Scholar] [CrossRef] [PubMed]
- Alzahrani, E. Gum Arabic-coated magnetic nanoparticles for methylene blue removal. Int. J. Innov. Res. Sci. Eng. Technol. 2014, 3, 15118–15129. [Google Scholar] [CrossRef]
- Musico, Y.L.F.; Santos, C.M.; Dalida, M.L.P.; Rodrigues, D.F. Improved removal of lead (ii) from water using a polymer-based graphene oxide nanocomposite. J. Mater. Chem. A 2013, 1, 3789–3796. [Google Scholar] [CrossRef]
- Sani, H.A.; Ahmad, M.B.; Hussein, M.Z.; Ibrahim, N.A.; Musa, A.; Saleh, T.A. Nanocomposite of ZnO with montmorillonite for removal of lead and copper ions from aqueous solutions. Process Saf. Environ. Prot. 2017, 109, 97–105. [Google Scholar] [CrossRef]
- Alebel Gebru, K.; Das, C. Removal of Pb (II) and Cu (II) ions from wastewater using composite electrospun cellulose acetate/titanium oxide (TiO2) adsorbent. J. Water Process. Eng. 2017, 16, 1–13. [Google Scholar] [CrossRef]
- Williams, D.N.; Gold, K.A.; Holoman, T.R.P.; Ehrman, S.H.; Wilson, O.C., Jr. Surface modification of magnetic nanoparticles using gum Arabic. J. Nanopart. Res. 2006, 8, 749–753. [Google Scholar] [CrossRef]
- Keshk, M.A.S.S.; El-Zahhar, A.A.; Youssef, M.S.A.; Bondock, S. Novel synthesis of flame-retardant magnetic nanoparticles/hydroxy acid cellulose-6-phosphate composite. Mater. Res. Express 2019, 6, 85310. [Google Scholar] [CrossRef]
- Sharma, G.; Kumar, A.; Devi, K.; Sharma, S.; Naushad, M.; Ghfar, A.A.; Ahamad, T.; Stadler, F.J. Guar gum-crosslinked-Soya lecithin nanohydrogel sheets as effective adsorbent for the removal of thiophanate methyl fungicide. Int. J. Biol. Macromol. 2018, 114, 295–305. [Google Scholar] [CrossRef]
- Farooq, M.; Sagbas, S.; Sahiner, M.; Siddiq, M.; Turk, M.; Aktas, N.; Sahiner, N. Synthesis, characterization and modification of Gum Arabic microgels for hemocompatibility and antimicrobial studies. Carbohydr. Polym. 2017, 156, 380–389. [Google Scholar] [CrossRef]
- Keshk, S.M.A.S.; El-Zahhar, A.A.; Alsulami, Q.A.; Jaremko, M.; Bondock, S.; Heinze, T. Synthesis, characterization and ampyrone drug release behavior of magnetite nanoparticle/2,3-dialdehyde cellulose-6-phosphate composite. Cellulose 2020, 27, 1603. [Google Scholar] [CrossRef] [Green Version]
- Zhuang, J.; Li, M.; Pu, Y.; Ragauskas, A.J.; Yoo, C.G. Observation of Potential Contaminants in Processed Biomass Using Fourier Transform Infrared Spectroscopy. Appl. Sci. 2020, 10, 4345. [Google Scholar] [CrossRef]
- Aguilera, G.; Berry, C.; West, R.; Gonzalez-Monterrubio, E.; Angulo-Molina, A.; Arias-Carrion, O.; Angel Mendez-Rojas, M. Carboxymethyl cellulose coated magnetic nanoparticles transport across a human lung microvascular endothelial cell model of the blood–brain barrier. Nanoscale Adv. 2019, 2, 671–685. [Google Scholar] [CrossRef] [Green Version]
- Bhakat, D.; Barik, P.; Bhattacharjee, A. Electrical conductivity behavior of Gum Arabic biopolymer-Fe3O4 nanocomposite. J. Phys. Chem. Solids 2018, 112, 73–97. [Google Scholar] [CrossRef]
- Hedayati, K.; Goodarzi, M.; Ghanbari, D. Hydrothermal Synthesis of Fe3O4 Nanoparticles and Flame Resistance Magnetic Poly styrene Nanocomposite. J. Nanostruct. 2017, 7, 32–39. [Google Scholar]
- Amer, M.W.; Ahmad, R.A.; Awwad, A.M. Biosorption of Cu (II), Ni (II), Zn (II) and Pb (II) ions from aqueous solution by Sophora japonica pods powder. Int. J. Ind. Chem. 2015, 6, 67–75. [Google Scholar] [CrossRef] [Green Version]
- Ouyang, D.; Zhuo, Y.; Hu, L.; Zeng, Q.; Hu, Y.; He, Z. Research on the Adsorption Behavior of Heavy Metal Ions by Porous Material Prepared with Silicate Tailings. Minerals 2019, 9, 291. [Google Scholar] [CrossRef] [Green Version]
- Elkhaleefa, A.; Ali, I.H.; Brima, E.I.; Elhag, A.B.; Karama, B. Efficient Removal of Ni (II) from Aqueous Solution by Date Seeds Powder Biosorbent: Adsorption Kinetics, Isotherm and Thermodynamics. Processes 2020, 8, 1001. [Google Scholar] [CrossRef]
- Alfaro-Cuevas-Villanueva, R.; Hidalgo-Vázquez, A.R.; Penagos, C.J.C.; Cortés-Martínez, R. Thermodynamic, Kinetic, and Equilibrium Parameters for the Removal of Lead and Cadmium from Aqueous Solutions with Calcium Alginate Beads. Sci. World J. 2014. [Google Scholar] [CrossRef]
- Kithome, M.; Paul, J.W.; Lavkulich, L.M.; Bomke, A.A. Kinetics of Ammonium Adsorption and Desorption by the Natural Zeolite Clinoptilolite. Soil Sci. Soc. Am. J. 1998, 62, 622–629. [Google Scholar] [CrossRef]
- Papageorgiou, S.K.; Katsaros, F.K.; Kouvelos, E.P.; Nolan, J.W.; Deit, H.L.; Kanellopoulos, N.K. Heavy metal sorption by calcium alginate beads from Laminaria digitata. J. Hazard. Mater. 2006, 137, 1765–1772. [Google Scholar] [CrossRef]
- Ali, I.H.; Alrafai, H.A. Kinetic, isotherm and thermodynamic studies on biosorption of chromium (VI) by using activated carbon from leaves of Ficus nitida. Chem. Cent. J. 2016, 10, 36. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mehrmand, N.; Moraveji, M.K.; Parvareh, A. Adsorption of Pb(II), Cu(II) andNi (II) ions from aqueous solutions by functionalised henna powder (Lawsonia Inermis); isotherm, kinetic and thermodynamic studies. Int. J. Environ. Anal. Chem. 2020. [Google Scholar] [CrossRef]
- El-Naggar, I.M.; Ahmed, S.A.; Shehata, N.E.S.; Fathy, S.M.; Shehata, A. A novel approach for the removal of lead (II) ion from wastewater using Kaolinite/Smectite natural composite adsorbent. Appl. Water Sci. 2019, 9, 7–19. [Google Scholar] [CrossRef] [Green Version]
- Khan, M.I.; Almesfer, M.K.; Danish, M.; Ali, I.H.; Shoukry, H.; Patel, R.; Gardy, J.; Nizami, A.S.; Rehan, M. Potential of Saudi natural clay as an effective adsorbent in heavy metals removal from wastewater. Desalin. Water Treat. 2019, 158, 140–151. [Google Scholar] [CrossRef]
- Ali, I.H.; Sulfab, Y. Concurrent two one-electron transfer in the oxidation of chromium (III) complexes with trans-1,2-diaminocyclohexane-N,N,N′,N′-tetraacetate and diethylenetriaminepentaacetate ligands by periodate ion. Int. J. Chem. Kinet. 2012, 44, 729–735. [Google Scholar] [CrossRef]
- Ali, I.H.; Sulfab, Y. One-step, two-electron oxidation of cis-diaquabis(1,10-phenanthroline)chromium (III) to cis-dioxobis (1,10-phenanthroline) chromium (V) by periodate in aqueous acidic solutions. Int. J. Chem. Kinet. 2011, 43, 563–568. [Google Scholar] [CrossRef]
- Mouni, L.; Merabet, D.; Bouzaza, A.; Belkhiri, L. Adsorption of Pb (II) from aqueous solutions using activated carbon developed from Apricot stone. Desalination 2011, 276, 148–153. [Google Scholar] [CrossRef]
- Kaur, M.; Kumari, S.; Sharma, P. Removal of Pb (II) from aqueous solution using nanoadsorbent of Oryza sativa husk: Isotherm, kinetic and thermodynamic studies. Biotechnol. Rep. 2020, 25, e00410. [Google Scholar] [CrossRef] [PubMed]
- Kardam, A.; Raj, K.R.; Srivastava, S.; Srivastava, M.M. Nanocellulose fibers for biosorption of cadmium, nickel, and lead ions from aqueous solution. Clean Technol. Environ. Policy 2014, 16, 385–393. [Google Scholar] [CrossRef]
- Alghamdi, A.A.; Al-Odayni, A.-B.; Saeed, W.S.; Al-Kahtani, A.; Alharthi, F.A.; Aouak, T. Efficient Adsorption of Lead (II) from Aqueous Phase Solutions Using Polypyrrole-Based Activated Carbon. Materials 2019, 12, 2020. [Google Scholar] [CrossRef] [Green Version]
- Tran, C.V.; Quang, D.V.; Thi, H.P.N.; Truong, T.N.; La, D.D. Effective Removal of Pb (II) from Aqueous Media by a New Design of Cu−Mg Binary Ferrite. ACS Omega 2020, 5, 7298–7306. [Google Scholar] [CrossRef] [PubMed]
- Mousavi, H.Z.; Hosseynifar, A.; Jahed, V.; Dehghani, S.A.M. Removal of lead from aqueous solution using waste tire rubber ash as an adsorbent. Braz. J. Chem. Eng. 2010, 27, 79–87. [Google Scholar] [CrossRef]
- Manyangadze, M.; Chikuruwo, N.M.H.; Narsaiah, T.B.; Chakra, C.H.; Charis, G.; Danha, G.; Mamvura, T.A. Adsorption of lead ions from wastewater using nano silica spheres synthesized on calcium carbonate templates. Heliyon 2020, 6, e05390. [Google Scholar] [CrossRef] [PubMed]
- Khatoon, A.; Kashif Uddin, M.; Rao, R.A.K. Adsorptive remediation of Pb (II) from aqueous media using Schleichera oleosa bark. Environ. Technol. Innov. 2018, 11, 1–14. [Google Scholar] [CrossRef]
- He, K.; Chen, Y.; Tang, Z.; Hu, Y. Removal of heavy metal ions from aqueous solution by zeolite synthesized from flyash. Environ. Sci. Pollut. Res. 2016, 23, 2778–2788. [Google Scholar] [CrossRef] [PubMed]




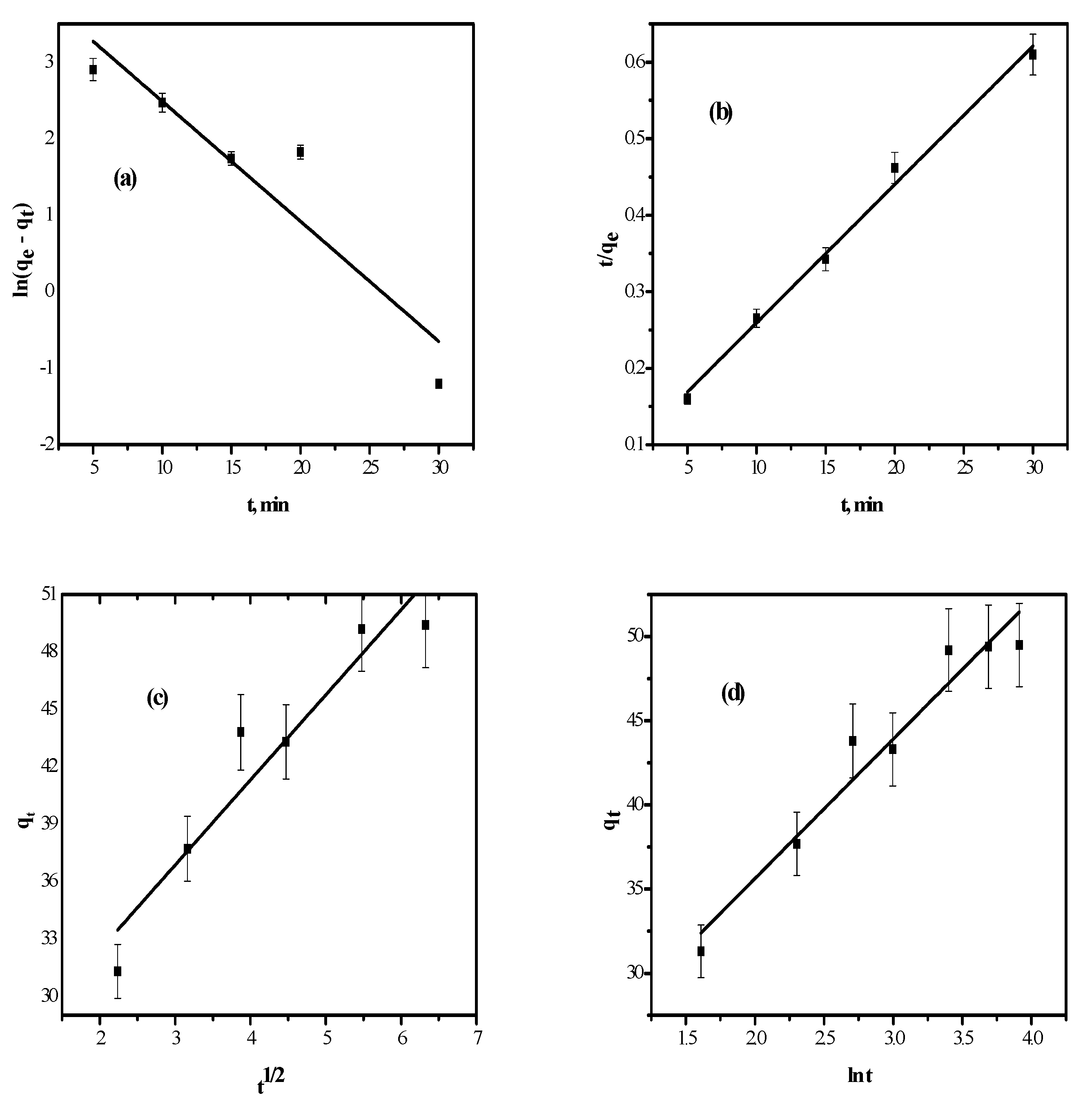
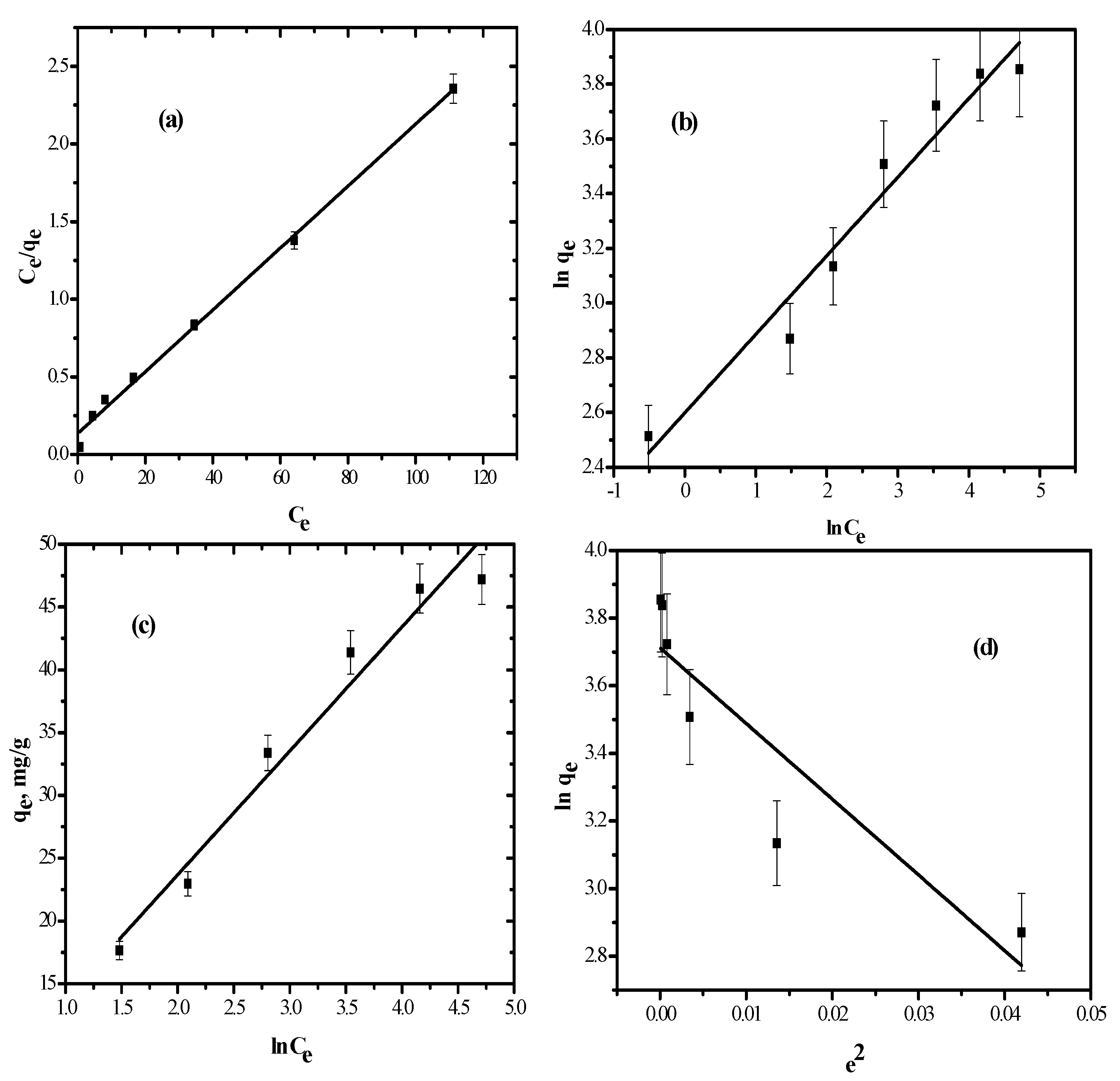
| Kinetic Model | Parameters | |
|---|---|---|
| first order | qe (mg/g) | 25.1 |
| k1 (min−1) | 0.0792 | |
| R2 | 0.8595 | |
| second order | qe (mg/g) | 55.2 |
| k2 (g/mg·min) | 0.0181 | |
| R2 | 0.9937 | |
| Intra-particle diffusion | kid (mg/g·min) | 4.45 |
| I | 23.5 | |
| R2 | 0.9043 | |
| Elovich | A | 30 × 1068 |
| Β | 0.121 | |
| R2 | 0.9378 | |
| Adsorption Model | Isotherm Parameters | Values |
|---|---|---|
| Langmuir | qmax (mg/g) | 50.5 |
| RL (L/g) | 0.029 | |
| R2 | 0.887 | |
| Freundlich | n | 0.67 |
| Kf (mg/g)/(mg/L) | 4.6 | |
| R2 | 0.9981 | |
| Temkin | A (L/g) | 2.1 |
| bt (kJ/mol) | 370.3 | |
| R2 | 0.899 | |
| DR | β | 22.4 |
| qm (mg/g) | 40.4 | |
| Ef (kJ/mol) | 0.15 | |
| R2 | 0.7864 |
| T, K | ∆G, kJ/mol | ∆S, J/mol K | ∆H, kJ/mol |
|---|---|---|---|
| 298 | −6.1 | 26.6 | 15.0 |
| 308 | −6.5 | ||
| 318 | −6.8 | ||
| 328 | −7.3 |
| Existing Metal Ion | Concentration of Each Metal | R% of Pb(II) Ions |
|---|---|---|
| Cr(III), Ni(II), Cu(II) | 5.0 mg/L | 92 |
| Cr(III), Ni(II), Cu(II) | 10.0 mg/L | 88 |
| Cr(III), Ni(II), Cu(II) | 15 mg/L | 84 |
| Material | qmax (mg/g) | Experimental Optimum Conditions | Ref. | ||||
|---|---|---|---|---|---|---|---|
| pH | T (K) | Contact Time (min) | Co (mg/L) | Adsorbent Mass (g/L) | |||
| Juniperus procera AC | 30.3 | 4.6 | 298 | 100 | 50 | 8 | [7] |
| Apricot stone | 21.4 | 6.0 | 293 | 300 | 50 | 2 | [40] |
| Oryza sativa husk | 6.1 | 8.0 | 333 | 70 | 10 | 12 | [41] |
| Nanocellulose fibers | 9.4 | 5.0 | 298 | 90 | 25 | 8 | [42] |
| Polypyrrole-based AC | 50.0 | 5.5 | 298 | 120 | 100 | 5 | [43] |
| Cu0.5Mg0.5Fe2O4 | 57.7 | 6.0 | 298 | 120 | 10 | 0.1 | [44] |
| Waste tire rubber ash | 22.4 | 6.0 | 303 | 90 | 400 | 2 | [45] |
| Nanosilica hollow spheres | 200.0 | 5.0 | 333 | 40 | 300 | 0.05 | [46] |
| S. Oleasea bark | 69.40 | 6.0 | 323 | 60 | 100 | 5.0 | [47] |
| Zeolite | 65.8 | 5.7 | 303 | 50 | 40 | 5.0 | [48] |
| GA/MNPs | 50.5 | 6.0 | 298 | 30 | 50 | 6 | This study |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, I.H.; Bani-Fwaz, M.Z.; El-Zahhar, A.A.; Marzouki, R.; Jemmali, M.; Ebraheem, S.M. Gum Arabic-Magnetite Nanocomposite as an Eco-Friendly Adsorbent for Removal of Lead(II) Ions from Aqueous Solutions: Equilibrium, Kinetic and Thermodynamic Studies. Separations 2021, 8, 224. https://doi.org/10.3390/separations8110224
Ali IH, Bani-Fwaz MZ, El-Zahhar AA, Marzouki R, Jemmali M, Ebraheem SM. Gum Arabic-Magnetite Nanocomposite as an Eco-Friendly Adsorbent for Removal of Lead(II) Ions from Aqueous Solutions: Equilibrium, Kinetic and Thermodynamic Studies. Separations. 2021; 8(11):224. https://doi.org/10.3390/separations8110224
Chicago/Turabian StyleAli, Ismat H., Mutasem Z. Bani-Fwaz, Adel A. El-Zahhar, Riadh Marzouki, Mosbah Jemmali, and Sara M. Ebraheem. 2021. "Gum Arabic-Magnetite Nanocomposite as an Eco-Friendly Adsorbent for Removal of Lead(II) Ions from Aqueous Solutions: Equilibrium, Kinetic and Thermodynamic Studies" Separations 8, no. 11: 224. https://doi.org/10.3390/separations8110224
APA StyleAli, I. H., Bani-Fwaz, M. Z., El-Zahhar, A. A., Marzouki, R., Jemmali, M., & Ebraheem, S. M. (2021). Gum Arabic-Magnetite Nanocomposite as an Eco-Friendly Adsorbent for Removal of Lead(II) Ions from Aqueous Solutions: Equilibrium, Kinetic and Thermodynamic Studies. Separations, 8(11), 224. https://doi.org/10.3390/separations8110224








