Accurate Analytical Approximation for the Bessel Function J2(x)
Abstract
:1. Introduction
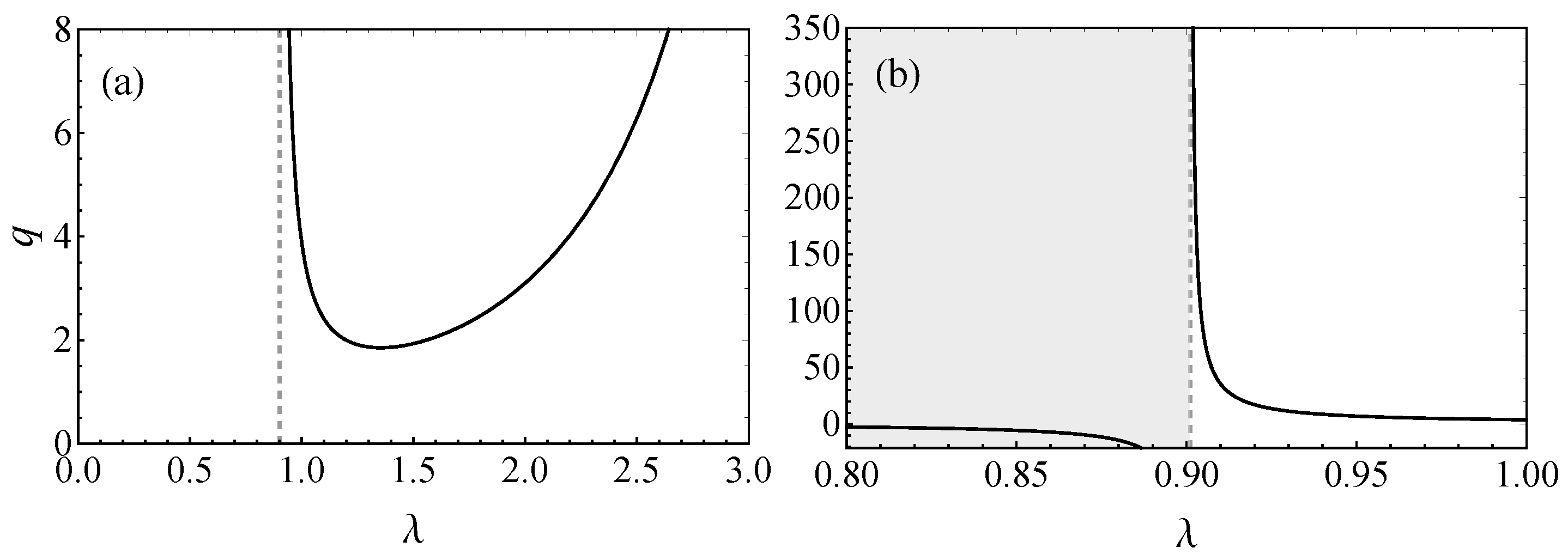
2. Theoretical Analysis
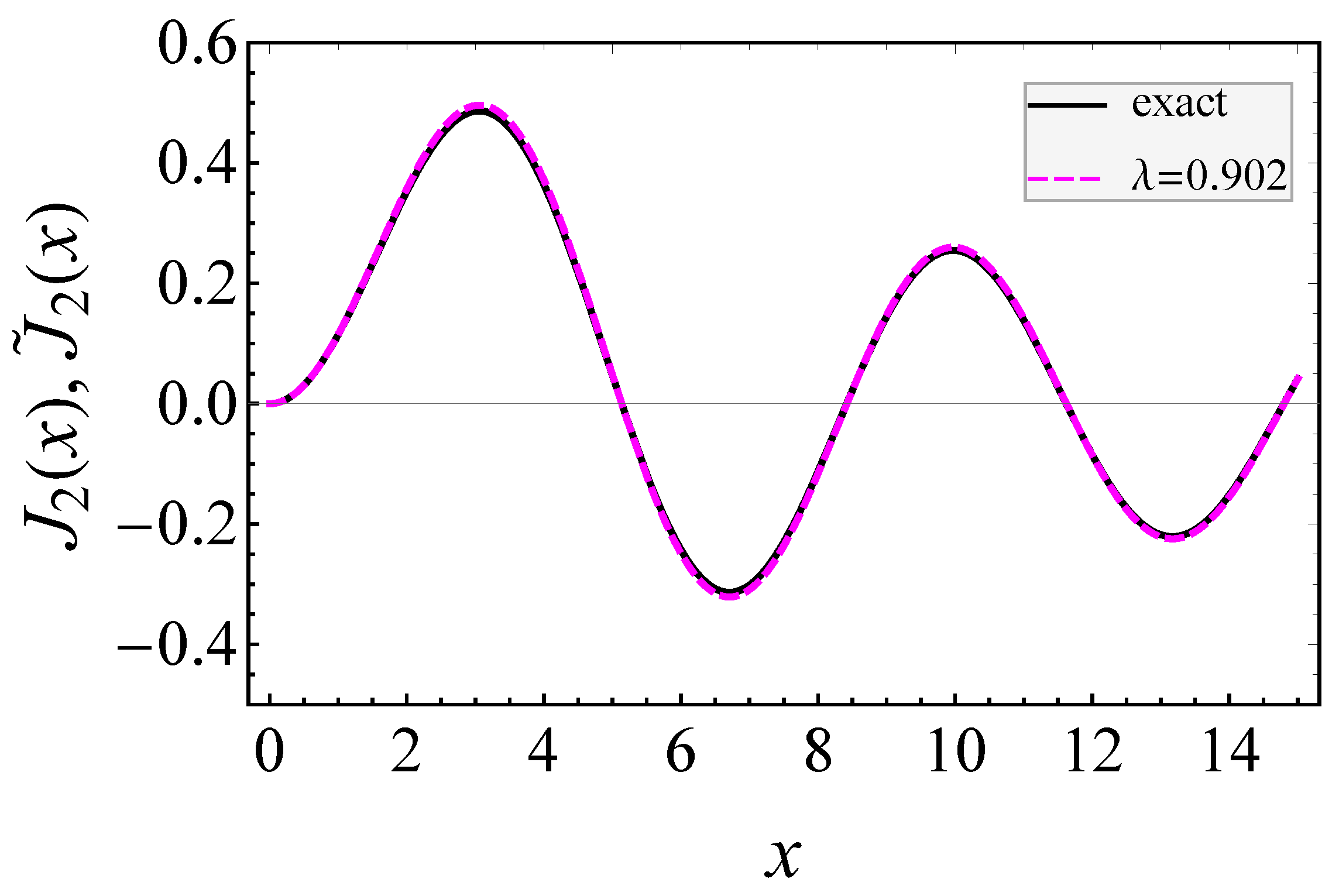
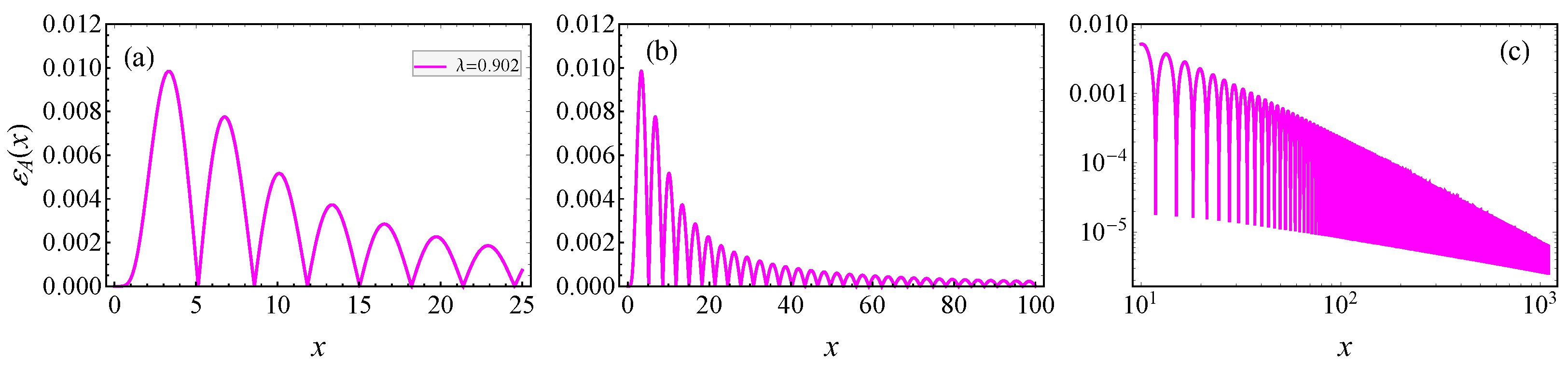
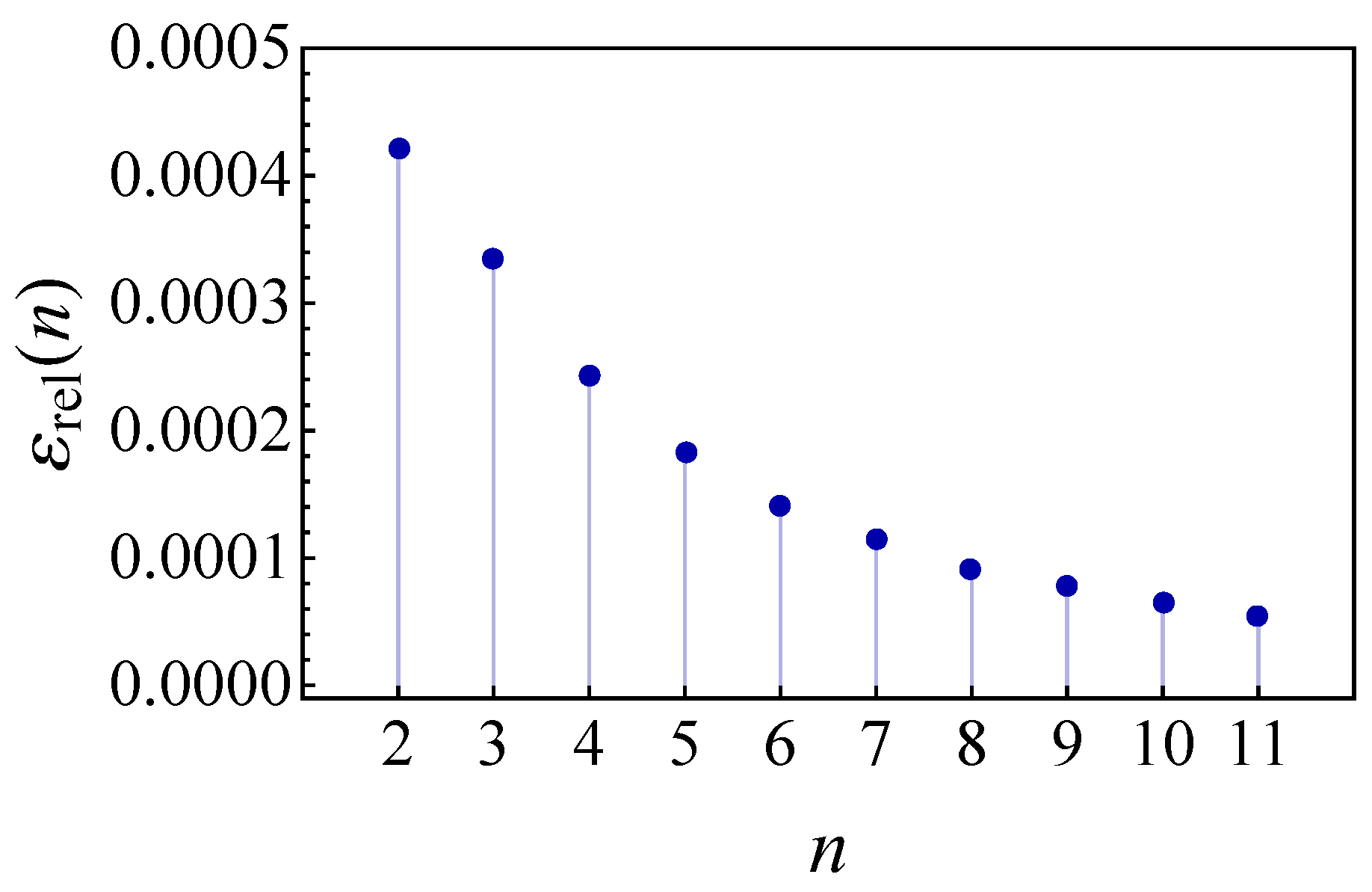
3. Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Watson, G.N. A Treatise on the Theory of Bessel Functions, 2nd ed.; Cambridge University Press: Cambridge, UK, 1966. [Google Scholar]
- Jackson, J.D. Classical Electrodynamics, 3rd ed.; John Willey and Sons, Inc.: New York, NY, USA, 1998; pp. 111–119. [Google Scholar]
- Khosravian-Arab, H.; Dehghan, M.; Eslahchi, M.R. Generalized Bessel functions. Theory and their applications. Math. Method. Appl. Sci. 2017, 40, 6389–6410. [Google Scholar] [CrossRef]
- Rothwell, E.J. Exponential Approximation of the Bessel Functions I0(x), I1(x), J0(x), J1(x), Y0(x) and H0(x) with Applications to Electromagnetic Scattering, Radiation and Diffraction [EM Programmer’s Notebooks]. IEEE Antennas Propag. Mg. 2009, 51, 138–147. [Google Scholar] [CrossRef]
- Maass, F.; Martin, P.; Olivares, J. Analytic Approximation to the Bessel Function J0(x). Comput. Appl. Math. 2020, 39, 222. [Google Scholar] [CrossRef]
- Maass, F.; Martin, P. Precise analytic approximations for the Bessel function J1(x). Results Phys. 2018, 8, 1234–1238. [Google Scholar] [CrossRef]
- Martin, P.; Castro, E.; Paz, J.L.; De Freitas, A. Multipoint quasi-rational approximations in Quantum Chemistry. In New Developments in Quantum Chemistry; Paz, J.L., Hernandez, J.A., Eds.; Transworld Research Network: Kerala, India, 2009; Chapter 3; pp. 55–76. [Google Scholar]
- Martin, P.; Guerrero, L. Fractional approximations for the Bessel function J0(x). J. Math. Phys. 1985, 26, 705–707. [Google Scholar] [CrossRef]
- Baker, G.A., Jr.; Travers-Morris, P. Padé Approximants; Cambrige University Press: Cambrige, UK, 1996. [Google Scholar]
- Peker, H.A. An Introduction to Padé Approximation. In Current Studies in Basic Sciences, Engineering and Tecnology; ISRES Publishing: Konya, Turkey, 2021; pp. 143–155. [Google Scholar]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions; National Bureau of Standards: Washington, DC, USA, 1972; pp. 481–482. [Google Scholar]
- Millane, R.P.; Eads, J.L. Polynomial approximations to Bessel Functions. IEEE Trans. Antenna Propagat. 2003, 51, 1398–1400. [Google Scholar] [CrossRef]
- Li, L.; Li, F.; Gross, F.B. A New Polynomial Approximations for Jv Bessel Functions. Appl. Math. Comp. 2006, 183, 1220–1225. [Google Scholar] [CrossRef]
- Relton, F.E. Applied Bessel Functions; Blackie and Son Limited: London, UK, 1946; pp. 124–151. [Google Scholar]
- Idris, F.A.; Buhari, A.L.; Adamu, T.U. Bessel Functions and Their Applications: Solution to Schrodinger Equation in a Cylindrical Function of the Second Kind and Hankel Functions. Int. J. Novel Res. Phys. Chem. Math. 2016, 3, 17–31. [Google Scholar]
- Eszes, A.; Szabó, Z.S.; Ladányi-Turóczy, B.; Kalácska, I. A Low Side Lobe Level Parabolic Antenna for Meteorological Applications. Prog. Electromagn. Res. Lett. 2024, 115, 91–98. [Google Scholar] [CrossRef]
| Parameters | Values |
|---|---|
| 0.9020 | |
| q | 327.974 |
| 1575.47 | |
| 2005.13 | |
| −2244.35 | |
| −1086.36 | |
| −1335.24 |
| Zeros of | Zeros of | Relative Error of Each Zero |
|---|---|---|
| 0.0004 | ||
| 0.0003 | ||
| 0.0002 | ||
| 0.0002 | ||
| 0.0001 | ||
| 0.0001 | ||
| 0.00009 | ||
| 0.00008 | ||
| 0.00007 | ||
| 0.00006 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martin, P.; Ramos-Andrade, J.P.; Caro-Pérez, F.; Lastra, F. Accurate Analytical Approximation for the Bessel Function J2(x). Math. Comput. Appl. 2024, 29, 63. https://doi.org/10.3390/mca29040063
Martin P, Ramos-Andrade JP, Caro-Pérez F, Lastra F. Accurate Analytical Approximation for the Bessel Function J2(x). Mathematical and Computational Applications. 2024; 29(4):63. https://doi.org/10.3390/mca29040063
Chicago/Turabian StyleMartin, Pablo, Juan Pablo Ramos-Andrade, Fabián Caro-Pérez, and Freddy Lastra. 2024. "Accurate Analytical Approximation for the Bessel Function J2(x)" Mathematical and Computational Applications 29, no. 4: 63. https://doi.org/10.3390/mca29040063






