A Staring Tracking Measurement Method of Resident Space Objects Based on the Star Tacker
Abstract
:1. Introduction
2. Materials and Methods
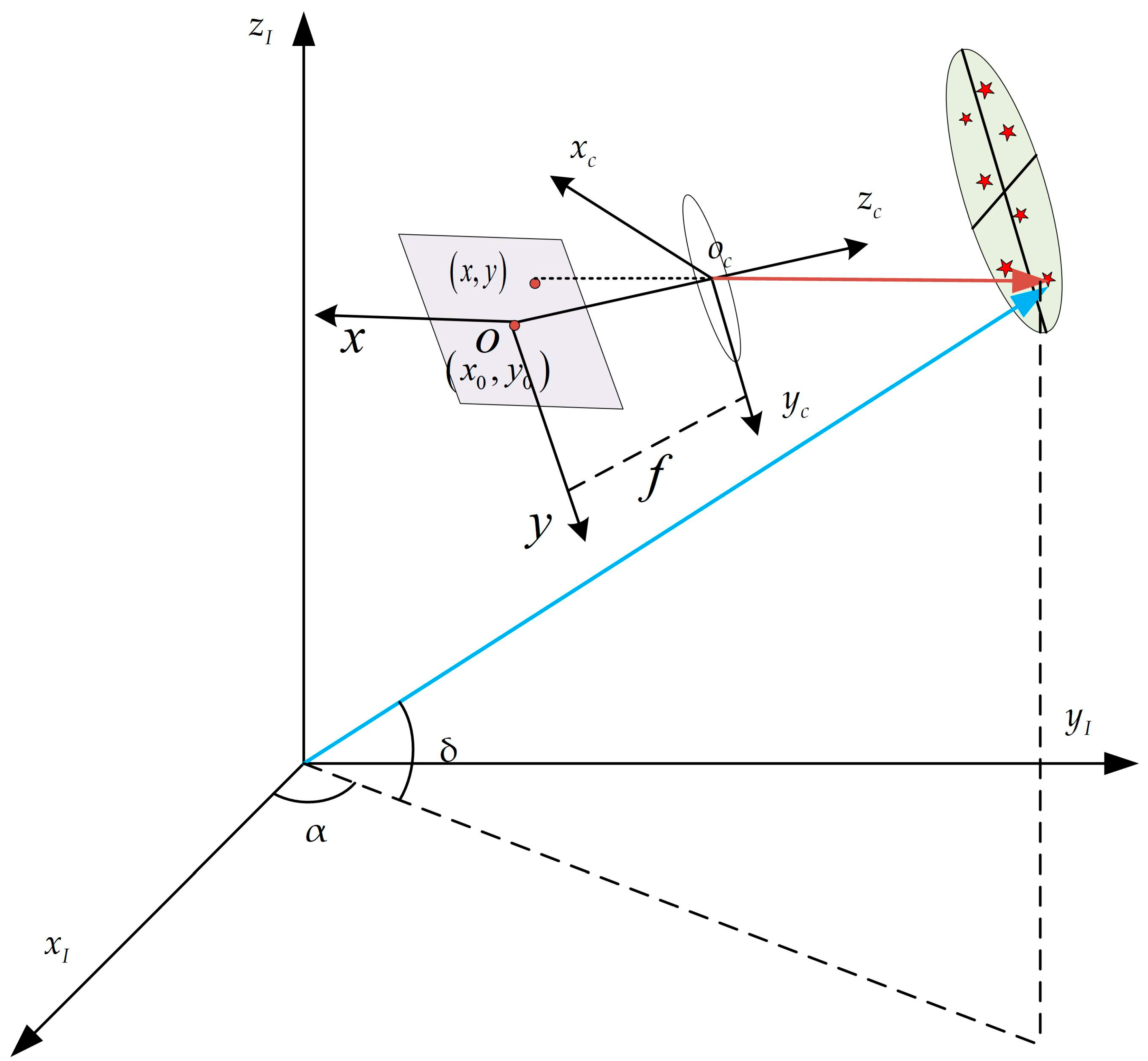
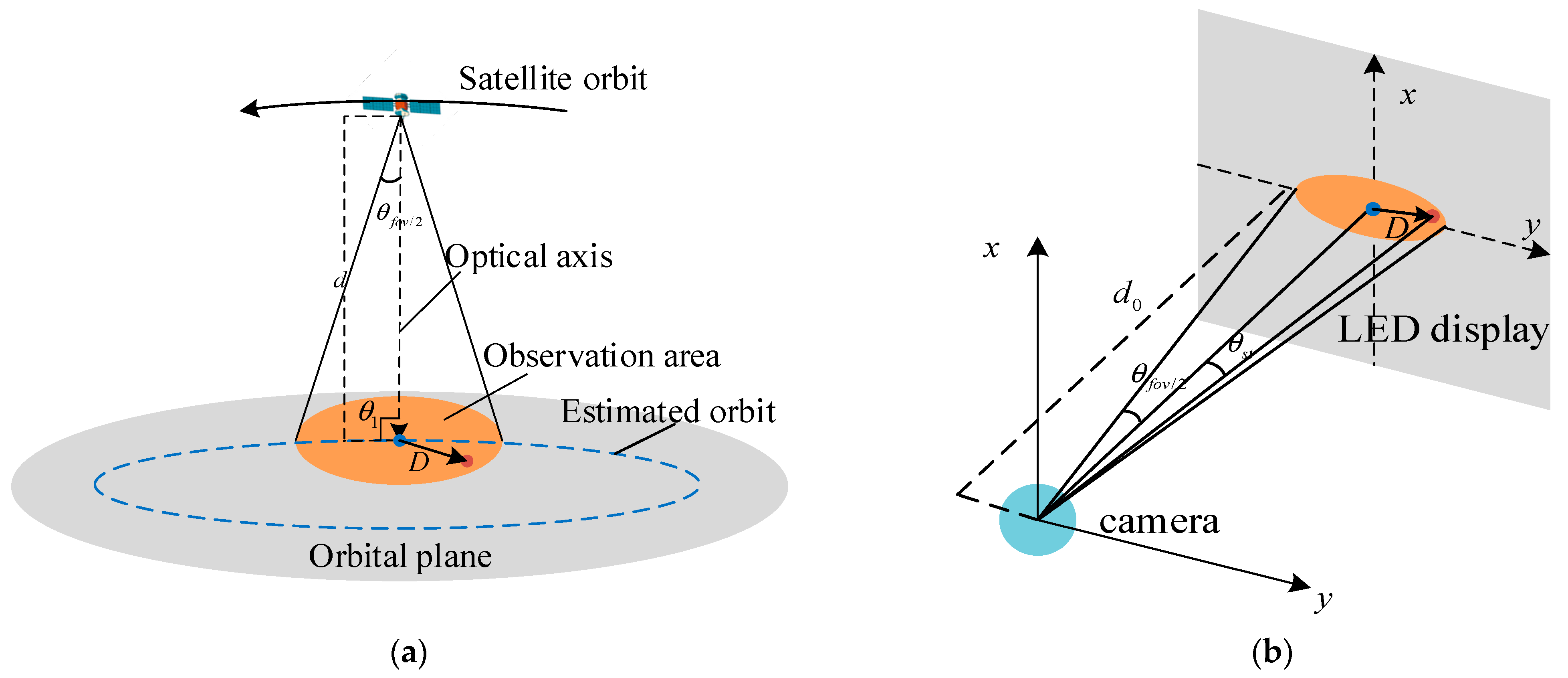
2.1. Three-Axis Attitude Model of the Satellite for RSOs Staring–Tracking Imaging
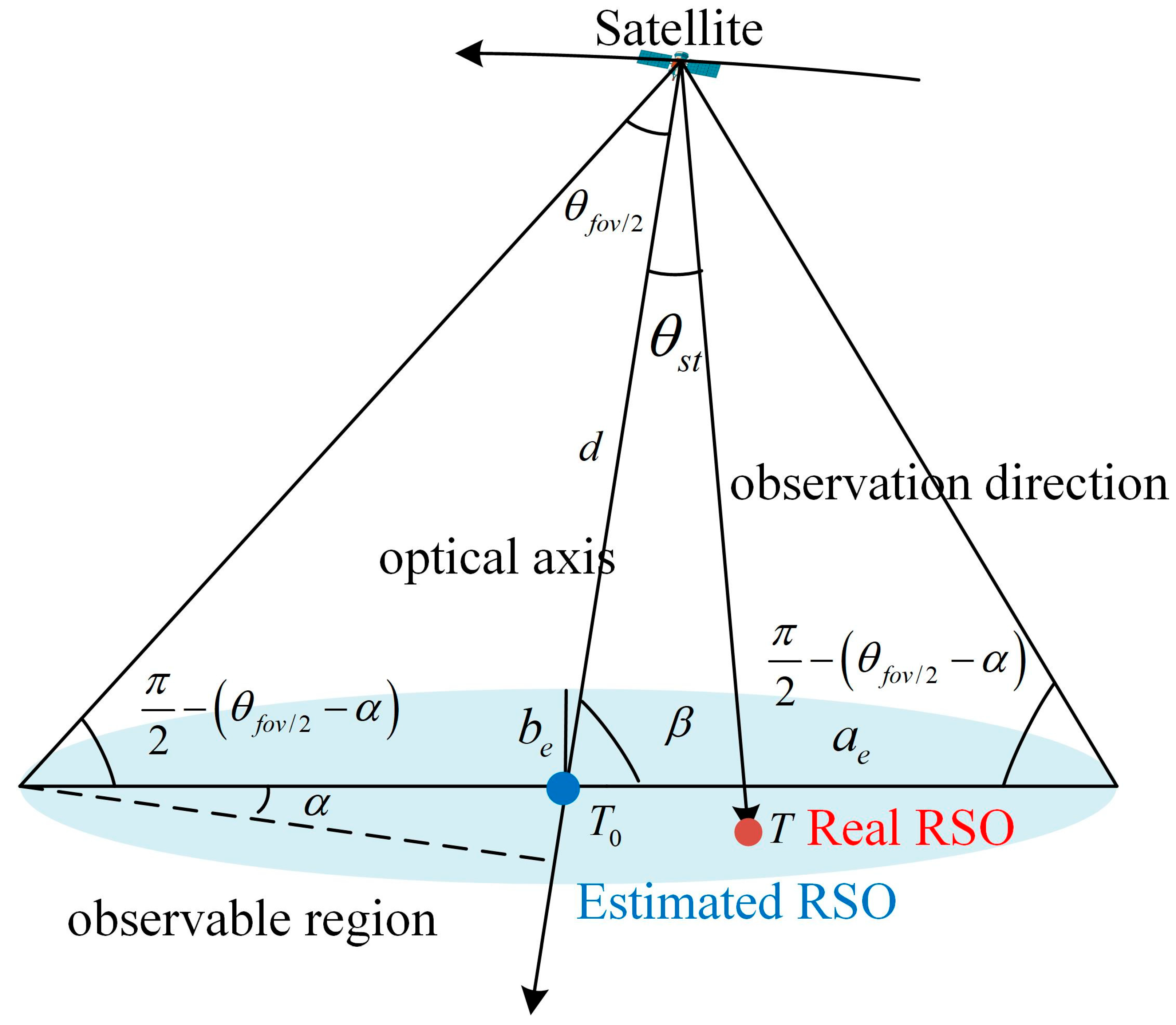
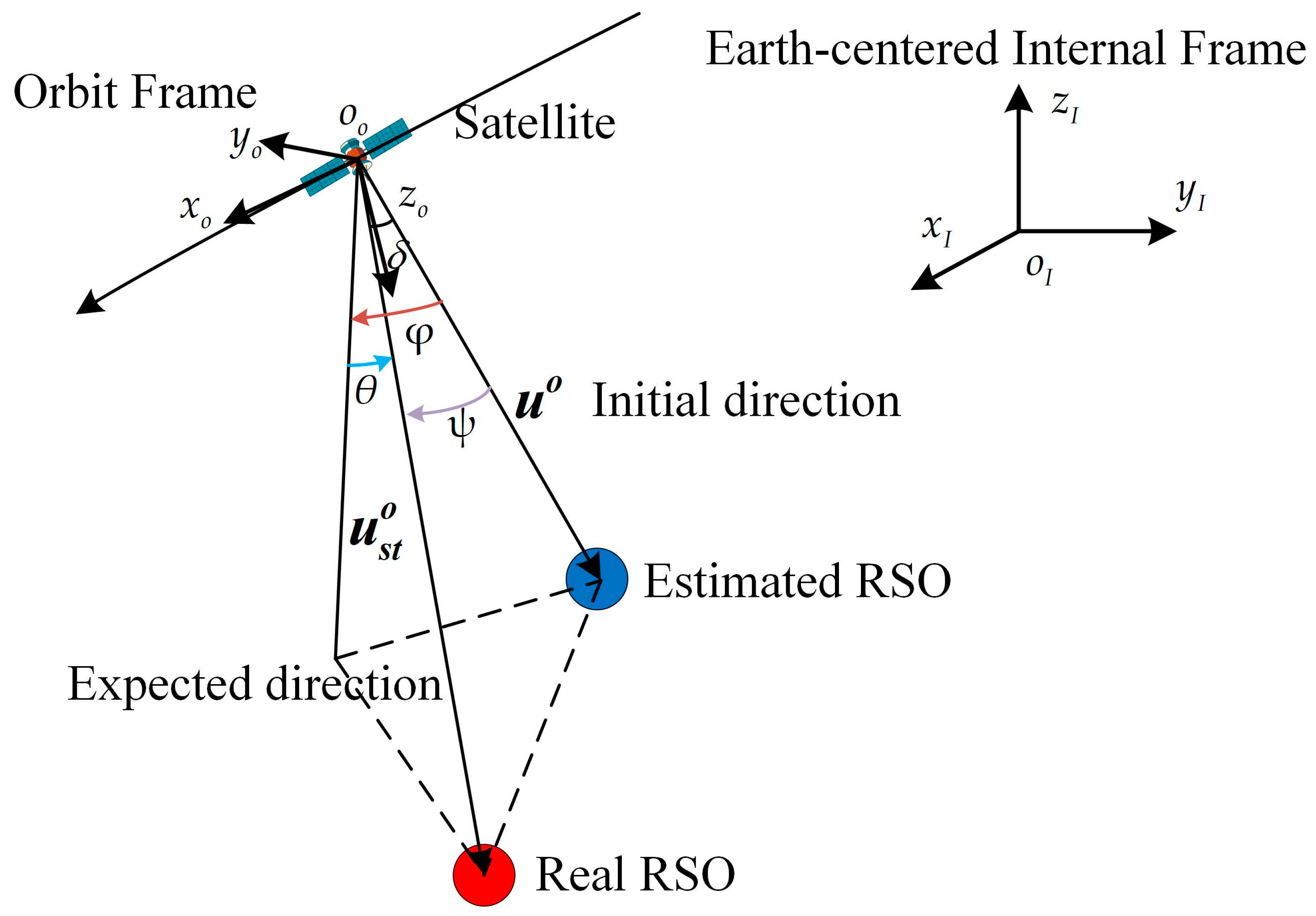
2.2. Observation Measurement Method Based on the Principle of Angular Distance Invariance
3. Results
3.1. Simulation Settings of the Proposed STM Method
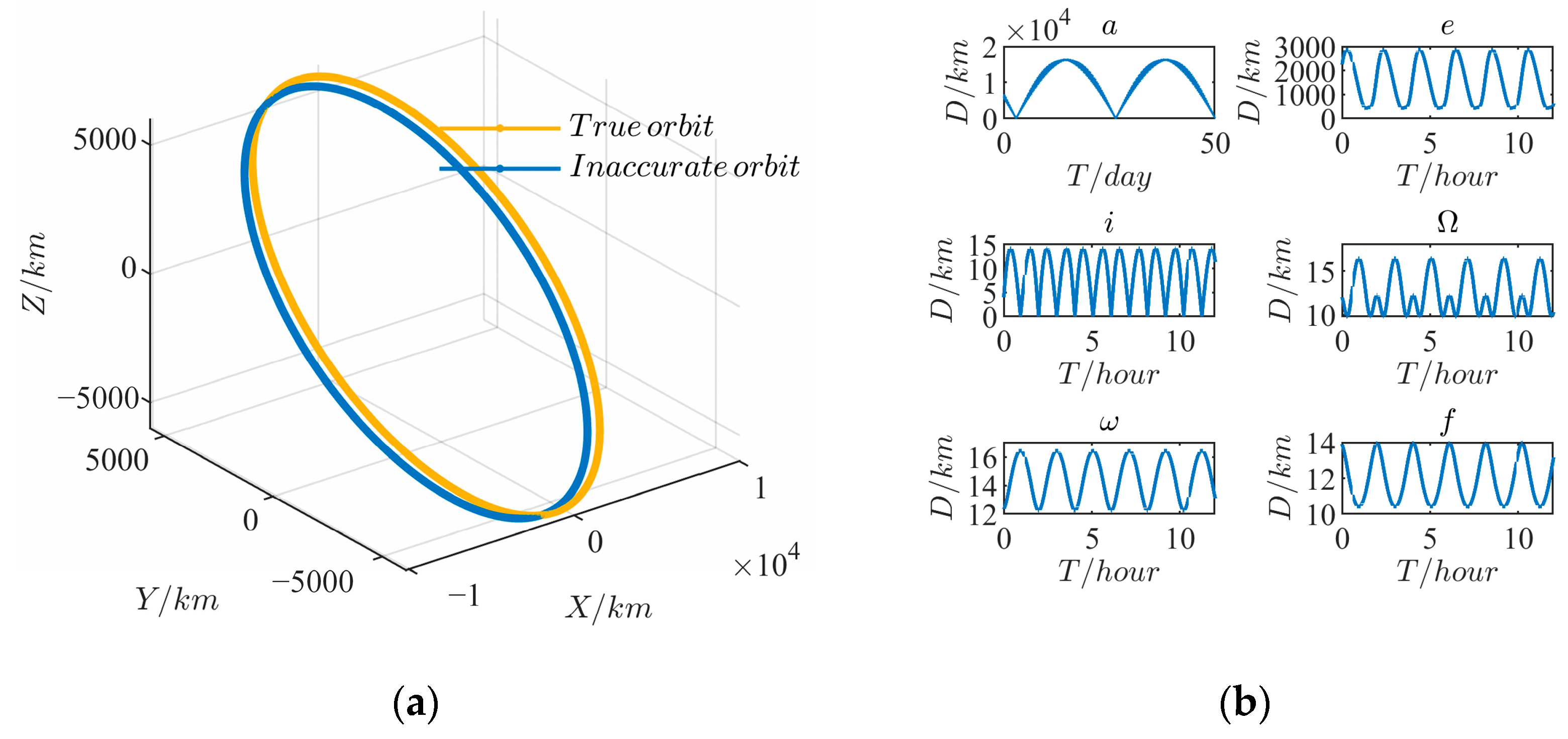
3.1.1. Orbit Parameters Settings of the Proposed STM Method
3.1.2. Star Tracker Parameters Settings
3.2. Numerical Simulation of the Proposed STM Method
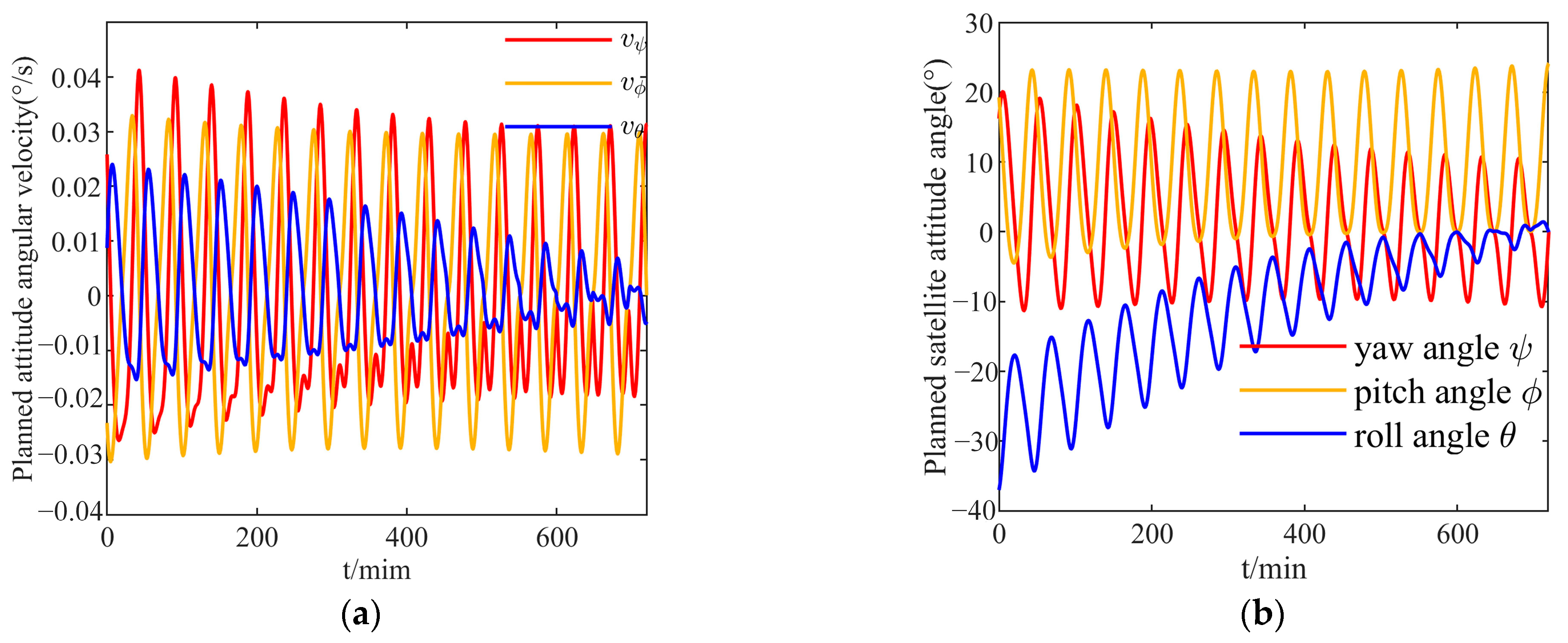
3.2.1. Simulation of the Observation Arcs in One Orbit Period of Staring–Tracking Imaging
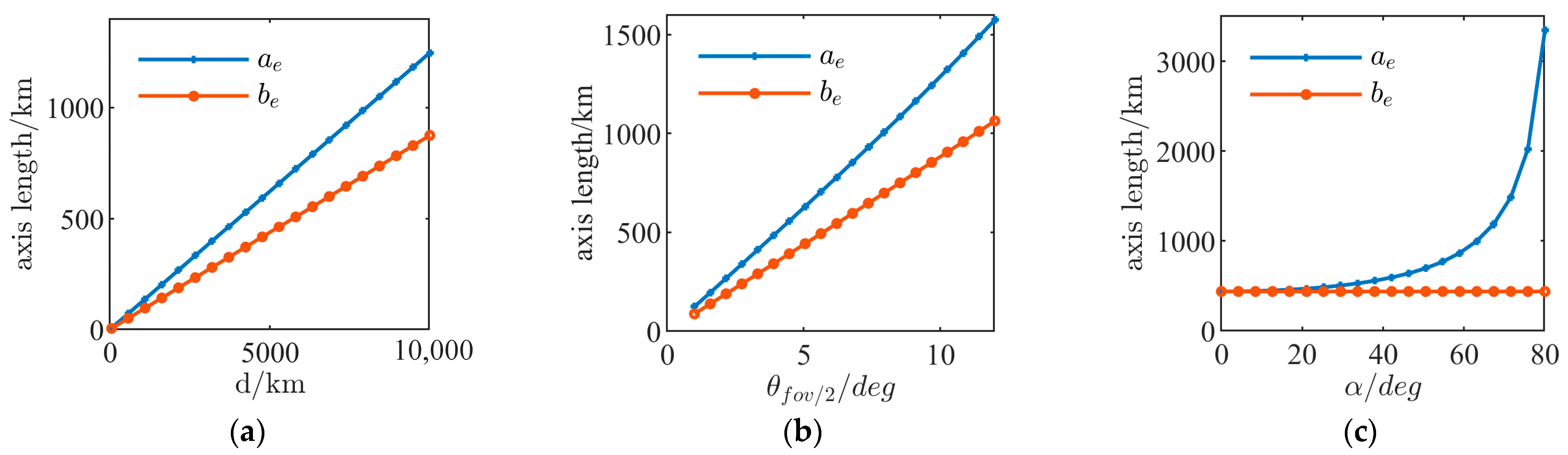
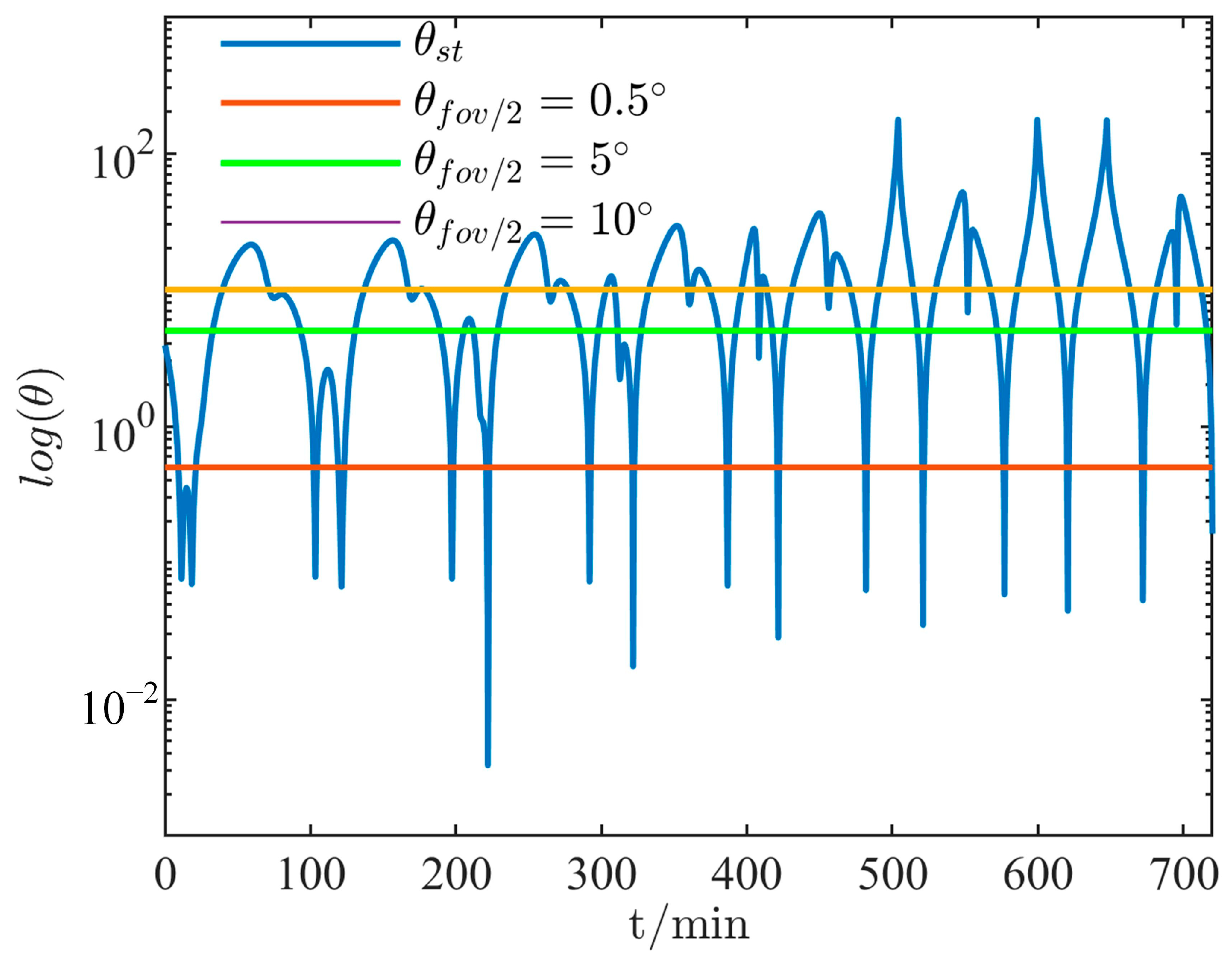
3.2.2. Simulation of the Measurement Accuracy
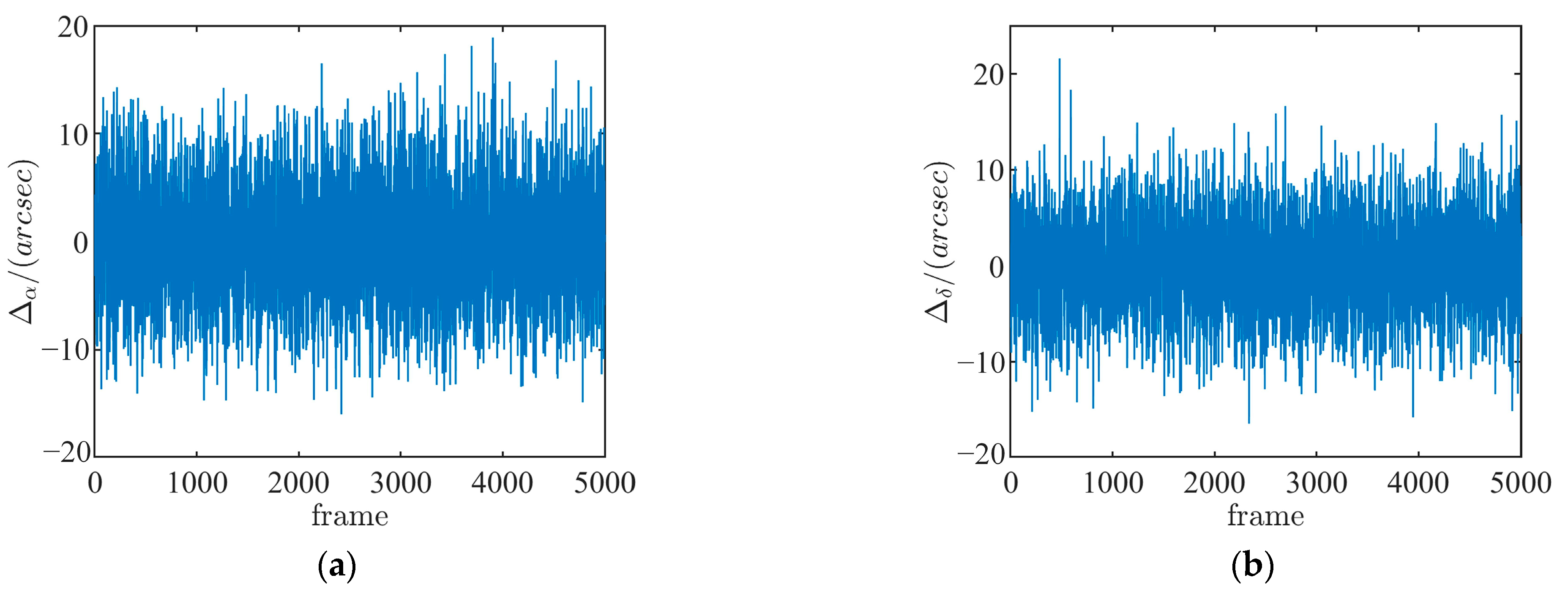
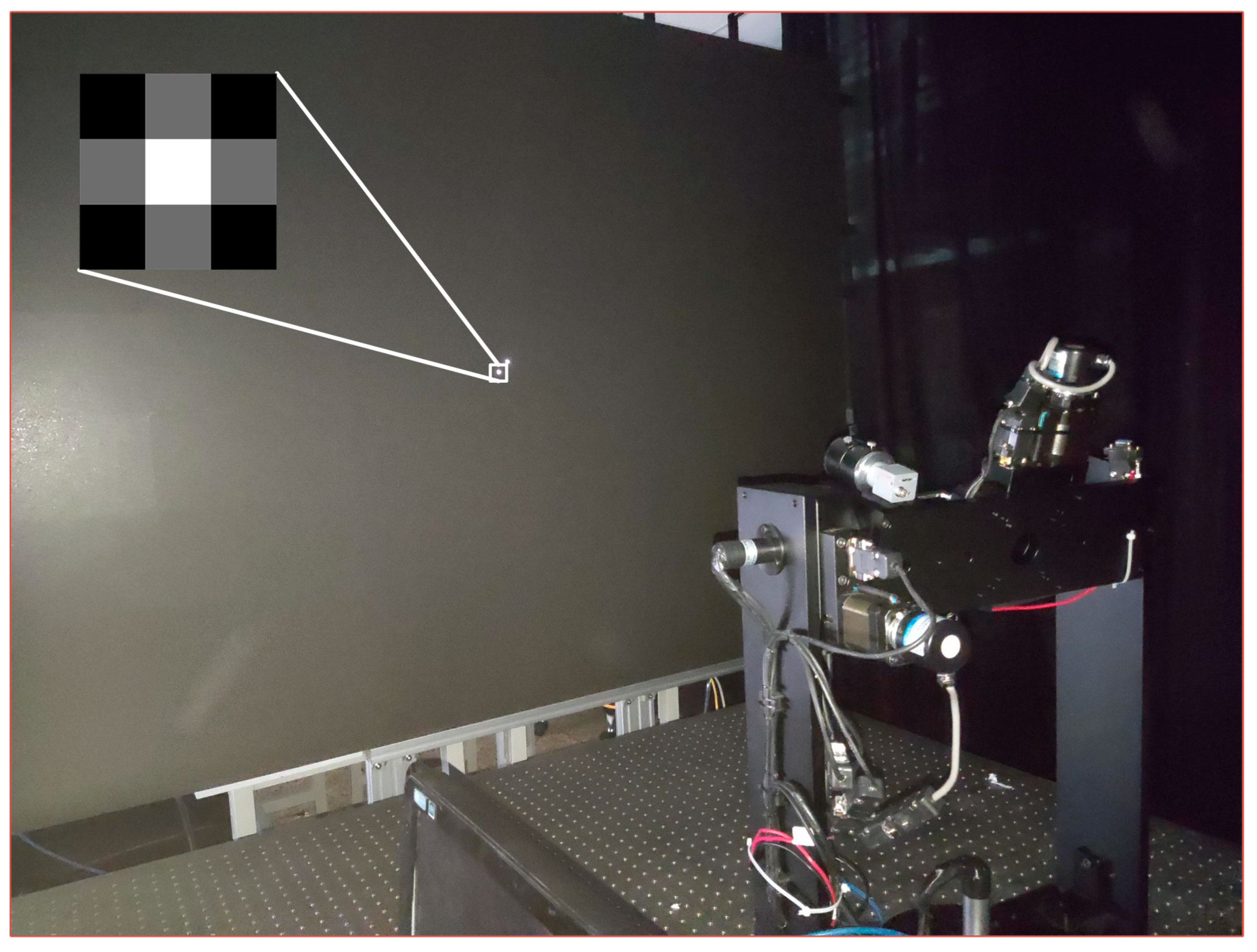
3.3. Physical Experiment
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, Y.; Li, B.; Liu, H.K.; Sang, J.Z. An analysis of close approaches and probability of collisions between LEO resident space objects and mega constellations. Geo-Spat. Inf. Sci. 2022, 25, 104–120. [Google Scholar] [CrossRef]
- Rodriguez-Villamizar, J.; Cordelli, E.; Schildknecht, T. The stare and chase observation strategy at the Swiss Optical Ground Station and Geodynamics Observatory Zimmerwald: From concept to implementation. Acta Astronaut. 2021, 189, 352–367. [Google Scholar] [CrossRef]
- Lagona, E.; Hilton, S.; Afful, A.; Gardi, A.; Sabatini, R. Autonomous Trajectory Optimisation for Intelligent Satellite Systems and Space Traffic Management. Acta Astronaut. 2022, 194, 185–201. [Google Scholar] [CrossRef]
- Pastor, A.; Sanjurjo-Rivo, M.; Escobar, D. Initial orbit determination methods for track-to-track association. Adv. Space Res. 2021, 68, 2677–2694. [Google Scholar] [CrossRef]
- AlDahoul, N.; Karim, H.A.; Momo, M.A. RGB-D based multi-modal deep learning for spacecraft and debris recognition. Sci. Rep. 2022, 12, 3924. [Google Scholar] [CrossRef] [PubMed]
- Schwab, D.; Singla, P.; O’Rourke, S. Angles-Only Initial Orbit Determination via Multivariate Gaussian Process Regression. Electronics 2022, 11, 588. [Google Scholar] [CrossRef]
- Feng, G.H.; Li, W.H.; Zhang, H. Geomagnetic Energy Approach to Space Debris Deorbiting in a Low Earth Orbit. Int. J. Aerosp. Eng. 2019, 2019, 5876861. [Google Scholar] [CrossRef]
- Du, J.L.; Chen, J.Y.; Li, B.; Sang, J.Z. Tentative design of SBSS constellations for LEO debris catalog maintenance. Acta Astronaut. 2019, 155, 379–388. [Google Scholar] [CrossRef]
- Yates, J.M.; Spanbauer, B.W.; Black, J.T. Geostationary orbit development and evaluation for space situational awareness. Acta Astronaut. 2012, 81, 256–272. [Google Scholar] [CrossRef]
- Utzmann, J.; Ferreira, L.; Strasser, N.; Vives, G.; Probst, D.; Lievre, N. Optical In-Situ Monitor a Breadboard System to Enable Space-Based Optical Observation of Space Debris. In Proceedings of the 7th European Conference on Space Debris, Darmstadt, Germany, 18–21 April 2017. [Google Scholar]
- Hu, Y.P.; Li, K.B.; Liang, Y.G.; Chen, L. Review on strategies of space-based optical space situational awareness. J. Syst. Eng. Electron. 2021, 32, 1152–1166. [Google Scholar] [CrossRef]
- Spiller, D.; Magionami, E.; Schiattarella, V.; Curti, F.; Facchinetti, C.; Ansalone, L.; Tuozzi, A. On-orbit recognition of resident space objects by using star trackers. Acta Astronaut. 2020, 177, 478–496. [Google Scholar] [CrossRef]
- Liebe, C.C. Accuracy performance of star trackers—A tutorial. IEEE Trans. Aerosp. Electron. Syst. 2002, 38, 587–599. [Google Scholar] [CrossRef]
- Liu, M.Y.; Wang, H.; Yi, H.W.; Xue, Y.K.; Wen, D.S.; Wang, F.; Shen, Y.; Pan, Y. Space Debris Detection and Positioning Technology Based on Multiple Star Trackers. Appl. Sci. 2022, 12, 3593. [Google Scholar] [CrossRef]
- Zhang, X.Y.; Xiang, J.H.; Zhang, Y.L. Error analysis of line-of-sight measurement for video satellite. Adv. Mech. Eng. 2017, 9, 1687814017742823. [Google Scholar] [CrossRef]
- Lei, X.X.; Wang, K.P.; Zhang, P.; Pan, T.; Li, H.F.; Sang, J.Z.; He, D.L. A geometrical approach to association of space-based very short-arc LEO tracks. Adv. Space Res. 2018, 62, 542–553. [Google Scholar] [CrossRef]
- Ansalone, L.; Curti, F. A genetic algorithm for Initial Orbit Determination from a too short arc optical observation. Adv. Space Res. 2013, 52, 477–489. [Google Scholar] [CrossRef]
- Hu, J.; Li, B.; Li, J. Initial orbit determination utilizing solution group optimization. IEEE Trans. Aerosp. Electron. Syst. 2019, 56, 897–911. [Google Scholar] [CrossRef]
- Xie, H.; Sun, S.; Xue, T.; Xu, W.; Liu, H.; Lei, L.; Zhang, Y. A Multimodal Differential Evolution Algorithm in Initial Orbit Determination for a Space-Based Too Short Arc. Remote Sens. 2022, 14, 5140. [Google Scholar] [CrossRef]
- Shang, H.T.; Chen, D.F.; Cao, H.W.; Fu, T.; Gao, M.C. Initial Orbit Determination Using Very Short Arc Data Based on Double-Station Observation. IEEE Trans. Aerosp. Electron. Syst. 2019, 55, 1596–1611. [Google Scholar] [CrossRef]
- Zhang, S.; Fu, T.; Chen, D.; Ding, S.; Gao, M. An Initial Orbit Determination Method Using Single-Site Very Short Arc Radar Observations. IEEE Trans. Aerosp. Electron. Syst. 2020, 56, 1856–1872. [Google Scholar] [CrossRef]
- Huang, Q.; Zhang, Y.; Feng, F. Space target synchronization association method under distributed star sensor. Chin. J. Sci. Instrum. 2019, 40, 106–113. [Google Scholar]
- Huang, Q.; Zhang, Y.; Feng, F. Algorithm of space track segment association under distributed star sensor. Syst. Eng. Electron. 2020, 42, 1007–1013. [Google Scholar]
- Huang, Q.; Zhang, Y.; Feng, F. Short-arc association algorithm for space target based on sine fitting. Chin. Space Sci. Technol. 2020, 40, 111–118. [Google Scholar] [CrossRef]
- Qian, Y.-J.; Zong, K.; Yang, X.-D.; Si, Z.; Gao, F. Forced resonance orbit analysis of binary asteroid system with consideration of solar radiation pressure. Nonlinear Dyn. 2022, 109, 1399–1422. [Google Scholar] [CrossRef]
- Xiao, Y.; de Ruiter, A.; Ye, D.; Sun, Z. Attitude Coordination Control for Flexible Spacecraft Formation Flying with Guaranteed Performance Bounds. IEEE Trans. Aerosp. Electron. Syst. 2022. [Google Scholar] [CrossRef]
- Zhang, H.Z.; Ye, D.; Xiao, Y.; Sun, Z.W. Adaptive Control on SE (3) for Spacecraft Pose Tracking with Harmonic Disturbance and Input Saturation. IEEE Trans. Aerosp. Electron. Syst. 2022, 58, 4578–4594. [Google Scholar] [CrossRef]
- d’Angelo, P.; Kuschk, G.; Reinartz, P. Evaluation of Skybox Video and Still Image Products. In Proceedings of the ISPRS Technical Commission I Symposium, Denver, CO, USA, 17–20 November 2014; pp. 95–99. [Google Scholar]
- Jiang, L.; Yang, X.B. Study on Enlarging the Searching Scope of Staring Area and Tracking Imaging of Dynamic Targets by Optical Satellites. IEEE Sens. J. 2021, 21, 5349–5358. [Google Scholar] [CrossRef]
- Wang, J.; Yu, P.; Ya, C.; Ren, J.; He, B. Space Optical Remote Sensor Image Motion Velocity Vector Computational Modeling. Acta Opt. Sin. 2004, 24, 1585–1589. [Google Scholar]
- Lu, K.L.; Liu, E.H.; Zhao, R.J.; Tian, H.; Zhang, H. A Fast Star Identification Algorithm of Star Sensors in the LIS Mode. Remote Sens. 2022, 14, 1739. [Google Scholar] [CrossRef]
- Chen, X.D.; Xing, F.; You, Z.; Zhong, X.; Qi, K.H. On-Orbit High-Accuracy Geometric Calibration for Remote Sensing Camera Based on Star Sources Observation. IEEE Trans. Geosci. Remote Sens. 2022, 60, 5608211. [Google Scholar] [CrossRef]
- Haibo, L.I.U.; Dezhi, S.U.; Jichun, T.A.N.; Jiankun, Y.; Xiujian, L.I. An Approach to Star Image Simulation for Star Sensor Considering Satellite Orbit Motion and Effect of Image Shift. J. Chin. Soc. Astronaut. 2011, 32, 1190–1194. [Google Scholar]
- Wan, X.; Wang, G.; Wei, X.; Li, J.; Zhang, G. ODCC: A Dynamic Star Spots Extraction Method for Star Sensors. IEEE Trans. Instrum. Meas. 2021, 70, 3073716. [Google Scholar] [CrossRef]




| Serial Number | Orbital Elements | Actual Value | Error Value |
|---|---|---|---|
| 1 | Semi-major axis/km | 6928.14 | 20 |
| 2 | Eccentricity | 0 | 0.1 |
| 3 | Inclination/(deg) | 97.5521 | 0.1 |
| 4 | Longitude of the ascending node/(deg) | 203.282 | 0.1 |
| 5 | Argument of periapsis/(deg) | 0 | 0.1 |
| 6 | True anomaly/(deg) | 0.10826 | 0.1 |
| Orbital Elements | Observation Satellite Orbit | Actual RSO Orbit | Estimated RSO Orbit |
|---|---|---|---|
| Semi-major axis/km | 6928.14 | 7000.14 | 6980.14 |
| Eccentricity | 0 | 0 | 0.1 |
| Inclination/(deg) | 97.5521 | 97.7141 | 97.7141 |
| Longitude of the ascending node/(deg) | 203.282 | 163.536 | 163.536 |
| Argument of periapsis/(deg) | 0 | 0 | 0 |
| True anomaly/(deg) | 0.108 26 | 0 | 0 |
| Parameter | Value |
|---|---|
| Focal length | 35.5 mm |
| Field of view | |
| Resolution | |
| Pixel length | |
| Exposure time | 100 ms |
| Star magnitude limit | 5.2 Mv |
| Serial Number | Ground Experiment Parameters | Value |
|---|---|---|
| 1 | Focal length of the camera | 7 mm |
| 2 | Pixel size of CMOS | 7 μm |
| 3 | Pixel size of LED | 2 mm |
| 4 | Size of the LED panel | 1.94 m × 1.44 m |
| 5 | Imaging frequency | 5 Hz |
| 6 | Object distance | 60 cm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, T.; Yang, X.; Fu, Z.; Wu, M.; Gao, S. A Staring Tracking Measurement Method of Resident Space Objects Based on the Star Tacker. Photonics 2023, 10, 288. https://doi.org/10.3390/photonics10030288
Xu T, Yang X, Fu Z, Wu M, Gao S. A Staring Tracking Measurement Method of Resident Space Objects Based on the Star Tacker. Photonics. 2023; 10(3):288. https://doi.org/10.3390/photonics10030288
Chicago/Turabian StyleXu, Tingting, Xiubin Yang, Zongqiang Fu, Mo Wu, and Suining Gao. 2023. "A Staring Tracking Measurement Method of Resident Space Objects Based on the Star Tacker" Photonics 10, no. 3: 288. https://doi.org/10.3390/photonics10030288
APA StyleXu, T., Yang, X., Fu, Z., Wu, M., & Gao, S. (2023). A Staring Tracking Measurement Method of Resident Space Objects Based on the Star Tacker. Photonics, 10(3), 288. https://doi.org/10.3390/photonics10030288




