Suppression of Nonlinear Optical Effects in DWDM-PON by Frequency Modulation Non-Coherent Detection
Abstract
1. Introduction
2. System Configuration and Simulation Model
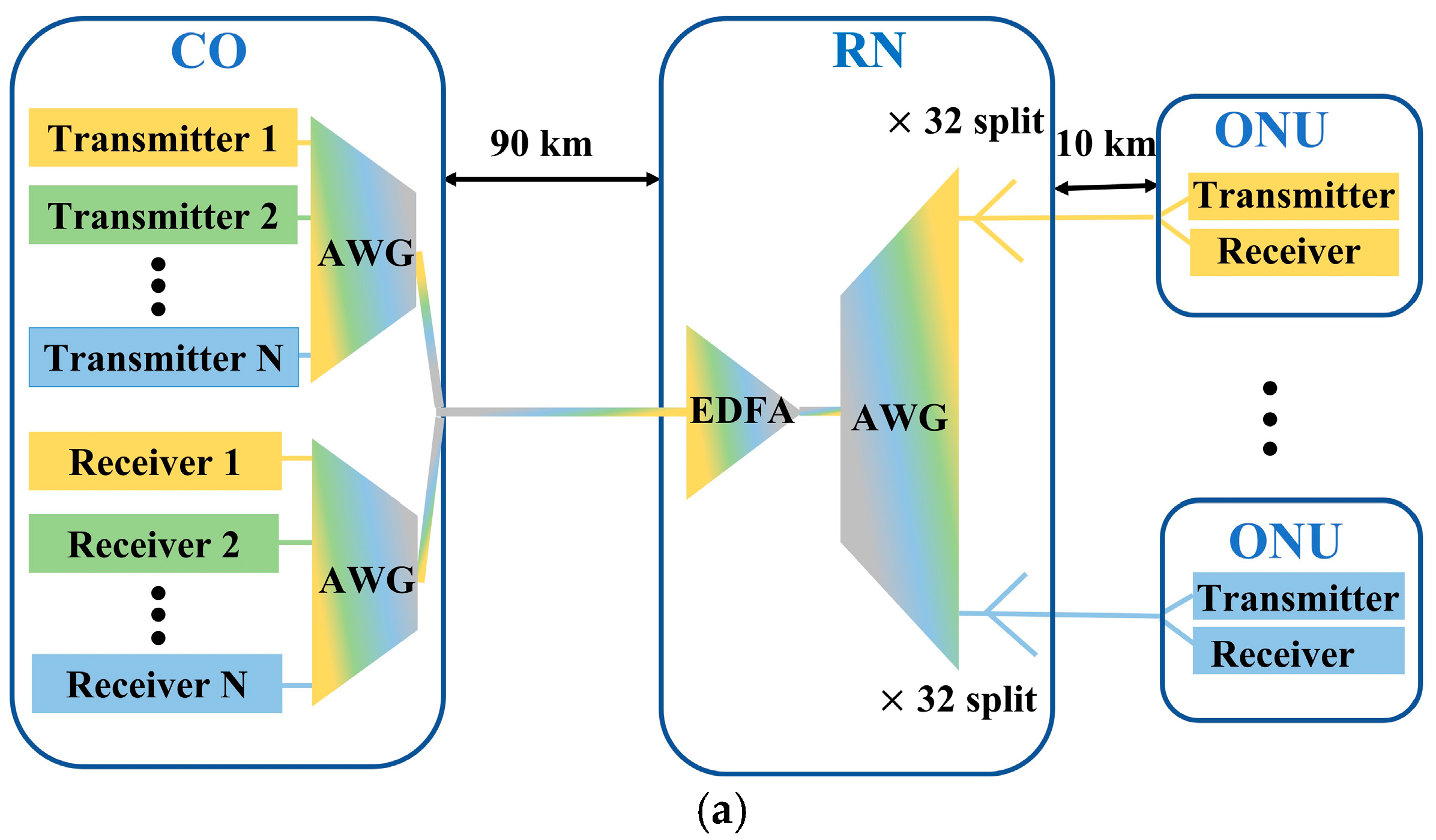
2.1. System Configuration
2.2. Simulation Model
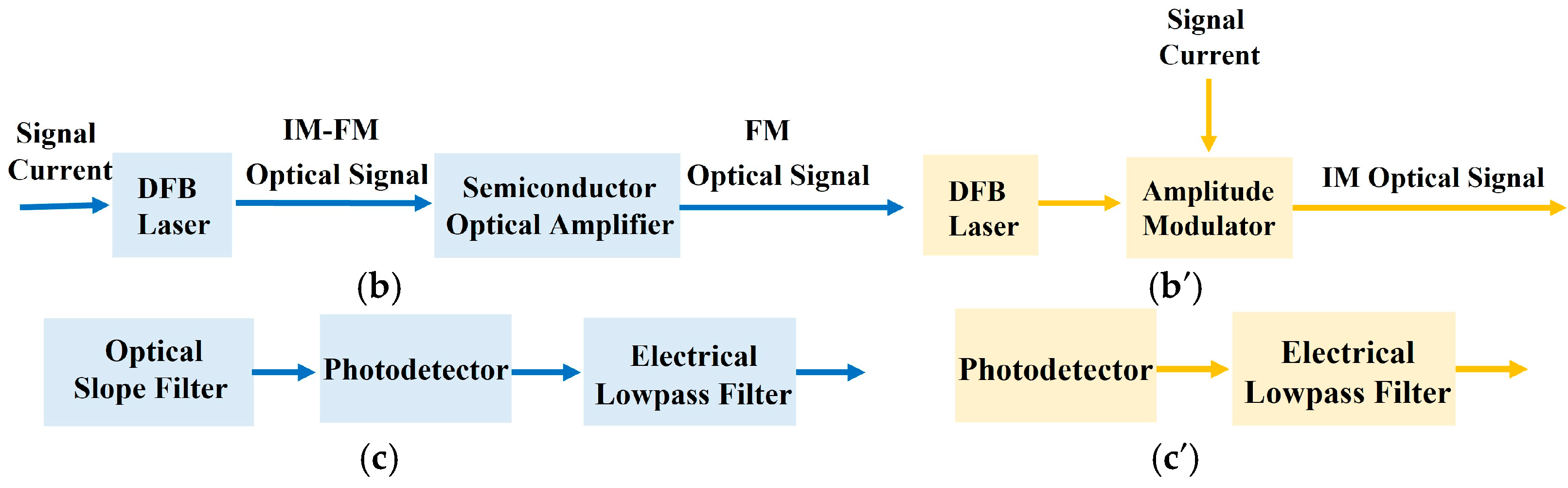
2.2.1. Optical Transmitter
2.2.2. Fiber-Optic Channel
2.2.3. Optical Receiver
2.2.4. The Other Devices
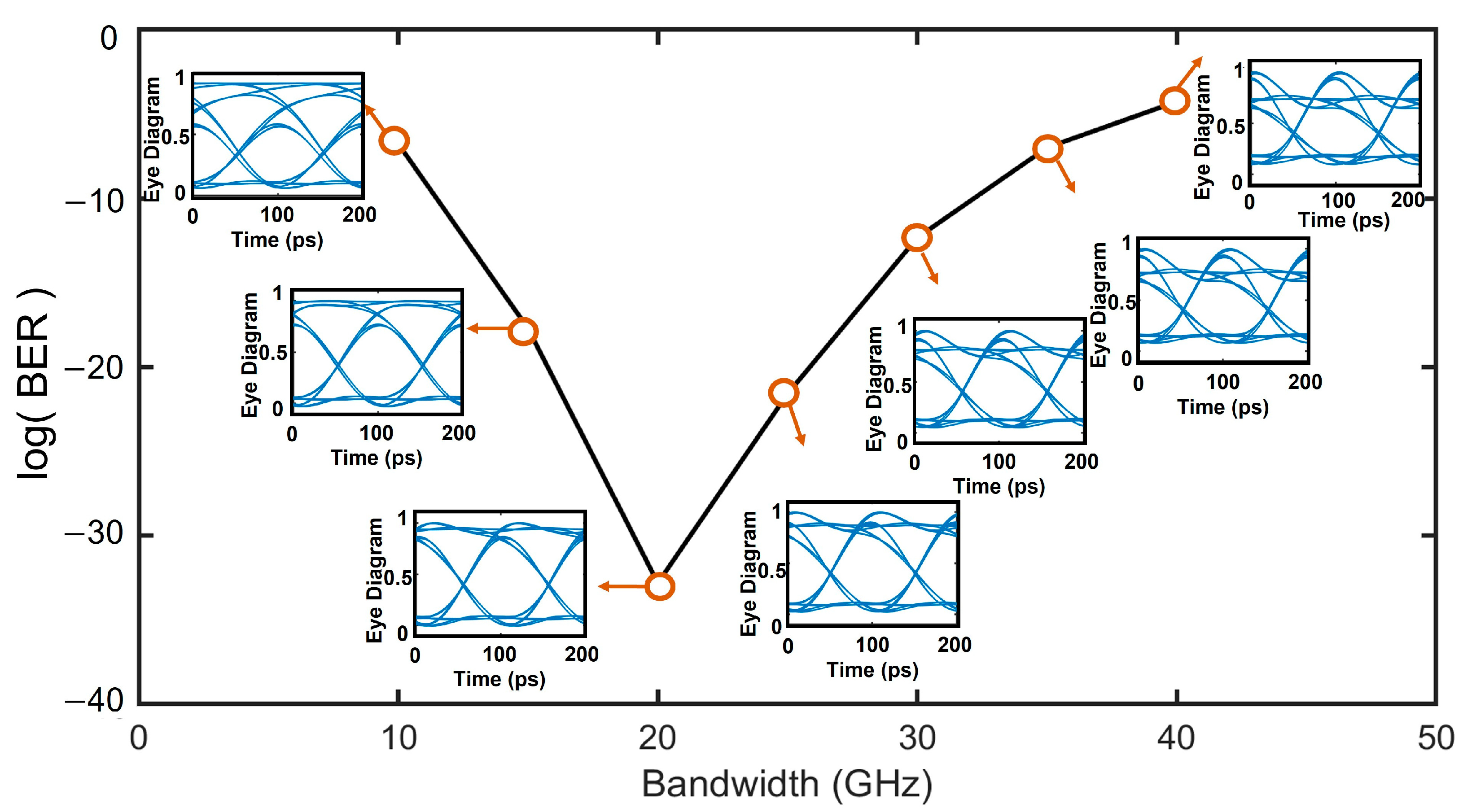
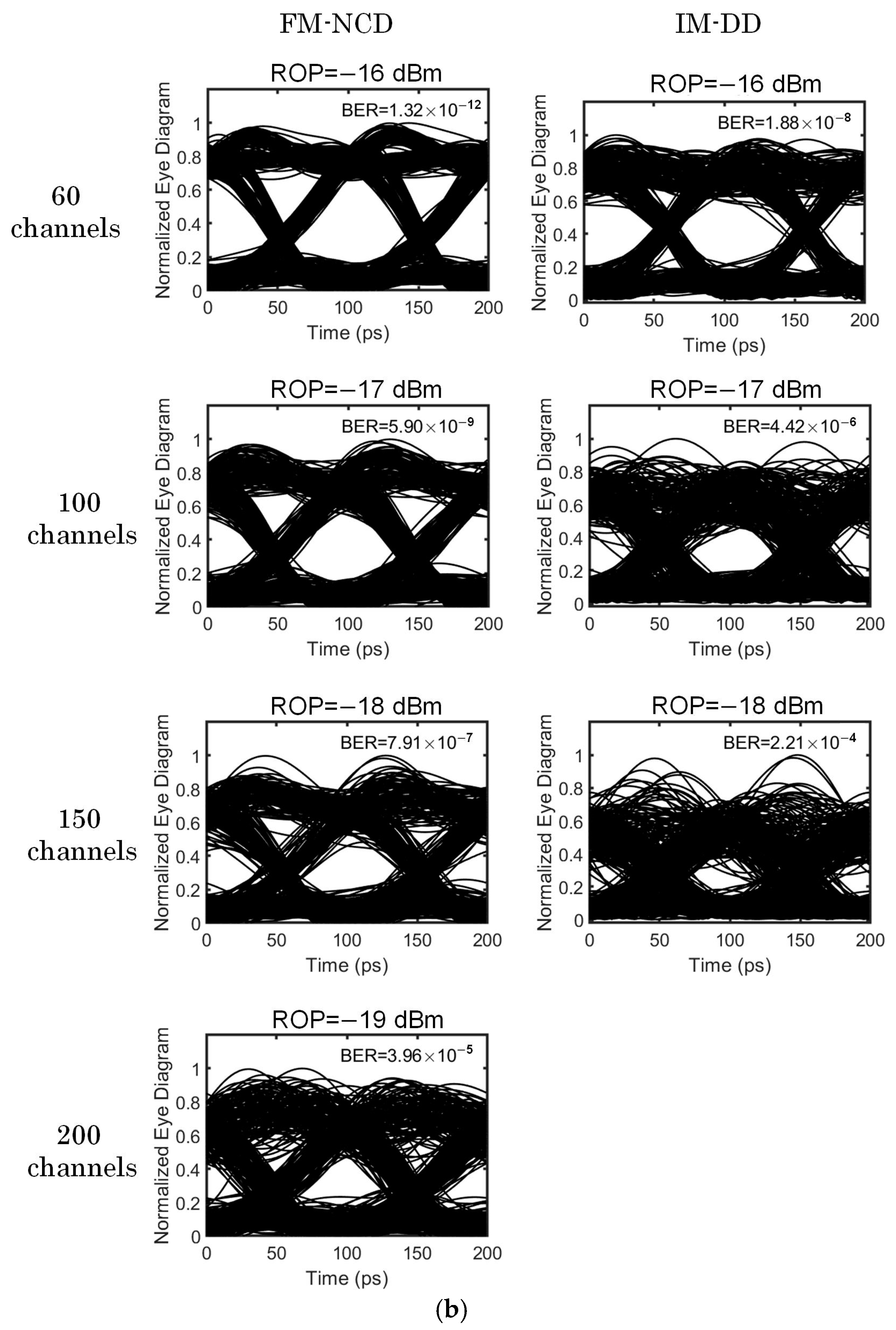
3. Numerical Simulation Results
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tommaso, M.; Fabio, G.; Vittorio, P. Passive optical access networks: State of the art and future evolution. Photonics 2014, 1, 323–346. [Google Scholar]
- ITUT, G.987.1; 10-Gigabit-Capable Passive Optical Networks (XG-PON): General Requirements. International Telecommunication Union: Geneve, Switzerland, 2016.
- ITUT, G.987.2; 10-Gigabit-Capable Passive Optical Networks (XG-PON): Physical Media Dependent (PMD) Layer Specification. International Telecommunication Union: Geneve, Switzerland, 2016.
- Banerjee, A.; Park, Y.; Clarke, F.; Song, H.; Yang, S.; Kramer, G.; Kim, K.; Mukherjee, B. Wavelength-division-multiplexed passive optical network (WDM-PON) technologies for broadband access: A review. J. Opt. Netw. 2005, 4, 737–758. [Google Scholar] [CrossRef]
- ITUT, G.989.1; 40-Gigabit-Capable Passive Optical Networks (NG-PON2): General Requirements. International Telecommunication Union: Geneve, Switzerland, 2013.
- Talli, G.; Chow, C.W.; Townsend, P.; Davey, R.; Ridder, T.D.; Qiu, X.Z.; Ossieur, P.; Krimmel, H.; Smith, D.; Lealman, I.; et al. Integrated metro and access network: PIEMAN. In Proceedings of the 12th European Conference on Networks and Optical Communications (NOC), Stockholm, Sweden, 18–21 June 2007; p. 493. [Google Scholar]
- Muciaccia, T.; Gargano, F.; Passaro, V.M.N. A TWDM-PON with Advanced Modulation Techniques and a Multi-Pump Raman Amplifier for Cost-Effective Migration to Future UDWDM-PONs. J. Light. Technol. 2015, 14, 2986–2996. [Google Scholar] [CrossRef]
- Davey, R.P.; Healey, P.; Hope, I.; Watkinson, P.; Payne, D.B.; Marmur, O. DWDM reach extension of a GPON to 135 km. J. Light. Technol. 2006, 24, 29–31. [Google Scholar] [CrossRef]
- Lee, S.M.; Mun, S.G.; Kim, M.H.; Lee, C.H. Demonstration of a long-reach DWDM-PON for consolidation of metro and access network. J. Light. Technol. 2007, 25, 271–276. [Google Scholar] [CrossRef]
- Boyd, R.W. Nonlinear Optics, 3rd ed.; Academic Press: Boston, MA, USA, 2008. [Google Scholar]
- Agrawal, G.P. Fiber Optic Communications Systems, 4th ed.; John Wiley & Sons: New York, NY, USA, 2010. [Google Scholar]
- Bellotti, G.; Bigo, S. Cross-phase modulation suppressor for multispan dispersion-managed WDM transmissions. IEEE Photon. Technol. Lett. 2000, 12, 726–728. [Google Scholar] [CrossRef]
- Marhic, M.E.; Yang, F.S.; Kazovsky, L.G. Cancellation of stimulated-Raman-scattering crosstalk in wavelength-division-multiplexed optical communication systems by series or parallel techniques. J. Opt. Soc. Am. B 1998, 15, 957–963. [Google Scholar] [CrossRef]
- Ip, E.; Kahn, J.M. Compensation of dispersion and nonlinear impairments using digital backpropagation. J. Lightw. Technol. 2008, 26, 3416–3425. [Google Scholar] [CrossRef]
- Kikuchi, K. Fundamentals of coherent optical fiber communications. J. Lightw. Technol. 2016, 34, 157–179. [Google Scholar] [CrossRef]
- Li, X. Optoelectronic Devices: Design, Modeling, and Simulation; Cambridge University Press: Cambridge, UK, 2009; pp. 1–361. [Google Scholar]
- Xin, L.; Zhao, J.; Li, X. Suppression of mode partition noise in FP Laser by frequency modulation non-coherent detection. IEEE Photon. J. 2022, 14, 7201310. [Google Scholar] [CrossRef]
- Zuo, C.; Li, X. Polarization-Discriminated RSOA–EAM for Colorless Transmitter in WDM–PON. Appl. Sci. 2020, 10, 9049. [Google Scholar] [CrossRef]
- Agrawal, G.P. Nonlinear Fiber Optics, 3rd ed.; Academic Press: New York, NY, USA, 2001. [Google Scholar]
- Lee, H.; Agrawal, G.P. Suppression of stimulated Brillouin scattering in optical fibers using fiber Bragg grating. Opt. Express 2003, 11, 3467–3472. [Google Scholar] [CrossRef] [PubMed]
- Willems, F.W.; Muys, W. Suppression of interferometric noise in externally modulated lightwave AM-CATV systems by phase modulation. Electron. Lett. 1993, 29, 2062–2063. [Google Scholar] [CrossRef]
- Boggio, J.M.C.; Marconi, J.D.; Fragnito, H.L. Experimental and numerical investigation of the SBS-threshold increase in an optical fiber by applying strain distributions. J. Lightwave Technol. 2005, 23, 3808–3814. [Google Scholar] [CrossRef]
- Sinkin, O.V.; Holzlohner, R.; Zweck, J.; Menyuk, C.R. Optimization of the split-step Fourier method in modeling optical fiber communication systems. J. Light. Technol. 2003, 1, 61–68. [Google Scholar] [CrossRef]
- Hardin, R.H.; Tappert, F.D. Applications of the split step fourier method to the numerical solution of nonlinear and variable coefficient wave equations. SIAM Rev. Chronicle. 1973, 15, 423. [Google Scholar]
- Carema, A.; Curri, V.; Gaudino, R.; Poggiolini, P.; Benedetto, S. A time-domain optical transmission system simulation package accounting for nonlinear and polarization-related effects in fiber. IEEE J. Select. Areas Commun. 1997, 15, 751–764. [Google Scholar] [CrossRef]
- Domash, L.; Wu, M.; Nemchuk, N.; Ma, E. Tunable and switchable multiple-cavity thin film filters. J. Lightw. Technol. 2004, 22, 126–135. [Google Scholar] [CrossRef]
- Saunders, A.; Patel, B.L.; Harvey, H.J.; Robinson, A. Impact of cross-phase modulation for WDM systems over positive and negative dispersion NZ-DSF and methods for its suppression. IEEE Electron. Lett. 1996, 32, 2206–2207. [Google Scholar] [CrossRef]
- Rapp, L. Experimental investigation of signal distortion induced by cross-phase modulation combined with dispersion. IEEE Photon. Technol. Lett. 1997, 9, 1592–1594. [Google Scholar] [CrossRef]
- Shtaif, M.; Eiselt, M.; Garrett, L.D. Cross-phase modulation distortion measurements in multispan WDM systems. IEEE Photon. Technol. Lett. 1997, 12, 1592–1594. [Google Scholar] [CrossRef]
- Ho, K.P. Statistical properties of stimulated Raman crosstalk in WDM systems. J. Lightw. Technol. 2000, 18, 915–921. [Google Scholar]
- Jiang, Z.; Fan, C. A comprehensive study on XPM- and SRS-induced noise in cascaded IM-DD optical fiber transmission systems. J. Lightw. Technol. 2003, 21, 953–960. [Google Scholar] [CrossRef]
- Jones, D.J.; Zhang, L.M.; Carroll, J.E.; Marcenac, D.D. Dynamics of monolithic passively mode-locked semiconductor lasers. IEEE J. Quantum Electron. 1995, 31, 1051–1058. [Google Scholar] [CrossRef]
- Zhao, J.; Shi, K.; Yu, Y.; Barry, L.P. Theoretical analysis of tunable three-section slotted Fabry-Perot lasers based on time-domain travelingwave model. IEEE J. Sel. Topics Quantum Electron. 2013, 19, 1–8. [Google Scholar] [CrossRef]
- Park, J.; Li, X.; Huang, W.P. Performance simulation and design optimization of gain-clamped semiconductor optical amplifiers based on distributed Bragg reflectors. IEEE J. Quantum Electron. 2003, 39, 1415–1423. [Google Scholar] [CrossRef]
- Connelly, M.J. Wideband semiconductor optical amplifier steady-state numerical model. IEEE J. Quantum Electron. 2001, 37, 439–447. [Google Scholar] [CrossRef]
- Park, J.; Li, X.; Huang, W.P. Comparative study of mixed frequency-time-domain models of semiconductor laser optical amplifiers. IEE Proc.-Optoelectron. 2005, 152, 151–159. [Google Scholar] [CrossRef]





| Parameter | Symbol | Value | Unit |
|---|---|---|---|
| Bragg grating period | 242.2 | nm | |
| Active region width | 2 | μm | |
| Total quantum well thickness | 0.05 | μm | |
| Active region length | 200 | μm | |
| Optical confinement factor | 0.06 | ||
| Grating coupling coefficient | 75 | cm−1 | |
| Carrier lifetime | 0.1 | ns | |
| Group index | 3.6 | ||
| Material gain coefficient | 2000 | cm−1 | |
| Transparent carrier density | 6 × 1017 | cm−3 | |
| Peak gain wavelength | 1550 | nm | |
| Nonlinear gain suppression coefficient | 3 × 10−17 | cm3 | |
| Optical modal loss | 15 | cm−1 | |
| Reflectivity of front facet | 0.3 | ||
| Reflectivity of back facet | 0.95 | ||
| Effective index without injection | 3.2 | ||
| Spontaneous coupling factor | 1 × 10−4 | ||
| Linewidth enhancement factor | 8 | ||
| IIR filter coefficient | 0.002 |
| Parameter | Symbol | Value | Unit |
|---|---|---|---|
| Active region width | 2 | μm | |
| Total quantum well thickness | 0.05 | μm | |
| Active region length | L | 150 | μm |
| Optical confinement factor | 0.06 | ||
| Carrier lifetime | 0.5 * | ns | |
| Group index | 3.6 | ||
| Material gain coefficient | 2000 | cm−1 | |
| Transparent carrier density | 6 × 1017 | cm−3 | |
| Gain profile width | 80 | nm | |
| Peak gain wavelength | 1550 | nm | |
| Nonlinear gain suppression coefficient | 3 × 10−17 | cm3 | |
| Optical modal loss | 15 | cm−1 | |
| Reflectivity of front facet | 0.001 | ||
| Reflectivity of back facet | 0.001 | ||
| Effective index without injection | 3.2 | ||
| Spontaneous coupling factor | 0.01 | ||
| Linewidth enhancement factor | 3 | ||
| Injected current | 100 | mA |
| Parameter | Symbol | Value | Unit |
|---|---|---|---|
| Dispersion parameter | D | 4, 17 * | ps/km/nm |
| Dispersion slope | S | 0.075, 0.056 * | ps/km/nm2 |
| Fiber loss | 0.2 | dB/km | |
| Nonlinear index coefficient | 2.6 × 10−20 | m2/W | |
| Mode field diameter | d | 9.5 | μm |
| Length | L | 90, 10 * | km |
| Slope of the Raman gain profile | 4.9 × 10−18 | m/W/GHz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xin, L.; Xu, X.; Du, L.; Sun, C.; Gao, F.; Zhao, J. Suppression of Nonlinear Optical Effects in DWDM-PON by Frequency Modulation Non-Coherent Detection. Photonics 2023, 10, 323. https://doi.org/10.3390/photonics10030323
Xin L, Xu X, Du L, Sun C, Gao F, Zhao J. Suppression of Nonlinear Optical Effects in DWDM-PON by Frequency Modulation Non-Coherent Detection. Photonics. 2023; 10(3):323. https://doi.org/10.3390/photonics10030323
Chicago/Turabian StyleXin, Lei, Xiao Xu, Liuge Du, Chonglei Sun, Feng Gao, and Jia Zhao. 2023. "Suppression of Nonlinear Optical Effects in DWDM-PON by Frequency Modulation Non-Coherent Detection" Photonics 10, no. 3: 323. https://doi.org/10.3390/photonics10030323
APA StyleXin, L., Xu, X., Du, L., Sun, C., Gao, F., & Zhao, J. (2023). Suppression of Nonlinear Optical Effects in DWDM-PON by Frequency Modulation Non-Coherent Detection. Photonics, 10(3), 323. https://doi.org/10.3390/photonics10030323




