Extending the Imaging Depth of Field through Scattering Media by Wavefront Shaping of Non-Diffraction Beams
Abstract
:1. Introduction
2. Materials and Methods
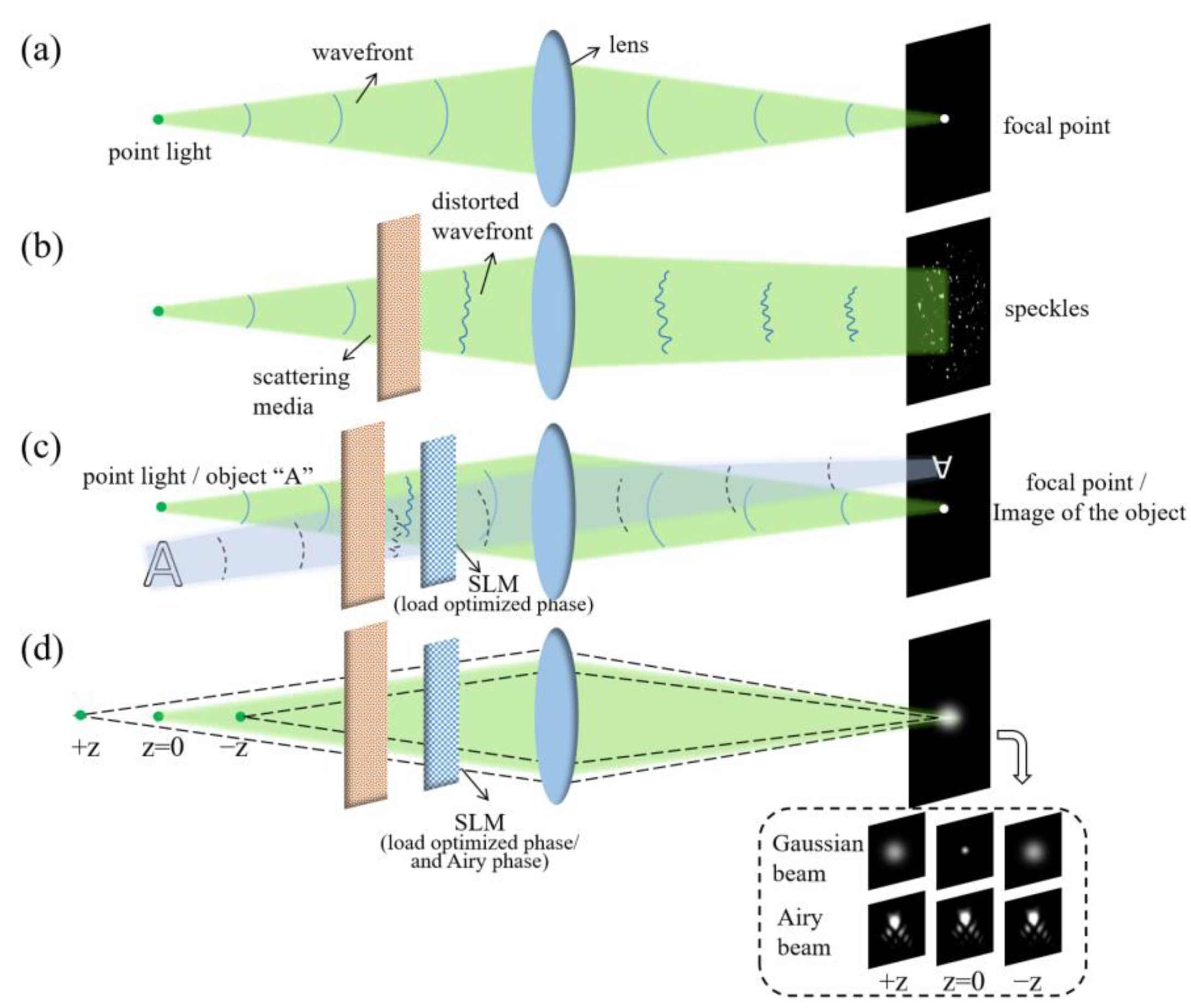
2.1. Principle
2.2. Experimental Setup
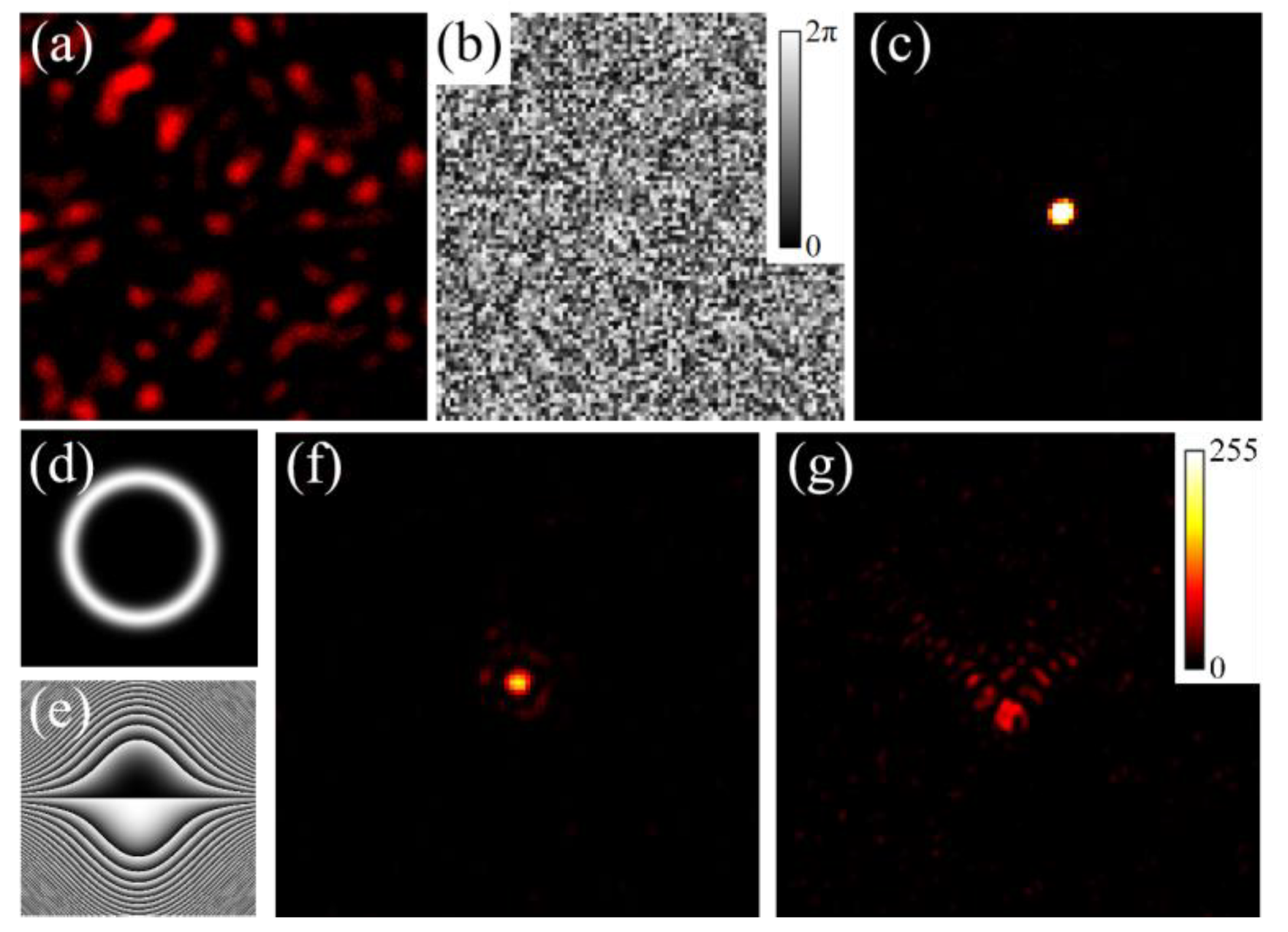
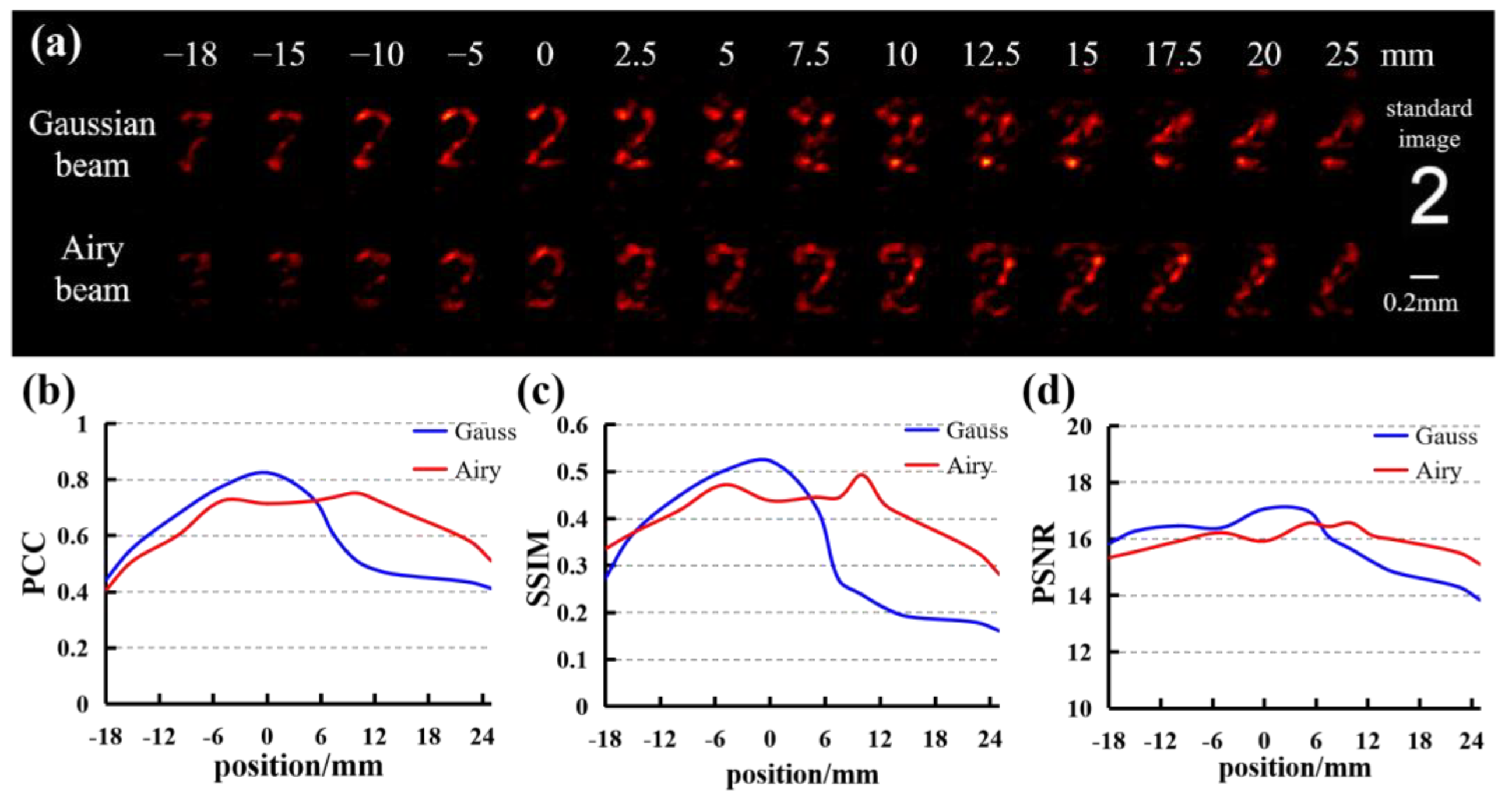
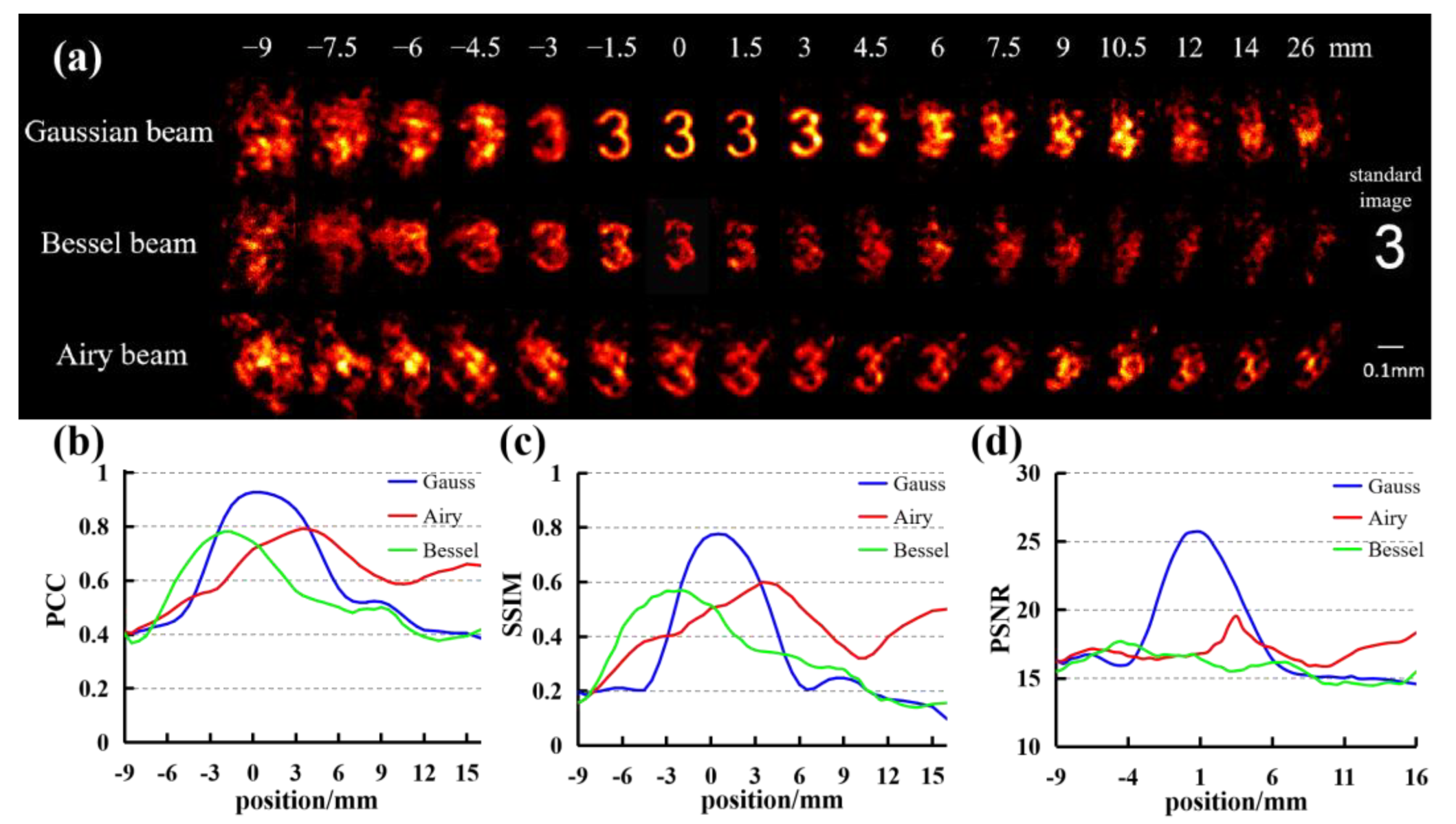
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hong, P.; Ojambati, O.S.; Lagendijk, A.; Mosk, A.P.; Vos, W.L. Three-dimensional spatially resolved optical energy density enhanced by wavefront shaping. Optica 2018, 5, 844–849. [Google Scholar] [CrossRef]
- Yaqood, Z.; Psaltis, D.; Feld, M.S.; Yang, C. Optical phase conjugation for turbidity suppression in biological samples. Nat. Photon. 2008, 2, 110–115. [Google Scholar]
- Popoff, S.M.; Lerosey, G.; Fink, M.; Boccara, A.C.; Gigan, S. Controlling Light Through Optical Disordered Media: Transmission Matrix Approach. New J. Phys. 2011, 13, 123021. [Google Scholar] [CrossRef]
- Wang, K.; Sun, W.; Richie, C.T.; Harvey, B.K.; Betzig, E.; Ji, N. Direct wavefront sensing for high-resolution in vivo imaging in scattering tissue. Nat. Commun. 2015, 6, 7276. [Google Scholar] [CrossRef]
- Hampson, K.M.; Turcotte, R.; Miller, D.T.; Kurokawa, K.; Males, J.R.; Ji, N.; Booth, M.J. Adaptive optics for high-resolution imaging. Nat. Rev. Methods Prim. 2021, 1, 68. [Google Scholar] [CrossRef]
- Chen, C.; Jones, S.M.; Silva, D.A.; Olivier, S.S. High-Resolution Adaptive Optics Scanning Laser Ophthalmoscope with Dual Deformable Mirrors. J. Opt. Soc. Am. A. 2006, 24, 1305–1312. [Google Scholar] [CrossRef]
- Katz, O.; Heidmann, P.; Fink, M.; Gigan, S. Non-invasive real-time imaging through scattering layers and around corners via speckle correlations. Nat. Photon. 2014, 8, 784–790. [Google Scholar] [CrossRef]
- Takasaki, K.T.; Fleischer, J.W. Phase-space measurement for depth-resolved memory-effect imaging. Opt. Express 2014, 22, 31426–31433. [Google Scholar] [CrossRef] [PubMed]
- Antipa, N.; Kuo, G.; Heckel, R.; Mildenhall, B.; Bostan, E.; Ng, R.; Waller, L. DiffuserCam: Lensless single-exposure 3D imaging. Optica 2018, 5, 1–9. [Google Scholar] [CrossRef]
- Xin, J.; Wang, Z.; Wang, X.; Dai, Q. Depth of field extended scattering imaging by light field estimation. Opt. Lett. 2018, 43, 4871–4874. [Google Scholar]
- Liao, M.; Lu, D.; Pedrini, G.; Osten, W.; Situ, G.; He, W.; Peng, X. Extending the depth-of-field of imaging systems with a scattering diffuser. Sci. Rep. 2019, 9, 7165–7172. [Google Scholar] [CrossRef]
- Vellekoop, I.M.; Mosk, A.P. Focusing coherent light through opaque strongly scattering media. Opt. Lett. 2007, 32, 2309–2311. [Google Scholar] [CrossRef]
- Stockbridge, C.; Lu, Y.; Moore, J.; Hoffman, S.; Paxman, R.; Toussaint, K.; Bifano, T. Focusing through dynamic scattering media. Opt. Express 2012, 20, 15086–15092. [Google Scholar] [CrossRef]
- Galaktionov, I.; Sheldakova, J.; Nikitin, A.; Samarkin, V.; Parfenov, V.; Kudryashov, A. Laser beam focusing through a moderately scattering medium using bimorph mirror. Opt. Express 2020, 28, 38061–38075. [Google Scholar] [CrossRef]
- He, C.; Shen, Y.; Forbes, A. Towards higher-dimensional structured light. Light Sci. Appl. 2022, 11, 205. [Google Scholar] [CrossRef]
- Galaktionov, I.; Nikitin, A.; Sheldakova, J.; Toporovsky, V.; Kudryashov, A. Focusing of a Laser Beam Passed through a Moderately Scattering Medium Using Phase-Only Spatial Light Modulator. Photonics 2022, 9, 296. [Google Scholar] [CrossRef]
- Horstmeyer, R.; Ruan, H.; Yang, C. Guidestar-assisted wavefront-shaping methods for focusing light into biological tissue. Nat. Photon. 2015, 9, 563–571. [Google Scholar] [CrossRef]
- Vellekoop, I.M.; Mosk, A.P. Phase control algorithms for focusing light through turbid media. Opt. Commun. 2008, 281, 3071–3080. [Google Scholar] [CrossRef]
- Katz, O.; Small, E.; Silberberg, Y. Looking around corners and through thin turbid layers in real time with scattered incoherent light. Nat. Photon. 2012, 6, 549–553. [Google Scholar] [CrossRef]
- Mikula, G.; Kolodziejczyk, A.; Makowski, M.; Prokopowicz, C.; Sypeket, M. Diffractive elements for imaging with extended depth of focus. Opt. Eng. 2005, 44, 058001. [Google Scholar] [CrossRef]
- Durnin, J.J.J.M.; Miceli, J.J., Jr.; Eberly, J.H. Diffraction-free beams. Phys. Rev. Lett. 1987, 58, 1499–1501. [Google Scholar] [CrossRef] [PubMed]
- Vasara, A.; Turunen, J.; Friberg, A.T. Realization of general nondiffracting beams with computer-generated holograms. J. Opt. Soc. Am. A. 1989, 6, 1748–1754. [Google Scholar] [CrossRef] [PubMed]
- Hu, Y.; Chen, Z.; Xiang, L.; Xing, D. Extended depth-of-field all-optical photoacoustic microscopy with a dual non-diffracting Bessel beam. Opt. Lett. 2019, 44, 1634–1637. [Google Scholar] [CrossRef] [PubMed]
- Planchon, T.A.; Gao, L.; Milkie, D.E.; Davidson, M.W.; Galbraith, J.A.; Galbraith, C.G.; Betzig, E. Rapid three-dimensional isotropic imaging of living cells using Bessel beam plane illumination. Nat. Meth. 2011, 8, 417–423. [Google Scholar] [CrossRef]
- Freund, I.I.; Rosenbluh, M.; Feng, S. Memory effects in propagation of optical waves through disordered media. Phys. Rev. Lett. 1988, 61, 2328–2331. [Google Scholar] [CrossRef]
- Li, R.; Peng, T.; Liang, Y.; Yang, Y.; Yao, B.; Yu, X.; Min, J.; Lei, M.; Yan, S.; Zhang, C.; et al. Interleaved segment correction achieves higher improvement factors in using genetic algorithm to optimize light focusing through scattering media. J. Opt. 2017, 19, 105602. [Google Scholar] [CrossRef]
- Wang, Z.; Bovik, A.C.; Sheikh, H.R.; Simoncelli, E.P. Image quality assessment: From error visibility to structural similarity. IEEE T. Image Process. 2004, 13, 600–612. [Google Scholar] [CrossRef]
- Huynh-Thu, Q.; Ghanbari, M. Scope of validity of PSNR in image/video quality assessment. Electron. Lett. 2008, 44, 800–801. [Google Scholar] [CrossRef]
- Somekh, M.G.; See, C.W.; Goh, J. Wide field amplitude and phase confocal microscope with speckle illumination. Opt. Commun. 2000, 174, 75–80. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, T.; Peng, T.; Li, R.; Wang, K.; Sun, D.; Yao, B. Extending the Imaging Depth of Field through Scattering Media by Wavefront Shaping of Non-Diffraction Beams. Photonics 2023, 10, 497. https://doi.org/10.3390/photonics10050497
Han T, Peng T, Li R, Wang K, Sun D, Yao B. Extending the Imaging Depth of Field through Scattering Media by Wavefront Shaping of Non-Diffraction Beams. Photonics. 2023; 10(5):497. https://doi.org/10.3390/photonics10050497
Chicago/Turabian StyleHan, Tongyu, Tong Peng, Runze Li, Kaige Wang, Dan Sun, and Baoli Yao. 2023. "Extending the Imaging Depth of Field through Scattering Media by Wavefront Shaping of Non-Diffraction Beams" Photonics 10, no. 5: 497. https://doi.org/10.3390/photonics10050497
APA StyleHan, T., Peng, T., Li, R., Wang, K., Sun, D., & Yao, B. (2023). Extending the Imaging Depth of Field through Scattering Media by Wavefront Shaping of Non-Diffraction Beams. Photonics, 10(5), 497. https://doi.org/10.3390/photonics10050497





