High-Efficiency and Compact Polarization-Insensitive Multi-Segment Linear Silicon Nitride Edge Coupler
Abstract
:1. Introduction
2. Materials and Methods
3. Simulation and Analysis
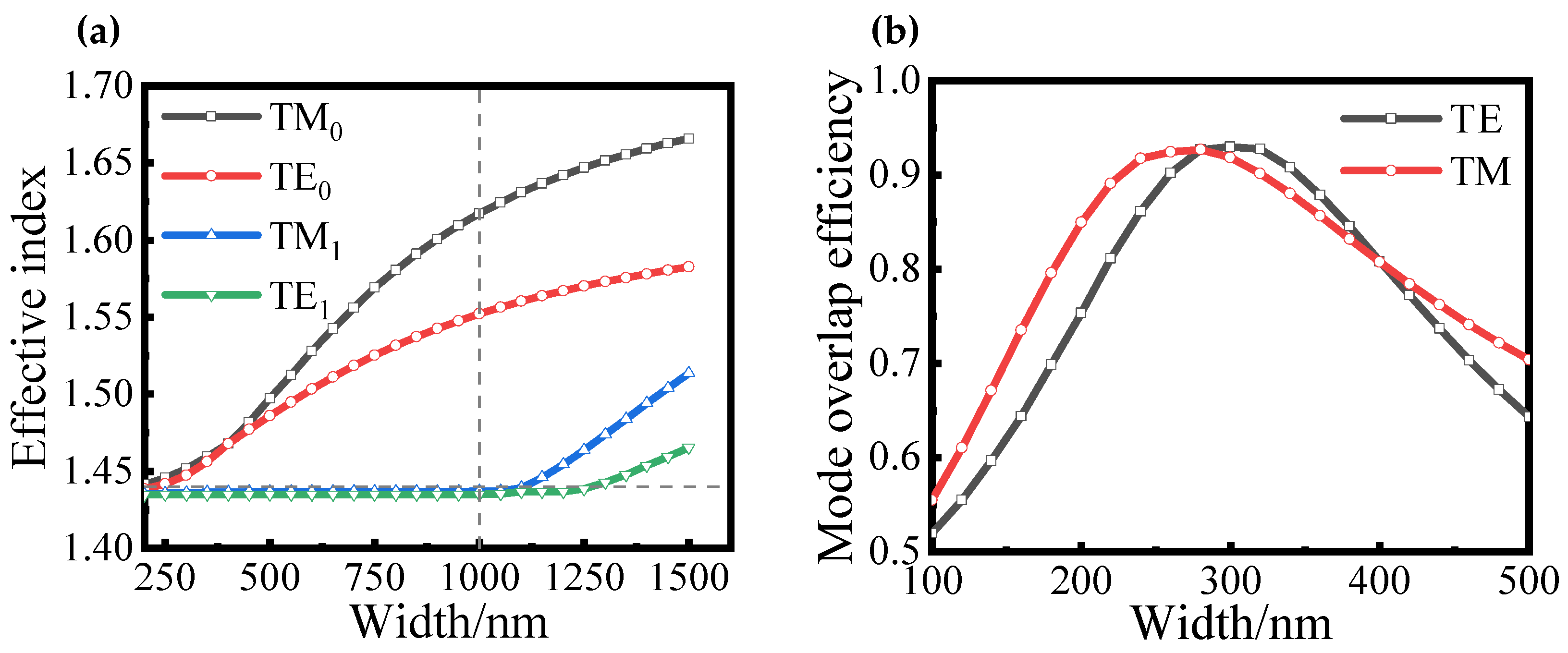
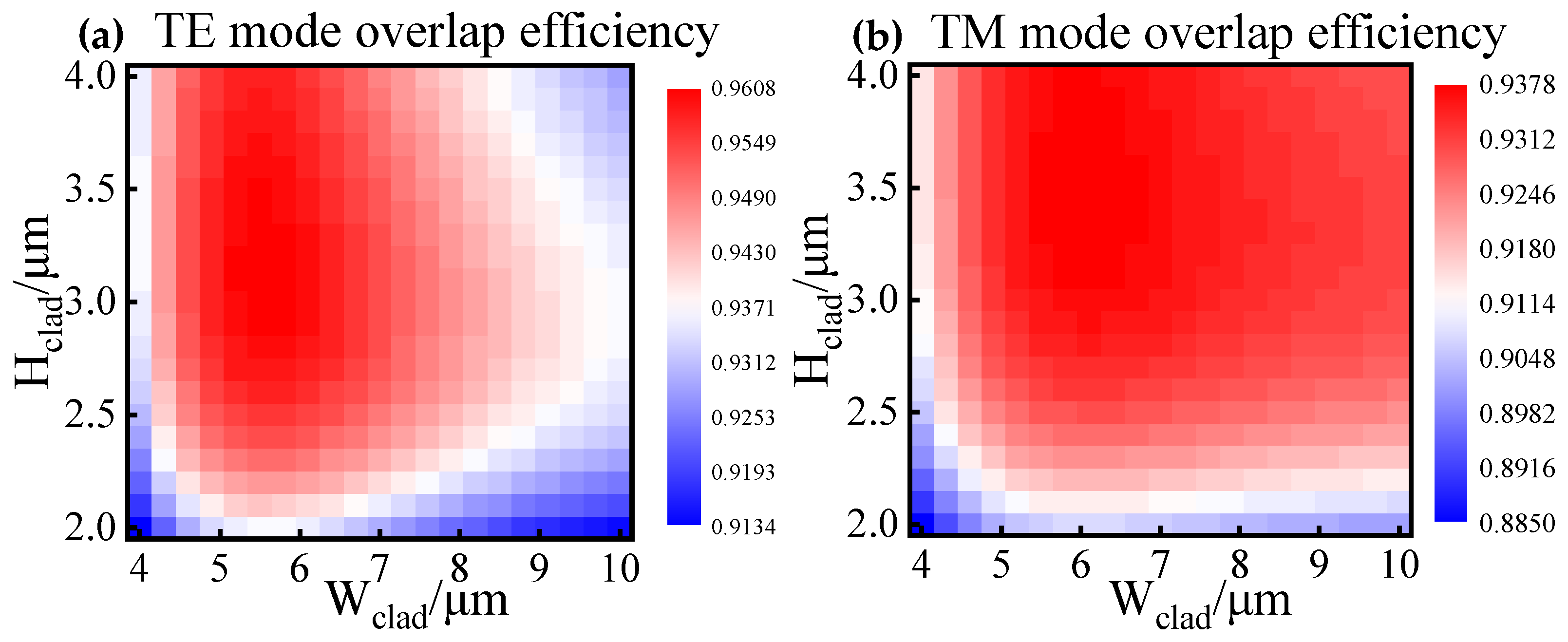
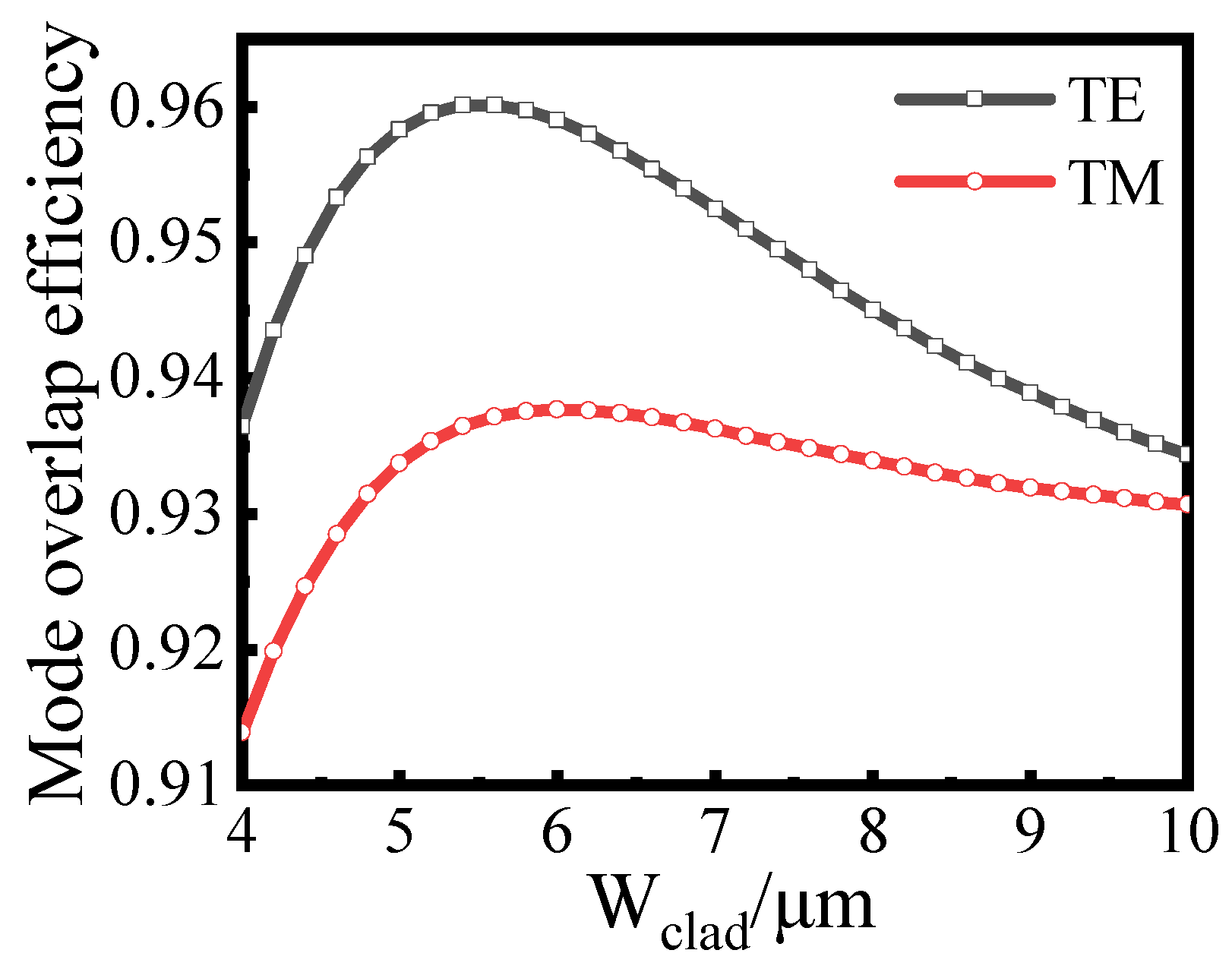
3.1. Mode Overlap Efficiency
3.2. Transmission Efficiency
3.3. Mode Field Distribution
3.4. Wavelength Dependence
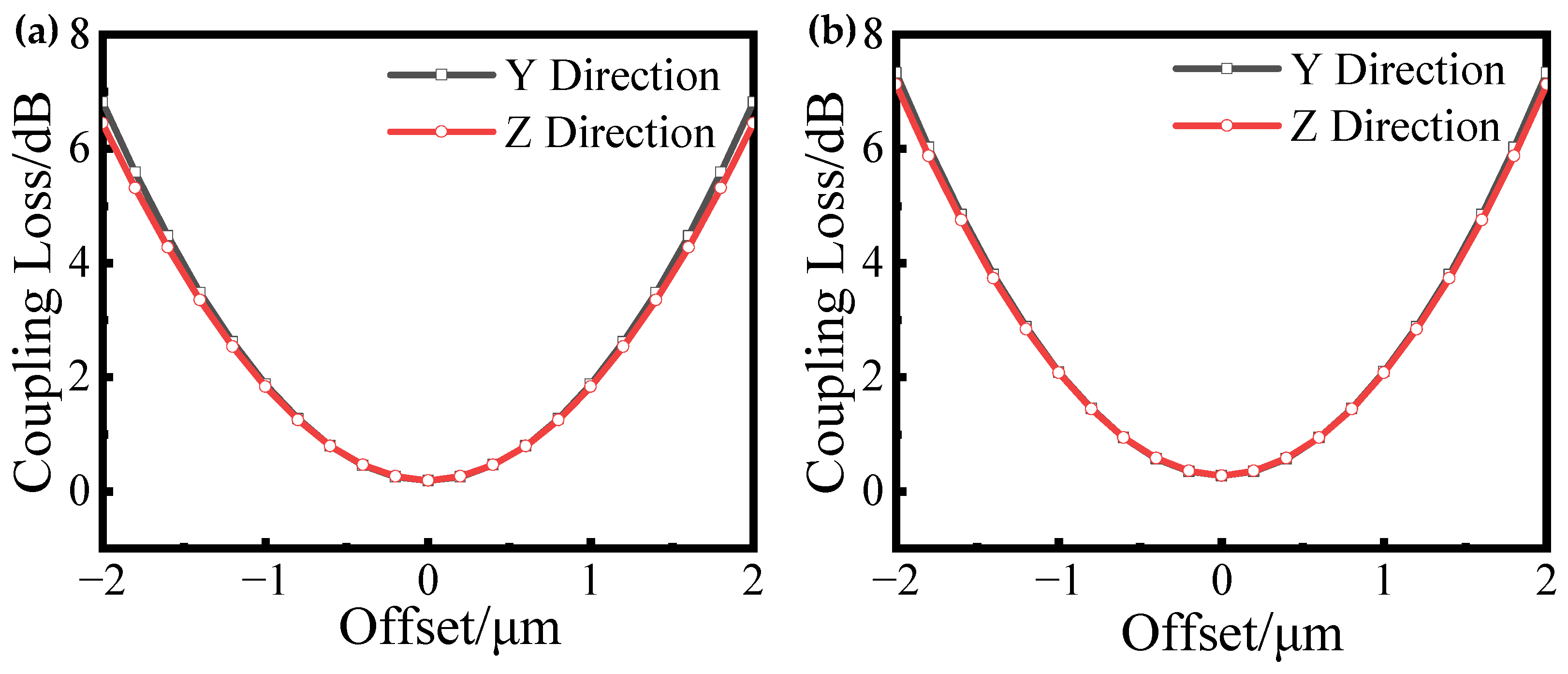
3.5. Alignment Tolerance
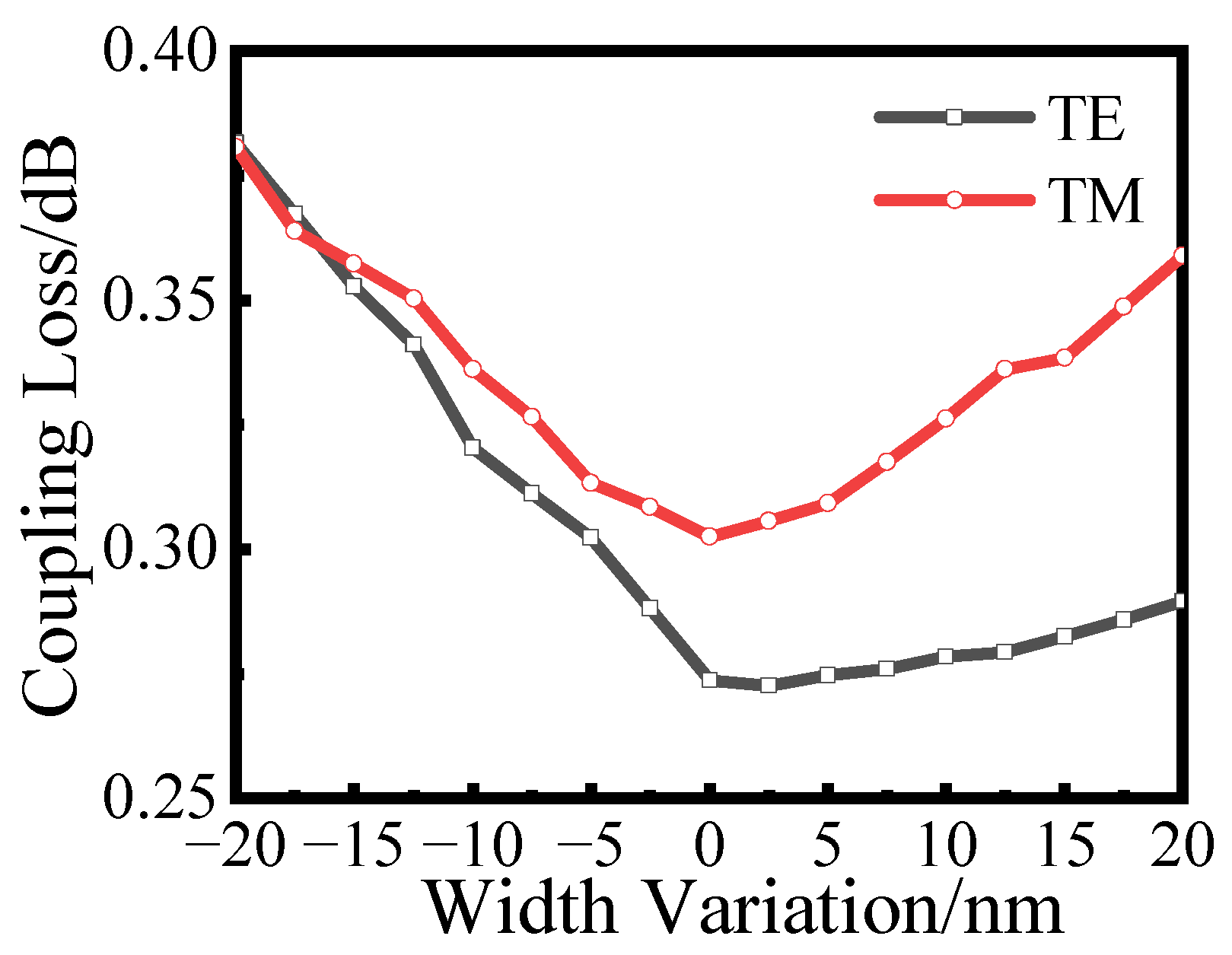
3.6. Fabrication Variation
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Siew, S.Y.; Li, B.; Gao, F.; Zheng, H.Y.; Zhang, W.; Guo, P.; Xie, S.W.; Song, A.; Dong, B.; Luo, L.W.; et al. Review of Silicon Photonics Technology and Platform Development. J. Light. Technol. 2021, 39, 4374–4389. [Google Scholar] [CrossRef]
- Margalit, N.; Xiang, C.; Bowers, S.M.; Bjorlin, A.; Blum, R.; Bowers, J.E. Perspective on the Future of Silicon Photonics and Electronics. Appl. Phys. Lett. 2021, 118, 220501. [Google Scholar] [CrossRef]
- Rickman, A. The Commercialization of Silicon Photonics. Nat. Photonics 2014, 8, 579–582. [Google Scholar] [CrossRef]
- Tang, M.; Park, J.-S.; Wang, Z.; Chen, S.; Jurczak, P.; Seeds, A.; Liu, H. Integration of III-V Lasers on Si for Si Photonics. Prog. Quantum Electron. 2019, 66, 1–18. [Google Scholar] [CrossRef]
- Dong, P.; Qian, W.; Liao, S.; Liang, H.; Kung, C.-C.; Feng, N.-N.; Shafiiha, R.; Fong, J.; Feng, D.; Krishnamoorthy, A.V.; et al. Low Loss Shallow-Ridge Silicon Waveguides. Opt. Express 2010, 18, 14474–14479. [Google Scholar] [CrossRef]
- Blumenthal, D.J.; Heideman, R.; Geuzebroek, D.; Leinse, A.; Roeloffzen, C. Silicon Nitride in Silicon Photonics. Proc. IEEE 2018, 106, 2209–2231. [Google Scholar] [CrossRef]
- Marchetti, R.; Lacava, C.; Carroll, L.; Gradkowski, K.; Minzioni, P. Coupling Strategies for Silicon Photonics Integrated Chips [Invited]. Photon. Res. 2019, 7, 201. [Google Scholar] [CrossRef]
- Moss, D.; Miao, Y.; Ta’eed, V.; Mägi, E.; Eggleton, B. Coupling to High-Index Waveguides via Tapered Microstructured Optical Fibre. Electron. Lett. 2005, 41, 1. [Google Scholar] [CrossRef]
- Mu, X.; Wu, S.; Cheng, L.; Fu, H.Y. Edge Couplers in Silicon Photonic Integrated Circuits: A Review. Appl. Sci. 2020, 10, 1538. [Google Scholar] [CrossRef]
- Hatori, N.; Shimizu, T.; Okano, M.; Ishizaka, M.; Yamamoto, T.; Urino, Y.; Mori, M.; Nakamura, T.; Arakawa, Y. A Hybrid Integrated Light Source on a Silicon Platform Using a Trident Spot-Size Converter. J. Light. Technol. 2014, 32, 1329–1336. [Google Scholar] [CrossRef]
- Hettrick, S.J.; Wang, J.; Li, C.; Wilkinson, J.S.; Shepherd, D.P. An Experimental Comparison of Linear and Parabolic Tapered Waveguide Lasers and a Demonstration of Broad-Stripe Diode Pumping. J. Light. Technol. 2004, 22, 845–849. [Google Scholar] [CrossRef]
- Ren, G.; Chen, S.; Cheng, Y.; Zhai, Y. Study on Inverse Taper Based Mode Transformer for Low Loss Coupling between Silicon Wire Waveguide and Lensed Fiber. Opt. Commun. 2011, 284, 4782–4788. [Google Scholar] [CrossRef]
- Bhandari, B.; Im, C.-S.; Lee, K.-P.; Kim, S.-M.; Oh, M.-C.; Lee, S.-S. Compact and Broadband Edge Coupler Based on Multi-Stage Silicon Nitride Tapers. IEEE Photonics J. 2020, 12, 1–11. [Google Scholar] [CrossRef]
- Teng, M.; Niu, B.; Han, K.; Kim, S.; Xuan, Y.; Lee, Y.J.; Qi, M. Trident Shape SOI Metamaterial Fiber-to-Chip Edge Coupler. In Proceedings of the Optical Fiber Communication Conference (OFC), San Diego, CA, USA, 3–7 March 2019; OSA: San Diego, CA, USA, 2019; p. Tu2J.6. [Google Scholar]
- Mu, X.; Wu, S.; Cheng, L.; Tu, X.; Fu, H.Y. Ultra-Compact Silicon Photonic Edge Coupler Based on Subwavelength Gratings. In Proceedings of the Asia Communications and Photonics Conference, Chengdu, China, 2–5 November 2019. [Google Scholar]
- Li, Y.; Wang, M.; Yang, S.; Quan, X.; Xu, J.; Wu, L.; Liu, M.; Cheng, X. Polarization Insensitive Edge Coupler Assisted by Subwavelength Grating and Suspended Structure. IEEE Photon. Technol. Lett. 2022, 34, 765–768. [Google Scholar] [CrossRef]
- Wang, X.; Quan, X.; Liu, M.; Cheng, X. Silicon-Nitride-Assisted Edge Coupler Interfacing With High Numerical Aperture Fiber. IEEE Photon. Technol. Lett. 2019, 31, 349–352. [Google Scholar] [CrossRef]
- Yao, Z.; Wan, Y.; Zhang, Y.; Ma, X.; Zheng, Z. Broadband High-Efficiency Triple-Tip Spot Size Converter for Edge Coupling with Improved Polarization Insensitivity. Opt. Commun. 2020, 475, 126301. [Google Scholar] [CrossRef]
- Sun, S.; Chen, Y.; Sun, Y.; Liu, F.; Cao, L. Novel Low-Loss Fiber-Chip Edge Coupler for Coupling Standard Single Mode Fibers to Silicon Photonic Wire Waveguides. Photonics 2021, 8, 79. [Google Scholar] [CrossRef]
- Wu, D.; Yan, W.; Yang, Y.; Song, X.; Wei, Z.; Qin, J.; Deng, L.; Bi, L. A Theoretical Sub-0.1 DB Loss Single Mode Fiber-To-Chip Edge Coupler for Silicon Nitride Waveguides. Photonics 2023, 10, 231. [Google Scholar] [CrossRef]
- Snyder, A.W.; Love, J.D. Optical Waveguide Theory; Chapman and Hall: London, UK, 1983; Volume 175. [Google Scholar]
- Liu, Y.; Chen, W.; Zhang, Y.; Cao, K.; Liu, Y.; Li, M.; Zhu, N. High-Efficiency Spot-Size Converter for Thin-Film Lithium Niobate Modulators. In Proceedings of the AOPC 2022: Optoelectronics and Nanophotonics, Beijing, China, 26–28 June 2022; Cheng, B., Guo, J., Qian, S., Eds.; SPIE: Beijing, China, 2023; p. 29. [Google Scholar]
- Yin, P.; Serafini, J.R.; Su, Z.; Shiue, R.-J.; Timurdogan, E.; Fanto, M.L.; Preble, S. Low Connector-to-Connector Loss through Silicon Photonic Chips Using Ultra-Low Loss Splicing of SMF-28 to High Numerical Aperture Fibers. Opt. Express 2019, 27, 24188. [Google Scholar] [CrossRef]
- Chuang, S.L. Physics of Photonic Devices; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Liang, Y.; Li, Z.; Fan, S.; Feng, J.; Liu, D.; Liao, H.; Yang, Z.; Feng, J.; Cui, N. Ultra-Low Loss SiN Edge Coupler Interfacing with a Single-Mode Fiber. Opt. Lett. 2022, 47, 4786. [Google Scholar] [CrossRef]
- Li, D.; Li, B.; Tang, B.; Zhang, P.; Yang, Y.; Liu, R.; Xie, L.; Li, Z. High Efficiency and Compact Vertical Interlayer Coupler for Silicon Nitride-on-Silicon Photonic Platform. Optik 2023, 274, 170572. [Google Scholar] [CrossRef]
- Tang, L.; Li, L.; Li, J.; Chen, M. Hybrid Integrated Ultralow-Linewidth and Fast-Chirped Laser for FMCW LiDAR. Opt. Express 2022, 30, 30420. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Gao, K.; Zhang, H.; Liu, H.; Lu, X.; Ren, X.; Huang, Y. Design of Silicon Nitride Edge Coupler for Monolithically Integrated Laser on Silicon Photonic Circuits With Relaxed Alignment Tolerance and High Efficiency. IEEE Photonics J. 2022, 14, 1–6. [Google Scholar] [CrossRef]
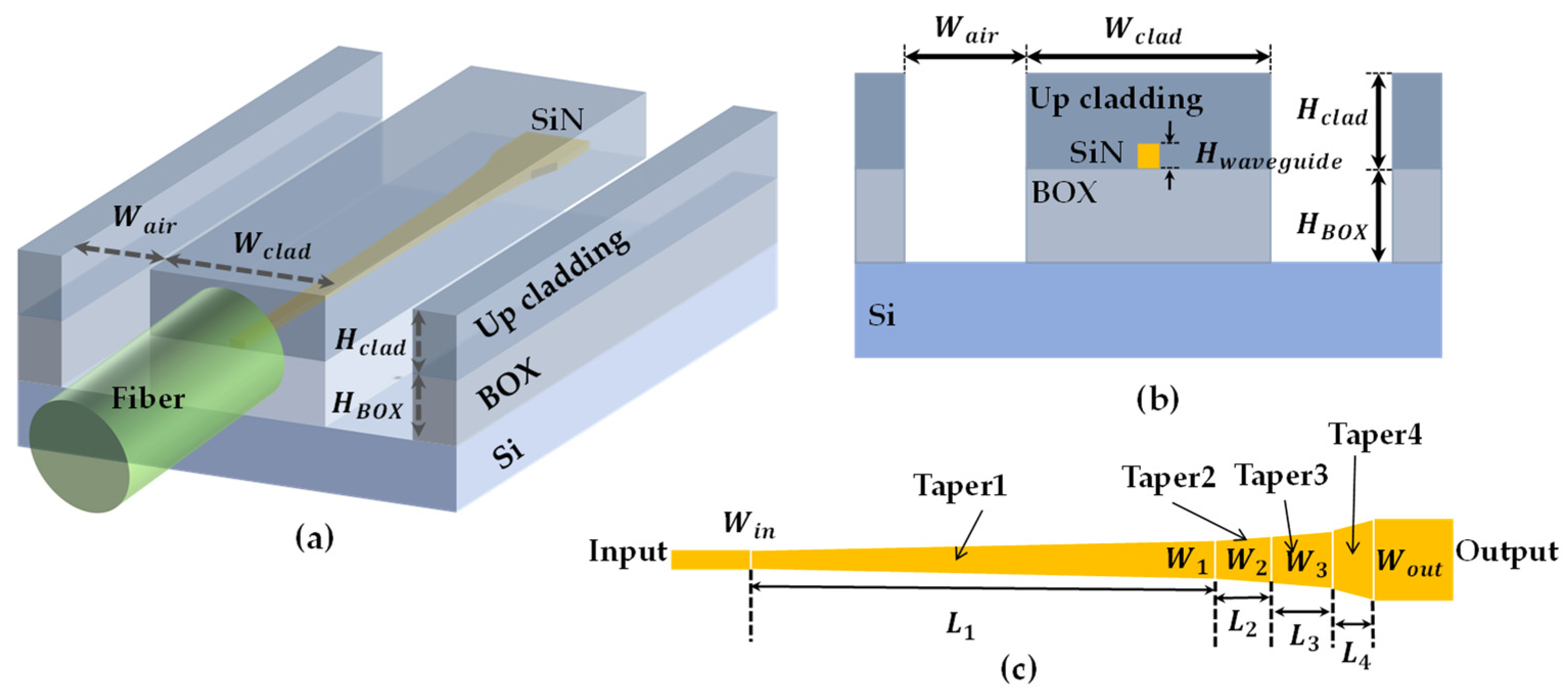




| Dimension | Size/μm | Dimension | Size/μm |
|---|---|---|---|
| HBOX | 4 | W2 | 0.5 |
| Hclad | 3.6 | W3 | 0.7 |
| Hwaveguide | 0.4 | Wout | 1 |
| Wclad | 6 | L1 | 26 |
| Wair | 2 | L2 | 6 |
| Win | 0.28 | L3 | 4 |
| W1 | 0.4 | L4 | 2 |
| Width of Input to Output for Single Linear Taper | Length | Taper Angle | Transmission Efficiency | Width of Input to Output | Taper Length | |
|---|---|---|---|---|---|---|
| 280 nm to 1000 nm | 156 μm | 0.26° | 97.5% | Taper1 | 280 nm to 400 nm | 26 μm |
| 400 nm to 1000 nm | 36 μm | 0.95° | 98.8% | Taper2 | 400 nm to 500 nm | 6 μm |
| 500 nm to 1000 nm | 10 μm | 2.86° | 99.55% | Taper3 | 500 nm to 700 nm | 4 μm |
| 700 nm to 1000 nm | 2 μm | 8.55° | 99.9% | Taper4 | 700 nm to 1000 nm | 2 μm |
| Structures | TE | TM | Length of TE Transmission Efficiency for Multi-Segment Taper |
| Linear taper | 89.3% | 98.1% | 338 μm |
| Parabolic taper (m = 2) | 91.9% | 98.9% | 142 μm |
| Triadic taper (m = 3) | 91.4% | 99.1% | 137 μm |
| Quadratic taper (m = 4) | 90.4% | 99.1% | 148 μm |
| Exponential taper | 92.4% | 99.3% | 173 μm |
| This structure | 99.34% | 99.9% | 38 μm |
| Device Stricture | Length/μm | Coupling Loss/dB | Bandwidth/nm | MFD/μm |
|---|---|---|---|---|
| Multi-Stage taper [13] | 76 | 0.36/0.55 * | 965 (<3 dB) | 4 [E] |
| Subwavelength grating [16] | 550 | 0.86/0.94 * | - | 10.4 [S] |
| Multi-layer structure [17] | 300 | 0.35/0.9 * | 95 (<1 dB) | 6.5 [E] |
| triple-tip taper [18] | 60 | 0.5/0.5 * | 580 (<1 dB) | 3 [S] |
| multiple structure [19] | 450 | 0.7(TE) | - | 8.2 [S] |
| inverted taper [25] | - | 0.85/1.09 * | 130 (<1 dB) | 10 [E] |
| bi-layer 5-tip [28] | 38.2 | 0.33(TE) | 362 (<1 dB) | N.A. [S] |
| This work | 38 | 0.22 /0.31 * | 450 (<1 dB) | 3.2 [S] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Liu, Y.; Cao, X.; Wang, L.; Mu, C.; Li, M.; Zhu, N.; Chen, W. High-Efficiency and Compact Polarization-Insensitive Multi-Segment Linear Silicon Nitride Edge Coupler. Photonics 2023, 10, 510. https://doi.org/10.3390/photonics10050510
Zhang Y, Liu Y, Cao X, Wang L, Mu C, Li M, Zhu N, Chen W. High-Efficiency and Compact Polarization-Insensitive Multi-Segment Linear Silicon Nitride Edge Coupler. Photonics. 2023; 10(5):510. https://doi.org/10.3390/photonics10050510
Chicago/Turabian StyleZhang, Yuhao, Yi Liu, Xuhua Cao, Li Wang, Chunyuan Mu, Ming Li, Ninghua Zhu, and Wei Chen. 2023. "High-Efficiency and Compact Polarization-Insensitive Multi-Segment Linear Silicon Nitride Edge Coupler" Photonics 10, no. 5: 510. https://doi.org/10.3390/photonics10050510
APA StyleZhang, Y., Liu, Y., Cao, X., Wang, L., Mu, C., Li, M., Zhu, N., & Chen, W. (2023). High-Efficiency and Compact Polarization-Insensitive Multi-Segment Linear Silicon Nitride Edge Coupler. Photonics, 10(5), 510. https://doi.org/10.3390/photonics10050510





