Generalized Asymmetric Hermite–Gaussian and Laguerre–Gaussian Beams
Abstract
1. Introduction
2. Generalized Hermite–Gaussian and Laguerre–Gaussian Beams
3. Asymmetric Generalized Hermite–Gaussian and Laguerre–Gaussian Beams
4. Non-Orthogonality and Power of gLG Beams
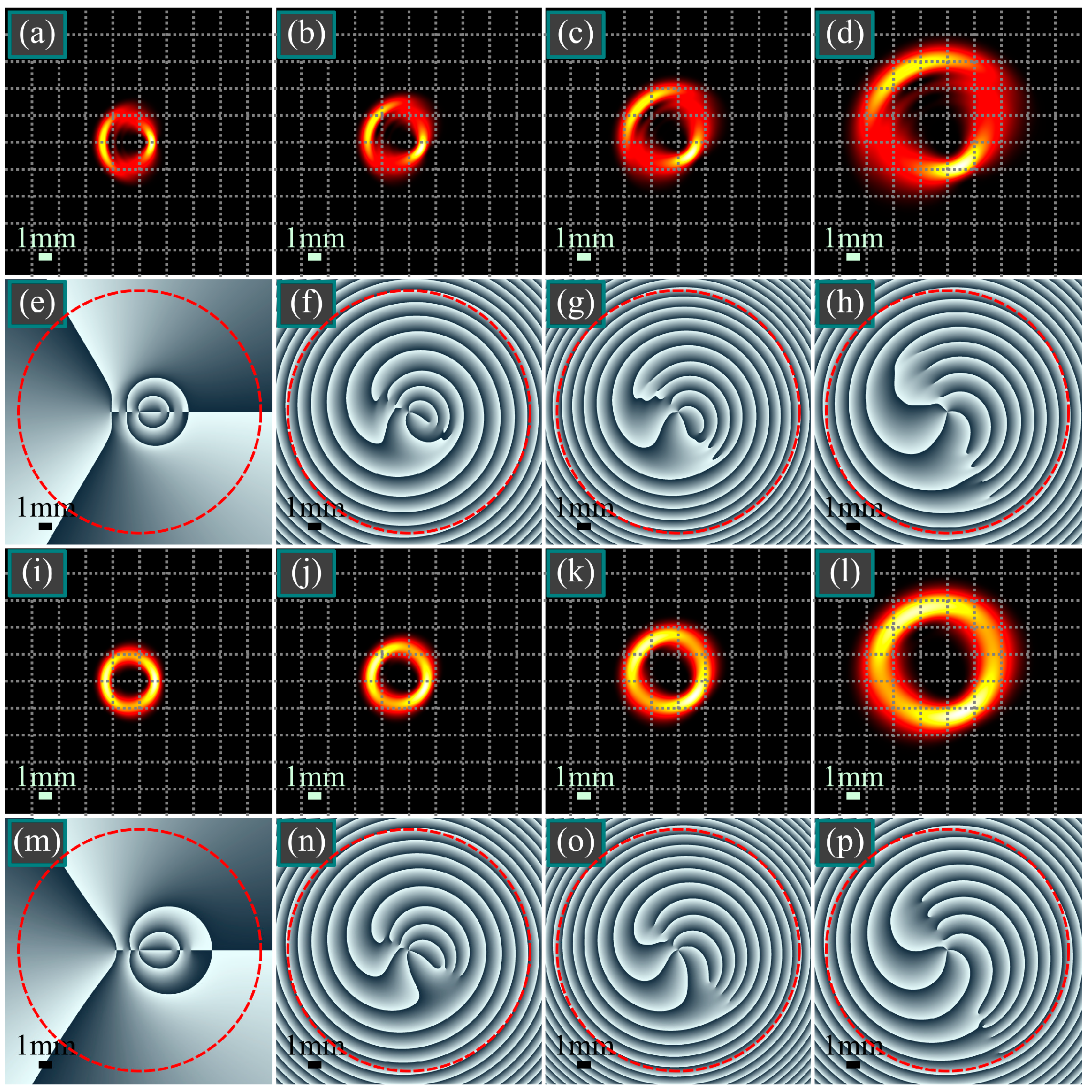
5. Topological Charge of gLG Beams and Their Anomalous Rotation upon Propagation
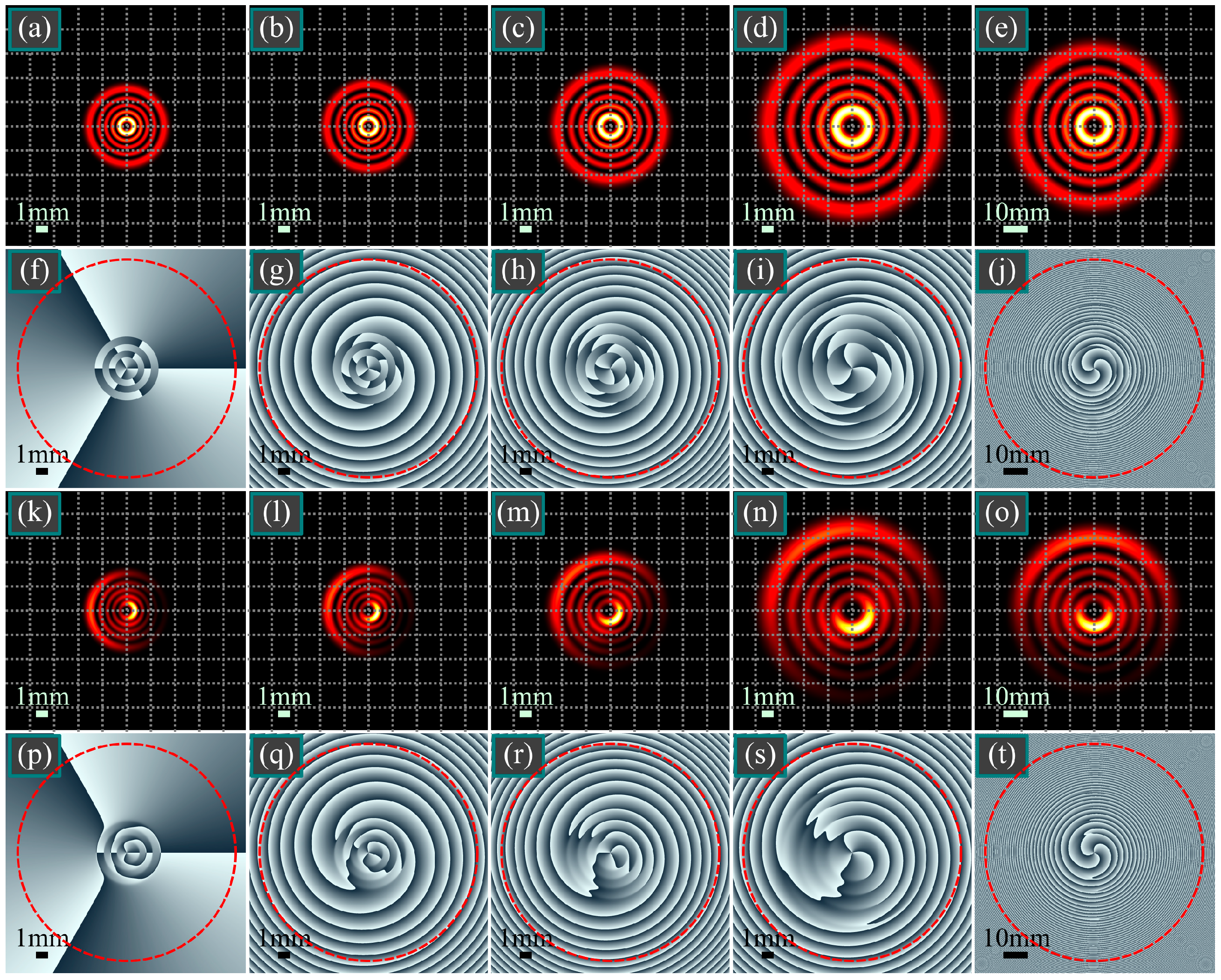
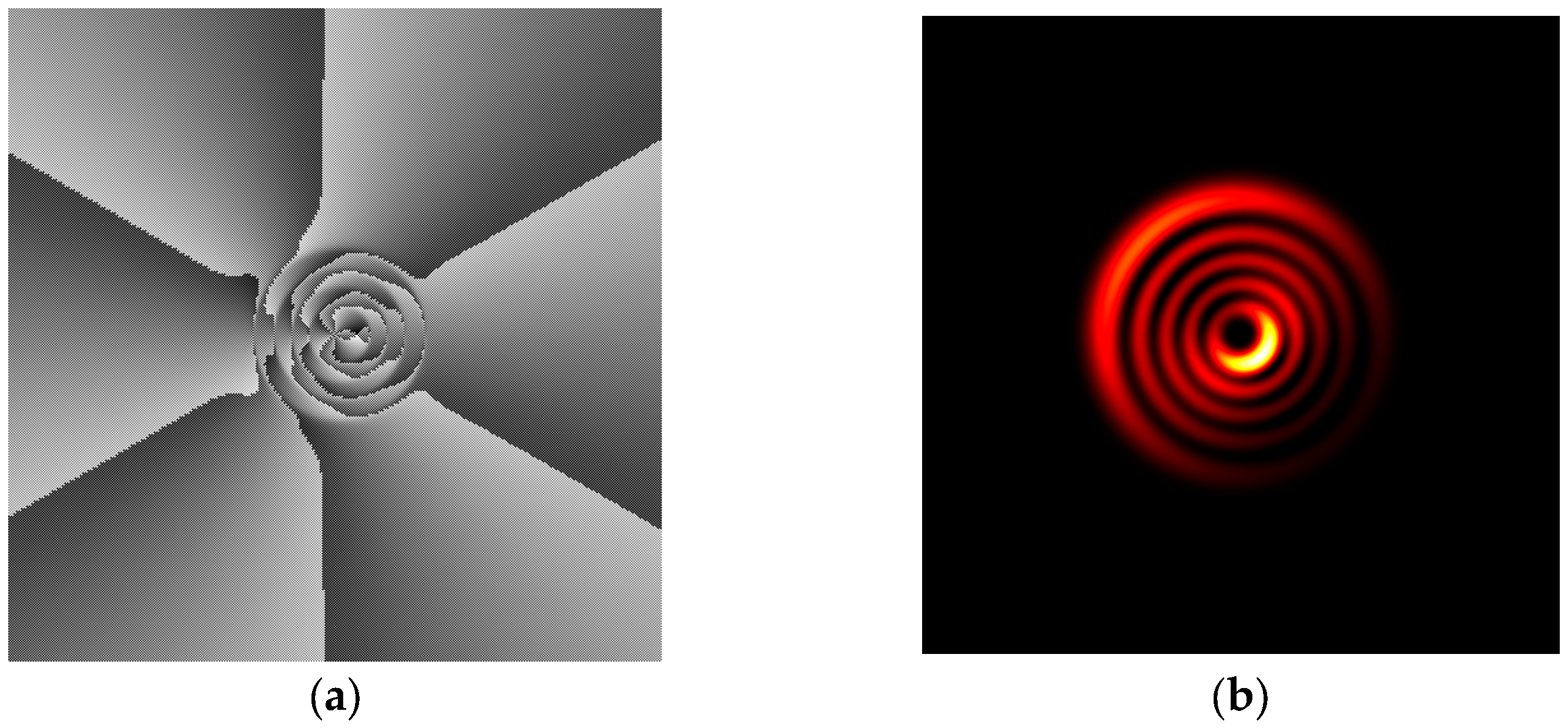
6. Numerical Modeling of gLG Beams
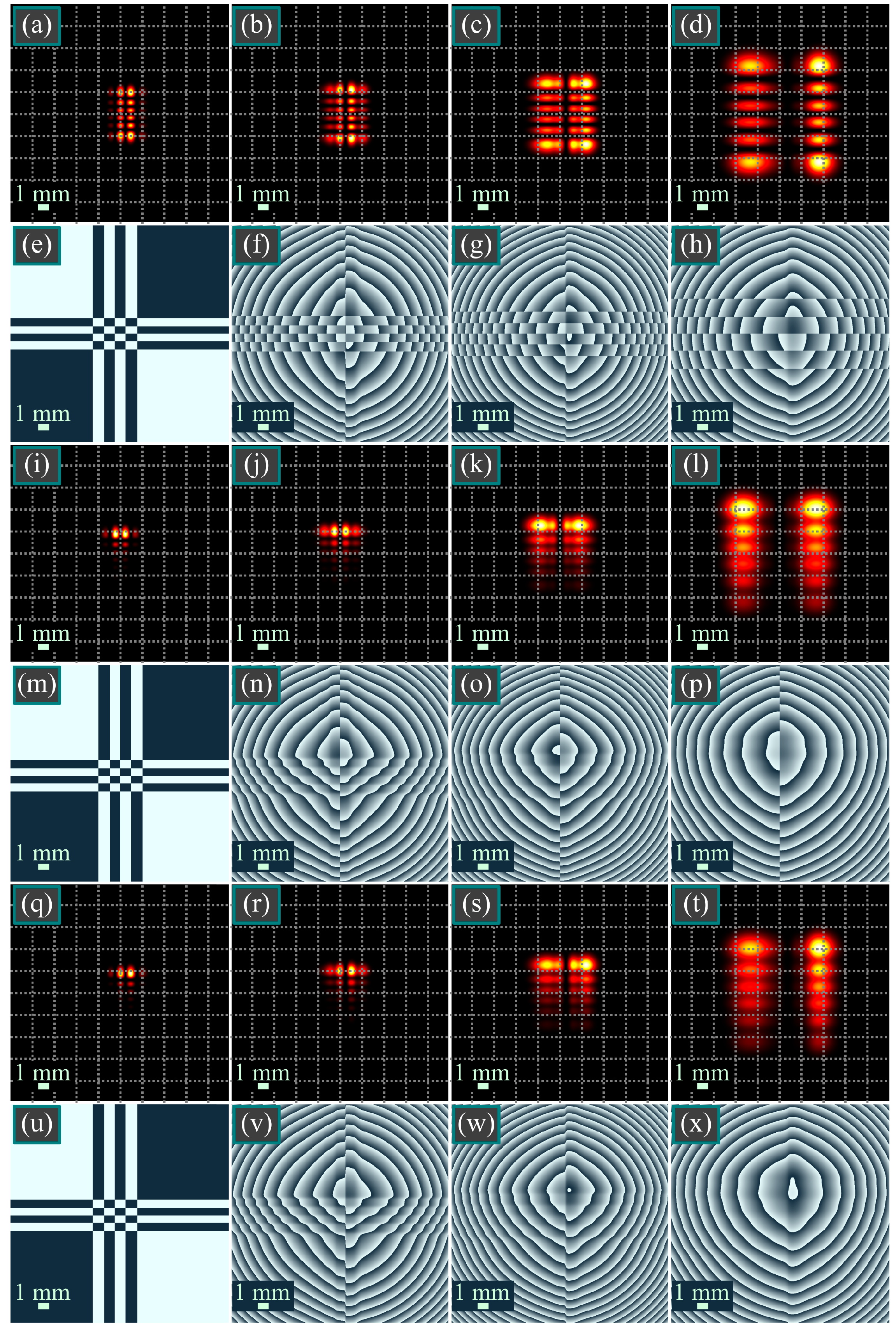
7. Numerical Modeling of gHG Beams
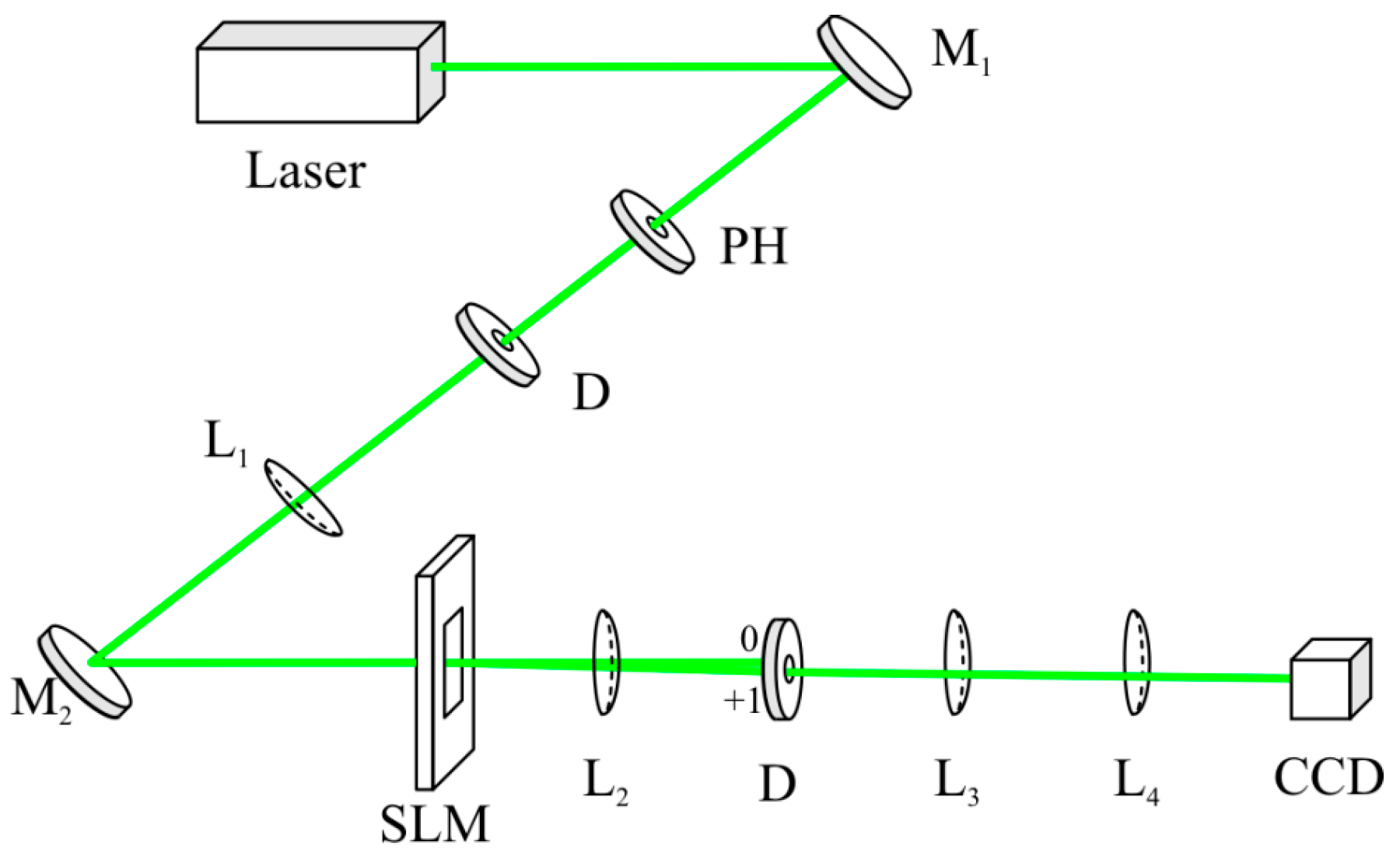
8. Experiment
9. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kogelnik, H.; Li, T. Laser beams and resonators. Appl. Opt. 1966, 5, 1550–1567. [Google Scholar] [CrossRef] [PubMed]
- Siegman, A.E. Hermite-Gaussian functions of complex argument as optical beam eigenfunction. J. Opt. Soc. Am. 1973, 63, 1093–1094. [Google Scholar] [CrossRef]
- Pratesi, R.; Ronchi, L. Generalized Gaussian beams in free space. J. Opt. Soc. Am. 1977, 67, 1274–1276. [Google Scholar] [CrossRef]
- Zauderer, E. Complex argument Hermite-Gaussian and Laguerre-Gaussian beams. J. Opt. Soc. Am. A 1986, 3, 465–469. [Google Scholar] [CrossRef]
- Wünsche, A. Generalized Gaussian beam solutions of paraxial optics and their connection to a hidden symmetry. J. Opt. Soc. Am. A 1989, 6, 1320–1329. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Kovalev, A.A. Hermite-Gaussian modal laser beams with orbital angular momentum. J. Opt. Soc. Am. A 2014, 31, 274–282. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, Y.; Zhang, Y.; Chen, H.; Yu, S. Generalised Hermite–Gaussian beams and mode transformations. J. Opt. 2016, 18, 055001. [Google Scholar] [CrossRef]
- Abramochkin, E.G.; Volostnikov, V.G. Generalized Gaussian beams. J. Opt. A Pure Appl. Opt. 2004, 6, S157–S161. [Google Scholar] [CrossRef]
- Abramochkin, E.G.; Volostnikov, V.G. Beam transformations and nontransformed beams. Opt. Commun. 1991, 83, 123–135. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Kovalev, A.A.; Porfirev, A.P. Vortex Hermite-Gaussian laser beams. Opt. Lett. 2015, 40, 701–704. [Google Scholar] [CrossRef]
- Mei, Z.; Zhao, D.; Gu, J.; Mao, H. Approximate analytical expressions of Laguerre-Gaussian beams passing through a paraxial optical system with an annular aperture. Optik 2004, 115, 311–316. [Google Scholar] [CrossRef]
- Arlt, J.; Kuhn, R.; Dholakia, K. Spatial transformation of Laguerre-Gaussian laser modes. J. Mod. Opt. 2001, 48, 783–787. [Google Scholar] [CrossRef]
- Jarutis, V.; Paškauskas, R.; Stabinis, A. Focusing of Laguerre-Gaussian beams by axicon. Opt. Commun. 2000, 184, 105–112. [Google Scholar] [CrossRef]
- Simon, R.; Agarwal, G.S. Wigner representation of Laguerre-Gaussian beams. Opt. Lett. 2000, 25, 1313–1315. [Google Scholar] [CrossRef] [PubMed]
- Kim, H.C.; Lee, Y.H. Hermite-Gaussian and Laguerre-Gaussian beams beyond the paraxial approximation. Opt. Commun. 1999, 169, 9–16. [Google Scholar] [CrossRef]
- Hasegawa, T.; Shimizu, T. Frequency-doubled Hermite-Gaussian beam and the mode conversion to the Laguerre-Gaussian beam. Opt. Commun. 1999, 160, 103–108. [Google Scholar] [CrossRef]
- Kovalev, A.A.; Kotlyar, V.V.; Porfirev, A.P. Asymmetric Laguerre-Gaussian beams. Phys. Rev. A 2016, 93, 063858. [Google Scholar] [CrossRef]
- Hsieh, Y.H.; Lai, Y.H.; Hsieh, M.X.; Huang, K.F.; Chen, Y.F. Generating high-power asymmetrical Laguerre-Gaussian modes and exploring topological charges distribution. Opt. Express 2018, 26, 31738–31749. [Google Scholar] [CrossRef]
- Ghaderi Goran Abad, M.; Mahmoudi, M. Laguerre-Gaussian modes generated vector beam via nonlinear magneto-optical rotation. Sci. Rep. 2021, 11, 5972. [Google Scholar] [CrossRef]
- Yang, Y.; Li, Y.; Wang, C. Generation and expansion of Laguerre–Gaussian beams. J. Opt. 2022, 51, 910–926. [Google Scholar] [CrossRef]
- Matsumoto, N.; Ando, T.; Inoue, T.; Ohtake, Y.; Fukuchi, N.; Hara, T. Generation of high-quality higher-order Laguerre-Gaussian beams using liquid-crystal-on-silicon spatial light modulators. J. Opt. Soc. Am. A 2008, 25, 1642–1651. [Google Scholar] [CrossRef] [PubMed]
- Volyar, A.; Abramochkin, E.; Akimova, Y.; Bretsko, M. Control of the orbital angular momentum via radial numbers of structured Laguerre–Gaussian beams. Opt. Lett. 2022, 47, 2402–2405. [Google Scholar] [CrossRef]
- Volyar, A.; Abramochkin, E.; Akimova, Y.; Bretsko, M. Astigmatic-Invariant Structured Singular Beams. Photonics 2022, 9, 842. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Khonina, S.N.; Almazov, A.A.; Soifer, V.A.; Jefimovs, K.; Turunen, J. Elliptic Laguerre-Gaussian beams. J. Opt. Soc. Am. A 2006, 23, 43–56. [Google Scholar] [CrossRef] [PubMed]
- Dwivedi, R.; Sharma, P.; Jaiswal, V.K.; Mehrotra, R. Elliptically squeezed axicon phase for detecting topological charge of vortex beam. Opt. Commun. 2021, 485, 126710. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Kovalev, A.A.; Soifer, V.A. Asymmetric Bessel modes. Opt. Lett. 2014, 39, 2395–2398. [Google Scholar] [CrossRef]
- Gong, L.; Qiu, X.Z.; Ren, Y.X.; Zhu, H.Q.; Liu, W.W.; Zhou, J.H.; Zhong, M.C.; Chu, X.X.; Li, Y.M. Observation of the asymmetric Bessel beams with arbitrary orientation using a digital micromirror device. Opt. Express 2014, 22, 26763–26776. [Google Scholar] [CrossRef]
- Rykov, M.A.; Skidanov, R.V. Modifying the laser beam intensity distribution for obtaining improved strength characteristics of an optical trap. Appl. Opt. 2014, 53, 156–164. [Google Scholar] [CrossRef]
- Beijersbergen, M.W.; Allen, L.; van der Veen, H.E.L.O.; Woerdman, J.P. Astigmatic laser mode converters and transfer of orbital angular momentum. Opt. Commun. 1993, 96, 123–132. [Google Scholar] [CrossRef]
- Heckenberg, N.R.; Mcduf, R.; Smith, C.P.; White, A.G. Generation of optical phase singularities by computer-generated holograms. Opt. Lett. 1992, 17, 3. [Google Scholar] [CrossRef]
- Dennis, M.R.; O’Holleran, K.; Padgett, M.J. Chapter 5 Singular Optics: Optical Vortices and Polarization Singularities. Prog. Opt. 2009, 53, 293–363. [Google Scholar] [CrossRef]
- Kim, G.H.; Jeon, J.H.; Ko, K.H.; Lee, J.H.; Chang, J.S. Optical vortices produced with a nonspiral phase plate. Appl. Opt. 1997, 36, 8614–8621. [Google Scholar] [CrossRef] [PubMed]
- Marrucci, L.; Manzo, C.; Paparo, D. Optical spin-to-orbital angular momentum conversion in inhomogeneous anisotropic media. Phys. Rev. Lett. 2006, 96, 163905. [Google Scholar] [CrossRef] [PubMed]
- Senatsky, Y.; Bisson, J.F.; Shelobolin, A.; Shirekawa, A.; Ueda, K. Circular modes selection in Yb:YAG laser using an intracavity lens with spherical aberration. Laser Phys. 2009, 19, 911–918. [Google Scholar] [CrossRef]
- Thirugnanasambandam, M.P.; Senatsky, Y.; Ueda, K. Generation of very-high order Laguerre-Gaussian modes in Yb:YAG ceramic laser. Laser Phys. Lett. 2010, 7, 637–643. [Google Scholar] [CrossRef]
- Tan, S.; Zhou, C.; Shirakakwa, A.; Ueda, K.; Li, J. Vortex Ti:Sapphire laser by using an intracavity spot-defect spatial filter. Opt. Laser Technol. 2017, 96, 76–80. [Google Scholar] [CrossRef]
- Ngcobo, S.; Litvin, I.; Burger, L.; Forbes, A. A digital laser for on-demand laser modes. Nat. Commun. 2013, 4, 2289. [Google Scholar] [CrossRef]
- Liu, S.S.; Chen, X.D.; Pu, J.X.; Lin, Z.L.; Chen, Z.Y. A V-folded digital laser for on-demand vortex beams by astigmatic transformation of Hermite-Gaussian modes. Chinese Phys. Lett. 2019, 36, 124203. [Google Scholar] [CrossRef]
- Ito, A.; Kozawa, Y.; Sato, S. Generation of hollow scalar and vector beams using a spot-defect mirror. J. Opt. Soc. Am. A. 2010, 27, 2072–2077. [Google Scholar] [CrossRef]
- Kano, K.; Kozawa, Y.; Sato, S. Generation of a Purely Single Transverse Mode Vortex Beam from a He-Ne Laser Cavity with a Spot-Defect Mirror. Int. J. Opt. 2012, 2012, 359141. [Google Scholar] [CrossRef]
- Vyas, S.; Kozawa, Y.; Sato, S. Generation of a vector doughnut beam from an internal mirror He–Ne laser. Opt. Lett. 2014, 39, 2080–2082. [Google Scholar] [CrossRef] [PubMed]
- Qiao, Z.; Xie, G.; Wu, Y.; Yuan, P.; Ma, J.; Qian, L.; Fan, D. Generating high-charge optical vortices directly from laser up to 288th order. Laser Photon. Rev. 2018, 12, 1800019. [Google Scholar] [CrossRef]
- Uesugi, Y.; Sato, S.; Kozawa, Y. Direct generation of the lowestorder vortex beam using a spot defect mirror in the ultraviolet region. Opt. Lett. 2020, 45, 2115–2118. [Google Scholar] [CrossRef] [PubMed]
- Yu, L.; Zhang, Y.; Wang, J. Propagation of asymmetric Bessel mode in turbulent atmosphere. IEEE Photonics J. 2022, 14, 7354106. [Google Scholar] [CrossRef]
- Watkins, R.J.; Dai, K.; White, G.; Li, W.; Miller, J.K.; Morgan, K.S.; Johnson, E.G. Experimental probing of turbulence using a continuous spectrum of asymmetric OAM beams. Opt. Express 2020, 28, 924–935. [Google Scholar] [CrossRef] [PubMed]
- Kovalev, A.A.; Kotlyar, V.V.; Porfirev, A.P. Optical trapping and moving of microparticles by using asymmetrical Laguerre–Gaussian beams. Opt. Lett. 2016, 41, 2426–2429. [Google Scholar] [CrossRef]
- Prudnikov, A.P.; Brychkov, Y.A.; Marichev, O.I. Integrals and Series. Special Functions; Gordon and Breach: New York, NY, USA, 1986. [Google Scholar]
- Brychkov, Y.A. Handbook of Special Functions: Derivatives, Integrals, Series and Other Formulas; CRC Press: Boca Raton, FL, USA, 2008. [Google Scholar]
- Berry, M.V. Optical vortices evolving from helicoidal integer and fractional phase steps. J. Opt. A Pure Appl. Opt. 2004, 6, 259. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions; Dover: New York, NY, USA, 1972. [Google Scholar]
- Abramochkin, E.G.; Volostnikov, V.G. Spiral light beams. Phys. Usp. 2004, 47, 1177–1203. [Google Scholar] [CrossRef]
- Mendoza-Yero, O.; Mínguez-Vega, G.; Lancis, J. Encoding complex fields by using a phase-only optical element. Opt. Lett. 2014, 39, 1740–1743. [Google Scholar] [CrossRef]
- Goorden, S.; Bertolotti, J.; Mosk, A. Superpixel-based spatial amplitude and phase modulation using a digital micromirror device. Opt. Express 2014, 22, 17999–18009. [Google Scholar] [CrossRef]
- Singh, S.K.; Adachi, Y.; Kinashi, K.; Tsutsumi, N.; Sakai, W.; Jackin, B.J. Tailoring Large Asymmetric Laguerre–Gaussian Beam Array Using Computer-Generated Holography. Photonics 2023, 10, 247. [Google Scholar] [CrossRef]
- Durnin, J. Exact solutions for nondiffracting beams. I. The scalar theory. J. Opt. Soc. Am. A 1987, 4, 651–654. [Google Scholar] [CrossRef]
- Siviloglou, G.A.; Broky, J.; Dogariu, A.; Christodoulides, D.N. Observation of Accelerating Airy Beams. Phys. Rev. Lett. 2007, 99, 213901. [Google Scholar] [CrossRef] [PubMed]
- Cox, M.A.; Maqondo, L.; Kara, R.; Milione, G.; Cheng, L.; Forbes, A. The Resilience of Hermite– and Laguerre–Gaussian Modes in Turbulence. J. Lightwave Technol. 2019, 37, 3911–3917. [Google Scholar] [CrossRef]
- Bochove, E.J.; Moore, G.T.; Scully, M.O. Acceleration of particles by an asymmetric Hermite-Gaussian laser beam. Phys. Rev. A 1992, 46, 6640–6653. [Google Scholar] [CrossRef]
- Guo, M.; Le, W.; Wang, C.; Rui, G.; Zhu, Z.; He, J.; Gu, B. Generation, Topological Charge, and Orbital Angular Momentum of Off-Axis Double Vortex Beams. Photonics 2023, 10, 368. [Google Scholar] [CrossRef]
- Pavani, S.R.P.; Piestun, R. High-efficiency rotating point spread functions. Opt. Express 2008, 16, 3484. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abramochkin, E.G.; Kotlyar, V.V.; Kovalev, A.A.; Stafeev, S.S. Generalized Asymmetric Hermite–Gaussian and Laguerre–Gaussian Beams. Photonics 2023, 10, 606. https://doi.org/10.3390/photonics10060606
Abramochkin EG, Kotlyar VV, Kovalev AA, Stafeev SS. Generalized Asymmetric Hermite–Gaussian and Laguerre–Gaussian Beams. Photonics. 2023; 10(6):606. https://doi.org/10.3390/photonics10060606
Chicago/Turabian StyleAbramochkin, Eugeny G., Victor V. Kotlyar, Alexey A. Kovalev, and Sergey S. Stafeev. 2023. "Generalized Asymmetric Hermite–Gaussian and Laguerre–Gaussian Beams" Photonics 10, no. 6: 606. https://doi.org/10.3390/photonics10060606
APA StyleAbramochkin, E. G., Kotlyar, V. V., Kovalev, A. A., & Stafeev, S. S. (2023). Generalized Asymmetric Hermite–Gaussian and Laguerre–Gaussian Beams. Photonics, 10(6), 606. https://doi.org/10.3390/photonics10060606




