A Generic and Effective System Dispersion Compensation Method: Development and Validation in Visible-Light OCT
Abstract
1. Introduction
2. Experiment and Theory
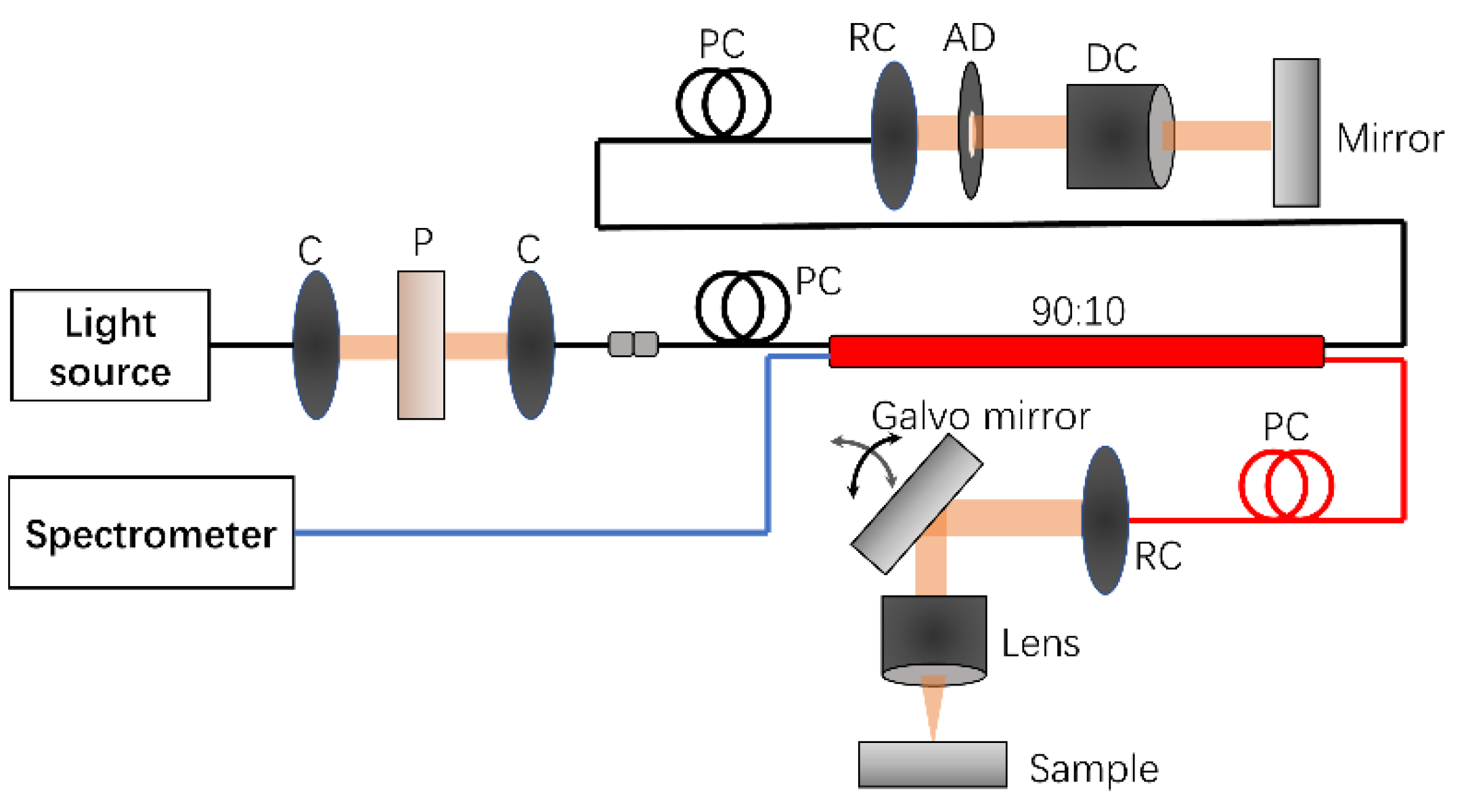
2.1. Vis-OCT System and Experiments
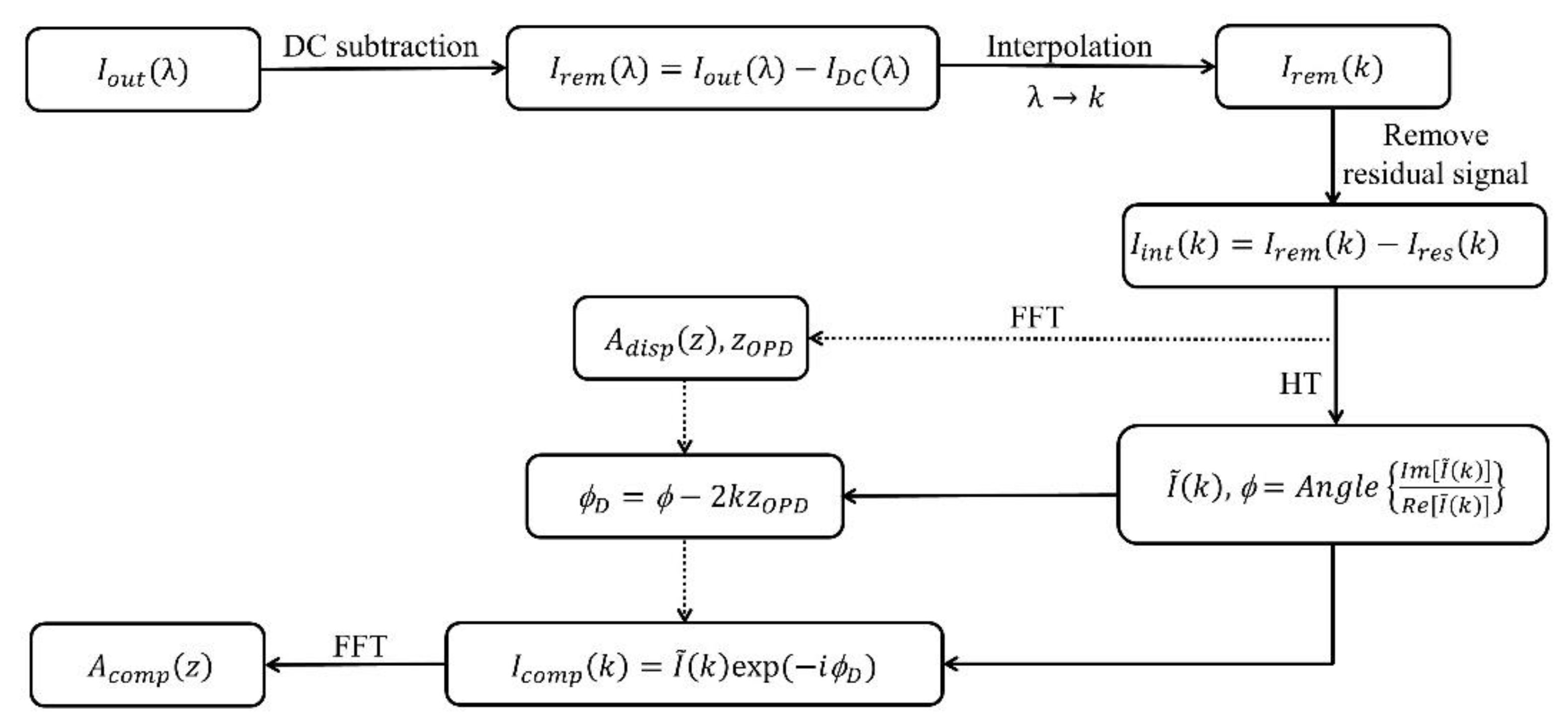
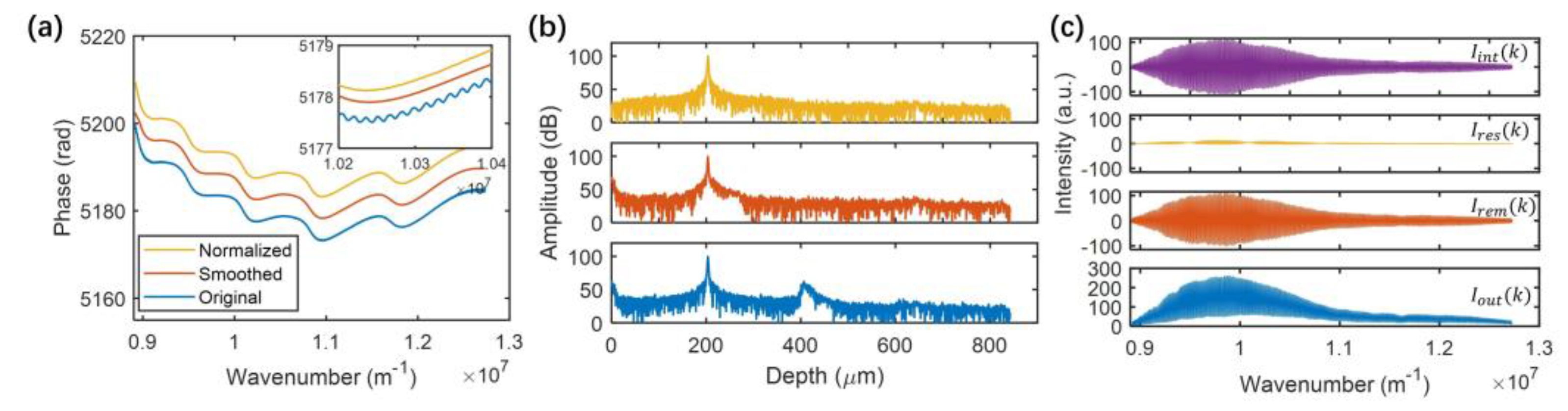
2.2. Dispersion Compensation Method
2.2.1. Taylor Series Iterative Fitting (TSIF) Method
2.2.2. Two Symmetrical Measurements of the Mirror-Reflection (TSMMR) Method
2.2.3. Single Arbitrary Measurement of Mirror-Reflection (SAMMR) Method
3. Result
3.1. Performance Evaluation
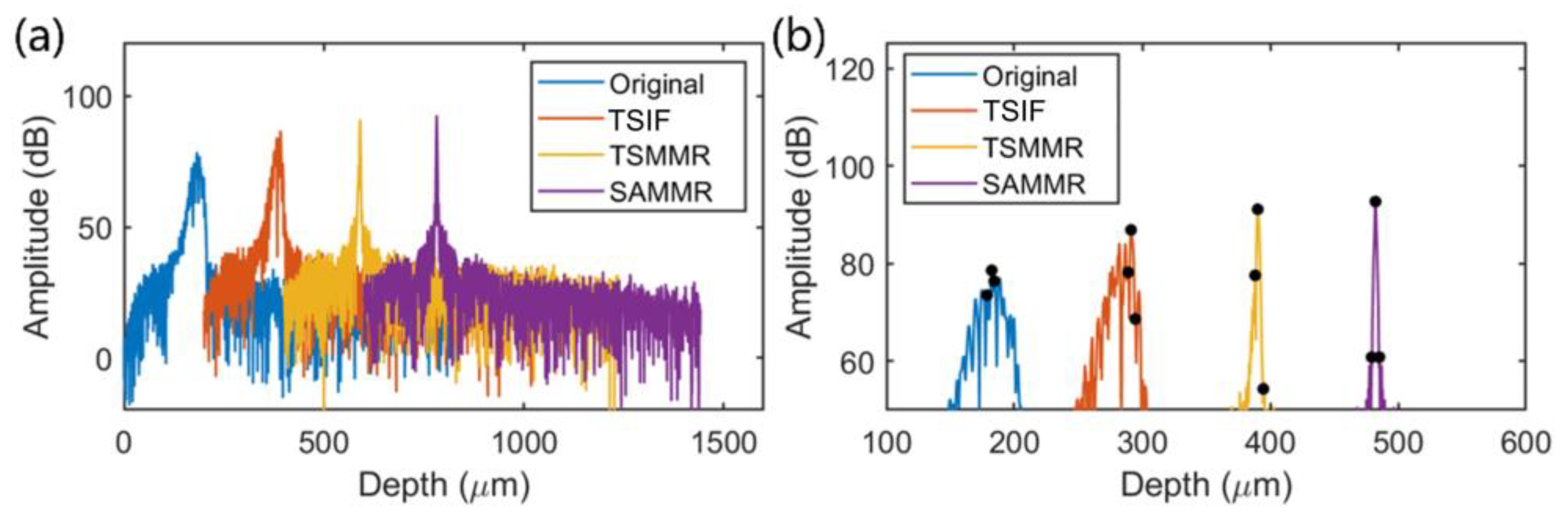
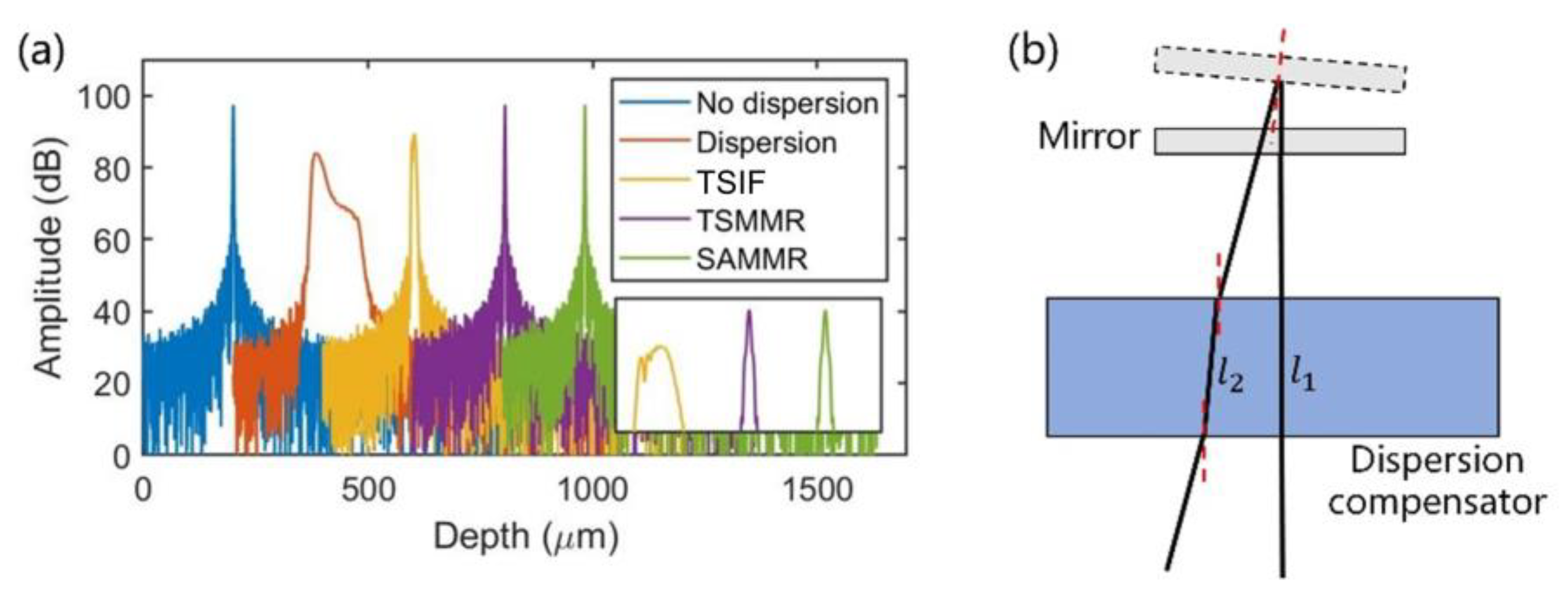
3.1.1. Dispersion-Compensated PSFs and Their Symmetrical Properties
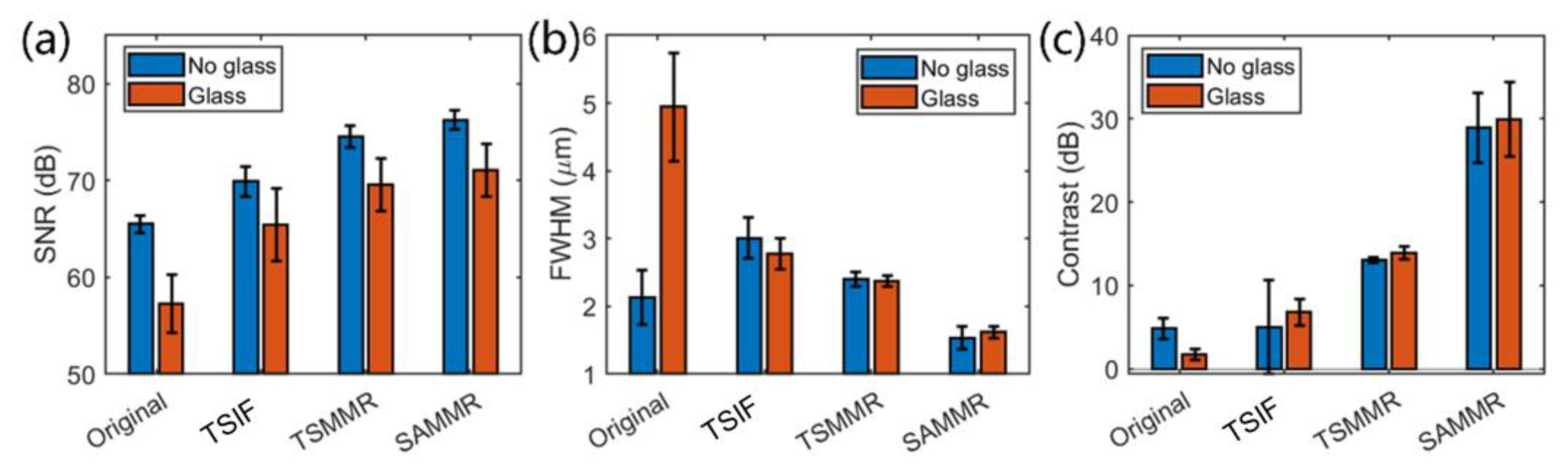
3.1.2. Signal-To-Noise Ratio, Full Width at Half Maximum, and Contrast of PSFs
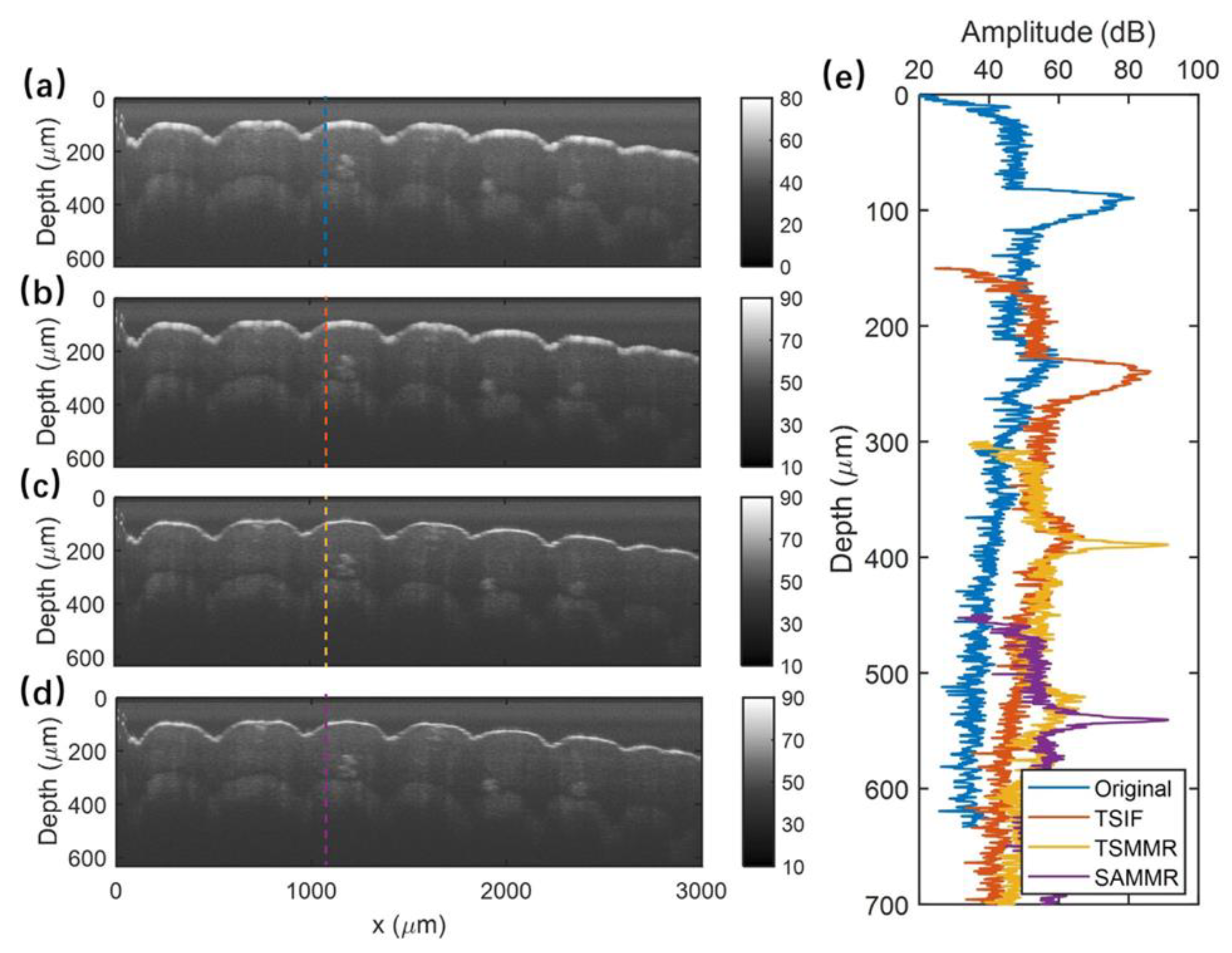
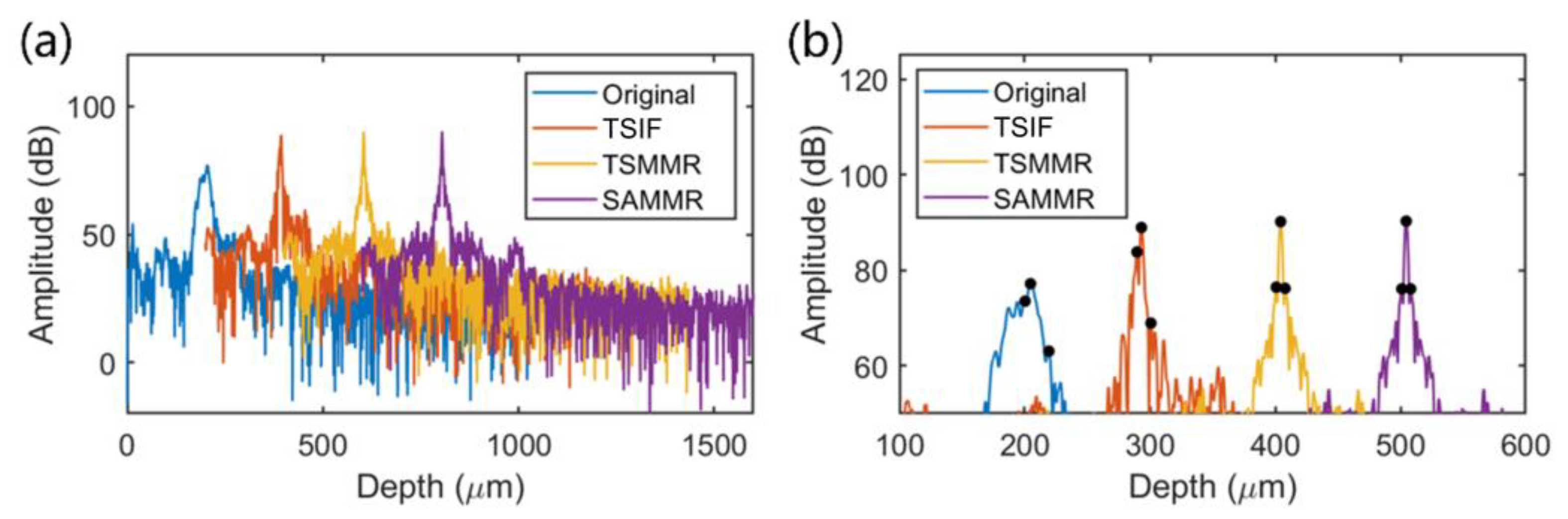
3.2. Improvement of Vis-OCT Images Using the SAMMR Method
3.3. Robustness of the SAMMR Method
3.3.1. Additional Dispersion in the Vis-OCT System
3.3.2. Higher-Order Dispersion in the Vis-OCT System
3.4. Oscillation of Phase Delay and Artificial Peaks
3.5. Application of the SAMMR Method to the 800 nm SD-OCT System
4. Discussion and Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Popescu, D.P.; Choo-Smith, L.-P.; Flueraru, C.; Mao, Y.; Chang, S.; Disano, J.; Sherif, S.; Sowa, M.G. Optical coherence tomography: Fundamental principles, instrumental designs and biomedical applications. Biophys. Rev. 2011, 3, 155–169. [Google Scholar] [CrossRef] [PubMed]
- Yun, S.; Tearney, G.; Bouma, B.; Park, B.; de Boer, J. High-speed spectral-domain optical coherence tomography at 1.3 μm wavelength. Opt. Express 2003, 11, 3598–3604. [Google Scholar] [CrossRef] [PubMed]
- Baran, U.; Choi, W.J.; Wang, R.K. Potential use of OCT-based microangiography in clinical dermatology. Skin Res. Technol. 2016, 22, 238–246. [Google Scholar] [CrossRef] [PubMed]
- Leitgeb, R.; Hitzenberger, C.; Fercher, A. Performance of fourier domain vs time domain optical coherence tomography. Opt. Express 2003, 11, 889–894. [Google Scholar] [CrossRef] [PubMed]
- Jia, Y.; Tan, O.; Tokayer, J.; Potsaid, B.; Wang, Y.; Liu, J.J.; Kraus, M.F.; Subhash, H.; Fujimoto, J.G.; Hornegger, J.; et al. Split-spectrum amplitude-decorrelation angiography with optical coherence tomography. Opt. Express 2012, 20, 4710–4725. [Google Scholar] [CrossRef]
- Pi, S.; Hormel, T.T.; Wei, X.; Cepurna, W.; Wang, B.; Morrison, J.C.; Jia, Y. Retinal capillary oximetry with visible light optical coherence tomography. Proc. Natl. Acad. Sci. USA 2020, 117, 11658–11666. [Google Scholar] [CrossRef]
- Mogensen, M.; Thrane, L.; Jørgensen, T.M.; Andersen, P.E.; Jemec, G.B.E. OCT imaging of skin cancer and other dermatological diseases. J. Biophotonics 2009, 2, 442–451. [Google Scholar] [CrossRef]
- Blatter, C.; Weingast, J.; Alex, A.; Grajciar, B.; Wieser, W.; Drexler, W.; Huber, R.; Leitgeb, R.A. In situ structural and microangiographic assessment of human skin lesions with high-speed OCT. Biomed. Opt. Express 2012, 3, 2636–2646. [Google Scholar] [CrossRef]
- Guagliumi, G.; Sirbu, V. Optical coherence tomography: High resolution intravascular imaging to evaluate vascular healing after coronary stenting. Catheter. Cardiovasc. Interv. 2008, 72, 237–247. [Google Scholar] [CrossRef]
- Adams, D.C.; Hariri, L.P.; Miller, A.J.; Wang, Y.; Cho, J.L.; Villiger, M.; Holz, J.A.; Szabari, M.V.; Hamilos, D.L.; Harris, R.S.; et al. Birefringence microscopy platform for assessing airway smooth muscle structure and function in vivo. Sci. Transl. Med. 2016, 8, 359ra131. [Google Scholar] [CrossRef]
- Yuan, W.; Brown, R.; Mitzner, W.; Yarmus, L.; Li, X. Super-achromatic monolithic microprobe for ultrahigh-resolution endoscopic optical coherence tomography at 800 nm. Nat. Commun. 2017, 8, 1531. [Google Scholar] [CrossRef] [PubMed]
- Luo, Y.; Cui, D.; Yu, X.; Bo, E.; Wang, X.; Wang, N.; Braganza, C.S.; Chen, S.; Liu, X.; Xiong, Q.; et al. Endomicroscopic optical coherence tomography for cellular resolution imaging of gastrointestinal tracts. J. Biophotonics 2018, 11, e201700141. [Google Scholar] [CrossRef] [PubMed]
- Yuan, W.; Feng, Y.; Chen, D.; Gharibani, P.; Chen, J.D.Z.; Yu, H.; Li, X. In vivo assessment of inflammatory bowel disease in rats with ultrahigh-resolution colonoscopic OCT. Biomed. Opt. Express 2022, 13, 2091–2102. [Google Scholar] [CrossRef] [PubMed]
- Jiao, S.; Ruggeri, M. Polarization effect on the depth resolution of optical coherence tomography. J. Biomed. Opt. 2008, 13, 060503. [Google Scholar] [CrossRef]
- Yuan, W.; Mavadia-Shukla, J.; Xi, J.; Liang, W.; Yu, X.; Yu, S.; Li, X. Optimal operational conditions for supercontinuum-based ultrahigh-resolution endoscopic OCT imaging. Opt. Lett. 2016, 41, 250–253. [Google Scholar] [CrossRef]
- Shu, X.; Beckmann, L.; Zhang, H.F. Visible-light optical coherence tomography: A review. J. Biomed. Opt. 2017, 22, 121707. [Google Scholar] [CrossRef]
- Yuan, W.; Chen, D.; Sarabia-Estrada, R.; Guerrero-Cázares, H.; Li, D.; Quiñones-Hinojosa, A.; Li, X. Theranostic OCT microneedle for fast ultrahigh-resolution deep-brain imaging and efficient laser ablation in vivo. Sci. Adv. 2020, 6, eaaz9664. [Google Scholar] [CrossRef]
- Yuan, W.; Thiboutot, J.; Park, H.-C.; Li, A.; Loube, J.; Mitzner, W.; Yarmus, L.; Brown, R.H.; Li, X. Direct Visualization and Quantitative Imaging of Small Airway Anatomy In Vivo Using Deep Learning Assisted Diffractive OCT. IEEE Trans. Biomed. Eng. 2022, 70, 238–246. [Google Scholar] [CrossRef]
- Wachulak, P.; Bartnik, A.; Fiedorowicz, H. Optical coherence tomography (OCT) with 2 nm axial resolution using a compact laser plasma soft X-ray source. Sci. Rep. 2018, 8, 8494. [Google Scholar] [CrossRef]
- Akcay, A.C.; Rolland, J.P.; Eichenholz, J.M. Spectral shaping to improve the point spread function in optical coherence tomography. Opt. Lett. 2003, 28, 1921–1923. [Google Scholar] [CrossRef]
- Pan, L.; Wang, X.; Li, Z.; Zhang, X.; Bu, Y.; Nan, N.; Chen, Y.; Wang, X. Depth-dependent dispersion compensation for full-depth OCT image. Opt. Express 2017, 25, 10345–10354. [Google Scholar] [CrossRef] [PubMed]
- Yaqoob, Z.; Wu, J.; Yang, C. Spectral domain optical coherence tomography: A better OCT imaging strategy. Biotechniques 2005, 39, S6–S13. [Google Scholar] [CrossRef] [PubMed]
- Kho, A.; Srinivasan, V.J. Compensating spatially dependent dispersion in visible light OCT. Opt. Lett. 2019, 44, 775–778. [Google Scholar] [CrossRef] [PubMed]
- Wojtkowski, M.; Srinivasan, V.J.; Ko, T.H.; Fujimoto, J.G.; Kowalczyk, A.; Duker, J.S. Ultrahigh-resolution, high-speed, Fourier domain optical coherence tomography and methods for dispersion compensation. Opt. Express 2004, 12, 2404–2422. [Google Scholar] [CrossRef] [PubMed]
- Cense, B.; Nassif, N.A.; Chen, T.C.; Pierce, M.C.; Yun, S.-H.; Park, B.H.; Bouma, B.E.; Tearney, G.J.; de Boer, J.F. Ultrahigh-resolution high-speed retinal imaging using spectral-domain optical coherence tomography. Opt. Express 2004, 12, 2435–2447. [Google Scholar] [CrossRef]
- Yasuno, Y.; Hong, Y.; Makita, S.; Yamanari, M.; Akiba, M.; Miura, M.; Yatagai, T. In vivo high-contrast imaging of deep posterior eye by 1-μm swept source optical coherence tomography and scattering optical coherence angiography. Opt. Express 2007, 15, 6121–6139. [Google Scholar] [CrossRef]
- Luo, S.; Holland, G.; Mikula, E.; Bradford, S.; Khazaeinezhad, R.; Jester, J.V.; Juhasz, T. Dispersion compensation for spectral domain optical coherence tomography by time-frequency analysis and iterative optimization. Opt. Contin. 2022, 1, 1117–1136. [Google Scholar] [CrossRef]
- Singh, K.; Sharma, G.; Tearney, G.J. Estimation and compensation of dispersion for a high-resolution optical coherence tomography system. J. Opt. 2018, 20, 025301. [Google Scholar] [CrossRef]
- Makita, S.; Fabritius, T.; Yasuno, Y. Full-range, high-speed, high-resolution 1-µm spectral-domain optical coherence tomography using BM-scan for volumetric imaging of the human posterior eye. Opt. Express 2008, 16, 8406–8420. [Google Scholar] [CrossRef]
- Uribe-Patarroyo, N.; Kassani, S.H.; Villiger, M.; Bouma, B.E. Robust wavenumber and dispersion calibration for Fourier-domain optical coherence tomography. Opt. Express 2018, 26, 8081–9094. [Google Scholar] [CrossRef]
- Attendu, X.; Ruis, R.M. Simple and robust calibration procedure for k-linearization and dispersion compensation in optical coherence tomography. J. Biomed. Opt. 2019, 24, 056001. [Google Scholar] [CrossRef]
- Ahmed, S.; Le, D.; Son, T.; Adejumo, T.; Ma, G.; Yao, X. ADC-Net: An Open-Source Deep Learning Network for Automated Dispersion Compensation in Optical Coherence Tomography. Front. Med. 2022, 9, 864879. [Google Scholar] [CrossRef] [PubMed]
- Yang, D.; Guo, W.; Cheng, T.; Wei, Z.; Xu, B. Artificial neural network (ANN) for dispersion compensation of spectral domain–Optical coherence tomography (SD-OCT). Instrum. Sci. Technol. 2022, 50, 560–576. [Google Scholar] [CrossRef]
- Chong, S.P.; Zhang, T.; Kho, A.; Bernucci, M.T.; Dubra, A.; Srinivasan, V.J. Ultrahigh resolution retinal imaging by visible light OCT with longitudinal achromatization. Biomed. Opt. Express 2018, 9, 1477–1491. [Google Scholar] [CrossRef] [PubMed]
- Song, W.; Zhou, L.; Zhang, S.; Ness, S.; Desai, M.; Yi, J. Fiber-based visible and near infrared optical coherence tomography (vnOCT) enables quantitative elastic light scattering spectroscopy in human retina. Biomed. Opt. Express 2018, 9, 3464–3480. [Google Scholar] [CrossRef]
- Chan, K.K.H.; Tang, S. High-speed spectral domain optical coherence tomography using non-uniform fast Fourier transform. Biomed. Opt. Express. 2010, 1, 1309–1319. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Xu, C.; Zhu, S.; Chen, D.; Qiu, H.; Lam, A.K.N.; Leung, C.K.S.; Yuan, W. A Generic and Effective System Dispersion Compensation Method: Development and Validation in Visible-Light OCT. Photonics 2023, 10, 892. https://doi.org/10.3390/photonics10080892
Wang J, Xu C, Zhu S, Chen D, Qiu H, Lam AKN, Leung CKS, Yuan W. A Generic and Effective System Dispersion Compensation Method: Development and Validation in Visible-Light OCT. Photonics. 2023; 10(8):892. https://doi.org/10.3390/photonics10080892
Chicago/Turabian StyleWang, Jiarui, Chao Xu, Shaodi Zhu, Defu Chen, Haixia Qiu, Alexander K. N. Lam, Christopher K. S. Leung, and Wu Yuan. 2023. "A Generic and Effective System Dispersion Compensation Method: Development and Validation in Visible-Light OCT" Photonics 10, no. 8: 892. https://doi.org/10.3390/photonics10080892
APA StyleWang, J., Xu, C., Zhu, S., Chen, D., Qiu, H., Lam, A. K. N., Leung, C. K. S., & Yuan, W. (2023). A Generic and Effective System Dispersion Compensation Method: Development and Validation in Visible-Light OCT. Photonics, 10(8), 892. https://doi.org/10.3390/photonics10080892







