The Design of a Photonic Crystal Fiber for Hydrogen Cyanide Gas Detection
Abstract
:1. Introduction
2. Proposed PCF-Based Geometrics
3. Essential Equations for PCF Characteristics
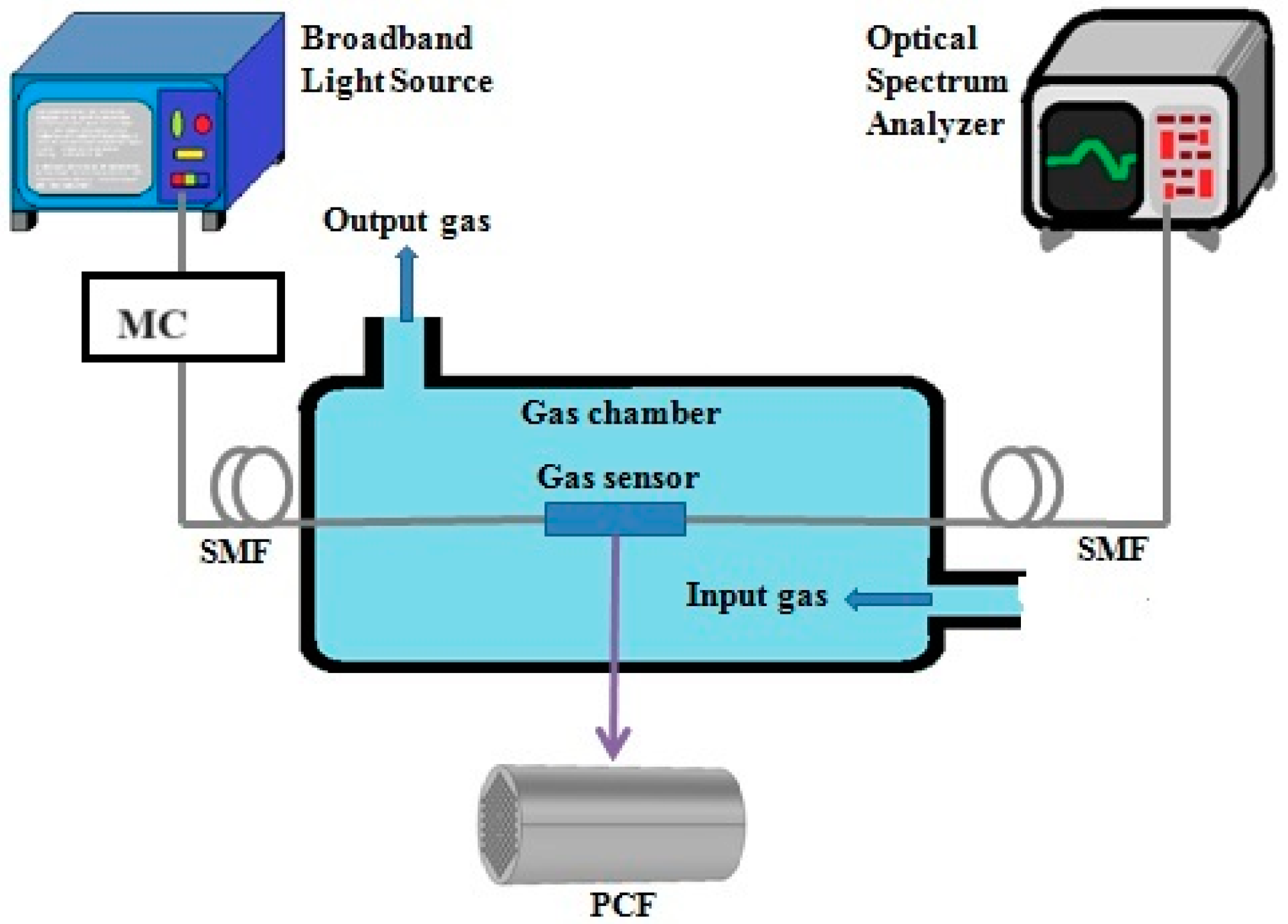
4. Simulation Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kawasaki, H.; Ueda, T.; Suda, Y.; Ohshima, T. Properties of metal doped tungsten oxide thin films for NOx gas sensors grown by PLD method combined with sputtering process. Sens. Actuators B Chem. 2004, 100, 266–269. [Google Scholar] [CrossRef]
- Walia, G.K.; Randhawa, D.K.K. Density-functional study of hydrogen cyanide adsorption on silicene nanoribbons. J. Mol. Model. 2018, 24, 242. [Google Scholar] [CrossRef]
- Siegien, I.; Bogatek, R. Cyanide action in plants—From toxic to regulatory. Acta Physiol. 2006, 28, 483–497. [Google Scholar] [CrossRef]
- Enderby, B.; Smith, D.; Carroll, W.; Lenney, W. Hydrogen cyanide as a biomarker for Pseudomonas aeruginosa in the breath of children with cystic fibrosis. Pediatr. Pulmonol. 2009, 44, 142–147. [Google Scholar] [CrossRef]
- Arslanov, D.D.; Castro, M.P.P.; Creemers, N.A.; Neerincx, A.H.; Spunei, M.; Mandon, J.; Cristescu, S.M.; Merkus, P.; Harren, F.J.M. Optical parametric oscillator-based photoacoustic detection of hydrogen cyanide for biomedical applications. J. Biomed. Opt. 2013, 18, 107002. [Google Scholar] [CrossRef]
- Arroyo, P.; Gómez-Suárez, J.; Herrero, J.L.; Lozano, J. Electrochemical gas sensing module combined with Unmanned Aerial Vehicles for air quality monitoring. Sens. Actuators B Chem. 2022, 364, 131815. [Google Scholar] [CrossRef]
- Kaaliveetil, S.; Yang, J.; Alssaidy, S.; Li, Z.; Cheng, Y.-H.; Menon, N.H.; Chande, C.; Basuray, S. Microfluidic Gas Sensors: Detection Principle and Applications. Micromachines 2022, 13, 1716. [Google Scholar] [CrossRef] [PubMed]
- Isaac, N.A.; Pikaar, I.; Biskos, G. Metal oxide semiconducting nanomaterials for air quality gas sensors: Operating principles, performance, and synthesis techniques. Microchim. Acta 2022, 189, 196. [Google Scholar] [CrossRef] [PubMed]
- Kumar, N.; Suthar, B.; Nayak, C.; Bhargava, A. Analysis of a gas sensor based on one-dimensional photonic crystal structure with a designed defect cavity. Phys. Scr. 2023, 98, 065506. [Google Scholar] [CrossRef]
- Hodgkinson, J.; Tatam, R.P. Optical gas sensing: A review. Meas. Sci. Technol. 2012, 24, 012004. [Google Scholar] [CrossRef]
- Knight, J.C.; Broeng, J.; Birks, T.A.; Russell, P.S.J. Photonic band gap guidance in optical fibers. Science 1998, 282, 1476–1478. [Google Scholar] [CrossRef] [PubMed]
- Fini, J.M. Microstructure fibers for optical sensing in gasses and liquids. Meas. Sci. Technol. 2004, 15, 1120–1128. [Google Scholar] [CrossRef]
- Knight, J.C.; Birks, T.A.; Russell, P.S.J.; Atkin, D.M. All-silica single-mode optical fiber with photonic crystal cladding. Opt. Lett. 1996, 21, 1547–1549. [Google Scholar] [CrossRef] [PubMed]
- Birks, T.A.; Knight, J.C.; Russell, P.S.J. Endlessly single-mode photonic crystal fiber. Opt. Lett. 1997, 22, 961–963. [Google Scholar] [CrossRef] [PubMed]
- Qin, J.; Zhu, B.; Du, Y.; Han, Z. Terahertz detection of toxic gas using a photonic crystal fiber. Opt. Fiber Technol. 2019, 52, 101990. [Google Scholar] [CrossRef]
- Henningsen, J.; Hald, J.; Peterson, J.C. Saturated absorption in acetylene and hydrogen cyanide in hollow-core photonic bandgap fibers. Opt. Express 2005, 13, 10475–10482. [Google Scholar] [CrossRef] [PubMed]
- Asaduzzaman, S.; Ahmed, K. Proposal of a gas sensor with high sensitivity, birefringence and nonlinearity for air pollution monitoring. Sens. Bio-Sens. Res. 2016, 10, 20–26. [Google Scholar] [CrossRef]
- Chowdhury, S.; Sen, S.; Ahmed, K.; Paul, B.K.; Alam Miah, M.B.; Asaduzzaman, S.; Islam, S.; Islam, I. Porous shaped photonic crystal fiber with strong confinement field in sensing applications: Design and analysis. Sens. Bio-Sens. Res. 2017, 13, 63–69. [Google Scholar] [CrossRef]
- Fard, A.M.; Sarraf, M.J.; Khatib, F. Design and optimization of index guiding photonic crystal fiber-based gas sensor. Optik 2021, 232, 166448. [Google Scholar] [CrossRef]
- Abbaszadeh, A.; Makouei, S.; Meshgini, S. Ammonia measurement in exhaled human breath using PCF sensor for medical applications. Photonics Nanostruct. Fundam. Appl. 2021, 44, 100917. [Google Scholar] [CrossRef]
- Dashtban, Z.; Salehi, M.R.; Abiri, E. High sensitivity all-optical sensor for detecting toxic gases using hollow-core photonic crystal fiber composed of magnesium fluoride. Opt. Fiber Technol. 2022, 72, 102958. [Google Scholar] [CrossRef]
- Mishra, G.P.; Kumar, D.; Chaudhary, V.S.; Kumar, S. Design and sensitivity improvement of microstructured-core photonic crystal fiber based sensor for methane and hydrogen fluoride detection. IEEE Sens. J. 2021, 22, 1265–1272. [Google Scholar] [CrossRef]
- Mortazavi, S.; Makouei, S.; Garamaleki, S.M. Hollow core photonic crystal fiber based carbon monoxide sensor design applicable for hyperbilirubinemia diagnosis. Opt. Eng. 2023, 62, 066105. [Google Scholar] [CrossRef]
- Nizar, S.M.; Britto, E.C.; Michael, M.; Sagadevan, K. Photonic crystal fiber sensor structure with vertical and horizontal cladding for the detection of hazardous gases. Opt. Quantum Electron. 2023, 55, 1186. [Google Scholar] [CrossRef]
- Azhar, M.; Mandon, J.; Neerincx, A.H.; Liu, Z.; Mink, J.; Merkus, P.J.F.M.; Cristescu, S.M.; Harren, F.J.M. A widely tunable, near-infrared laser-based trace gas sensor for hydrogen cyanide (HCN) detection in exhaled breath. Appl. Phys. B Laser Opt. 2017, 123, 268. [Google Scholar] [CrossRef]
- Shi, X.; Zhao, Z.; Han, Z. Highly sensitive and selective gas sensing using the defect mode of a compact terahertz photonic crystal cavity. Sens. Actuators B Chem. 2018, 274, 188–193. [Google Scholar] [CrossRef]
- Yoshida, M.; Yoshida, K.; Kasai, K.; Nakazawa, M. 155 μm hydrogen cyanide optical frequency-stabilized and 10 GHz repetition-rate-stabilized mode-locked fiber laser. Opt. Express 2016, 24, 24287–24296. [Google Scholar] [CrossRef] [PubMed]
- Monro, T.M.; Belardi, W.; Furusawa, K.; Baggett, J.C.; Broderick, N.G.R.; Richardson, D.J. Sensing with microstructured optical fibres. Meas. Sci. Technol. 2001, 12, 854–858. [Google Scholar] [CrossRef]
- Wanstall, C.T.; Agrawal, A.K.; Bittle, J.A. Implications of real-gas behavior on refractive index calculations for optical diagnostics of fuel–air mixing at high pressures. Combust. Flame 2020, 214, 47–56. [Google Scholar] [CrossRef]
- Kuwabara, M. Photonic Crystals Fabricated by Sol-Gel Process, Handbook of Sol-Gel Science and Technology; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- Sun, X.H.; Tao, X.M.; Wang, Y.Y. Various photonic crystal structures fabricated by using a top-cut hexagonal prism. Appl. Phys. A 2010, 98, 255–261. [Google Scholar] [CrossRef]
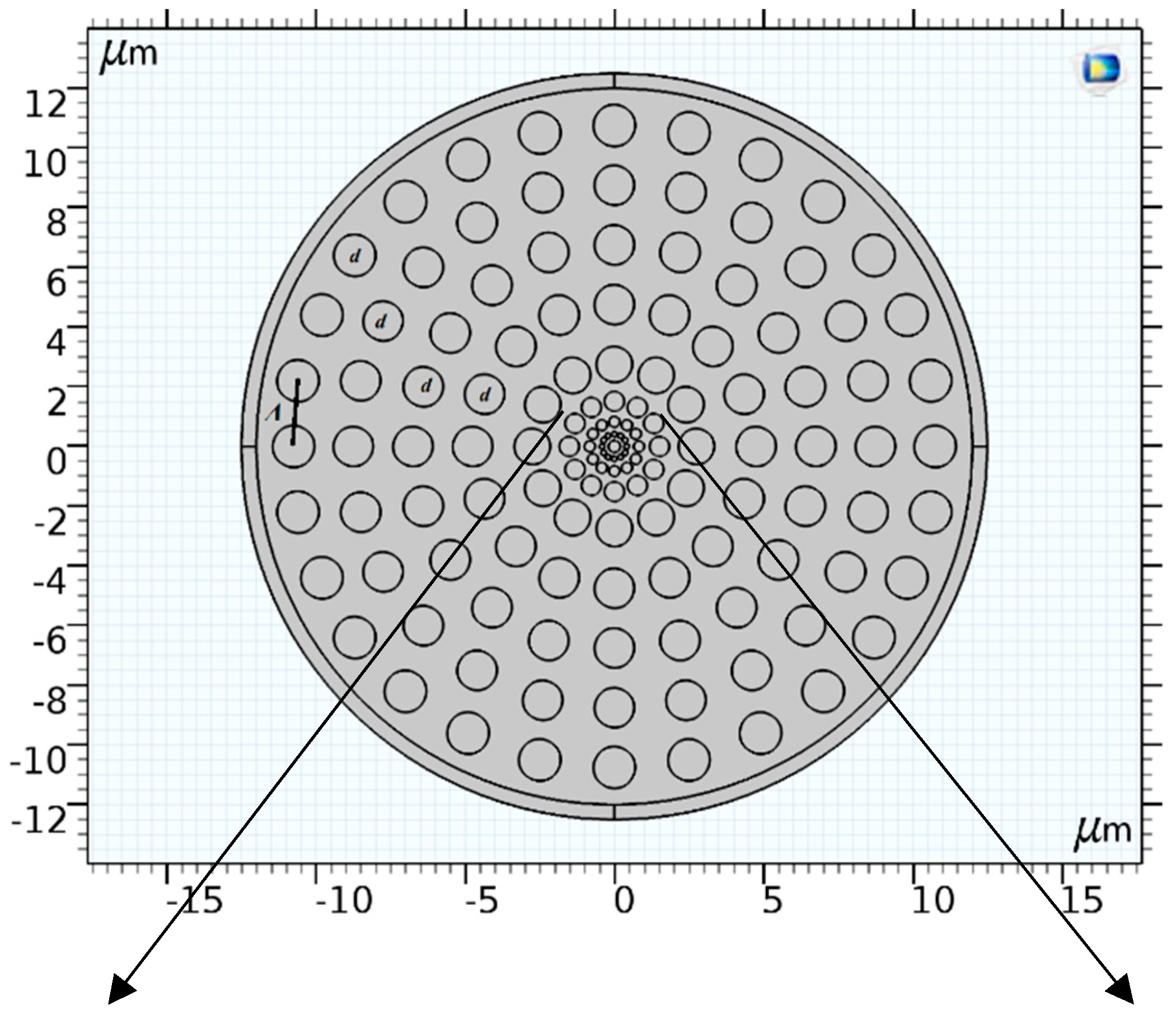

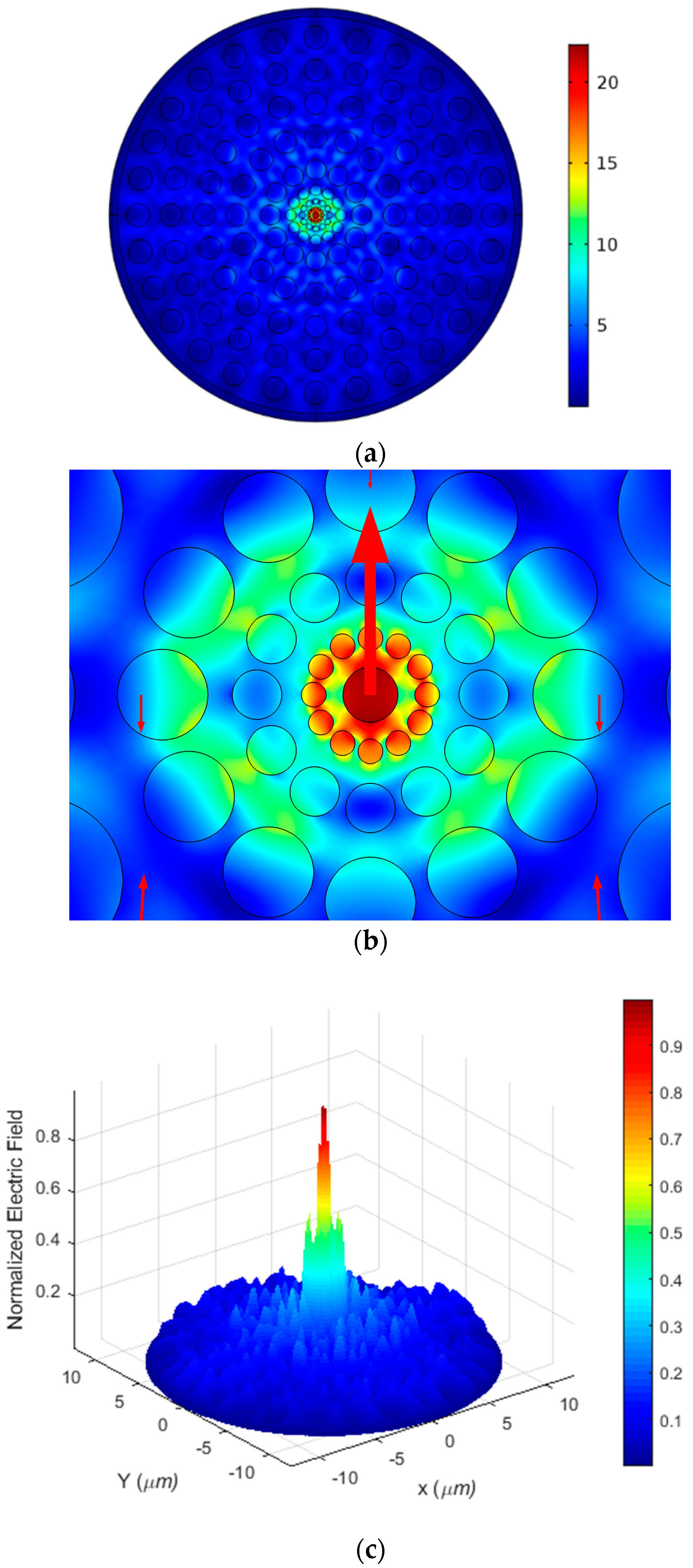
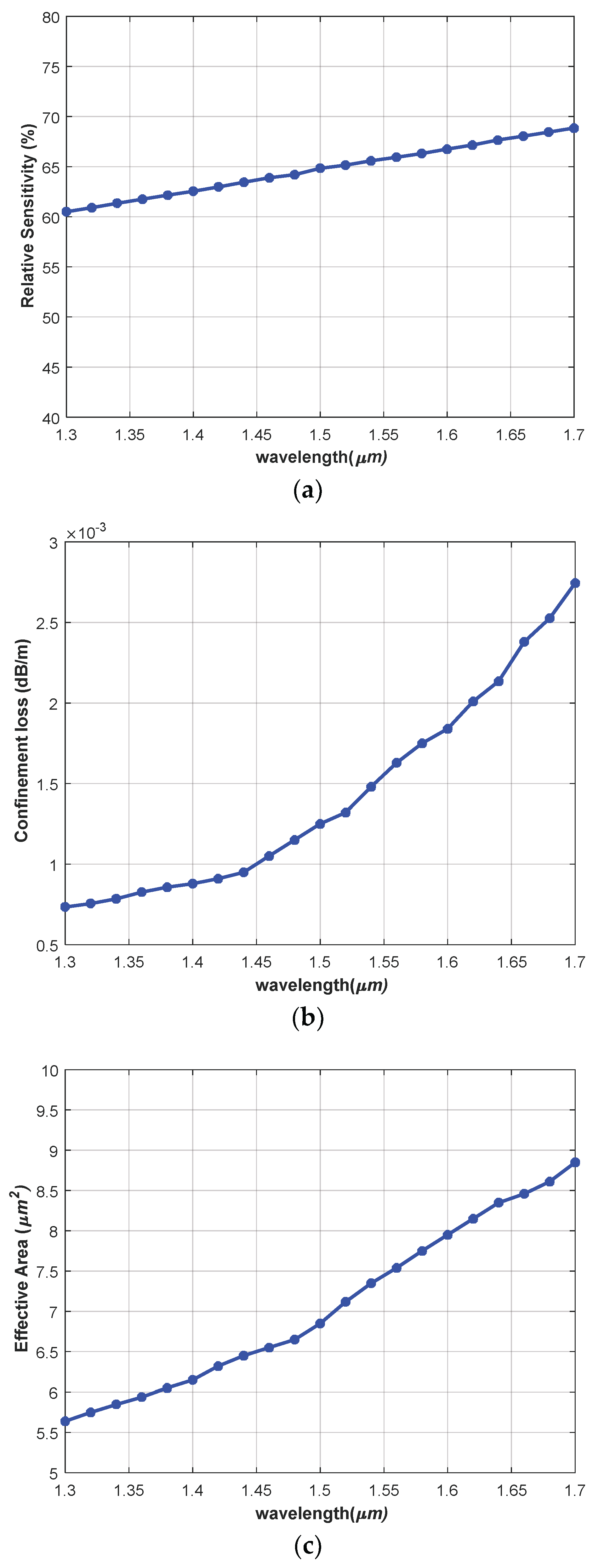
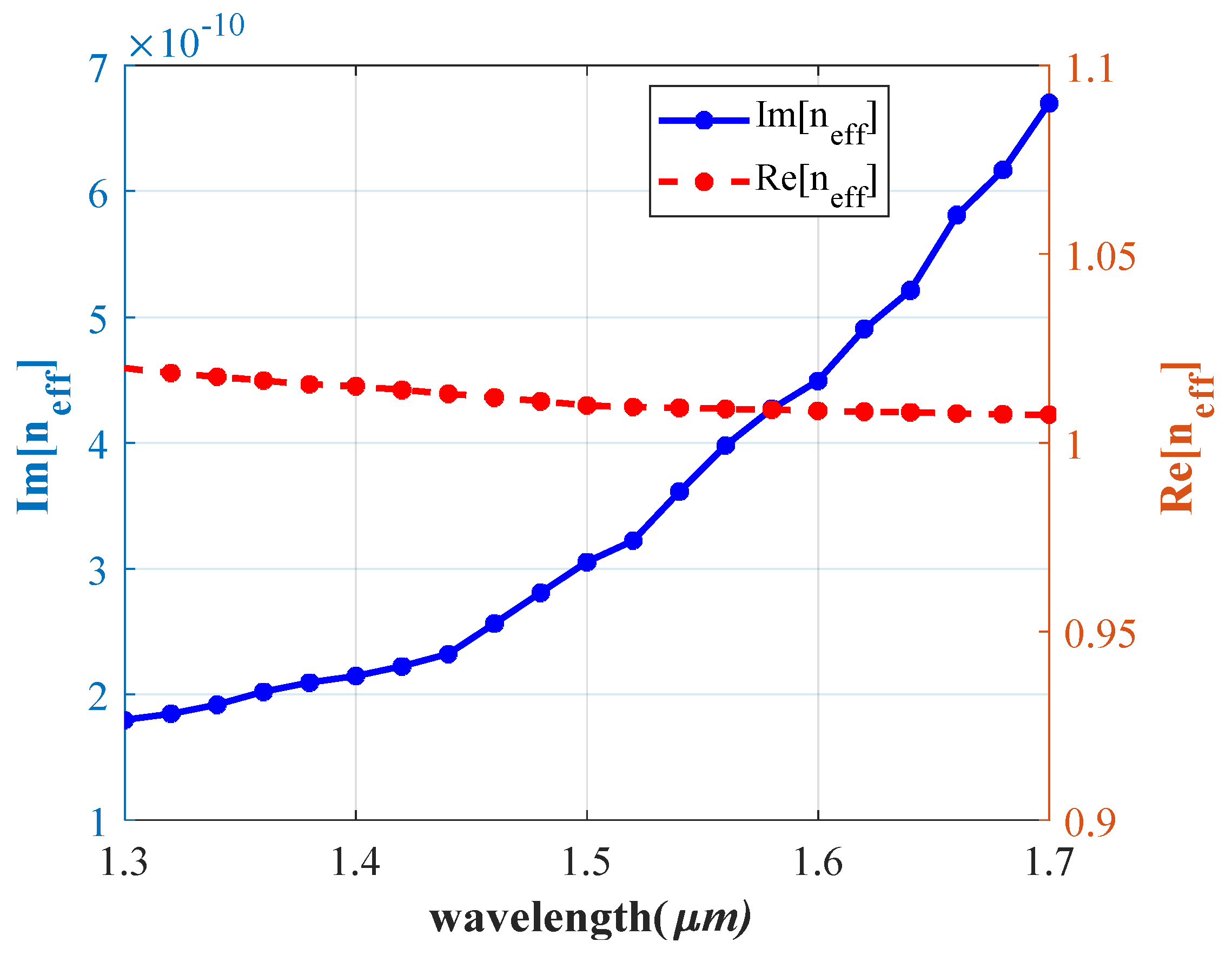
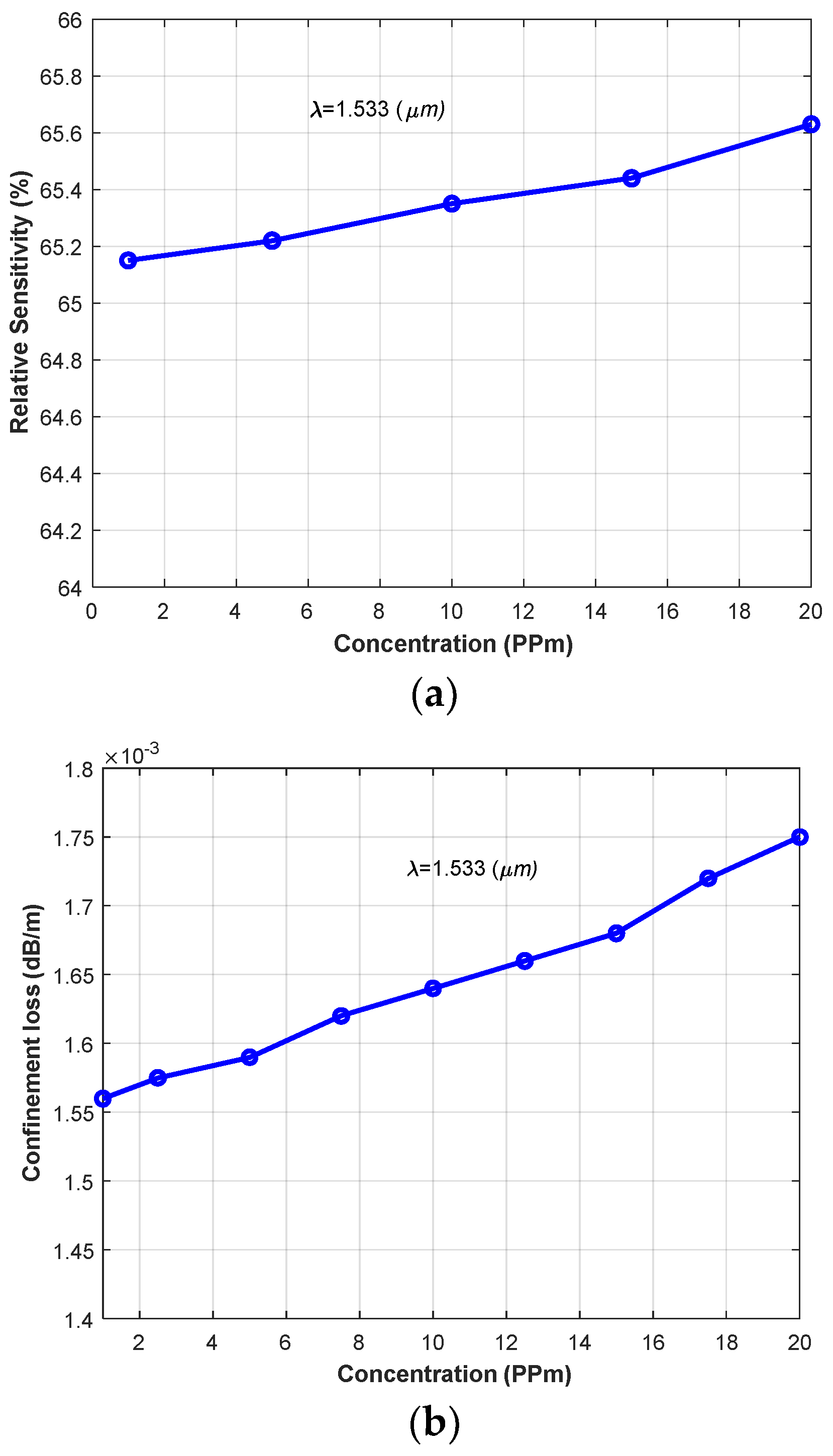
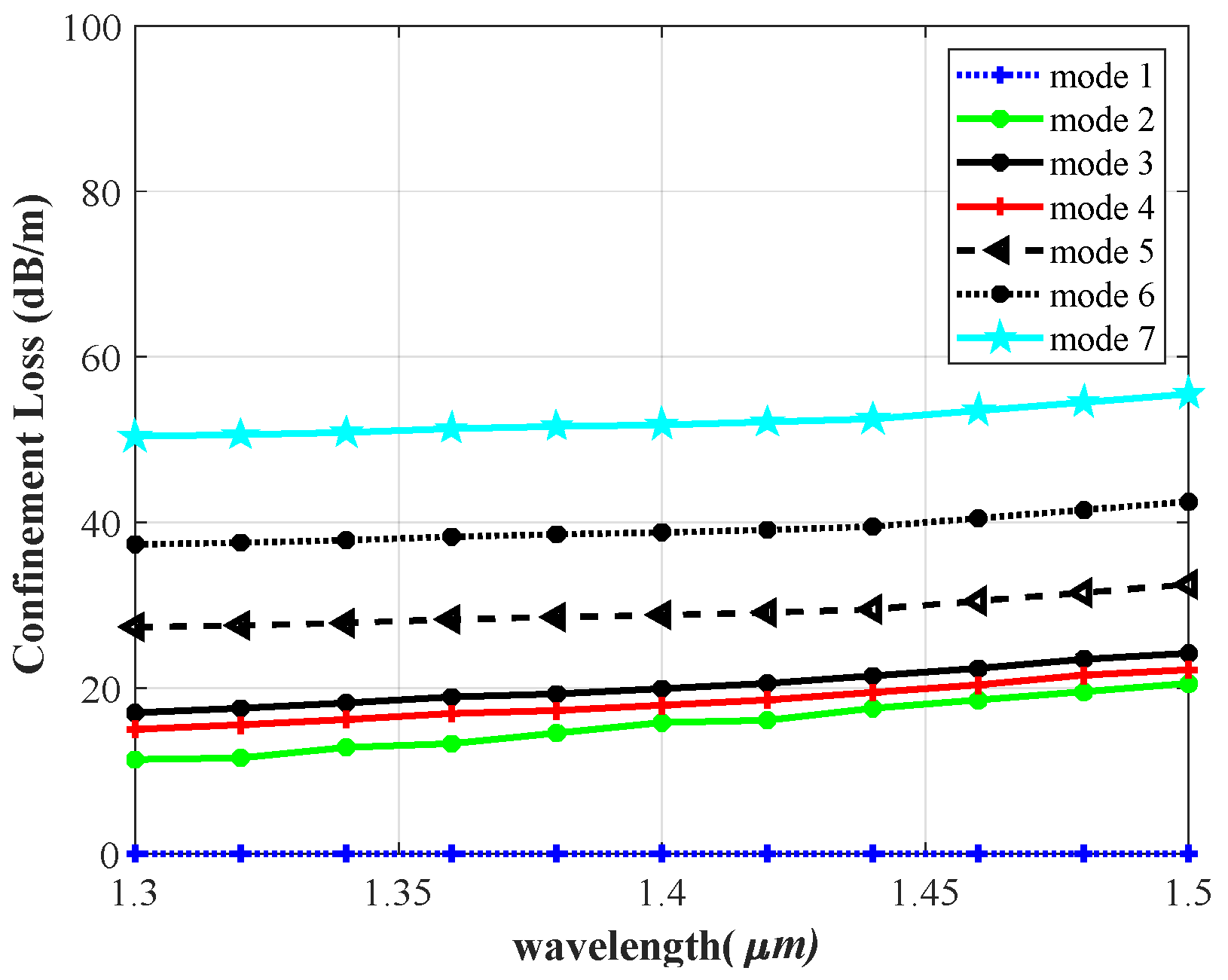
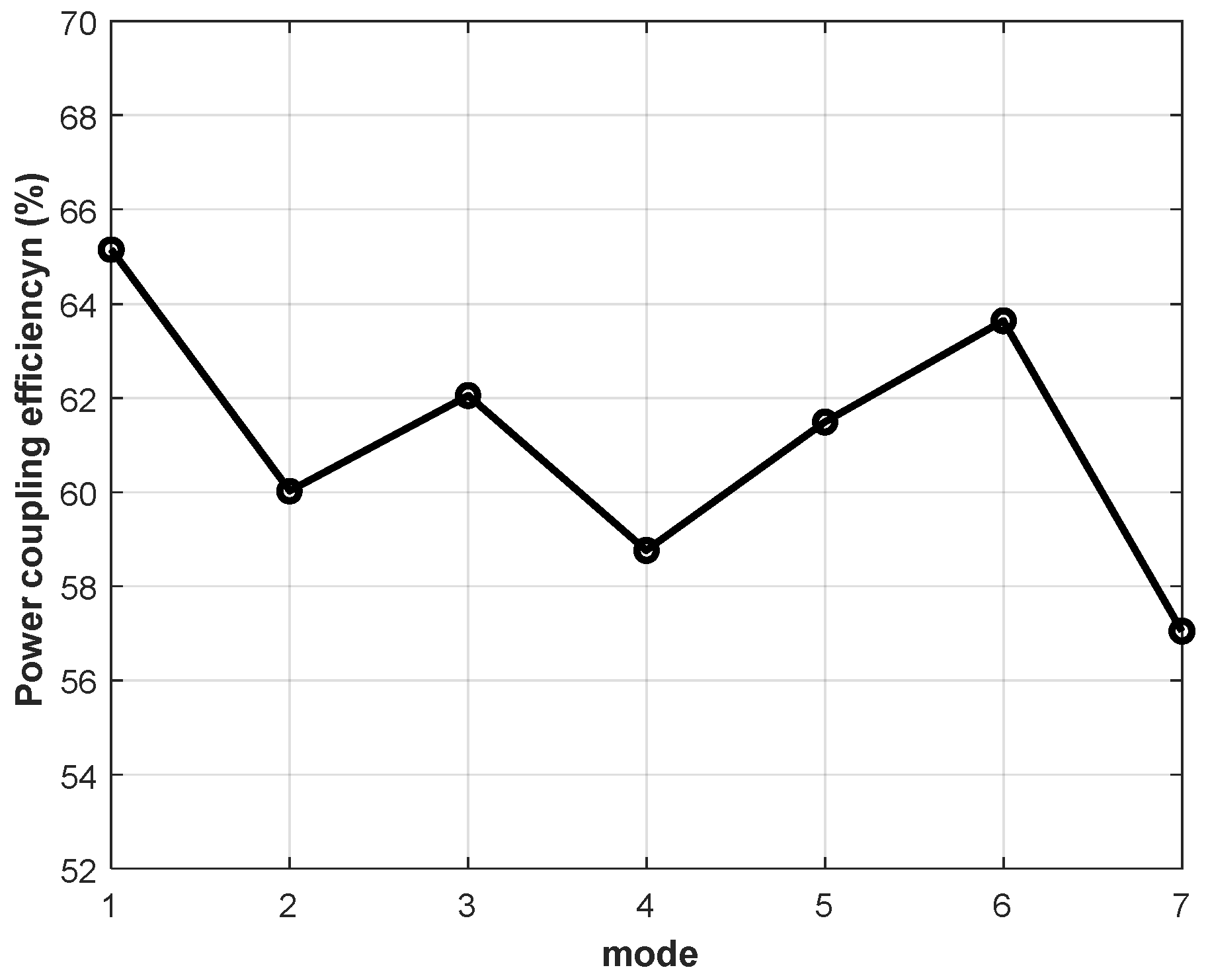
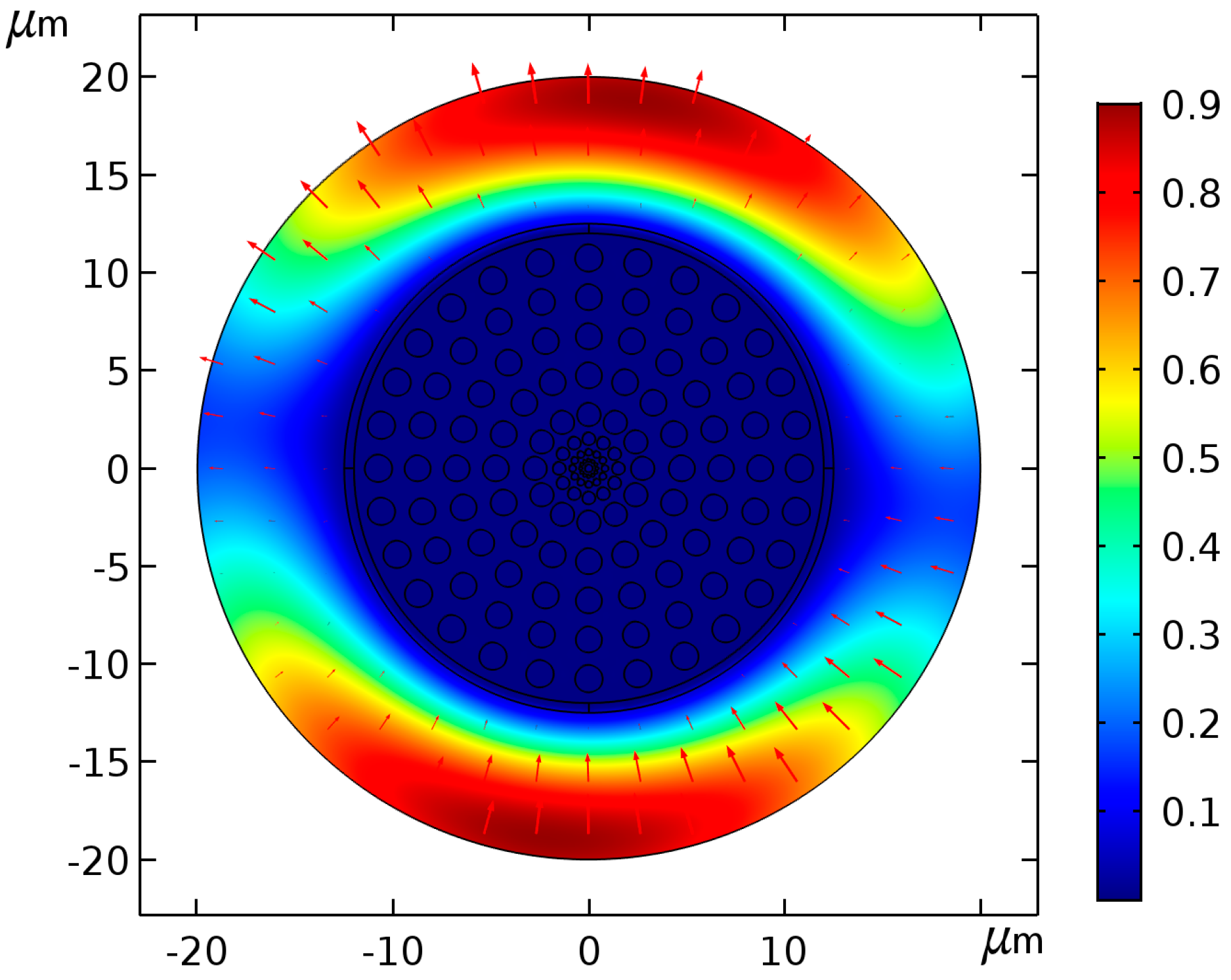
| Parameter | d | Λ | d1 | d2 | d3 | d4 | Λ1 | Λ2 | Λ3 |
|---|---|---|---|---|---|---|---|---|---|
| Value(µm) | 1.33 | 1.44 | 1.2 | 0.66 | 0.36 | 0.8 | 1.26 | 0.72 | 0.4 |
| PCF Structure | Sensing Gas | Sensitivity (%) | Confinement Loss (dB/m) | Wavelength (µm) |
|---|---|---|---|---|
| Five hexagonal rings in cladding Eight horizontally arranged rings in elliptical hole–core [17] | HF | 53 | 3.21 × 10−6 | 1.33 |
| Porous cladding Porous core [18] | - | 60.57 | 8.7 × 10−8 | 1.33 |
| Five hexagonal rings in cladding Two circular rings in core [20] | NH3 | 63 | 1.98 × 10−4 | 1.544 |
| Six hexagonal rings in cladding One circular ring in core Four noncircular quarter sectors between the core and cladding [19] | H2S/CH4 | 67 | 8.61 × 10−5 | 1.578 |
| Air holes around the core with quasi-crystalline arrangement [21] | H2S/CH4 | 50 | 2.1 × 10−6 | 1.55 |
| Five hexagonal rings in cladding Two circular rings in core [22] | HF | 44.47 | 1.83 × 10−8 | 1.33 |
| Five hexagonal rings in cladding Circular and elliptical hole–core [23] | CO | 64 | 3.8 × 10−3 | 1.567 |
| Two hexagonal rings with vertical/horizontal elliptical holes in the cladding Two circular rings with elliptical holes in the core [24] | C10H16 | 65 | 2.32 × 10−3 | 1 |
| Five circular rings in cladding Three circular rings in core (this work) | HCN | 65.13 | 1.5 × 10−3 | 1.533 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pourfathi Fard, A.; Makouei, S.; Danishvar, M.; Danishvar, S. The Design of a Photonic Crystal Fiber for Hydrogen Cyanide Gas Detection. Photonics 2024, 11, 178. https://doi.org/10.3390/photonics11020178
Pourfathi Fard A, Makouei S, Danishvar M, Danishvar S. The Design of a Photonic Crystal Fiber for Hydrogen Cyanide Gas Detection. Photonics. 2024; 11(2):178. https://doi.org/10.3390/photonics11020178
Chicago/Turabian StylePourfathi Fard, Abdolreza, Somayeh Makouei, Morad Danishvar, and Sebelan Danishvar. 2024. "The Design of a Photonic Crystal Fiber for Hydrogen Cyanide Gas Detection" Photonics 11, no. 2: 178. https://doi.org/10.3390/photonics11020178
APA StylePourfathi Fard, A., Makouei, S., Danishvar, M., & Danishvar, S. (2024). The Design of a Photonic Crystal Fiber for Hydrogen Cyanide Gas Detection. Photonics, 11(2), 178. https://doi.org/10.3390/photonics11020178








