Wide-Angle, Polarization-Independent Broadband Metamaterial Absorber by Using Plasmonic Metasurface-Based Split-Circular Structure
Abstract
:1. Introduction
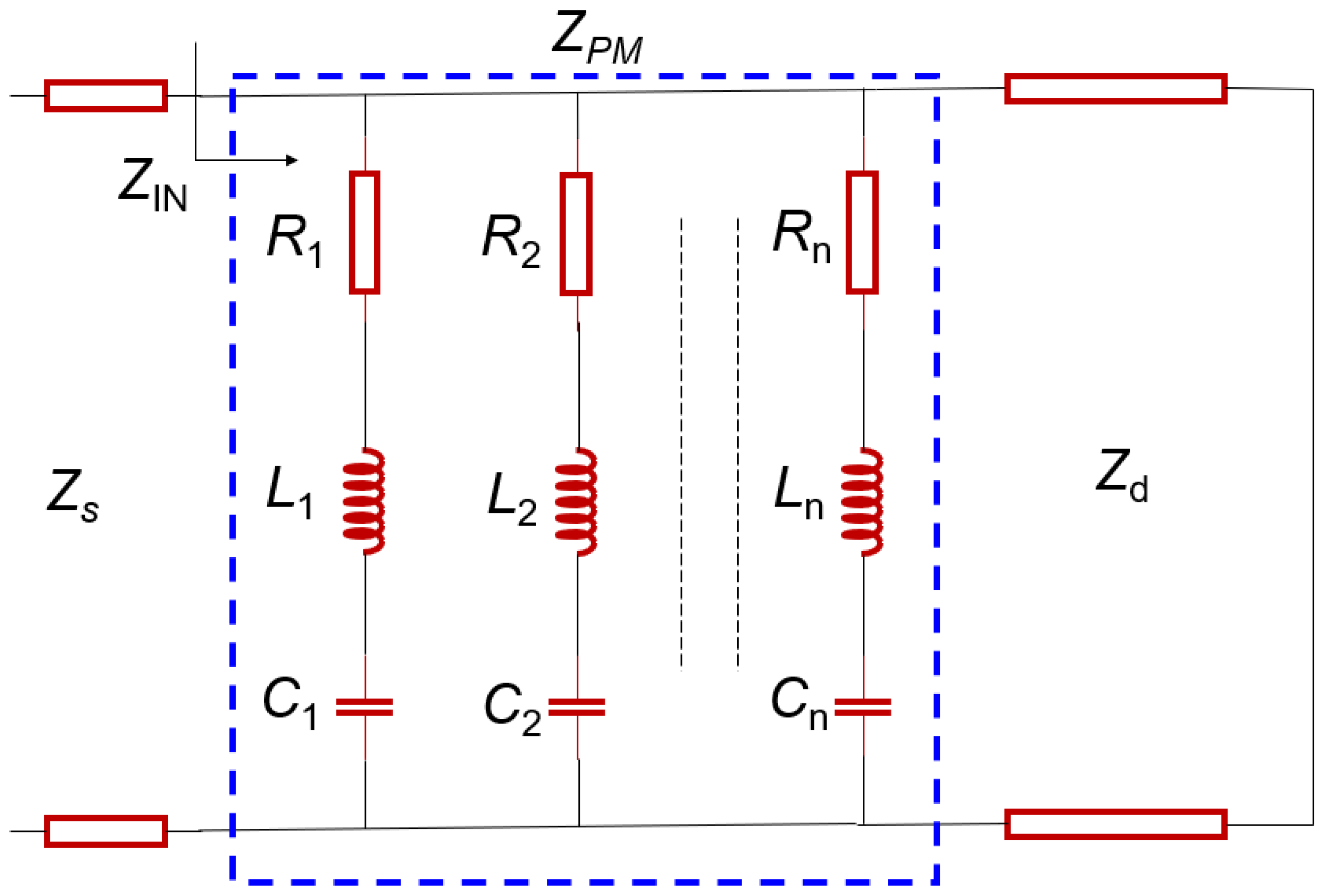
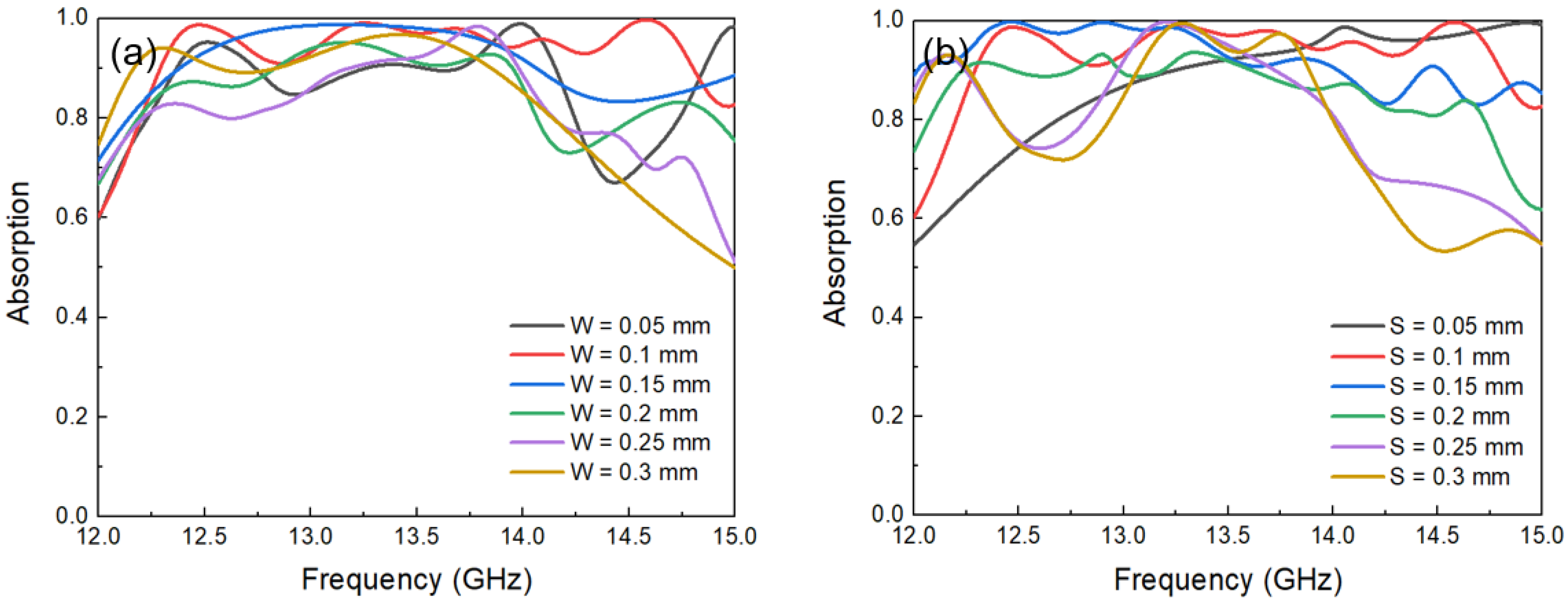
2. Design and Analysis
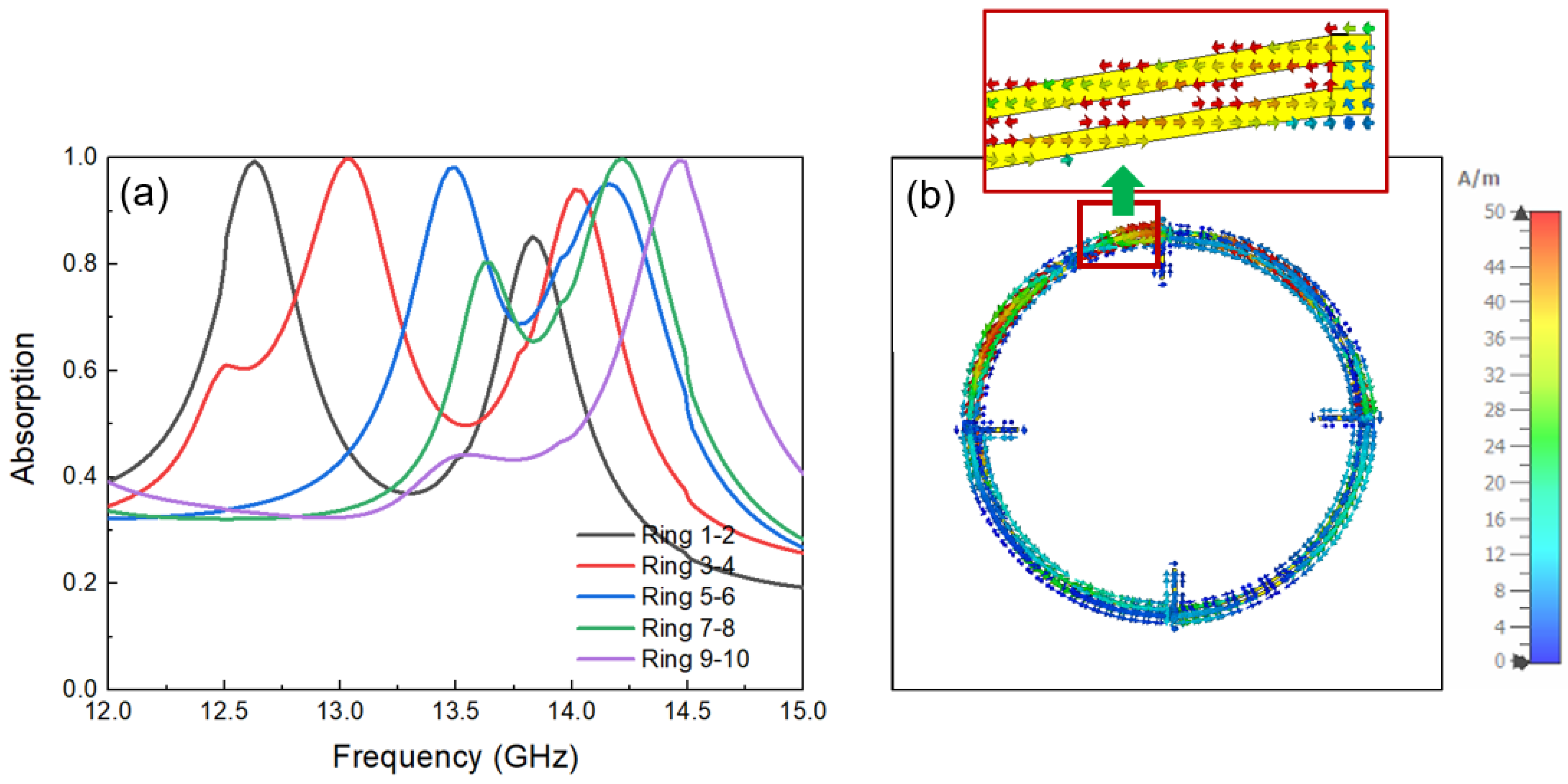
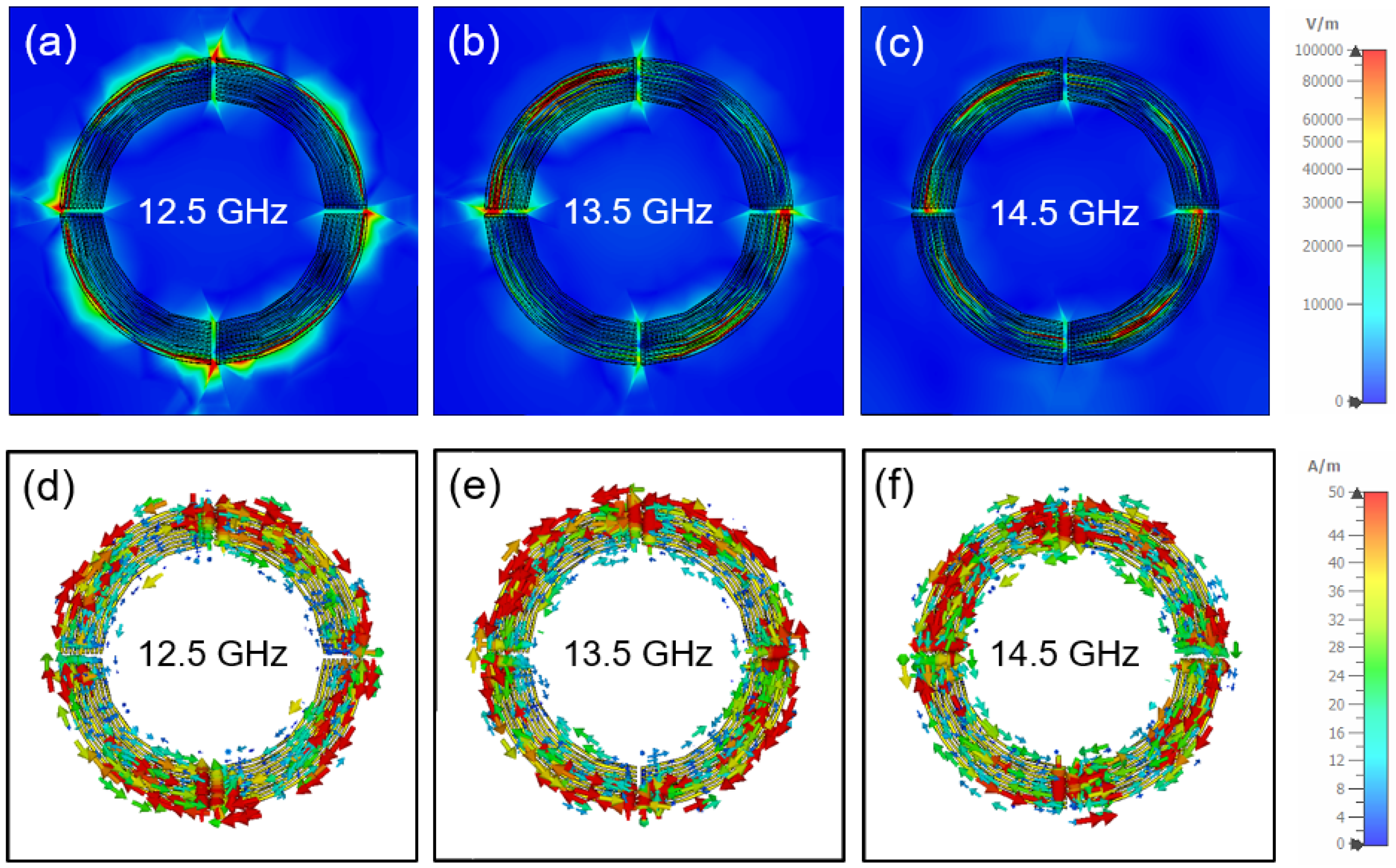
3. Experimental Verification for the Proposed Plasmonic Metasurface
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xiao, S.; Wang, T.; Liu, T.; Zhou, C.; Jiang, X.; Zhang, J. Active Metamaterials and Metadevices: A Review. J. Phys. D Appl. Phys. 2020, 53, 503002. [Google Scholar] [CrossRef]
- Kadic, M.; Milton, G.W.; van Hecke, M.; Wegener, M. 3D Metamaterials. Nat. Rev. Phys. 2019, 1, 198–210. [Google Scholar] [CrossRef]
- Nguyen, H.A.; Pham, T.S.; Tung, B.S.; Khuyen, B.X.; Le, D.T.; Vu, H.Y.; Vu, D.L.; Hien, N.T. Metamaterials Based on Hyperbolic-Graphene Composite: A Pathway from Positive to Negative Refractive Index at Terahertz. Comput. Mater. Sci. 2025, 248, 113574. [Google Scholar] [CrossRef]
- Fowler, C.; Silva, S.; Thapa, G.; Zhou, J. High Efficiency Ambient RF Energy Harvesting by a Metamaterial Perfect Absorber. Opt. Mater. Express OME 2022, 12, 1242–1250. [Google Scholar] [CrossRef]
- Hiep, L.T.H.; Bui, H.N.; Tung, B.S.; Lam, V.D.; Khuyen, B.X.; Pham, T.S. Enhanced Efficiency of Magnetic Resonant Wireless Power Transfer System Using Rollable and Foldable Metasurface Based on Polyimide Substrate. Appl. Phys. A 2024, 130, 521. [Google Scholar] [CrossRef]
- Rakhshani, M.R.; Rashki, M. Metamaterial Perfect Absorber Using Elliptical Nanoparticles in a Multilayer Metasurface Structure with Polarization Independence. Opt. Express OE 2022, 30, 10387–10399. [Google Scholar] [CrossRef]
- Bhati, R.; Malik, A.K. Multiband Terahertz Metamaterial Perfect Absorber for Microorganisms Detection. Sci. Rep. 2023, 13, 19685. [Google Scholar] [CrossRef]
- Tung, B.S.; Viet, N.N.; Khuyen, B.X.; Pham, T.S.; Do, P.X.; Hoa, N.T.; Lam, V.D.; Tung, D.K. Multi-Band and Polarization-Insensitive Electromagnetically-Induced Transparency Based on Coupled-Resonators in a Metamaterial Operating at GHz Frequencies. Phys. Scr. 2024, 99, 115502. [Google Scholar] [CrossRef]
- Shruti; Pahadsingh, S.; Appasani, B. Metamaterial-Based Terahertz Absorbers for Refractive Index Sensing: Types, Mechanism, and Applications. Plasmonics 2024. [Google Scholar] [CrossRef]
- Saadeldin, A.S.; Sayed, A.M.; Amr, A.M.; Sayed, M.O.; Hameed, M.F.O.; Obayya, S.S.A. Wideband Ultrathin and Polarization Insensitive Metamaterial Absorber for Ku-Band Applications. J. Mater. Sci. Mater. Electron. 2023, 34, 1797. [Google Scholar] [CrossRef]
- Tan, T.; Yan, Z.; Zou, H.; Ma, K.; Liu, F.; Zhao, L.; Peng, Z.; Zhang, W. Renewable Energy Harvesting and Absorbing via Multi-Scale Metamaterial Systems for Internet of Things. Appl. Energy 2019, 254, 113717. [Google Scholar] [CrossRef]
- Wang, C. Design, Calculation, and Measurement of a Single Resonant Mode Metamaterial Absorber and Its Tunable Performance. Opt. Mater. 2024, 148, 114810. [Google Scholar] [CrossRef]
- Liu, Y.; Ma, W.-Z.; Wu, Y.-C.; Meng, D.; Cheng, Y.-Y.; Chen, Y.-S.; Liu, J.; Gu, Y. Multi-Peak Narrow-Band Metamaterial Absorber for Visible to near-Infrared Wavelengths. Results Phys. 2023, 47, 106374. [Google Scholar] [CrossRef]
- Zheng, H.; Pham, T.S.; Chen, L.; Lee, Y. Metamaterial Perfect Absorbers for Controlling Bandwidth: Single-Peak/Multiple-Peaks/Tailored-Band/Broadband. Crystals 2024, 14, 19. [Google Scholar] [CrossRef]
- Ullah, N.; Islam, M.S.; Hoque, A.; Yong, W.H.; Alrashdi, A.M.; Soliman, M.S.; Islam, M.T. An Efficient, Compact, Wide-Angle, Wide-Band, and Polarization-Insensitive Metamaterial Electromagnetic Energy Harvester. Alex. Eng. J. 2023, 82, 377–388. [Google Scholar] [CrossRef]
- Zhang, C.; Yin, S.; Long, C.; Dong, B.W.; He, D.; Cheng, Q. Hybrid Metamaterial Absorber for Ultra-Low and Dual-Broadband Absorption. Opt. Express OE 2021, 29, 14078–14086. [Google Scholar] [CrossRef]
- Liang, C.; Kong, X.; Wang, F.; Xu, R.; Fu, Y.; Pang, X.; Zhang, S.; Shen, X.; Zhao, L. A Broadband Perfect Metamaterial Absorber with Angle-Insensitive Characteristics. J. Electromagn. Waves Appl. 2023, 37, 401–410. [Google Scholar] [CrossRef]
- Feng, S.; Yang, L.; Cai, B.; Yang, W.; Wu, L.; Cheng, Y.; Chen, F.; Luo, H.; Li, X. Tri-Band Terahertz Metamaterial Absorber Based on Structural Ti3C2Tx MXene for Enhanced Sensing Application. IEEE Sens. J. 2024, 24, 28889–28896. [Google Scholar] [CrossRef]
- You, X.; Upadhyay, A.; Cheng, Y.; Bhaskaran, M.; Sriram, S.; Fumeaux, C.; Withayachumnankul, W. Ultra-Wideband Far-Infrared Absorber Based on Anisotropically Etched Doped Silicon. Opt. Lett. OL 2020, 45, 1196–1199. [Google Scholar] [CrossRef]
- Shen, Z.; Huang, D.; Lin, X. Dual-Band Chirality-Selective Absorbing by Plasmonic Metasurfaces with Breaking Mirror and Rotational Symmetry. Opt. Express OE 2023, 31, 35730–35741. [Google Scholar] [CrossRef]
- Pendry, J.B.; Martín-Moreno, L.; Garcia-Vidal, F.J. Mimicking Surface Plasmons with Structured Surfaces. Science 2004, 305, 847–848. [Google Scholar] [CrossRef] [PubMed]
- Pham, T.S.; Zheng, H.; Chen, L.; Khuyen, B.X.; Lee, Y. Wide-Incident-Angle, Polarization-Independent Broadband-Absorption Metastructure without External Resistive Elements by Using a Trapezoidal Structure. Sci. Rep. 2024, 14, 10198. [Google Scholar] [CrossRef] [PubMed]
- Zhu, R.; Wang, J.; Sui, S.; Meng, Y.; Qiu, T.; Jia, Y.; Wang, X.; Han, Y.; Feng, M.; Zheng, L.; et al. Wideband Absorbing Plasmonic Structures via Profile Optimization Based on Genetic Algorithm. Front. Phys. 2020, 8, 231. [Google Scholar] [CrossRef]
- Garcia-Vidal, F.J.; Fernández-Domínguez, A.I.; Martin-Moreno, L.; Zhang, H.C.; Tang, W.; Peng, R.; Cui, T.J. Spoof Surface Plasmon Photonics. Rev. Mod. Phys. 2022, 94, 025004. [Google Scholar] [CrossRef]
- Tang, W.X.; Zhang, H.C.; Ma, H.F.; Jiang, W.X.; Cui, T.J. Concept, Theory, Design, and Applications of Spoof Surface Plasmon Polaritons at Microwave Frequencies. Adv. Opt. Mater. 2019, 7, 1800421. [Google Scholar] [CrossRef]
- Cheng, Z.W.; Wang, M.; You, Z.H.; Ma, H.F.; Cui, T.J. Spoof Surface Plasmonics: Principle, Design, and Applications. J. Phys. Condens. Matter 2022, 34, 263002. [Google Scholar] [CrossRef]
- Gu, Z.; Ma, Q.; Gao, X.; You, J.W.; Cui, T.J. Direct Electromagnetic Information Processing with Planar Diffractive Neural Network. Sci. Adv. 2024, 10, eado3937. [Google Scholar] [CrossRef]
- Gao, X.; Gu, Z.; Ma, Q.; Chen, B.J.; Shum, K.-M.; Cui, W.Y.; You, J.W.; Cui, T.J.; Chan, C.H. Terahertz Spoof Plasmonic Neural Network for Diffractive Information Recognition and Processing. Nat. Commun. 2024, 15, 6686. [Google Scholar] [CrossRef]
- Jidi, L.; Cao, X.; Gao, J.; Li, T.; Yang, H.; Li, S. Ultra-Wideband Absorber for Electromagnetic Waves under Large Incident Angle Based on Spoof Surface Plasmon Polaritons. Opt. Mater. Express OME 2021, 11, 3917–3929. [Google Scholar] [CrossRef]
- Bai, J.; Yang, Q.; Liang, Y.; Gao, X. Broadband Frequency Selective Rasorber Based on Spoof Surface Plasmon Polaritons. Micromachines 2022, 13, 1969. [Google Scholar] [CrossRef]
- Jiang, W.; Ma, H.; Wang, J.; Yang, J.; Yan, L.; Fan, Y.; Qu, S. Spoof Surface Plasmon Polaritons Realized by Unidirectional Carbon Fibers Arrays and Applications in Structure/Function Integrated Sandwich Structure. Results Phys. 2020, 17, 103081. [Google Scholar] [CrossRef]
- Zhou, S.; Liang, X.; Xing, J.; Fan, Y.; Zhang, L.; Li, D.; Li, E.-P. Ultra-Broadband Metamaterial Absorbers Based on Spoof Surface Plasmon Polaritons Structure. Microw. Opt. Technol. Lett. 2022, 64, 489–495. [Google Scholar] [CrossRef]
- Xu, J.; Zhao, Z.; Yu, H.; Yang, L.; Gou, P.; Cao, J.; Zou, Y.; Qian, J.; Shi, T.; Ren, Q.; et al. Design of Triple-Band Metamaterial Absorbers with Refractive Index Sensitivity at Infrared Frequencies. Opt. Express OE 2016, 24, 25742–25751. [Google Scholar] [CrossRef]
- CST Studio Suite 3D EM Simulation and Analysis Software. Available online: https://www.3ds.com/products-services/simulia/products/cst-studio-suite/ (accessed on 10 October 2023).
- Chen, H.; Yang, X.; Wu, S.; Zhang, D.; Xiao, H.; Huang, K.; Zhu, Z.; Yuan, J. Flexible and Conformable Broadband Metamaterial Absorber with Wide-Angle and Polarization Stability for Radar Application. Mater. Res. Express 2018, 5, 015804. [Google Scholar] [CrossRef]
- Jeong, H.; Lim, S. Broadband Frequency-Reconfigurable Metamaterial Absorber Using Switchable Ground Plane. Sci. Rep. 2018, 8, 9226. [Google Scholar] [CrossRef]
- Yoo, Y.J.; Kim, Y.J.; Hwang, J.S.; Rhee, J.Y.; Kim, K.W.; Kim, Y.H.; Cheong, H.; Chen, L.Y.; Lee, Y.P. Triple-Band Perfect Metamaterial Absorption, Based on Single Cut-Wire Bar. Appl. Phys. Lett. 2015, 106, 071105. [Google Scholar] [CrossRef]
- Sandeep, D.R.; Sivanvitha, G.V. Design of Low-Cost Compact FR-4 Microstrip Patch Antenna for 5G, ISM, and X-Band Applications. In Proceedings of the 2024 IEEE Wireless Antenna and Microwave Symposium (WAMS), Visakhapatnam, India, 29 February–3 March 2024; pp. 1–5. [Google Scholar]
- Li, S.; Gao, J.; Cao, X.; Li, W.; Zhang, Z.; Zhang, D. Wideband, Thin, and Polarization-Insensitive Perfect Absorber Based the Double Octagonal Rings Metamaterials and Lumped Resistances. J. Appl. Phys. 2014, 116, 043710. [Google Scholar] [CrossRef]
- Li, S.-J.; Cao, X.-Y.; Gao, J.; Liu, T.; Zheng, Y.-J.; Zhang, Z. Analysis and Design of Three-Layer Perfect Metamaterial-Inspired Absorber Based on Double Split-Serration-Rings Structure. IEEE Trans. Antennas Propag. 2015, 63, 5155–5160. [Google Scholar] [CrossRef]
- Nguyen, T.Q.H.; Nguyen, T.K.T.; Cao, T.N.; Nguyen, H.; Bach, L.G. Numerical Study of a Broadband Metamaterial Absorber Using a Single Split Circle Ring and Lumped Resistors for X-Band Applications. AIP Adv. 2020, 10, 035326. [Google Scholar] [CrossRef]
- Shukoor, M.A.; Dey, S. A Novel Modified Circular Ring-Based Broadband Polarization-Insensitive Angular Stable Circuit Analog Absorber (CAA) for RCS Applications. Int. J. Microw. Wirel. Technol. 2023, 15, 440–453. [Google Scholar] [CrossRef]
- Sambhav, S.; Ghosh, J.; Singh, A.K. Ultra-Wideband Polarization Insensitive Thin Absorber Based on Resistive Concentric Circular Rings. IEEE Trans. Electromagn. Compat. 2021, 63, 1333–1340. [Google Scholar] [CrossRef]
- Zheng, Y.; Chen, K.; Jiang, T.; Zhao, J.; Feng, Y. Multi-Octave Microwave Absorption via Conformal Metamaterial Absorber with Optical Transparency. J. Phys. D Appl. Phys. 2019, 52, 335101. [Google Scholar] [CrossRef]
- Hakim, M.L.; Alam, T.; Soliman, M.S.; Sahar, N.M.; Baharuddin, M.H.; Almalki, S.H.A.; Islam, M.T. Polarization Insensitive Symmetrical Structured Double Negative (DNG) Metamaterial Absorber for Ku-Band Sensing Applications. Sci. Rep. 2022, 12, 479. [Google Scholar] [CrossRef]
- Hannan, S.; Islam, M.T.; Almutairi, A.F.; Faruque, M.R.I. Wide Bandwidth Angle- and Polarization-Insensitive Symmetric Metamaterial Absorber for X and Ku Band Applications. Sci. Rep. 2020, 10, 10338. [Google Scholar] [CrossRef]

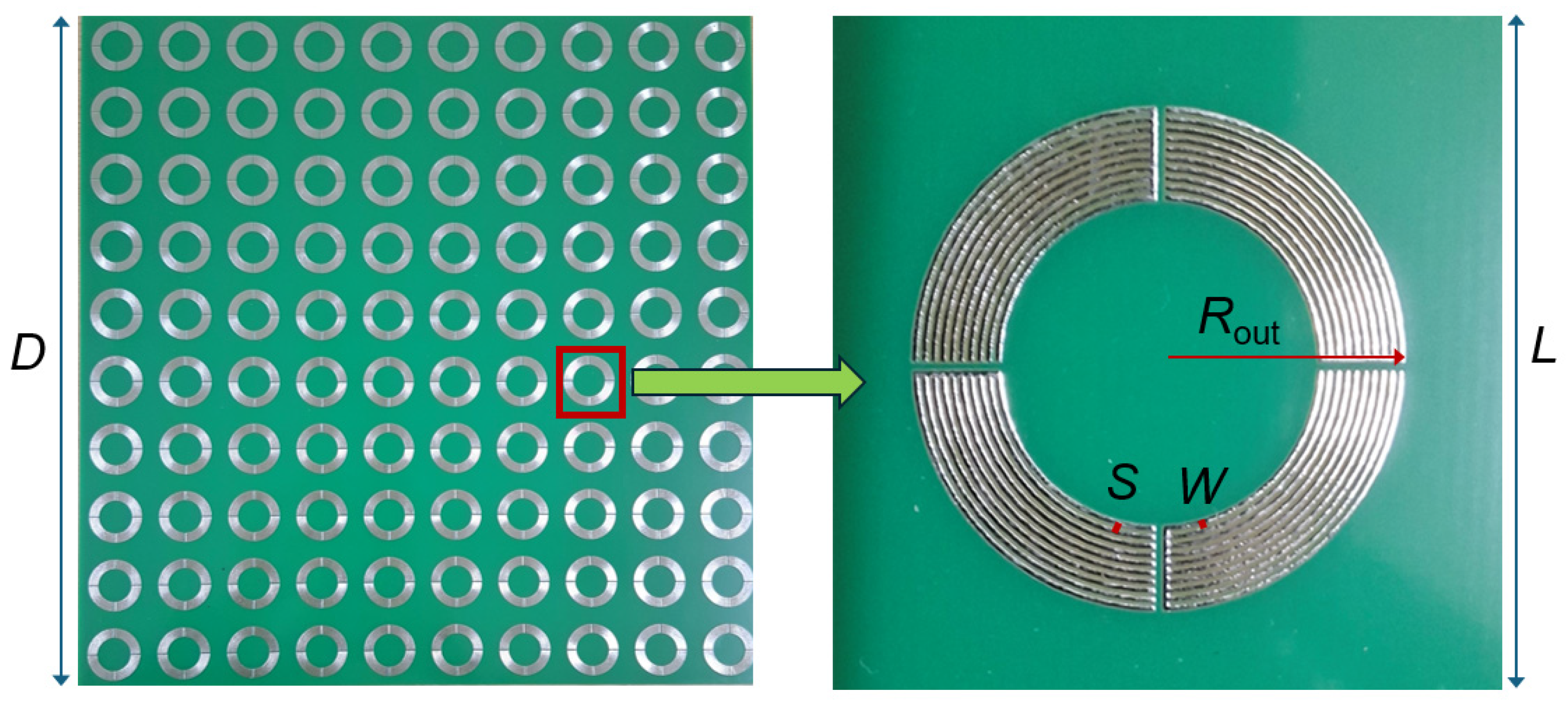
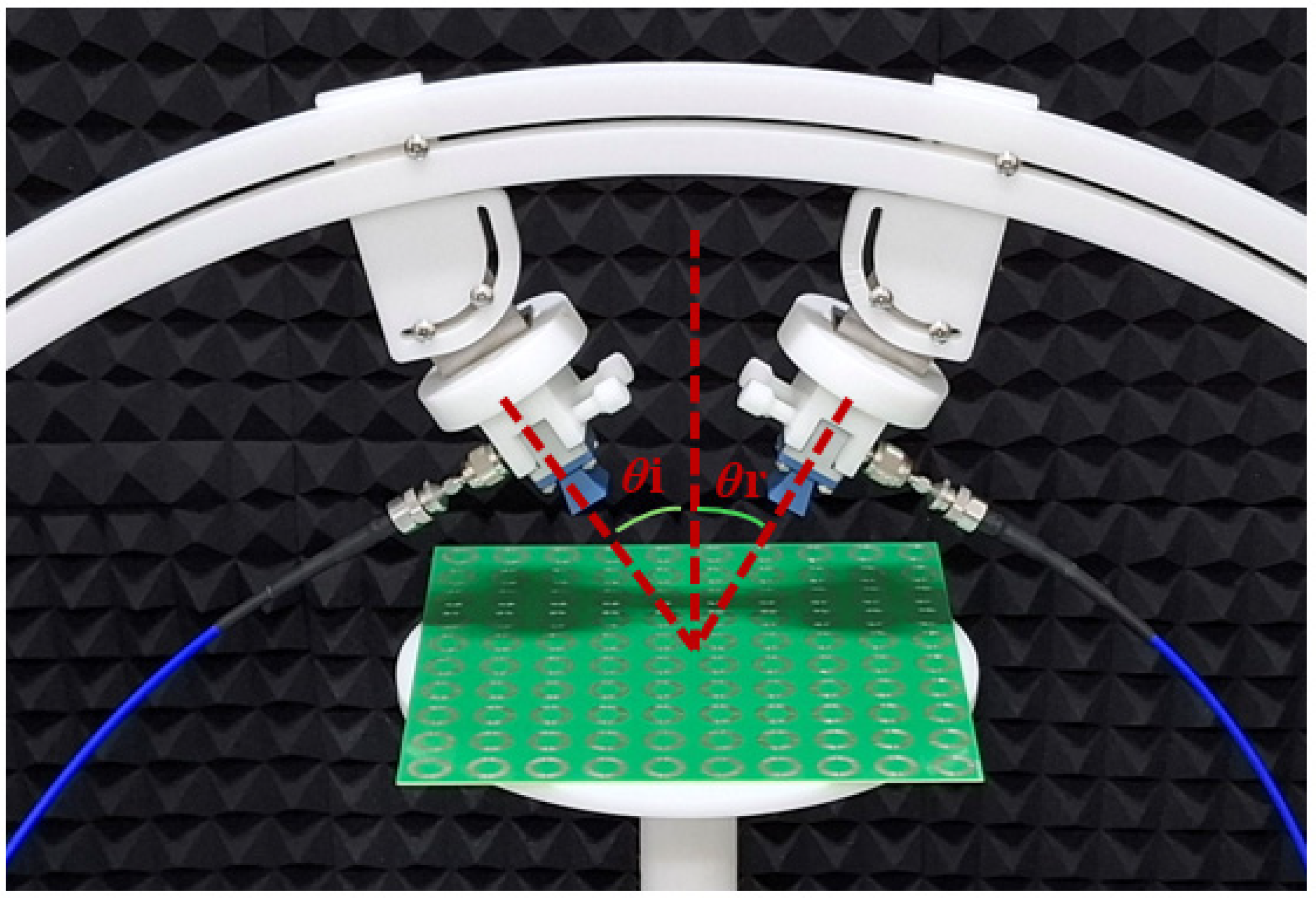
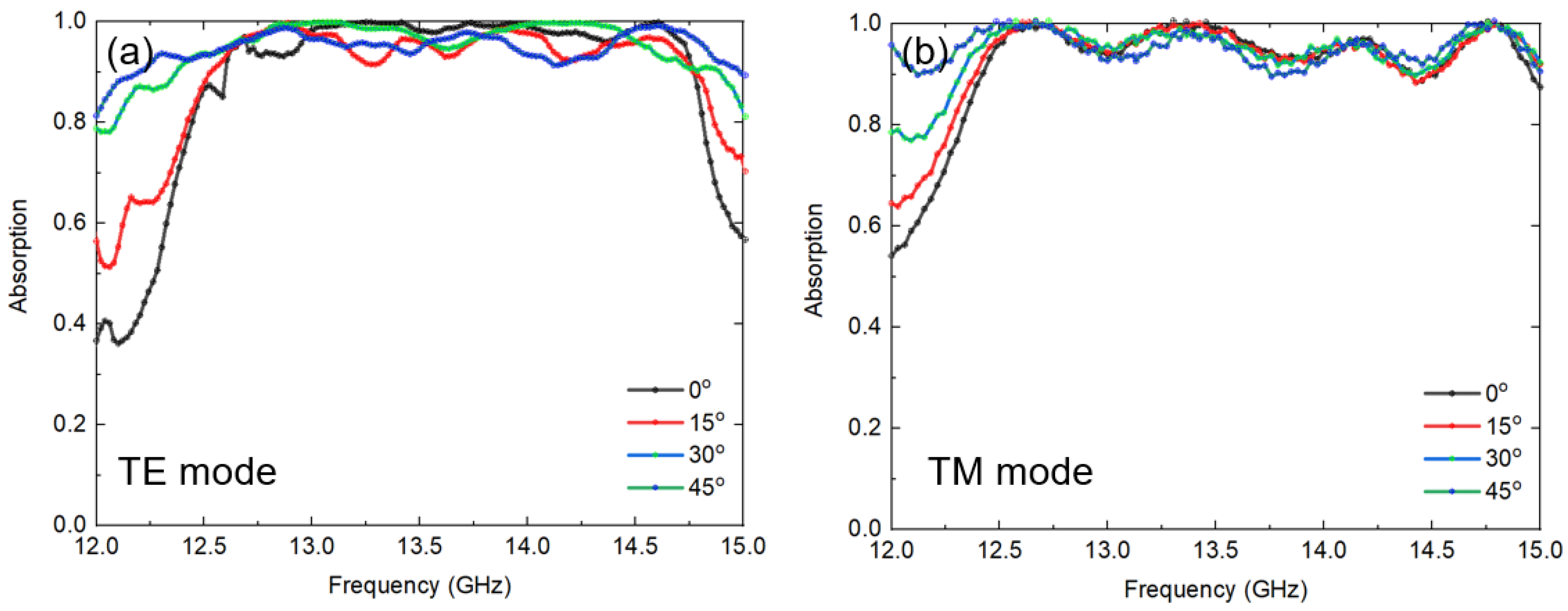
| Structure Parameters | Value |
|---|---|
| Unit-cell periodicity (L) | 18.7 mm |
| Dielectric thickness (td) | 3.2 mm |
| Dielectric constant (ε) | 4.3 |
| Loss tangent (tan δ) | 0.025 |
| Metal thickness (tm) | 0.035 mm |
| Width of metal trace (W) | 0.1 mm |
| Spacing between turns (S) | 0.1 mm |
| Outer radius (Rout) | 7 mm |
| Number of rings (n) | 10 |
| Total size of plasmonic metasurface | 187 × 187 mm |
| Ref. | Bandwidth (GHz) | Relative Absorption Bandwidth | Absorption | Lumped Elements/Multilayer | Polarization (Both TE and TM?) | Incident Angle |
|---|---|---|---|---|---|---|
| [39] | 7.93–17.18 | 73.67% | >90% | Yes/No | Both | 0–20° |
| [40] | 5.46–14.75 | 91.93% | >90% | No/Yes | Both | 0–30° |
| [41] | 6.80–11.80 | 53.76% | >70% | Yes/No | Both | 0–30° |
| [43] | 6.70–20.58 | 101.75% | >90% | Yes/Yes | Both | 0–30° |
| [44] | 6.21–19.31 | 102.66% | >90% | No/Yes | Both | 0° |
| [45] | Peaks at 14.50, 16.50 | n/a | >90% | No/No | Both | 0, 45°, 90° |
| [46] | 11.21–11.49 | 2.46% | >90% | No/No | Both | 0–45° |
| 13.92–14.85 | 6.46% | |||||
| 17.66–17.87 | 1.18% | |||||
| This paper | 12.30–14.80 | 18.45% | >90% | No/No | Both | 0–45° |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pham, T.S.; Khuyen, B.X.; Lam, V.D.; Chen, L.; Lee, Y. Wide-Angle, Polarization-Independent Broadband Metamaterial Absorber by Using Plasmonic Metasurface-Based Split-Circular Structure. Photonics 2025, 12, 334. https://doi.org/10.3390/photonics12040334
Pham TS, Khuyen BX, Lam VD, Chen L, Lee Y. Wide-Angle, Polarization-Independent Broadband Metamaterial Absorber by Using Plasmonic Metasurface-Based Split-Circular Structure. Photonics. 2025; 12(4):334. https://doi.org/10.3390/photonics12040334
Chicago/Turabian StylePham, Thanh Son, Bui Xuan Khuyen, Vu Dinh Lam, Liangyao Chen, and Youngpak Lee. 2025. "Wide-Angle, Polarization-Independent Broadband Metamaterial Absorber by Using Plasmonic Metasurface-Based Split-Circular Structure" Photonics 12, no. 4: 334. https://doi.org/10.3390/photonics12040334
APA StylePham, T. S., Khuyen, B. X., Lam, V. D., Chen, L., & Lee, Y. (2025). Wide-Angle, Polarization-Independent Broadband Metamaterial Absorber by Using Plasmonic Metasurface-Based Split-Circular Structure. Photonics, 12(4), 334. https://doi.org/10.3390/photonics12040334








