Wavefront Characterization of an Optical Parametric Oscillator as a Function of Wavelength
Abstract
:1. Introduction
2. Methods
2.1. Experimental System
2.2. Wavefront Aberration and Zernike Polynomials
3. Results
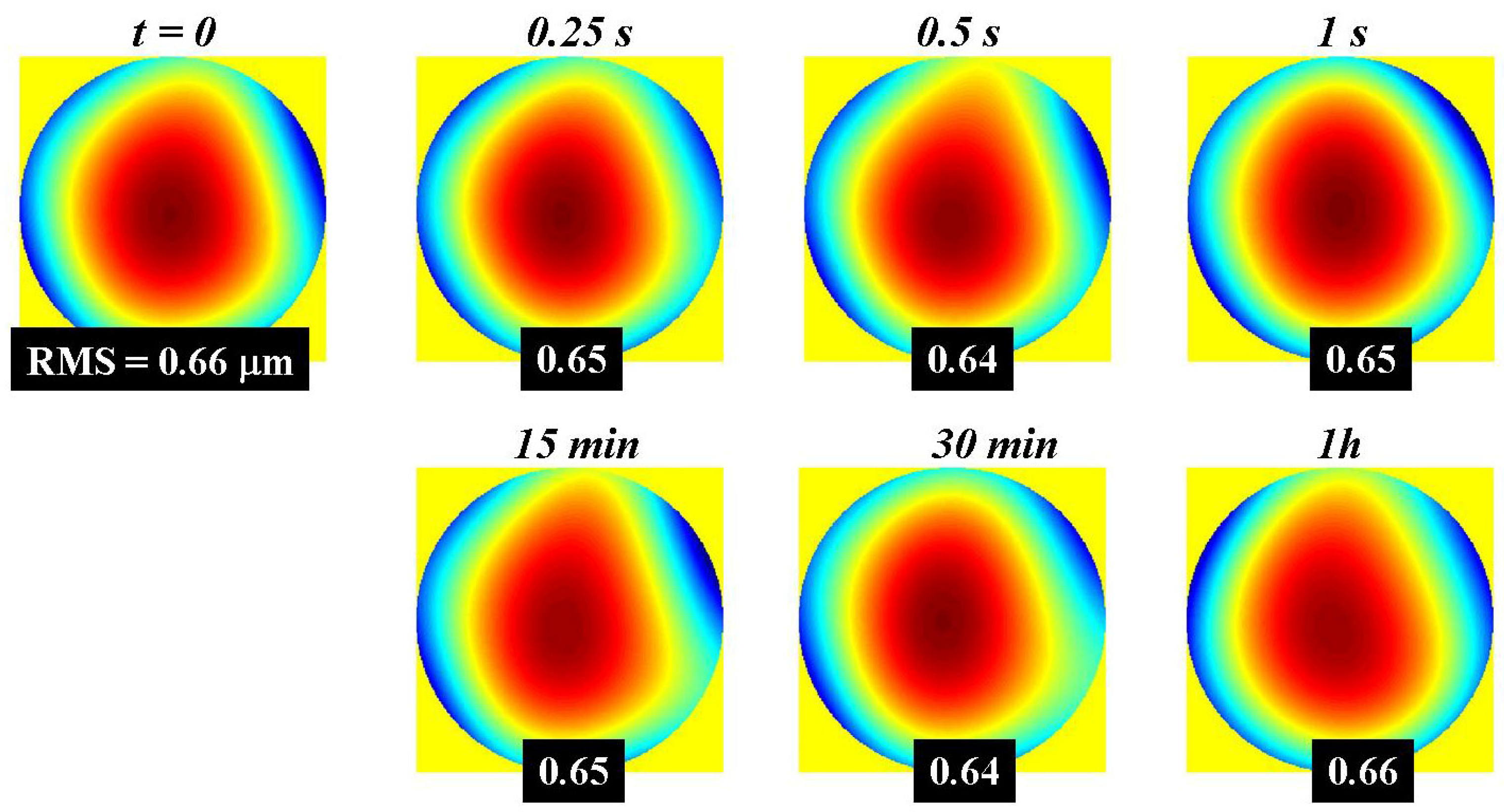
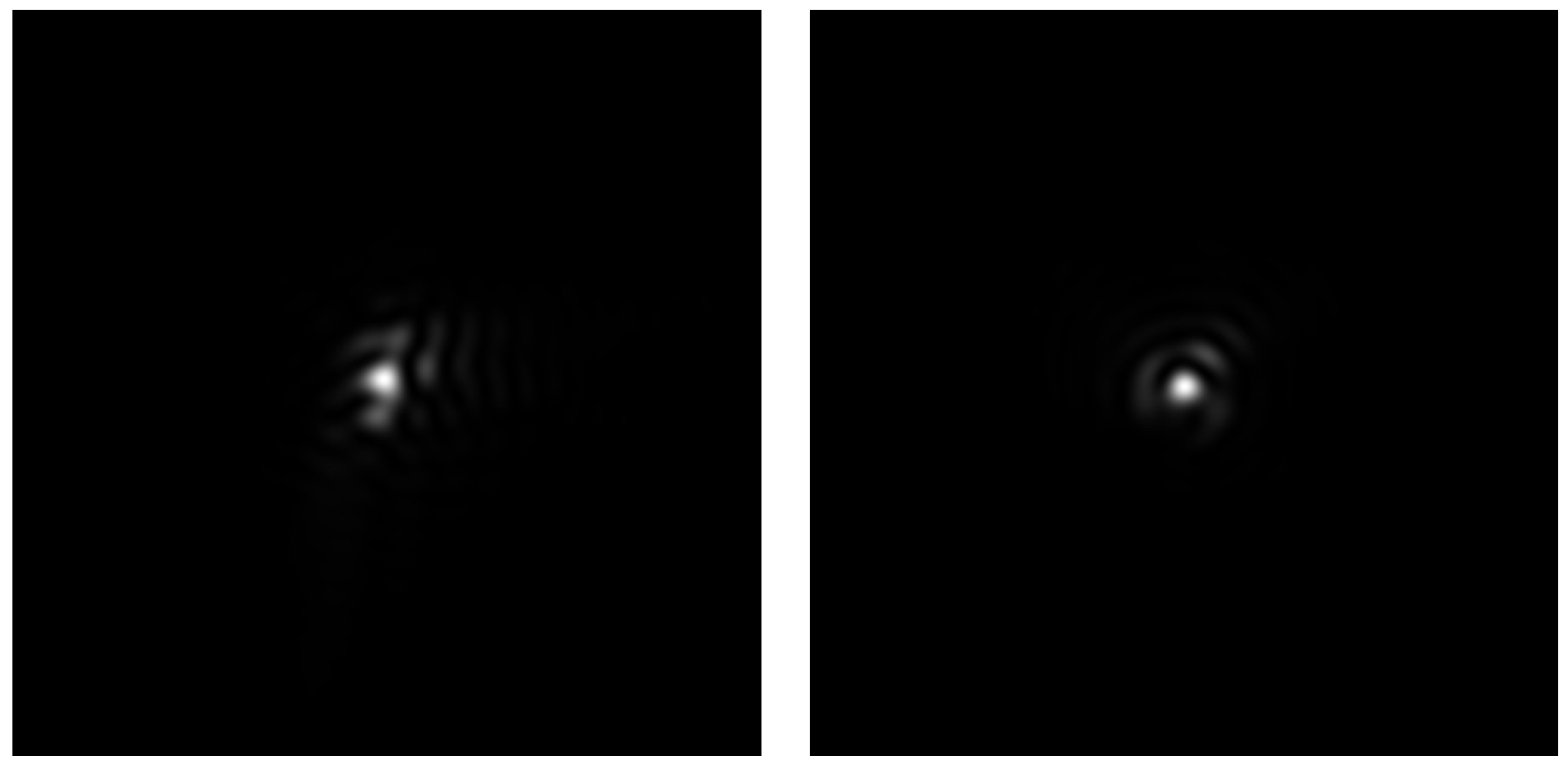
3.1. Wavefront Temporal Stability
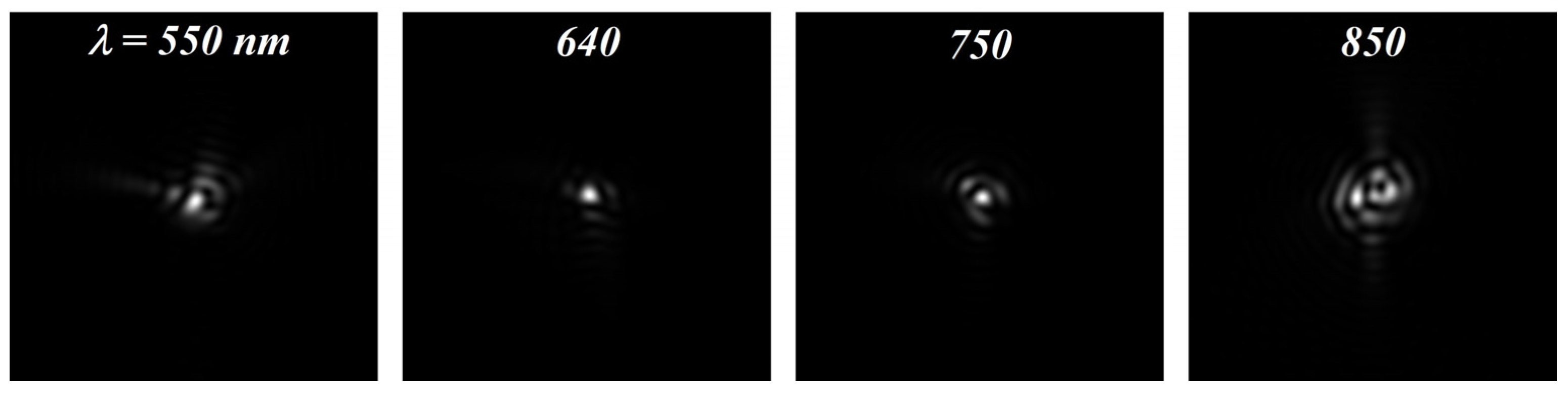
3.2. Wavefront vs. Wavelength
4. Discussion
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Helmchen, F.; Denk, W. Deep tissue two-photon microscopy. Nat. Methods 2005, 2, 932–939. [Google Scholar] [CrossRef] [PubMed]
- Campagnola, P.J.; Loew, L.M. Second-harmonic imaging microscopy for visualizing biomolecular arrays in cells, tissues, and organisms. Nat. Biotechnol. 2003, 21, 1356–1360. [Google Scholar] [CrossRef]
- Squier, J.A.; Müller, M.; Brakenhoff, G.J.; Wilson, K.R. Third-harmonic generation microscopy. Opt. Express 1998, 3, 315–324. [Google Scholar] [CrossRef] [PubMed]
- Zipfel, W.R.; Williams, R.M.; Webb, W.W. Nonlinear magic: Multiphoton microscopy in the biosciences. Nat. Biotechnol. 2003, 21, 1369–1377. [Google Scholar] [CrossRef]
- Helmchen, F. Two-photon functional imaging of neuronal activity. In In Vivo Optical Imaging of Brain Function, 2nd ed.; Frostig, R.D., Ed.; CRC Press/Taylor & Francis: Boca Raton, FL, USA, 2009; Chapter 2. [Google Scholar]
- Mohanty, S.K.; Lakshminarayananan, V. Optical techniques in optogenetics. J. Mod. Opt. 2015, 62, 949–970. [Google Scholar] [CrossRef]
- Dzhagalov, I.L.; Melichar, H.J.; Ross, J.O.; Herzmark, P.; Robey, E.A. Two-photon imaging of the immune system. Curr. Protoc. Cytom. 2012, 60, 12–26. [Google Scholar] [CrossRef]
- Hao, Q.; Song, Y.; Cao, J.; Liu, H.; Liu, Q.; Li, J.; Luo, Q.; Cheng, Y.; Cui, H.; Liu, L. The development of snapshot multispectral imaging technology based on artificial compound eyes. Electronics 2023, 12, 812. [Google Scholar] [CrossRef]
- Hwang, S.; Shin, H.K.; Park, J.M.; Kwon, B.; Kang, M.-G. Classification of dog skin diseases using deep learning with images captured from multispectral imaging device. Mol. Cell Toxicol. 2022, 18, 299–309. [Google Scholar] [CrossRef]
- Lin, S.-J.; Wu, R.; Tan, H.-Y.; Lo, W.; Lin, W.-C.; Young, T.-H.; Hsu, C.-J.; Chen, J.-S.; Jee, S.-H.; Dong, C.-Y. Evaluating cutaneous photoaging by use of multiphoton fluorescence and second harmonic. Opt. Lett. 2005, 30, 2275–2277. [Google Scholar] [CrossRef]
- Bueno, J.M.; Martínez-Ojeda, R.M.; Pérez-Zabalza, M.; García-Mendívil, L.; Asensio, M.C.; Ordovás, L.; Pueyo, E. Analysis of age-related changes in the left ventricular myocardium with multiphoton microscopy. Biomed. Opt. Express 2024, 15, 3251–3264. [Google Scholar] [CrossRef]
- Berezin, M.Y.; Achilefu, S. Fluorescence lifetime measurements and biological imaging. Chem. Rev. 2010, 110, 2641–2684. [Google Scholar] [CrossRef] [PubMed]
- Walters, S.; Feeks, J.A.; Huynh, K.T.; Hunter, J.J. Adaptive optics two-photon excited fluorescence lifetime imaging ophthalmoscopy of photoreceptors and retinal pigment epithelium in the living non-human primate eye. Biomed. Opt. Express 2022, 13, 389–407. [Google Scholar] [CrossRef] [PubMed]
- Ding, L.; Knox, W.H.; Bühren, J.; Nagy, L.J.; Huxlin, K.R. Intratissue refractive index shaping (IRIS) of the cornea and lens using a low-pulse-energy femtosecond laser oscillator. Investig. Ophthalmol. Vis. Sci. 2008, 49, 5332–5339. [Google Scholar] [CrossRef]
- Xu, L.; Knox, W.H.; DeMagistris, M.; Wang, N.; Huxlin, K.R. Noninvasive intratissue refractive index shaping (IRIS) of the cornea with blue femtosecond laser light. Investig. Ophthalmol. Vis. Sci. 2011, 52, 8148–8155. [Google Scholar] [CrossRef]
- Gualda, E.J.; de Aldana, J.R.V.; Martínez-García, M.C.; Moreno, P.; Hernández-Toro, J.; Roso, L.; Artal, P.; Bueno, J.M. Femtosecond infrared intrastromal ablation and backscattering-mode adaptive-optics multiphoton microscopy in chicken corneas. Biomed. Opt. Express 2011, 2, 2950–2960. [Google Scholar] [CrossRef]
- Savage, N. Optical parametric oscillators. Nat. Photonics 2010, 4, 124–125. [Google Scholar] [CrossRef]
- Bueno, J.M.; Vohnsen, B.; Roso, L.; Artal, P. Temporal wavefront stability of an ultrafast high-power laser beam. Appl. Opt. 2009, 48, 770–777. [Google Scholar] [CrossRef]
- Thibos, L.N.; Applegate, R.A.; Schwiegerling, J.T.; Webb, R. VSIA Standards Taskforce Members. Vision science and its applications. Standards for reporting the optical aberrations of eyes. J. Refract. Surg. 2002, 18, S652–S660. [Google Scholar] [CrossRef]
- Goodman, J. Introduction to Fourier Optics, 3rd ed.; Roberts and Company Publishers: Greenwood Village, CO, USA, 2004. [Google Scholar]
- Giordmaine, J.A.; Miller, R.C. Tunable coherent parametric oscillation in LiNbO3 at optical frequencies. Phys. Rev. Lett. 1965, 14, 973. [Google Scholar] [CrossRef]
- Akaoka, K.; Harayama, S.; Tei, K.; Maruyama, Y.; Arisawa, T. Close loop wavefront correction of Ti: Sapphire chirped pulse amplification laser beam. In Proceedings of the Solid State Lasers VII, San Jose, CA, USA, 24–30 January 1998; SPIE: St Bellingham, WA, USA, 1998; Volume 3265. [Google Scholar] [CrossRef]
- Fourmaux, S.; Payeur, S.; Alexandrov, A.; Serbanescu, C.; Martin, F.; Ozaki, T.; Kudryashov, A.; Kieffer, J.C. Laser beam wavefront correction for ultra high intensities with the 200 TW laser system at the advanced laser light source. Opt. Express 2008, 16, 11987–11994. [Google Scholar] [CrossRef]
- Hauri, C.P.; Biegert, J.; Keller, U.; Schaefer, B.; Mann, K.; Marowski, G. Validity of wave-front reconstruction and propagation of ultrabroadband pulses measured with a Hartmann-Shack sensor. Opt. Lett. 2005, 30, 1563–1565. [Google Scholar] [CrossRef] [PubMed]
- Schäfer, B.; Mann, K.; Marowsky, G.; Hauri, C.P.; Biegert, J.; Keller, U. Characterization, wavefront reconstruction and propagation of ultra-broadband laser pulses from Hartmann-Shack measurements. In Proceedings of the SPIE 5918, Laser-Generated, Synchrotron, and Other Laboratory X-Ray and EUV Sources, Optics, and Applications II, San Diego, CA, USA, 2–4 August 2005; p. 59180. [Google Scholar] [CrossRef]
- Cousin, S.L.; Bueno, J.M.; Forget, N.; Austin, D.R.; Biegert, J. Three-dimensional spatiotemporal pulse characterization with an acousto-optic pulse shaper and a Hartmann–Shack wavefront sensor. Opt. Lett. 2012, 37, 3291–3293. [Google Scholar] [CrossRef] [PubMed]
- Wu, L.-A.; Xiao, M.; Kimble, H.J. Squeezed states of light from an optical parametric oscillator. J. Opt. Soc. Am. B 1987, 4, 1465–1475. [Google Scholar] [CrossRef]
- Horodecki, R.; Horodecki, P.; Horodecki, M.; Horodecki, K. Quantum entanglement. Rev. Mod. Phys. 2009, 81, 865–942. [Google Scholar] [CrossRef]
- Roy, A.; Ledezma, L.; Costa, L.; Gray, R.; Sekine, R.; Guo, Q.; Liu, M.; Briggs, R.M.; Marandi, A. Visible-to-mid-IR tunable frequency comb in nanophotonics. Nat. Commun. 2023, 14, 6549. [Google Scholar] [CrossRef]
- Campbell, P.; Moore, I.D.; Pearson, M.R. Laser spectroscopy for nuclear structure physics. Prog. Part. Nucl. Phys. 2016, 86, 127–180. [Google Scholar] [CrossRef]
- Holzwarth, R.; Udem, T.; Hänsch, T.W.; Knight, J.C.; Wadsworth, W.J.; Russell, P.S.J. Optical frequency synthesizer for precision spectroscopy. Phys. Rev. Lett. 2000, 85, 2264–2267. [Google Scholar] [CrossRef]
- Picqué, N.; Hänsch, T.W. Frequency comb spectroscopy. Nat. Photonics 2019, 13, 146–157. [Google Scholar] [CrossRef]
- Booth, M.J. Adaptive optical microscopy: The ongoing quest for a perfect image. Light Sci. Appl. 2014, 3, e165. [Google Scholar] [CrossRef]
- Ji, N. Adaptive optical fluorescence microscopy. Nat. Methods 2017, 14, 374–380. [Google Scholar] [CrossRef]
- Rodríguez, C.; Ji, N. Adaptive optical microscopy for neurobiology. Curr. Opin. Neurobiol. 2018, 50, 83–91. [Google Scholar] [CrossRef] [PubMed]
- Bueno, J.M.; Skorsetz, M.; Bonora, S.; Artal, P. Wavefront correction in two-photon microscopy with a multi-actuator adaptive lens. Opt. Express 2018, 26, 14278–14287. [Google Scholar] [CrossRef] [PubMed]
- Yan, W.; Huang, Y.; Wang, L.; Guo, Y.; Li, J.; Zhu, Y.; Yang, Z.; Qu, J. Aberration correction to optimize the performance of two-photon fluorescence microscopy using the genetic algorithm. Microsc. Microanal. 2022, 25, 383–389. [Google Scholar] [CrossRef]
- Kao, F.J. The use of optical parametric oscillator for harmonic generation and two-photon UV fluorescence microscopy. Microsc. Res. Tech. 2004, 63, 175–181. [Google Scholar] [CrossRef] [PubMed]
- Li, D.; Zheng, W.; Qu, J.Y. Two-photon autofluorescence microscopy of multicolor excitation. Opt. Lett. 2009, 34, 202–204. [Google Scholar] [CrossRef] [PubMed]
- Rousset, F.; Ducros, N.; Peyrin, F.; Valentini, G.; D’Andrea, C.; Farina, A. Time-resolved multispectral imaging based on an adaptive single-pixel camera. Opt. Express 2018, 26, 10550–10558. [Google Scholar] [CrossRef]
- Wang, J.; Schuele, G.; Palanker, D. Finesse of transparent tissue cutting by ultrafast lasers at various wavelengths. J. Biomed. Opt. 2015, 20, 125004. [Google Scholar] [CrossRef]
- Yang, Q.X.; Yu, M.H.; Chen, Z.X.; Ai, S.; Kentsch, U.; Zhou, S.; Jia, Y.; Chen, F.; Liu, H. A novel approach towards robust construction of physical colors on lithium niobate crystal. Opto-Electron. Adv. 2025, 8, 240193. [Google Scholar] [CrossRef]
- Niu, P.; Geng, J.; Jiang, Q.; Wang, Y.; Sang, J.; Wang, Z.; Shi, L. Femtosecond laser-induced recrystallized nanotexturing for identity document security with physical unclonable functions. Adv. Sci. 2025, 12, 2411449. [Google Scholar] [CrossRef]
- Varlamov, P.; Marx, J.; Elgueta, Y.U.; Ostendorf, A.; Kim, J.-W.; Vavassori, P.; Temnov, V. Femtosecond laser ablation and delamination of functional magnetic multilayers at the nanoscale. Nanomaterials 2024, 14, 1488. [Google Scholar] [CrossRef]
- Amigó, A.; Martínez-Sorribes, P.; Recuerda, M. Refractive changes induced by spherical aberration in laser correction procedures: An adaptive optics study. J. Refract. Surg. 2017, 33, 470–474. [Google Scholar] [CrossRef] [PubMed]
- Campbell, S.; Triphan, S.M.F.; El-Agmy, R.; Greenaway, A.H.; Reid, D.T. Direct optimization of femtosecond laser ablation using adaptive wavefront shaping. J. Opt. A Pure Appl. Opt. 2007, 9, 1100–1104. [Google Scholar] [CrossRef]
- Schwertner, M.; Booth, M.J.; Wilson, T. Adaptive optics for microscopy, optical data storage, and micromachining. In Proceedings of the SPIE 6306, Advanced Wavefront Control: Methods, Devices, and Applications IV, San Diego, CA, USA, 14–15 August 2006; p. 63060A. [Google Scholar] [CrossRef]
- Parry, J.; Beck, R.J.; Weston, N.; Shephard, J.; Hand, D. Application of adaptive optics to laser micromachining. In ICALEO 2010—Congress Proceedings 103, Proceedings of the 29th International Congress on Applications of Lasers and Electro-Optics, Anaheim, CA, USA, 26–30 September 2010; AIP Publishing: Melville, NY, USA, 2010; pp. 767–776. [Google Scholar] [CrossRef]
- Salter, P.S.; Booth, M.J. Adaptive optics in laser processing. Light Sci. Appl. 2019, 8, 110. [Google Scholar] [CrossRef] [PubMed]
- Shoham, S. Optogenetics meets optical wavefront sensing. Nat. Methods 2010, 7, 798–799. [Google Scholar] [CrossRef]
- Deisseroth, K. Optogenetics. Nat. Methods 2011, 8, 26–29. [Google Scholar] [CrossRef]
- Girkin, J.M.; Buckley, C.B.; Torres-Carvalho, M.; Young, L.; Rider, S.; Mullins, J.J. Adaptive optis for precise cell ablation in vivo. In Computational Optical Sensing and Imaging; Optica Publishing Group: Washington, DC, USA, 2018; p. JTu5B.3. [Google Scholar] [CrossRef]
- Wang, H.; He, H. Optical precise ablation of targeted individual neurons in vivo. ACS Chem. Neurosci. 2025, 16, 374–383. [Google Scholar] [CrossRef] [PubMed]


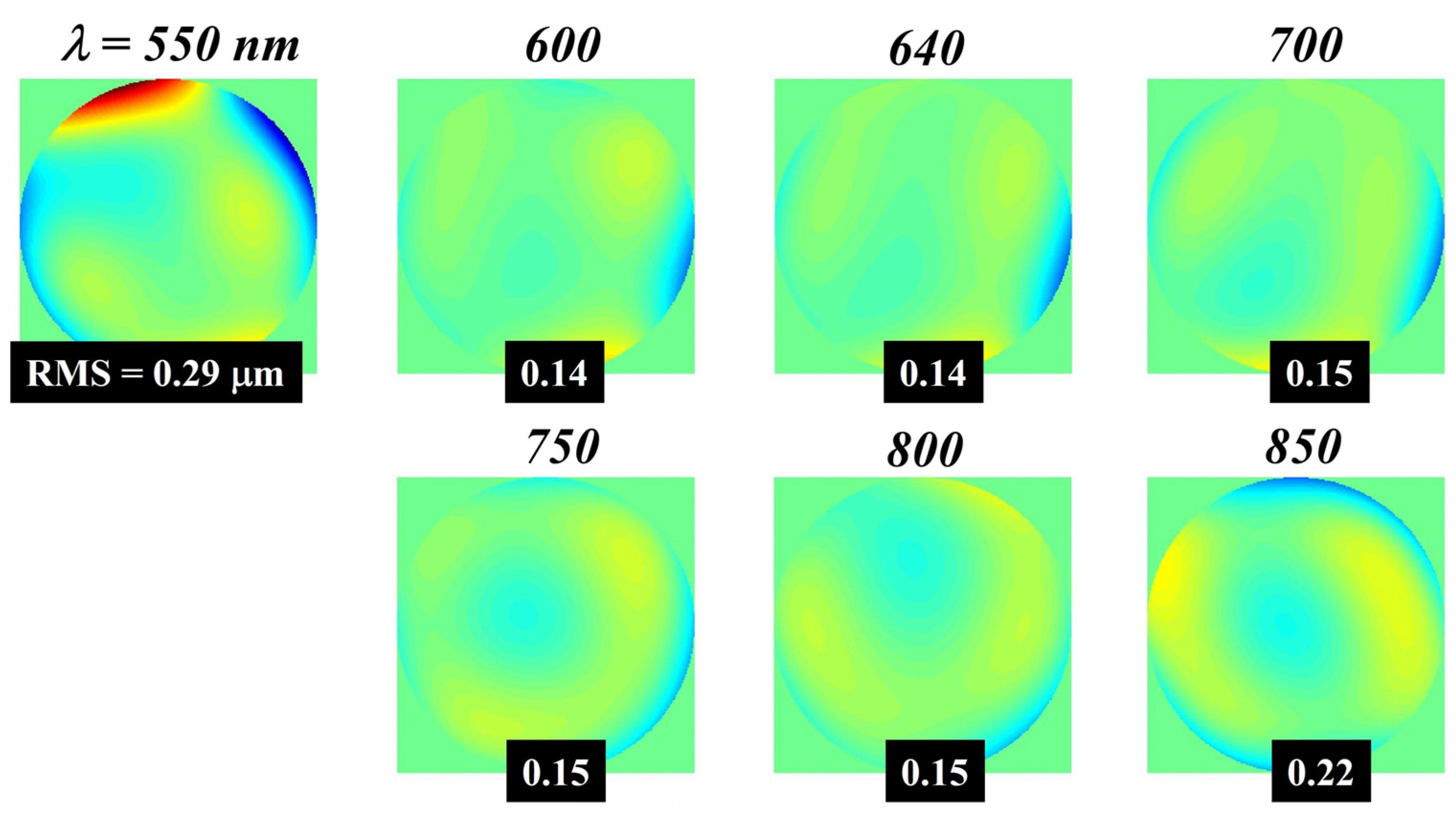
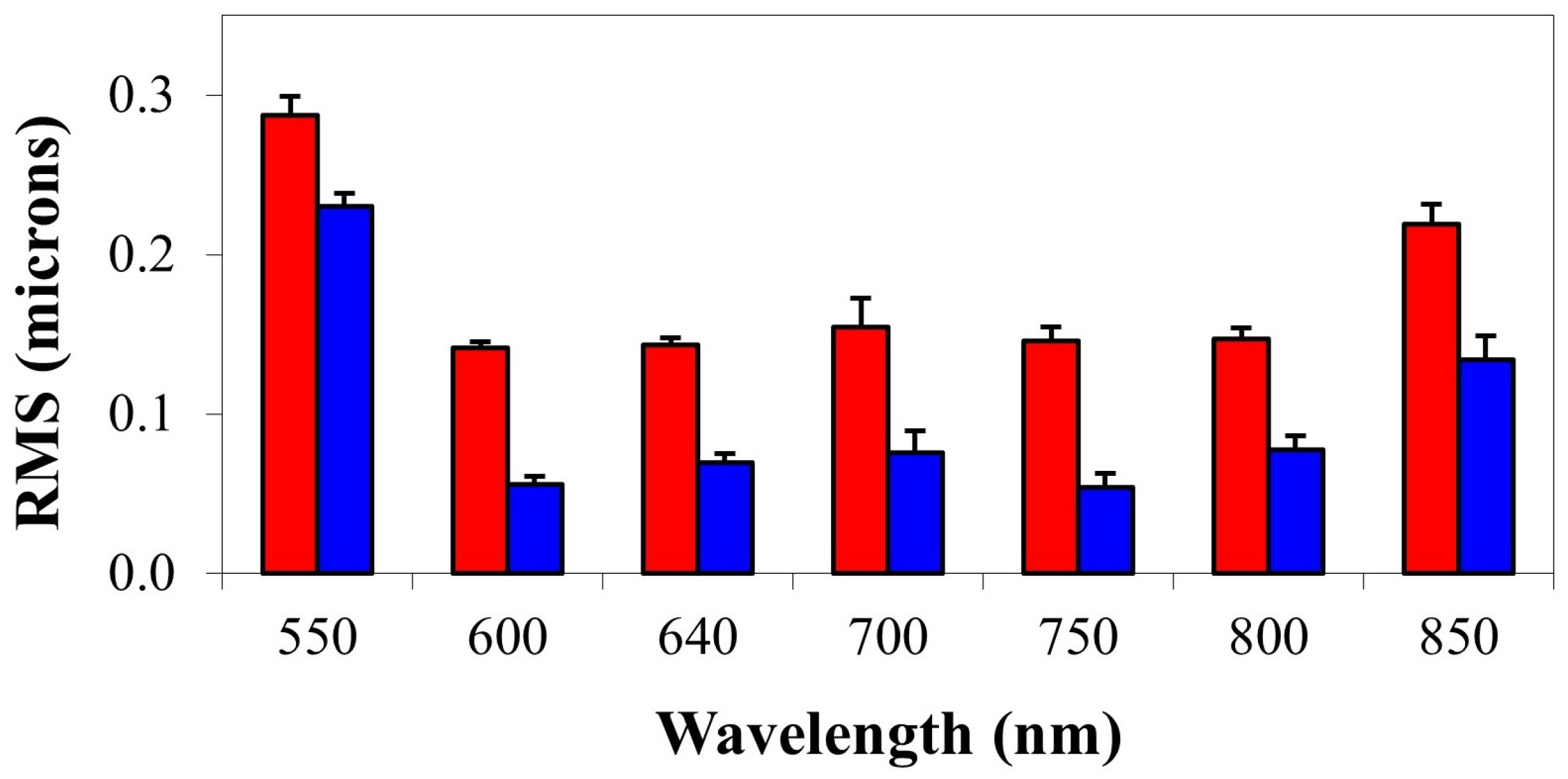
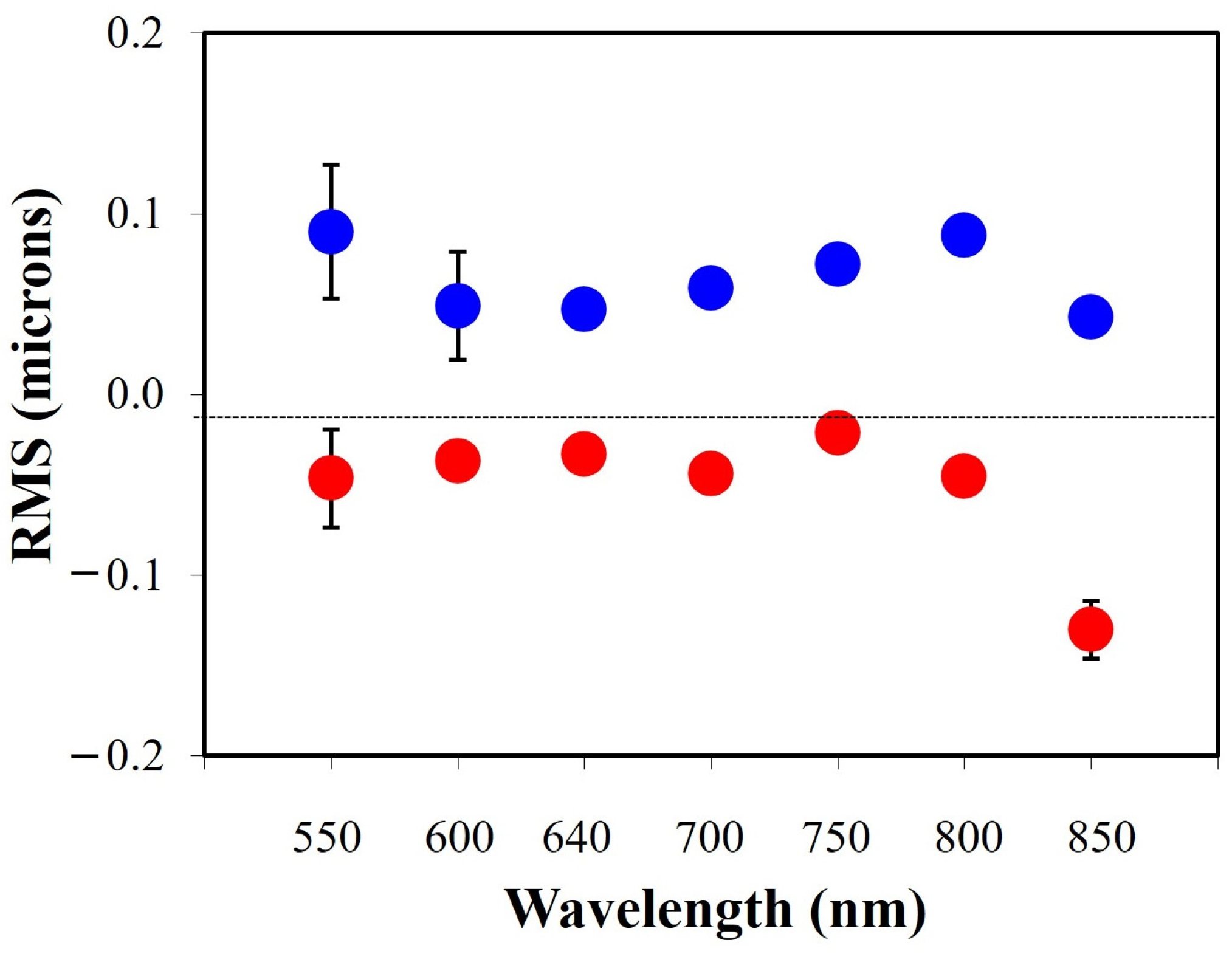
| RMS (μm) | 550 | 600 | 640 | 700 | 750 | 800 | 850 |
|---|---|---|---|---|---|---|---|
| Total (no defocus) | 0.287 | 0.141 | 0.143 | 0.154 | 0.146 | 0.147 | 0.219 |
| Astigmatism | 0.230 | 0.056 | 0.069 | 0.075 | 0.054 | 0.077 | 0.134 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bueno, J.M. Wavefront Characterization of an Optical Parametric Oscillator as a Function of Wavelength. Photonics 2025, 12, 347. https://doi.org/10.3390/photonics12040347
Bueno JM. Wavefront Characterization of an Optical Parametric Oscillator as a Function of Wavelength. Photonics. 2025; 12(4):347. https://doi.org/10.3390/photonics12040347
Chicago/Turabian StyleBueno, Juan M. 2025. "Wavefront Characterization of an Optical Parametric Oscillator as a Function of Wavelength" Photonics 12, no. 4: 347. https://doi.org/10.3390/photonics12040347
APA StyleBueno, J. M. (2025). Wavefront Characterization of an Optical Parametric Oscillator as a Function of Wavelength. Photonics, 12(4), 347. https://doi.org/10.3390/photonics12040347





