High-Spatial-Resolution High-Accuracy OFDR Distributed Sensors Based on Seamless fs-WFBG Array
Abstract
:1. Introduction
2. Experimental Configuration
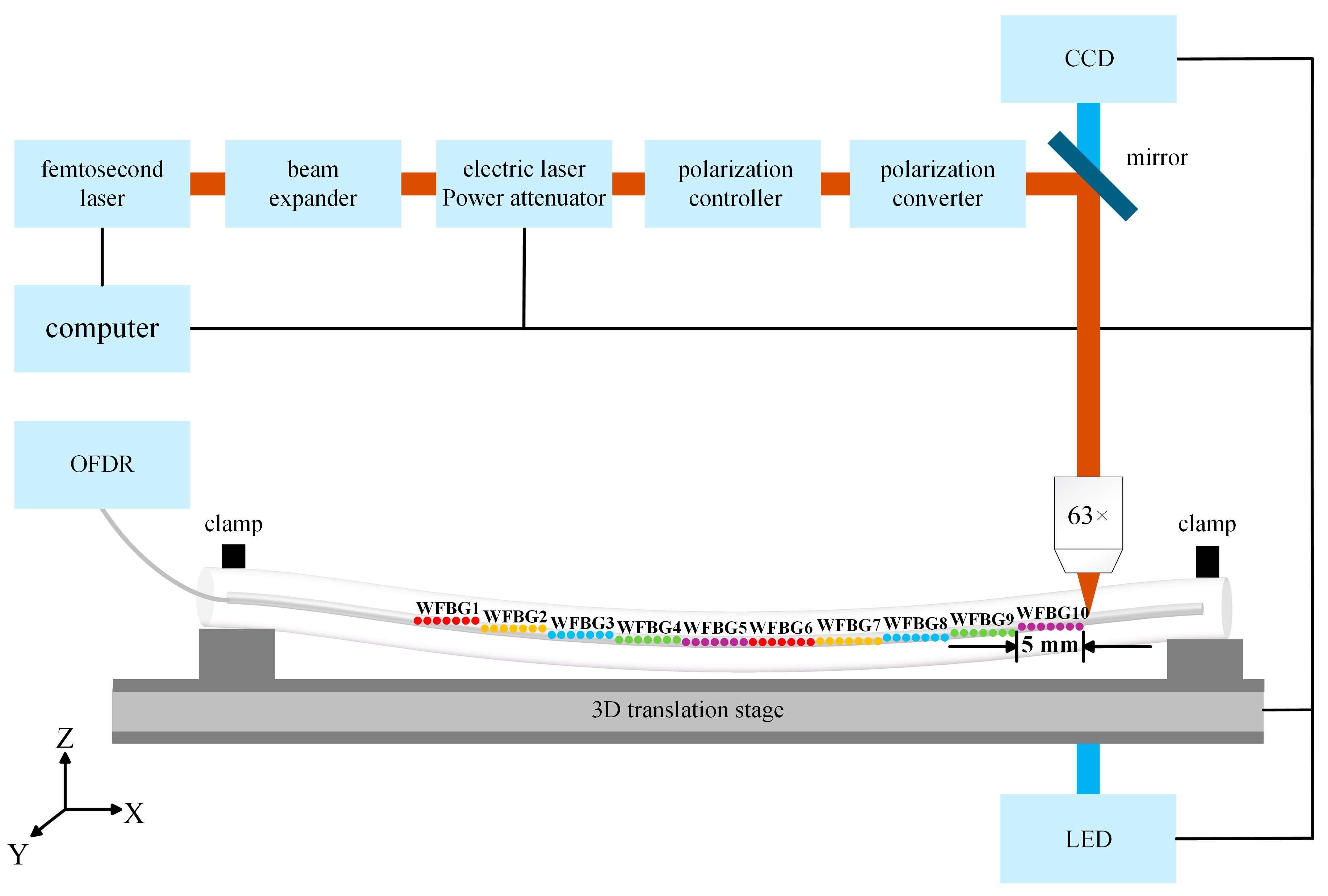
2.1. Experimental Setup for Seamless fs-WFBG Array
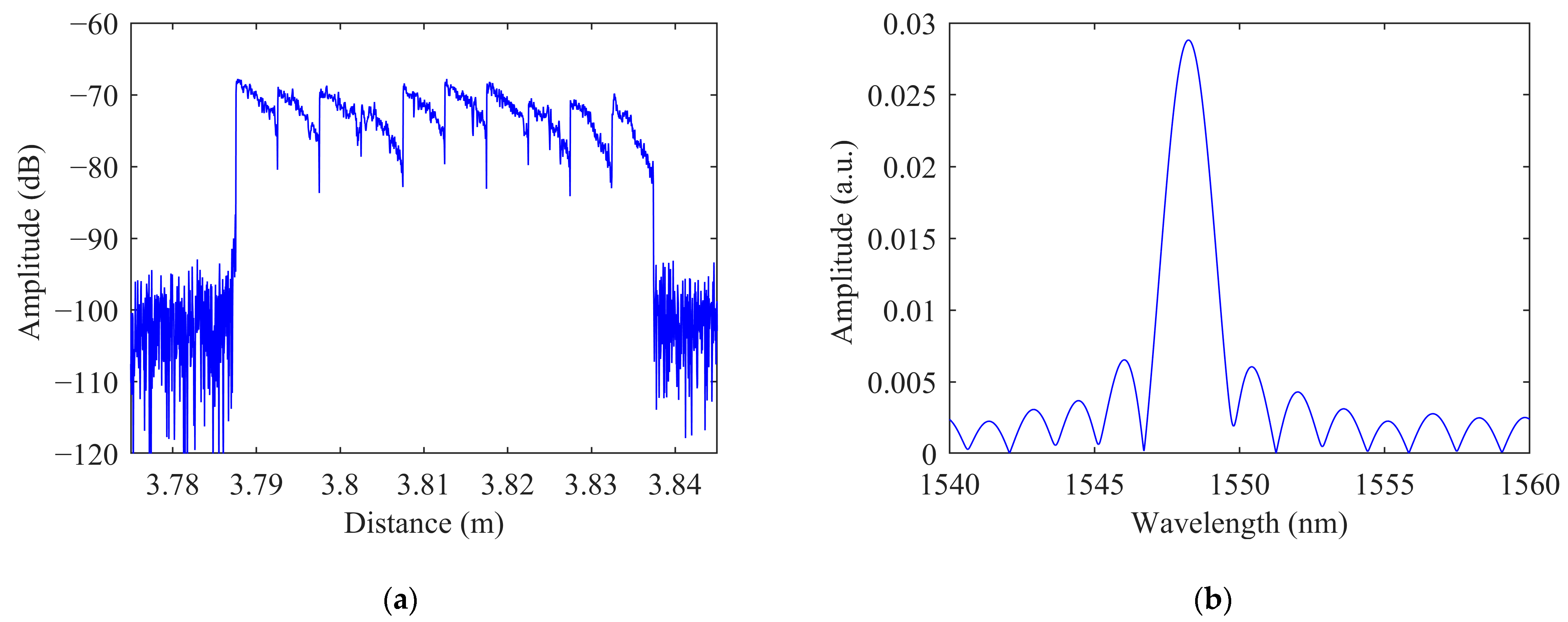
2.2. OFDR-Based Wavelength Demodulation
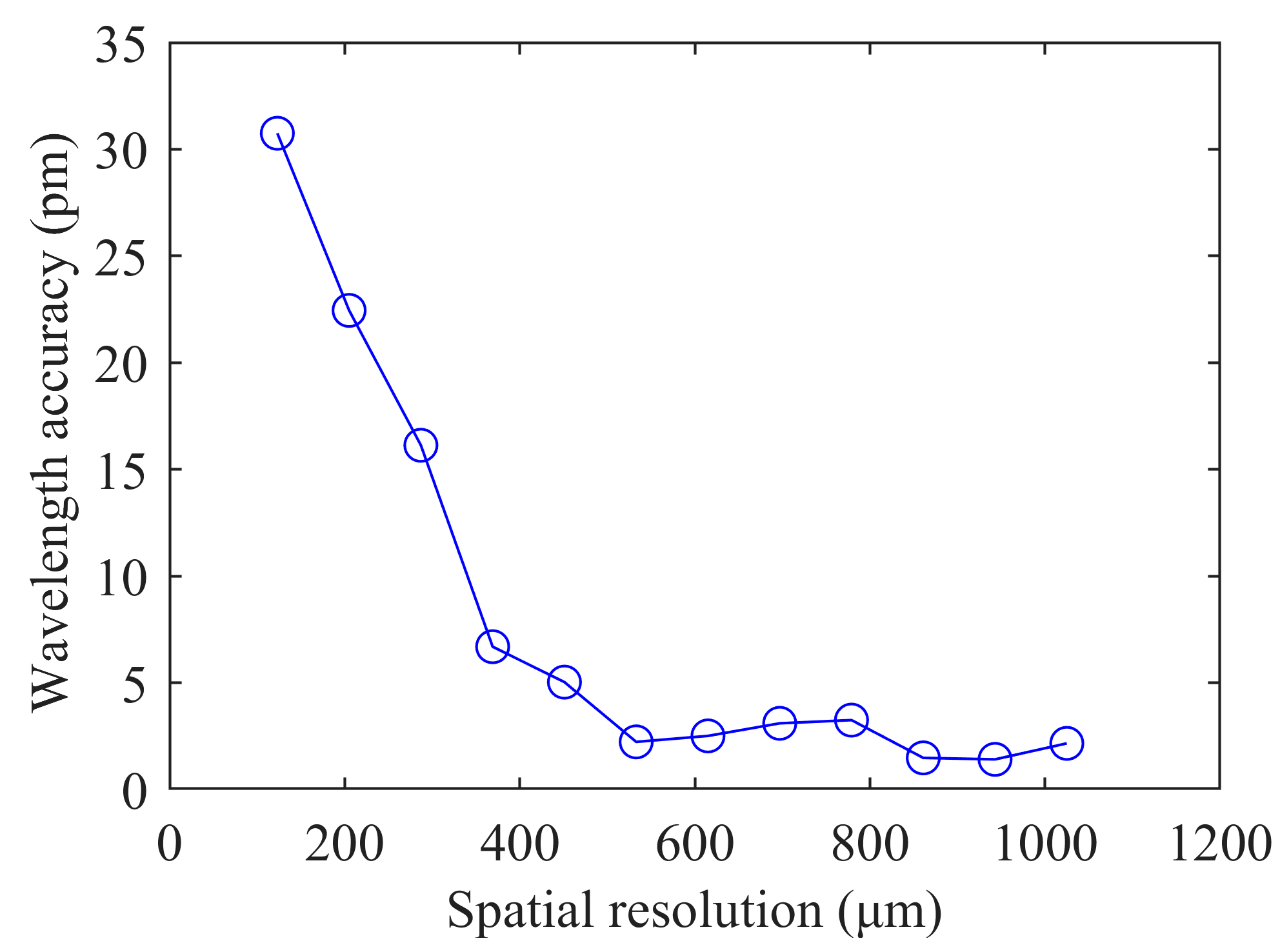
3. Experimental Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Eickhoff, W.; Ulrich, R. Optical frequency domain reflectometry in single-mode fiber. Appl. Phys. Lett. 1981, 39, 693–695. [Google Scholar] [CrossRef]
- Ma, Z.; Chen, X. Fiber Bragg Gratings Sensors for Aircraft Wing Shape Measurement: Recent Applications and Technical Analysis. Sensors 2018, 19, 55. [Google Scholar] [CrossRef] [PubMed]
- Fu, C.; Xiao, S.; Meng, Y.; Shan, R.; Liang, W.; Zhong, H.; Liao, C.; Yin, X.; Wang, Y. OFDR shape sensor based on a femtosecond-laser-inscribed weak fiber Bragg grating array in a multicore fiber. Opt. Lett. 2024, 49, 1273–1276. [Google Scholar] [CrossRef] [PubMed]
- Froggatt, M.; Moore, J. High-spatial-resolution distributed strain measurement in optical fiber with Rayleigh scatter. Appl. Opt. 1998, 37, 1735–1740. [Google Scholar] [CrossRef] [PubMed]
- Suo, L.; Lei, Z.; Zhao, S.; Wu, Z.; Takezawa, A. Study on sliding-window length based on Rayleigh backscattering spectrum correlation in distributed optical-fiber strain measurement. Opt. Fiber Technol. 2019, 47, 126–132. [Google Scholar] [CrossRef]
- Fu, C.; Peng, Z.; Li, P.; Meng, Y.; Zhong, H.; Du, C.; Wang, Y. Research on distributed fiber temperature/strain/shape sensing based on OFDR. Laser Optoelectron. Prog. 2023, 60, 1106007. [Google Scholar]
- Xu, B.; He, J.; Du, B.; Xiao, X.; Xu, X.; Fu, C.; He, J.; Liao, C.; Wang, Y. Femtosecond laser point-by-point inscription of an ultra-weak fiber Bragg grating array for distributed high-temperature sensing. Opt. Express 2021, 29, 32615–32626. [Google Scholar] [CrossRef] [PubMed]
- Zhong, H.; Liu, X.; Fu, C.; Xu, B.; He, J.; Li, P.; Meng, Y.; Du, C.; Chen, L.; Tang, J.; et al. Quasi-Distributed Temperature and Strain Sensors Based on Series-Integrated Fiber Bragg Gratings. Nanomaterials 2022, 12, 1540. [Google Scholar] [CrossRef] [PubMed]
- Fu, C.; Sui, R.; Peng, Z.; Meng, Y.; Zhong, H.; Shan, R.; Liang, W.; Liao, C.; Yin, X.; Wang, Y. Wide-range OFDR strain sensor based on the femtosecond-laser-inscribed weak fiber Bragg grating array. Opt. Lett. 2023, 48, 5819–5822. [Google Scholar] [CrossRef] [PubMed]
- Chen, B.; Li, A.; Yang, J.; Zhang, D.; Li, J.; Zhang, M.; Cheng, Q.; Zhu, J.; Li, Y. Real-Time Monitoring of Strain Processes With Large-Range and High-Spatial Resolution Using the Method of Weak Reflection FBG Measurement Based on OFDR. IEEE Trans. Instrum. Meas. 2024, 73, 3000111. [Google Scholar] [CrossRef]
- Qu, S.; Qin, Z.; Xu, Y.; Cong, Z.; Wang, Z.; Liu, Z. Improvement of Strain Measurement Range via Image Processing Methods in OFDR System. J. Light. Technol. 2021, 39, 6340–6347. [Google Scholar] [CrossRef]
- Ding, Z.; Yao, X.S.; Liu, T.; Du, Y.; Liu, K.; Jiang, J.; Meng, Z.; Chen, H. Compensation of laser frequency tuning nonlinearity of a long range OFDR using deskew filter. Opt. Express 2013, 21, 3826–3834. [Google Scholar] [CrossRef] [PubMed]
- Pan, M.; Hua, P.; Ding, Z.; Zhu, D.; Liu, K.; Jiang, J.; Wang, C.; Guo, H.; Zhang, T.; Li, S.; et al. Long Distance Distributed Strain Sensing in OFDR by BM3D-SAPCA Image Denoising. J. Light. Technol. 2022, 40, 7952–7960. [Google Scholar] [CrossRef]
- Ahn, T.J.; Lee, J.Y.; Kim, D.Y. Suppression of nonlinear frequency sweep in an optical frequency-domain reflectometer by use of Hilbert transformation. Appl. Opt. 2005, 44, 7630–7634. [Google Scholar] [CrossRef] [PubMed]
- Feng, B.; Liu, K.; Liu, T.; Jiang, J.; Du, Y. Improving OFDR spatial resolution by reducing external clock sampling error. Opt. Commun. 2016, 363, 74–79. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, Z.; Huang, W.; Zhao, Y.; Zhang, W. High-Spatial-Resolution High-Accuracy OFDR Distributed Sensors Based on Seamless fs-WFBG Array. Photonics 2025, 12, 352. https://doi.org/10.3390/photonics12040352
Jin Z, Huang W, Zhao Y, Zhang W. High-Spatial-Resolution High-Accuracy OFDR Distributed Sensors Based on Seamless fs-WFBG Array. Photonics. 2025; 12(4):352. https://doi.org/10.3390/photonics12040352
Chicago/Turabian StyleJin, Zhengze, Wenzhu Huang, Yuanjing Zhao, and Wentao Zhang. 2025. "High-Spatial-Resolution High-Accuracy OFDR Distributed Sensors Based on Seamless fs-WFBG Array" Photonics 12, no. 4: 352. https://doi.org/10.3390/photonics12040352
APA StyleJin, Z., Huang, W., Zhao, Y., & Zhang, W. (2025). High-Spatial-Resolution High-Accuracy OFDR Distributed Sensors Based on Seamless fs-WFBG Array. Photonics, 12(4), 352. https://doi.org/10.3390/photonics12040352






