Deformation Measurement of a SS304 Stainless Steel Sheet Using Digital Image Correlation Method
Abstract
:1. Introduction
2. DIC Methodology

Stress and Strain Calculation from the Correlation Function
- The digital image is first separated into smaller parts known as subsets.
- Subset shape functions are imposed on the reference subset to account for the deformed shape of the subset in the deformed picture.
- The distorted position of the subset may not be at the integer location.
- The correlation function (C) is constructed to match the similarity of the subset in the un-deformed and deformed images.
3. Experiments
4. Results
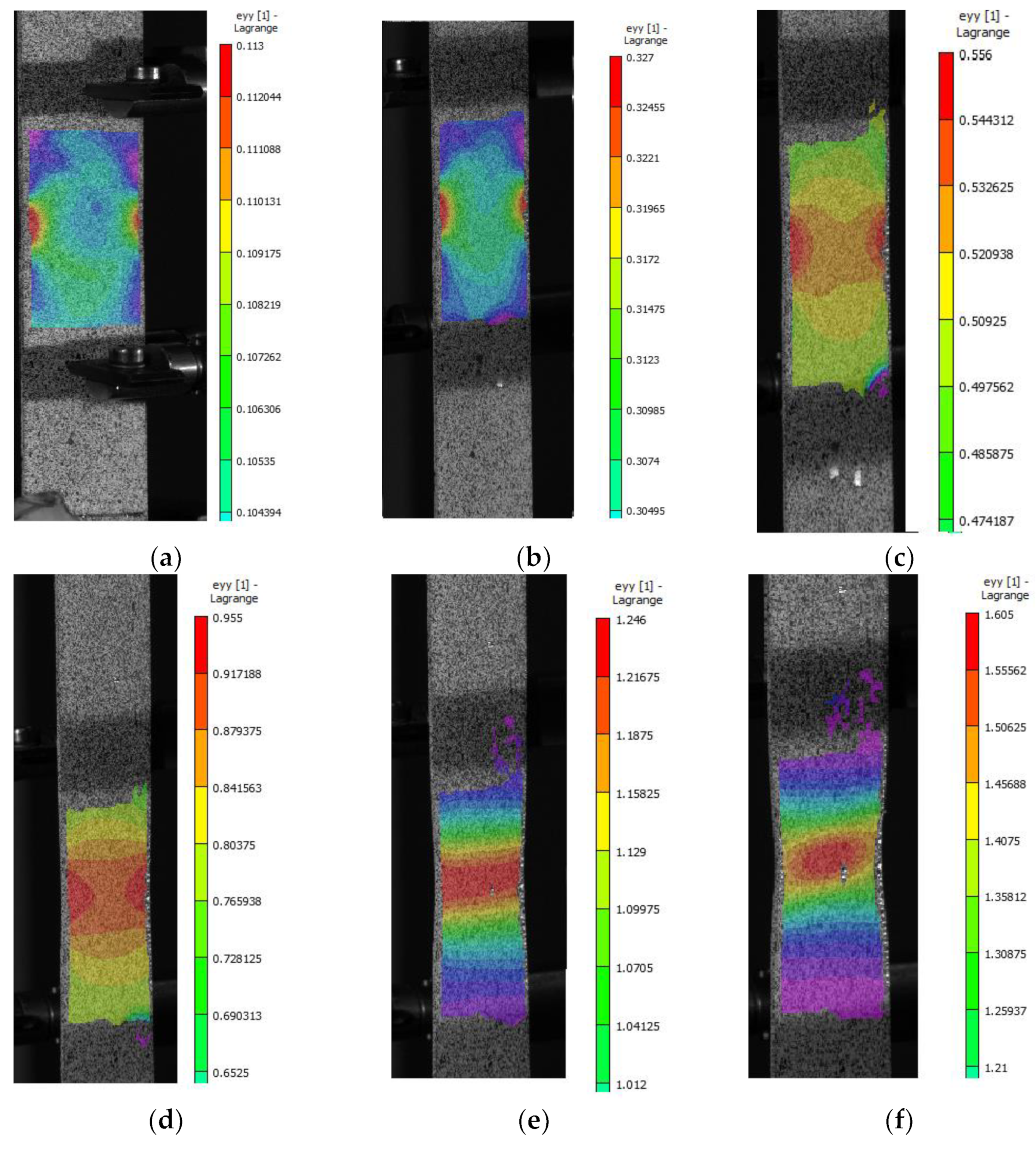
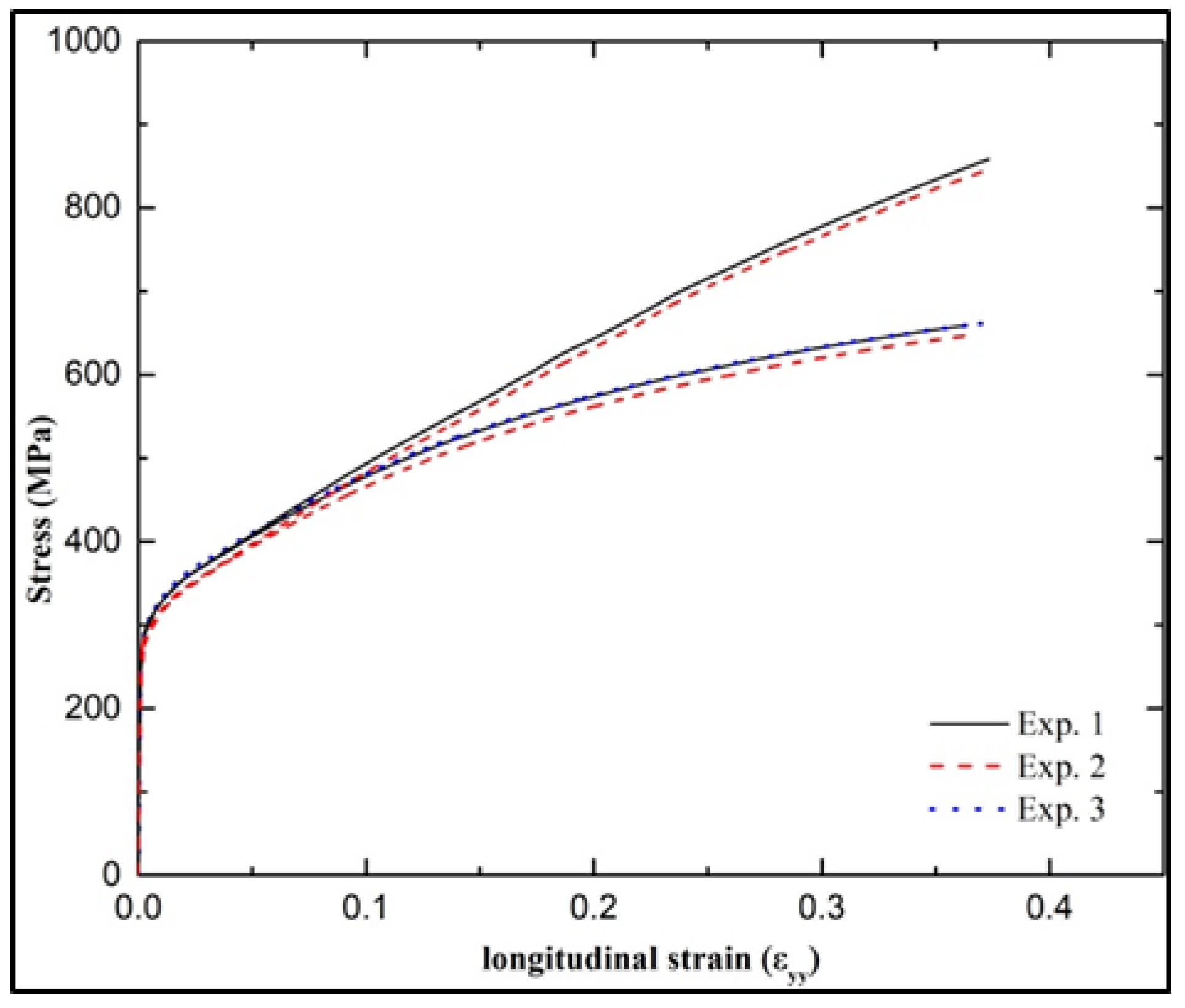
4.1. Experimental Results and Discussion
4.2. Numerical Results and Discussion
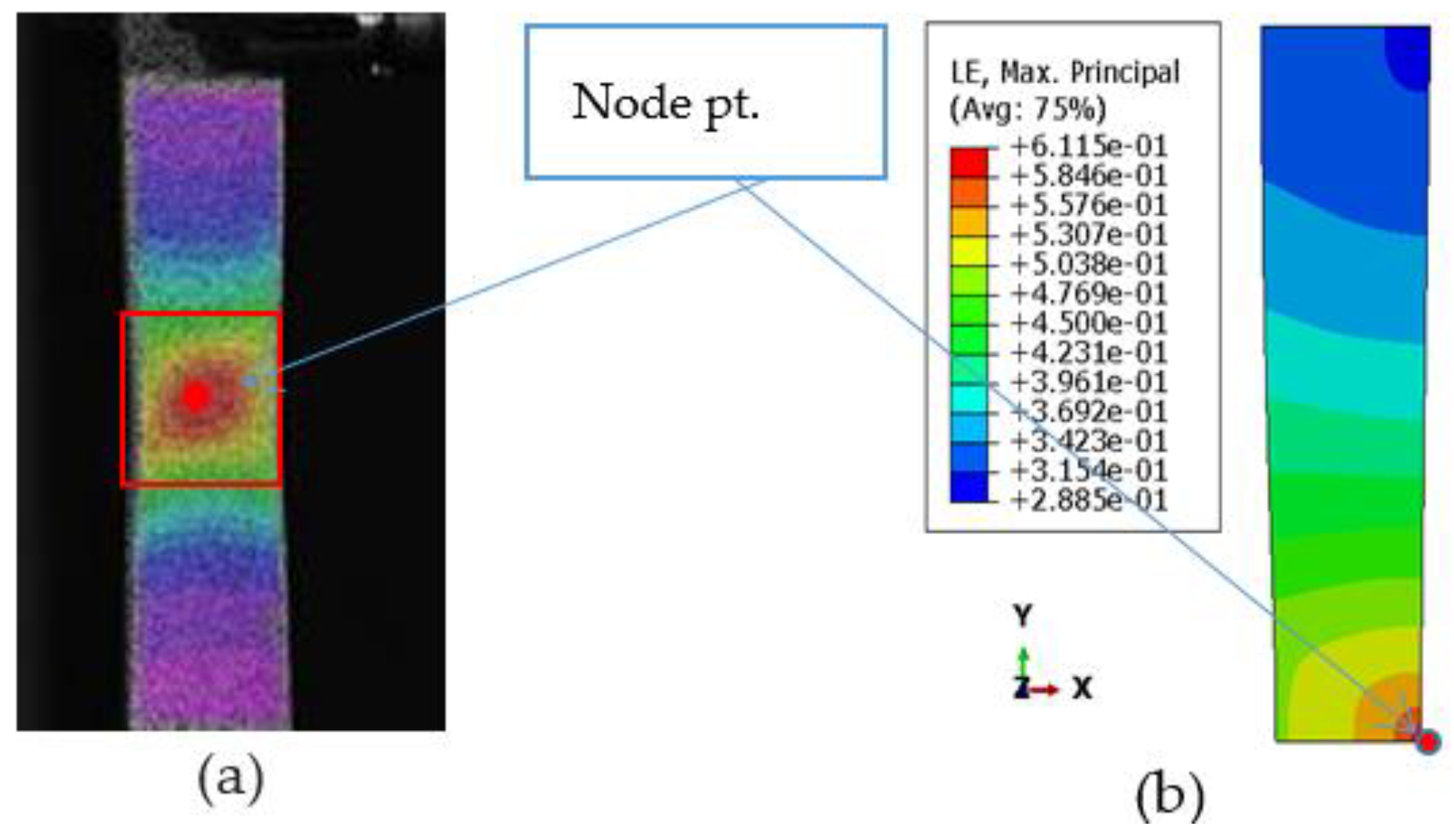
5. Validation
5.1. Simulation
5.2. Experimental
5.3. Reverse Retrieve Strategy
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Pandre, S.; Morchhale, A.; Kotkunde, N.; Singh, S.K. Influence of Processing Temperature on Formability of Thin-Rolled DP590 Steel Sheet. Mater. Manuf. Process. 2020, 35, 901–909. [Google Scholar] [CrossRef]
- Harikrishna, C.; Nagaraju, C. Modeling of Cylindrical Upsetting Process for Enhanced Ductile Fracture. Mater. Today Proc. 2020, 39, 1629–1634. [Google Scholar] [CrossRef]
- Habibi, N.; Zarei-Hanzaki, A.; Abedi, H.R. An Investigation into the Fracture Mechanisms of Twinning-Induced-Plasticity Steel Sheets under Various Strain Paths. J. Mater. Process. Technol. 2015, 224, 102–116. [Google Scholar] [CrossRef]
- Mishra, A.; Leguen, C.; Thuillier, S.; Maire, E. Investigation of Ductile Damage in DP980 Steel Sheets Using Mechanical Tests and X-ray Micro-Tomography. AIP Conf. Proc. 2011, 1353, 1464–1469. [Google Scholar] [CrossRef]
- Kim, J.H.; Serpantié, A.; Barlat, F.; Pierron, F.; Lee, M.G. Characterization of the Post-Necking Strain Hardening Behavior Using the Virtual Fields Method. Int. J. Solids Struct. 2013, 50, 3829–3842. [Google Scholar] [CrossRef] [Green Version]
- Jain, A.; Mishra, A. Comparative Study of Macroscopic Rupture Criteria. Mater. Today Proc. 2019, 18, 3394–3400. [Google Scholar] [CrossRef]
- Chen, Y.; Clausen, A.H.; Hopperstad, O.S.; Langseth, M. Stress-Strain Behaviour of Aluminium Alloys at a Wide Range of Strain Rates. Int. J. Solids Struct. 2009, 46, 3825–3835. [Google Scholar] [CrossRef] [Green Version]
- Gharehbaghi, S.; Gandomi, M.; Plevris, V.; Gandomi, A.H.; Abdulameer Kadhim, A.; Mohammed Kadhim, H.; Ham, S.W.; Cho, J.U.; Cheon, S.S.; Paresi, P.R.; et al. Fracture Prediction in Plastic Deformation Processes. Ductile Fract. Met. Form. 2020, 7, 1–17. [Google Scholar]
- Jain, M.; Lloyd, D.J.; Macewen, S.R. Hardening Laws, Surface Roughness and Biaxial Tensile Limit Strains of Sheet Aluminium Alloys. Int. J. Mech. Sci. 1996, 38, 219–232. [Google Scholar] [CrossRef]
- Gia Hai, V.; Thi Hong Minh, N.; Nguyen, D.T. A Study on Experiment and Simulation to Predict the Spring-Back of SS400 Steel Sheet in Large Radius of V-Bending Process. Mater. Res. Express 2020, 7, 016562. [Google Scholar] [CrossRef]
- Lou, Y.; Yoon, J.W. A User-Friendly Anisotropic Ductile Fracture Criterion for Sheet Metal under Proportional Loading. Int. J. Solids Struct. 2021, 217, 48–59. [Google Scholar] [CrossRef]
- Boyce, B.L.; Reu, P.L.; Robino, C.V. The Constitutive Behavior of Laser Welds in 304L Stainless Steel Determined by Digital Image Correlation. Metall. Mater. Trans. A Phys. Metall. Mater. Sci. 2006, 37, 2481–2492. [Google Scholar] [CrossRef]
- Khare, S.; Kumar, K.; Choudhary, S.; Singh, P.K.; Verma, R.K.; Mahajan, P. Determination of Johnson–Cook Material Parameters for Armour Plate Using DIC and FEM. Met. Mater. Int. 2021, 27, 4984–4995. [Google Scholar] [CrossRef]
- Jang, I.; Bae, G.; Song, J.; Kim, H.; Park, N. Fracture Envelopes on the 3D-DIC and Hybrid Inverse Methods Considering Loading History. Mater. Des. 2020, 194, 108934. [Google Scholar] [CrossRef]
- Iosipescu, N. New Accurate Procedure for Single Shear Testing of Metals. J. Mater. 1967, 2, 537–566. [Google Scholar]
- Jain, A.; Mishra, A.; Tiwari, V. Investigation of Rupture in SS304 Steel Sheet Using Macroscopic Criteria. IOP Conf. Ser. Mater. Sci. Eng. 2020, 998, 012015. [Google Scholar] [CrossRef]
- Wang, L.; Park, J.H.; Choi, N.S. Observation of Notch Effect in Al6061-T6 Specimens under Tensile Loading Using Digital Image Correlation and Finite Element Method. J. Mech. Sci. Technol. 2020, 34, 1049–1058. [Google Scholar] [CrossRef]
- Gardner, K.A. Experimental Techniques for Shear Testing of Thin Sheet Metals and Compression Testing at Intermediate Strain Rates. Master’s Thesis, The Ohio State University, Columbus, OH, USA, 2013; p. 133. [Google Scholar]
- Paul, S.K.; Roy, S.; Sivaprasad, S.; Bar, H.N.; Tarafder, S. Identification of Post-Necking Tensile Stress–Strain Behavior of Steel Sheet: An Experimental Investigation Using Digital Image Correlation Technique. J. Mater. Eng. Perform. 2018, 27, 5736–5743. [Google Scholar] [CrossRef]
- Versaillot, P.D.; Wu, Y.F.; Zhao, Z.L. An Investigation into the Phenomenon of Macroscopic Plastic Deformation Localization in Metals. J. Phys. Conf. Ser. 2021, 1777, 012067. [Google Scholar] [CrossRef]
- Pham, C.H.; Thuillier, S.; Manach, P.Y. Mechanical Properties Involved in the Micro-Forming of Ultra-Thin Stainless Steel Sheets. Metall. Mater. Trans. A Phys. Metall. Mater. Sci. 2015, 46, 3502–3515. [Google Scholar] [CrossRef]
- Field, J.E.; Walley, S.M.; Proud, W.G.; Goldrein, H.T.; Siviour, C.R. Review of Experimental Techniques for High Rate Deformation and Shock Studies. Int. J. Impact Eng. 2004, 30, 725. [Google Scholar] [CrossRef]
- Peters, W.H.; Ranson, W.F. Digital Imaging Techniques In Experimental Stress Analysis. Opt. Eng. 1982, 21, 213427. [Google Scholar] [CrossRef]
- Hild, F.; Roux, S. Digital Image Correlation: From Displacement Measurement to Identification of Elastic Properties—A Review. Strain 2006, 42, 69–80. [Google Scholar] [CrossRef] [Green Version]
- Tiwari, V.; Sutton, M.A.; McNeill, S.R. Assessment of High Speed Imaging Systems for 2D and 3D Deformation Measurements: Methodology Development and Validation. Exp. Mech. 2007, 47, 561–579. [Google Scholar] [CrossRef]
- Bornert, M.; Brémand, F.; Doumalin, P.; Dupré, J.C.; Fazzini, M.; Grédiac, M.; Hild, F.; Mistou, S.; Molimard, J.; Orteu, J.J.; et al. Assessment of Digital Image Correlation Measurement Errors: Methodology and Results. Exp. Mech. 2009, 49, 353–370. [Google Scholar] [CrossRef] [Green Version]
- Schreier, H.; Orteu, J.J.; Sutton, M.A. Image Correlation for Shape, Motion and Deformation Measurements: Basic Concepts, Theory and Applications. In Image Correlation for Shape, Motion and Deformation Measurements; Springer: Berlin/Heidelberg, Germany, 2009; 321p. [Google Scholar] [CrossRef]
- Motra, H.B.; Hildebrand, J.; Dimmig-Osburg, A. Assessment of Strain Measurement Techniques to Characterise Mechanical Properties of Structural Steel. Eng. Sci. Technol. Int. J. 2014, 17, 260–269. [Google Scholar] [CrossRef] [Green Version]
- Jia, B.; Rusinek, A.; Pesci, R.; Bernier, R.; Bahi, S.; Wood, P. A Novel Technique for Dynamic Shear Testing of Bulk Metals with Application to 304 Austenitic Stainless Steel. Int. J. Solids Struct. 2020, 204, 153–171. [Google Scholar] [CrossRef]
- Kajberg, J.; Sundin, K.G.; Melin, L.G.; Ståhle, P. High Strain-Rate Tensile Testing and Viscoplastic Parameter Identification Using Microscopic High-Speed Photography. Int. J. Plast. 2004, 20, 561–575. [Google Scholar] [CrossRef] [Green Version]
- Hung, P.C.; Voloshin, A.S. In-Plane Strain Measurement by Digital Image Correlation. J. Braz. Soc. Mech. Sci. Eng. 2003, 25, 215–221. [Google Scholar] [CrossRef] [Green Version]
- Schwab, R.; Harter, A. Extracting True Stresses and Strains from Nominal Stresses and Strains in Tensile Testing. Strain 2021, 57, e12396. [Google Scholar] [CrossRef]






| Type | Approach | Mechanism of Mechanical Strain Evaluation | Test | Image Analysis | Advantage | Disadvantage |
|---|---|---|---|---|---|---|
| Electro-based | The deformation of the material induces the electrical signal changes, which can be converted into the strain values of the materials. | Discrete In vivo Ex vivo | N/A | Cheap; offline work | Invasive; low anti-interference | |
| Light -based |
| The relative strain is assessed by comparing the images before and after the material deformation. | Serial Ex vivo | Marker-tracking algorithm | Cheap; easy operation | Transparent or translucent samples |
| Strain measurement based on load-induced (stress-induced) birefringence. | Cheap; easy application | time-consuming | Regional phase unwrapping algorithm | ||
| Texture-correlation-based |
| Strain is quantified from changed search space patterns in the images during the deformation of the materials. | Serial In vivo Ex vivo | Simple structure | Complex analysis | Baseband speckle tracking algorithm; Registration algorithm |
| Imaging-based |
| The Moiré effect is the mechanical interference of light by a superimposed network of lines. | In-plane fringes; out-plane fringes | Fast; non-invasive; portable; cost-efficient and has no harmful radiation | Resolution; costly | Moiré fringe phase shifting measurement algorithm |
| Strain is evaluated by tracking the subsets including markers or speckles on the surface of tissues. | Serial Ex vivo | Relatively fast imaging; relatively low cost | Expensive; high contrast | Computed tomography | |
| Strain is evaluated by tracking image subsets by tracking the natural pattern in the tissues. | Serial In vivo Ex vivo | Correlation-based algorithm |
| DIC System | Externsiometer |
|---|---|
| Non-contact measurement | Contact measurement |
| Unlimited number of deformation measurements | An extensometer can be used only once (a glued extensometer cannot be peeled off without damaging it) |
| The possibility of testing samples of any shape and material; the tested surface of the sample does not have to be flat | A surface on which the extensometer is glued has to be flat |
| The ability to measure deformation in all directions (along the X-axis, Y-axis, and Z-axis), on a plane or in three-dimensional space | The ability to measure deformation only in the chosen direction |
| Full-field deformation analysis | Results of the deformation at selected points of the sample, i.e., at the points where the sensors are attached |
| A measurement of the real maximum displacements and deformations | A measurement limited by the maximum value of the deformation of an extensometer |
| A quick preparation of a random pattern of black dots on a white background on the sample surface by spraying paint | A time-consuming process of placing the extensometer on the surface of a sample (gluing, etc.) |
| The need to clean the surface of a sample before testing | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jain, A.; Mishra, A.; Tiwari, V.; Singh, G.; Singh, R.P.; Singh, S. Deformation Measurement of a SS304 Stainless Steel Sheet Using Digital Image Correlation Method. Photonics 2022, 9, 912. https://doi.org/10.3390/photonics9120912
Jain A, Mishra A, Tiwari V, Singh G, Singh RP, Singh S. Deformation Measurement of a SS304 Stainless Steel Sheet Using Digital Image Correlation Method. Photonics. 2022; 9(12):912. https://doi.org/10.3390/photonics9120912
Chicago/Turabian StyleJain, Appurva, Abhishek Mishra, Vikrant Tiwari, Gurminder Singh, Ravinder Pal Singh, and Sunpreet Singh. 2022. "Deformation Measurement of a SS304 Stainless Steel Sheet Using Digital Image Correlation Method" Photonics 9, no. 12: 912. https://doi.org/10.3390/photonics9120912
APA StyleJain, A., Mishra, A., Tiwari, V., Singh, G., Singh, R. P., & Singh, S. (2022). Deformation Measurement of a SS304 Stainless Steel Sheet Using Digital Image Correlation Method. Photonics, 9(12), 912. https://doi.org/10.3390/photonics9120912






