Design of Matching Imaging on Agile Satellite with Wide-Swath Whiskbroom Payloads along the Coastal Zone
Abstract
1. Introduction
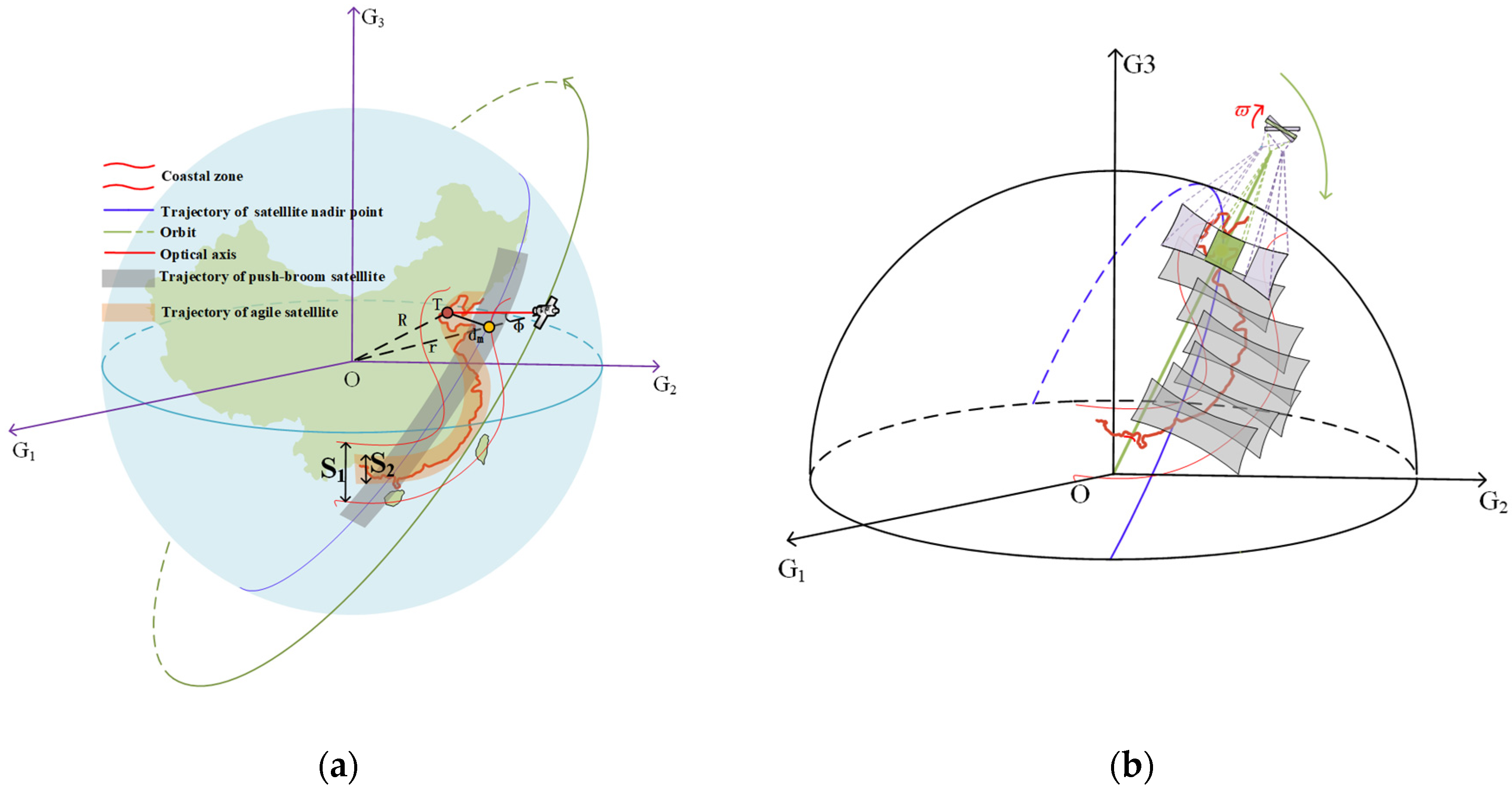
2. Attitude Adjustment and Swing Mirror Scanning of Agile Satellites Imaging the Coastal Zone
2.1. Attitude Adjustment of Agile Satellite
2.2. Seamless Imaging by the Swing Mirror
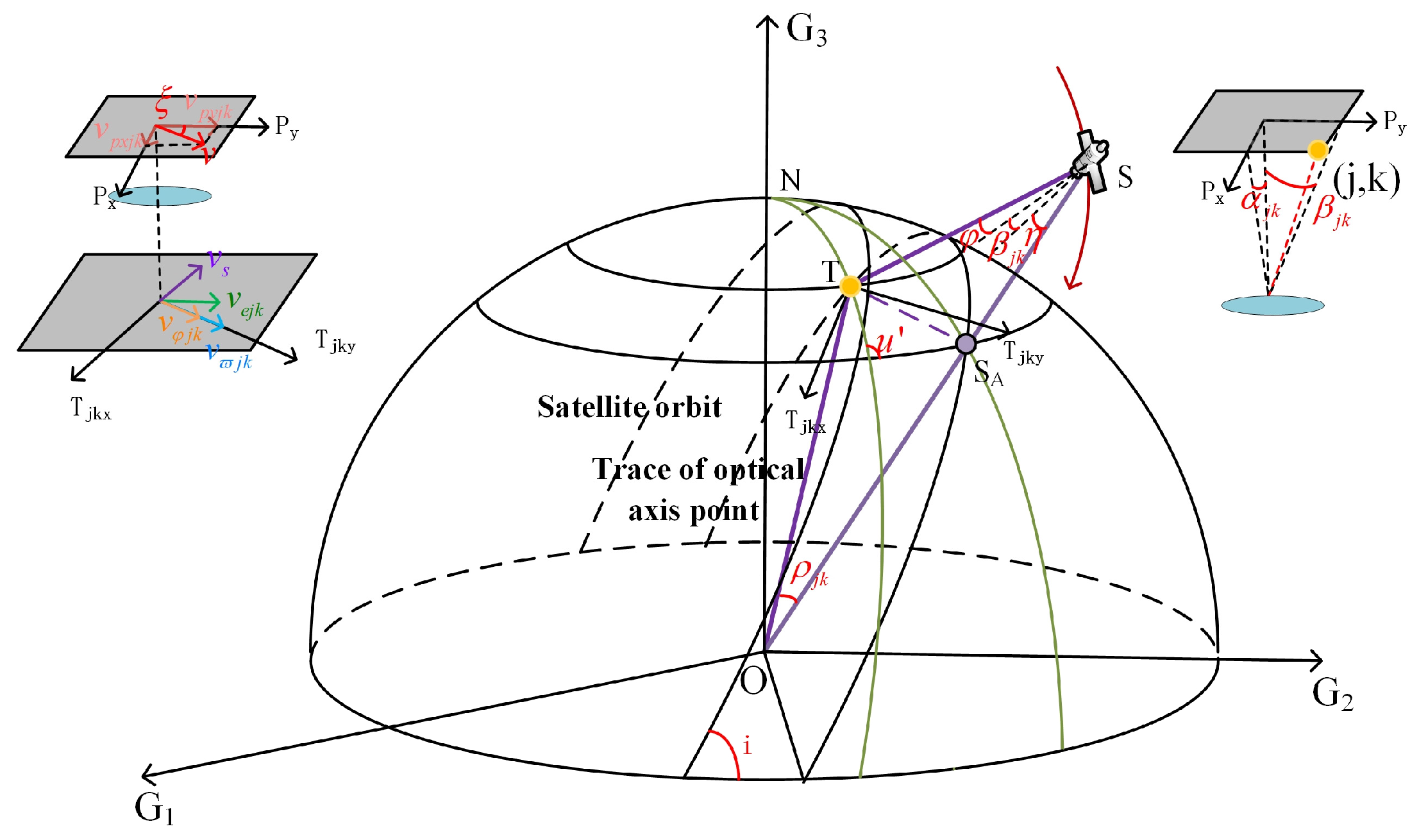
3. Matching Strategy Based on Image Velocity Vector Field
3.1. Velocity Vector Model on the Image Plane
3.1.1. The Position of Objects
3.1.2. Velocity Vector
- 1.
- The projected linear velocity of the satellite’s flight velocity at the project point: ;
- 2.
- The linear velocity of the Earth’s rotation at the object point: ;
- 3.
- The linear velocity of roll angle at the object point: .
- 4.
- The line velocity of the swing mirror at the object point: .
3.2. Velocity Vector Matching
- 1.
- Adjustment of yaw angle: first, during imaging along the coast, the satellite continuously adjusts the yaw angle to ensure that the imaging direction of the detector coincides with the direction of resultant velocity in the center of the image plane. The value of yaw angle is ;
- 2.
- Tilt of detectors: adjustment of yaw angle only matches the velocity vector in the center pixel of the image plane. However, the residual mismatched angles of other pixels still exist. These residual mismatched angles can be compensated by tilting the detectors. The tilt angles of detectors are ;
- 3.
- Adjustment of the imaging time of detectors: step 1 and step 2 only consider the direction of the velocity vector. The value of the velocity vector is compensated by adjusting the imaging time of detectors separately.
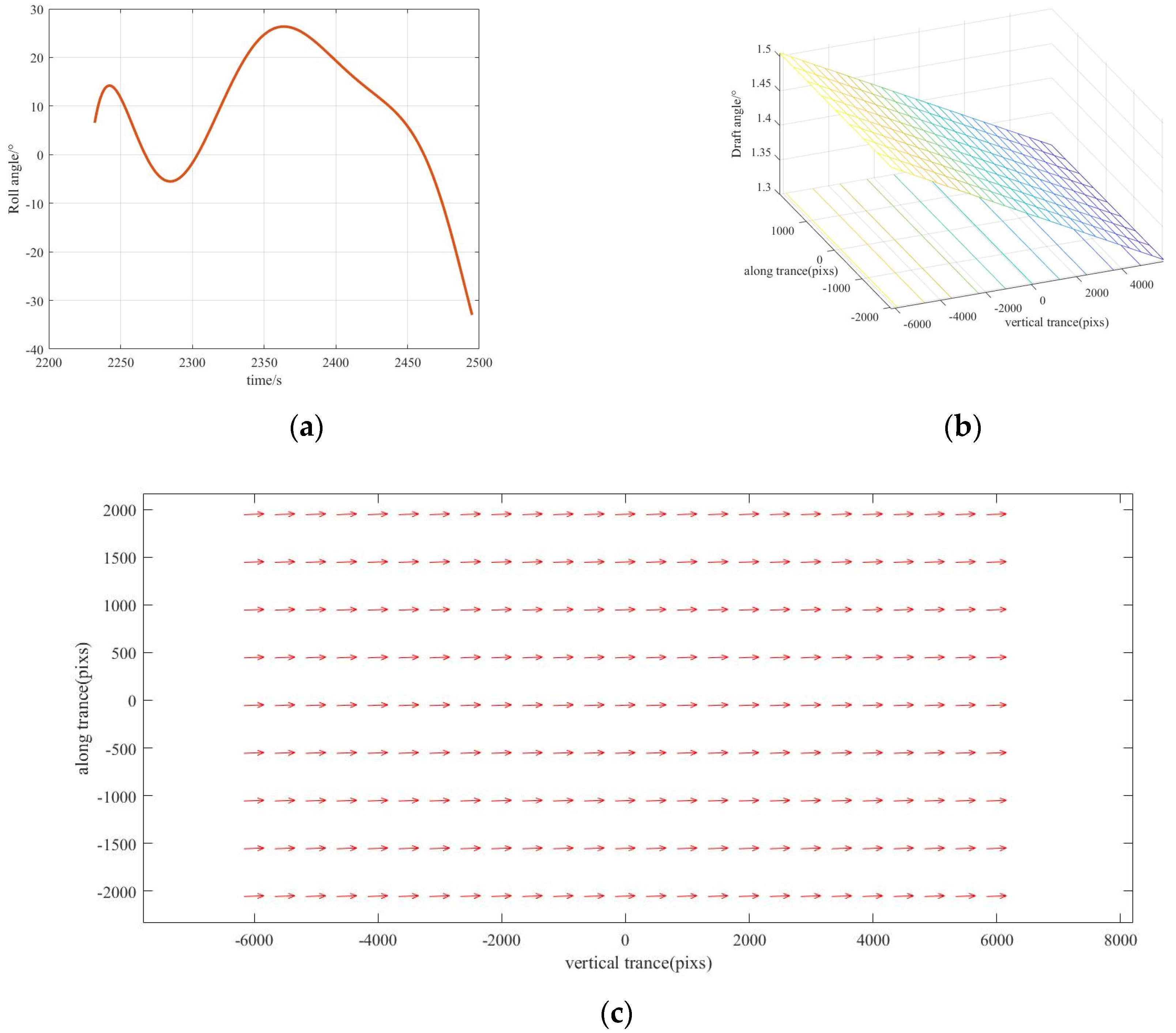
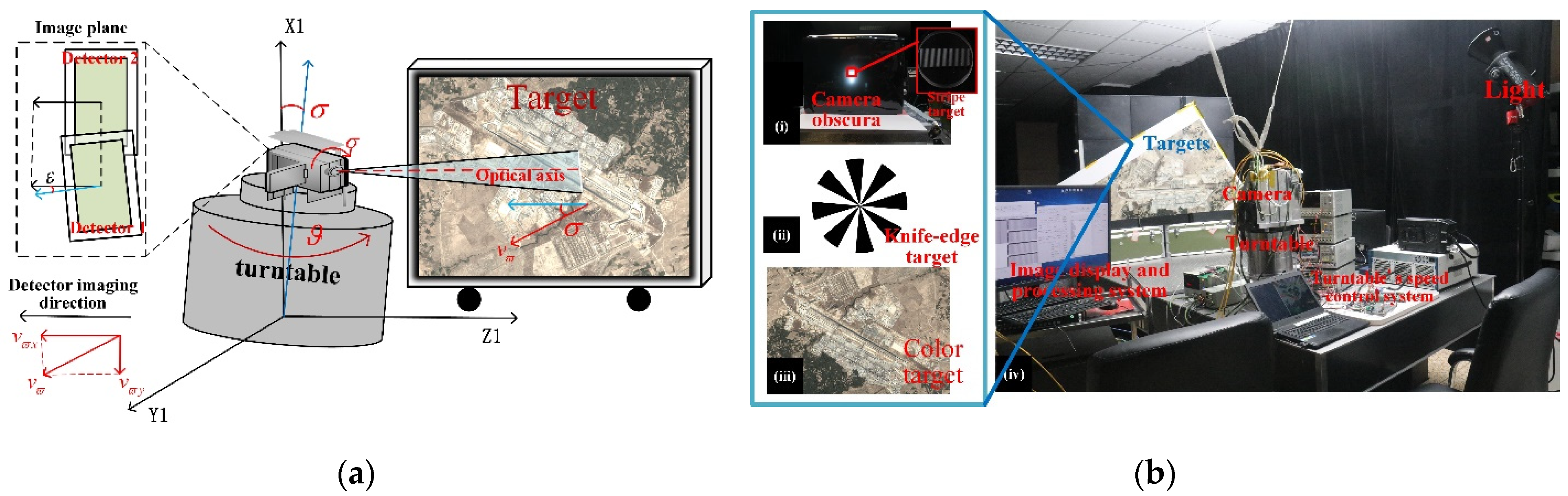
4. Experiment
4.1. Design of Experiment
- 1.
- The lens and detectors are rotated by an angle , which is equivalent to adjustment of yaw angle;
- 2.
- The side detectors are tilted by an angle to compensate for residual mismatched angles;
- 3.
- Adjustment of the imaging time of detectors to compensate for the value of the velocity vector. The imaging time of the center detector is . The imaging time of the side detector is .
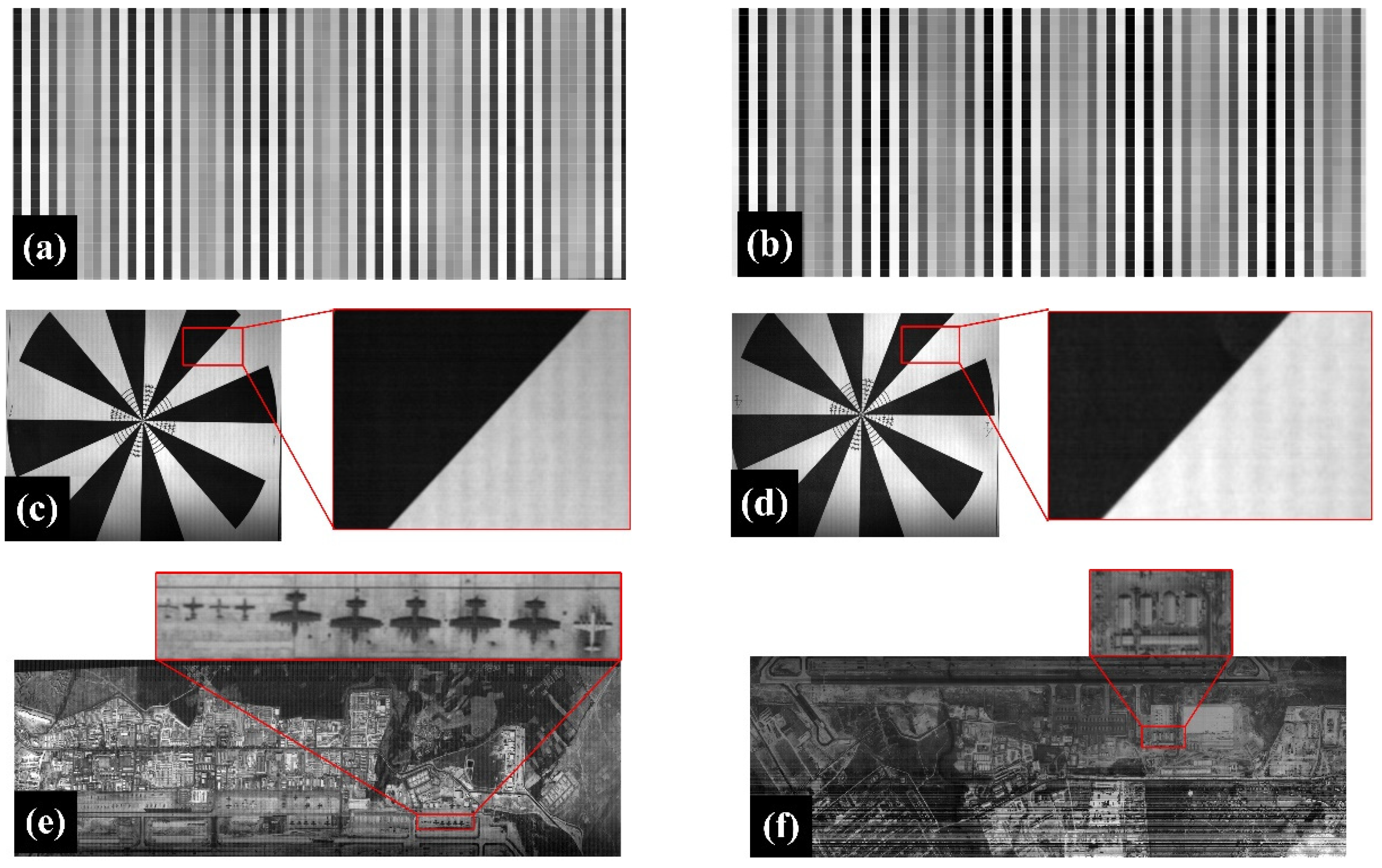
4.2. Experiment Procedure and Results
4.2.1. Resolution
4.2.2. Modulation Transfer Function
4.2.3. Color image
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jain, A.; Ramakrishnan, R.; Thomaskutty, A.V.; Agrawal, R.; Rajawat, A.S.; Solanki, H. Topography and morphodynamic study of intertidal mudflats along the eastern coast of the Gulf of Khambhat, India using remote sensing techniques. Remote Sens. Appl.-Soc. Environ. 2022, 27, 100798. [Google Scholar] [CrossRef]
- Mao, Y.J.; Harris, D.L.; Xie, Z.Y.; Phinn, S. Global coastal geomorphology-integrating earth observation and geospatial data. Remote Sens. Environ. 2022, 278, 113082. [Google Scholar] [CrossRef]
- Amani, M.; Mohseni, F.; Layegh, N.F.; Nazari, M.E.; Fatolazadeh, F.; Salehi, A.; Ahmadi, S.A.; Ebrahimy, H.; Ghorbanian, A.; Jin, S.G.; et al. Remote Sensing Systems for Ocean: A Review (Part 2: Active Systems). IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2022, 15, 1421–1453. [Google Scholar] [CrossRef]
- Amani, M.; Ghorbanian, A.; Asgarimehr, M.; Yekkehkhany, B.; Moghimi, A.; Jin, S.G.; Naboureh, A.; Mohseni, F.; Mahdavi, S.; Layegh, N.F. Remote Sensing Systems for Ocean: A Review (Part 1: Passive Systems). IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2022, 15, 210–234. [Google Scholar] [CrossRef]
- Benveniste, J.; Mandea, M.; Melet, A.; Ferrier, P. Earth Observations for Coastal Hazards Monitoring and International Services: A European Perspective. Surv. Geophys. 2020, 41, 1185–1208. [Google Scholar] [CrossRef]
- Melet, A.; Teatini, P.; Le Cozannet, G.; Jamet, C.; Conversi, A.; Benveniste, J.; Almar, R. Earth Observations for Monitoring Marine Coastal Hazards and Their Drivers. Surv. Geophys. 2020, 41, 1489–1534. [Google Scholar] [CrossRef]
- Xu, C.; Yang, X.; Xu, T.; Zhu, L.; Chang, L.; Jin, G.; Qi, X. Study of space optical dynamic push-broom imaging along the trace of targets. Optik 2020, 202, 163640. [Google Scholar] [CrossRef]
- Chen, F.S.; Hu, Z.Y.; Li, X.Y.; Yang, L.; Hu, X.J.; Zhang, Y. Research on Wide-Range and High-Resolution Thermal Infrared Remote Sensing Imaging Technology. Chin. J. Lasers-Zhongguo Jiguang 2021, 48, 334–342. [Google Scholar] [CrossRef]
- Dolloff, J.; Settergren, R. An Assessment of WorldView-1 Positional Accuracy based on Fifty Contiguous Stereo Pairs of Imagery. Photogramm. Eng. Remote Sens. 2010, 76, 935–943. [Google Scholar] [CrossRef]
- Capaldo, P.; Crespi, M.; Fratarcangeli, F.; Nascetti, A.; Pieralice, F. DSM generation from high resolution imagery: Applications with WorldView-1 and Geoeye-1. Ital. J. Remote Sens.-Riv. Ital. Di Telerilevamento 2012, 44, 41–53. [Google Scholar] [CrossRef]
- Alkan, M.; Buyuksalih, G.; Sefercik, U.G.; Jacobsen, K. Geometric accuracy and information content of WorldView-1 images. Opt. Eng. 2013, 52, 026201. [Google Scholar] [CrossRef]
- Davranche, A.; Lefebvre, G.; Poulin, B. Wetland monitoring using classification trees and SPOT-5 seasonal time series. Remote Sens. Environ. 2010, 114, 552–562. [Google Scholar] [CrossRef]
- Candra, D.S.; Sciences, E. Analysis of spot-6 data fusion using gram-schmidt spectral sharpening on rural areas. Int. J. Remote Sens. Earth Sci. 2014, 10, 839–844. [Google Scholar] [CrossRef][Green Version]
- Pu, R.L.; Landry, S.; Yu, Q.Y. Assessing the potential of multi-seasonal high resolution Pleiades satellite imagery for mapping urban tree species. Int. J. Appl. Earth Obs. Geoinf. 2018, 71, 144–158. [Google Scholar] [CrossRef]
- Bagnardi, M.; Gonzalez, P.J.; Hooper, A. High-resolution digital elevation model from tri-stereo Pleiades-1 satellite imagery for lava flow volume estimates at Fogo Volcano. Geophys. Res. Lett. 2016, 43, 6267–6275. [Google Scholar] [CrossRef]
- Zhi, Z.; Qu, H.; Tao, S.; Zheng, L.; Ying, S.; Zhu, H. The Design of Cone and Pendulum Scanning Mode Using Dual-Camera with Multi-Dimensional Motion Imaging Micro-Nanosatellite. Remote Sens. 2022, 14, 4613. [Google Scholar] [CrossRef]
- Lee, L.S.; Lo, K.; Chiang, Y.J. Ocean Color Imager: Instrument description and its performance. Terr. Atmos. Ocean. Sci. 1999, 10, 43–61. [Google Scholar] [CrossRef]
- Qiu, W.; Xu, C.; Ren, Z.G.; Teo, K.L. Scheduling and Planning Framework for Time Delay Integration Imaging by Agile Satellite. IEEE Trans. Aerosp. Electron. Syst. 2022, 58, 189–205. [Google Scholar] [CrossRef]
- Wahballah, W.A.; Eltohamy, F.; Bazan, T.M. Influence of Attitude Parameters on Image Quality of Very High-Resolution Satellite Telescopes. IEEE Trans. Aerosp. Electron. Syst. 2021, 57, 1177–1183. [Google Scholar] [CrossRef]
- Qiu, W.; Xu, C. Attitude Maneuver Planning of Agile Satellites for Time Delay Integration Imaging. J. Guid. Control. Dyn. 2020, 43, 46–59. [Google Scholar] [CrossRef]
- Xu, T.; Yang, X.; Wang, S.; Han, J.; Chang, L.; Yue, W. Imaging Velocity Fields Analysis of Space Camera for Dynamic Circular Scanning. IEEE Access 2020, 8, 191574–191585. [Google Scholar] [CrossRef]
- Wang, S.E.; Yang, X.B.; Feng, R.P.; Gao, S.N.; Han, J.L. Dynamic Disturbance Analysis of Whiskbroom Area Array Imaging of Aerospace Optical Camera. IEEE Access 2021, 9, 137099–137106. [Google Scholar] [CrossRef]
- Prithiviraj, S.; Alyson, L.; Avery, T.; Konstantinos, F. Variable shearing holography with applications to phase imaging and metrology. Light Adv. Manuf. 2022, 3, 193–210. [Google Scholar] [CrossRef]
- Han, J.; Yang, X.; Yue, W.; Xu, T.; Wang, S.; Chang, L.; Yang, C. Image Motion of Remote Sensing Camera with Wide Field of View Over the Antarctic and Arctic. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2021, 14, 3475–3484. [Google Scholar] [CrossRef]
- Sun, T.; Long, H.; Liu, B.-C.; Li, Y. Application of side-oblique image-motion blur correction to Kuaizhou-1 agile optical images. Opt. Express 2016, 24, 6665–6679. [Google Scholar] [CrossRef]
- Li, W.; Hu, C.; Zhang, L.; Yan, C. Drift angle compensation method for a high-resolution and wide-range space camera. Measurement 2020, 158, 107710. [Google Scholar] [CrossRef]
- Guo, Q.; Guan, M. Image rotation modeling for two-dimensional plane mirror scanning and its application in precise image positioning for linear sensor imager on geostationary platform. J. Appl. Remote Sens. 2007, 1, 013524. [Google Scholar] [CrossRef]
- Liu, Z.Y.; Gao, L.X.; Wang, J.K.; Tian, Y. Research on the influence of swing mirror of infrared imaging system with image-space scanning. Infrared Phys. Technol. 2018, 92, 459–465. [Google Scholar] [CrossRef]
- Stollenwerk, T.; Michaud, V.; Lobe, E.; Picard, M.; Basermann, A.; Botter, T. Agile Earth Observation Satellite Scheduling with a Quantum Annealer. IEEE Trans. Aerosp. Electron. Syst. 2021, 57, 3520–3528. [Google Scholar] [CrossRef]

| Parameters | Meaning | Value |
|---|---|---|
| Orbital inclination | 97° | |
| Semi-major axis | 6878.14 km | |
| The eccentricity of satellite orbit | 0 | |
| Right ascension of ascending node | 295° | |
| The argument of perigee | 0° | |
| The true perigee angle | 0° | |
| Epoch time | 12 July 2021 04:00:00 | |
| Orbit altitude | ||
| Greenwich sidereal time | 6.11 rad | |
| The focal length of satellite | ||
| The size of single pixel of satellite | ||
| The field of view of the satellite | ||
| Ground resolution of the satellite | ||
| The coverage width of satellite |
| Parameter | Meaning | Value |
|---|---|---|
| Focal length of lens | 200 mm | |
| The size of single pixel in the experiment | ||
| The distance between target and lens | 2.2 m | |
| The curvature of target | ||
| Angular velocity of turntable | ||
| Detector tilt angle |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, J.; Yang, X.; Wu, M.; He, X.; Fu, Z.; Gao, S. Design of Matching Imaging on Agile Satellite with Wide-Swath Whiskbroom Payloads along the Coastal Zone. Photonics 2022, 9, 930. https://doi.org/10.3390/photonics9120930
Du J, Yang X, Wu M, He X, Fu Z, Gao S. Design of Matching Imaging on Agile Satellite with Wide-Swath Whiskbroom Payloads along the Coastal Zone. Photonics. 2022; 9(12):930. https://doi.org/10.3390/photonics9120930
Chicago/Turabian StyleDu, Jiamin, Xiubin Yang, Mo Wu, Xi He, Zongqiang Fu, and Suining Gao. 2022. "Design of Matching Imaging on Agile Satellite with Wide-Swath Whiskbroom Payloads along the Coastal Zone" Photonics 9, no. 12: 930. https://doi.org/10.3390/photonics9120930
APA StyleDu, J., Yang, X., Wu, M., He, X., Fu, Z., & Gao, S. (2022). Design of Matching Imaging on Agile Satellite with Wide-Swath Whiskbroom Payloads along the Coastal Zone. Photonics, 9(12), 930. https://doi.org/10.3390/photonics9120930




