Stability of the Discrete Time-Crystalline Order in Spin-Optomechanical and Open Cavity QED Systems
Abstract
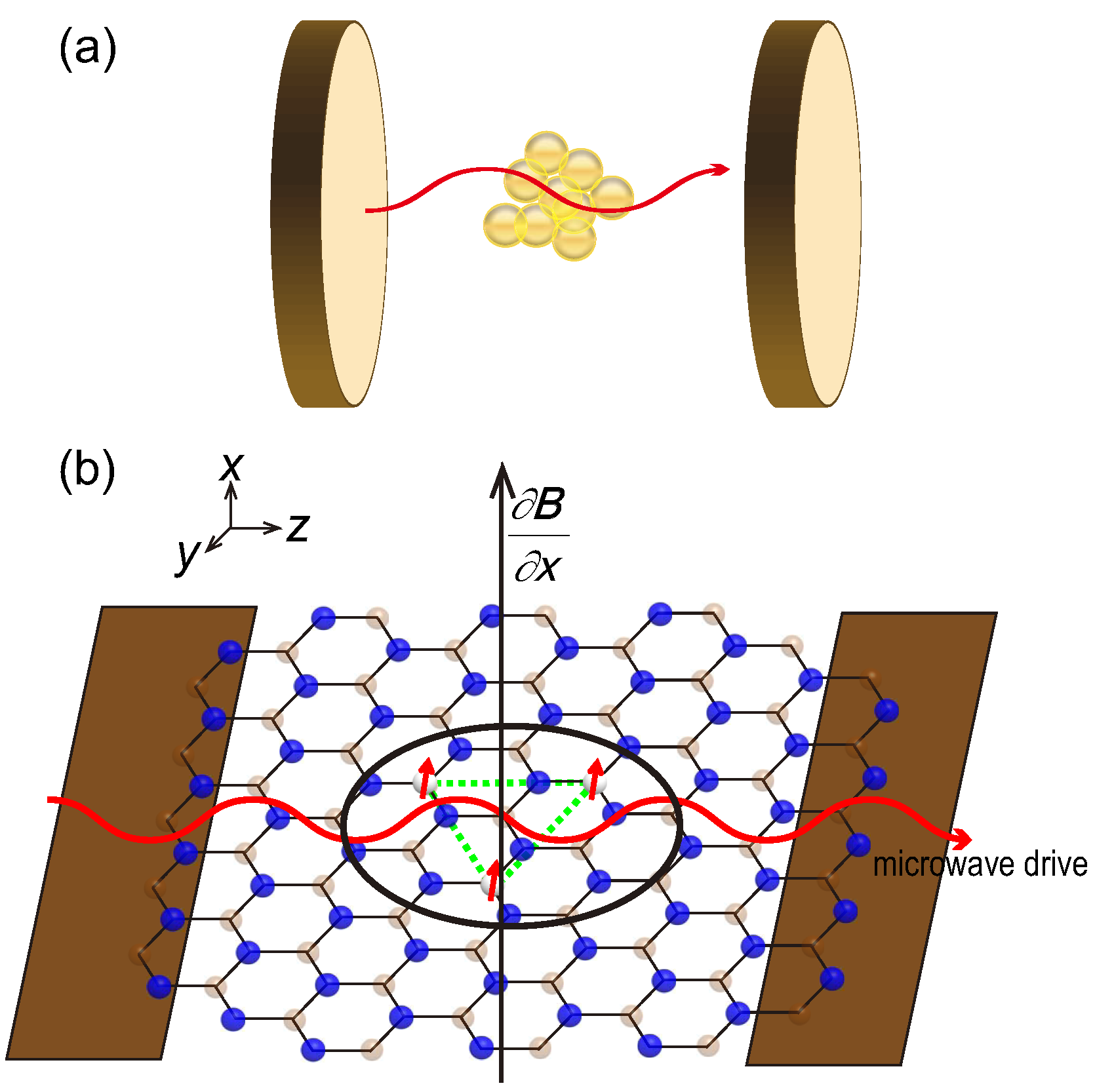
:1. Introduction
2. Perfect DTC in the Thermodynamic Limit
3. Transient DTC Behavior in the Deep Quantum Regime
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Abanin, D.A.; Altman, E.; Bloch, I.; Serbyn, M. Colloquium: Many-body localization, thermalization, and entanglement. Rev. Mod. Phys. 2019, 91, 021001. [Google Scholar] [CrossRef] [Green Version]
- Lyu, C.; Choudhury, S.; Lv, C.; Yan, Y.; Zhou, Q. Eternal discrete time crystal beating the Heisenberg limit. Phys. Rev. Res. 2020, 2, 033070. [Google Scholar] [CrossRef]
- Sacha, K. Modeling spontaneous breaking of time-translation symmetry. Phys. Rev. A 2015, 91, 033617. [Google Scholar] [CrossRef] [Green Version]
- Else, D.V.; Bauer, B.; Nayak, C. Floquet time crystals. Phys. Rev. Lett. 2016, 117, 090402. [Google Scholar] [CrossRef] [Green Version]
- Khemani, V.; Lazarides, A.; Moessner, R.; Sondhi, S.L. Phase structure of driven quantum systems. Phys. Rev. Lett. 2016, 116, 250401. [Google Scholar] [CrossRef]
- Wilczek, F. Quantum time crystals. Phys. Rev. Lett. 2012, 109, 160401. [Google Scholar] [CrossRef] [Green Version]
- Li, T.; Gong, Z.-X.; Yin, Z.-Q.; Quan, H.T.; Yin, X.; Zhang, P.; Duan, L.-M.; Zhang, X. Space-time crystals of trapped ions. Phys. Rev. Lett. 2012, 109, 163001. [Google Scholar] [CrossRef] [Green Version]
- Huang, Y.; Li, T.; Yin, Z.-Q. Symmetry-breaking dynamics of the finite-size Lipkin-Meshkov-Glick model near ground state. Phys. Rev. A 2018, 97, 012115. [Google Scholar] [CrossRef] [Green Version]
- Huang, Y.; Guo, Q.; Xiong, A.; Li, T.; Yin, Z.-Q. Classical and quantum time crystals in a levitated nanoparticle without drive. Phys. Rev. A 2020, 102, 023113. [Google Scholar] [CrossRef]
- Sacha, K. Time Crystals; Springer International Publishing: Cham, Switzerland, 2020. [Google Scholar]
- Choi, S.; Choi, J.; Landig, R.; Kucsko, G.; Zhou, H.; Isoya, J.; Jelezko, F.; Onoda, S.; Sumiya, H.; Khemani, V.; et al. Observation of discrete time-crystalline order in a disordered dipolar many-body system. Nature 2017, 543, 221. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J.; Hess, P.W.; Kyprianidis, A.; Becker, P.; Lee, A.; Smith, J.; Pagano, G.; Potirniche, I.-D.; Potter, A.C.; Vishwanath, A.; et al. Observation of a discrete time crystal. Nature 2017, 543, 217. [Google Scholar] [CrossRef] [PubMed]
- Randall, J.; Bradley, C.E.; van der Gronden, F.V.; Galicia, A.; Abobeih, M.H.; Markham, M.; Twitchen, D.J.; Machado, F.; Yao, N.Y.; Taminiau, T.H. Many-body–localized discrete time crystal with a programmable spin-based quantum simulator. Science 2021, 374, 1474. [Google Scholar] [CrossRef] [PubMed]
- Kyprianidis, A.; Machado, F.; Morong, W.; Becker, P.; Collins, K.S.; Elsel, D.V.; Feng, L.; Hess, P.W.; Nayak, C.; Pagano, G.; et al. Observation of a prethermal discrete time crystal. Science 2021, 372, 1192. [Google Scholar] [CrossRef] [PubMed]
- von Keyserlingk, C.W.; Khemani, V.; Sondhi, S.L. Absolute stability and spatiotemporal long-range order in Floquet systems. Phys. Rev. B 2016, 94, 085112. [Google Scholar] [CrossRef] [Green Version]
- Yao, N.Y.; Potter, A.C.; Potirniche, I.-D.; Vishwanath, A. Discrete time crystals: Rigidity, criticality, and realizations. Phys. Rev. Lett. 2017, 118, 030401. [Google Scholar] [CrossRef]
- Lazarides, A.; Moessner, R. Fate of a discrete time crystal in an open system. Phys. Rev. B 2017, 95, 195135. [Google Scholar] [CrossRef] [Green Version]
- Else, D.V.; Bauer, B.; Nayak, C. Prethermal phases of matter protected by time-translation symmetry. Phys. Rev. X 2017, 7, 011026. [Google Scholar] [CrossRef] [Green Version]
- Gong, Z.; Hamazaki, R.; Ueda, M. Discrete time-crystalline order in cavity and circuit QED systems. Phys. Rev. Lett. 2018, 120, 040404. [Google Scholar] [CrossRef] [Green Version]
- Zhu, B.; Marino, J.; Yao, N.Y.; Lukin, M.D.; Demler, E.A. Dicke time crystals in driven-dissipative quantum many-body systems. New J. Phys. 2019, 21, 073028. [Google Scholar] [CrossRef]
- Keßler, H.; Kongkhambut, P.; Georges, C.; Mathey, M.; Cosme, J.G.; Hemmerich, A. Observation of a dissipative time crystal. Phys. Rev. Lett. 2021, 127, 043602. [Google Scholar] [CrossRef]
- Lazarides, A.; Roy, S.; Piazza, F.; Moessner, R. Time crystallinity in dissipative Floquet systems. Phys. Rev. Res. 2020, 2, 022002. [Google Scholar] [CrossRef] [Green Version]
- Riera-Campeny, A.; Moreno-Cardoner, M.; Sanpera, A. Time crystallinity in open quantum systems. Quantum 2020, 4, 270. [Google Scholar] [CrossRef]
- Fabre, C.; Pinard, M.; Bourzeix, S.; Heidmann, A.; Giacobino, E.; Reynaud, S. Quantum-noise reduction using a cavity with a movable mirror. Phys. Rev. A 1994, 49, 1337. [Google Scholar] [CrossRef] [PubMed]
- Mancini, S.; Tombesi, P. Quantum noise reduction by radiation pressure. Phys. Rev. A 1994, 49, 4055. [Google Scholar] [CrossRef] [PubMed]
- Aspelmeyer, M.; Kippenberg, T.J.; Marquardt, F. Cavity optomechanics. Rev. Mod. Phys. 2014, 86, 1391. [Google Scholar] [CrossRef]
- Yin, Z.; Zhao, N.; Li, T. Hybrid opto-mechanical systems with nitrogen-vacancy centers. Sci. China Phys. Mech. Astron. 2015, 58, 1. [Google Scholar] [CrossRef] [Green Version]
- Xu, Z.; Gao, X.; Bang, J.; Jacob, Z.; Li, T. Non-reciprocal energy transfer through the Casimir effect. Nat. Nanotechnol. 2021. [Google Scholar] [CrossRef]
- Chan, J.; Alegre, T.P.M.; Safavi-Naeini, A.H.; Hill, J.T.; Krause, A.; Gröblacher, S.; Aspelmeyer, M.; Painter, O. Laser cooling of a nanomechanical oscillator into its quantum ground state. Nature 2011, 478, 89. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.-c.; Hu, Y.-W.; Wong, C.W.; Xiao, Y.F. Review of cavity optomechanical cooling. Chin. Phys. B 2013, 22, 114213. [Google Scholar] [CrossRef] [Green Version]
- Xiong, H.; Liu, Z.X.; Wu, Y. Highly sensitive optical sensor for precision measurement of electrical charges based on optomechanically induced difference-sideband generation. Opt. Lett. 2017, 42, 3630. [Google Scholar] [CrossRef] [Green Version]
- Liu, Z.-X.; Wang, B.; Kong, C.; Si, L.-G.; Xiong, H.; Wu, Y. A proposed method to measure weak magnetic field based on a hybrid optomechanical system. Sci. Rep. 2017, 7, 12521. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Krause, A.G.; Winger, M.; Blasius, T.D.; Lin, Q.; Painter, O. A high-resolution microchip optomechanical accelerometer. Nat. Photonics 2012, 6, 768. [Google Scholar] [CrossRef] [Green Version]
- Ahn, J.; Xu, Z.; Bang, J.; Ju, P.; Gao, X.; Li, T. Ultrasensitive torque detection with an optically levitated nanorotor. Nat. Nanotechnol. 2020, 15, 89. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Li, B.-B.; Ou, L.; Lei, Y.; Liu, Y.-C. Cavity optomechanical sensing. Nanophotonics 2021, 10, 2799. [Google Scholar]
- Stannigel, K.; Rabl, P.; Sørensen, A.S.; Zoller, P.; Lukin, M.D. Optomechanical transducers for long-distance quantum communication. Phys. Rev. Lett. 2010, 105, 220501. [Google Scholar] [CrossRef] [Green Version]
- Stannigel, K.; Komar, P.; Habraken, S.J.M.; Bennett, S.D.; Lukin, M.D.; Zoller, P.; Rabl, P. Optomechanical quantum information processing with photons and phonons. Phys. Rev. Lett. 2012, 109, 013603. [Google Scholar] [CrossRef] [Green Version]
- Karg, T.M.; Gouraud, B.; Ngai, C.T.; Schmid, G.-L.; Hammerer, K.; Treutlein, P. Light-mediated strong coupling between a mechanical oscillator and atomic spins 1 meter apart. Science 2020, 369, 174. [Google Scholar] [CrossRef]
- Frisk Kockum, A.; Miranowicz, A.; De Liberato, S.; Savasta, S.; Nori, F. Ultrastrong coupling between light and matter. Nat. Rev. Phys. 2019, 1, 19. [Google Scholar]
- Akinwande, D.; Brennan, C.J.; Bunch, J.S.; Egberts, P.; Felts, J.R.; Gao, H.; Huang, R.; Kim, J.-S.; Li, T.; Li, Y.; et al. A Review on mechanics and mechanical properties of 2D materials–graphene and beyond. Extreme Mech. Lett. 2017, 13, 42. [Google Scholar]
- Tran, T.T.; Bray, K.; Ford, M.J.; Toth, M.; Aharonovich, I. Quantum emission from hexagonal Boron Nitride monolayers. Nat. Nanotechnol. 2016, 11, 37. [Google Scholar] [CrossRef]
- Liu, X.; Hersam, M.C. 2D materials for quantum information science. Nat. Rev. Mater. 2019, 4, 669. [Google Scholar]
- Xia, F.; Wang, H.; Xiao, D.; Dubey, M.; Ramasubramaniam, A. Two-dimensional material nanophotonics. Nat. Photonics 2014, 8, 899. [Google Scholar]
- Sławińska, J.; Zasada, I.; Kosiński, P.; Klusek, Z. Reversible modifications of linear dispersion: Graphene between boron nitride monolayers. Phys. Rev. B 2010, 82, 085431. [Google Scholar] [CrossRef] [Green Version]
- Cadiz, F.; Robert, C.; Courtade, E.; Manca, M.; Martinelli, L.; Taniguchi, T.; Watanabe, K.; Amand, T.; Rowe, A.C.H.; Paget, D.; et al. Exciton diffusion in WSe2 monolayers embedded in a van der Waals heterostructure. Appl. Phys. Lett. 2018, 112, 152106. [Google Scholar] [CrossRef] [Green Version]
- Tran, T.T.; Wang, D.; Xu, Z.-Q.; Yang, A.; Toth, M.; Odom, T.W.; Aharonovich, I. Deterministic coupling of quantum emitters in 2D materials to plasmonic nanocavity arrays. Nano Lett. 2017, 17, 2634. [Google Scholar] [CrossRef]
- Caldwell, J.D.; Aharonovich, I.; Cassabois, G.; Edgar, J.H.; Gil, B.; Basov, D. Photonics with hexagonal boron nitride. Nat. Rev. Mater. 2019, 4, 552. [Google Scholar]
- Gao, X.; Yin, Z.; Li, T. High-speed quantum transducer with a single-photon emitter in a 2D resonator. Annalen Physik 2020, 532, 2000233. [Google Scholar] [CrossRef]
- Wu, K.; Zhang, H.; Chen, Y.; Luo, K.; Xu, K. All-silicon microdisplay using efficient hot-carrier electroluminescence in standard 0.18 μm CMOS technology. IEEE Electron Device Lett. 2021, 42, 541. [Google Scholar] [CrossRef]
- Xu, K. Silicon electro-optic micro-modulator fabricated in standard CMOS technology as components for all silicon monolithic integrated optoelectronic systems. J. Micromech. Microeng. 2021, 31, 054001. [Google Scholar] [CrossRef]
- Kim, S.; Fröch, J.E.; Christian, J.; Straw, M.; Bishop, J.; Totonjian, D.; Watanabe, K.; Taniguchi, T.; Toth, M.; Aharonovich, I. Photonic crystal cavities from hexagonal Boron Nitride. Nat. Commun. 2018, 9, 2623. [Google Scholar] [CrossRef]
- Shandilya, P.K.; Fröch, J.E.; Mitchell, M.; Lake, D.P.; Kim, S.; Toth, M.; Behera, B.; Healey, C.; Aharonovich, I.; Barclay, P.E. Hexagonal Boron Nitride cavity optomechanics. Nano Lett. 2019, 19, 1343. [Google Scholar] [CrossRef] [PubMed]
- Abdi, M.; Hwang, M.-J.; Aghtar, M.; Plenio, M.B. Spin-mechanical scheme with color centers in hexagonal Boron Nitride membranes. Phys. Rev. Lett. 2017, 119, 233602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Abdi, M.; Plenio, M.B. Quantum effects in a mechanically modulated single-photon emitter. Phys. Rev. Lett. 2019, 122, 023602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gottscholl, A.; Kianinia, M.; Soltamov, V.; Orlinskii, S.; Mamin, G.; Bradac, C.; Kasper, C.; Krambrock, K.; Sperlich, A.; Toth, M.; et al. Initialization and read-out of intrinsic spin defects in a van der waals crystal at room temperature. Nat. Mater. 2020, 19, 540. [Google Scholar] [CrossRef]
- Chejanovsky, N.; Mukherjee, A.; Geng, J.; Chen, Y.-C.; Kim, Y.; Denisenko, A.; Finkler, A.; Taniguchi, T.; Watanabe, K.; Dasari, D.B.R.; et al. Single-spin resonance in a van der Waals embedded paramagnetic defect. Nat. Mater. 2021, 20, 1079. [Google Scholar] [CrossRef]
- Gao, X.; Jiang, B.; Allcca, A.E.L.; Shen, K.; Sadi, M.A.; Solanki, A.B.; Ju, P.; Xu, Z.; Upadhyaya, P.; Chen, Y.P.; et al. High-contrast plasmonic-enhanced shallow spin defects in hexagonal Boron Nitride for quantum sensing. Nano Lett. 2021, 21, 7708. [Google Scholar] [CrossRef]
- Li, B.; Li, X.; Li, P.; Li, T. Preparing squeezed spin states in a spin–mechanical hybrid system with silicon-vacancy centers. Adv. Quantum Technol. 2020, 3, 2000034. [Google Scholar] [CrossRef]
- Morrison, S.; Parkins, A.S. Dynamical quantum phase transitions in the dissipative Lipkin-Meshkov-Glick model with proposed realization in optical cavity QED. Phys. Rev. Lett. 2008, 100, 040403. [Google Scholar] [CrossRef] [Green Version]
- Russomanno, A.; Iemini, F.; Dalmonte, M.; Fazio, R. Floquet time crystal in the Lipkin-Meshkov-Glick model. Phys. Rev. B 2017, 95, 214307. [Google Scholar] [CrossRef] [Green Version]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, Z.; Gao, X.; Li, T. Stability of the Discrete Time-Crystalline Order in Spin-Optomechanical and Open Cavity QED Systems. Photonics 2022, 9, 61. https://doi.org/10.3390/photonics9020061
Hu Z, Gao X, Li T. Stability of the Discrete Time-Crystalline Order in Spin-Optomechanical and Open Cavity QED Systems. Photonics. 2022; 9(2):61. https://doi.org/10.3390/photonics9020061
Chicago/Turabian StyleHu, Zhengda, Xingyu Gao, and Tongcang Li. 2022. "Stability of the Discrete Time-Crystalline Order in Spin-Optomechanical and Open Cavity QED Systems" Photonics 9, no. 2: 61. https://doi.org/10.3390/photonics9020061
APA StyleHu, Z., Gao, X., & Li, T. (2022). Stability of the Discrete Time-Crystalline Order in Spin-Optomechanical and Open Cavity QED Systems. Photonics, 9(2), 61. https://doi.org/10.3390/photonics9020061





