Optical Frequency Comb-Based Direct Two-Photon Cooling for Cold Atom Clock
Abstract
:1. Introduction
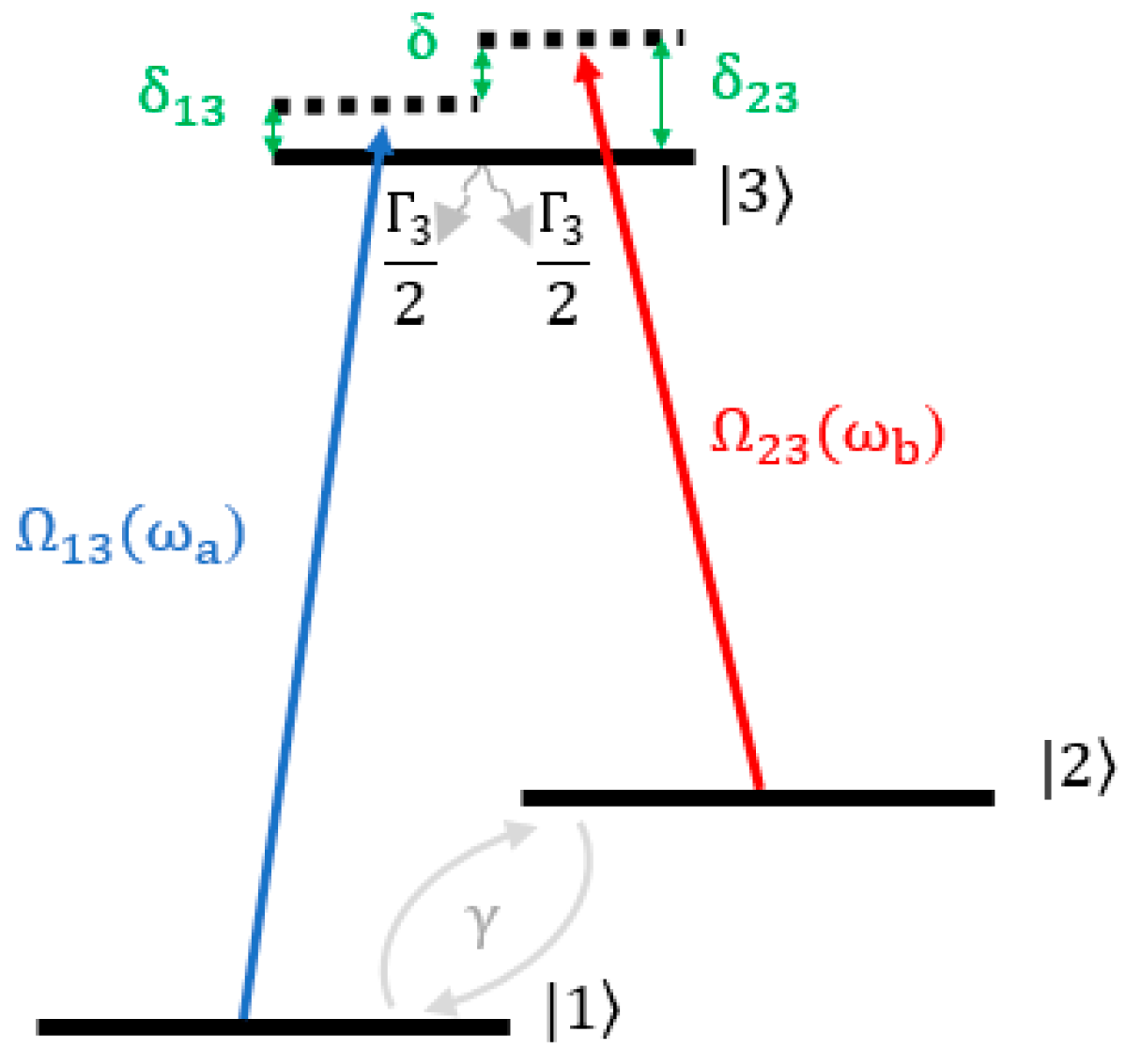
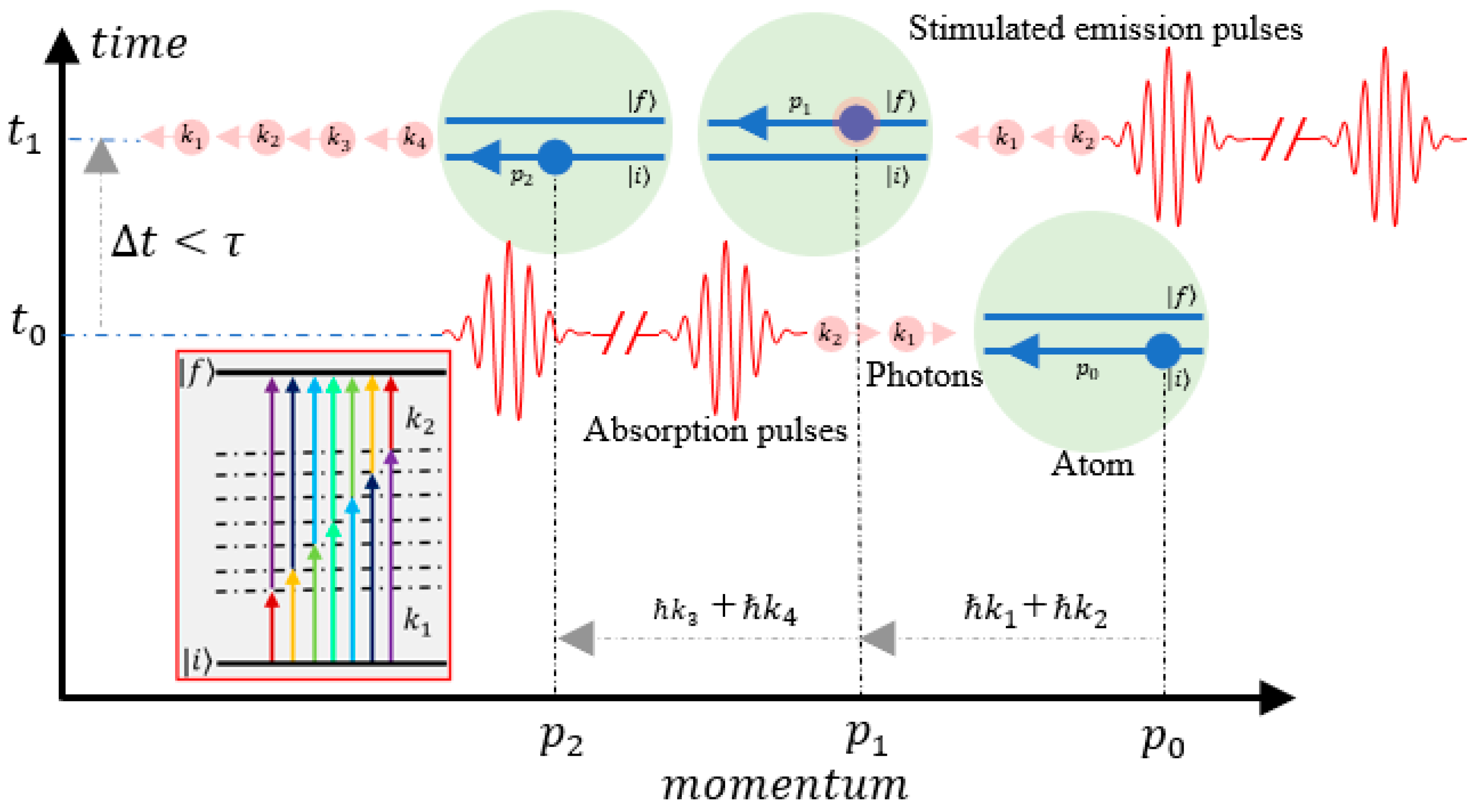
2. Two-Photon Cooling Model by Pulses
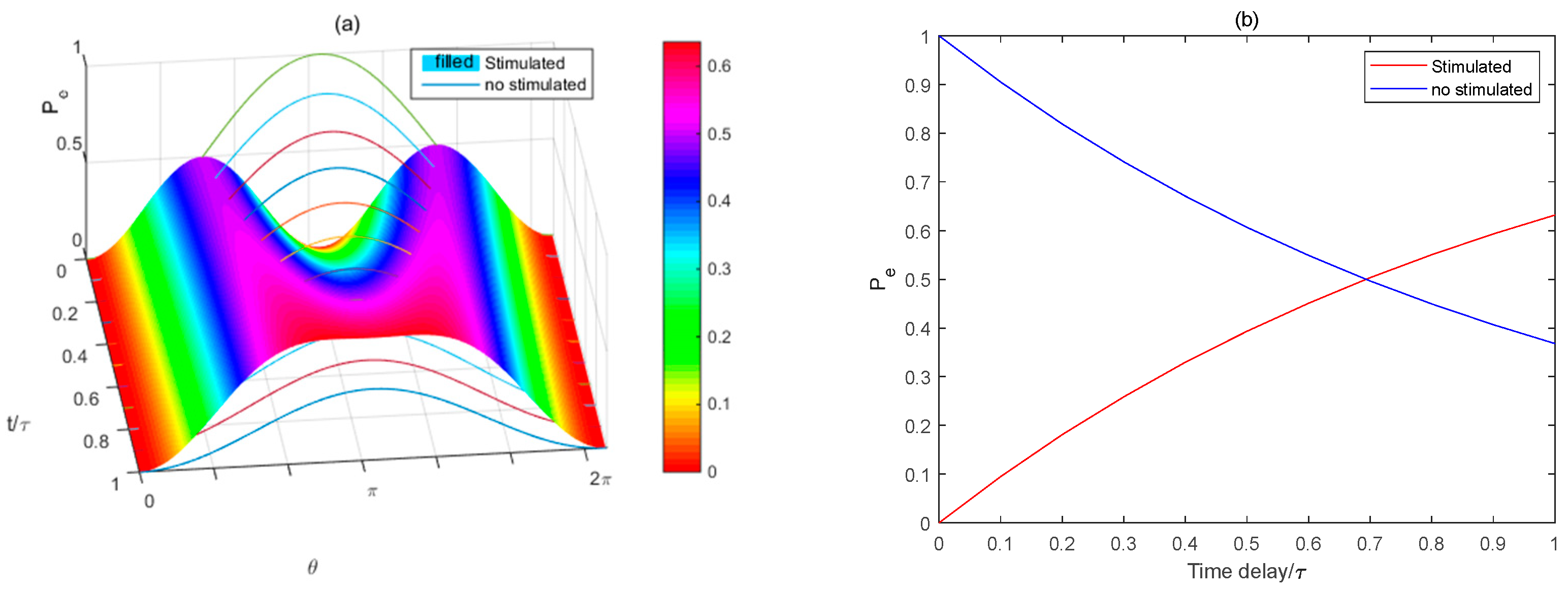
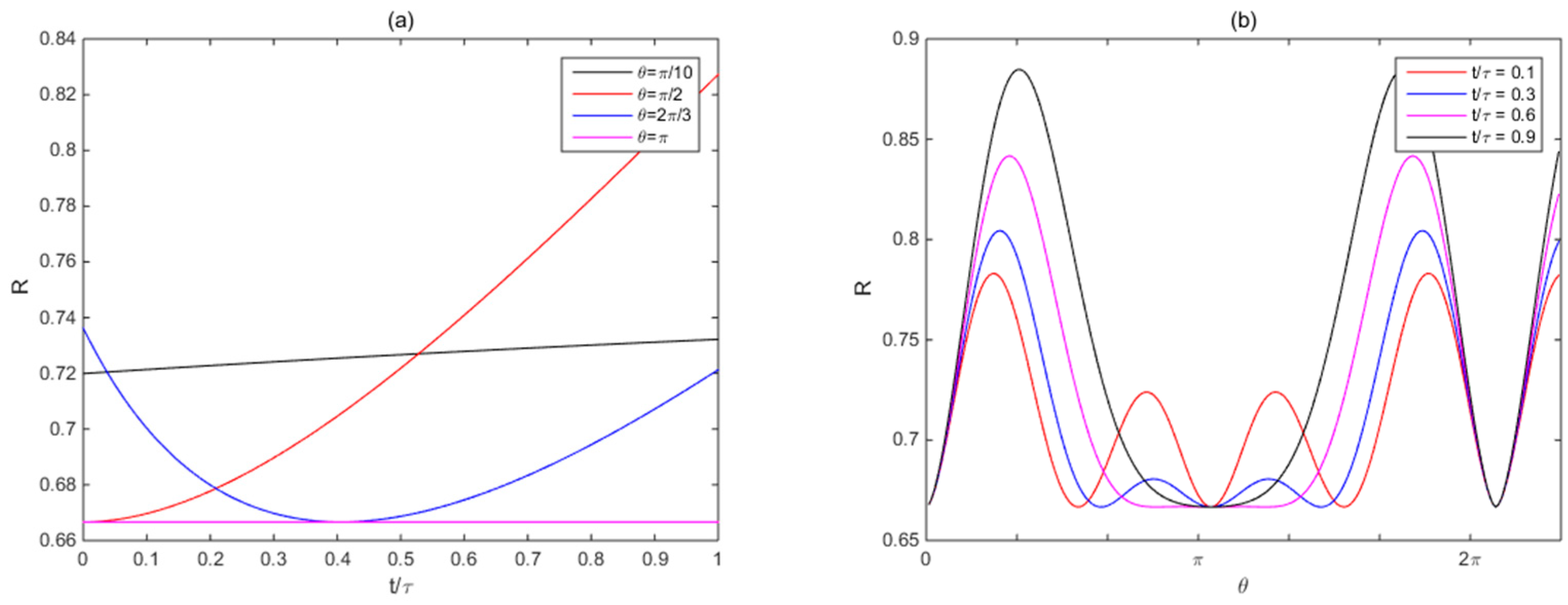
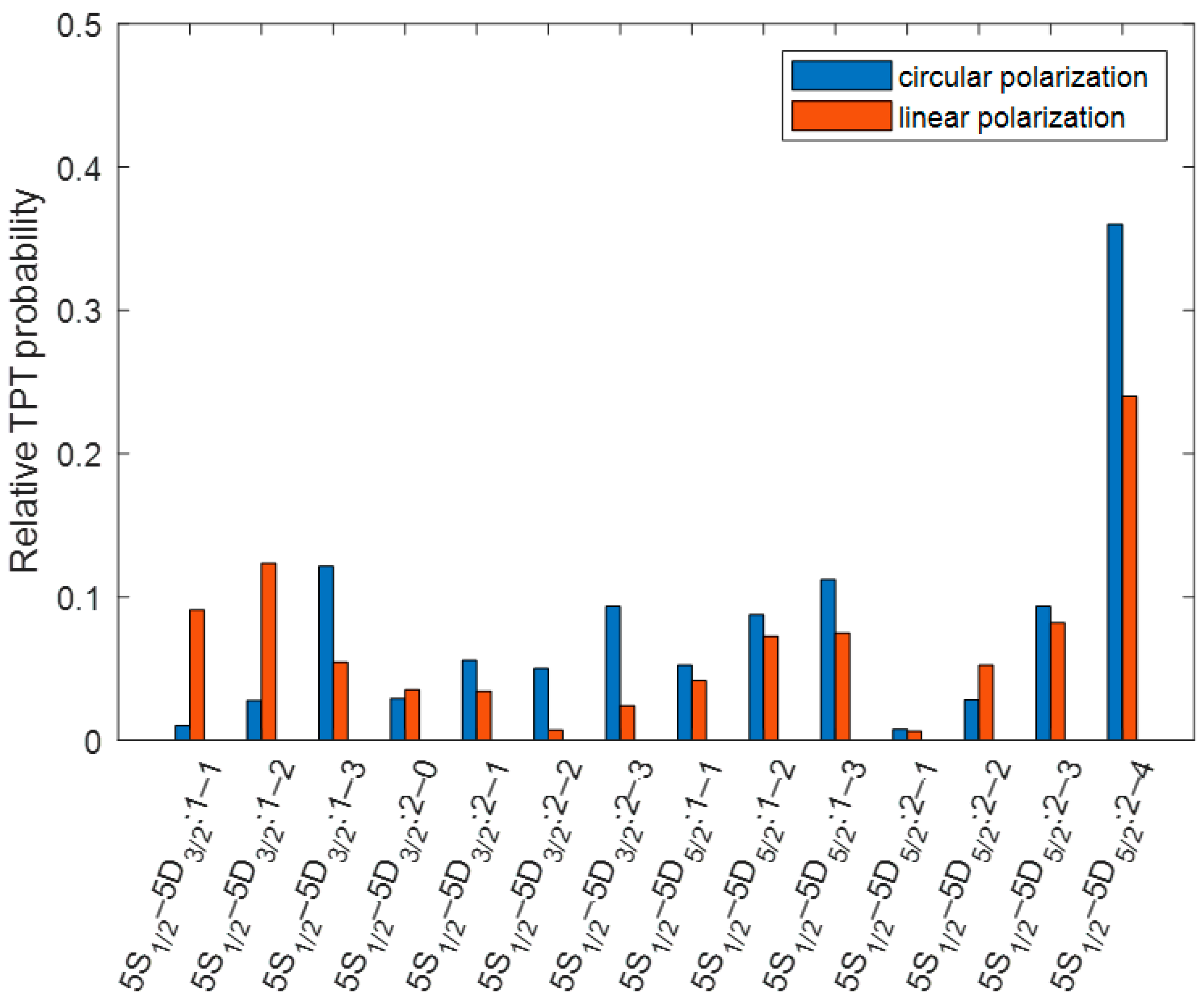
3. Results
4. Discussion and Application
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Vanier, J. Atomic clocks based on coherent population trapping: A review. Appl. Phys. B 2005, 81, 421–442. [Google Scholar] [CrossRef]
- Vanier, J.; Levine, M.W.; Kendig, S.; Janssen, D.; Everson, C.; Delaney, M.J. Practical realization of a passive coherent population trapping frequency standard. IEEE Trans. Instrum. Meas. 2005, 54, 2531–2539. [Google Scholar] [CrossRef]
- Wang, Z. Review of chip-scale atomic clocks based on coherent population trapping. Chin. Phys. B 2014, 23, 030601. [Google Scholar] [CrossRef]
- Jau, Y.Y.; Miron, E.; Post, A.B.; Kuzma, N.N.; Happer, W. Push-pull optical pumping of pure superposition states. Phys. Rev. Lett. 2004, 93, 160802. [Google Scholar] [CrossRef] [PubMed]
- Kargapoltsev, S.V.; Kitching, J.; Hollberg, L.; Taichenachev, A.V.; Velichansky, V.L.; Yudin, V.I. High-contrast dark resonance in sigma(+)-sigma(−) optical field. Laser Phys. Lett. 2004, 1, 495–499. [Google Scholar] [CrossRef] [Green Version]
- Taichenachev, A.V.; Yudin, V.I.; Velichansky, V.L.; Zibrov, S.A. On the unique possibility of significantly increasing the contrast of dark resonances on the D1 line of Rb-87. J. Exp. Lett. 2005, 82, 398–403. [Google Scholar] [CrossRef] [Green Version]
- Zanon, T.; Guerandel, S.; De Clercq, E.; Holleville, D.; Dimarcq, N.; Clairon, A. High contrast Ramsey fringes with coherent-population-trapping pulses in a double lambda atomic system. Phys. Rev. Lett. 2005, 94, 193002. [Google Scholar] [CrossRef]
- Knappe, S.; Shah, V.; Schwindt, P.D.D.; Hollberg, L.; Kitching, J.; Liew, L.-A.; Moreland, J. A microfabricated atomic clock. Appl. Phys. Lett. 2004, 85, 1460. [Google Scholar] [CrossRef] [Green Version]
- Knappe, S.; Gerginov, V.; Schwindt, P.D.D.; Shah, V.; Robinson, H.G.; Hollberg, L.; Kitching, J. Atomic vapor cells for chip-scale atomic clocks with improved long-term frequency stability. Opt. Express 2005, 30, 2351. [Google Scholar] [CrossRef] [Green Version]
- Datasheets of Microchips CSAC SA.45S. Available online: https://www.microchip.com/en-us/product/CSAC-SA65 (accessed on 22 February 2022).
- Yun, P.; Tricot, F.; Calosso, C.E.; Micalizio, S.; Francois, B.; Boudot, R.; Guerandel, S.; De Clercq, E. High-Performance Coherent Population Trapping Clock with Polarization Modulation. Phys. Rev. Appl. 2017, 7, 014018. [Google Scholar] [CrossRef] [Green Version]
- Boudot, R.; Guerandel, S.; De Clercq, E.; Dimarcq, N.; Clairon, A. Current Status of a Pulsed CPT Cs Cell Clock. IEEE Trans. Instrum. Meas. 2009, 58, 1217–1222. [Google Scholar] [CrossRef]
- Castagna, N.; Boudot, R.; Guerandel, S.; De Clercq, E.; Dimarcq, N.; Clairon, A. Investigations on Continuous and Pulsed Interrogation for a CPT Atomic Clock. IEEE Trans. Ultrason. Ferroelectr. 2009, 56, 246–253. [Google Scholar] [CrossRef] [PubMed]
- Esnault, F.X.; Blanshan, E.; Ivanov, E.N.; Scholten, R.E.; Kitching, J.; Donley, E.A. Cold-atom double-Λ coherent population trapping clock. Phy. Rev. A 2013, 88, 042120. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.; Ivanov, E.; Yudin, V.I.; Kitching, J.; Donley, E.A. Low-Drift Coherent Population Trapping Clock Based on Laser-Cooled Atoms and High-Coherence Excitation Fields. Phys. Rev. Appl. 2017, 8, 054001. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.; Yudin, V.I.; Taichenachev, A.V.; Kitching, J.; Donley, E.A. High contrast dark resonances in a cold-atom clock probed with counterpropagating circularly polarized beams. Appl. Phys. Lett. 2017, 111, 224102. [Google Scholar] [CrossRef]
- Moreno, M.P.; Nogueira, G.T.; Felinto, D.; Vianna, S.S. Sub-Doppler two-photon absorption induced by the combination of a single-mode laser and a frequency comb. Phys. Rev. A 2017, 96, 023430. [Google Scholar] [CrossRef] [Green Version]
- Malossi, N.; Damkjaer, S.; Hansen, P.L.; Jacobsen, L.B.; Kindt, L.; Sauge, S.; Thomsen, J.W.; Cruz, F.C.; Allegrini, M.; Arimondo, E. Two-photon cooling of magnesium atoms. Phys. Rev. A 2005, 72, 051403. [Google Scholar] [CrossRef] [Green Version]
- Magno, W.C.; Cavasso, R.L.; Cruz, F.C. Two-photon Doppler cooling of alkaline-earth-metal and ytterbium atoms. Phys. Rev. A 2003, 67, 043407. [Google Scholar] [CrossRef] [Green Version]
- Rooijakkers, W.; Hogervorst, W.; Vassen, W. 2-Color Excitation of Metastable Helium-Atoms for Efficient Laser Cooling. Phys. Rev. Lett. 1995, 74, 3348–3351. [Google Scholar] [CrossRef] [Green Version]
- Zehnle, V.; Garreau, J.C. Continuous-wave Doppler cooling of hydrogen atoms with two-photon transitions. Phys. Rev. A 2001, 63, 021402. [Google Scholar] [CrossRef] [Green Version]
- Marian, A.; Stowe, M.C.; Lawall, J.R.; Felinto, D.; Ye, J. United time-frequency spectroscopy for dynamics and global structure. Science 2004, 306, 2063–2068. [Google Scholar] [CrossRef] [PubMed]
- Rubiola, E.; Santarelli, G. The purest microwave oscillations. Nat. Photonics 2013, 7, 269–271. [Google Scholar] [CrossRef]
- Kocharovskaya, O.A.; Khanin, Y.I. Population trapping and coherent bleaching of a three-level medium by a periodic train of ultrashort pulses. Zh. Eksp. Teor. Fiz. 1986, 63, 945–950. [Google Scholar]
- Aumiler, D. Coherent population trapping in 87Rb atoms induced by the optical frequency comb excitation. Phys. Rev. A 2010, 82, 055402. [Google Scholar] [CrossRef]
- Malitskiy, R.A.; Khodakovskiy, V.M.; Negriyko, A.M.; Romanenko, V.I.; Matsnev, I.V.; Yatsenko, L.P. Magneto-optical CPT resonances in rubidium excited by femtosecond laser comb. In ICONO 2010: International Conference on Coherent and Nonlinear Optics; SPIE: Bellingham, WA, USA, 2011; Volume 7993, p. 799312. [Google Scholar]
- Leng, J.X.; Xu, H.; Lu, H.Y.; Fan, Y.Y.; Zhao, J.Y. Optical communication frequency standard using a fiber laser to excite cold rubidium two-photon transition. J. Opt. Soc. Am. B 2019, 36, 1183–1188. [Google Scholar] [CrossRef]
- Ban, T.; Aumiler, D.; Skenderovic, H.; Pichler, G. Mapping of the optical frequency comb to the atom-velocity comb. Phys. Rev. A 2006, 73, 043407. [Google Scholar] [CrossRef]
- Blinov, B.B.; Kohn, R.N.; Madsen, M.J.; Maunz, P.; Moehring, D.L.; Monroe, C. Broadband laser cooling of trapped atoms with ultrafast pulses. J. Opt. Soc. Am. B 2006, 23, 1170. [Google Scholar] [CrossRef] [Green Version]
- Wu, S.; Plisson, T.; Brown, R.C.; Phillips, W.D.; Porto, J.V. Multiphoton Magnetooptical Trap. Phys. Rev. Lett. 2009, 103, 173003. [Google Scholar] [CrossRef] [Green Version]
- Ilinova, E.; Ahmad, M.; Derevianko, A. Doppler cooling with coherent trains of laser pulses and a tunable velocity comb. Phys. Rev. A 2011, 84, 033421. [Google Scholar] [CrossRef] [Green Version]
- Aumiler, D.; Ban, T. Simultaneous laser cooling of multiple atomic species using an optical frequency comb. Phys. Rev. A 2012, 85, 063412. [Google Scholar] [CrossRef]
- Kregar, G.; Santic, N.; Aumiler, D.; Ban, T. Radiation pressure force on cold rubidium atoms due to excitation to a non-cooling hyperfine level. Eur. Phys. J. D 2014, 68, 360. [Google Scholar] [CrossRef]
- Kregar, G.; Santic, N.; Aumiler, D.; Buljan, H.; Ban, T. Frequency-comb-induced radiative force on cold rubidium atoms. Phys. Rev. A 2014, 89, 053421. [Google Scholar] [CrossRef] [Green Version]
- Davila-Rodriguez, J.; Ozawa, A.; Haensch, T.W.; Udem, T. Doppler Cooling Trapped Ions with a UV Frequency Comb. Phys. Rev. Lett. 2016, 116, 043002. [Google Scholar] [CrossRef] [PubMed]
- Šantić, N.; Buhin, D.; Kovačić, D.; Krešić, I.; Aumiler, D.; Ban, T. Cooling of atoms using an optical frequency comb. Sci. Rep. 2019, 9, 2510. [Google Scholar] [CrossRef] [Green Version]
- Buhin, D.; Kovacic, D.; Schmid, F.; Kruljac, M.; Vulic, V.; Ban, T.; Aumiler, D. Simultaneous dual-species laser cooling using an optical frequency comb. Phys. Rev. A 2020, 102, 021101. [Google Scholar] [CrossRef]
- Kielpinski, D. Laser cooling of atoms and molecules with ultrafast pulses. Phys. Rev. A 2006, 73, 063407. [Google Scholar] [CrossRef] [Green Version]
- Long, X.P.; Yu, S.S.; Jayich, A.M.; Campbell, W.C. Suppressed Spontaneous Emission for Coherent Momentum Transfer. Phys. Rev. Lett. 2019, 123, 033603. [Google Scholar] [CrossRef] [Green Version]
- Corder, C.; Arnold, B.; Metcalf, H. Laser Cooling without Spontaneous Emission. Phys. Rev. Lett. 2015, 114, 043002. [Google Scholar] [CrossRef] [Green Version]
- Kozyryev, I.; Baum, L.; Aldridge, L.; Yu, P.; Eyler, E.E.; Doyle, J.M. Coherent Bichromatic Force Deflection of Molecules. Phys. Rev. Lett. 2018, 120, 063205. [Google Scholar] [CrossRef] [Green Version]
- Jayich, A.M.; Vutha, A.C.; Hummon, M.T.; Porto, J.V.; Campbell, W.C. Continuous all-optical deceleration and single-photon cooling of molecular beams. Phys. Rev. A 2014, 89, 023425. [Google Scholar] [CrossRef] [Green Version]
- Ketterle, W.; Pritchard, D.E. Atom cooling by time-dependent potentials. Phys. Rev. A 1992, 46, 4051–4054. [Google Scholar] [CrossRef] [PubMed]
- Metcalf, H. Entropy exchange in laser cooling. Phys. Rev. A 2008, 77, 061401. [Google Scholar] [CrossRef] [Green Version]
- Jayich, A.M.; Long, X.; Campbell, W.C. Direct Frequency Comb Laser Cooling and Trapping. Phys. Rev. X 2016, 6, 041004. [Google Scholar] [CrossRef] [Green Version]
- Xu, H.; Lu, H.Y.; Li, Z.L.; Zhao, J.Y. Deep-ultraviolet femtosecond laser source at 243 nm for hydrogen spectroscopy. Opt. Express 2021, 29, 17398. [Google Scholar] [CrossRef]
- Moreno, M.P.; Vianna, S.S. Coherence induced by a train of ultrashort pulses in a Λ-type system. J. Opt. Soc. Am. B 2011, 28, 1124–1129. [Google Scholar] [CrossRef]

| Schemes | Linewidth/Contrast | |
|---|---|---|
| cw CPT cw CPT | 1.06 | |
| 0.70 | ||
| DTPT cooling + cw CPT DTPT cooling + ML CPT | 0.69 | |
| 0.11 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dan, L.; Xu, H.; Guo, P.; Zhao, J. Optical Frequency Comb-Based Direct Two-Photon Cooling for Cold Atom Clock. Photonics 2022, 9, 268. https://doi.org/10.3390/photonics9040268
Dan L, Xu H, Guo P, Zhao J. Optical Frequency Comb-Based Direct Two-Photon Cooling for Cold Atom Clock. Photonics. 2022; 9(4):268. https://doi.org/10.3390/photonics9040268
Chicago/Turabian StyleDan, Lin, Hao Xu, Ping Guo, and Jianye Zhao. 2022. "Optical Frequency Comb-Based Direct Two-Photon Cooling for Cold Atom Clock" Photonics 9, no. 4: 268. https://doi.org/10.3390/photonics9040268
APA StyleDan, L., Xu, H., Guo, P., & Zhao, J. (2022). Optical Frequency Comb-Based Direct Two-Photon Cooling for Cold Atom Clock. Photonics, 9(4), 268. https://doi.org/10.3390/photonics9040268





