Generation of 8–20 μm Mid-Infrared Ultrashort Femtosecond Laser Pulses via Difference Frequency Generation
Abstract
1. Introduction
1.1. Motivations for the Research of the Mid-Infrared Region
1.2. Methods for Mid-Infrared Generation Technology
2. Experimental Study of Long-Wavelength Mid-Infrared Laser Sources Based on DFG
3. Theoretical Study of the DFG for Mid-Infrared Generation
4. Feasibility of Mid-Infrared Power Scaling by Nonlinear-Amplification DFG
5. Methods for Measuring the Power of DFG-Based Mid-Infrared
6. Methods for Obtaining a High Beam Quality of DFG-Based Mid-Infrared
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ebrahim-Zadeh, M.; Sorokina, I.T. Mid-Infrared Coherent Sources and Applications: Preface; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2008; ISBN 9781402064395. [Google Scholar]
- Schliesser, A.; Picqué, N.; Hänsch, T.W. Mid-infrared frequency combs. Nat. Photonics 2012, 6, 440–449. [Google Scholar] [CrossRef]
- Pires, H.; Baudisch, M.; Sanchez, D.; Hemmer, M.; Biegert, J. Ultrashort pulse generation in the mid-IR. Prog. Quantum Electron. 2015, 43, 1–30. [Google Scholar] [CrossRef]
- Petrov, V. Frequency down-conversion of solid-state laser sources to the mid-infrared spectral range using non-oxide nonlinear crystals. Prog. Quantum Electron. 2015, 42, 1–106. [Google Scholar] [CrossRef]
- Vodopyanov, K.L. Laser-Based Mid-Infrared Sources and Applications; John Wiley & Sons: Hoboken, NJ, USA, 2020. [Google Scholar]
- Haas, J.; Mizaikoff, B. Advances in Mid-Infrared Spectroscopy for Chemical Analysis. Annu. Rev. Anal. Chem. 2016, 9, 45–68. [Google Scholar] [CrossRef] [PubMed]
- Popa, D.; Udrea, F. Towards integrated mid-infrared gas sensors. Sensors 2019, 19, 2076. [Google Scholar] [CrossRef] [PubMed]
- Du, Z.; Zhang, S.; Li, J.; Gao, N.; Tong, K. Mid-infrared tunable laser-based broadband fingerprint absorption spectroscopy for trace gas sensing: A review. Appl. Sci. 2019, 9, 338. [Google Scholar] [CrossRef]
- Seddon, A.B. Mid-infrared (IR)—A hot topic: The potential for using mid-IR light for non-invasive early detection of skin cancer in vivo. Phys. Status Solidi Basic Res. 2013, 250, 1020–1027. [Google Scholar] [CrossRef]
- Tseng, Y.-P.; Bouzy, P.; Pedersen, C.; Stone, N.; Tidemand-Lichtenberg, P. Upconversion raster scanning microscope for long-wavelength infrared imaging of breast cancer microcalcifications. Biomed. Opt. Express 2018, 9, 4979–4987. [Google Scholar] [CrossRef]
- Ma, P.; Li, J.; Chen, Y.; Zhou Montano, B.A.; Luo, H.; Zhang, D.; Zheng, H.; Liu, Y.; Lin, H.; Zhu, W.; et al. Non-invasive exhaled breath diagnostic and monitoring technologies. Microw. Opt. Technol. Lett. 2021, 1–14. [Google Scholar] [CrossRef]
- Pejcic, B.; Myers, M.; Ross, A. Mid-infrared sensing of organic pollutants in aqueous environments. Sensors 2009, 9, 6232–6253. [Google Scholar] [CrossRef]
- Siciliani de Cumis, M.; Viciani, S.; Borri, S.; Patimisco, P.; Sampaolo, A.; Scamarcio, G.; De Natale, P.; D’Amato, F.; Spagnolo, V. Widely-tunable mid-infrared fiber-coupled quartz-enhanced photoacoustic sensor for environmental monitoring. Opt. Express 2014, 22, 28222–28231. [Google Scholar] [CrossRef] [PubMed]
- Baudet, E.; Gutierrez-Arroyo, A.; Němec, P.; Bodiou, L.; Lemaitre, J.; De Sagazan, O.; Lhermitte, H.; Rinnert, E.; Michel, K.; Bureau, B.; et al. Selenide sputtered films development for MIR environmental sensor. Opt. Mater. Express 2016, 6, 2616–2627. [Google Scholar] [CrossRef]
- Dong, X.; Jochmann, M.A.; Elsner, M.; Meyer, A.H.; Bäcker, L.E.; Rahmatullah, M.; Schunk, D.; Lens, G.; Meckenstock, R.U. Monitoring Microbial Mineralization Using Reverse Stable Isotope Labeling Analysis by Mid-Infrared Laser Spectroscopy. Environ. Sci. Technol. 2017, 51, 11876–11883. [Google Scholar] [CrossRef] [PubMed]
- Schulte, S.M.; Köster, D.; Jochmann, M.A.; Meckenstock, R.U. Applying reverse stable isotope labeling analysis by mid-infrared laser spectroscopy to monitor BDOC in recycled wastewater. Sci. Total Environ. 2019, 665, 1064–1072. [Google Scholar] [CrossRef] [PubMed]
- Wang, T.; Rodriguez-Saona, L.E. Rapid Determination of Sugar Level in Snack Products Using Infrared Spectroscopy. J. Food Sci. 2012, 77, C874–C879. [Google Scholar] [CrossRef] [PubMed]
- Maurice-Van Eijndhoven, M.H.T.; Soyeurt, H.; Dehareng, F.; Calus, M.P.L. Validation of fatty acid predictions in milk using mid-infrared spectrometry across cattle breeds. Animal 2013, 7, 348–354. [Google Scholar] [CrossRef]
- Li, X.; Huo, G.; Wang, Y.; Sun, H.; Kong, Q. Research on rapid detection method of protein and fat in raw milk based on mid-infrared spectrum. Int. J. Multimed. Ubiquitous Eng. 2016, 11, 131–142. [Google Scholar] [CrossRef]
- Ferreira, D.S.; Galão, O.F.; Pallone, J.A.L.; Poppi, R.J. Comparison and application of near-infrared (NIR) and mid-infrared (MIR) spectroscopy for determination of quality parameters in soybean samples. Food Control 2014, 35, 227–232. [Google Scholar] [CrossRef]
- Cocchi, M.; Foca, G.; Lucisano, M.; Marchetti, A.; Pagani, M.A.; Tassi, L.; Ulrici, A. Classification of Cereal Flours by Chemometric Analysis of MIR Spectra. J. Agric. Food Chem. 2004, 52, 1062–1067. [Google Scholar] [CrossRef]
- López-Lorente, Á.I.; Mizaikoff, B. Mid-infrared spectroscopy for protein analysis: Potential and challenges. Anal. Bioanal. Chem. 2016, 408, 2875–2889. [Google Scholar] [CrossRef]
- Dabrowska, A.; David, M.; Freitag, S.; Andrews, A.M.; Strasser, G.; Hinkov, B.; Schwaighofer, A.; Lendl, B. Broadband laser-based mid-infrared spectroscopy employing a quantum cascade detector for milk protein analysis. Sens. Actuators B Chem. 2022, 350, 130873. [Google Scholar] [CrossRef]
- Borri, S.; Santambrogio, G. Laser spectroscopy of cold molecules. Adv. Phys. X 2016, 1, 368–386. [Google Scholar] [CrossRef]
- Kowligy, A.S.; Timmers, H.; Lind, A.J.; Elu, U.; Cruz, F.C.; Schunemann, P.G.; Biegert, J.; Diddams, S.A. Infrared electric field sampled frequency comb spectroscopy. Sci. Adv. 2019, 5, 36–38. [Google Scholar] [CrossRef] [PubMed]
- Etezadi, D.; Warner, J.B.; Ruggeri, F.S.; Dietler, G.; Lashuel, H.A.; Altug, H. Nanoplasmonic mid-infrared biosensor for in vitro protein secondary structure detection. Light Sci. Appl. 2017, 6, e17029. [Google Scholar] [CrossRef] [PubMed]
- Rodrigo, D.; Limaj, O.; Janner, D.; Etezadi, D.; García De Abajo, F.J.; Pruneri, V.; Altug, H. Mid-infrared plasmonic biosensing with graphene. Science 2015, 349, 165–168. [Google Scholar] [CrossRef]
- Akin, B.; Linford, M.R.; Ahmadivand, A.; Altindal, S. All-Dielectric Fabry–Pérot Cavity Design for Spectrally Selective Mid-Infrared Absorption. Phys. Status Solidi Basic Res. 2021, 2100464, 1–7. [Google Scholar] [CrossRef]
- Sheehy, B.; Martin, J.D.D.; Di Mauro, L.F.; Agostini, P.; Schafer, K.J.; Gaarde, M.B.; Kulander, K.C. High harmonic generation at long wavelengths. Phys. Rev. Lett. 1999, 83, 5270–5273. [Google Scholar] [CrossRef]
- Günter, G.; Anappara, A.A.; Hees, J.; Sell, A.; Biasiol, G.; Sorba, L.; De Liberato, S.; Ciuti, C.; Tredicucci, A.; Leitenstorfer, A.; et al. Sub-cycle switch-on of ultrastrong light-matter interaction. Nature 2009, 458, 178–181. [Google Scholar] [CrossRef]
- Dura, J.; Camus, N.; Thai, A.; Britz, A.; Hemmer, M.; Baudisch, M.; Senftleben, A.; Schröter, C.D.; Ullrich, J.; Moshammer, R.; et al. Ionization with low-frequency fields in the tunneling regime. Sci. Rep. 2013, 3, 2675. [Google Scholar] [CrossRef]
- Wolter, B.; Pullen, M.G.; Baudisch, M.; Sclafani, M.; Hemmer, M.; Senftleben, A.; Schröter, C.D.; Ullrich, J.; Moshammer, R.; Biegert, J. Strong-field physics with Mid-IR fields. Phys. Rev. X 2015, 5, 021034. [Google Scholar] [CrossRef]
- Panagiotopoulos, P.; Whalen, P.; Kolesik, M.; Moloney, J.V. Super high power mid-infrared femtosecond light bullet. Nat. Photonics 2015, 9, 543–548. [Google Scholar] [CrossRef]
- Woodbury, D.; Feder, L.; Shumakova, V.; Gollner, C.; Miao, B.; Schwartz, R.; Pugžlys, A.; Baltuška, A.; Milchberg, H.M. Laser wakefield acceleration with mid-IR laser pulses. Opt. InfoBase Conf. Pap. 2017, 43, 1131–1134. [Google Scholar]
- Xiao, Y.; Guo, M.; Parker, K.; Hutson, M.S. Wavelength-dependent collagen fragmentation during Mid-IR laser ablation. Biophys. J. 2006, 91, 1424–1432. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Zavalin, A.; Hachey, D.L.; Sundaramoorthy, M.; Banerjee, S.; Morgan, S.; Feldman, L.; Tolk, N.; Piston, D.W. Kinetics of a collagen-like polypeptide fragmentation after mid-IR free-electron laser ablation. Biophys. J. 2008, 95, 1371–1381. [Google Scholar] [CrossRef]
- Edwards, G.S. Mechanisms for soft-tissue ablation and the development of alternative medical lasers based on investigations with mid-infrared free-electron lasers. Laser Photonics Rev. 2009, 3, 545–555. [Google Scholar] [CrossRef]
- Serebryakov, V.S.; Bo, É.V.; Kalintsev, A.G.; Kornev, A.F.; Narivonchik, A.S. Mid-IR laser for high-precision surgery. J. Opt. Technol. 2015, 82, 781–788. [Google Scholar] [CrossRef]
- Serebryakov, V.A.; Narivonchik, A.S.; Kalintseva, N.A.; Skvortsov, D.V.; Doroganov, S.V. Repetitively-pulsed mid-IR laser for precise microsurgery. Proc.-Int. Conf. Laser Opt. 2018, 788, 479. [Google Scholar]
- Toor, F.; Jackson, S.; Shang, X.; Arafin, S.; Yang, H. Mid-infrared Lasers for Medical Applications: Introduction to the feature issue. Biomed. Opt. Express 2018, 9, 6255–6257. [Google Scholar] [CrossRef]
- Larson, E.; Hines, M.; Tanas, M.; Miller, B.; Coleman, M.; Toor, F. Mid-infrared absorption by soft tissue sarcoma and cell ablation utilizing a mid-infrared interband cascade laser. J. Biomed. Opt. 2021, 26, 043012. [Google Scholar] [CrossRef]
- Popmintchev, T.; Chen, M.C.; Popmintchev, D.; Arpin, P.; Brown, S.; Ališauskas, S.; Andriukaitis, G.; Balčiunas, T.; Mücke, O.D.; Pugzlys, A.; et al. Bright coherent ultrahigh harmonics in the kev x-ray regime from mid-infrared femtosecond lasers. Science 2012, 336, 1287–1291. [Google Scholar] [CrossRef]
- Chini, M.; Zhao, K.; Chang, Z. The generation, characterization and applications of broadband isolated attosecond pulses. Nat. Photonics 2014, 8, 178–186. [Google Scholar] [CrossRef]
- Wagner, M.; Fei, Z.; McLeod, A.S.; Rodin, A.S.; Bao, W.; Iwinski, E.G.; Zhao, Z.; Goldflam, M.; Liu, M.; Dominguez, G.; et al. Ultrafast and nanoscale plasmonic phenomena in exfoliated graphene revealed by infrared pump-probe nanoscopy. Nano Lett. 2014, 14, 894–900. [Google Scholar] [CrossRef] [PubMed]
- Weichman, M.L.; Changala, P.B.; Ye, J.; Chen, Z.; Yan, M.; Picqué, N. Broadband molecular spectroscopy with optical frequency combs. J. Mol. Spectrosc. 2019, 355, 66–78. [Google Scholar] [CrossRef]
- Aerts, A.; Kockaert, P.; Gorza, S.-P.; Brown, A.; Vander Auwera, J.; Vaeck, N. Laser control of a dark vibrational state of acetylene in the gas phase—Fourier transform pulse shaping constraints and effects of decoherence. J. Chem. Phys. 2022, 156, 084302. [Google Scholar] [CrossRef] [PubMed]
- Shea, P.G.O.; Freund, H.P. Free-Electron Lasers: Status and Applications. Science 2001, 292, 1853–1859. [Google Scholar] [CrossRef]
- Barletta, W.A.; Bisognano, J.; Corlett, J.N.; Emma, P.; Huang, Z.; Kim, K.J.; Lindberg, R.; Murphy, J.B.; Neil, G.R.; Nguyen, D.C.; et al. Free electron lasers: Present status and future challenges. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2010, 618, 69–96. [Google Scholar] [CrossRef]
- Hillbrand, J.; Opačak, N.; Piccardo, M.; Schneider, H.; Strasser, G.; Capasso, F.; Schwarz, B. Mode-locked short pulses from an 8 μm wavelength semiconductor laser. Nat. Commun. 2020, 11, 5788. [Google Scholar] [CrossRef]
- Täschler, P.; Bertrand, M.; Schneider, B.; Singleton, M.; Jouy, P.; Kapsalidis, F.; Beck, M.; Faist, J. Femtosecond pulses from a mid-infrared quantum cascade laser. Nat. Photonics 2021, 15, 919–924. [Google Scholar] [CrossRef]
- Seidel, M. A New Generation of High-Power, Waveform Controlled, Few-Cycle Light Sources; Springer: Berlin/Heidelberg, Germany, 2019. [Google Scholar]
- Sylvestre, T.; Genier, E.; Ghosh, A.N.; Bowen, P.; Genty, G.; Troles, J.; Mussot, A.; Peacock, A.C.; Klimczak, M.; Heidt, A.M.; et al. Recent advances in supercontinuum generation in specialty optical fibers [Invited]. J. Opt. Soc. Am. B 2021, 38, F90–F103. [Google Scholar] [CrossRef]
- Saini, T.S.; Sinha, R.K. Mid-infrared supercontinuum generation in soft-glass specialty optical fibers: A review. Prog. Quantum Electron. 2021, 78, 100342. [Google Scholar] [CrossRef]
- Maidment, L.; Schunemann, P.G.; Reid, D.T. Molecular fingerprint-region spectroscopy from 5 to 12 μm using an orientation-patterned gallium phosphide optical parametric oscillator. Opt. Lett. 2016, 41, 4261–4264. [Google Scholar] [CrossRef] [PubMed]
- Iwakuni, K.; Porat, G.; Bui, T.Q.; Bjork, B.J.; Schoun, S.B.; Heckl, O.H.; Fermann, M.E.; Ye, J. Phase-stabilized 100 mW frequency comb near 10 μm. Appl. Phys. B Lasers Opt. 2018, 124, 128. [Google Scholar] [CrossRef] [PubMed]
- Maidment, L.; Kara, O.; Schunemann, P.G.; Piper, J.; McEwan, K.; Reid, D.T. Long-wave infrared generation from femtosecond and picosecond optical parametric oscillators based on orientation-patterned gallium phosphide. Appl. Phys. B Lasers Opt. 2018, 124, 143. [Google Scholar] [CrossRef]
- Junginger, F.; Sell, A.; Schubert, O.; Mayer, B.; Brida, D.; Marangoni, M.; Cerullo, G.; Leitenstorfer, A.; Huber, R. Single-cycle multiterahertz transients with peak fields above 10 MV/cm. Opt. Lett. 2010, 35, 2645–2647. [Google Scholar] [CrossRef]
- Krauth, J.; Steinmann, A.; Hegenbarth, R.; Conforti, M.; Giessen, H. Broadly tunable femtosecond near- and mid-IR source by direct pumping of an OPA with a 417 MHz Yb:KGW oscillator. Opt. Express 2013, 21, 11516–11522. [Google Scholar] [CrossRef]
- Namboodiri, M.; Golz, T.; Bus, J.H.; Schulz, M.; Riedel, R.; Laarmann, T.; Prandolini, M.J. Review of thermal parameters of Li-based nonlinear crystals for high power 8 µm sources. In Proceedings of the Conference on Lasers and Electro-Optics Science and Innovations 2021, San Jose, CA, USA, 9–14 May 2021; pp. 8–9. [Google Scholar]
- Heiner, Z.; Petrov, V.; Panyutin, V.L.; Badikov, V.V.; Kato, K.; Miyata, K.; Mero, M. Efficient generation of few-cycle pulses beyond 10 μm from an optical parametric amplifier pumped by a 1-µm laser system. Sci. Rep. 2022, 12, 5082. [Google Scholar] [CrossRef]
- Sanchez, D.; Hemmer, M.; Baudisch, M.; Cousin, S.L.; Zawilski, K.; Schunemann, P.; Chalus, O.; Simon-Boisson, C.; Biegert, J. 7 μm, ultrafast, sub-millijoule-level mid-infrared optical parametric chirped pulse amplifier pumped at 2 μm. Optica 2016, 3, 147–150. [Google Scholar] [CrossRef]
- Qu, S.; Liang, H.; Liu, K.; Zou, X.; Li, W.; Wang, Q.J.; Zhang, Y. 9 μm few-cycle optical parametric chirped-pulse amplifier based on LiGaS2. Opt. Lett. 2019, 44, 2422–2425. [Google Scholar] [CrossRef]
- Elu, U.; Steinle, T.; Sánchez, D.; Maidment, L.; Zawilski, K.; Schunemann, P.; Zeitner, U.D.; Simon-Boisson, C.; Biegert, J. Table-top high-energy 7 μm OPCPA and 260 mJ Ho:YLF pump laser. Opt. Lett. 2019, 44, 3194–3197. [Google Scholar] [CrossRef]
- Liang, H.; Krogen, P.; Wang, Z.; Park, H.; Kroh, T.; Zawilski, K.; Schunemann, P.; Moses, J.; Dimauro, L.F.; Kärtner, F.X.; et al. High-energy mid-infrared sub-cycle pulse synthesis from a parametric amplifier. Nat. Commun. 2017, 8, 141. [Google Scholar] [CrossRef]
- Seidel, M.; Xiao, X.; Hussain, S.A.; Arisholm, G.; Hartung, A.; Zawilski, K.T.; Schunemann, P.G.; Habel, F.; Trubetskov, M.; Pervak, V.; et al. Multi-watt, multi-octave, mid-infrared femtosecond source. Sci. Adv. 2018, 4, eaaq1526. [Google Scholar] [CrossRef] [PubMed]
- Penwell, S.B.; Whaley-Mayda, L.; Tokmakoff, A. Single-stage MHz mid-IR OPA using LiGaS2 and a fiber laser pump source. Opt. Lett. 2018, 43, 1363–1366. [Google Scholar] [CrossRef] [PubMed]
- Liu, K.; Liang, H.; Wang, L.; Qu, S.; Lang, T.; Li, H.; Wang, Q.J.; Zhang, Y. Multimicrojoule GaSe-based midinfrared optical parametric amplifier with an ultrabroad idler spectrum covering 4.2–16 μm. Opt. Lett. 2019, 44, 1003–1006. [Google Scholar] [CrossRef] [PubMed]
- Jakob, M.A.; Namboodiri, M.; Prandolini, M.J.; Laarmann, T. Generation and characterization of tailored MIR waveforms for steering molecular dynamics. Opt. Express 2019, 27, 26979–26987. [Google Scholar] [CrossRef]
- Chen, B.-H.; Wittmann, E.; Morimoto, Y.; Baum, P.; Riedle, E. Octave-spanning single-cycle middle-infrared generation through optical parametric amplification in LiGaS2. Opt. Express 2019, 27, 21306–21316. [Google Scholar] [CrossRef]
- Heiner, Z.; Petrov, V.; Mero, M. Efficient, sub-4-cycle, 1-µm-pumped optical parametric amplifier at 10 µm based on BaGa4S7. Opt. Lett. 2020, 45, 5692–5695. [Google Scholar] [CrossRef]
- Budriūnas, R.; Jurkus, K.; Varanavičius, A. Yb-laser-based sub-60 fs mid-infrared source tunable from 2.5 μm to 10 μm. Opt. InfoBase Conf. Pap. 2021, 202, 10233. [Google Scholar]
- Petersen, C.R.; Møller, U.; Kubat, I.; Zhou, B.; Dupont, S.; Ramsay, J.; Benson, T.; Sujecki, S.; Abdel-Moneim, N.; Tang, Z.; et al. Mid-infrared supercontinuum covering the 1.4–13.3 μm molecular fingerprint region using ultra-high NA chalcogenide step-index fibre. Nat. Photonics 2014, 8, 830–834. [Google Scholar] [CrossRef]
- Yu, Y.; Zhang, B.; Gai, X.; Zhai, C.; Qi, S.; Guo, W.; Yang, Z.; Wang, R.; Choi, D.-Y.; Madden, S.; et al. 1.8–10 μm Mid-Infrared Supercontinuum Generated in a Step-Index Chalcogenide Fiber Using Low Peak Pump Power. Opt. Lett. 2015, 40, 1081–1084. [Google Scholar] [CrossRef]
- Guo, K.; Martinez, R.A.; Plant, G.; Maksymiuk, L.; Janiszewski, B.; Freeman, M.J.; Maynard, R.L.; Islam, M.N.; Terry, F.L.; Bedford, R.; et al. Generation of near-diffraction-limited, high-power supercontinuum from 1.57 μm to 12 μm with cascaded fluoride and chalcogenide fibers. Appl. Opt. 2018, 57, 2519–2532. [Google Scholar] [CrossRef]
- Martinez, R.A.; Plant, G.; Guo, K.; Janiszewski, B.; Freeman, M.J.; Maynard, R.L.; Islam, M.N.; Terry, F.L.; Alvarez, O.; Chenard, F.; et al. Mid-infrared supercontinuum generation from 1.6 to >11 μm using concatenated step-index fluoride and chalcogenide fibers. Opt. Lett. 2018, 43, 296–299. [Google Scholar] [CrossRef] [PubMed]
- Wu, Z.; Yang, L.; Xu, Y.; Zhang, P.; Nie, Q.; Wang, X. 1.8–13 μm supercontinuum generation by pumping at normal dispersion regime of As–Se–Te glass fiber. J. Am. Ceram. Soc. 2019, 102, 5025–5032. [Google Scholar] [CrossRef]
- Butler, T.P.; Lilienfein, N.; Xu, J.; Nagl, N.; Hofer, C.; Gerz, D.; Mak, K.F.; Gaida, C.; Heuermann, T.; Gebhardt, M.; et al. Multi-octave spanning, Watt-level ultrafast mid-infrared source. J. Phys. Photonics 2019, 1, 044006. [Google Scholar] [CrossRef]
- Li, G.; Peng, X.; Dai, S.; Wang, Y.; Xie, M.; Yang, L.; Yang, C.; Wei, W.; Zhang, P. Highly coherent 1.5–8.3 μm broadband supercontinuum generation in tapered As—S chalcogenide fibers. J. Lightwave Technol. 2019, 37, 1847–1852. [Google Scholar] [CrossRef]
- Lemière, A.; Désévédavy, F.; Mathey, P.; Froidevaux, P.; Gadret, G.; Jules, J.-C.; Aquilina, C.; Kibler, B.; Béjot, P.; Billard, F.; et al. Mid-infrared supercontinuum generation from 2 to 14 μm in arsenic- and antimony-free chalcogenide glass fibers. J. Opt. Soc. Am. B 2019, 36, A183–A192. [Google Scholar] [CrossRef]
- Jiao, K.; Yao, J.; Wang, X.; Wang, X.; Zhao, Z.; Zhang, B.; Si, N.; Liu, J.; Shen, X.; Zhang, P.; et al. 1.2–15.2 μm Supercontinuum Generation in a Low-Loss Chalcohalide Fiber Pumped At a Deep Anomalous-Dispersion Region. Opt. Lett. 2019, 44, 5545–5548. [Google Scholar] [CrossRef]
- Jiao, K.; Yao, J.; Zhao, Z.; Wang, X.; Si, N.; Wang, X.; Chen, P.; Xue, Z.; Tian, Y.; Zhang, B.; et al. Mid-infrared flattened supercontinuum generation in all-normal dispersion tellurium chalcogenide fiber. Opt. Express 2019, 27, 2036–2043. [Google Scholar] [CrossRef]
- Montesinos-Ballester, M.; Lafforgue, C.; Frigerio, J.; Ballabio, A.; Vakarin, V.; Liu, Q.; Ramirez, J.M.; Roux, X.L.; Bouville, D.; Barzaghi, A.; et al. On-Chip Mid-Infrared Supercontinuum Generation from 3 to 13 μm Wavelength. ACS Photonics 2020, 7, 3423–3429. [Google Scholar] [CrossRef]
- Qu, S.; Chaudhary Nagar, G.; Li, W.; Liu, K.; Zou, X.; Hon Luen, S.; Dempsey, D.; Hong, K.-H.; Jie Wang, Q.; Zhang, Y.; et al. Long-wavelength-infrared laser filamentation in solids in the near-single-cycle regime. Opt. Lett. 2020, 45, 2175–2178. [Google Scholar] [CrossRef]
- Zhang, B.; Yu, Y.; Zhai, C.; Qi, S.; Wang, Y.; Yang, A.; Gai, X.; Wang, R.; Yang, Z.; Luther-Davies, B.; et al. High Brightness 2.2–12 μm Mid-Infrared Supercontinuum Generation in a Nontoxic Chalcogenide Step-Index Fiber. J. Am. Ceram. Soc. 2016, 99, 2565–2568. [Google Scholar] [CrossRef]
- Venck, S.; St-Hilaire, F.; Brilland, L.; Ghosh, A.N.; Chahal, R.; Caillaud, C.; Meneghetti, M.; Troles, J.; Joulain, F.; Cozic, S.; et al. 2–10 µm Mid-Infrared Fiber-Based Supercontinuum Laser Source: Experiment and Simulation. Laser Photonics Rev. 2020, 14, 2000011. [Google Scholar] [CrossRef]
- Lemière, A.; Bizot, R.; Désévédavy, F.; Gadret, G.; Jules, J.C.; Mathey, P.; Aquilina, C.; Béjot, P.; Billard, F.; Faucher, O.; et al. 1.7–18 µm mid-infrared supercontinuum generation in a dispersion-engineered step-index chalcogenide fiber. Results Phys. 2021, 26, 104397. [Google Scholar] [CrossRef]
- Woyessa, G.; Kwarkye, K.; Dasa, M.K.; Petersen, C.R.; Sidharthan, R.; Chen, S.; Yoo, S.; Bang, O. Power stable 1.5–10.5 µm cascaded mid-infrared supercontinuum laser without thulium amplifier. Opt. Lett. 2021, 46, 1129–1132. [Google Scholar] [CrossRef] [PubMed]
- Yu, Y.; Gai, X.; Ma, P.; Vu, K.; Yang, Z.; Wang, R.; Choi, D.-Y.; Madden, S.; Luther-Davies, B. Experimental demonstration of linearly polarized 2–10 μm supercontinuum generation in a chalcogenide rib waveguide. Opt. Lett. 2016, 41, 958–961. [Google Scholar] [CrossRef] [PubMed]
- Cheng, T.; Nagasaka, K.; Tuan, T.H.; Xue, X.; Matsumoto, M.; Tezuka, H.; Suzuki, T.; Ohishi, Y. Mid-infrared supercontinuum generation spanning 2.0 to 15.1 μm in a chalcogenide step-index fiber. Opt. Lett. 2016, 41, 2117–2120. [Google Scholar] [CrossRef]
- Zhao, Z.; Wang, X.; Dai, S.; Pan, Z.; Liu, S.; Sun, L.; Zhang, P.; Liu, Z.; Nie, Q.; Shen, X.; et al. 1.5–14 μm midinfrared supercontinuum generation in a low-loss Te-based chalcogenide step-index fiber. Opt. Lett. 2016, 41, 5222–5225. [Google Scholar] [CrossRef]
- Zhao, Z.; Wu, B.; Wang, X.; Pan, Z.; Liu, Z.; Zhang, P.; Shen, X.; Nie, Q.; Dai, S.; Wang, R. Mid-infrared supercontinuum covering 2.0–16 μm in a low-loss telluride single-mode fiber. Laser Photonics Rev. 2017, 11, 2–6. [Google Scholar] [CrossRef]
- Stingel, A.M.; Vanselous, H.; Petersen, P.B. Covering the vibrational spectrum with microjoule mid-infrared supercontinuum pulses in nonlinear optical applications. J. Opt. Soc. Am. B 2017, 34, 1163–1169. [Google Scholar] [CrossRef]
- Hudson, D.D.; Antipov, S.; Li, L.; Alamgir, I.; Hu, T.; Amraoui, M.E.; Messaddeq, Y.; Rochette, M.; Jackson, S.D.; Fuerbach, A. Toward all-fiber supercontinuum spanning the mid-infrared. Optica 2017, 4, 1163–1166. [Google Scholar] [CrossRef]
- Wang, Y.; Dai, S.; Han, X.; Zhang, P.; Liu, Y.; Wang, X.; Sun, S. Broadband mid-infrared supercontinuum generation in novel As2Se3-As2Se2S step-index fibers. Opt. Commun. 2018, 410, 410–415. [Google Scholar] [CrossRef]
- de Barros, M.R.X.; Miranda, R.S.; Jedju, T.M.; Becker, P.C. High-repetition-rate femtosecond mid-infrared pulse generation. Opt. Lett. 1995, 20, 480–482. [Google Scholar] [CrossRef] [PubMed]
- Fraser, J.M.; Wang, D.; Haché, A.; Allan, G.R.; van Driel, H.M. Generation of high-repetition-rate femtosecond pulses from 8 to 18 µm. Appl. Opt. 1997, 36, 5044–5047. [Google Scholar] [CrossRef] [PubMed]
- Ehret, S.; Schneider, H. Generation of subpicosecond infrared pulses tunable between 5.2 μm and 18 μm at a repetition rate of 76 MHz. Appl. Phys. B Lasers Opt. 1998, 66, 27–30. [Google Scholar] [CrossRef]
- Kaindl, R.A.; Smith, D.C.; Joschko, M.; Hasselbeck, M.P.; Woerner, M.; Elsaesser, T. Femtosecond infrared pulses tunable from 9 to 18 µm at an 88-MHz repetition rate. Opt. Lett. 1998, 23, 861–863. [Google Scholar] [CrossRef] [PubMed]
- Kaindl, R.A.; Wurm, M.; Reimann, K.; Hamm, P.; Weiner, A.M.; Woerner, M. Generation, shaping, and characterization of intense femtosecond pulses tunable from 3 to 20 μm. J. Opt. Soc. Am. B 2000, 17, 2086–2094. [Google Scholar] [CrossRef]
- Song, J.; Xia, J.F.; Zhang, Z.; Strickland, D. Mid-infrared pulses generated from the mixing output of an amplified, dual-wavelength Ti:sapphire system. Opt. Lett. 2002, 27, 200–202. [Google Scholar] [CrossRef]
- Witte, T.; Kompa, K.L.; Motzkus, M. Femtosecond pulse shaping in the mid infrared by difference-frequency mixing. Appl. Phys. B Lasers Opt. 2003, 76, 467–471. [Google Scholar] [CrossRef]
- Foreman, S.M.; Jones, D.J.; Ye, J. Flexible and rapidly configurable femtosecond pulse generation in the mid-IR. Opt. Lett. 2003, 28, 370–372. [Google Scholar] [CrossRef]
- Beutler, M.; Rimke, I.; Büttner, E.; Petrov, V.; Isaenko, L. Femtosecond mid-IR difference-frequency generation in LiInSe2. Opt. Mater. Express 2013, 3, 1834–1838. [Google Scholar] [CrossRef]
- Beutler, M.; Rimke, I.; Büttner, E.; Panyutin, V.; Petrov, V. 80-MHz difference-frequency generation of femtosecond pulses in the mid-infrared using GaS0.4Se0.6. Laser Phys. Lett. 2013, 10, 075406. [Google Scholar] [CrossRef]
- Beutler, M.; Rimke, I.; Edlef, B.; Petrov, V.; Isaenko, L. Difference-frequency generation of fs and ps mid-IR pulses in LiInSe2 based on Yb-fiber laser pump sources. Opt. Lett. 2014, 39, 4353–4355. [Google Scholar] [CrossRef] [PubMed]
- Beutler, M.; Rimke, I.; Büttner, E.; Farinello, P.; Agnesi, A.; Badikov, V.; Badikov, D.; Petrov, V. Difference-frequency generation of ultrashort pulses in the mid-IR using Yb-fiber pump systems and AgGaSe2. Opt. Express 2015, 23, 2730–2736. [Google Scholar] [CrossRef] [PubMed]
- Hegenbarth, R.; Steinmann, A.; Sarkisov, S.; Giessen, H. Milliwatt-level mid-infrared (10.5–16.5 μm) difference frequency generation with a femtosecond dual-signal-wavelength optical parametric oscillator. Opt. Lett. 2012, 37, 3513–3515. [Google Scholar] [CrossRef] [PubMed]
- Steinle, T.; Mörz, F.; Steinmann, A.; Giessen, H. Ultra-stable high average power femtosecond laser system tunable from 1.33 to 20 μm. Opt. Lett. 2016, 41, 4863–4866. [Google Scholar] [CrossRef]
- Wilson, D.J.; Summers, A.M.; Zigo, S.; Davis, B.; Robatjazi, S.J.; Powell, J.A.; Rolles, D.; Rudenko, A.; Trallero-Herrero, C.A. An intense, few-cycle source in the long-wave infrared. Sci. Rep. 2019, 9, 3–9. [Google Scholar] [CrossRef]
- Keilmann, F.; Amarie, S. Mid-infrared frequency comb spanning an octave based on an Er fiber laser and difference-frequency generation. J. Infrared Millim. Terahertz Waves 2012, 33, 479–484. [Google Scholar] [CrossRef]
- Gambetta, A.; Coluccelli, N.; Cassinerio, M.; Gatti, D.; Laporta, P.; Galzerano, G.; Marangoni, M. Milliwatt-level frequency combs in the 8–14 μm range via difference frequency generation from an Er:fiber oscillator. Opt. Lett. 2013, 38, 1155–1157. [Google Scholar] [CrossRef]
- Yao, Y.; Knox, W.H. Broadly tunable femtosecond mid-infrared source based on dual photonic crystal fibers. Opt. Express 2013, 21, 26612–26619. [Google Scholar] [CrossRef]
- Churin, D.; Kieu, K.; Norwood, R.A.; Peyghambarian, N. Efficient frequency comb generation in the 9-μm region using compact fiber sources. IEEE Photonics Technol. Lett. 2014, 26, 2271–2274. [Google Scholar] [CrossRef]
- Zhou, G.; Cao, Q.; Kärtner, F.X.; Chang, G. Energy scalable, offset-free ultrafast mid-infrared source harnessing self-phase-modulation-enabled spectral selection. Opt. Lett. 2018, 43, 2953–2956. [Google Scholar] [CrossRef]
- Sotor, J.; Martynkien, T.; Schunemann, P.G.; Mergo, P.; Rutkowski, L.; Soboń, G. All-fiber mid-infrared source tunable from 6 to 9 μm based on difference frequency generation in OP-GaP crystal. Opt. Express 2018, 26, 11756–11763. [Google Scholar] [CrossRef] [PubMed]
- Krzempek, K.; Tomaszewska, D.; Głuszek, A.; Martynkien, T.; Mergo, P.; Sotor, J.; Foltynowicz, A.; Soboń, G. Stabilized all-fiber source for generation of tunable broadband fCEO-free mid-IR frequency comb in the 7–9 µm range. Opt. Express 2019, 27, 37435–37445. [Google Scholar] [CrossRef] [PubMed]
- Ma, J.; Lu, Q.; Duan, D.; Yao, B.; Mao, Q. A Broadband Infrared DFG Optical Comb Using All-PM Nonlinear Pulse Fiber Amplification Technique. IEEE Photonics Technol. Lett. 2019, 31, 439–442. [Google Scholar] [CrossRef]
- Pupeza, I.; Sanchez, D.; Zhang, J.; Lilienfein, N.; Seidel, M.; Karpowicz, N.; Paasch-Colberg, T.; Znakovskaya, I.; Pescher, M.; Schweinberger, W.; et al. High-power sub-two-cycle mid-infrared pulses at 100 MHz repetition rate. Nat. Photonics 2015, 9, 721–724. [Google Scholar] [CrossRef]
- Chen, B.-H.; Nagy, T.; Baum, P. Efficient middle-infrared generation in LiGaS2 by simultaneous spectral broadening and difference-frequency generation. Opt. Lett. 2018, 43, 1742–1745. [Google Scholar] [CrossRef] [PubMed]
- Novák, O.; Krogen, P.R.; Kroh, T.; Mocek, T.; Kärtner, F.X.; Hong, K.-H. Femtosecond 8.5 μm source based on intrapulse difference-frequency generation of 2.1 μm pulses. Opt. Lett. 2018, 43, 1335–1338. [Google Scholar] [CrossRef]
- Timmers, H.; Kowligy, A.; Lind, A.; Cruz, F.C.; Nader, N.; Silfies, M.; Ycas, G.; Allison, T.K.; Schunemann, P.G.; Papp, S.B.; et al. Molecular fingerprinting with bright, broadband infrared frequency combs. Optica 2018, 5, 727–732. [Google Scholar] [CrossRef]
- Zhang, J.; Fai Mak, K.; Nagl, N.; Seidel, M.; Bauer, D.; Sutter, D.; Pervak, V.; Krausz, F.; Pronin, O. Multi-mW, few-cycle mid-infrared continuum spanning from 500 to 2250 cm−1. Light Sci. Appl. 2018, 7, 17180. [Google Scholar] [CrossRef]
- Gaida, C.; Gebhardt, M.; Heuermann, T.; Stutzki, F.; Jauregui, C.; Antonio-Lopez, J.; Schülzgen, A.; Amezcua-Correa, R.; Tünnermann, A.; Pupeza, I.; et al. Watt-scale super-octave mid-infrared intrapulse difference frequency generation. Light Sci. Appl. 2018, 7, 94. [Google Scholar] [CrossRef]
- Wang, Q.; Zhang, J.; Kessel, A.; Nagl, N.; Pervak, V.; Pronin, O.; Mak, K.F. Broadband mid-infrared coverage (2–17 μm) with few-cycle pulses via cascaded parametric processes. Opt. Lett. 2019, 44, 2566–2569. [Google Scholar] [CrossRef]
- Liu, K.; Liang, H.; Qu, S.; Li, W.; Zou, X.; Zhang, Y.; Wang, Q.J. High-energy mid-infrared intrapulse difference-frequency generation with 53% conversion efficiency driven at 3 µm. Opt. Express 2019, 27, 37706–37713. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Fritsch, K.; Wang, Q.; Krausz, F.; Mak, K.F.; Pronin, O. Intra-pulse difference-frequency generation of mid-infrared (2.7–20 μm) by random quasi-phase-matching. Opt. Lett. 2019, 44, 2986–2989. [Google Scholar] [CrossRef] [PubMed]
- Kowligy, A.S.; Timmers, H.; Lind, A.J.; Karlen, S.; Cruz, F.; Schunemann, P.G.; Biegert, J.; DIddams, S.A. Near-Single-Cycle Long-Wave Infrared Pulses for Coherent Linear and Nonlinear Optics. In Proceedings of the Conference on Lasers and Electro-Optics Science and Innovations 2019, San Jose, CA, USA, 5–10 May 2019; Volume 1, pp. 39–40. [Google Scholar]
- Vasilyev, S.; Moskalev, I.S.; Smolski, V.O.; Peppers, J.M.; Mirov, M.; Muraviev, A.V.; Zawilski, K.; Schunemann, P.G.; Mirov, S.B.; Vodopyanov, K.L.; et al. Super-octave longwave mid-infrared coherent transients produced by optical rectification of few-cycle 2.5-μm pulses. Optica 2019, 6, 111–114. [Google Scholar] [CrossRef]
- Butler, T.P.; Gerz, D.; Hofer, C.; Xu, J.; Gaida, C.; Heuermann, T.; Gebhardt, M.; Vamos, L.; Schweinberger, W.; Gessner, J.A.; et al. Watt-scale 50-MHz source of single-cycle waveform-stable pulses in the molecular fingerprint region. Opt. Lett. 2019, 44, 1730–1733. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, Q.; Hao, J.; Liu, H.; Yao, J.; Li, Z.; Liu, J.; Mak, K.F. Broadband, few-cycle mid-infrared continuum based on the intra-pulse difference frequency generation with BGSe crystals. Opt. Express 2020, 28, 37903–37909. [Google Scholar] [CrossRef] [PubMed]
- Kowligy, A.S.; Carlson, D.R.; Hickstein, D.D.; Timmers, H.; Lind, A.J.; Schunemann, P.G.; Papp, S.B.; Diddams, S.A. Mid-infrared frequency combs at 10 GHz. Opt. Lett. 2020, 45, 3677–3680. [Google Scholar] [CrossRef]
- Lesko, D.M.B.; Timmers, H.; Xing, S.; Kowligy, A.; Lind, A.J.; Diddams, S.A. A six-octave optical frequency comb from a scalable few-cycle erbium fibre laser. Nat. Photonics 2021, 15, 281–286. [Google Scholar] [CrossRef]
- Wang, W.; Wu, H.; Liu, C.; Sun, B.; Liang, H. Multigigawatt 50 fs Yb:CALGO regenerative amplifier system with 11 W average power and mid-infrared generation. Photonics Res. 2021, 9, 1439–1445. [Google Scholar] [CrossRef]
- Xing, S.; Lesko, D.M.B.; Umeki, T.; Lind, A.J.; Hoghooghi, N.; Wu, T.H.; Diddams, S.A. Single-cycle all-fiber frequency comb. APL Photonics 2021, 6, 086110. [Google Scholar] [CrossRef]
- Nakamura, T.; Badarla, V.R.; Hashimoto, K.; Schunemann, P.G.; Ideguchi, T. A simple approach of broadband mid-infrared pulse generation with a mode-locked Yb-doped fiber laser. Opt. Lett. 2022, 47, 1790–1793. [Google Scholar] [CrossRef]
- Romero-Alvarez, R.; Pettus, R.; Wu, Z.; Strickland, D. Two-color fiber amplifier for short-pulse, mid-infrared generation. Opt. Lett. 2008, 33, 1065–1067. [Google Scholar] [CrossRef] [PubMed]
- Al-Kadry, A.M.; Strickland, D. Generation of 400 μW at 17.5 μm using a two-color Yb fiber chirped pulse amplifier. Opt. Lett. 2011, 36, 1080–1082, Erratum in Opt. Lett. 2018, 43, 316–316. [Google Scholar]
- Hajialamdari, M.; Strickland, D. Tunable mid-infrared source from an ultrafast two-color Yb:fiber chirped-pulse amplifier. Opt. Lett. 2012, 37, 3570–3572, Erratum in Opt. Lett. 2018, 43, 353–353. [Google Scholar] [CrossRef]
- Su, X.; Hoang, T.; Long, P.; Zheng, Y.; Strickland, D. A Compact High-Average-Power Femtosecond Fiber-Coupled Two-Color CPA System. IEEE J. Sel. Top. Quantum Electron. 2018, 24, 0902905. [Google Scholar] [CrossRef]
- Su, X.; Lyu, M.; Hoang, T.; Xu, Z.; Zheng, Y.; Strickland, D. Investigation of long wavelength mid-infrared generation in the tight focusing limit. Opt. Express 2019, 27, 24945–24952. [Google Scholar] [CrossRef]
- Hajialamdari, M. Tunable Two-Color Ultrafast Yb: Fiber Chirped Pulse Amplifier: Modeling, Experiment, and Application in Tunable Short-Pulse Mid-Infrared Generation; University of Waterloo: Waterloo, ON, Canada, 2013. [Google Scholar]
- Cao, Q.; Kärtner, F.X.; Chang, G. Towards high power longwave mid-IR frequency combs: Power scalability of high repetition-rate difference-frequency generation. Opt. Express 2020, 28, 1369–1384. [Google Scholar] [CrossRef]
- Morris, J.R.; Shen, Y.R. Theory of far-infrared generation by optical mixing. Phys. Rev. A 1977, 15, 1143–1156. [Google Scholar] [CrossRef]
- Giusfredi, G.; Mazzotti, D.; Cancio, P.; De Natale, P. Spatial mode control of radiation generated by frequency difference in periodically poled crystals. Phys. Rev. Lett. 2001, 87, 113901. [Google Scholar] [CrossRef]
- Malara, P.; Maddaloni, P.; Mincuzzi, G.; De Nicola, S.; De Natale, P. Non-collinear quasi phase matching and annular profiles in difference frequency generation with focused Gaussian beams. Opt. Express 2008, 16, 8056–8066. [Google Scholar] [CrossRef]
- Kurus, A.; Yelisseyev, A.; Lobanov, S.; Plyusnin, P.; Molokeev, M.; Solovyev, L.; Samoshkin, D.; Stankus, S.; Melnikova, S.; Isaenko, L. Thermophysical properties of lithium thiogallate that are important for optical applications. RSC Adv. 2021, 11, 39177–39187. [Google Scholar] [CrossRef]








| Pump | MIR | Optical-Optical Conversion Efficiency | Repetition Rate | Method | Crystal | Reference |
|---|---|---|---|---|---|---|
| 800 nm, 4 W, 150 fs | 2.3 mW@11.5 μm Tunable 4–11.5 μm 410 fs@7.2 μm | 0.058%@11.5 μm | 80 MHz | DFG | LiInSe2 | [103] |
| 800 nm, 4 W, 150 fs | >0.5 mW@12 μm Tunable 4–12 μm | 0.013%@12 μm | 80 MHz | DFG | GaS0.4Se0.6 | [104] |
| 1034 nm, 5 W, 260 fs | ~25 mW@9 μm Tunable 5–12 μm 313 fs@7.2 μm | ~0.5%@9 μm | 53 MHz | DFG | LiInSe2 | [105] |
| 1034 nm, 5 W, 260 fs | 69 mW@6 μm ~25 mW@8.2 μm Tunable 5–17 μm 305 fs@7.2 μm | 1.4%@6 μm ~0.5%@8.2 μm | 53 MHz | DFG | AgGaSe2 | [106] |
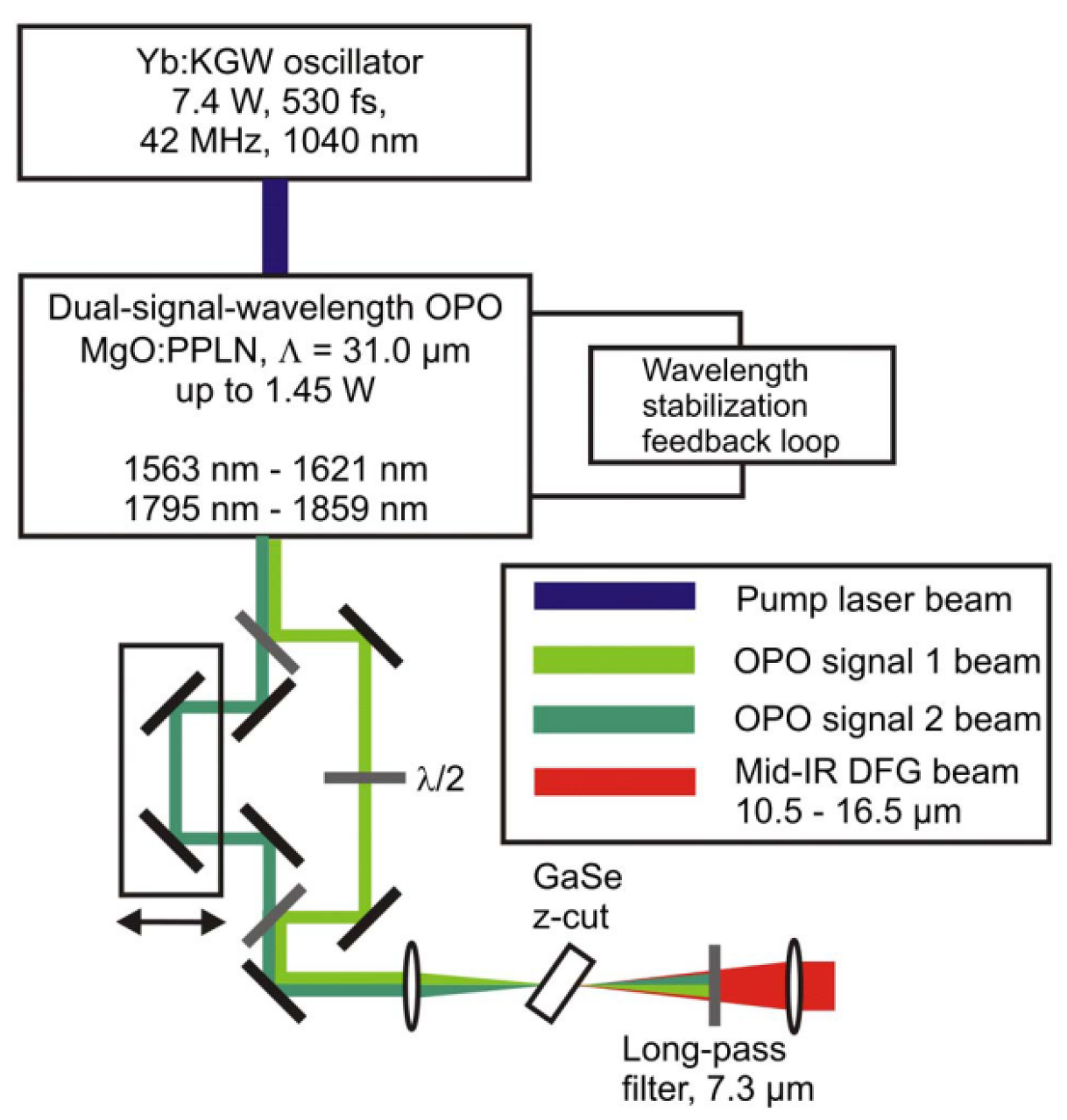
| 1040 nm, 1.45 W, 250 fs | 4.3 mW@13.2 μm Tunable 10.5–16.5 μm | 0.29%@13.2μm | 42 MHz | DFG | AgGaSe2 | [107] |
| 1.03 μm, 7 W, 450 fs | 16 mW@15 μm, 0.6 mW@20 μm Tunable 1.33–20 μm 350 fs | 0.23%@15 μm 0.085%@20 μm | 43 MHz | DFG | AgGaSe2 | [108] |
| 800 nm, 18 mJ, 26 fs | 80 μJ@8.9 μm Tunable 3–9 μm 70–90 fs | 0.44%@8.9 μm | 1 kHz | DFG | AgGaS2 | [109] |
| 1.55 μm, 360 mW, 100 fs | 0.6–0.9 mW Tunable 3.7–20 μm | 0.16–0.25% | 40 MHz | DFG | GaSe | [110] |
| 1.55 μm, 550 mW, 50 fs | 4 mW@7.8 μm 1 mW@10.2 μm 0.11 mW@13.6 μm Tunable 8–14 μm | 0.72%@7.8 μm 0.18%@10.2 μm 0.02%@13.6 μm | 250 MHz | DFG | GaSe | [111] |
| 1035 nm, 1.3 W, 300 fs | ~135 µW@9 µm Tunable 4.2–9 μm | ~0.01%@9 µm | 40 MHz | DFG | AgGaS2 | [112] |
| 1.56 μm, 65 mW, 80 fs | 1.55 mW Spanning 7.5–11.6 μm 80 fs | 2.3% | 40 MHz | DFG | AgGaS2 | [113] |
| 1.03 μm, 6 W, 165 fs | 5.4 mW@9.5 μm 1.7 mW@16.7 μm Tunable 7–18 μm | 0.09%@9.5 μm 0.03%@16.7 μm | 30 MHz | DFG | GaSe | [114] |
| 1.56 μm, 175 mW, 76 fs | 7.4 mW@7.5 μm Tunable 6–9 μm | 4.2%@7.5 μm | 48 MHz | DFG | OP-GaP | [115] |
| 1.56 μm, 300 mW, 65 fs | 5 mW Tunable 6.5–9 µm | 1.7% | 125 MHz | DFG | OP-GaP | [116] |
| 1.56 μm, 150 mW, 47 fs | 120 µW@8 µm Tunable 7–10.5 µm | 0.08%@8 µm | 100 MHz | DFG | GaSe | [117] |
| 940 nm, 50 W, 19 fs | 103 mW Spanning 6.8–16.4 μm 66 fs@11.5 μm | 0.21% | 100 MHz | IDFG | GaSe | [118] |
| 1030 nm, 1 W, 30 fs | ~0.350 mW Spanning 8–11µm | ~0.035% | 50 kHz | IDFG | LiGaS2 | [119] |
| 2.1 μm, 250 μJ, 26 fs | 2 μJ@8.5 μm Spanning 7–11 μm | 0.8%@8.5 μm | 1 kHz | IDFG | AgGaSe2 | [120] |
| 600 nm, 350 mW, 10.6 fs | 0.25 mW Spanning 4–12 μm | 0.071% | 100 MHz | IDFG | OP-GaP | [121] |
| 2 μm, 18.7 W, 15 fs | 24 mW Spanning 4.5–20 μm | 0.13% | 77 MHz | IDFG | GaSe | [122] |
| 1.92 μm, 31.4 W, 110 fs | 450 mW Spanning 3.7–18 μm | 1.4% | 1.25 MHz | IDFG | GaSe | [123] |
| 2.1 μm, 1 W, 45 fs, | 15 mW Spanning 2–17 μm | 1.5% | 68.7 MHz | IDFG | GaSe | [124] |
| 3 μm, 95 μJ, 35 fs | 5 µJ, 50 mW Spanning 6–13.2 μm 68 fs@9.7 μm | 5.3% | 10 kHz | IDFG | GaSe | [125] |
| 2.1 μm, 7 W, 15 fs | 35 mW Spanning 2.7–20 μm | 0.5% | 77 MHz | IDFG | ZnSe/ZnS | [126] |
| 1.55 μm, 3.5 W, 30 fs | 25 mW Spanninng 4–20 μm | 0.71% | 100 MHz | IDFG | OP-GaP | [127] |
| 2.5 μm, 5.9 W, 20 fs | 13 mW Spanning 5.8–17.6 μm | 0.22% | 78 MHz | IDFG | GaSe | [128] |
| 2.5 μm, 4.5 W, 20 fs | 148 mW Spanning 5.8–12.5 μm | 3.3% | 78 MHz | IDFG | ZGP | [128] |
| 2 μm, 30 W, 32 fs | 0.5 W Spanning 6–18 μm 43 fs | 1.7% | 50 MHz | IDFG | GaSe | [129] |
| 2.4 µm, 1.1 W, 28 fs, | 1.9 mW Spanning 6–18 μm | 0.17% | 69 MHz | IDFG | BGSe | [130] |
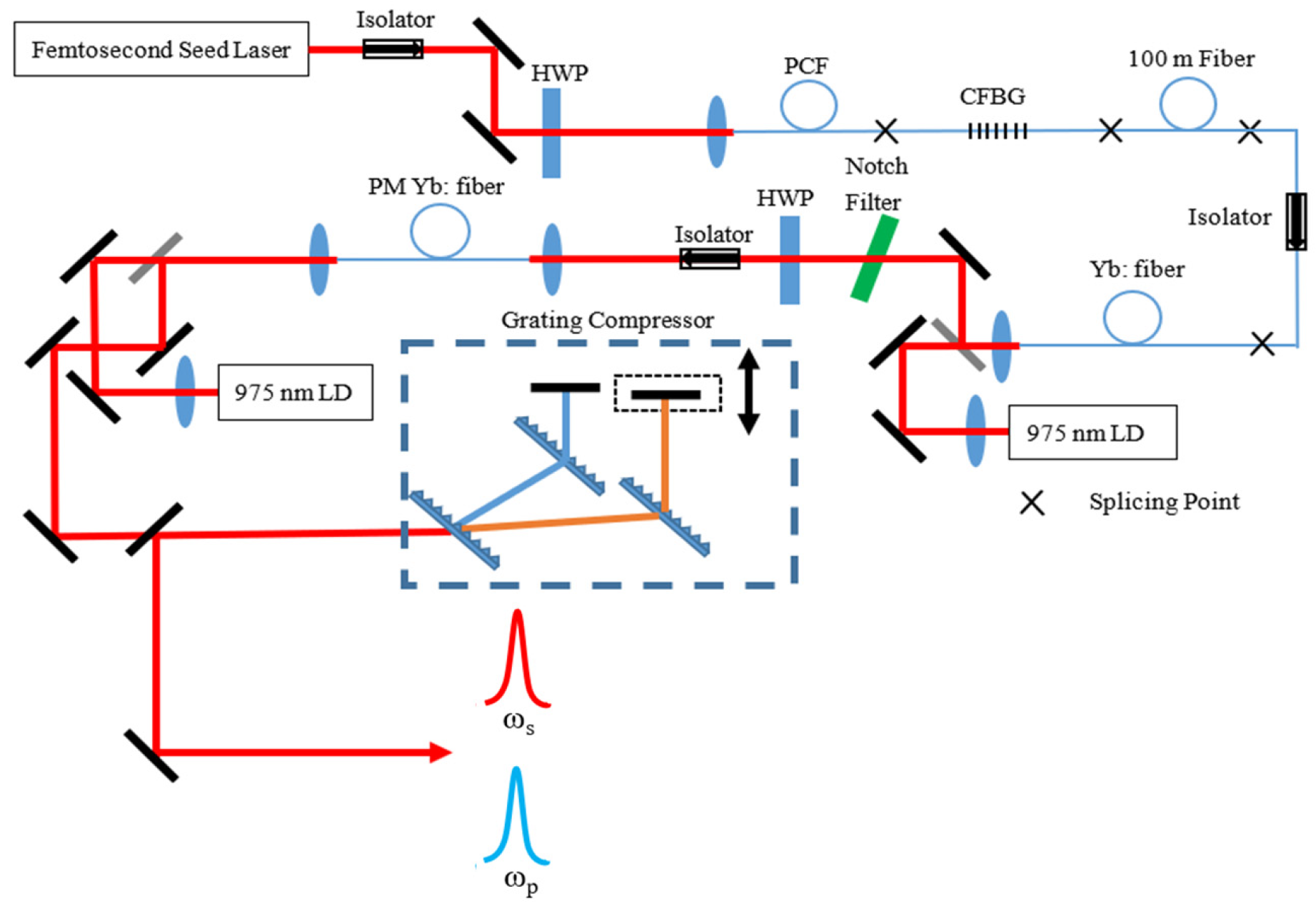
| 1.55 μm, 800 mW, 15 fs | 70–100 μW Spanning 7–11 μm | 0.008–0.013% | 10 GHz | IDFG | OP-GaP | [131] |
| 1550 nm, 1.5 W, 10 fs | N/A Spanning 0.35–22.5 μm | N/A | 100 MHz | IDFG | PPLN/CdSiP2/GaSe | [132] |
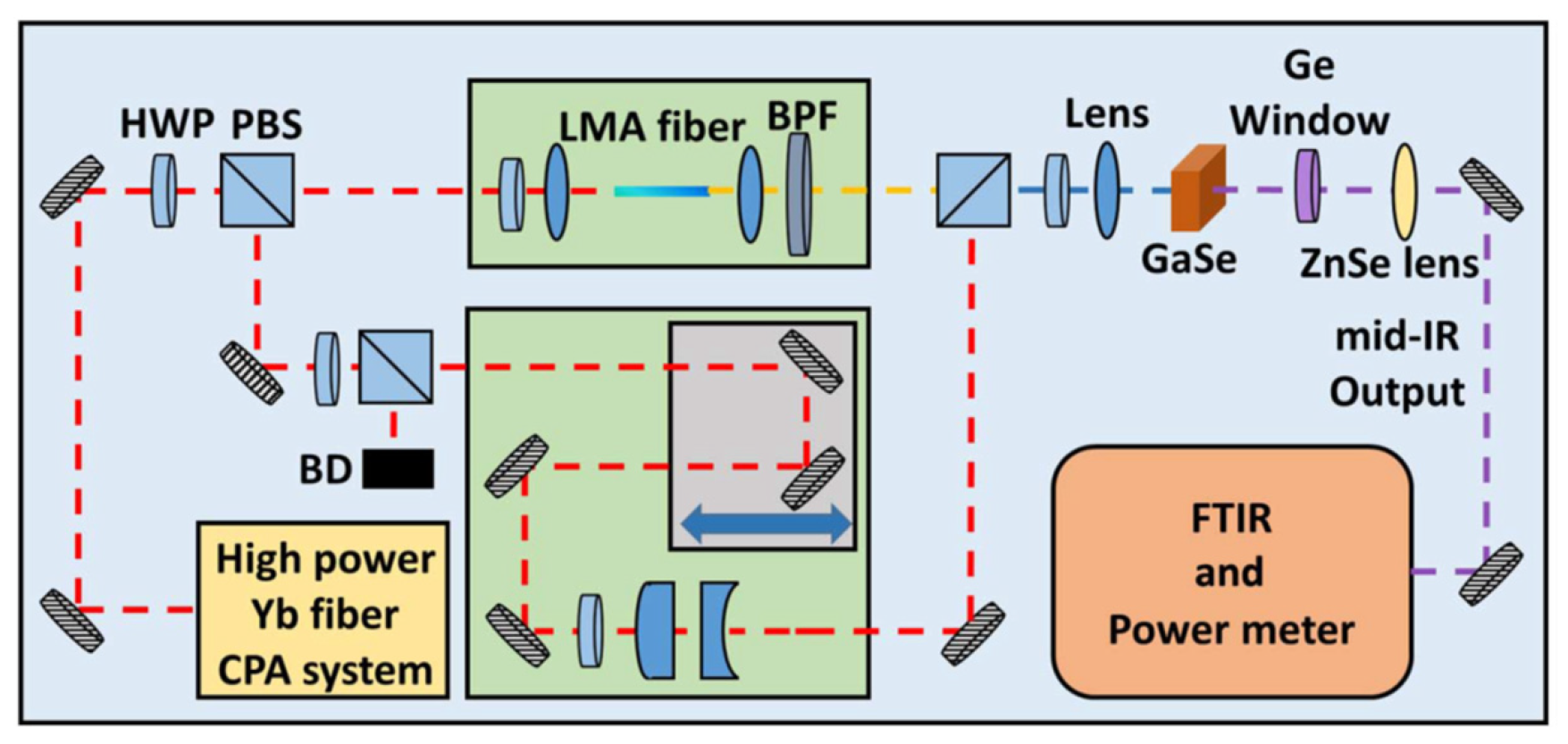
| 1040 nm, 11 W, 50 fs | 3.3 mW Tunable 7.5–11.2 μm | 0.03% | 43 kHz | IDFG | LiGaS2 | [133] |
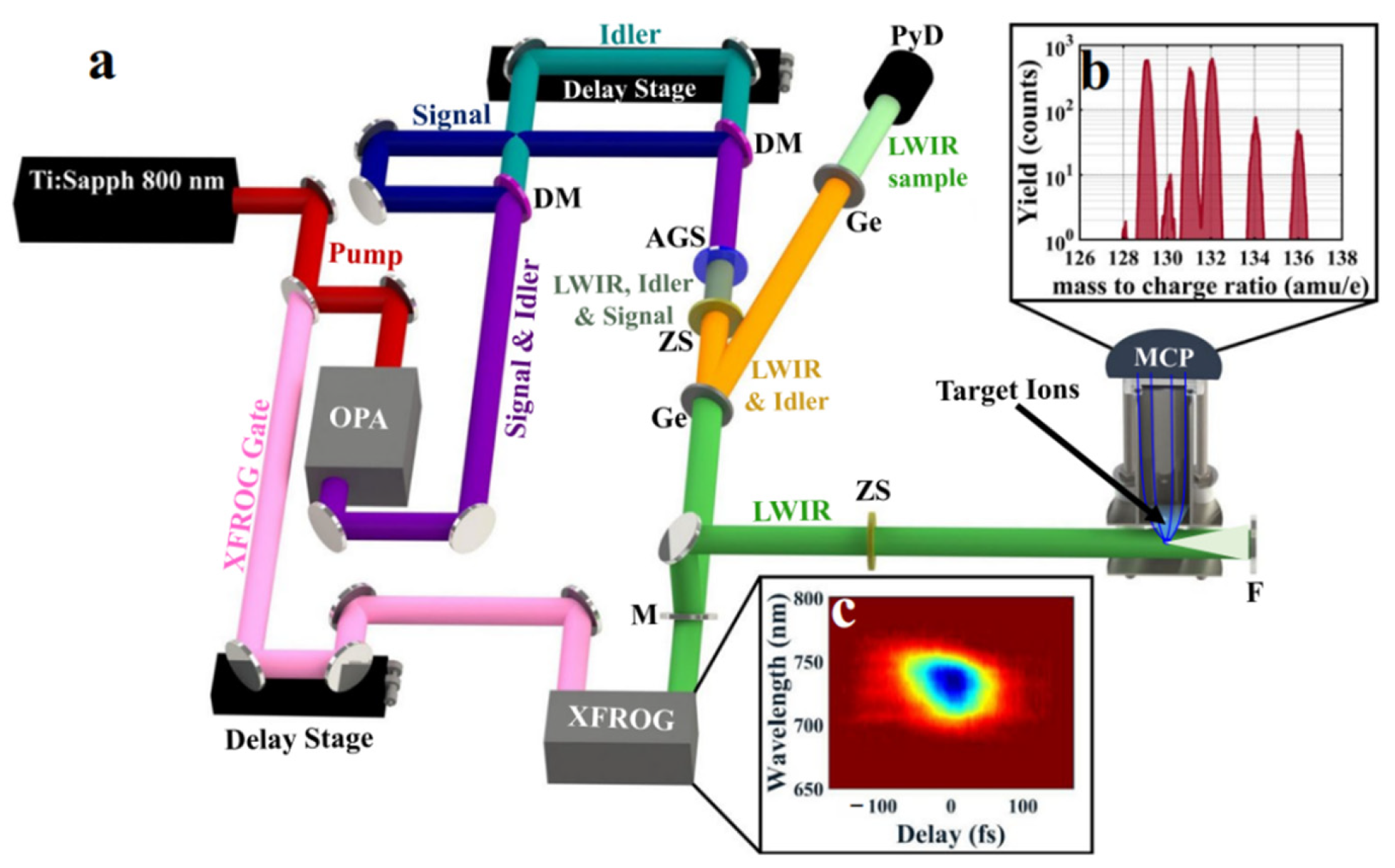
| 2 μm, 374 mW, 6.8 fs | 860 μW@8.5 μm Spanning 6–22 μm 63 fs@8.5 μm 120 fs@10.7 μm | 0.23% | 100 MHz | IDFG | OP-GaAs/CdSiP2 | [134] |
| 1030 nm, 3.3 W, 12.1 fs | 1.2 mW Spanning 8.1–13.1 µm | 0.036% | 50 MHz | IDFG | OP-GaP | [135] |
| Method 1 | Method 2 | Method 3 | |
|---|---|---|---|
| Power (mW) | 2.50 ± 0.19 | 2.19 ± 0.54 | 5.94 ± 0.58 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Su, X.; Zhu, R.; Wang, B.; Bai, Y.; Ding, T.; Sun, T.; Lü, X.; Peng, J.; Zheng, Y. Generation of 8–20 μm Mid-Infrared Ultrashort Femtosecond Laser Pulses via Difference Frequency Generation. Photonics 2022, 9, 372. https://doi.org/10.3390/photonics9060372
Su X, Zhu R, Wang B, Bai Y, Ding T, Sun T, Lü X, Peng J, Zheng Y. Generation of 8–20 μm Mid-Infrared Ultrashort Femtosecond Laser Pulses via Difference Frequency Generation. Photonics. 2022; 9(6):372. https://doi.org/10.3390/photonics9060372
Chicago/Turabian StyleSu, Xinyang, Ruixue Zhu, Bolin Wang, Yu Bai, Tao Ding, Tianran Sun, Xing Lü, Jiying Peng, and Yi Zheng. 2022. "Generation of 8–20 μm Mid-Infrared Ultrashort Femtosecond Laser Pulses via Difference Frequency Generation" Photonics 9, no. 6: 372. https://doi.org/10.3390/photonics9060372
APA StyleSu, X., Zhu, R., Wang, B., Bai, Y., Ding, T., Sun, T., Lü, X., Peng, J., & Zheng, Y. (2022). Generation of 8–20 μm Mid-Infrared Ultrashort Femtosecond Laser Pulses via Difference Frequency Generation. Photonics, 9(6), 372. https://doi.org/10.3390/photonics9060372






