Holographic Tailoring of Structured Light Field with Digital Device
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
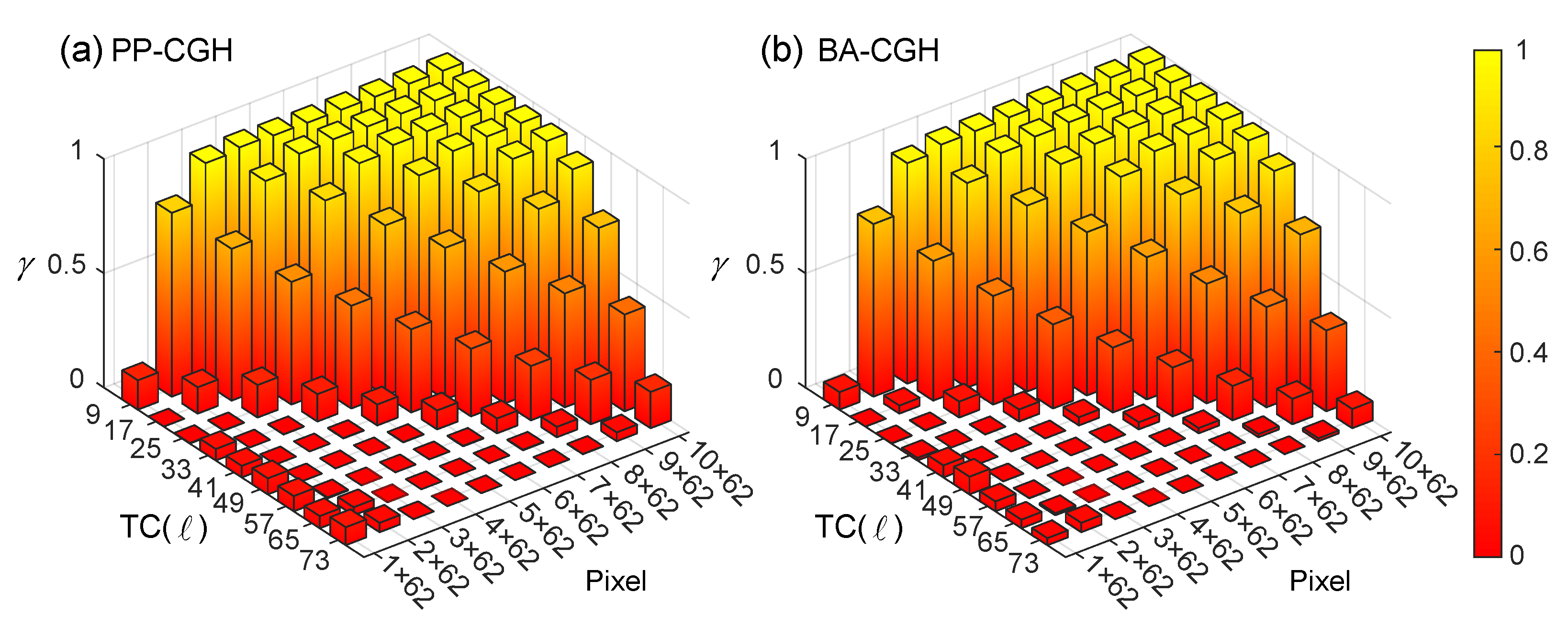
3.1. Precision of Holographic Tailoring
3.2. Efficiency of Holographic Tailoring
3.3. Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
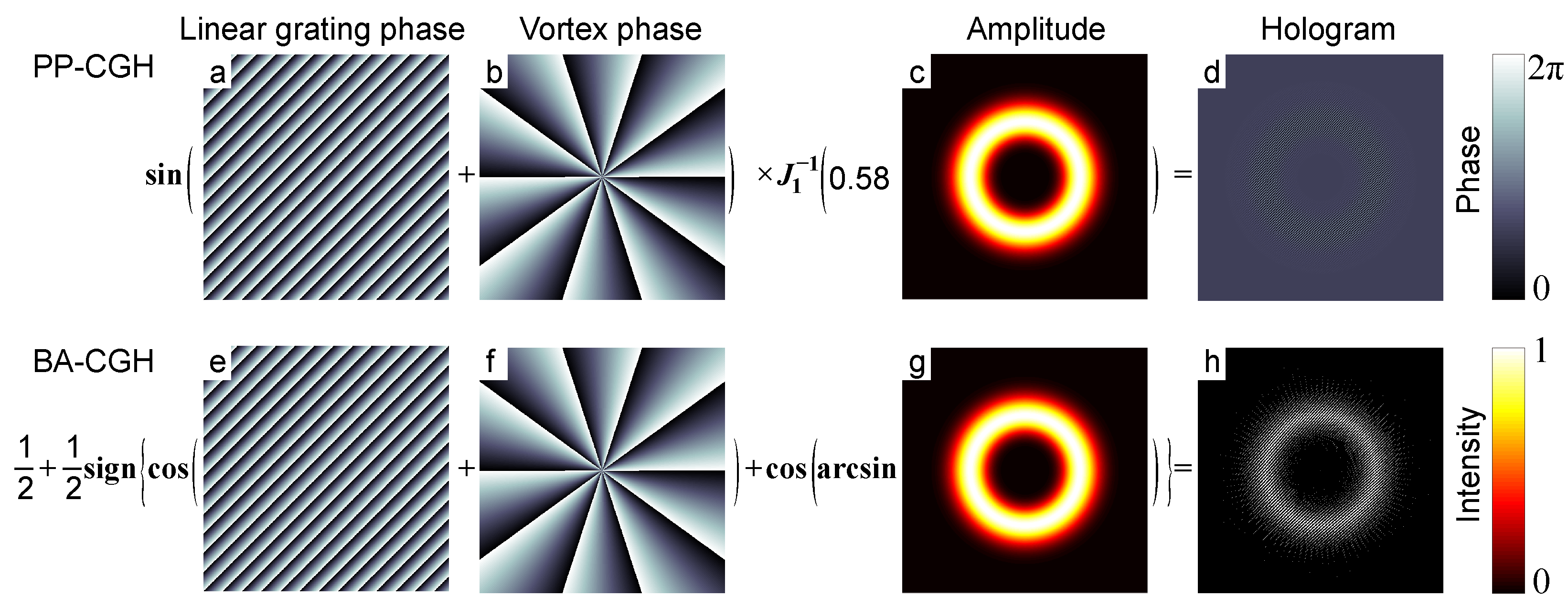
Appendix A. Generation of Pure Phase Holograms
Appendix B. Generation of Binary Amplitude Holograms
References
- Forbes, A.; de Oliveira, M.; Dennis, M.R. Structured light. Nat. Photonics 2021, 15, 253–262. [Google Scholar] [CrossRef]
- Rubinsztein-Dunlop, H.; Forbes, A.; Berry, M.V.; Dennis, M.R.; Andrews, D.L.; Mansuripur, M.; Denz, C.; Alpmann, C.; Banzer, P.; Bauer, T.; et al. Roadmap on structured light. J. Opt. 2016, 19, 013001. [Google Scholar] [CrossRef]
- Wan, Z.; Shen, Y.; Wang, Z.; Shi, Z.; Liu, Q.; Fu, X. Divergence-degenerate spatial multiplexing towards future ultrahigh capacity, low error-rate optical communications. Light Sci. Appl. 2022, 11, 144. [Google Scholar] [CrossRef] [PubMed]
- Allen, L.; Beijersbergen, M.W.; Spreeuw, R.; Woerdman, J. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 1992, 45, 8185. [Google Scholar] [CrossRef] [PubMed]
- Shen, Y.; Wang, X.; Xie, Z.; Min, C.; Fu, X.; Liu, Q.; Gong, M.; Yuan, X. Optical vortices 30 years on: OAM manipulation from topological charge to multiple singularities. Light Sci. Appl. 2019, 8, 90. [Google Scholar] [CrossRef] [Green Version]
- Yao, A.M.; Padgett, M.J. Orbital angular momentum: Origins, behavior and applications. Adv. Opt. Photonics 2011, 3, 161–204. [Google Scholar] [CrossRef] [Green Version]
- Shen, Y.; Meng, Y.; Fu, X.; Gong, M. Hybrid topological evolution of multi-singularity vortex beams: Generalized nature for helical-Ince–Gaussian and Hermite–Laguerre–Gaussian modes. J. Opt. Soc. Am. A 2019, 36, 578–587. [Google Scholar] [CrossRef]
- Chen, R.; Zhou, H.; Moretti, M.; Wang, X.; Li, J. Orbital angular momentum waves: Generation, detection, and emerging applications. IEEE Commun. Surv. Tutor. 2019, 22, 840–868. [Google Scholar] [CrossRef] [Green Version]
- Ettorre, M.; Pavone, S.C.; Casaletti, M.; Albani, M.; Mazzinghi, A.; Freni, A. Near-field focusing by non-diffracting Bessel beams. In Aperture Antennas for Millimeter and Sub-Millimeter Wave Applications; Springer: Cham, Switzerland, 2018; pp. 243–288. [Google Scholar]
- Zhan, Q. Cylindrical vector beams: From mathematical concepts to applications. Adv. Opt. Photonics 2009, 1, 1–57. [Google Scholar] [CrossRef]
- Willner, A.E.; Pang, K.; Song, H.; Zou, K.; Zhou, H. Orbital angular momentum of light for communications. Appl. Phys. Rev. 2021, 8, 041312. [Google Scholar] [CrossRef]
- Yang, Y.; Ren, Y.; Chen, M.; Arita, Y.; Rosales-Guzmán, C. Optical trapping with structured light: A review. Adv. Photonics 2021, 3, 034001. [Google Scholar] [CrossRef]
- Liu, K.; Cheng, Y.; Gao, Y.; Li, X.; Qin, Y.; Wang, H. Super-resolution radar imaging based on experimental OAM beams. Appl. Phys. Lett. 2017, 110, 164102. [Google Scholar] [CrossRef]
- Neo, R.; Leon-Saval, S.; Bland-Hawthorn, J.; Molina-Terriza, G. OAM interferometry: The detection of the rotational Doppler shift. Opt. Express 2017, 25, 21159–21170. [Google Scholar] [CrossRef]
- Arrizón, V.; Méndez, G.; Sánchez-de La-Llave, D. Accurate encoding of arbitrary complex fields with amplitude-only liquid crystal spatial light modulators. Opt. Express 2005, 13, 7913–7927. [Google Scholar] [CrossRef]
- Kirk, J.P.; Jones, A.L. Phase-only complex-valued spatial filter. J. Opt. Soc. Am. 1971, 61, 1023–1028. [Google Scholar] [CrossRef]
- Lee, W.H. Binary computer-generated holograms. Appl. Opt. 1979, 18, 3661–3669. [Google Scholar] [CrossRef]
- Davis, J.A.; Cottrell, D.M.; Campos, J.; Yzuel, M.J.; Moreno, I. Encoding amplitude information onto phase-only filters. Appl. Opt. 1999, 38, 5004–5013. [Google Scholar] [CrossRef] [PubMed]
- Huang, D.; Timmers, H.; Roberts, A.; Shivaram, N.; Sandhu, A.S. A low-cost spatial light modulator for use in undergraduate and graduate optics labs. Am. J. Phys. 2012, 80, 211–215. [Google Scholar] [CrossRef]
- Sampsell, J.B. Digital micromirror device and its application to projection displays. J. Vac. Sci. Technol. B Microelectron. Nanometer Struct. Process. Meas. Phenom. 1994, 12, 3242–3246. [Google Scholar] [CrossRef]
- Ren, Y.X.; Lu, R.D.; Gong, L. Tailoring light with a digital micromirror device. Ann. Phys. 2015, 527, 447–470. [Google Scholar] [CrossRef] [Green Version]
- Arrizón, V.; Ruiz, U.; Carrada, R.; González, L.A. Pixelated phase computer holograms for the accurate encoding of scalar complex fields. J. Opt. Soc. Am. A 2007, 24, 3500–3507. [Google Scholar] [CrossRef] [PubMed]
- Davis, J.A.; Valadéz, K.O.; Cottrell, D.M. Encoding amplitude and phase information onto a binary phase-only spatial light modulator. Appl. Opt. 2003, 42, 2003–2008. [Google Scholar] [CrossRef] [PubMed]
- Forbes, A.; Dudley, A.; McLaren, M. Creation and detection of optical modes with spatial light modulators. Adv. Opt. Photonics 2016, 8, 200–227. [Google Scholar] [CrossRef]
- Qu, G.; Yang, W.; Song, Q.; Liu, Y.; Qiu, C.W.; Han, J.; Tsai, D.P.; Xiao, S. Reprogrammable meta-hologram for optical encryption. Nat. Commun. 2020, 11, 5484. [Google Scholar] [CrossRef] [PubMed]
- Yepes, I.S.; Vieira, T.A.; Suarez, R.A.; Fernandez, S.R.; Gesualdi, M.R. Phase and intensity analysis of non-diffracting beams via digital holography. Opt. Commun. 2019, 437, 121–127. [Google Scholar] [CrossRef]
- Hu, X.; Rosales-Guzmán, C. Generation and characterization of complex vector modes with digital micromirror devices: A tutorial. J. Opt. 2021, 24, 034001. [Google Scholar] [CrossRef]
- Wan, Z.; Wang, Z.; Yang, X.; Shen, Y.; Fu, X. Digitally tailoring arbitrary structured light of generalized ray-wave duality. Opt. Express 2020, 28, 31043–31056. [Google Scholar] [CrossRef]
- Wan, Z.; Shen, Y.; Gong, M.; Fu, X. Quadrant-separable multi-singularity vortices manipulation by coherent superposed mode with spatial-energy mismatch. Opt. Express 2018, 26, 34940–34955. [Google Scholar] [CrossRef]
- He, T.; Meng, Y.; Liu, Z.; Hu, F.; Wang, R.; Li, D.; Yan, P.; Liu, Q.; Gong, M.; Xiao, Q. Guided mode meta-optics: Metasurface-dressed waveguides for arbitrary mode couplers and on-chip OAM emitters with a configurable topological charge. Opt. Express 2021, 29, 39406–39418. [Google Scholar] [CrossRef]
- da Silva, B.P.; Marques, B.; Rodrigues, R.; Ribeiro, P.S.; Khoury, A. Machine-learning recognition of light orbital-angular-momentum superpositions. Phys. Rev. A 2021, 103, 063704. [Google Scholar] [CrossRef]





| Modulator | DMD | |
|---|---|---|
| Traits | Low refresh rate (50–500 Hz); polarization-sensitive; wavelength-sensitive; temperature-sensitive; relatively high efficiency(≤20%); expensive | High refresh rate (10 kHz–30 kHz); polarization-insensitive; wavelength-insensitive; temperature-insensitive; low efficiency(≤5%); relatively cheap |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wan, Z.; Shi, Z.; Liu, Q.; Fu, X. Holographic Tailoring of Structured Light Field with Digital Device. Photonics 2022, 9, 506. https://doi.org/10.3390/photonics9070506
Wan Z, Shi Z, Liu Q, Fu X. Holographic Tailoring of Structured Light Field with Digital Device. Photonics. 2022; 9(7):506. https://doi.org/10.3390/photonics9070506
Chicago/Turabian StyleWan, Zhensong, Zijian Shi, Qiang Liu, and Xing Fu. 2022. "Holographic Tailoring of Structured Light Field with Digital Device" Photonics 9, no. 7: 506. https://doi.org/10.3390/photonics9070506
APA StyleWan, Z., Shi, Z., Liu, Q., & Fu, X. (2022). Holographic Tailoring of Structured Light Field with Digital Device. Photonics, 9(7), 506. https://doi.org/10.3390/photonics9070506





