Effect of Granule Sizes on Acoustic Properties of Protein-Based Silica Aerogel Composites via Novel Inferential Transmission Loss Method
Abstract
:1. Introduction
2. Results
2.1. Silica Aerogel (SA) Granule Optimization
2.2. Transmission Loss of SA Granules
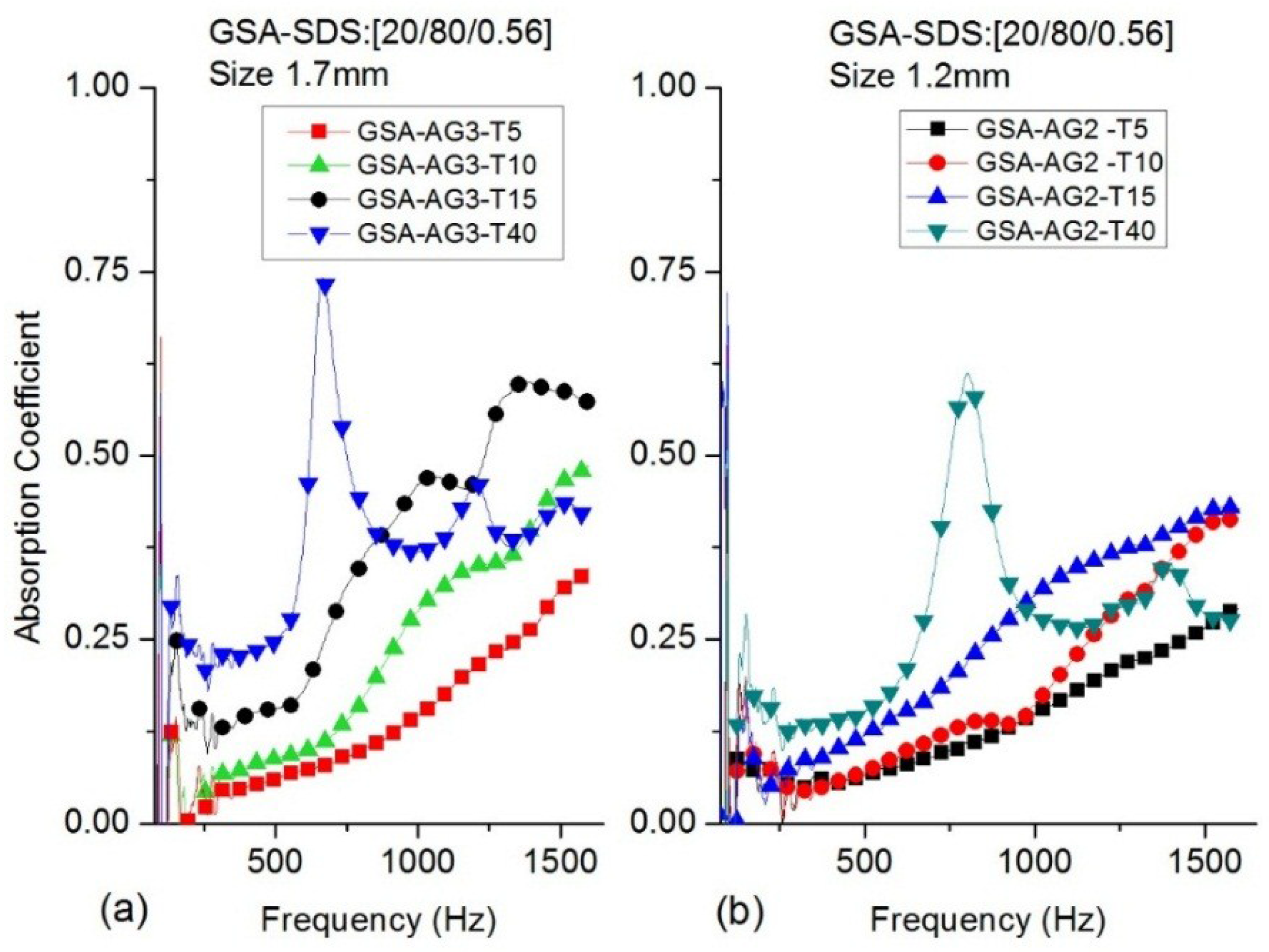
2.3. Acoustic Performance of Gelatin Silica Aerogels Doped with Sodium Dodecyl Sulfate (GSA–SDS)
2.4. Comparative Analysis with Other Traditional Materials
2.5. Acoustic Activity
3. Conclusions
4. Experimental Procedures
4.1. Specimen Preparation
4.2. Transfer Function Method (Two-Microphone)
- and are the sound amplitude of the incident and reflected pressure respectively.
- is the wavenumber of the incident sound pressure, therefore
- f is the frequency and is the speed of sound.
- are the distances from the specimen to the microphones 1 and 2, respectively.
4.3. Inferential Transmission Loss Method (InTLM)
4.4. Sound Meter Measurements
5. Material and Equipment
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Gesser, H.D.; Goswami, P.C. Aerogels and related porous materials. Chem. Rev. 1989, 89, 765–788. [Google Scholar] [CrossRef]
- Hunt, A.J.; Jantzen, C.A.; Cao, W. Aerogel. A High Performance Insulating Material at 0.1 Bar. In Insulation Materials: Testing and Applications; ASTM: Gatlinburg, TN, USA, 1991; pp. 455–463. [Google Scholar]
- Kistler, S.S.; Fischer, E.A.; Freeman, I.R. Sorption and surface area in silica aerogel. J. Am. Chem. Soc. 1943, 65, 1909–1919. [Google Scholar] [CrossRef]
- Koebel, M.; Rigacci, A.; Achard, P. Aerogel-based thermal superinsulation: An overview. J. Sol-Gel Sci. Technol. 2012, 63, 315–339. [Google Scholar] [CrossRef]
- Zu, G.Q.; Shen, J.; Wei, X.Q.; Ni, X.Y.; Zhang, Z.H.; Wang, J.C.; Liu, G.W. Preparation and characterization of monolithic alumina aerogels. J. Non-Crystalline Solids 2011, 357, 2903–2906. [Google Scholar] [CrossRef]
- Juzkow, M. Aerogel capacitors support pulse, hold-up, and main power applications. Power Electron. Technol. 2002, 28, 58–60. [Google Scholar]
- Nuckols, M.L.; Chao, J.C.; Swiergosz, M.J. Manned Evaluation of a Prototype Composite Cold Water Diving Garment Using Liquids and Superinsulation Aerogel Materials; Navy Experimental Diving Unit, Naval Sea Systems Command: Panama, FL, USA, 2005; p. 28. [Google Scholar]
- Erik, B.; Joseph, M.; David, P. Thermal resistance and compressive strain of underwater aerogel-syntactic foam hybrid insulation at atmospheric and elevated hydrostatic pressure. J. Phys. D Appl. Phys. 2006, 39, 1908. [Google Scholar]
- Kobayashi, Y.; Saito, T.; Isogai, A. Aerogels with 3D ordered nanofiber skeletons of liquid-crystalline nanocellulose derivatives as tough and transparent insulators. Angew. Chem. Int. Ed. 2014, 53, 10394–10397. [Google Scholar] [CrossRef] [PubMed]
- Wang, D.; Zhang, F.; Tang, J. Sodium alginate decorated carbon nanotubes-graphene composite aerogel for heavy metal ions detection. Electrochemistry 2015, 83, 84–90. [Google Scholar] [CrossRef]
- Bag, S.; Trikalitis, P.N.; Chupas, P.J.; Armatas, G.S.; Kanatzidis, M.G. Porous semiconducting gels and aerogels from chalcogenide clusters. Science 2007, 317, 490–493. [Google Scholar] [CrossRef] [PubMed]
- Ratke, L.; Brück, S. Mechanical properties of aerogel composites for casting purposes. J. Mater. Sci. 2006, 41, 1019–1024. [Google Scholar] [CrossRef]
- Hong, C.-Q.; Han, J.-C.; Zhang, X.-H.; Du, J.-C. Novel nanoporous silica aerogel impregnated highly porous ceramics with low thermal conductivity and enhanced mechanical properties. Scr. Mater. 2013, 68, 599–602. [Google Scholar] [CrossRef]
- Gurav, J.L.; Jung, I.-K.; Park, H.-H.; Kang, E.S.; Nadargi, D.Y. Silica aerogel: Synthesis and applications. J. Nanomater. 2010, 2010, 11. [Google Scholar] [CrossRef]
- Schmidt, M.; Schwertfeger, F. Applications for silica aerogel products. J. Non-Crystalline Solids 1998, 225, 364–368. [Google Scholar] [CrossRef]
- Gibiat, V.; Lefeuvre, O.; Woignier, T.; Pelous, J.; Phalippou, J. Acoustic properties and potential applications of silica aerogels. J. Non-Crystalline Solids 1995, 186, 244–255. [Google Scholar] [CrossRef]
- Clark, S. World Aerogel Market—Opportunities and Forecasts, 2013 to 2020; Allied Market Research: Portland, OR, USA, 2013; p. 157. [Google Scholar]
- Rao, A.V.; Kulkarni, M.M.; Pajonk, G.M.; Amalnerkar, D.P.; Seth, T. Synthesis and characterization of hydrophobic silica aerogels using trimethylethoxysilane as a co-precursor. J. Sol-Gel Sci. Technol. 2003, 27, 103–109. [Google Scholar] [CrossRef]
- Bhagat, S.D.; Rao, A.V. Surface chemical modification of teos based silica aerogels synthesized by two step (acid–base) sol–gel process. Appl. Surf. Sci. 2006, 252, 4289–4297. [Google Scholar] [CrossRef]
- Bhagat, S.D.; Kim, Y.-H.; Moon, M.-J.; Ahn, Y.-S.; Yeo, J.-G. A cost-effective and fast synthesis of nanoporous sio2 aerogel powders using water-glass via ambient pressure drying route. Solid State Sci. 2007, 9, 628–635. [Google Scholar] [CrossRef]
- Mahesh, S.; Joshi, S.C. Thermal conductivity variations with composition of gelatin-silica aerogel-sodium dodecyl sulfate with functionalized multi-walled carbon nanotube doping in their composites. Int. J. Heat Mass Transf. 2015, 87, 606–615. [Google Scholar] [CrossRef]
- Gronauer, M.; Fricke, J. Acoustic properties of microporous sio-aerogel. Acta Acust. United Acust. 1986, 59, 177–181. [Google Scholar]
- Fricke, J.; Reichenauer, G. Thermal. Acoustical and structural properties of silica aerogels. MRS Proc. 2011, 73, 775. [Google Scholar] [CrossRef]
- Gross, J.; Fricke, J.; Hrubesh, L.W. Sound-propagation in sio2 aerogels. J. Acoust. Soc. Am. 1992, 91, 2004–2006. [Google Scholar] [CrossRef]
- Forest, L.; Gibiat, V.; Hooley, A. Impedance matching and acoustic absorption in granular layers of silica aerogels. J. Non-Crystalline Solids 2001, 285, 230–235. [Google Scholar] [CrossRef]
- Cotana, F.; Pisello, A.L.; Moretti, E.; Buratti, C. Multipurpose characterization of glazing systems with silica aerogel: In-field experimental analysis of thermal-energy, lighting and acoustic performance. Build. Environ. 2014, 81, 92–102. [Google Scholar] [CrossRef]
- Buratti, C.; Moretti, E. Silica nanogel for energy-efficient windows. In Nanotechnology in Eco-Efficient Construction; Pacheco-Torgal, F., Diamanti, M.V., Nazari, A., Granqvist, C.G., Eds.; Woodhead Publishing: Cambridge, UK, 2013; Charpter10; pp. 207–235. [Google Scholar]
- Forest, L.; Gibiat, V.; Woignier, T. Evolution of the acoustical properties of silica alcogels during their formation. Ultrasonics 1998, 36, 477–481. [Google Scholar] [CrossRef]
- Kim, Y.-H. Vibration and waves. In Sound Propagation; John Wiley & Sons, Ltd: New York, NY, USA, 2010; pp. 1–68. [Google Scholar]
- Riffat, S.B.; Qiu, G. A review of state-of-the-art aerogel applications in buildings. Int. J. Low-Carbon Technol. 2013, 8, 1–6. [Google Scholar] [CrossRef]
- Vigran, T.E. Normal incidence sound transmission loss in impedance tube—Measurement and prediction methods using perforated plates. Appl. Acoust. 2012, 73, 454–459. [Google Scholar] [CrossRef]
- Smith, C.D.; Parrott, T.L. Comparison of three methods for measuring acoustic properties of bulk materials. J. Acoust. Soc. Am. 1983, 74, 1577–1582. [Google Scholar] [CrossRef]
- Palumbo, D.L.; Jones, M.G.; Klos, J. Improvements to the two-thickness method for deriving acoustic properties of materials. In Proceeding of the NOISE Conference 2004, Baltimore, MA, USA, 9 April 2004; National Technical Information Service: Baltimore, MA, USA, 2004. [Google Scholar]
- Sachithanadam, M.; Joshi, S.C. High strain recovery with improved mechanical properties of gelatin-silica aerogel composites post-binding treatment. J. Mater. Sci. 2013, 49, 163–179. [Google Scholar] [CrossRef]
- Sachithanadam, M.; Joshi, S.C. A new phenomenon of compressive strain recovery in gelatin-silica aerogel composites with SDS. Procedia Eng. 2014, 75, 51–55. [Google Scholar] [CrossRef]
- Kim, Y.-H. Acoustic wave equation and its basic physical measures. In Sound Propagation; John Wiley & Sons, Ltd.: New York, NY, USA, 2010; pp. 69–128. [Google Scholar]
- Dowling, A.P.; Williams, J.E.F. Sound and Sources of Sound; Ellis Horwood: West Sussex, England, 1983. [Google Scholar]
- Basirjafari, S.; Malekfar, R.; Esmaielzadeh Khadem, S. Low loading of carbon nanotubes to enhance acoustical properties of poly(ether)urethane foams. J. Appl. Phys. 2012, 112, 104312. [Google Scholar] [CrossRef]
- Verdejo, R.; Stämpfli, R.; Alvarez-Lainez, M.; Mourad, S.; Rodriguez-Perez, M.A.; Brühwiler, P.A.; Shaffer, M. Enhanced acoustic damping in flexible polyurethane foams filled with carbon nanotubes. Compos. Sci. Technol. 2009, 69, 1564–1569. [Google Scholar] [CrossRef]
- Jung, S.S.; Kim, Y.T.; Lee, Y.B.; Cho, S.I.; Lee, J.K. Measurement of sound transmission loss by using impedance tubes. J. Korean Phys. Soc. 2008, 53, 596–600. [Google Scholar] [CrossRef]
- Feng, L. Modified impedance tube measurements and energy dissipation inside absorptive materials. Appl. Acoust. 2013, 74, 1480–1485. [Google Scholar] [CrossRef]
- Henderson, W.; Goggans, P.; Xiang, N.; Botts, J. Bayesian inference approach to room-acoustic modal analysis. AIP Conf. Proc. 2013, 1553, 38–45. [Google Scholar]
- Botts, J.; Xiang, N. Bayesian inference for acoustic impedance boundaries in room-acoustic finite difference time-domain modeling. AIP Conf. Proc. 2012, 1443, 306–313. [Google Scholar]
- Robinson, P.; Xiang, N. On the subtraction method for in-situ reflection and diffusion coefficient measurements. J. Acoust. Soc. Am. 2010, 127, EL99–EL104. [Google Scholar] [CrossRef] [PubMed]
- Azevedo, M.; Xiang, N.; Fackler, C. Low frequency absorption and attenuation of granular aerogel composites. In Proceedings of the 40th International Congress and Exposition on Noise Control Engineering 2011, INTER-NOISE 2011, Osaka, Japan, 4–7 September 2011; pp. 2038–2043.
- Fackler, C.; Xiang, N.; Churu, G.; Mohite, D.P.; Leventis, N.; Sotiriou-Leventis, C.; Lu, H. Experimental investigation of the acoustic attenuation by monolithic polyurea aerogels. In Proceedings of the 41st International Congress and Exposition on Noise Control Engineering 2012, INTER-NOISE 2012, New York, NY, USA, 19–22 August 2012; pp. 5883–5887.
- ASTM International. Standard Test Method for Impedance and Absorption of Acoustical Materials Using a Tube, Two Microphones and a Digital Frequency Analysis System; ASTM E1050-12; ASTM International: West Conshohocken, PA, USA, 2012. [Google Scholar]











| Classification | Absorption Coefficient (10 mm) | Absorption Coefficient (15 mm) | ||
|---|---|---|---|---|
| 800 Hz | 1600 Hz | 800 Hz | 1600 Hz | |
| AG1 | 0.14 | 0.31 | 0.14 | 0.31 |
| AG2 | 0.14 | 0.32 | 0.20 | 0.52 |
| AG3 | 0.14 | 0.29 | 0.21 | 0.48 |
| AG4 | 0.10 | 0.23 | 0.20 | 0.47 |
| AG5 | 0.10 | 0.25 | 0.10 | 0.24 |
| AG6 | 0.11 | 0.24 | 0.11 | 0.24 |
| AGMX | 0.11 | 0.25 | 0.20 | 0.48 |
| Air | 0.00 | 0.00 | 0.00 | 0.00 |
| Porous Ply | 0.01 | 0.01 | 0.01 | 0.01 |
| Granule Size (mm) | Density (g/cm3) | No. of Layers | Thickness, T (mm) | Frequency (Hz) | Absorption Coefficient | Transmission Loss (dB) | TL(avg) (dB) | Sound Meter TL(avg) (dB) |
|---|---|---|---|---|---|---|---|---|
| GSA–AG2 (1.2 mm) | 0.079 | 1 | 5 | 800/1600 | 0.11/0.29 | 26.0/11.2 | 11.3 | 11.0 |
| 0.073 | 1 | 10 | 800/1600 | 0.14/0.41 | 12.0/15.5 | 11.7 | 12.8 | |
| 0.071 | 1 | 15 | 800/1600 | 0.22/0.43 | 15.2/12.6 | 14.6 | 11.3 | |
| 0.074 | 4 | 40 | 800/1600 | 0.61/0.28 | 15.7/18.6 | 16.4 | 15.2 | |
| GSA–AG3 (1.7 mm) | 0.084 | 1 | 5 | 800/1600 | 0.10/0.34 | 16.1/12.5 | 10.7 | 10.9 |
| 0.074 | 1 | 10 | 800/1600 | 0.17/0.49 | 16.9/13.3 | 11.8 | 12.6 | |
| 0.072 | 1 | 15 | 800/1600 | 0.36/0.57 | 11.4/15.8 | 14.5 | 13.3 | |
| 0.075 | 4 | 40 | 800/1600 | 0.43/0.42 | 33.0/42.9 | 20.3 | 18.6 |
| Classification | Size Range d (mm) | Median d (cm) | Density (g/cm3) | Distribution % | Aspect Ratio |
|---|---|---|---|---|---|
| AG1 | 0.50 ≤ x < 1.00 | 0.075 | 0.0682 | 2.10 | 1.98 |
| AG2 | 1.00 ≤ x < 1.40 | 0.12 | 0.0693 | 7.01 | 1.39 |
| AG3 | 1.40 ≤ x < 2.00 | 0.17 | 0.0719 | 63.15 | 1.42 |
| AG4 | 2.00 ≤ x < 2.36 | 0.218 | 0.0727 | 19.69 | 1.18 |
| AG5 | 2.36 ≤ x < 2.8 | 0.258 | 0.0732 | 5.96 | 1.18 |
| AG6 | 2.8 ≤ x < 3.35 | 0.307 | 0.0748 | 1.22 | 1.19 |
| AGMX | 0.10 < x ≤ 4.0 | 0.200 | 0.0723 | 100.0 | 1.35 |
| Classification | Type | Density (g/cm3) |
|---|---|---|
| Grey FPF | Foam, flexible | 0.0068 |
| Acrylic | Transparent, rigid, hard | 1.2072 |
| HeraDesign Inc Magnesite wood wool | Normal | 0.4082 |
| Coated with smooth micro-ceramic layer | 0.6395 | |
| Coated with rough micro-ceramic layer | 0.5660 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sachithanadam, M.; Joshi, S.C. Effect of Granule Sizes on Acoustic Properties of Protein-Based Silica Aerogel Composites via Novel Inferential Transmission Loss Method. Gels 2016, 2, 11. https://doi.org/10.3390/gels2010011
Sachithanadam M, Joshi SC. Effect of Granule Sizes on Acoustic Properties of Protein-Based Silica Aerogel Composites via Novel Inferential Transmission Loss Method. Gels. 2016; 2(1):11. https://doi.org/10.3390/gels2010011
Chicago/Turabian StyleSachithanadam, Mahesh, and Sunil C. Joshi. 2016. "Effect of Granule Sizes on Acoustic Properties of Protein-Based Silica Aerogel Composites via Novel Inferential Transmission Loss Method" Gels 2, no. 1: 11. https://doi.org/10.3390/gels2010011
APA StyleSachithanadam, M., & Joshi, S. C. (2016). Effect of Granule Sizes on Acoustic Properties of Protein-Based Silica Aerogel Composites via Novel Inferential Transmission Loss Method. Gels, 2(1), 11. https://doi.org/10.3390/gels2010011








