The Twofold Role of 12-Hydroxyoctadecanoic Acid (12-HOA) in a Ternary Water—Surfactant—12-HOA System: Gelator and Co-Surfactant

Abstract

Gelled lyotropic liquid crystals can be formed by adding a gelator to a mixture of surfactant and solvent. If the gel network and the liquid-crystalline phase coexist without influencing each other, the self-assembly is called orthogonal. In this study, the influence of the organogelator 12-hydroxyoctadecanoic acid (12-HOA) on the lamellar and hexagonal liquid crystalline phases of the binary system H₂O–C₁₂E₇ (heptaethylene glycol monododecyl ether) is investigated. More precisely, we added 12-HOA at mass fractions from 0.015 to 0.05 and studied the resulting phase diagram of the system H₂O–C₁₂E₇ by visual observation of birefringence and by ²H NMR spectroscopy. In addition, the dynamic shear moduli of the samples were measured in order to examine their gel character. The results show that 12-HOA is partly acting as co-surfactant, manifested by the destabilization of the hexagonal phase and the stabilization of the lamellar phase. The higher the total surfactant concentration, the more 12-HOA is incorporated in the surfactant layer. Accordingly, its gelation capacity is substantially reduced in the surfactant solution compared to the system 12-HOA–n-decane, and large amounts of gelator are required for gels to form, especially in the lamellar phase.

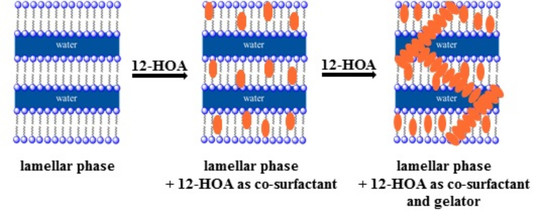
1. Introduction

Gelled complex fluids [1], in which the mechanical stability of a gel is combined with the microstructure of a complex fluid, are interesting candidates for (trans-)dermal drug delivery systems and tissue healing applications [1,2]. Additional applications of this class of materials can be found in a recent review by Stubenrauch and Gießelmann [1]. Gelled complex fluids can be obtained by either adding a gelator to a complex fluid or by replacing the solvent of a gel by a complex fluid. A special class of gelled complex fluids are gelled lyotropic liquid crystals (gelled LCs), such as gelled lamellar phases L_α (gelled L_α). Examples of gelled L_α phases_, known in literature are the cell [3], lamellar biogels [4], and gelled lamellar phases of synthetic surfactants. Only two systems of the latter type have been studied to date: The gelled lamellar phase of the system H₂O–n-decane/12-hydroxyoctadecanoic acid (12-HOA)—tetraethylene glycol monodecyl ether (C₁₀E₄) [5] and the gelled lamellar phase L_α of the system H₂O–2C₁₂DAB–12-HOA [6]. The cell surely is the most prominent example. Proteins form filaments, which provide mechanical stability of the cell and coexist independently with the lipid bilayer of phospholipids, i.e., the building block of the cell membrane [3]. Warriner et al. introduced lamellar biological hydrogels that consist of the lipid dimyristoyl phosphatidyl choline (DMPC), the co-surfactant pentanol and tiny amounts of poly (ethylene glycol)-derived polymer lipids (PEG-lipids) [4]. The domains of the lamellar membranes built by DMPC and pentanol are randomly orientated and provide the gel properties, while the PEG-lipids are attached to these membranes, but can diffuse freely within the lamellar bilayers, which are separated by water. These PEG-lipids stabilize defects occurring in high curvature regions and lead to highly flexible membranes with large distances from each other caused by long-range repulsive forces of the PEG-lipids. Xu et al. studied the gelled lamellar phase L_α of the system H₂O–n-decane/12-HOA–C₁₀E₄ with a focus on the question whether the formation of the gel network and of the lamellar phase formation is simultaneous, but independent [5]. Koitani et al. tried to answer the same question by studying the lamellar phase L_α of the system H₂O–2C₁₂DAB–12-HOA [6].

Introduced by Laibinis et al. for alkanethiols and alkane carboxylic acids on gold and alumina, respectively, the simultaneous (but independent) formation of two coexisting structures is called orthogonal self-assembly [7]. However, the term orthogonal self-assembly is not restricted to surface chemistry. Orthogonal self-assembled structures have been studied in bulk systems as well [8,9,10,11,12]. The cell, once again, is the most prominent example of an orthogonal self-assembled system, since proteins self-assemble to fibers forming the cytoskeleton, while phospholipids self-assemble to membranes [3]. The expression orthogonal self-assembly was first used for gelled complex fluids by the group of van Esch [13]. They reported on surfactant micelles, worm-like micelles, liposomes and phospholipids entrapped in self-assembled fibrillary networks of low molecular weight gelators (LMWG) [13,14,15,16,17]. Laupheimer studied the system H₂O–n-decane/12-HOA–C₁₀E₄ and proved that the gelled bicontinuous microemulsion is an orthogonal self-assembled system in which the formation of the nanostructured microemulsion is nearly independent on the formation of the gel network [18,19,20]. In the special case of the gelled L_α phase of the very same system, the question whether it is a truly orthogonal self-assembled system could not be answered satisfactorily. On the one hand, phase behaviour and rheological studies supported the idea of an orthogonal self-assembled gelled lamellar L_α phase. On the other hand, freeze fracture electron microscopy revealed that the gel network and the lamellar L_α phase influence each other: The presence of the gel network leads to a higher order of the L_α phase while the presence of the L_α phase results in gel fibers which are not twisted anymore [5]. Moreover, Laupheimer et al. showed in a previous SANS study of the system H₂O–n-decane/12-HOA–C₁₀E₄ that 12-HOA is only partly involved in the gel formation, since it also acts as co-surfactant, which results in a shift of the phase boundaries to lower temperatures [20]. Koitani et al. studied the gelled lamellar phase L_α of the system H₂O–didodecyldimethylammonium bromide (2C₁₂DAB)–12-HOA at surfactant mass fractions γ_a = 0.10 and γ_a = 0.20 in order to answer the question of orthogonal self-assembly [6]. However, they also found that 12-HOA seems to have two roles in surfactant-containing systems, namely as gelator and as co-surfactant. The slightly surface active 12-HOA molecules are partly incorporated in the surfactant bilayers of the system H₂O–2C₁₂DAB–12-HOA and are thus not available for the gel formation which leads to a weaker gel compared to the binary gel n-decane–12-HOA. Additionally, the incorporation of 12-HOA in the bilayers caused a slightly larger interlayer spacing compared to the non-gelled L_α phase. In order to elucidate the role of 12-HOA in surfactant-containing systems and its ability of gelling lyotropic liquid crystals of different phase structures, we chose the binary system H₂O–heptaethylene glycol monododecyl ether (C₁₂E₇) instead of the ternary system H₂O–n-decane–C₁₀E₄ or the system H₂O–2C₁₂DAB, for the following reasons. Firstly, studying the system H₂O–C₁₂E₇ ensures easy handling and a broader access to gelled lyotropic liquid crystals as it forms three lyotropic liquid crystalline phases, namely the hexagonal phase H₁, the bicontinuous cubic phase V₁ and the lamellar phase L_α in specific surfactant concentration ranges and with melting points at moderate temperatures (T ≈ 50 °C) [21]. Secondly, it allows us to prove the concept of gelling lyotropic liquid crystals of binary water–surfactant systems with the organogelator 12-HOA at much higher surfactant concentrations as compared to the binary system H₂O–2C₁₂DAB. We first investigated the influence of the 12-HOA concentration on the phase boundaries of the system H₂O–C₁₂E₇ by means of visual observation in water basins and determined qualitatively whether a gelled lyotropic liquid crystalline phase was formed. ²H NMR spectroscopy complemented the visual phase studies, since it is a well-known and powerful tool to detect phase transitions of lyotropic liquid crystalline phases to an isotropic phase and the coexistence of two phases, respectively [22,23,24,25,26,27,28,29,30]. In addition, we investigated the rheological properties of the lyotropic liquid crystalline phases in the presence of 12-HOA in order to identify gelled lyotropic liquid crystalline phases.

2. Results and Discussion

2.1. Visual Phase Studies

The visual phase studies allowed us to determine the occurrence of lyotropic liquid crystals via optical birefringence. In addition, they helped identifying transition temperatures, namely the melting points of the lyotropic liquid crystals and the sol-gel transition of the gel network. Therefore, we were able to measure the influence of 12-HOA on the phase boundaries of the lyotropic liquid crystalline phases of the system H₂O–C₁₂E₇. As already mentioned, the system H₂O–C₁₂E₇ forms three liquid crystalline phases as a function of the surfactant concentration, namely the hexagonal phase H₁, the bicontinuous cubic phase V₁ and the lamellar phase L_α. The visually determined T-γ_a phase diagram of the binary system H₂O–C₁₂E₇, as well as those of the systems in the presence of 12-HOA, i.e., H₂O–C₁₂E₇–12-HOA with 12-HOA mass fractions η = 0.015, 0.025, 0.05, are shown in Figure 1.

The black circles refer to the binary system H₂O–C₁₂E₇ and we note that the phase boundaries are in good agreement with literature data [21]. The highly viscous and transparent hexagonal H₁ phase occurs at a surfactant concentration range of γ_a ≈ 0.40–0.68 and melts at temperatures T ≈ 50 °C. At higher surfactant concentrations, in a range of γ_a ≈ 0.72–0.85, the lamellar L_α phase is formed. The L_α phase melts at about the same temperatures as the hexagonal H₁ phase, but is less viscous and appears slightly turbid. The highly viscous, transparent bicontinuous cubic phase V₁ occurs between the hexagonal phase H₁ and the lamellar phase L_α in a surfactant concentration range γ_α ≈ 0.68–0.72 and melts at temperatures T ≈ 46 °C. In contrast to the anisotropic H₁ and L_α phases, the bicontinuous cubic phase V₁ is characterized by optical isotropy. The V₁ phase will not be discussed further as it is difficult to identify, especially in the presence of 12-HOA. The upper miscibility gap (2φ), which is typical for oligo (ethylene oxide) alkyl ethers, was detected at higher temperatures for surfactant concentrations up to γ_a = 0.50.

The open circles in Figure 1 represent the phase boundaries of the system H₂O–C₁₂E₇ at three different 12-HOA concentrations. Comparing the binary system with the systems in the presence of 12-HOA, it is obvious that 12-HOA has an enormous influence on the phase boundaries of the liquid crystalline phases. For a 12-HOA mass fraction of η = 0.015, the melting point of the lamellar phase L_α is increased by ΔT ≈ 5 K, whereas at the same time the melting point of the hexagonal phase H₁ is decreased by the same value. The upper miscibility gap is also shifted towards lower temperatures in the presence of 12-HOA. The influence of 12-HOA on the upper miscibility gap at surfactant concentrations γ_a < 0.3 could not be determined via visual observations, because 12-HOA precipitated at these surfactant concentrations. After addition of 12-HOA (η = 0.015) the appearance and viscosity of the lamellar phase L_α did not change. It maintained its slight turbidity and also its flow ability, i.e., no gelled lamellar L_α phase was formed. On the contrary, the initially transparent hexagonal phase H₁ became turbid after addition of 12-HOA (η = 0.015), but whether a gel was formed, could not be detected by visual observation, because the viscosity of the hexagonal phase H₁ is high a priori. Moreover, the turbidity of the hexagonal phase complicated the visual observation, especially at low temperatures. For a 12-HOA concentration of η = 0.025 we observed the same trend, i.e., a stabilized lamellar L_α phase and a destabilized hexagonal H₁ phase, but a bit more pronounced as for η = 0.015. Again, even after increasing the 12-HOA concentration to η = 0.025, the appearance of the lamellar phase L_α did not change, i.e., still no gelled lamellar phase L_α was formed. In the case of the hexagonal phase H₁, the effect of 12-HOA is also slightly more pronounced for η = 0.025 than for η = 0.015. The hexagonal H₁ phase is marginally less temperature stable for η = 0.025, but again its appearance changes from transparent to turbid. For the highest 12-HOA concentration, i.e., η = 0.05, the lamellar phase L_α is extended to higher temperatures and lower surfactant concentrations γ_a, i.e., it additionally occurs in the range of γ_a = 0.40–0.60 at temperatures T = 50–70 °C. Unlike for η = 0.015 and 0.025, gelled L_α phases were obtained for η = 0.05 in a surfactant concentration range of γ_a = 0.72–0.85. In addition, gelled isotropic solutions were formed at lower concentrations of γ_a = 0.40–0.60, since the hexagonal H₁ phase did not form anymore in the presence of 12-HOA at η = 0.05. In Figure 1, bottom, the approximate sol-gel transition temperature line is added to the phase diagram of the system H₂O–C₁₂E₇–12-HOA (η = 0.05) and it can be seen that the sol-gel transition temperatures decrease with increasing surfactant concentration γ_a.

Based on these results it is clear that 12-HOA has two roles in the system H₂O–C₁₂E₇–12-HOA. On the one hand, it acts as co-surfactant, which results in the stabilization of the lamellar phase L_α and at the same time in the destabilization of the hexagonal phase H₁. This effect can be explained by Israelachvili’s packing parameter, which is the ratio of hydrophobic area to the effective hydrophilic head group area [31]. By adding 12-HOA to the system H₂O–C₁₂E₇, a C₁₈ chain is introduced which increases the volume of the hydrophobic chains. As a consequence, the packing parameter is increased, and the surfactant layer has a lower curvature. This stabilizes the lamellar phase L_α, and simultaneously destabilizes the hexagonal phase H₁ [31,32,33]. On the other hand, it seems that at sufficient high gelator concentrations, i.e., for η > 0.025–0.05, the bilayers of the lamellar phase are saturated by 12-HOA and 12-HOA starts to act as gelator. The sol-gel transition temperature of the system H₂O–C₁₂E₇–12-HOA (η = 0.05) decreases with increasing surfactant concentration γ_a which can be explained by an increasing surfactant layer at higher surfactant concentrations γ_a. The higher the surfactant concentrations γ_a, the more likely 12-HOA is incorporated in the surfactant layer instead of forming the gelator network, which leads to weaker gels with lower sol-gel transition temperatures T_sol-gel. The system H₂O–C₁₂E₇ thus is a better solvent for 12-HOA at high surfactant concentrations γ_a than at low ones. This concentration dependence is already evident at the lowest 12-HOA concentration η = 0.015. While the appearance of the lamellar L_α phase at high surfactant mass fractions does not change in the presence of 12-HOA at η = 0.015, the otherwise clear hexagonal H₁ phase becomes turbid, which may indicate the start of a gel formation. Therefore, we focused our study on the system H₂O–C₁₂E₇–12-HOA at η = 0.015, in order to answer the question whether 12-HOA acts as both gelator and co-surfactant already at the lowest 12-HOA mass fraction.

2.2. ²H NMR

We performed ²H NMR measurements in order to complement the visual phase studies of the system H₂O–C₁₂E₇–12-HOA at η = 0.015 as the determination of the phase boundary of the hexagonal phase H₁ at surfactant concentrations γ_a ≈ 0.40 is difficult by means of visual observation, in particular, in the presence of 12-HOA which makes the sample turbid. For this purpose, H₂O needed to be replaced by D₂O, which, in turn, requires a phase study of D₂O–C₁₂E₇. The phase diagram of D₂O–C₁₂E₇ is available in the Appendix A (Figure A1). It was found that the lyotropic liquid crystalline phases were shifted to slightly smaller surfactant mass fractions γ_a. Moreover, the phase boundary of the hexagonal phase H₁ was shifted by ΔT = 1–2 °C to lower values, whereas the phase boundary of the lamellar phase L_α was shifted to higher temperatures by ΔT = 2–3 °C. The miscibility gap at low surfactant concentrations and at high temperatures was shifted to lower temperatures by ΔT = 2–3 °C, i.e., it seems that the surfactant is more hydrophobic in the presence of D₂O [29]. The temperature shifts caused by replacing H₂O by D₂O are the same for the systems without and with 12-HOA. We performed temperature dependent ²H NMR measurements at γ_a = 0.37 and 0.38 for the system D₂O–C₁₂E₇ and at γ_a = 0.36 and 0.38 for the system D₂O–C₁₂E₇–12-HOA (η = 0.015). In the following, we will discuss the ²H NMR spectra of the systems D₂O–C₁₂E₇ and D₂O–C₁₂E₇–12-HOA (η = 0.015) at γ_a = 0.38. The spectra of the systems D₂O–C₁₂E₇ at γ_a = 0.37 and D₂O–C₁₂E₇–12-HOA (η = 0.015) at γ_a = 0.36 are shown in the Appendix A (Figure A2).

In the anisotropic hexagonal phase H₁, the quadrupolar ²H nucleus with a spin quantum number I = 1 shows a splitting which depends on the local orientation order of the D₂O molecules, as well as on the orientation of the director of the hexagonal phase with respect to the magnetic field. The D₂O molecules are on average slightly aligned, since they interact with the aligned surfactant molecules resulting in a non-zero residual quadrupole coupling. Hexagonal phases H₁ are not macroscopically aligned by the magnetic field, because of their high viscosity, i.e., domains with all possible orientations of the hexagonal axis are present in the sample. The superposition of the spectral contributions from all domains leads to a characteristic line shape, called the Pake powder pattern [34]. On the contrary, isotropic phases are indicated by a single peak, since the temporal average over the random orientations of the molecules, which have no preferred orientation with respect to the magnetic field eliminates the quadrupole coupling.

In Figure 2a, the ²H NMR spectra of the system D₂O–C₁₂E₇ at γ_a = 0.38 as a function of temperature are shown. The bottom two spectra show the Pake powder pattern with two inner peaks and two outer shoulders, corresponding to domains with the hexagonal axis perpendicular and parallel to the magnetic field, respectively. The shape of the Pake pattern represents the isotropic distribution of domains in a macroscopically unaligned sample. As we studied the system D₂O–C₁₂E₇ by means of visual observation, and since the general phase behavior is known in literature, the splitting can be assigned to the hexagonal phase H₁. The spectrum at T = 33 °C shows an additional peak that can be assigned to an isotropic phase that coexists with the hexagonal phase. The two phase region can be detected up to T = 40 °C, though the peaks that indicate the hexagonal phase are at minimum intensity. At T = 41 °C the single peak corresponds to an isotropic phase, therefore this temperature is set as phase transition temperature from the hexagonal phase H₁ to the isotropic phase. The ²H NMR spectra of the system D₂O–C₁₂E₇ at γ_a = 0.37 shown in the Appendix A indicate that the system consists of an isotropic and a hexagonal phase H₁ from room temperature up to T = 42 °C.

The respective temperature dependent ²H NMR spectra of the system D₂O–C₁₂E₇–12-HOA (η = 0.015) at γ_a = 0.38 are shown in Figure 2b. As opposed to the spectra of the system D₂O–C₁₂E₇, the line shape is less sharp in the presence of 12-HOA, though the general phase behavior is maintained in presence of 12-HOA, but shifted to lower temperatures by ΔT = 8 °C, which is in accordance with the visual phase studies. At T = 25 °C up to T = 31 °C again the Pake pattern can be observed and can also be assigned to the hexagonal phase H₁. At T > 32 °C an additional peak occurs, which can be assigned to the isotropic phase. At T = 34 °C, the Pake spectrum is still visible, indicating that the system is still in the two phase region. The phase transition temperature from the hexagonal phase H₁ to the isotropic phase was set to T = 35 °C—since a single peak, indicative of a pure isotropic phase, was recorded. In the Appendix A the spectra of the 12-HOA containing sample at γ_a = 0.36 are shown.

In Figure 3, the phase transitions determined by ²H NMR are added to the phase diagram of the system H₂O–C₁₂E₇–12-HOA (η = 0.015) determined by visual observation in water basins (see Figure 1, top). We added ΔT = +1 to the phase transitions determined by ²H NMR before we added them to the phase diagram, since D₂O influences the phase boundaries in that way (see Figure A1). The results of visual observations and ²H NMR are in good agreement and complement each other. We were able to detect the phase boundaries of the hexagonal phase H₁ at γ_a < 0.40 in the presence of 12-HOA, which was difficult by means of visual observations, due to turbidity caused by 12-HOA. Moreover, the ²H NMR measurements enabled the detection of the two-phase region of isotropic and hexagonal phase at γ_a = 0.38–0.40. To summarize, ²H NMR measurements confirm the phase behavior and the role of 12-HOA as co-surfactant determined by visual observations.

2.3. Rheometry

Looking at the phase diagrams and the NMR spectra one can conclude that 12-HOA acts as co-surfactant up to η = 0.015. Somewhere between a 12-HOA concentration of η = 0.025 and η = 0.05 its second role of a gelator becomes obvious. However, at the lowest 12-HOA concentration of η = 0.015, the hexagonal H₁ phase went turbid as discussed in Section 2.1, which could indicate the beginning of a gel formation. In other words, it can well be that 12-HOA acts already as both co-surfactant and gelator at η = 0.015. In order to determine whether or not the hexagonal phase H₁ was gelled at a 12-HOA concentration of η = 0.015, we performed frequency sweeps by oscillation shear rheometry and compared the results with the pure hexagonal phase H₁ at γ_a = 0.50 and with the binary gel n-decane–12-HOA at η = 0.015. For comparison, we also studied the lamellar L_α phase at γ_a = 0.76 under the same conditions. The results of the frequency sweeps are shown in Figure 4 for the lamellar phase L_α and in Figure 5 for the hexagonal phase H₁, respectively.

For all systems, except for the pure hexagonal phase H₁ (Figure 5, left) the storage modulus G’ is higher than the loss modulus G’’ over the whole frequency range investigated, i.e., the binary gel n-decane–12-HOA, the pure lamellar phase L_α, as well as both the lamellar phase L_α and the hexagonal phase H₁ in presence of 12-HOA at η = 0.015 show solid like behavior. In case of the pure hexagonal phase H₁ a crossover point between G’ and G’’ is observed at frequencies of ω ≈ 0.1 s⁻¹, which indicates a change from solid like, elastic behavior (G’ > G’’) to liquid like, viscous behavior (G’ < G’’) [35]. Moreover, G’ of the pure hexagonal phase H₁ changes with the frequency. Characteristic for gels and soft solids is the frequency independence of G’ as can be seen in the frequency sweep of the binary gel n-decane–12-HOA [36,37,38,39]. The frequency sweeps of the pure lamellar phase L_α, as well as of the lamellar phase L_α and the hexagonal phase H₁ in presence of 12-HOA show a slight frequency dependence of G’. Comparing the absolute values of G’ and G’’, one sees that the pure hexagonal phase H₁ is much more viscous than the pure lamellar phase L_α. This results from the densely packed cylinders compared to the parallel orientated lamellar bilayers, which can easily slip against each other [40]. Moreover, the absolute G’ and G’’ values of the pure lamellar phase L_α are lower than those of the binary gel and do not change in the presence of 12-HOA. This shows that no gel was formed in case of the lamellar phase L_α. In the frequency sweep of the hexagonal phase H₁ in presence of 12-HOA no crossover between G’ and G’’ can be seen anymore, since the slope of G’ is lower, i.e., the solid like behavior predominates in the whole frequency range and indicates that a gel was indeed formed. However, as the storage modulus G’ is still frequency dependent, a gel weaker (but stiffer) than the binary gel was formed. Note that the absolute values of G’ and G’’ cannot be used as measure for gel formation as they are a priori high for the pure hexagonal phase H₁. In conclusion, one can say that for the lowest 12-HOA mass fraction of η = 0.015, 12-HOA is only co-surfactant in case of the lamellar L_α phase, but acts as both co-surfactant and gelator in case of the hexagonal phase. Again, it becomes obvious that 12-HOA is more likely incorporated in the surfactant layer of the system H₂O–C₁₂E₇ at higher surfactant mass fractions than at low ones—since at higher surfactant mass fractions most of the 12-HOA molecules are dissolved in the surfactant layer, no gel can be formed.

3. Conclusions

Both visual observation and ²H NMR clearly show that 12-HOA stabilizes the lamellar phase and destabilizes the hexagonal phase of the binary system H₂O–C₁₂E₇ (heptaethylene glycol monododecyl ether). The strong influence of 12-HOA on the phase behavior is clear evidence that the organogelator acts as a co-surfactant. With increasing mass fraction η of 12-HOA the effect is more pronounced (at the highest gelator mass fraction investigated, namely η = 0.05, the hexagonal phase is completely absent), but the gelation capacity is increased, too. At η = 0.05, a sol-gel transition can be observed by visual inspection. The sol-gel transition temperature decreases with increasing surfactant concentration, which proves a decreasing gelation capacity, due to an increasing incorporation of 12-HOA in the surfactant layer. Note that the total gelator mass fraction is kept constant, while the amount of surfactant thus the surfactant layer increases.

The ²H NMR measurements show broadened features of the Pake powder pattern of the hexagonal phase, which indicates an increased viscosity, even at the lowest gelator mass fraction of η = 0.015. Dynamic rheometry proves that a weak gel is formed at this low gelator concentration in the case of the hexagonal phase (i.e., at low surfactant concentrations), whereas no gel is formed in the case of the lamellar phase (i.e., at high surfactant concentrations). This is in excellent agreement with the decreasing gelation capacity deduced from decrease of the sol-gel transition temperature as a function of surfactant concentration.

Our investigations clearly show that 12-HOA is not a suitable gelator for binary systems consisting of H₂O and an alkyl polyglycol ether (C_iE_j), since its gelation capacity is hampered by its competing role as co-surfactant. The search for a suitable gelator for H₂O–C_iE_j systems that solely acts as gelator is continued; tests of other organogelators, as well as hydrogelators, are in progress. Once a suitable gelator is found, we will investigate (a) if the lyotropic liquid crystalline phases serve as template for the gelator network leading to well aligned gelator fibers and (b) if the gel network influences the structure of the lyotropic liquid crystalline phases. For this purpose, the sol-gel transition line has to be adjusted via the gelator concentration, such that (a) the sol-gel transition temperature T_sol-gel of the gelator is lower than the melting point of the lyotropic liquid crystalline phases, and (b) the sol-gel transition temperature T_sol-gel of the gelator is higher than the melting point of the lyotropic liquid crystalline phases. First experimental evidence that the chronology—that is, whether gelation or liquid crystal phase formation occurs first—may indeed play a role is the work published by Kato et al. They studied gelled thermotropic liquid crystals (thermotropic LC), focusing on the influence of the chronology on the resulting structure [41].

4. Materials and Methods

4.1. Materials and Sample Preparation

We purchased heptaethylene glycol monododecyl ether (C₁₂E₇) from Sigma Aldrich (≥98%) (St. Louis, MO, USA), Nikkol (Tokyo, Japan) and TCI (Tokyo, Japan), 12-hydroxyoctadecanoic acid (12-HOA) from Alfa Aesar (95%) (Karlsruhe, Germany), and D₂O from euriso-top (99.9 atom % D). Note that Alfa Aesar does not specify whether 12-HOA is a racemate or a pure enantiomer. We thus measured the optical rotation angle of 12-HOA in methanol and found out that the substance is the (R)-enantiomer. In a previous study we proved that 12-HOA provided by Sigma Aldrich is also (R)-12-HOA. [42] All chemicals were used without further purification.

The composition of the systems is defined by the surfactant mass fraction,

$${\mathsf{\gamma }}_{\mathrm{a}}= \frac{m_{{\mathrm{C}}_{12}{\mathrm{E}}_7}}{m_{{\mathrm{C}}_{12}{\mathrm{E}}_7}{+ m}_{{\mathrm{H}}_2\mathrm{O}}},$$

(1)

and the 12-HOA mass fraction,

$$\mathsf{\eta } = \frac{m_{12\text{-}\mathrm{HOA}}}{m_{{\mathrm{C}}_{12}{\mathrm{E}}_7}{+ m}_{{\mathrm{H}}_2\mathrm{O}}{+ m}_{12\text{-}\mathrm{HOA}} }.$$

(2)

We used η = 0.015, 0.025, 0.05 as 12-HOA mass fractions. The samples were weighed in glass tubes, equipped with a stirring bar and sealed with plugs. The samples had to be heated in a water bath up to T = 85 °C as 12-HOA has to be molten and they were then stirred at this temperature for at least 5 min in order to ensure homogenous mixing. Subsequently, the samples were put into an ice bath for gelation for 5 min. Gelation was indicated by a change of turbidity in the case of the otherwise clear hexagonal phase and a change in viscosity in the case of the lamellar phase L_α. It is important to note that changes in turbidity and viscosity are no proofs of gelation per se. However, in the present case they can be used to identify gelation, since there are no other (phase) transitions, which could explain these changes. The samples measured by ²H NMR were prepared with D₂O instead of H₂O, but the surfactant mass fraction, as well as the 12-HOA mass fraction were calculated in the same way.

4.2. Visual Phase Studies

The phase boundaries of the binary system H₂O–C₁₂E₇ and of the systems H₂O–C₁₂E_7–12-HOA with η = 0.015, 0.025, 0.05 were determined by visual observation in water basins equipped with a thermostat (Thermo Scientific DC30, Waltham, MA, USA). The phase boundaries of the anisotropic lyotropic LC phases to the transparent, isotropic phase were detected with the help of a lamp behind the water basin and two crossed polarizers, one behind and one in front of the sample. The lyotropic LC phases were distinguished due to anisotropy, viscosity and turbidity. The hexagonal phase H₁ is transparent and highly viscous, whereas the lamellar phase L_α is slightly turbid and less viscous. Accordingly, the turbid upper miscibility gap at higher temperature was identified. The approximate sol-gel transition temperature was determined by fading turbidity in the case of the hexagonal phase H₁ and by a change in viscosity in the case of the lamellar phase L_α.

4.3. 2H NMR

²H NMR measurements were carried out with a Tecmag Apollo 300 MHz spectrometer (Erfurt, Germany) at a ²H resonance frequency of 46.02 MHz with a pulse width of 6.4 µs and a relaxation delay time of 2 s. The samples were filled in 4 cm glass tubes with a diameter of 5 mm. The tubes, equipped with a Teflon spacer and sealed with a Teflon plug and parafilm, were put in a goniometer probe such that its axis was perpendicular to the magnetic field. By applying a quadrupole echo pulse sequence, temperature-dependent ²H-NMR spectra of each sample were recorded. The sample temperature in the goniometer probe was set according to the temperature calibration of a reference probe, which was calibrated by a known phase transition of the system that was determined by visual observation before. The target temperatures were reached after a 30 min heating ramp followed by an additional 30 min equilibration time. 64 scans were averaged before Fourier transformation was applied.

4.4. Rheometry

The measurements were carried out with a Physica MCR 501 rheometer from Anton Paar (Ostfildern, Germany). A cone-plate geometry was used with an upper moving cone of 2.5 cm diameter and a cone angle of 1°. The samples were transferred to the plate with a spatula. After the upper cone was lowered to the measuring position (gap width z = 1 mm), the samples were kept at T = 22 °C for 30 min to reach equilibrium. Then, frequency (ω) sweeps were performed with a constant strain amplitude γ = 1% and constant temperature T = 22 °C of the lower plate. The frequency ω = 0.01 s⁻¹ is the lower limit of our rheometer. The temperature was set with a precision of ±0.1 K by a Peltier element. The strain amplitude γ was set such that it was in the linear viscoelastic (LVE) region determined through prior oscillating stress sweeps. The storage modulus G’ and the loss modulus G’’ were determined in the frequency sweeps. The pure hexagonal H₁ phase at a surfactant mass fraction γ_a = 0.50 and the pure lamellar L_α phase at γ_a = 0.76 as well as both lyotropic liquid crystalline phases in the presence of 12-HOA with a gelator mass fraction η = 0.015 were measured. For comparison, the rheological behaviour of the binary gel n-decane–12-HOA at η = 0.015 was investigated.