Performance of Dye Removal from Single and Binary Component Systems by Adsorption on Composite Hydrogel Beads Derived from Fruits Wastes Entrapped in Natural Polymeric Matrix
Abstract
:1. Introduction
2. Results and Discussion
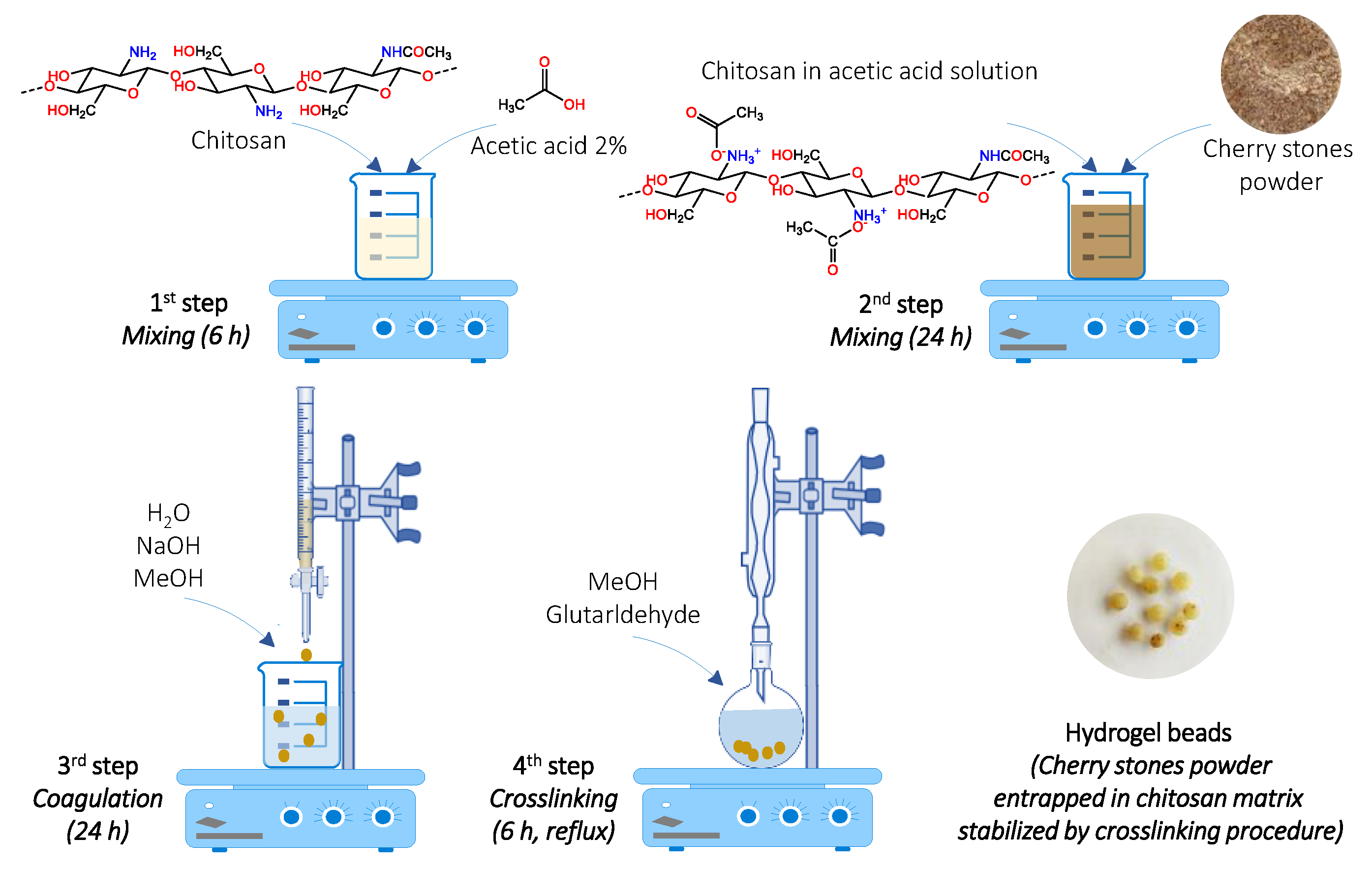
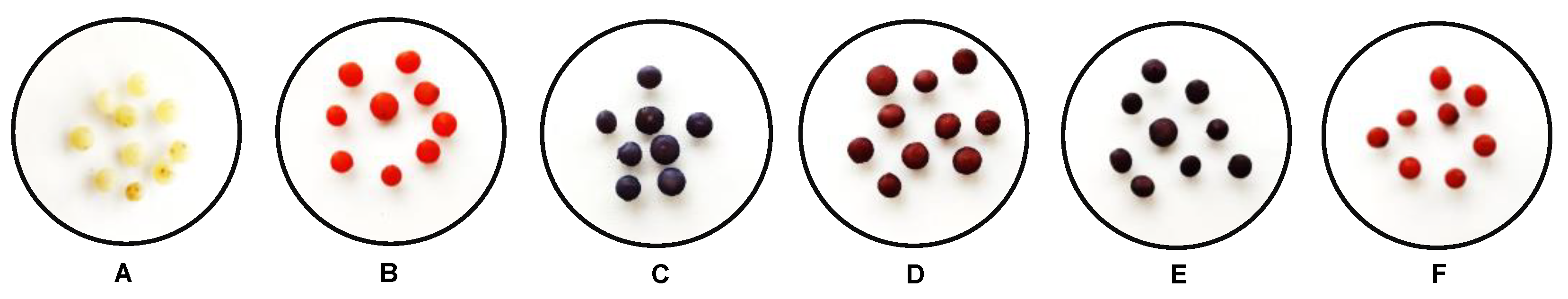
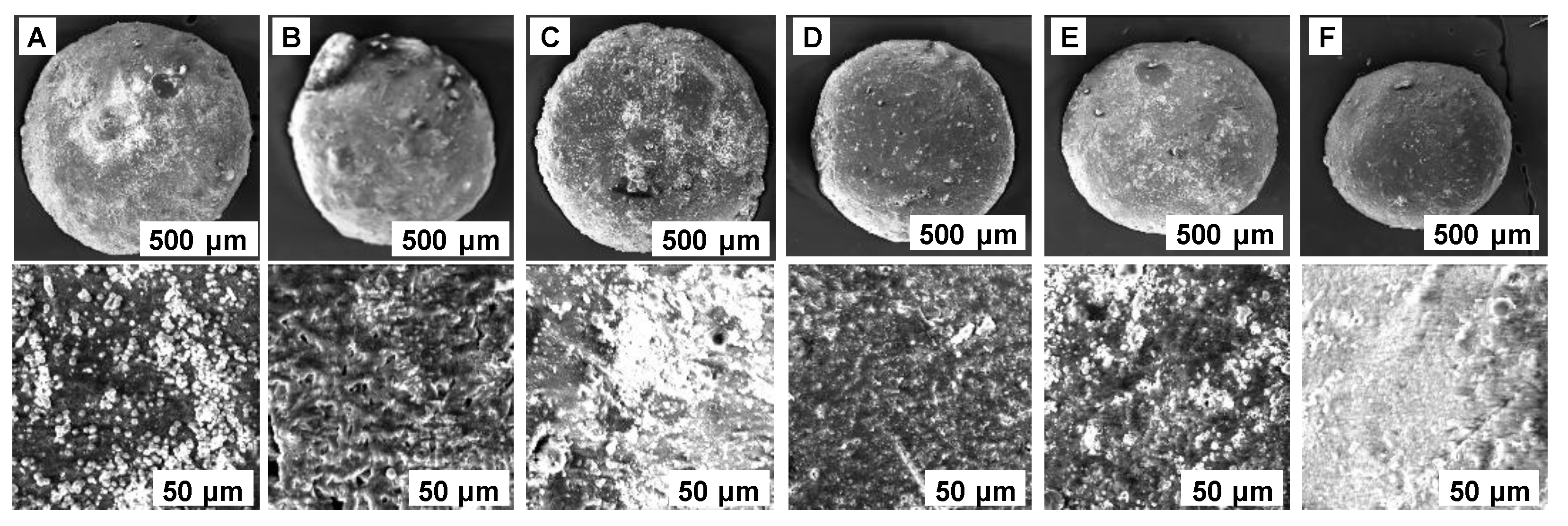
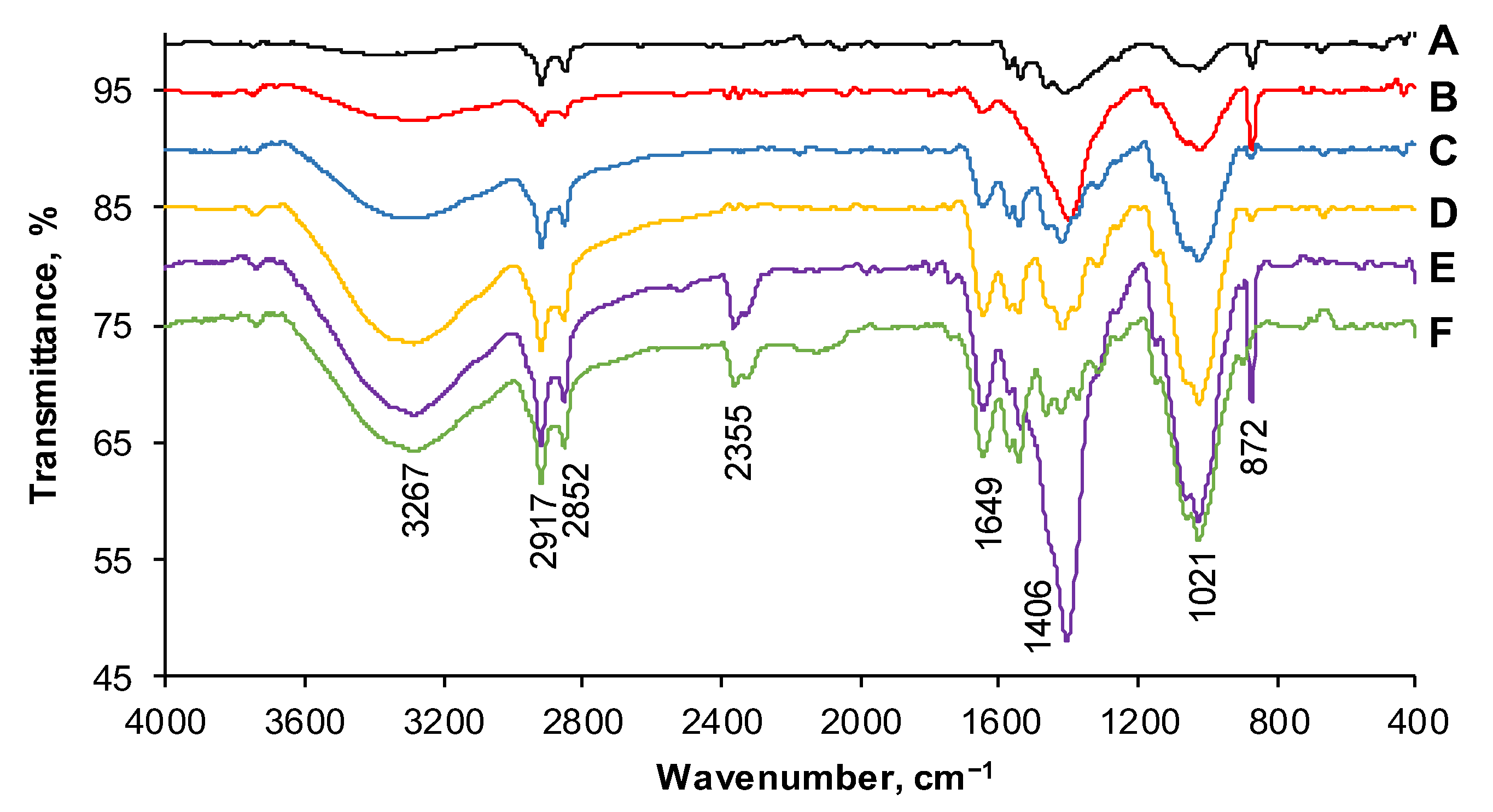
2.1. Adsorbent Synthesis and Characterization
2.2. Optimization of Adsorption Process Main Parameters by Response Surface Methodology
2.3. Adsorption Process Kinetic
2.4. Equilibrium Isotherms in Single and Binary Component Dye Systems
2.5. Adsorption Mechanism Exploration
3. Conclusions
4. Materials and Methods
4.1. Chemical Reagents
4.2. Analytical Procedure
4.3. Adsorbent Synthesis
4.4. Adsorbent Characterization
4.5. Optimization of Adsorption Process Main Parameters
4.6. Kinetic Study and Equilibrium Isotherms
4.7. Statiscal Analysis
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Benkhaya, S.; M’Rabet, S.; El Harfi, A. Classifications, properties, recent synthesis and applications of azo dyes. Heliyon 2020, 6, e03271. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Benkhaya, S.; M’ rabet, S.; El Harfi, A. A review on classifications, recent synthesis and applications of textile dyes. Inorg. Chem. Commun. 2020, 115, 107891. [Google Scholar] [CrossRef]
- Do, K.L.; Su, M.; Zhao, F. From historical dye to bio-colourant: Processing, identification in historical textiles and potential applications of anthraquinone-based morindone. Dye. Pigment. 2022, 205, 110482. [Google Scholar] [CrossRef]
- Vázquez-Ortega, F.; Lagunes, I.; Trigos, Á. Cosmetic dyes as potential photosensitizers of singlet oxygen generation. Dye. Pigment. 2020, 176, 108248. [Google Scholar] [CrossRef]
- Lee, M.; Nam, K.T.; Kim, J.; Lim, S.E.; Yeon, S.H.; Lee, B.; Lee, J.Y.; Lim, K.-M. Evaluation of ocular irritancy of coal-tar dyes used in cosmetics employing reconstructed human cornea-like epithelium and short time exposure tests. Food Chem. Toxicol. 2017, 108, 236–243. [Google Scholar] [CrossRef]
- Guerra, E.; Alvarez-Rivera, G.; Llompart, M.; Garcia-Jares, C. Simultaneous determination of preservatives and synthetic dyes in cosmetics by single-step vortex extraction and clean-up followed by liquid chromatography coupled to tandem mass spectrometry. Talanta 2018, 188, 251–258. [Google Scholar] [CrossRef]
- Durazzo, A.; Carocho, M.; Heleno, S.; Barros, L.; Souto, E.B.; Santini, A.; Lucarini, M. Food dyes and health: Literature quantitative research analysis. Meas. Food 2022, 7, 100050. [Google Scholar] [CrossRef]
- Lehmkuhler, A.; Miller, M.D.; Bradman, A.; Castorina, R.; Chen, M.-A.; Xie, T.; Mitchell, A.E. Levels of FD&C certified food dyes in foods commonly consumed by children. J. Food Compos. Anal. 2022, 112, 104649. [Google Scholar] [CrossRef]
- Jang, G.-W.; Choi, S.-I.; Choi, S.-H.; Han, X.; Men, X.; Kwon, H.-Y.; Choi, Y.-E.; Lee, O.-H. Method validation of 12 kinds of food dye in chewing gums and soft drinks, and evaluation of measurement uncertainty for soft drinks. Food Chem. 2021, 356, 129705. [Google Scholar] [CrossRef]
- Velho, S.R.K.; Brum, L.F.W.; Petter, C.O.; dos Santos, J.H.Z.; Šimunić, Š.; Kappa, W.H. Development of structured natural dyes for use into plastics. Dye. Pigment. 2017, 136, 248–254. [Google Scholar] [CrossRef]
- Choi, P.J.; Cooper, E.A.; Park, T.I.H.; Denny, W.A.; Jose, J. Novel synthetic approach for accessing drug–dye conjugates for targeted tumour therapy. Results Chem. 2022, 4, 100343. [Google Scholar] [CrossRef]
- Choi, P.J.; Cooper, E.; Schweder, P.; Mee, E.; Faull, R.; Denny, W.A.; Dragunow, M.; Park, T.I.H.; Jose, J. The synthesis of a novel Crizotinib heptamethine cyanine dye conjugate that potentiates the cytostatic and cytotoxic effects of Crizotinib in patient-derived glioblastoma cell lines. Bioorganic Med. Chem. Lett. 2019, 29, 2617–2621. [Google Scholar] [CrossRef] [PubMed]
- Kim, K.-H.; Kabir, E.; Jahan, S.A. The use of personal hair dye and its implications for human health. Environ. Int. 2016, 89–90, 222–227. [Google Scholar] [CrossRef]
- Al-Tohamy, R.; Ali, S.S.; Li, F.; Okasha, K.M.; Mahmoud, Y.A.G.; Elsamahy, T.; Jiao, H.; Fu, Y.; Sun, J. A critical review on the treatment of dye-containing wastewater: Ecotoxicological and health concerns of textile dyes and possible remediation approaches for environmental safety. Ecotoxicol. Environ. Saf. 2022, 231, 113160. [Google Scholar] [CrossRef]
- Oladoye, P.O.; Ajiboye, T.O.; Omotola, E.O.; Oyewola, O.J. Methylene blue dye: Toxicity and potential elimination technology from wastewater. Results Eng. 2022, 16, 100678. [Google Scholar] [CrossRef]
- Hadi, P.; Sharma, S.K.; McKay, G. Removal of Dyes from Effluents Using Biowaste-Derived Adsorbents. In Green Chemistry for Dyes Removal from Wastewater; Wiley: Hoboken, NJ, USA, 2015; pp. 139–201. [Google Scholar]
- Singh, A.; Pal, D.B.; Mohammad, A.; Alhazmi, A.; Haque, S.; Yoon, T.; Srivastava, N.; Gupta, V.K. Biological remediation technologies for dyes and heavy metals in wastewater treatment: New insight. Bioresour. Technol. 2022, 343, 126154. [Google Scholar] [CrossRef]
- Dadban Shahamat, Y.; Masihpour, M.; Borghei, P.; Hoda Rahmati, S. Removal of azo red-60 dye by advanced oxidation process O3/UV from textile wastewaters using Box-Behnken design. Inorg. Chem. Commun. 2022, 143, 109785. [Google Scholar] [CrossRef]
- Bessegato, G.G.; de Souza, J.C.; Cardoso, J.C.; Zanoni, M.V.B. Assessment of several advanced oxidation processes applied in the treatment of environmental concern constituents from a real hair dye wastewater. J. Environ. Chem. Eng. 2018, 6, 2794–2802. [Google Scholar] [CrossRef] [Green Version]
- Hassaan, M.A.; El Nemr, A.; Madkour, F.F. Testing the advanced oxidation processes on the degradation of Direct Blue 86 dye in wastewater. Egypt. J. Aquat. Res. 2017, 43, 11–19. [Google Scholar] [CrossRef] [Green Version]
- Wang, J.; Yuan, R.; Feng, Z.; Ma, F.; Zhou, B.; Chen, H. The advanced treatment of textile printing and dyeing wastewater by hydrodynamic cavitation and ozone: Degradation, mechanism, and transformation of dissolved organic matter. Environ. Res. 2022, 215, 114300. [Google Scholar] [CrossRef]
- Turhan, K.; Turgut, Z. Decolorization of direct dye in textile wastewater by ozonization in a semi-batch bubble column reactor. Desalination 2009, 242, 256–263. [Google Scholar] [CrossRef]
- Koulini, G.V.; Laiju, A.R.; Ramesh, S.T.; Gandhimathi, R.; Nidheesh, P.V. Effective degradation of azo dye from textile wastewater by electro-peroxone process. Chemosphere 2022, 289, 133152. [Google Scholar] [CrossRef]
- Kothai, A.; Sathishkumar, C.; Muthupriya, R.; Sankar, K.S.; Dharchana, R. Experimental investigation of textile dyeing wastewater treatment using aluminium in electro coagulation process and Fenton’s reagent in advanced oxidation process. Mater. Today Proc. 2021, 45, 1411–1416. [Google Scholar] [CrossRef]
- Chairungsri, W.; Subkomkaew, A.; Kijjanapanich, P.; Chimupala, Y. Direct dye wastewater photocatalysis using immobilized titanium dioxide on fixed substrate. Chemosphere 2022, 286, 131762. [Google Scholar] [CrossRef]
- Dihom, H.R.; Al-Shaibani, M.M.; Radin Mohamed, R.M.S.; Al-Gheethi, A.A.; Sharma, A.; Khamidun, M.H.B. Photocatalytic degradation of disperse azo dyes in textile wastewater using green zinc oxide nanoparticles synthesized in plant extract: A critical review. J. Water Process Eng. 2022, 47, 102705. [Google Scholar] [CrossRef]
- Thambiliyagodage, C. Efficient photocatalysis of carbon coupled TiO2 to degrade pollutants in wastewater—A review. Environ. Nanotechnol. Monit. Manag. 2022, 18, 100737. [Google Scholar] [CrossRef]
- Nnaji, P.C.; Anadebe, V.C.; Ezemagu, I.G.; Onukwuli, O.D. Potential of Luffa cylindrica seed as coagulation-flocculation (CF) agent for the treatment of dye wastewater: Kinetic, mass transfer, optimization and CF adsorption studies. Arab. J. Chem. 2022, 15, 103629. [Google Scholar] [CrossRef]
- Liang, C.-Z.; Sun, S.-P.; Li, F.-Y.; Ong, Y.-K.; Chung, T.-S. Treatment of highly concentrated wastewater containing multiple synthetic dyes by a combined process of coagulation/flocculation and nanofiltration. J. Membr. Sci. 2014, 469, 306–315. [Google Scholar] [CrossRef]
- Xiang, J.; Wang, X.; Ding, M.; Tang, X.; Zhang, S.; Zhang, X.; Xie, Z. The role of lateral size of MXene nanosheets in membrane filtration of dyeing wastewater: Membrane characteristic and performance. Chemosphere 2022, 294, 133728. [Google Scholar] [CrossRef] [PubMed]
- Xiang, J.; Li, H.; Hei, Y.; Tian, G.; Zhang, L.; Cheng, P.; Zhang, J.; Tang, N. Preparation of highly permeable electropositive nanofiltration membranes using quaternized polyethyleneimine for dye wastewater treatment. J. Water Process Eng. 2022, 48, 102831. [Google Scholar] [CrossRef]
- Hao, J.; Ji, L.; Li, C.; Hu, C.; Wu, K. Rapid, efficient and economic removal of organic dyes and heavy metals from wastewater by zinc-induced in-situ reduction and precipitation of graphene oxide. J. Taiwan Inst. Chem. Eng. 2018, 88, 137–145. [Google Scholar] [CrossRef]
- Li, Y.; An, Y.; Zhao, R.; Zhong, Y.; Long, S.; Yang, J.; Li, J.; Zheng, H. Synergetic removal of oppositely charged dyes by co-precipitation and amphoteric self-floating capturer: Mechanism investigation by molecular simulation. Chemosphere 2022, 296, 134033. [Google Scholar] [CrossRef]
- Liu, X.; Chen, Z.; Du, W.; Liu, P.; Zhang, L.; Shi, F. Treatment of wastewater containing methyl orange dye by fluidized three dimensional electrochemical oxidation process integrated with chemical oxidation and adsorption. J. Environ. Manag. 2022, 311, 114775. [Google Scholar] [CrossRef]
- Jing, X.; Yuan, J.; Cai, D.; Li, B.; Hu, D.; Li, J. Concentrating and recycling of high-concentration printing and dyeing wastewater by a disc tube reverse osmosis-Fenton oxidation/low temperature crystallization process. Sep. Purif. Technol. 2021, 266, 118583. [Google Scholar] [CrossRef]
- Khan, S.A.; Abbasi, N.; Hussain, D.; Khan, T.A. Sustainable Mitigation of Paracetamol with a Novel Dual-Functionalized Pullulan/Kaolin Hydrogel Nanocomposite from Simulated Wastewater. Langmuir 2022, 38, 8280–8295. [Google Scholar] [CrossRef] [PubMed]
- Bichave, M.S.; Kature, A.Y.; Koranne, S.V.; Shinde, R.S.; Gongle, A.S.; Choudhari, V.P.; Topare, N.S.; Raut-Jadhav, S.; Bokil, S.A. Nano-metal oxides-activated carbons for dyes removal: A review. Mater. Today Proc. 2022, in press. [Google Scholar] [CrossRef]
- Goswami, R.; Kumar Dey, A. Synthesis and application of treated activated carbon for cationic dye removal from modelled aqueous solution. Arab. J. Chem. 2022, 15, 104290. [Google Scholar] [CrossRef]
- Sultana, M.; Rownok, M.H.; Sabrin, M.; Rahaman, M.H.; Alam, S.M.N. A review on experimental chemically modified activated carbon to enhance dye and heavy metals adsorption. Clean. Eng. Technol. 2022, 6, 100382. [Google Scholar] [CrossRef]
- Wang, W.; Zhao, W.; Zhang, H.; Xu, J.; Zong, L.; Kang, Y.; Wang, A. Mesoporous polymetallic silicate derived from naturally abundant mixed clay: A potential robust adsorbent for removal of cationic dye and antibiotic. Powder Technol. 2021, 390, 303–314. [Google Scholar] [CrossRef]
- Tochetto, G.A.; Simão, L.; de Oliveira, D.; Hotza, D.; Immich, A.P.S. Porous geopolymers as dye adsorbents: Review and perspectives. J. Clean. Prod. 2022, 374, 133982. [Google Scholar] [CrossRef]
- Bilal, M.; Ihsanullah, I.; Hassan Shah, M.U.; Bhaskar Reddy, A.V.; Aminabhavi, T.M. Recent advances in the removal of dyes from wastewater using low-cost adsorbents. J. Environ. Manag. 2022, 321, 115981. [Google Scholar] [CrossRef]
- Ali, N.S.; Jabbar, N.M.; Alardhi, S.M.; Majdi, H.S.; Albayati, T.M. Adsorption of methyl violet dye onto a prepared bio-adsorbent from date seeds: Isotherm, kinetics, and thermodynamic studies. Heliyon 2022, 8, e10276. [Google Scholar] [CrossRef] [PubMed]
- Amalina, F.; Abd Razak, A.S.; Krishnan, S.; Zularisam, A.W.; Nasrullah, M. A review of eco-sustainable techniques for the removal of Rhodamine B dye utilizing biomass residue adsorbents. Phys. Chem. Earth Parts A/B/C 2022, 128, 103267. [Google Scholar] [CrossRef]
- Moradi, O.; Panahandeh, S. Fabrication of different adsorbents based on zirconium oxide, graphene oxide, and dextrin for removal of green malachite dye from aqueous solutions. Environ. Res. 2022, 214, 114042. [Google Scholar] [CrossRef]
- Hassanien, R.; Hassan, Z.A.; Al-Assy, W.; Ibrahim, S.M. Removal of toxic thymol sulfone phthalein dye from wastewater by using efficient adsorbent NiO nanoparticles. J. Mol. Struct. 2022, 1269, 133864. [Google Scholar] [CrossRef]
- Zeng, S.; Long, J.; Sun, J.; Wang, G.; Zhou, L. A review on peach gum polysaccharide: Hydrolysis, structure, properties and applications. Carbohydr. Polym. 2022, 279, 119015. [Google Scholar] [CrossRef]
- Tabasum, A.; Razzaq, H.; Razzaque, S.; Bibi, A.; Farooq, S.; Yaqub, A.; Siddique, A.; Amir, T.; Rehman, S.-u. Protonated polyaniline and its derivatives as potential adsorbents for simultaneous reclamation of textile dyes and oil/water separation. Mater. Chem. Phys. 2023, 293, 126913. [Google Scholar] [CrossRef]
- Mohammad-Rezaei, R.; Khalilzadeh, B.; Rahimi, F.; Moradi, S.; Shahlaei, M.; Derakhshankhah, H.; Jaymand, M. Simultaneous removal of cationic and anionic dyes from simulated industrial effluents using a nature-inspired adsorbent. Environ. Res. 2022, 214, 113966. [Google Scholar] [CrossRef]
- Angin, D. Production and characterization of activated carbon from sour cherry stones by zinc chloride. Fuel 2014, 115, 804–811. [Google Scholar] [CrossRef]
- Olivares-Marín, M.; Fernández-González, C.; Macías-García, A.; Gómez-Serrano, V. Preparation of activated carbons from cherry stones by activation with potassium hydroxide. Appl. Surf. Sci. 2006, 252, 5980–5983. [Google Scholar] [CrossRef]
- Olivares-Marín, M.; Fernández-González, C.; Macías-García, A.; Gómez-Serrano, V. Preparation of activated carbon from cherry stones by physical activation in air. Influence of the chemical carbonisation with H2SO4. J. Anal. Appl. Pyrolysis 2012, 94, 131–137. [Google Scholar] [CrossRef]
- Durán-Valle, C.J.; Gómez-Corzo, M.; Gómez-Serrano, V.; Pastor-Villegas, J.; Rojas-Cervantes, M.L. Preparation of charcoal from cherry stones. Appl. Surf. Sci. 2006, 252, 5957–5960. [Google Scholar] [CrossRef]
- Nowicki, P.; Kazmierczak, J.; Pietrzak, R. Comparison of physicochemical and sorption properties of activated carbons prepared by physical and chemical activation of cherry stones. Powder Technol. 2015, 269, 312–319. [Google Scholar] [CrossRef]
- Grigoras, C.; Simion, A.-I.; Favier, L.; Gavrilă, L. Congo Red Removal from Aqueous Effluents by Adsorption on Cherry Stones Activated Carbon. Environ. Eng. Manag. J. 2020, 19, 247–254. [Google Scholar]
- Al-Rooqi, M.M.; Hassan, M.M.; Moussa, Z.; Obaid, R.J.; Suman, N.H.; Wagner, M.H.; Natto, S.S.A.; Ahmed, S.A. Advancement of chitin and chitosan as promising biomaterials. J. Saudi Chem. Soc. 2022, 26, 101561. [Google Scholar] [CrossRef]
- Nasir, A.; Inaam-ul-Hassan, M.; Raza, A.; Tahir, M.; Yasin, T. Removal of copper using chitosan beads embedded with amidoxime grafted graphene oxide nanohybids. Int. J. Biol. Macromol. 2022, 222, 750–758. [Google Scholar] [CrossRef]
- Altun, T. Chitosan-coated sour cherry kernel shell beads: An adsorbent for removal of Cr(VI) from acidic solutions. J. Anal. Sci. Technol. 2019, 10, 14. [Google Scholar] [CrossRef]
- Rosli, M.I.; Abdullah, M.; Krishnan, G.; Harun, S.W.; Aziz, M.S. Power-dependent nonlinear optical behaviours of ponceau BS chromophore at 532 nm via Z-scan technique. J. Photochem. Photobiol. A Chem. 2020, 397, 112574. [Google Scholar] [CrossRef]
- Vinayak, A.; Singh, G.B. Biodecolorization of reactive black 5 using magnetite nanoparticles coated Bacillus sp. RA5. Mater. Today: Proc. 2022, 48, 1523–1526. [Google Scholar] [CrossRef]
- Joksimović, K.; Kodranov, I.; Randjelović, D.; Slavković Beškoski, L.; Radulović, J.; Lješević, M.; Manojlović, D.; Beškoski, V.P. Microbial fuel cells as an electrical energy source for degradation followed by decolorization of Reactive Black 5 azo dye. Bioelectrochemistry 2022, 145, 108088. [Google Scholar] [CrossRef]
- Khan, M.; Sarwar, A. Determination of points of zero charge of natural and treated adsorbents. Surf. Rev. Lett. 2007, 14, 461–469. [Google Scholar] [CrossRef]
- Bakatula, E.N.; Richard, D.; Neculita, C.M.; Zagury, G.J. Determination of point of zero charge of natural organic materials. Environ. Sci. Pollut. Res. 2018, 25, 7823–7833. [Google Scholar] [CrossRef]
- Olivares-Marín, M.; Del Prete, V.; Garcia-Moruno, E.; Fernández-González, C.; Macías-García, A.; Gómez-Serrano, V. The development of an activated carbon from cherry stones and its use in the removal of ochratoxin A from red wine. Food Control 2009, 20, 298–303. [Google Scholar] [CrossRef]
- Bushra, R.; Mohamad, S.; Alias, Y.; Jin, Y.; Ahmad, M. Current approaches and methodologies to explore the perceptive adsorption mechanism of dyes on low-cost agricultural waste: A review. Microporous Mesoporous Mater. 2021, 319, 111040. [Google Scholar] [CrossRef]
- Karimifard, S.; Alavi Moghaddam, M.R. Application of response surface methodology in physicochemical removal of dyes from wastewater: A critical review. Sci. Total Environ. 2018, 640–641, 772–797. [Google Scholar] [CrossRef] [PubMed]
- Ye, X.; Wu, L.; Zhu, M.; Wang, Z.; Huang, Z.-H.; Wang, M.-X. Lotus pollen-derived hierarchically porous carbons with exceptional adsorption performance toward Reactive Black 5: Isotherms, kinetics and thermodynamics investigations. Sep. Purif. Technol. 2022, 300, 121899. [Google Scholar] [CrossRef]
- Priya; Sharma, A.K.; Kaith, B.S.; Vipula; Chandel, K.; Singh, A.; Isha. Chemically modified chitosan-sodium alginate as chemo-sensor adsorbent for the detection of picric acid and removal of biebrich scarlet. Int. J. Biol. Macromol. 2020, 147, 582–594. [Google Scholar] [CrossRef]
- Priya; Sharma, A.K.; Kaith, B.S.; Tanwar, V.; Bhatia, J.K.; Sharma, N.; Bajaj, S.; Panchal, S. RSM-CCD optimized sodium alginate/gelatin based ZnS-nanocomposite hydrogel for the effective removal of biebrich scarlet and crystal violet dyes. Int. J. Biol. Macromol. 2019, 129, 214–226. [Google Scholar] [CrossRef]
- Yadav, B.S.; Dasgupta, S. Effect of time, pH, and temperature on kinetics for adsorption of methyl orange dye into the modified nitrate intercalated MgAl LDH adsorbent. Inorg. Chem. Commun. 2022, 137, 109203. [Google Scholar] [CrossRef]
- de Farias Silva, C.E.; da Gama, B.M.V.; da Silva Gonçalves, A.H.; Medeiros, J.A.; de Souza Abud, A.K. Basic-dye adsorption in albedo residue: Effect of pH, contact time, temperature, dye concentration, biomass dosage, rotation and ionic strength. J. King Saud Univ.-Eng. Sci. 2020, 32, 351–359. [Google Scholar] [CrossRef]
- Plazinski, W.; Rudzinski, W.; Plazinska, A. Theoretical models of sorption kinetics including a surface reaction mechanism: A review. Adv. Colloid Interface Sci. 2009, 152, 2–13. [Google Scholar] [CrossRef]
- Tran, H.N.; You, S.-J.; Chao, H.-P. Thermodynamic parameters of cadmium adsorption onto orange peel calculated from various methods: A comparison study. J. Environ. Chem. Eng. 2016, 4, 2671–2682. [Google Scholar] [CrossRef]
- Azizian, S. Kinetic models of sorption: A theoretical analysis. J. Colloid Interface Sci. 2004, 276, 47–52. [Google Scholar] [CrossRef] [PubMed]
- dos Santos Lins, P.V.; Henrique, D.C.; Ide, A.H.; de Paiva e Silva Zanta, C.L.; Meili, L. Evaluation of caffeine adsorption by MgAl-LDH/biochar composite. Environ. Sci. Pollut. Res. 2019, 26, 31804–31811. [Google Scholar] [CrossRef] [PubMed]
- Wu, F.-C.; Tseng, R.-L.; Juang, R.-S. Characteristics of Elovich equation used for the analysis of adsorption kinetics in dye-chitosan systems. Chem. Eng. J. 2009, 150, 366–373. [Google Scholar] [CrossRef]
- George, R.; Sugunan, S. Kinetics of adsorption of lipase onto different mesoporous materials: Evaluation of Avrami model and leaching studies. J. Mol. Catal. B Enzym. 2014, 105, 26–32. [Google Scholar] [CrossRef]
- Rong, X.; Qiu, F.; Qin, J.; Zhao, H.; Yan, J.; Yang, D. A facile hydrothermal synthesis, adsorption kinetics and isotherms to Congo Red azo-dye from aqueous solution of NiO/graphene nanosheets adsorbent. J. Ind. Eng. Chem. 2015, 26, 354–363. [Google Scholar] [CrossRef]
- Abramian, L.; El-Rassy, H. Adsorption kinetics and thermodynamics of azo-dye Orange II onto highly porous titania aerogel. Chem. Eng. J. 2009, 150, 403–410. [Google Scholar] [CrossRef]
- Felista, M.M.; Wanyonyi, W.C.; Ongera, G. Adsorption of anionic dye (Reactive black 5) using macadamia seed Husks: Kinetics and equilibrium studies. Sci. Afr. 2020, 7, e00283. [Google Scholar] [CrossRef]
- Shahinpour, A.; Tanhaei, B.; Ayati, A.; Beiki, H.; Sillanpää, M. Binary dyes adsorption onto novel designed magnetic clay-biopolymer hydrogel involves characterization and adsorption performance: Kinetic, equilibrium, thermodynamic, and adsorption mechanism. J. Mol. Liq. 2022, 366, 120303. [Google Scholar] [CrossRef]
- You, X.; Zhou, R.; Zhu, Y.; Bu, D.; Cheng, D. Adsorption of dyes methyl violet and malachite green from aqueous solution on multi-step modified rice husk powder in single and binary systems: Characterization, adsorption behavior and physical interpretations. J. Hazard. Mater. 2022, 430, 128445. [Google Scholar] [CrossRef]
- Osagie, C.; Othmani, A.; Ghosh, S.; Malloum, A.; Kashitarash Esfahani, Z.; Ahmadi, S. Dyes adsorption from aqueous media through the nanotechnology: A review. J. Mater. Res. Technol. 2021, 14, 2195–2218. [Google Scholar] [CrossRef]
- Foo, K.Y.; Hameed, B.H. Insights into the modeling of adsorption isotherm systems. Chem. Eng. J. 2010, 156, 2–10. [Google Scholar] [CrossRef]
- Hamdaoui, O.; Naffrechoux, E. Modeling of adsorption isotherms of phenol and chlorophenols onto granular activated carbon: Part I. Two-parameter models and equations allowing determination of thermodynamic parameters. J. Hazard. Mater. 2007, 147, 381–394. [Google Scholar] [CrossRef] [PubMed]
- Diaz de Tuesta, J.L.; Silva, A.M.T.; Faria, J.L.; Gomes, H.T. Adsorption of Sudan-IV contained in oily wastewater on lipophilic activated carbons: Kinetic and isotherm modelling. Environ. Sci. Pollut. Res. 2020, 27, 20770–20785. [Google Scholar] [CrossRef]
- Mozaffari Majd, M.; Kordzadeh-Kermani, V.; Ghalandari, V.; Askari, A.; Sillanpää, M. Adsorption isotherm models: A comprehensive and systematic review (2010−2020). Sci. Total Environ. 2022, 812, 151334. [Google Scholar] [CrossRef] [PubMed]
- Ali, B.; Naceur, B.; Abdelkader, E.; Karima, E.; Nourredine, B. Competitive adsorption of binary dye from aqueous solutions using calcined layered double hydroxides. Int. J. Environ. Anal. Chem. 2022, 102, 3207–3226. [Google Scholar] [CrossRef]
- Ojediran, J.O.; Dada, A.O.; Aniyi, S.O.; David, R.O.; Adewumi, A.D. Mechanism and isotherm modeling of effective adsorption of malachite green as endocrine disruptive dye using Acid Functionalized Maize Cob (AFMC). Sci. Rep. 2021, 11, 21498. [Google Scholar] [CrossRef] [PubMed]
- Archin, S.; Sharifi, S.H.; Asadpour, G. Optimization and modeling of simultaneous ultrasound-assisted adsorption of binary dyes using activated carbon from tobacco residues: Response surface methodology. J. Clean. Prod. 2019, 239, 118136. [Google Scholar] [CrossRef]
- Balistrieri, L.; Murray, J. The Surface Chemistry of Goethite (a-FeOOH) in Major Ion Seawater. Am. J. Sci. 1981, 281, 788–806. [Google Scholar] [CrossRef]
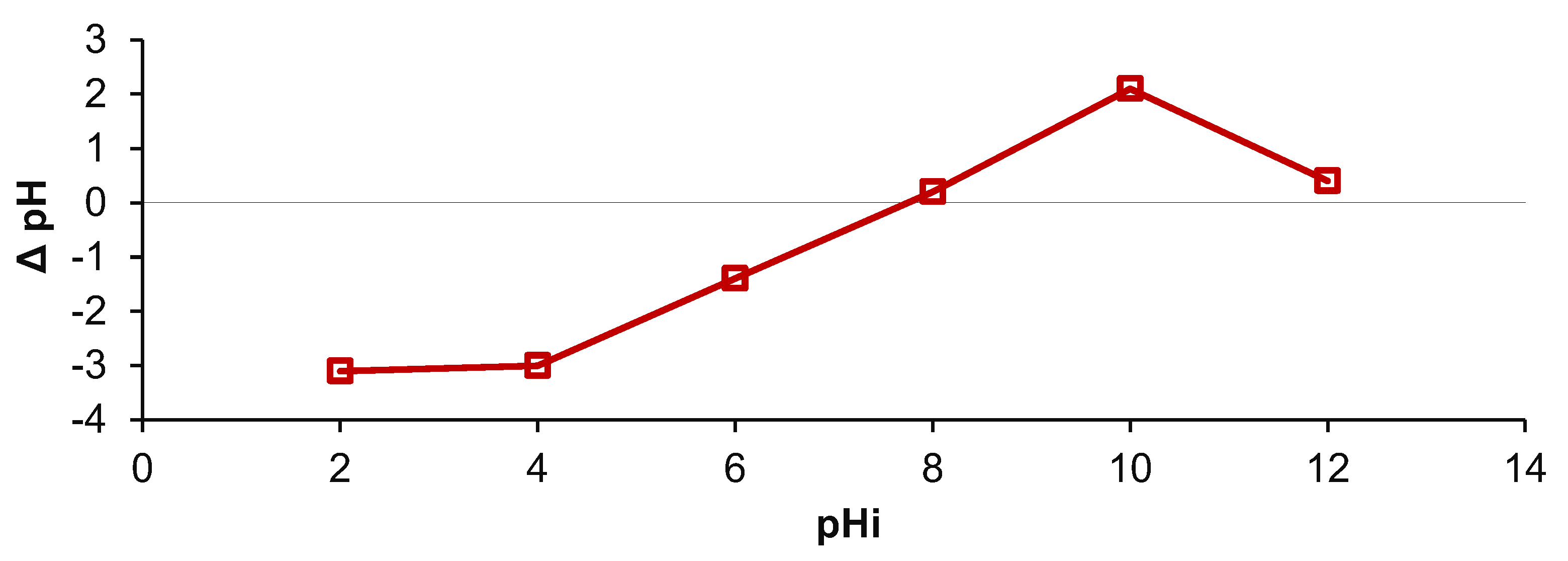
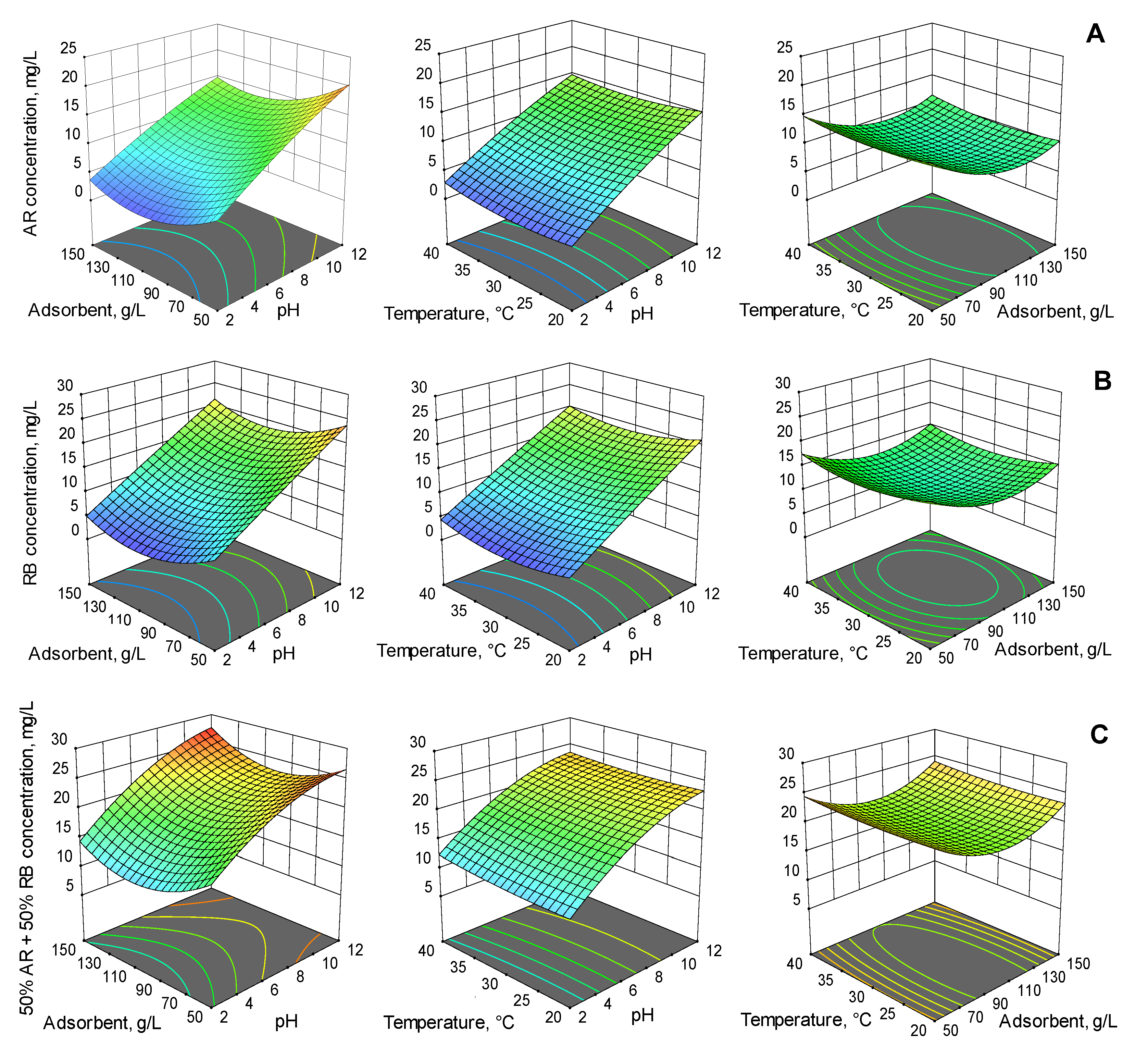

| Run | Variables | Final Contaminant Concentration, mg/L | |||||||
|---|---|---|---|---|---|---|---|---|---|
| X1 | X2 | X3 | AR | RB | 50% AR + 50% RB | ||||
| Observed | Predicted | Observed | Predicted | Observed | Predicted | ||||
| 1 | 2 | 50 | 20 | 8.07 | 7.48 | 9.79 | 9.84 | 18.12 | 16.37 |
| 2 | 2 | 50 | 30 | 7.16 | 6.54 | 7.71 | 7.69 | 17.29 | 16.25 |
| 3 | 2 | 50 | 40 | 8.07 | 7.53 | 9.67 | 9.57 | 18.81 | 17.48 |
| 4 | 2 | 100 | 20 | 2.08 | 2.19 | 4.63 | 4.45 | 9.65 | 11.06 |
| 5 | 2 | 100 | 30 | 1.14 | 1.58 | 2.40 | 2.39 | 7.07 | 10.98 |
| 6 | 2 | 100 | 40 | 1.84 | 2.90 | 4.55 | 4.37 | 11.23 | 12.26 |
| 7 | 2 | 150 | 20 | 3.75 | 3.97 | 6.73 | 6.94 | 14.22 | 14.31 |
| 8 | 2 | 150 | 30 | 3.47 | 3.68 | 4.92 | 4.98 | 16.65 | 14.28 |
| 9 | 2 | 150 | 40 | 5.60 | 5.32 | 6.87 | 7.06 | 15.56 | 15.60 |
| 10 | 7 | 50 | 20 | 14.37 | 15.39 | 18.20 | 18.23 | 22.52 | 23.76 |
| 11 | 7 | 50 | 30 | 13.65 | 14.15 | 15.92 | 15.89 | 22.12 | 23.39 |
| 12 | 7 | 50 | 40 | 14.19 | 14.85 | 17.26 | 17.58 | 22.78 | 24.37 |
| 13 | 7 | 100 | 20 | 9.08 | 9.45 | 12.82 | 12.94 | 20.54 | 19.22 |
| 14 | 7 | 100 | 30 | 8.78 | 8.54 | 10.24 | 10.69 | 19.80 | 18.89 |
| 15 | 7 | 100 | 40 | 10.32 | 9.57 | 12.62 | 12.48 | 22.12 | 19.91 |
| 16 | 7 | 150 | 20 | 11.49 | 10.57 | 15.88 | 15.53 | 23.34 | 23.23 |
| 17 | 7 | 150 | 30 | 10.22 | 9.99 | 13.73 | 13.38 | 22.97 | 22.95 |
| 18 | 7 | 150 | 40 | 11.77 | 11.34 | 15.32 | 15.27 | 23.57 | 24.02 |
| 19 | 12 | 50 | 20 | 22.43 | 21.90 | 26.59 | 26.33 | 26.97 | 27.09 |
| 20 | 12 | 50 | 30 | 20.36 | 20.38 | 23.75 | 23.80 | 26.59 | 26.47 |
| 21 | 12 | 50 | 40 | 20.73 | 20.79 | 25.32 | 25.30 | 27.18 | 27.20 |
| 22 | 12 | 100 | 20 | 15.40 | 15.32 | 21.00 | 21.14 | 23.73 | 23.31 |
| 23 | 12 | 100 | 30 | 14.57 | 14.12 | 18.57 | 18.70 | 23.70 | 22.73 |
| 24 | 12 | 100 | 40 | 15.31 | 14.86 | 20.64 | 20.30 | 24.03 | 23.50 |
| 25 | 12 | 150 | 20 | 15.40 | 15.79 | 23.60 | 23.84 | 27.35 | 28.09 |
| 26 | 12 | 150 | 30 | 14.56 | 14.92 | 21.77 | 21.50 | 27.33 | 27.56 |
| 27 | 12 | 150 | 40 | 15.31 | 15.98 | 22.86 | 23.19 | 27.44 | 28.37 |
| Source | Sum of Squares | df | Mean Square | F-Value | p-Value |
|---|---|---|---|---|---|
| AR | |||||
| Model | 890.28 | 9 | 98.92 | 260.08 | <0.0001 |
| X1—pH | 707.86 | 1 | 707.86 | 1861.08 | <0.0001 |
| X2—Adsorbent dose | 77.92 | 1 | 77.92 | 204.86 | <0.0001 |
| X3—Temperature | 0.0642 | 1 | 0.0642 | 0.1687 | 0.6857 |
| X1X2 | 5.06 | 1 | 5.06 | 13.31 | 0.0016 |
| X1X3 | 1.02 | 1 | 1.02 | 2.67 | 0.1177 |
| X2X3 | 1.28 | 1 | 1.28 | 3.38 | 0.0811 |
| X12 | 3.76 | 1 | 3.76 | 9.89 | 0.0051 |
| X22 | 83.08 | 1 | 83.08 | 218.44 | <0.0001 |
| X32 | 5.76 | 1 | 5.76 | 15.15 | 0.0009 |
| Residual | 7.61 | 20 | 0.3803 | ||
| Lack of Fit | 7.60 | 17 | 0.4472 | 254.31 | 0.0004 |
| Pure Error | 0.0053 | 3 | 0.0018 | ||
| Cor Total | 897.89 | 29 | |||
| RB | |||||
| Model | 1383.54 | 9 | 153.73 | 1877.61 | <0.0001 |
| X1—pH | 1197.72 | 1 | 1197.72 | 14,628.93 | <0.0001 |
| X2—Adsorbent dose | 28.20 | 1 | 28.20 | 344.43 | <0.0001 |
| X3—Temperature | 0.9476 | 1 | 0.9476 | 11.57 | 0.0028 |
| X1X2 | 0.1240 | 1 | 0.1240 | 1.51 | 0.2327 |
| X1X3 | 0.4447 | 1 | 0.4447 | 5.43 | 0.0304 |
| X2X3 | 0.1141 | 1 | 0.1141 | 1.39 | 0.2517 |
| X12 | 0.1900 | 1 | 0.1900 | 2.32 | 0.1433 |
| X22 | 105.38 | 1 | 105.38 | 1287.11 | <0.0001 |
| X32 | 27.34 | 1 | 27.34 | 333.96 | <0.0001 |
| Residual | 1.64 | 20 | 0.0819 | ||
| Lack of Fit | 0.9900 | 17 | 0.0582 | 0.2698 | 0.9678 |
| Pure Error | 0.6475 | 3 | 0.2158 | ||
| Cor Total | 1385.18 | 29 | |||
| 50% AR + 50% RB | |||||
| Model | 767.58 | 9 | 85.29 | 34.43 | <0.0001 |
| X1—pH | 620.92 | 1 | 620.92 | 250.64 | <0.0001 |
| X2—Adsorbent dose | 0.8736 | 1 | 0.8736 | 0.3526 | 0.5593 |
| X3—Temperature | 2.19 | 1 | 2.19 | 0.8849 | 0.3581 |
| X1X2 | 7.01 | 1 | 7.01 | 2.83 | 0.1080 |
| X1X3 | 0.7594 | 1 | 0.7594 | 0.3065 | 0.5859 |
| X2X3 | 0.0215 | 1 | 0.0215 | 0.0087 | 0.9266 |
| X12 | 35.51 | 1 | 35.51 | 14.34 | 0.0012 |
| X22 | 111.81 | 1 | 111.81 | 45.13 | <0.0001 |
| X32 | 1.28 | 1 | 1.28 | 0.5163 | 0.4807 |
| Residual | 49.55 | 20 | 2.48 | ||
| Lack of Fit | 48.87 | 17 | 2.87 | 12.65 | 0.0295 |
| Pure Error | 0.6819 | 3 | 0.2273 | ||
| Cor Total | 817.13 | 29 | |||
| Kinetic Model | Parameters | Dye System | ||
|---|---|---|---|---|
| AR | RB | 50% AR + 50% RB | ||
| Pseudo-first-order | Qe | 266.0630 | 246.2734 | 263.1561 |
| k1 | 0.0357 | 0.0241 | 0.0502 | |
| Pseudo-second-order | Qe | 291.7014 | 277.7586 | 282.5912 |
| k2 | 0.0001 | 0.0001 | 0.0002 | |
| Elovich | α | 68.9711 | 25.4416 | 46.8997 |
| β | 0.0215 | 0.0193 | 0.0008 | |
| Avrami | Qe | 266.0640 | 246.2756 | 263.1573 |
| kAv | 0.0048 | 0.0037 | 0.0097 | |
| nAv | 7.3976 | 6.5119 | 5.1388 | |
| Kinetic Model | Statistical Error | Dye System | ||
|---|---|---|---|---|
| AR | RB | 50% AR + 50% RB | ||
| Pseudo-1st-order | RMSE | 16.3835 | 24.9997 | 19.0718 |
| MPSD | 10.7211 | 18.4442 | 10.0718 | |
| HYBRID | 176.581 | 468.3276 | 216.2806 | |
| Χ2 | 31.1769 | 99.9418 | 36.2142 | |
| R2 | 0.8889 | 0.7755 | 0.7631 | |
| Pseudo-2nd-order | RMSE | 7.1010 | 16.9111 | 8.8147 |
| MPSD | 4.8352 | 12.6683 | 5.1598 | |
| HYBRID | 34.4764 | 217.7377 | 47.5154 | |
| Χ2 | 5.3919 | 39.4300 | 7.2024 | |
| R2 | 0.9791 | 0.8972 | 0.9494 | |
| Elovich | RMSE | 6.0496 | 7.8381 | 4.6171 |
| MPSD | 3.6209 | 5.7136 | 2.1060 | |
| HYBRID | 22.3955 | 45.6905 | 10.1622 | |
| Χ2 | 3.0401 | 6.8212 | 1.4145 | |
| R2 | 0.9848 | 0.9779 | 0.9861 | |
| Avrami | RMSE | 16.3834 | 24.9997 | 19.0718 |
| MPSD | 11.1263 | 19.1411 | 11.2905 | |
| HYBRID | 190.1722 | 504.3717 | 232.9243 | |
| Χ2 | 31.1797 | 99.9527 | 36.2170 | |
| R2 | 0.8888 | 0.7755 | 0.7631 | |
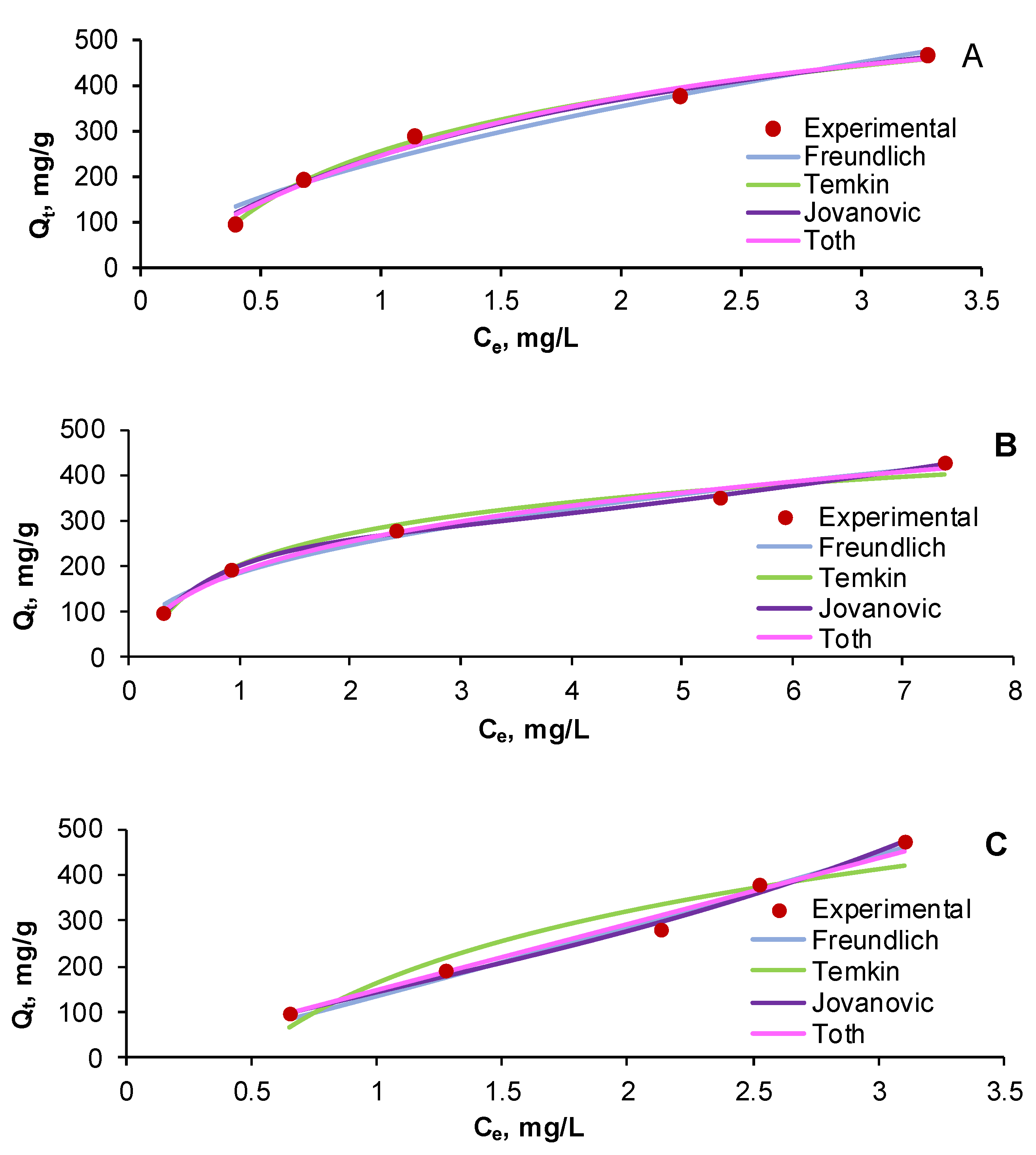
| Equilibrium Isotherm | Parameters | Dye System | ||
|---|---|---|---|---|
| AR | RB | 50% AR + 50% RB | ||
| Freundlich | KF | 234.4442 | 183.2031 | 134.9317 |
| nF | 1.6768 | 2.4246 | 0.9236 | |
| Temkin | KT | 4.5322 | 25.5301 | 11.1164 |
| bT | 14.8290 | 7.6888 | 2.0320 | |
| Jovanovic-multilayer | QJ | 356.0906 | 222.6863 | 120.8938 |
| KJ | 1.0000 | 1.7227 | 1.4182 | |
| K’J | 0.0917 | 0.0867 | 0.4418 | |
| Toth | QTo | 1157.282 | 226.8143 | 145.5048 |
| KTo | 3.0521 | 0.2571 | 3.8275 | |
| nTo | 1.1041 | 0.8255 | 0.00006 | |
| Equilibrium Isotherm | Statistical Error | Dye System | ||
|---|---|---|---|---|
| AR | RB | 50% AR + 50% RB | ||
| Freundlich | RMSE | 23.8625 | 14.8366 | 14.9614 |
| MPSD | 24.4571 | 12.0034 | 8.6964 | |
| HYBRID | 676.8724 | 198.3764 | 153.0725 | |
| Χ2 | 16.3826 | 5.5098 | 4.4373 | |
| R2 | 0.9668 | 0.9834 | 0.9872 | |
| Temkin | RMSE | 9.5809 | 16.8941 | 38.2854 |
| MPSD | 3.8981 | 7.6475 | 24.0721 | |
| HYBRID | 44.5042 | 145.4721 | 1004.89 | |
| Χ2 | 1.3125 | 4.4617 | 32.6841 | |
| R2 | 0.9946 | 0.9785 | 0.9167 | |
| Jovanovic-multilayer | RMSE | 16.6873 | 4.7557 | 11.2649 |
| MPSD | 19.0802 | 2.3253 | 6.9566 | |
| HYBRID | 418.4485 | 17.2520 | 115.684 | |
| Χ2 | 7.1594 | 0.3431 | 2.2864 | |
| R2 | 0.9856 | 0.9983 | 0.9927 | |
| Toth | RMSE | 16.0543 | 12.7540 | 16.6615 |
| MPSD | 17.4819 | 7.9484 | 8.8027 | |
| HYBRID | 379.7791 | 140.7021 | 227.6069 | |
| Χ2 | 6.7054 | 2.7508 | 4.1725 | |
| R2 | 0.9850 | 0.9877 | 0.9842 | |
| Properties | Acid Red 66 | Reactive Black 5 |
|---|---|---|
| Structure |  |  |
| Chemical name | Sodium 6-(2-hydroxynaphthylazo)-3,4′-azodibenzenesulfonate | Tetrasodium 4-amino-5-hydroxy-3,6[[4-[[2-(sulphonatooxy)ethyl]supphonyl]pehnyl]azo]naphthalene-2,7-disulphonate |
| Molecular formula | C22H14N4Na2O7S2 | C26H21N5Na4O19S6 |
| Molecular weight | 556.48 g/mol | 991.82 g/mol |
| CAS Number | 4196.99-0 | 17095-24-8 |
| EC Number | 224-084-5 | 241-164-5 |
| Maximum wavelength | 500 nm | 600 nm |
| Variable | Levels of Variation | |||
|---|---|---|---|---|
| Coded | Uncoded | Low (−1) | Central (0) | High (+1) |
| X1 | pH | 2 | 7 | 12 |
| X2 | Adsorbent dose, mg/L | 50 | 100 | 150 |
| X3 | Temperature, °C | 20 | 30 | 40 |
| Kinetic Model | Equation | Parameters Significance * |
|---|---|---|
| Pseudo-1st-order | k1—pseudo-first-order constant rate, min−1 | |
| Pseudo-2nd-order | k2—pseudo-2nd-order constant rate, g/(mg·min) | |
| Elovich | β—extent of surface coverage and activation energy for chemisorption, g/mg α—initial adsorption rate, mg/(g·min) | |
| Avrami | kAv—global rate constant, min−1 nAv—factor related to adsorption, dimensionless |
| Equilibrium Isotherm | Equation | Parameters Significance * |
|---|---|---|
| Langmuir | QL—maximum Langmuir uptake, mg/g KL—Langmuir constant, L/mg | |
| Freundlich | KF—Freundlich constant, (mg/g)(L/mg)1/n nF—Freundlich constant, dimensionless | |
| Temkin | R—gas constant, R = 8.314 J/(mol K) T—temperature, K KT—Temkin constant, L/mg bT—Temkin constant, J/mg | |
| Jovanovic-multilayer | QJ—Jovanovic maximum uptake, mg/g KJ—Jovanovic constant, L/mg K’J—Jovanovic constant, L/mg | |
| Toth | QTo—Toth maximum uptake, mg/g KTo—Toth constant, L/mg nTo—Toth constant, dimensionless |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grigoraș, C.-G.; Simion, A.-I.; Favier, L.; Drob, C.; Gavrilă, L. Performance of Dye Removal from Single and Binary Component Systems by Adsorption on Composite Hydrogel Beads Derived from Fruits Wastes Entrapped in Natural Polymeric Matrix. Gels 2022, 8, 795. https://doi.org/10.3390/gels8120795
Grigoraș C-G, Simion A-I, Favier L, Drob C, Gavrilă L. Performance of Dye Removal from Single and Binary Component Systems by Adsorption on Composite Hydrogel Beads Derived from Fruits Wastes Entrapped in Natural Polymeric Matrix. Gels. 2022; 8(12):795. https://doi.org/10.3390/gels8120795
Chicago/Turabian StyleGrigoraș, Cristina-Gabriela, Andrei-Ionuț Simion, Lidia Favier, Cătălin Drob, and Lucian Gavrilă. 2022. "Performance of Dye Removal from Single and Binary Component Systems by Adsorption on Composite Hydrogel Beads Derived from Fruits Wastes Entrapped in Natural Polymeric Matrix" Gels 8, no. 12: 795. https://doi.org/10.3390/gels8120795
APA StyleGrigoraș, C.-G., Simion, A.-I., Favier, L., Drob, C., & Gavrilă, L. (2022). Performance of Dye Removal from Single and Binary Component Systems by Adsorption on Composite Hydrogel Beads Derived from Fruits Wastes Entrapped in Natural Polymeric Matrix. Gels, 8(12), 795. https://doi.org/10.3390/gels8120795










