Thermal Irreversibility in Nano-Enhanced Phase Change Material Liquefaction
Abstract
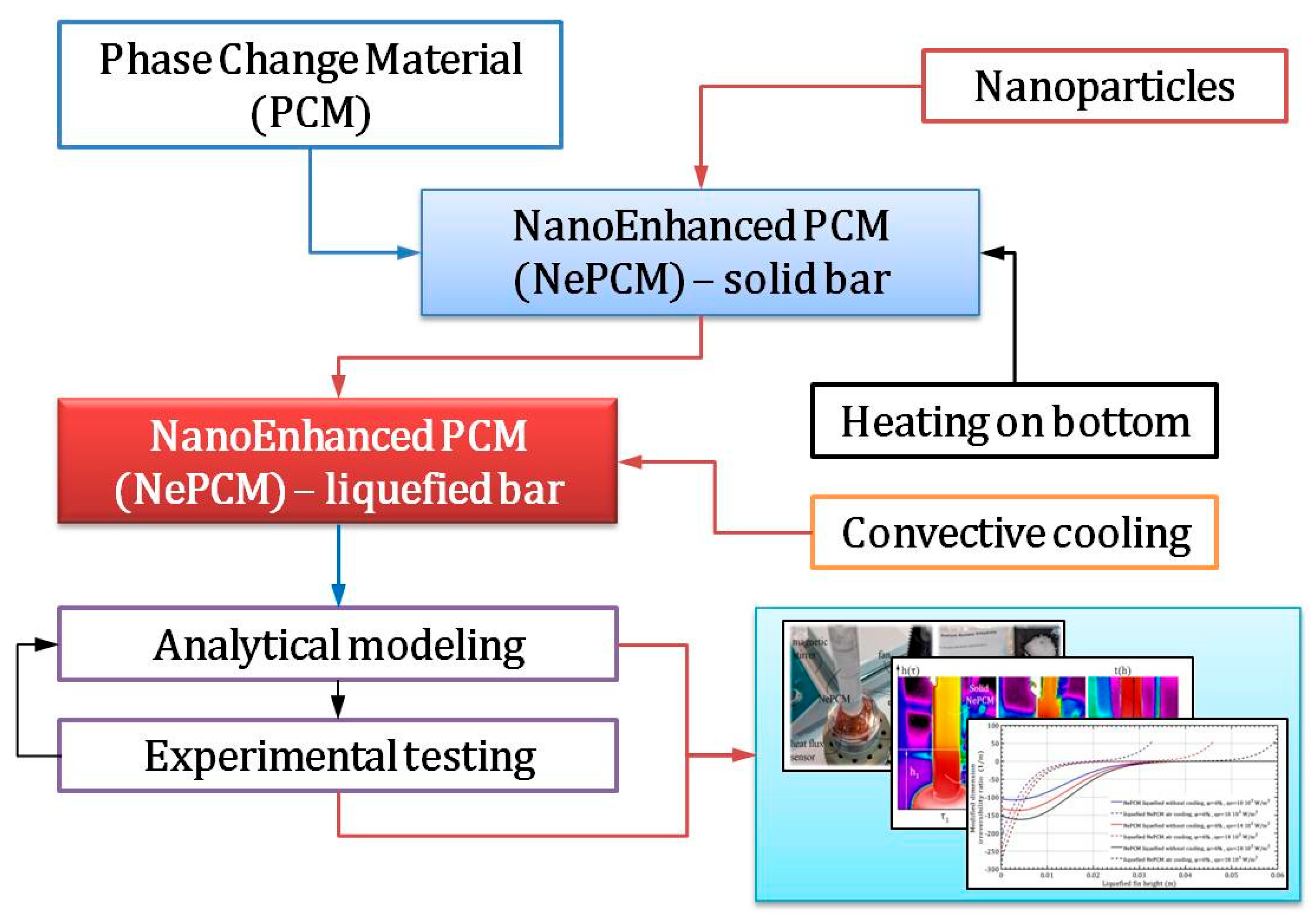
1. Introduction
1.1. The Methodology
1.2. Novelty and Objective
- -
- A well-defined methodology for analytically modeling transient thermal irreversibilities in a NePCM bar during combined conductive-convective heat transfer.
- -
- The ability to optimize process and geometric parameters of the NePCM bar by maximizing the modified irreversibility ratio.
- -
- The novelty covered by the established methodology is based on a combined analytical model and experimental testing that includes:
- -
- The transient temperature field of a NePCM bar during its liquefaction by an external heat source.
- -
- The transient temperature field of the liquefied NePCM bar during forced cooling of its outer surface.
- -
- Thermal transient irreversibilities are generated in both cases, which enable the establishment of an efficient optimization model based on minimizing them.
2. Methodology
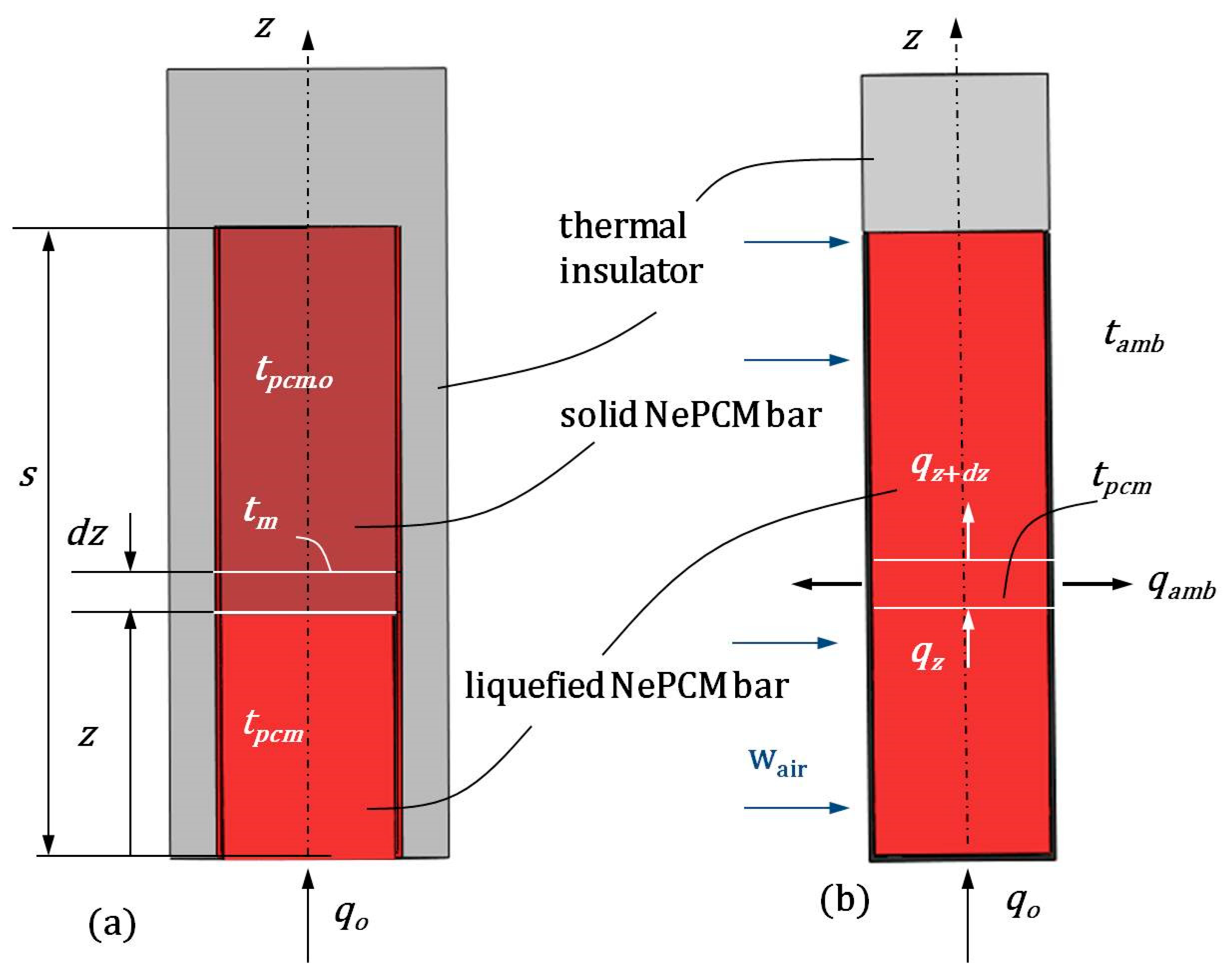
2.1. Temperature Distribution of a NePCM Cylindrical Bar
- -
- The temperature field of the cylindrical bar is one-dimensional and depends only on the z coordinate.
- -
- Incoming heat flux at the bottom of the bar is uniform across its circular cross-section.
- -
- The physical properties of NePCM are consistent throughout its volume.
- -
- The volume concentration of nanoparticles does not alter the liquefaction temperature of the NePCM bar.
2.2. Temperature Distribution During the Cooling Process of a Cylindrical Bar Using Liquefied NePCM
2.3. The Thermal Entropy of the Liquefied NePCM Bar Due to Heat Conduction
2.4. Entransy Dissipation Rate
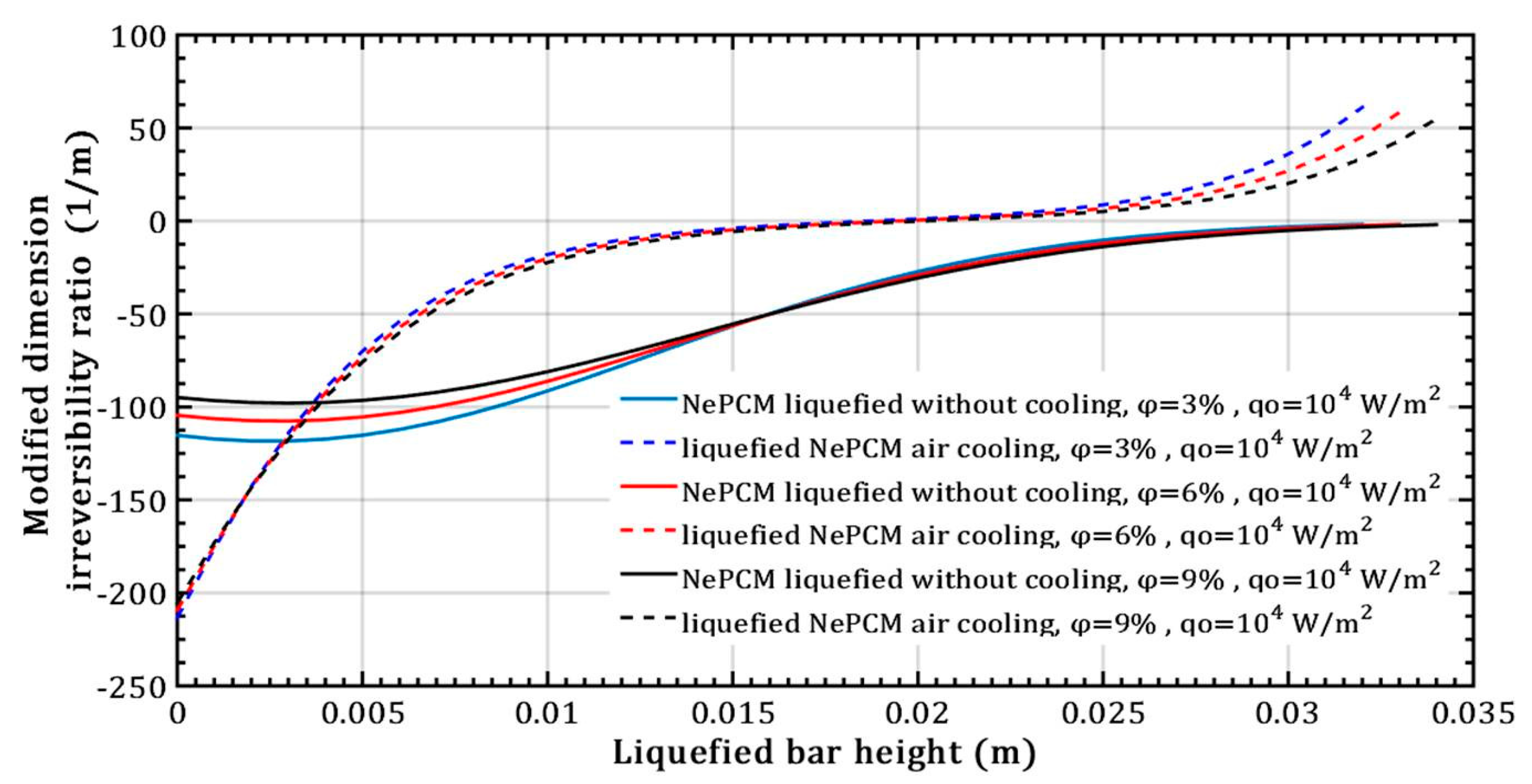
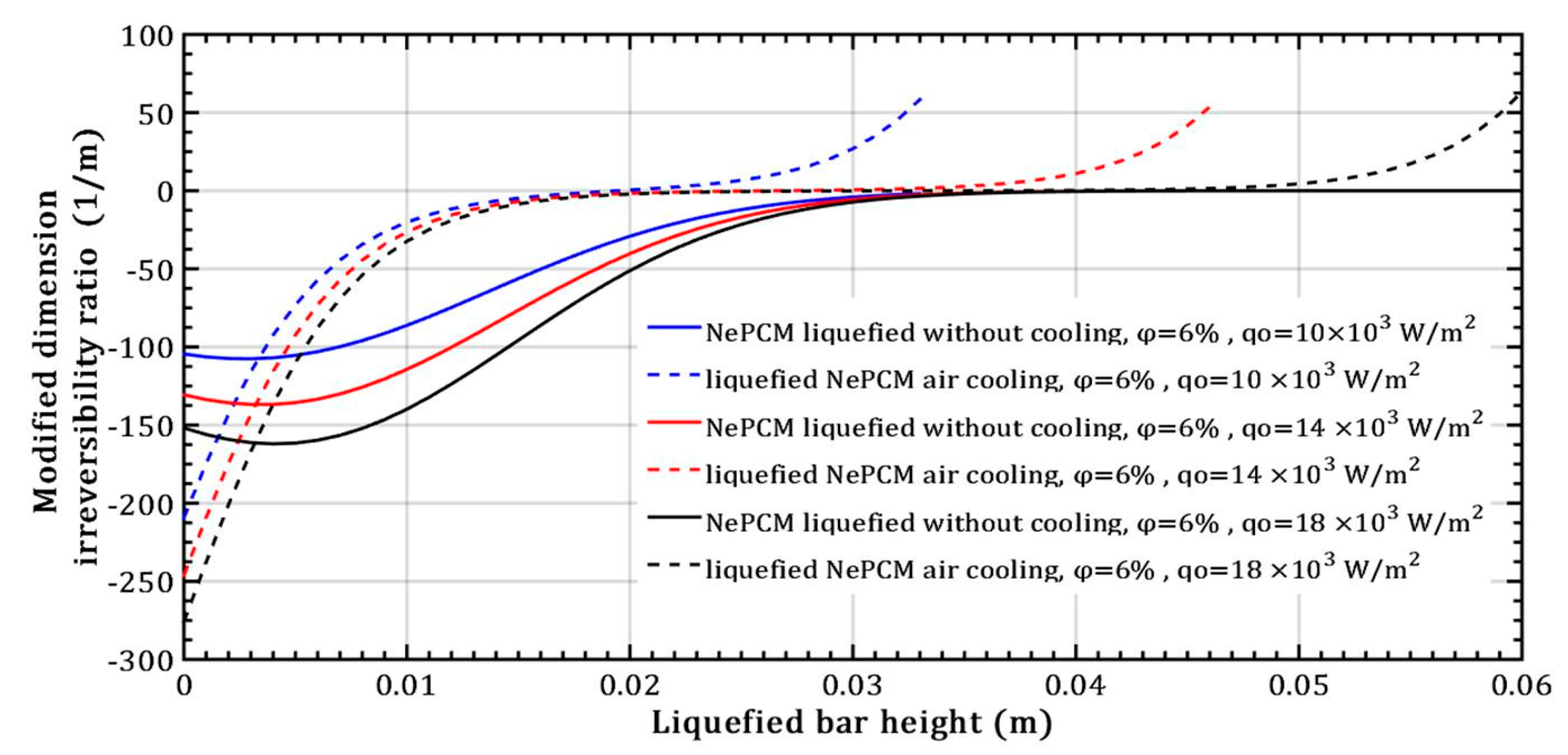
2.5. Modified Dimension Irreversibility Ratio
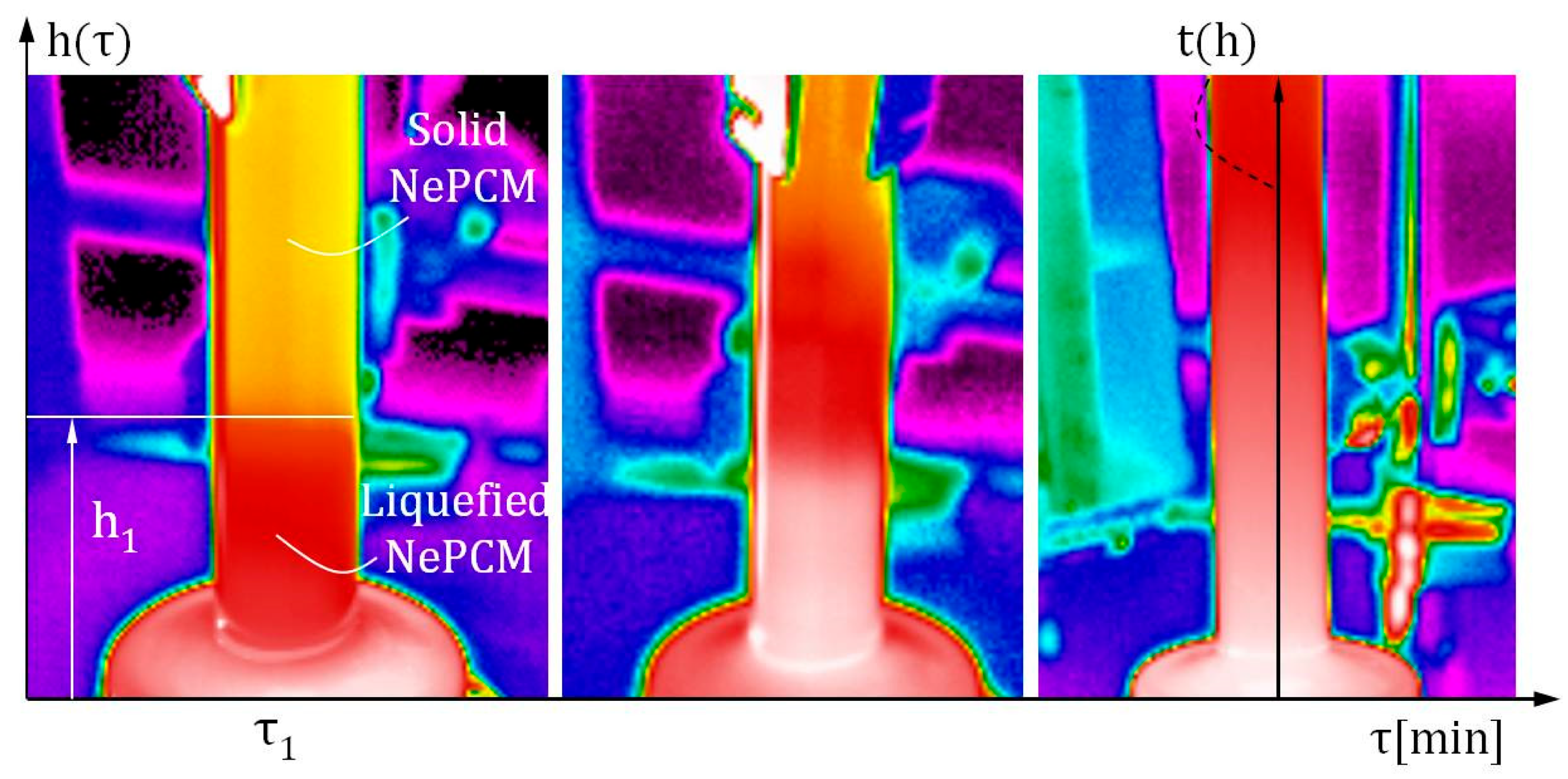
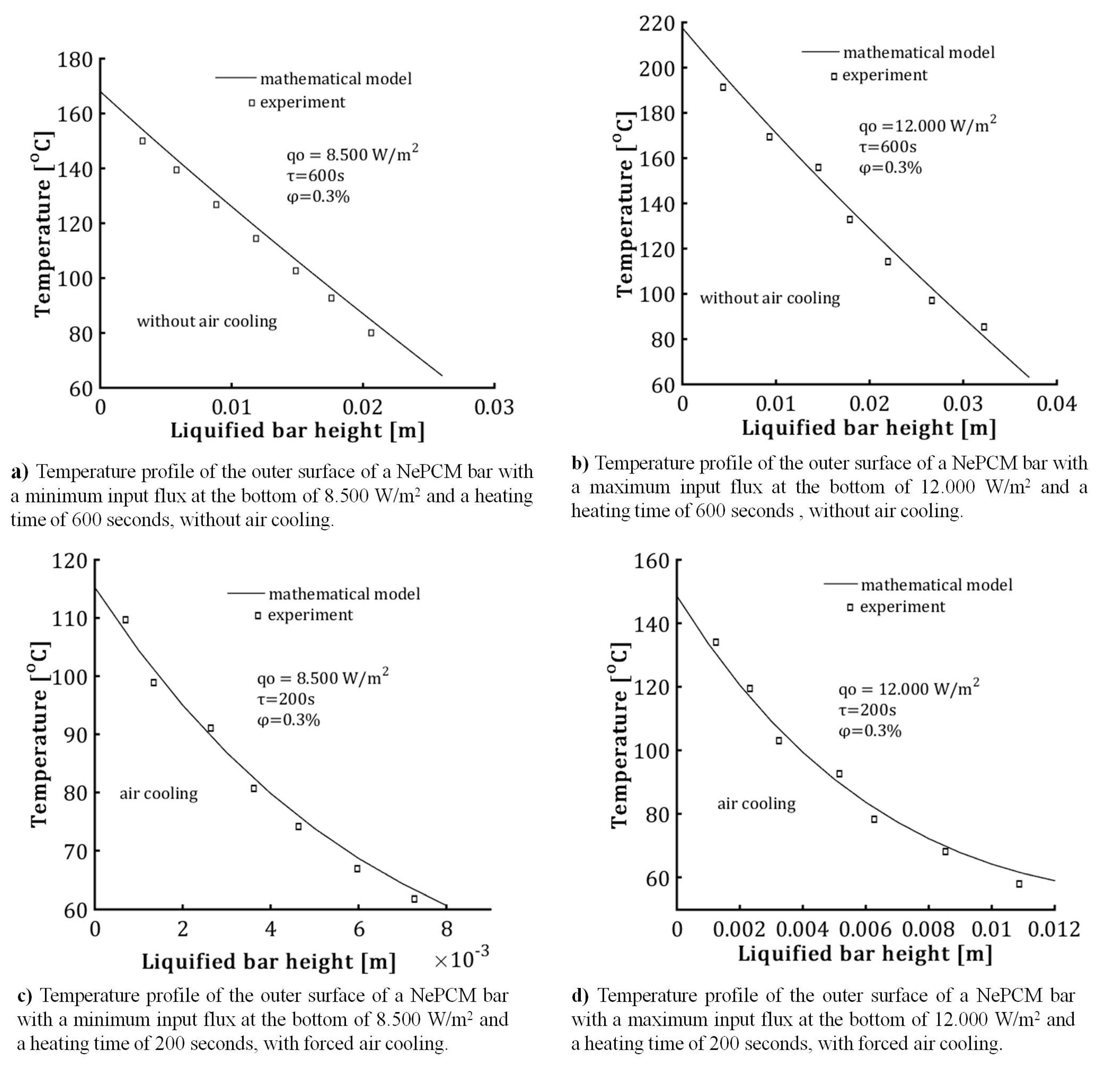
2.6. Experimental Testing
3. Results and Discussion
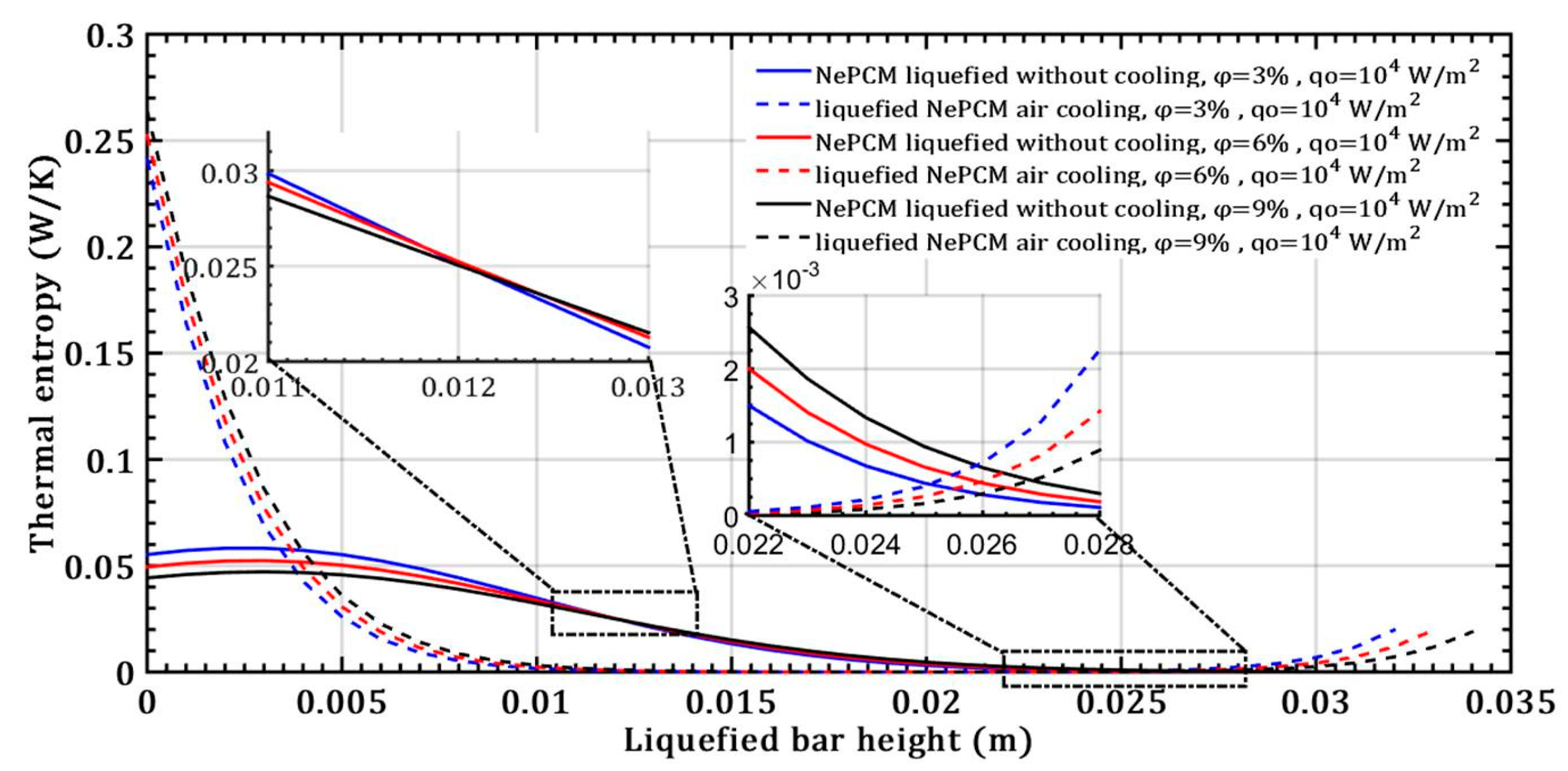
- -
- There is no effect on the thermal entropy of the liquefied NePCM bar when increasing the volume fraction ratio from 3% to 9%. This is observed at a constant heat flux of 104 Wm−2 and a height of the liquefied bar of 0.012 m.
- -
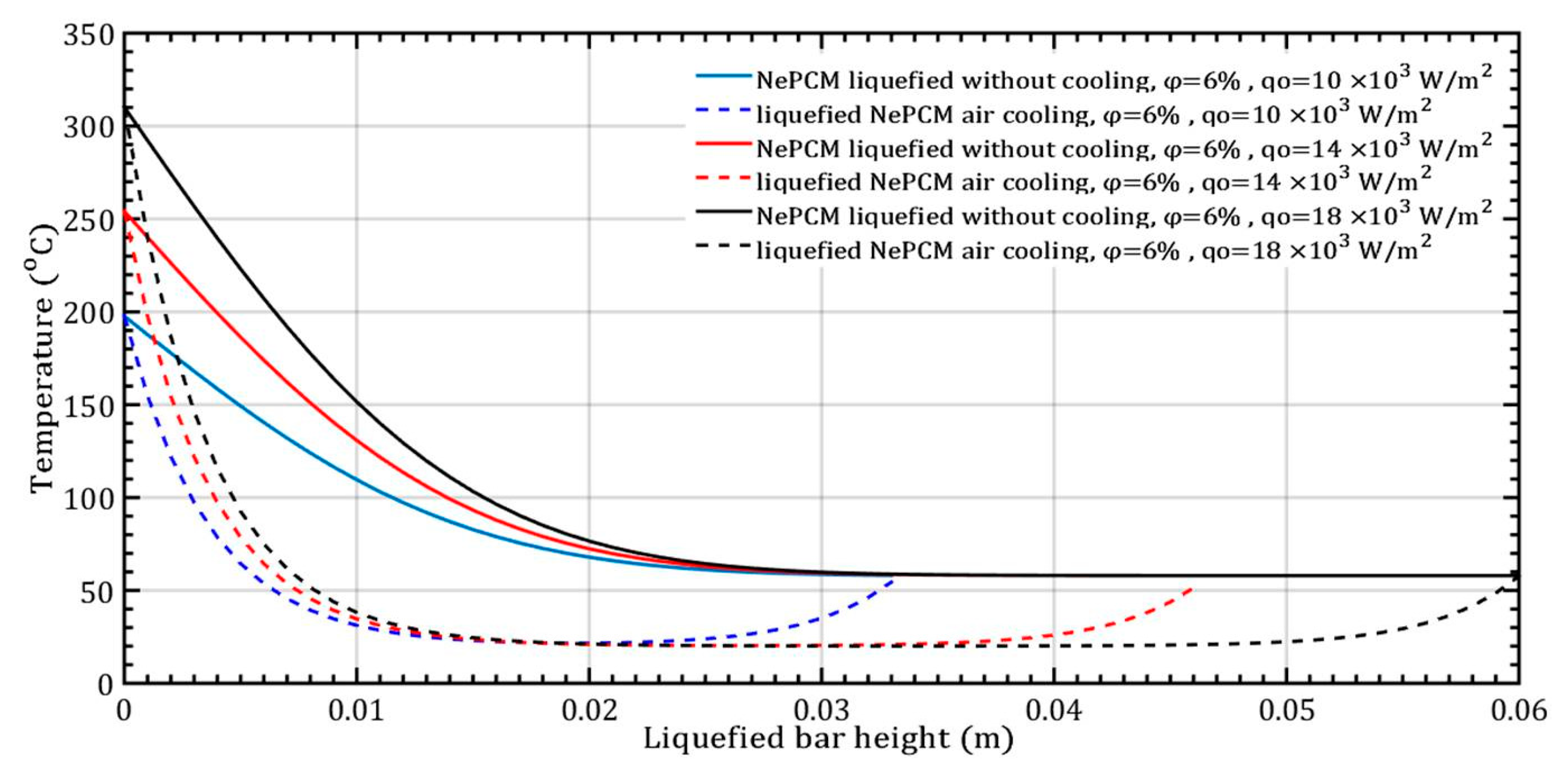
- However, with an increase in the volume fraction ratio from 3% to 9%, at a constant heat flux of 104 Wm−2 and a height of the liquefied bar of 0.02 m, the NePCM bar efficiency decreases by 99%.
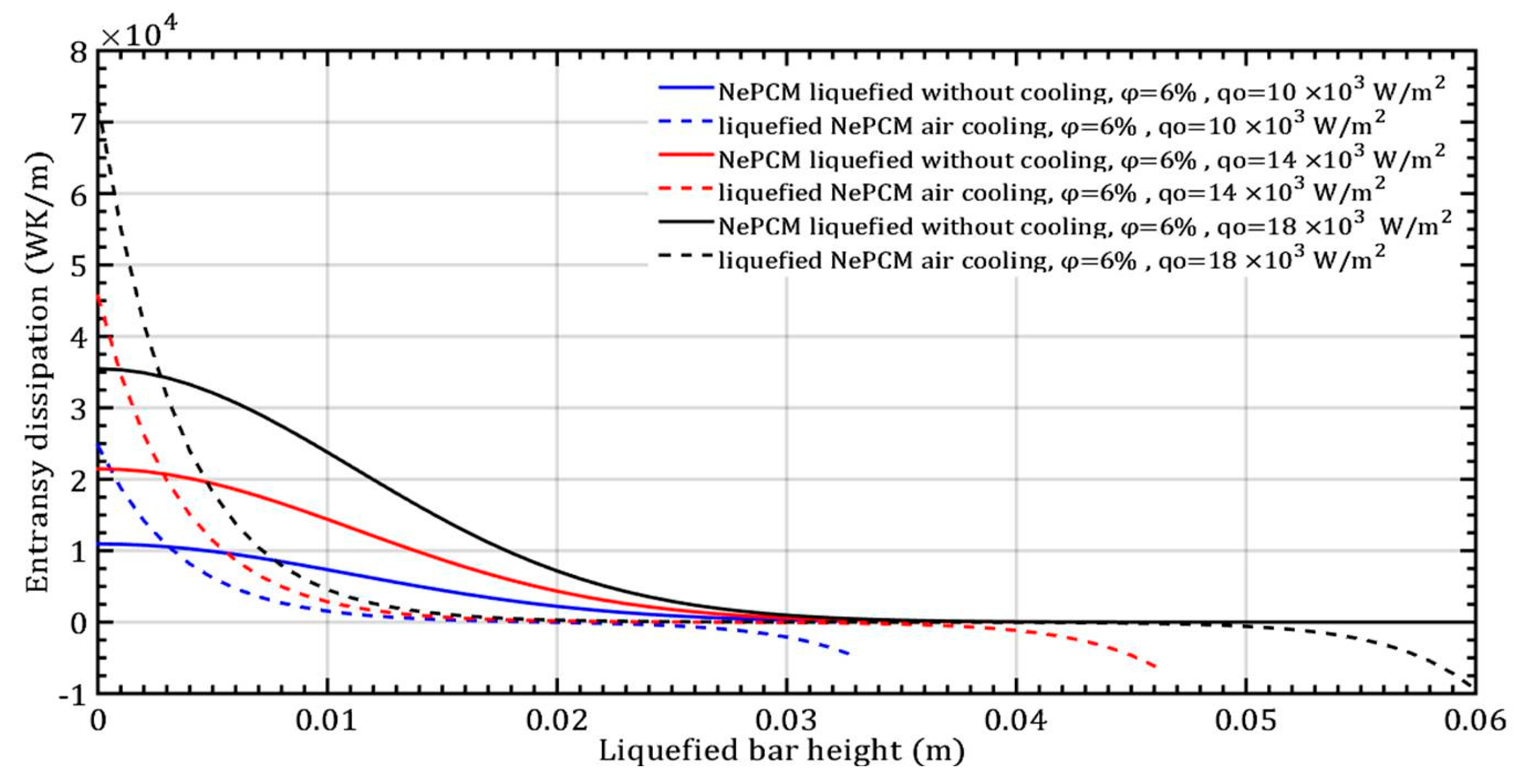
- -
- The entransy dissipation value decreases by 50% and is not affected by the volume fraction ratio at a liquefied bar height of 0.015 m and a heat flux of 104 Wm−2 for the case without bar cooling. However, with a liquefied bar height of 0.02 m and a heat flux of 104 Wm−2, entransy dissipation decreases by 100% with bar cooling, becoming negative for bar heights over 0.02 m.
- -
- The thermal entropy value of the liquefied NePCM bar increases by an average of 110% without cooling at a volume fraction ratio of 6% and an 80% increase in heat flux, with the bar height reaching up to 0.02 m.
- -
- The entransy dissipation ratio of the liquefied NePCM bar at 0.035 m without cooling is not affected by the increase in heat flux. However, with bar cooling the entransy dissipation decreases by about 98% when the heat flux increases by 80% and the bar height is up to 0.01 m.
4. Conclusions
- -
- Increasing the volume fraction ratio of Al2O3 nanoparticles in basic PCM leads to an increase in melting height and a decrease in the rate of forced convective air cooling of NePCM bars.
- -
- The transient thermal entropy of the liquefied NePCM bar is significantly lower when not cooled compared to when cooled using forced convective air cooling.
- -
- Changing the value of the input heat flux has a significant impact on both the intensity and rate of change of the transient thermal entropy.
- -
- When it comes to the efficiency of the NePCM bar, varying the input heat flux has no significant effect at lower heights of the liquefied NePCM bar.
- -
- The impact of the volume fraction ratio of Al2O3 nanoparticles on the entransy dissipation rate of NePCM bars is negligible compared to the variation in the input heat flux.
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| cpcm.o | specific heat capacity of PCM, kJkg−1K−1 |
| cp | specific heat capacity of nanoparticles, kJkg−1K−1 |
| cpcm | specific heat capacity of nano-enhanced phase change material, kJkg−1K−1 |
| ipcm | specific enthalpy of nano-enhanced phase change material, kJkg−1 |
| ip | specific enthalpy of nanoparticles, kJkg−1 |
| s | liquefied bar height, m |
| tpcm | temperature of liquefied bar in NePCM, °C |
| tpcm.o | temperature of solid bar in NePCM, °C |
| tm | temperature of phase change in PCM, °C |
| apcm | thermal diffusivity of nano-enhanced phase change material, m2 s−1 |
| tair | ambient air temperature, °C |
| Epcm.conv | entransy dissipation rate of NePCM in a liquefied bar is influenced by convective heat transfer, Wm−2K |
| Spcm.cond | thermal entropy of NePCM in a liquefied bar is influenced by conductive heat transfer, Wm−1K−1 |
| Spcm.conv | thermal entropy of NePCM in a liquefied bar is influenced by convective cooling, Wm−1K−1 |
| Epcm.cond | entransy dissipation rate of NePCM in a liquefied bar is influenced by conductive heat transfer, Wm−2K |
| Greek symbols | |
| φ | volume fraction ratio of nanoparticles,- |
| qo | inlet heat flux into the bottom of the bar, Wm−2 |
| τ | time, s |
| λpcm.o | thermal conductivity coefficient of PCM, Wm−1 K−1 |
| λpcm | thermal conductivity coefficient of NePCM, Wm−1 K−1 |
| λp | thermal conductivity coefficient of nanoparticles, Wm−1 K−1 |
| αpcm | convection heat transfer coefficient, Wm−2 K−1 |
| ρpcm.o | density of PCM, kgm−3 |
| ρpcm | density of NePCM, kgm−3 |
| ρp | density of nanoparticles, kgm−3 |
| ψ | dimension irreversibility ratio, K2 |
References
- Dhaidan, N.S.; Hassan, A.F.; Al-Gaheeshi, A.M.R.; Al-Mousawi, F.N.; Homod, R.Z. Experimental investigation of thermal characteristics of phase change material in finned heat exchangers. J. Energy Storage 2023, 71, 108162. [Google Scholar] [CrossRef]
- Sarani, I.; Payan, S.; Nada, S.A.; Payan, A. Numerical investigation of an innovative discontinuous distribution of fins for solidification rate enhancement in PCM with and without nanoparticles. Appl. Therm. Eng. 2020, 176, 115017. [Google Scholar] [CrossRef]
- Al-Salami, H.A.; Dhaidan, N.S.; Abbas, H.H.; Al-Mousawi, F.N.; Homod, R.Z. Review of PCM charging in latent heat thermal energy storage systems with fins. Therm. Sci. Eng. Prog. 2024, 51, 102640. [Google Scholar] [CrossRef]
- Shahsavar, A.; Shaham, A.; Yıldız, Ç.; Arıcı, M. Entropy generation characteristics of phase change material in a variable wavy walled triplex tube latent heat storage unit for battery thermal management system. J. Energy Storage 2022, 51, 104374. [Google Scholar] [CrossRef]
- Zhang, Z.; Duan, Z.; Wang, J. Sodium acetate trihydrate-based composite phase change material with enhanced thermal performance for energy storage. J. Energy Storage 2021, 34, 102186. [Google Scholar] [CrossRef]
- Sharma, S.; Tahir, A.A.; Reddy, K.S.; Mallick, T.K. Nano-enhanced Phase Change Material for thermal management of BICPV. Appl. Energy 2017, 208, 719–733. [Google Scholar] [CrossRef]
- Colla, L.; Fedele, L.; Mancin, S.; Danza, L.; Manca, O. Nano-PCMs for enhanced energy storage and passive cooling applications. Appl. Therm. Eng. 2017, 110, 584–589. [Google Scholar] [CrossRef]
- Leong, K.Y.; Rahman, M.R.A.; Gurunathan, B.A. Nano-enhanced phase change materials: A review of thermo-physical properties, applications and challenges. J. Energy Storage 2019, 21, 18–31. [Google Scholar] [CrossRef]
- He, M.; Yang, L.; Lin, W.; Chen, J.; Mao, X.; Ma, Z. Preparation, thermal characterization and examination of phase change materials (PCMs) enhanced by carbon-based nanoparticles for solar thermal energy storage. J. Energy Storage 2019, 25, 100874. [Google Scholar] [CrossRef]
- Dhaidan, N.S.; Khodadadi, J.M.; Al-Hattab, T.A.; Al-Mashat, S.M. Experimental and numerical investigation of melting of phase change material/nanoparticle suspensions in a square container subjected to a constant heat flux. Int. J. Heat Mass Transf. 2013, 66, 672–683. [Google Scholar] [CrossRef]
- Al-Jethelah, M.S.M.; Tasnim, S.H.; Mahmud, S.; Dutta, A. Melting of nano-phase change material inside a porous enclosure. Int. J. Heat Mass Transf. 2016, 102, 773–787. [Google Scholar] [CrossRef]
- Keshteli, A.N.; Iasiello, M.; Langella, G.; Bianco, N. Using metal foam and nanoparticle additives with different fin shapes for PCM-based thermal storage in flat plate solar collectors. Therm. Sci. Eng. Prog. 2024, 52, 102690. [Google Scholar]
- Sharma, A.; Kothadia, H.B.; Singh, S.; Mondal, B. Solidification of nanoparticle-based PCM in a fin-aided triplex-tube energy storage system for cooling applications. Therm. Sci. Eng. Prog. 2023, 42, 101872. [Google Scholar] [CrossRef]
- Zullo, F. Entropy Production in the Theory of Heat Conduction in Solids. Entropy 2016, 18, 87. [Google Scholar] [CrossRef]
- Din, Z.U.; Ali, A.; De la Sen, M.; Zaman, G. Entropy generation from convective–radiative moving exponential porous bars with variable thermal conductivity and internal heat generations. Sci. Rep. 2022, 12, 1791. [Google Scholar] [CrossRef]
- Tian, X.; Wang, L. Heat conduction in cylinders: Entropy generation and mathematical inequalities. Int. J. Heat Mass Transf. 2018, 121, 1137–1145. [Google Scholar] [CrossRef]
- Alic, F. The modified dimension and dimensionless irreversibility analysis of low conductivity material of concentric hollow cylinders. J. Therm. Anal. Calorim. 2022, 147, 14555–14569. [Google Scholar] [CrossRef]
- Aziz, A.; Khan, W.A. Classical and minimum entropy generation analyses for steady state conduction with temperature dependent thermal conductivity and asymmetric thermal boundary conditions: Regular and functionally graded materials. Energy 2011, 36, 6195–6207. [Google Scholar] [CrossRef]
- Alic, F. Transient Entropy Generation Analysis of Liquid vortex Isolated by Hollow Heated Cylinder. Appl. Math. Model. 2017, 44, 321–335. [Google Scholar] [CrossRef]
- Chen, C.; Wang, J.; Zhao, H.; Yu, Z.; Han, J.; Chen, J.; Liu, C. Entropy Flow Analysis of Thermal Transmission Process in Integrated Energy System Part I: Theoretical Approach Study. Processes 2022, 10, 1717. [Google Scholar] [CrossRef]
- Lu, D.; Afridi, M.I.; Allauddin, U.; Farooq, U.; Qasim, M. Entropy Generation in a Dissipative Nanofluid Flow under the Influence of Magnetic Dissipation and Transpiration. Energies 2020, 13, 5506. [Google Scholar] [CrossRef]
- Alic, F. The non-dimensional analysis of nanofluid irreversibility within novel adaptive process electric heaters. Appl. Therm. Eng. 2019, 152, 13–23. [Google Scholar] [CrossRef]
- Guelpa, E.; Sciacovelli, A.; Verda, V. Entropy generation analysis for the design improvement of a latent heat storage system. Energy 2013, 53, 128–138. [Google Scholar] [CrossRef]
- Zadeh, S.M.H.; Mehryan, S.A.M.; Sheremet, M.; Ghodrat, M.; Ghalambaz, M. Thermo-hydrodynamic and entropy generation analysis of a dilute aqueous suspension enhanced with nano-encapsulated phase change material. Int. J. Mech. Sci. 2020, 178, 105609. [Google Scholar]
- Guo, Z.Y.; Zhu, H.Y.; Liang, X.G. Entransy-A Physical Quantity describing Heat Transfer Ability. Int. J. Heat Mass Transf. 2007, 50, 2545–2556. [Google Scholar] [CrossRef]
- Cheng, X.; Zhang, Q.; Liang, X. Analyses of entransy dissipation, entropy generation and entransy-dissipation-based thermal resistance on heat exchanger optimization. Appl. Therm. Eng. 2012, 38, 31–39. [Google Scholar] [CrossRef]
- Brinkman, H.C. The viscosity of concentrated suspensions and solution. J. Chem. Phys. 1952, 20, 571–581. [Google Scholar] [CrossRef]
- Pak, B.C.; Cho, Y.I. Hydrodynamic and heat transfer study of dispersed fluids with submicron metallic oxide particles. Exp. Heat Transf. 1998, 11, 151–170. [Google Scholar] [CrossRef]
- Maxwell, J.C.A. Treatise on Electricity and Magnetism; Clarendon Press: Oxford, UK, 1881. [Google Scholar]
- Yu, W.; Choi, S.U.S. The role of interfacial layers in the enhanced thermal conductivity of nanofluids: A renovated Maxwell model. J. Nanopart. Res. 2003, 5, 167–171. [Google Scholar] [CrossRef]
- Hamilton, R.; Crosser, O.K. Thermal Conductivity of Heterogeneous Two-Component Systems. Ind. Eng. Chem. Fundam. 1962, 1, 187–191. [Google Scholar] [CrossRef]
- Warrier, P.; Teja, A. Effect of particle size on the thermal conductivity of nanofluids containing metallic nanoparticles. Nanoscale Res. Lett. 2011, 6, 247. [Google Scholar] [CrossRef] [PubMed]
- Alic, F. Entransy Dissipation Analysis and New Irreversibility Dimension Ratio of Nanofluid Flow. Energies 2020, 13, 114. [Google Scholar] [CrossRef]
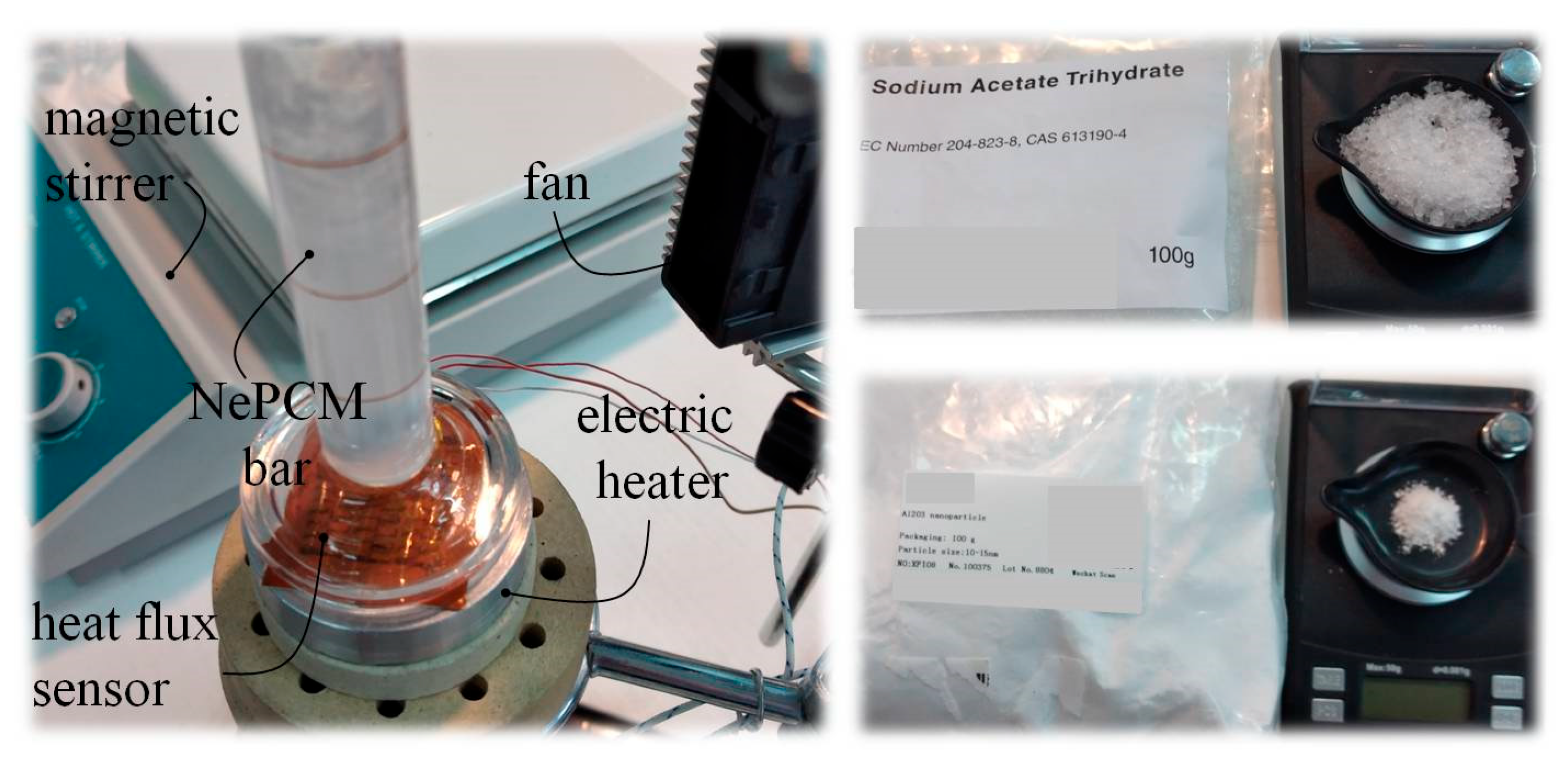





| Properties of Nanoparticles (Al2O3)s (Al2O3) | Properties of the Base PCM—Sodium Acetate Trihydrate | |||||
|---|---|---|---|---|---|---|
| λp [Wm−1K−1] | ρp [kgm−3] | cp [Jkg−1K−1] | ibf [kJkg−1] | λbf [Wm−1K−1] | ρbf [kgm−3] | cbf [Jkg−1K−1] |
| 40 | 3970 | 756 | 264 | 0.387 | 1450 | 3100 |
| Control Parameter | Value |
|---|---|
| heat flux | 8500 Wm2, 10,000 Wm2, 12,000 Wm2, 14,000 Wm2, 18,000 Wm2 |
| heating time | 200 s, 500 s, 600 s |
| volume fraction ratio of Al2O3 nanoparticles | 3%, 6%, 9% |
| liquified bar height | to 0.035 m |
| bottom bar temperature | from 115 °C to 350 °C |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alić, F. Thermal Irreversibility in Nano-Enhanced Phase Change Material Liquefaction. Fluids 2025, 10, 102. https://doi.org/10.3390/fluids10040102
Alić F. Thermal Irreversibility in Nano-Enhanced Phase Change Material Liquefaction. Fluids. 2025; 10(4):102. https://doi.org/10.3390/fluids10040102
Chicago/Turabian StyleAlić, Fikret. 2025. "Thermal Irreversibility in Nano-Enhanced Phase Change Material Liquefaction" Fluids 10, no. 4: 102. https://doi.org/10.3390/fluids10040102
APA StyleAlić, F. (2025). Thermal Irreversibility in Nano-Enhanced Phase Change Material Liquefaction. Fluids, 10(4), 102. https://doi.org/10.3390/fluids10040102






