A Relaxation Filtering Approach for Two-Dimensional Rayleigh–Taylor Instability-Induced Flows
Abstract
:1. Introduction
2. Governing Equations
3. Numerical Methods
3.1. ILES Approach
3.1.1. Weno Reconstruction
3.1.2. Roe Riemann Solver
3.1.3. Rusanov Riemann Solver
3.2. Central Scheme with Relaxation Filtering (Cs+Rf) Approach
4. Results
4.1. Two-Dimensional RTI Test Problem: Case Setup
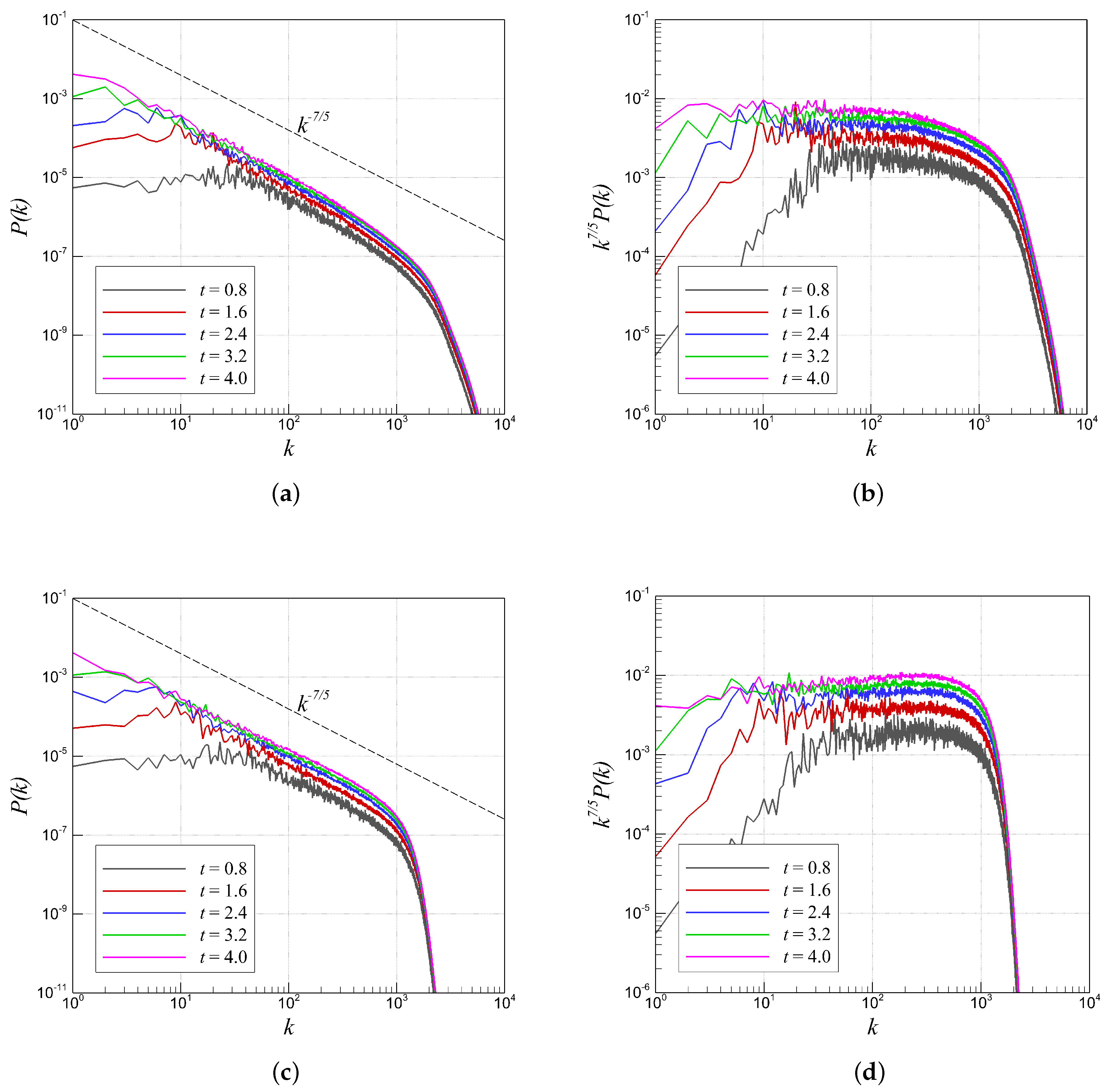
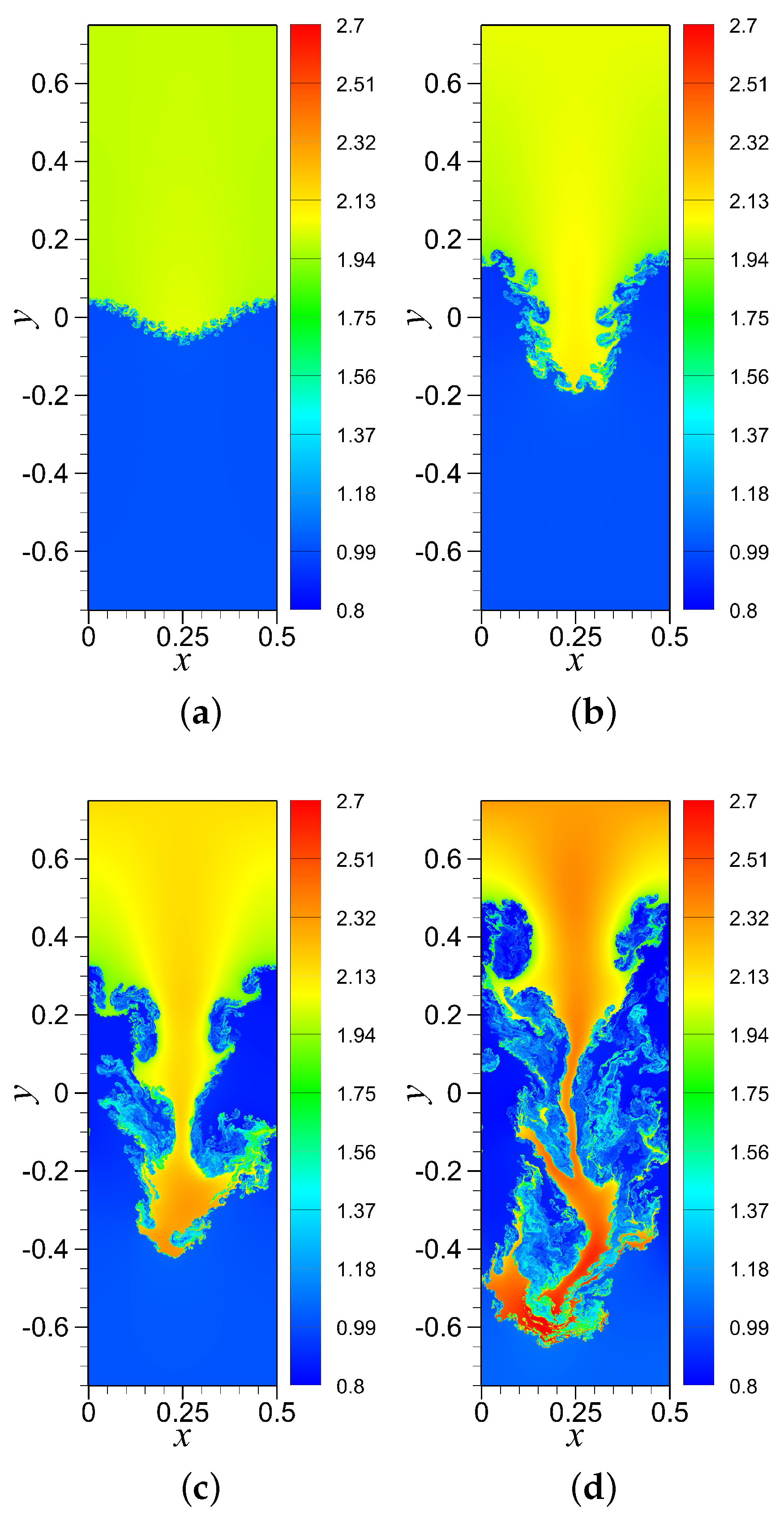
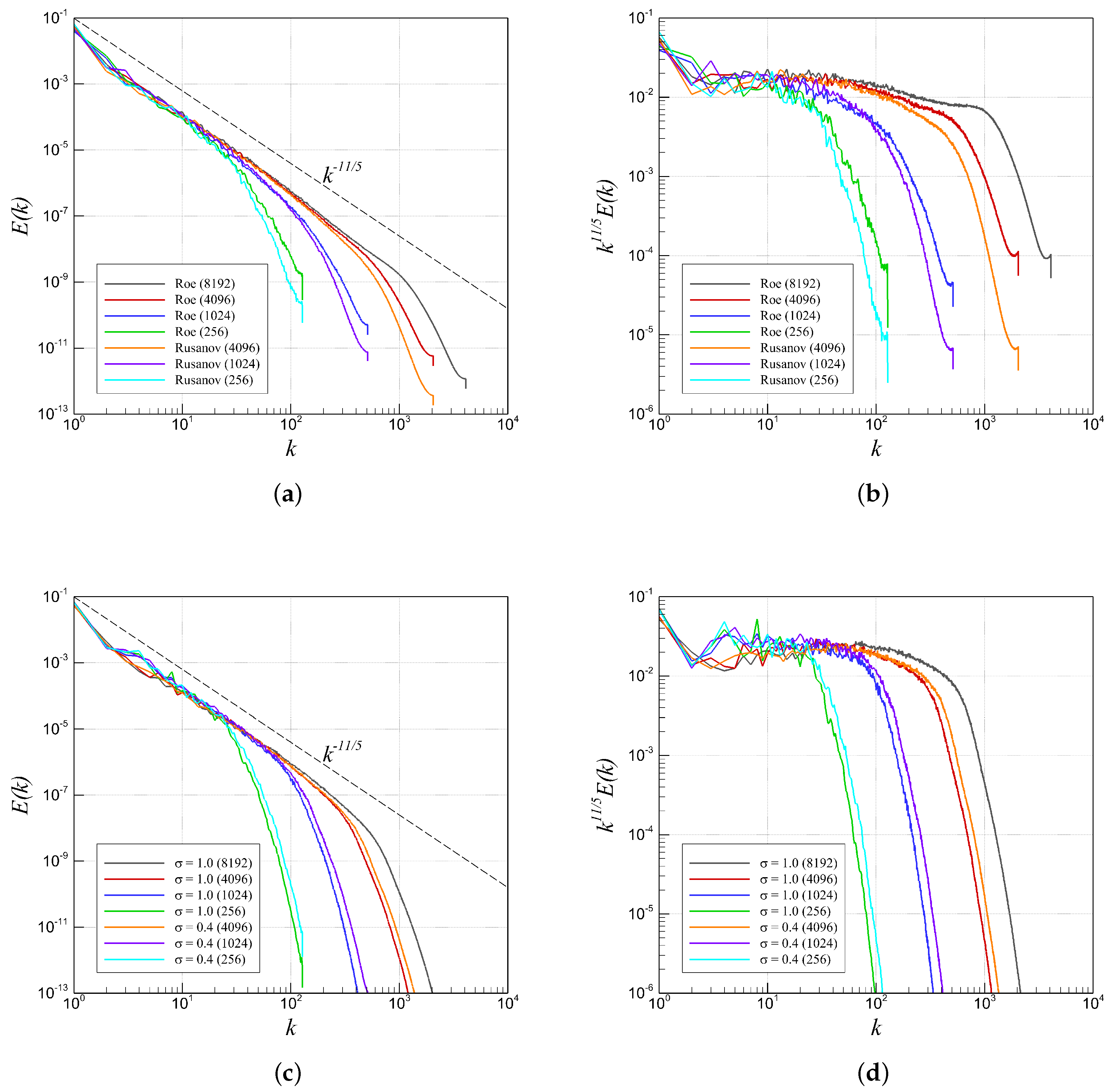
4.2. RTI with Random (Multi-Mode) Perturbation
4.3. RTI with Single-Mode Perturbation
5. Summary and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Taylor, G.I. The instability of liquid surfaces when accelerated in a direction perpendicular to their planes. I. Proc. R. Soc. Lond. Ser. Math. Phys. Sci. 1950, 201, 192–196. [Google Scholar]
- Cui, A.; Street, R.L. Large-eddy simulation of coastal upwelling flow. Environ. Fluid Mech. 2004, 4, 197–223. [Google Scholar] [CrossRef]
- Jevons, W.S., II. On the cirrous form of cloud. Lond. Edinburgh Dublin Philos. Mag. J. Sci. 1857, 14, 22–35. [Google Scholar] [CrossRef]
- Bradley, P. The effect of mix on capsule yields as a function of shell thickness and gas fill. Phys. Plasmas 2014, 21, 062703. [Google Scholar] [CrossRef]
- Bell, J.; Day, M.; Rendleman, C.; Woosley, S.; Zingale, M. Direct numerical simulations of type Ia supernovae flames. II. The Rayleigh-Taylor instability. Astrophys. J. 2004, 608, 883. [Google Scholar] [CrossRef]
- Hillebrandt, W.; Niemeyer, J.C. Type Ia supernova explosion models. Annu. Rev. Astron. Astrophys. 2000, 38, 191–230. [Google Scholar] [CrossRef]
- Racca, R.A.; Annett, C.H. Simple demonstration of Rayleigh-Taylor instability. Am. J. Phys. 1985, 53, 484–486. [Google Scholar] [CrossRef]
- Chertkov, M.; Lebedev, V.; Vladimirova, N. Reactive Rayleigh–Taylor turbulence. J. Fluid Mech. 2009, 633, 1–16. [Google Scholar] [CrossRef]
- Veynante, D.; Trouvé, A.; Bray, K.; Mantel, T. Gradient and counter-gradient scalar transport in turbulent premixed flames. J. Fluid Mech. 1997, 332, 263–293. [Google Scholar] [CrossRef]
- Petrasso, R.D. Rayleigh’s challenge endures. Nature 1994, 367, 217. [Google Scholar] [CrossRef]
- Lewis, D.J. The instability of liquid surfaces when accelerated in a direction perpendicular to their planes. II. Proc. R. Soc. Lond. Ser. Math. Phys. Sci. 1950, 202, 81–96. [Google Scholar]
- Lord, R. Investigation of the character of the equilibrium of an incompressible heavy fluid of variable density. Sci. Pap. 1900, 200–207. [Google Scholar]
- Zhou, Y. Rayleigh–Taylor and Richtmyer–Meshkov instability induced flow, turbulence, and mixing. I. Phys. Rep. 2017, 720–722, 1–136. [Google Scholar]
- Zhou, Y. Rayleigh–Taylor and Richtmyer–Meshkov instability induced flow, turbulence, and mixing. II. Phys. Rep. 2017, 723–725, 1–160. [Google Scholar] [CrossRef]
- Piriz, A.; Cortazar, O.; Lopez Cela, J.; Tahir, N. The Rayleigh-Taylor instability. Am. J. Phys. 2006, 74, 1095–1098. [Google Scholar] [CrossRef]
- Boffetta, G.; Mazzino, A. Incompressible Rayleigh–Taylor turbulence. Annu. Rev. Fluid Mech. 2017, 49, 119–143. [Google Scholar] [CrossRef]
- Sharp, D.H. Overview of Rayleigh-Taylor Instability; Technical Report; Los Alamos National Lab.: Los Alamos, NM, USA, 1983. [Google Scholar]
- Dimonte, G.; Youngs, D.; Dimits, A.; Weber, S.; Marinak, M.; Wunsch, S.; Garasi, C.; Robinson, A.; Andrews, M.; Ramaprabhu, P.; et al. A comparative study of the turbulent Rayleigh–Taylor instability using high-resolution three-dimensional numerical simulations: The Alpha-Group collaboration. Phys. Fluids 2004, 16, 1668–1693. [Google Scholar] [CrossRef]
- Mueschke, N.J.; Schilling, O. Investigation of Rayleigh–Taylor turbulence and mixing using direct numerical simulation with experimentally measured initial conditions. I. Comparison to experimental data. Phys. Fluids 2009, 21, 014106. [Google Scholar] [CrossRef]
- Wei, T.; Livescu, D. Late-time quadratic growth in single-mode Rayleigh-Taylor instability. Phys. Rev. E 2012, 86, 046405. [Google Scholar] [CrossRef]
- Dalziel, S.; Linden, P.; Youngs, D. Self-similarity and internal structure of turbulence induced by Rayleigh–Taylor instability. J. Fluid Mech. 1999, 399, 1–48. [Google Scholar] [CrossRef]
- Ristorcelli, J.; Clark, T. Rayleigh–Taylor turbulence: Self-similar analysis and direct numerical simulations. J. Fluid Mech. 2004, 507, 213–253. [Google Scholar] [CrossRef]
- Xin, J.; Yan, R.; Wan, Z.H.; Sun, D.J.; Zheng, J.; Zhang, H.; Aluie, H.; Betti, R. Two mode coupling of the ablative Rayleigh-Taylor instabilities. Phys. Plasmas 2019, 26, 032703. [Google Scholar] [CrossRef]
- Jun, B.I.; Norman, M.L.; Stone, J.M. A numerical study of Rayleigh-Taylor instability in magnetic fluids. Astrophys. J. 1995, 453, 332. [Google Scholar] [CrossRef]
- Cabot, W. Comparison of two-and three-dimensional simulations of miscible Rayleigh-Taylor instability. Phys. Fluids 2006, 18, 045101. [Google Scholar] [CrossRef]
- Anuchina, N.; Volkov, V.; Gordeychuk, V.; Es’ kov, N.; Ilyutina, O.; Kozyrev, O. Numerical simulations of Rayleigh–Taylor and Richtmyer–Meshkov instability using MAH-3 code. J. Comput. Appl. Math. 2004, 168, 11–20. [Google Scholar] [CrossRef]
- Zhao, D.; Aluie, H. Inviscid criterion for decomposing scales. Phys. Rev. Fluids 2018, 3, 054603. [Google Scholar] [CrossRef]
- Livescu, D.; Ristorcelli, J. Buoyancy-driven variable-density turbulence. J. Fluid Mech. 2007, 591, 43–71. [Google Scholar] [CrossRef]
- Kokkinakis, I.; Drikakis, D.; Youngs, D.; Williams, R. Two-equation and multi-fluid turbulence models for Rayleigh–Taylor mixing. Int. J. Heat Fluid Flow 2015, 56, 233–250. [Google Scholar] [CrossRef]
- Youngs, D. Large eddy simulation and 1D/2D engineering models for Rayleigh-Taylor mixing. In Proceedings of the IWPCTM: 12th International Workshop on the Physics of Compressible Turbulent Mixing, Moscow, Russia, 12–17 July 2010; pp. 12–17. [Google Scholar]
- Ramaprabhu, P.; Dimonte, G.; Woodward, P.; Fryer, C.; Rockefeller, G.; Muthuraman, K.; Lin, P.H.; Jayaraj, J. The late-time dynamics of the single-mode Rayleigh-Taylor instability. Phys. Fluids 2012, 24, 074107. [Google Scholar] [CrossRef]
- Glimm, J.; Li, X.l.; Lin, A.D. Nonuniform approach to terminal velocity for single mode Rayleigh-Taylor instability. Acta Math. Appl. Sin. 2002, 18, 1–8. [Google Scholar] [CrossRef]
- Daly, B.J. Numerical study of two fluid Rayleigh-Taylor instability. Phys. Fluids 1967, 10, 297–307. [Google Scholar] [CrossRef]
- Kane, J.; Arnett, D.; Remington, B.; Glendinning, S.; Bazán, G.; Müller, E.; Fryxell, B.; Teyssier, R. Two-dimensional versus three-dimensional supernova hydrodynamic instability growth. Astrophys. J. 2000, 528, 989. [Google Scholar] [CrossRef]
- Goncharov, V. Analytical model of nonlinear, single-mode, classical Rayleigh-Taylor instability at arbitrary Atwood numbers. Phys. Rev. Lett. 2002, 88, 134502. [Google Scholar] [CrossRef]
- Young, Y.N.; Tufo, H.; Dubey, A.; Rosner, R. On the miscible Rayleigh–Taylor instability: Two and three dimensions. J. Fluid Mech. 2001, 447, 377–408. [Google Scholar] [CrossRef]
- Youngs, D.L. Three-dimensional numerical simulation of turbulent mixing by Rayleigh–Taylor instability. Phys. Fluids A Fluid Dyn. 1991, 3, 1312–1320. [Google Scholar] [CrossRef]
- Youngs, D.L. Numerical simulation of mixing by Rayleigh–Taylor and Richtmyer–Meshkov instabilities. Laser Part. Beams 1994, 12, 725–750. [Google Scholar] [CrossRef]
- Shvarts, D.; Alon, U.; Ofer, D.; McCrory, R.; Verdon, C. Nonlinear evolution of multimode Rayleigh–Taylor instability in two and three dimensions. Phys. Plasmas 1995, 2, 2465–2472. [Google Scholar] [CrossRef]
- Oron, D.; Arazi, L.; Kartoon, D.; Rikanati, A.; Alon, U.; Shvarts, D. Dimensionality dependence of the Rayleigh–Taylor and Richtmyer–Meshkov instability late-time scaling laws. Phys. Plasmas 2001, 8, 2883–2889. [Google Scholar] [CrossRef]
- Zhou, Y. A scaling analysis of turbulent flows driven by Rayleigh–Taylor and Richtmyer–Meshkov instabilities. Phys. Fluids 2001, 13, 538–543. [Google Scholar] [CrossRef]
- Shvarts, D.; Oron, D.; Kartoon, D.; Rikanati, A.; Sadot, O.; Srebro, Y.; Yedvab, Y.; Ofer, D.; Levin, A.; Sarid, E.; et al. Scaling laws of nonlinear Rayleigh-Taylor and Richtmyer-Meshkov instabilities in two and three dimensions (IFSA 1999). In Edward Teller Lectures: Lasers and Inertial Fusion Energy; World Scientific: Singapore, 2016; pp. 253–260. [Google Scholar]
- Chertkov, M. Phenomenology of Rayleigh-Taylor turbulence. Phys. Rev. Lett. 2003, 91, 115001. [Google Scholar] [CrossRef]
- Celani, A.; Mazzino, A.; Vozella, L. Rayleigh-Taylor turbulence in two dimensions. Phys. Rev. Lett. 2006, 96, 134504. [Google Scholar] [CrossRef]
- Margolin, L. The reality of artificial viscosity. Shock Waves 2019, 29, 27–35. [Google Scholar] [CrossRef]
- Brehm, C.; Barad, M.F.; Housman, J.A.; Kiris, C.C. A comparison of higher-order finite-difference shock capturing schemes. Comput. Fluids 2015, 122, 184–208. [Google Scholar] [CrossRef]
- Margolin, L.G.; Rider, W.J.; Grinstein, F.F. Modeling turbulent flow with implicit LES. J. Turbul. 2006, 7, N15. [Google Scholar] [CrossRef]
- Zhou, Y.; Thornber, B. A comparison of three approaches to compute the effective Reynolds number of the implicit large-eddy simulations. J. Fluids Eng. 2016, 138, 070905. [Google Scholar] [CrossRef]
- Maulik, R.; San, O. Resolution and energy dissipation characteristics of implicit LES and explicit filtering models for compressible turbulence. Fluids 2017, 2, 14. [Google Scholar] [CrossRef]
- San, O.; Kara, K. Numerical assessments of high-order accurate shock capturing schemes: Kelvin–Helmholtz type vortical structures in high-resolutions. Comput. Fluids 2014, 89, 254–276. [Google Scholar] [CrossRef]
- Titarev, V.A.; Toro, E.F. Finite-volume WENO schemes for three-dimensional conservation laws. J. Comput. Phys. 2004, 201, 238–260. [Google Scholar] [CrossRef]
- Pirozzoli, S. Numerical methods for high-speed flows. Annu. Rev. Fluid Mech. 2011, 43, 163–194. [Google Scholar] [CrossRef]
- Brehm, C. On consistent boundary closures for compact finite-difference WENO schemes. J. Comput. Phys. 2017, 334, 573–581. [Google Scholar] [CrossRef]
- Wong, M.L.; Lele, S.K. High-order localized dissipation weighted compact nonlinear scheme for shock-and interface-capturing in compressible flows. J. Comput. Phys. 2017, 339, 179–209. [Google Scholar] [CrossRef]
- Zhao, S.; Lardjane, N.; Fedioun, I. Comparison of improved finite-difference WENO schemes for the implicit large eddy simulation of turbulent non-reacting and reacting high-speed shear flows. Comput. Fluids 2014, 95, 74–87. [Google Scholar] [CrossRef]
- Hu, X.; Wang, Q.; Adams, N.A. An adaptive central-upwind weighted essentially non-oscillatory scheme. J. Comput. Phys. 2010, 229, 8952–8965. [Google Scholar] [CrossRef]
- Mathew, J.; Foysi, H.; Friedrich, R. A new approach to LES based on explicit filtering. Int. J. Heat Fluid Flow 2006, 27, 594–602. [Google Scholar] [CrossRef]
- Lund, T. The use of explicit filters in large eddy simulation. Comput. Math. Appl. 2003, 46, 603–616. [Google Scholar] [CrossRef]
- Berland, J.; Lafon, P.; Daude, F.; Crouzet, F.; Bogey, C.; Bailly, C. Filter shape dependence and effective scale separation in large-eddy simulations based on relaxation filtering. Comput. Fluids 2011, 47, 65–74. [Google Scholar] [CrossRef]
- Bose, S.T.; Moin, P.; You, D. Grid-independent large-eddy simulation using explicit filtering. Phys. Fluids 2010, 22, 105103. [Google Scholar] [CrossRef]
- Vasilyev, O.V.; Lund, T.S.; Moin, P. A general class of commutative filters for LES in complex geometries. J. Comput. Phys. 1998, 146, 82–104. [Google Scholar] [CrossRef]
- San, O. Analysis of low-pass filters for approximate deconvolution closure modelling in one-dimensional decaying Burgers turbulence. Int. J. Comput. Fluid Dyn. 2016, 30, 20–37. [Google Scholar] [CrossRef]
- Najafi-Yazdi, A.; Najafi-Yazdi, M.; Mongeau, L. A high resolution differential filter for large eddy simulation: Toward explicit filtering on unstructured grids. J. Comput. Phys. 2015, 292, 272–286. [Google Scholar] [CrossRef]
- Shivamoggi, B.K. Spectral laws for the compressible isotropic turbulence. In Instability, Transition, and Turbulence; Springer: New York, NY, USA, 1992; pp. 524–534. [Google Scholar]
- Shivamoggi, B.K. Multi-fractal aspects of the fine-scale structure of temperature fluctuations in isotropic turbulence. Phys. A Stat. Mech. Its Appl. 1995, 221, 460–477. [Google Scholar] [CrossRef]
- Shivamoggi, B.K. Intermittency in the enstrophy cascade of two-dimensional fully developed turbulence: Universal features. Ann. Phys. 2008, 323, 444–460. [Google Scholar] [CrossRef]
- Shivamoggi, B. Compressible turbulence: Multi-fractal scaling in the transition to the dissipative regime. Phys. A Stat. Mech. Its Appl. 2011, 390, 1534–1538. [Google Scholar] [CrossRef]
- Sun, B. The temporal scaling laws of compressible turbulence. Mod. Phys. Lett. B 2016, 30, 1650297. [Google Scholar] [CrossRef]
- Sun, B. Scaling laws of compressible turbulence. Appl. Math. Mech. 2017, 38, 765–778. [Google Scholar] [CrossRef]
- Parent, B. Positivity-preserving high-resolution schemes for systems of conservation laws. J. Comput. Phys. 2012, 231, 173–189. [Google Scholar] [CrossRef]
- Gottlieb, S.; Shu, C.W. Total variation diminishing Runge-Kutta schemes. Math. Comput. Am. Math. Soc. 1998, 67, 73–85. [Google Scholar] [CrossRef]
- Godunov, S.K. A difference method for numerical calculation of discontinuous solutions of the equations of hydrodynamics. Mat. Sb. 1959, 89, 271–306. [Google Scholar]
- Grinstein, F.F.; Margolin, L.G.; Rider, W.J. Implicit Large Eddy Simulation: Computing Turbulent Fluid Dynamics; Cambridge University Press: New York, NY, USA, 2007. [Google Scholar]
- Denaro, F.M. What does finite volume-based implicit filtering really resolve in large-eddy simulations? J. Comput. Phys. 2011, 230, 3849–3883. [Google Scholar] [CrossRef]
- Liu, X.D.; Osher, S.; Chan, T. Weighted essentially non-oscillatory schemes. J. Comput. Phys. 1994, 115, 200–212. [Google Scholar] [CrossRef]
- Harten, A.; Engquist, B.; Osher, S.; Chakravarthy, S.R. Uniformly high order accurate essentially non-oscillatory schemes, III. In Upwind and High-Resolution Schemes; Springer: New York, NY, USA, 1987; pp. 218–290. [Google Scholar]
- Shu, C.W.; Osher, S. Efficient implementation of essentially non-oscillatory shock-capturing schemes. J. Comput. Phys. 1988, 77, 439–471. [Google Scholar] [CrossRef]
- Jiang, G.S.; Shu, C.W. Efficient implementation of weighted ENO schemes. J. Comput. Phys. 1996, 126, 202–228. [Google Scholar] [CrossRef]
- Henrick, A.K.; Aslam, T.D.; Powers, J.M. Mapped weighted essentially non-oscillatory schemes: Achieving optimal order near critical points. J. Comput. Phys. 2005, 207, 542–567. [Google Scholar] [CrossRef]
- Borges, R.; Carmona, M.; Costa, B.; Don, W.S. An improved weighted essentially non-oscillatory scheme for hyperbolic conservation laws. J. Comput. Phys. 2008, 227, 3191–3211. [Google Scholar] [CrossRef]
- Roe, P.L. Approximate Riemann solvers, parameter vectors, and difference schemes. J. Comput. Phys. 1981, 43, 357–372. [Google Scholar] [CrossRef]
- Harten, A. High resolution schemes for hyperbolic conservation laws. J. Comput. Phys. 1983, 49, 357–393. [Google Scholar] [CrossRef]
- Rusanov, V.V. The calculation of the interaction of non-stationary shock waves with barriers. Zhurnal Vychislitel’Noi Mat. Mat. Fiz. 1961, 1, 267–279. [Google Scholar]
- Lax, P.D. Weak solutions of nonlinear hyperbolic equations and their numerical computation. Commun. Pure Appl. Math. 1954, 7, 159–193. [Google Scholar] [CrossRef]
- Toro, E.F. Riemann Solvers and Numerical Methods for Fluid Dynamics: A Practical Introduction; Springer Science & Business Media: Berlin, Germany, 2013. [Google Scholar]
- Hyman, J.M.; Knapp, R.J.; Scovel, J.C. High order finite volume approximations of differential operators on nonuniform grids. Phys. D Nonlinear Phenom. 1992, 60, 112–138. [Google Scholar] [CrossRef]
- Fauconnier, D.; Bogey, C.; Dick, E. On the performance of relaxation filtering for large-eddy simulation. J. Turbul. 2013, 14, 22–49. [Google Scholar] [CrossRef]
- Bogey, C.; Bailly, C. Computation of a high Reynolds number jet and its radiated noise using large eddy simulation based on explicit filtering. Comput. Fluids 2006, 35, 1344–1358. [Google Scholar] [CrossRef]
- Bull, J.R.; Jameson, A. Explicit filtering and exact reconstruction of the sub-filter stresses in large eddy simulation. J. Comput. Phys. 2016, 306, 117–136. [Google Scholar] [CrossRef]
- San, O. A dynamic eddy-viscosity closure model for large eddy simulations of two-dimensional decaying turbulence. Int. J. Comput. Fluid Dyn. 2014, 28, 363–382. [Google Scholar] [CrossRef]
- Gropp, W.; Lusk, E.; Doss, N.; Skjellum, A. A high-performance, portable implementation of the MPI message passing interface standard. Parallel Comput. 1996, 22, 789–828. [Google Scholar] [CrossRef]
- Gropp, W.D.; Gropp, W.; Lusk, E.; Skjellum, A.; Lusk, E. Using MPI: Portable Parallel Programming with the Message-Passing Interface; MIT Press: Cambridge, MA, USA, 1999; Volume 1. [Google Scholar]
- Banerjee, A.; Andrews, M.J. 3D simulations to investigate initial condition effects on the growth of Rayleigh–Taylor mixing. Int. J. Heat Mass Transf. 2009, 52, 3906–3917. [Google Scholar] [CrossRef]
- Vladimirova, N.; Chertkov, M. Self-similarity and universality in Rayleigh–Taylor, Boussinesq turbulence. Phys. Fluids 2009, 21, 015102. [Google Scholar] [CrossRef]
- Xu, Z.; Duo-Wang, T. Direct Numerical Simulation of the Rayleigh- Taylor Instability with the Spectral Element Method. Chin. Phys. Lett. 2009, 26, 084703. [Google Scholar] [CrossRef]
- Liska, R.; Wendroff, B. Comparison of several difference schemes on 1D and 2D test problems for the Euler equations. Siam J. Sci. Comput. 2003, 25, 995–1017. [Google Scholar] [CrossRef]
- Stone, J.M.; Gardiner, T.A.; Teuben, P.; Hawley, J.F.; Simon, J.B. Athena: A new code for astrophysical MHD. Astrophys. Suppl. Ser. 2008, 178, 137–177. [Google Scholar] [CrossRef]
- Poinsot, T.J.; Lele, S.K. Boundary conditions for direct simulations of compressible viscous flows. J. Comput. Phys. 1992, 101, 104–129. [Google Scholar] [CrossRef]
- Youngs, D.L. Rayleigh–Taylor mixing: Direct numerical simulation and implicit large eddy simulation. Phys. Scr. 2017, 92, 074006. [Google Scholar] [CrossRef]
- San, O.; Kara, K. Evaluation of Riemann flux solvers for WENO reconstruction schemes: Kelvin–Helmholtz instability. Comput. Fluids 2015, 117, 24–41. [Google Scholar] [CrossRef]
- Lele, S.K. Compressibility effects on turbulence. Annu. Rev. Fluid Mech. 1994, 26, 211–254. [Google Scholar] [CrossRef]
- Kritsuk, A.G.; Norman, M.L.; Padoan, P.; Wagner, R. The statistics of supersonic isothermal turbulence. Astrophys. J. 2007, 665, 416. [Google Scholar] [CrossRef]
- Aluie, H. Scale decomposition in compressible turbulence. Phys. D Nonlinear Phenom. 2013, 247, 54–65. [Google Scholar] [CrossRef]
- San, O.; Maulik, R. Stratified Kelvin–Helmholtz turbulence of compressible shear flows. Nonlinear Process. Geophys. 2018, 25. [Google Scholar] [CrossRef]
- Press, W.H.; Teukolsky, S.A.; Vetterling, W.T.; Flannery, B.P. Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed.; Cambridge University Press: New York, NY, USA, 1992. [Google Scholar]
- Kida, S.; Orszag, S.A. Energy and spectral dynamics in forced compressible turbulence. J. Sci. Comput. 1990, 5, 85–125. [Google Scholar] [CrossRef]
- Bolgiano, R., Jr. Turbulent spectra in a stably stratified atmosphere. J. Geophys. Res. 1959, 64, 2226–2229. [Google Scholar] [CrossRef]
- Livescu, D.; Wei, T.; Petersen, M. Direct Numerical Simulations of Rayleigh-Taylor Instability; Conference Series; IOP Publishing: Bristol, UK, 2011; Volume 318, p. 082007. [Google Scholar]
- Youngs, D.L. Numerical simulation of turbulent mixing by Rayleigh-Taylor instability. Phys. D Nonlinear Phenom. 1984, 12, 32–44. [Google Scholar] [CrossRef]
- Ramaprabhu, P.; Dimonte, G.; Andrews, M. A numerical study of the influence of initial perturbations on the turbulent Rayleigh–Taylor instability. J. Fluid Mech. 2005, 536, 285–319. [Google Scholar] [CrossRef]
- Shadloo, M.; Zainali, A.; Yildiz, M. Simulation of single mode Rayleigh–Taylor instability by SPH method. Comput. Mech. 2013, 51, 699–715. [Google Scholar] [CrossRef]
- Lee, H.G.; Kim, K.; Kim, J. On the long time simulation of the Rayleigh–Taylor instability. Int. J. Numer. Methods Eng. 2011, 85, 1633–1647. [Google Scholar] [CrossRef]
- Livescu, D.; Ristorcelli, J.; Petersen, M.; Gore, R. New phenomena in variable-density Rayleigh–Taylor turbulence. Phys. Scr. 2010, 2010, 014015. [Google Scholar] [CrossRef]
- Shi, J.; Zhang, Y.T.; Shu, C.W. Resolution of high order WENO schemes for complicated flow structures. J. Comput. Phys. 2003, 186, 690–696. [Google Scholar] [CrossRef]
- Fu, L.; Hu, X.Y.; Adams, N.A. A family of high-order targeted ENO schemes for compressible-fluid simulations. J. Comput. Phys. 2016, 305, 333–359. [Google Scholar] [CrossRef]
- Latini, M.; Schilling, O.; Don, W.S. Effects of WENO flux reconstruction order and spatial resolution on reshocked two-dimensional Richtmyer–Meshkov instability. J. Comput. Phys. 2007, 221, 805–836. [Google Scholar] [CrossRef]
- Tritschler, V.; Hu, X.; Hickel, S.; Adams, N. Numerical simulation of a Richtmyer–Meshkov instability with an adaptive central-upwind sixth-order WENO scheme. Phys. Scr. 2013, 2013, 014016. [Google Scholar] [CrossRef]























© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rahman, S.M.; San, O. A Relaxation Filtering Approach for Two-Dimensional Rayleigh–Taylor Instability-Induced Flows. Fluids 2019, 4, 78. https://doi.org/10.3390/fluids4020078
Rahman SM, San O. A Relaxation Filtering Approach for Two-Dimensional Rayleigh–Taylor Instability-Induced Flows. Fluids. 2019; 4(2):78. https://doi.org/10.3390/fluids4020078
Chicago/Turabian StyleRahman, Sk. Mashfiqur, and Omer San. 2019. "A Relaxation Filtering Approach for Two-Dimensional Rayleigh–Taylor Instability-Induced Flows" Fluids 4, no. 2: 78. https://doi.org/10.3390/fluids4020078
APA StyleRahman, S. M., & San, O. (2019). A Relaxation Filtering Approach for Two-Dimensional Rayleigh–Taylor Instability-Induced Flows. Fluids, 4(2), 78. https://doi.org/10.3390/fluids4020078





