Computational Analysis of Lung and Isolated Airway Bifurcations under Mechanical Ventilation and Normal Breathing
Abstract
:1. Introduction
2. Methods
2.1. Construction of Tracheobronchial (TB) Model
2.2. Continuous and Discrete Phase Transport Equations
2.3. Flow Rates and Boundary Conditions
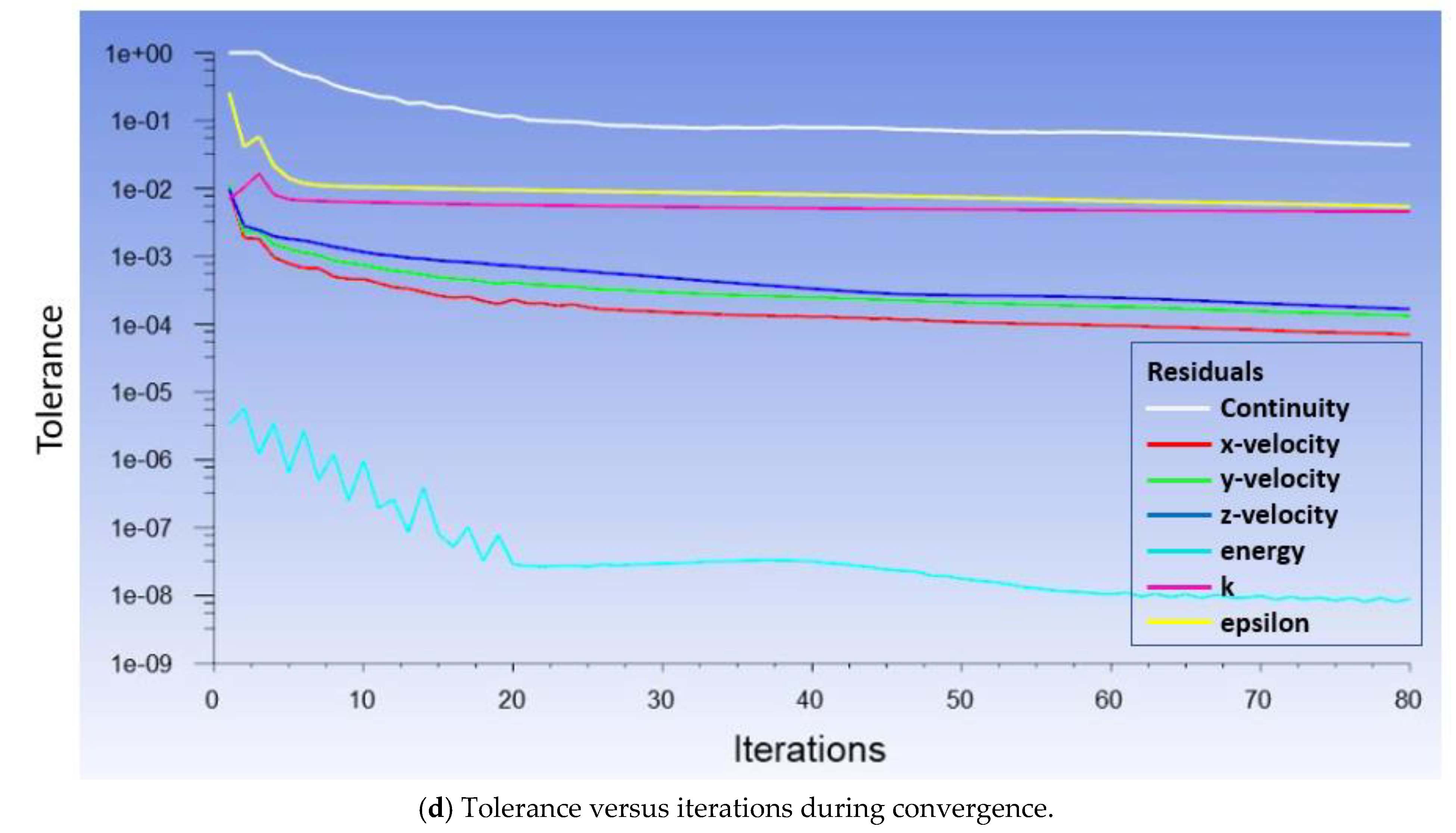
2.4. Computational Simulations
3. Results
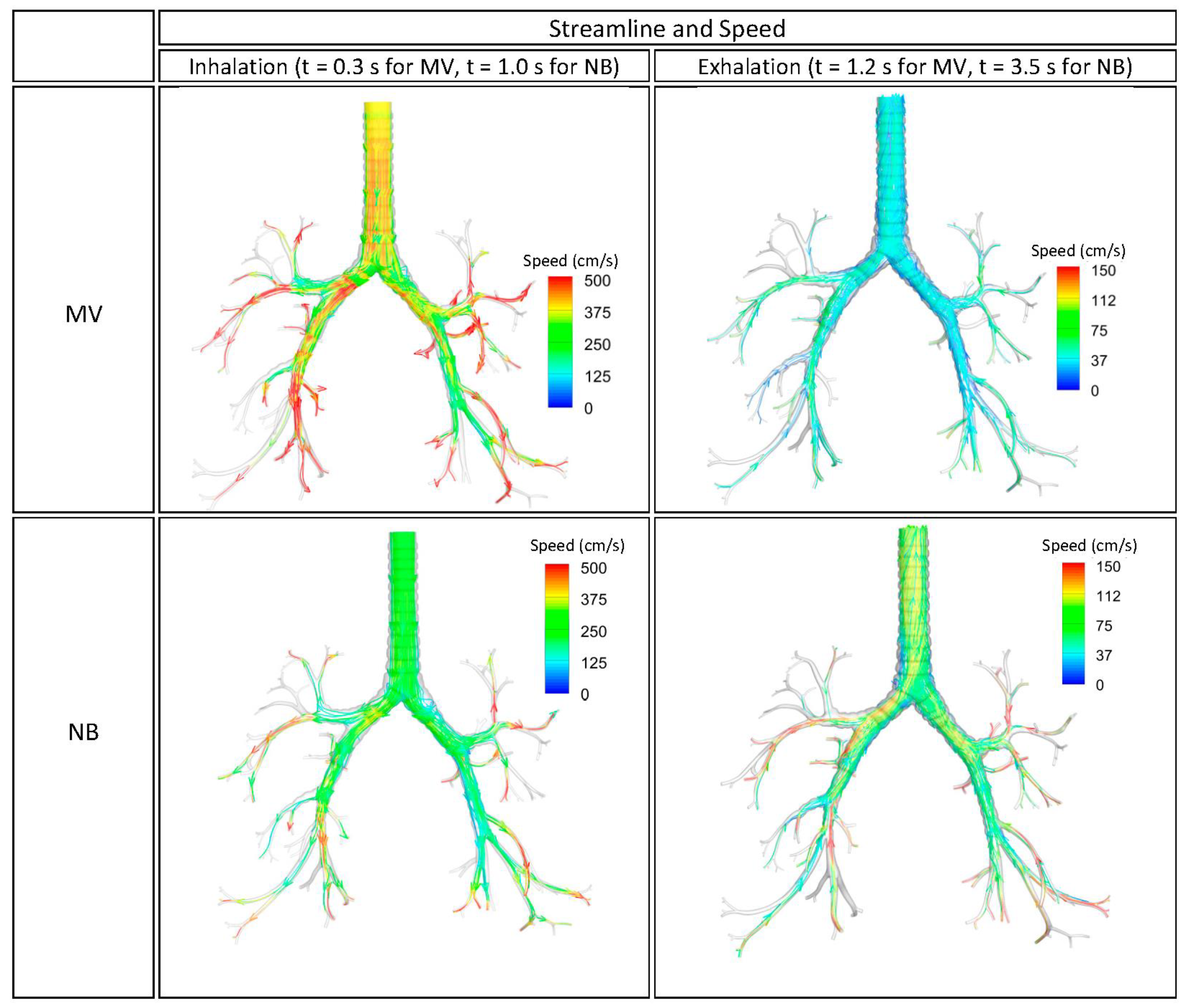
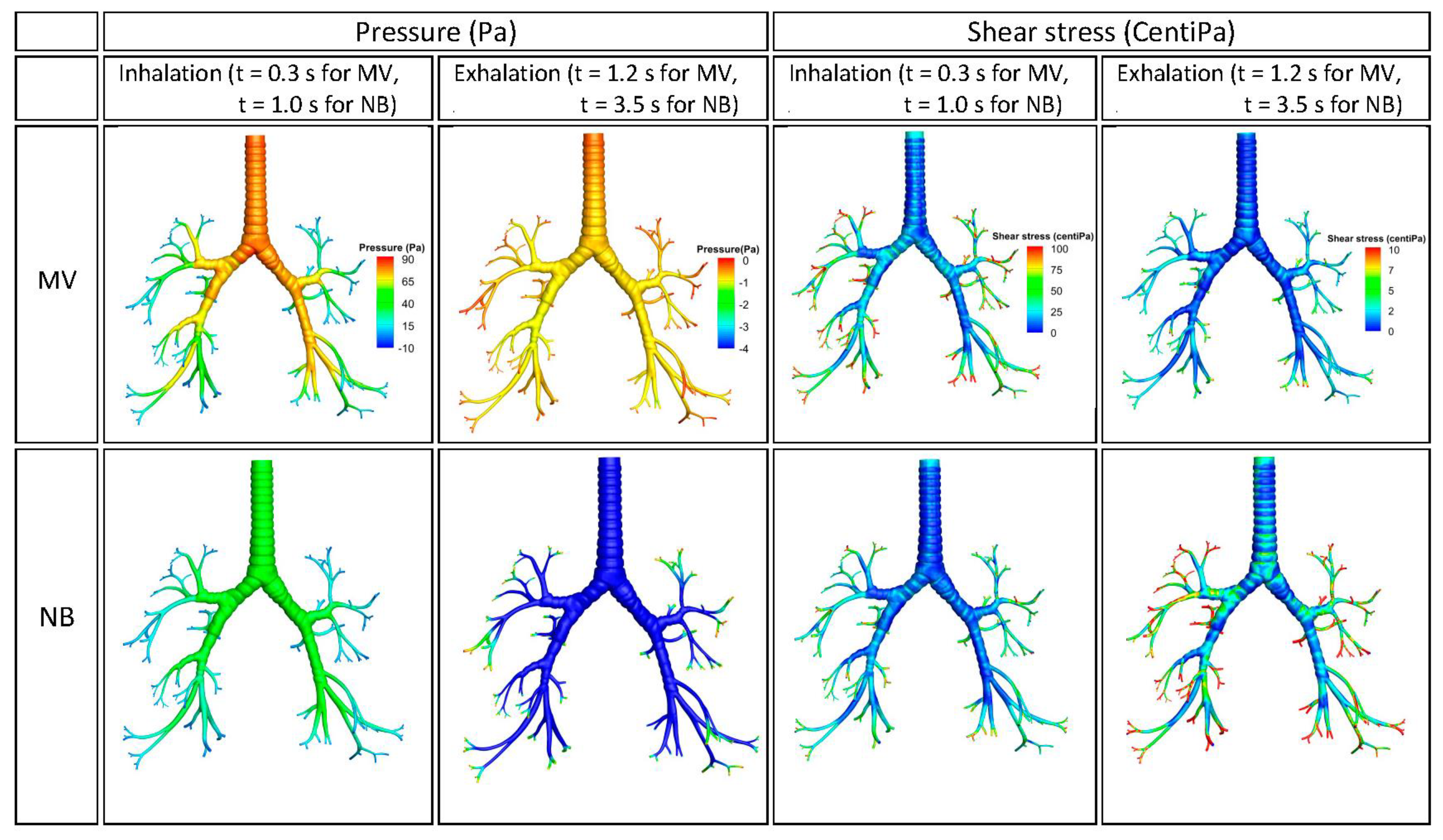
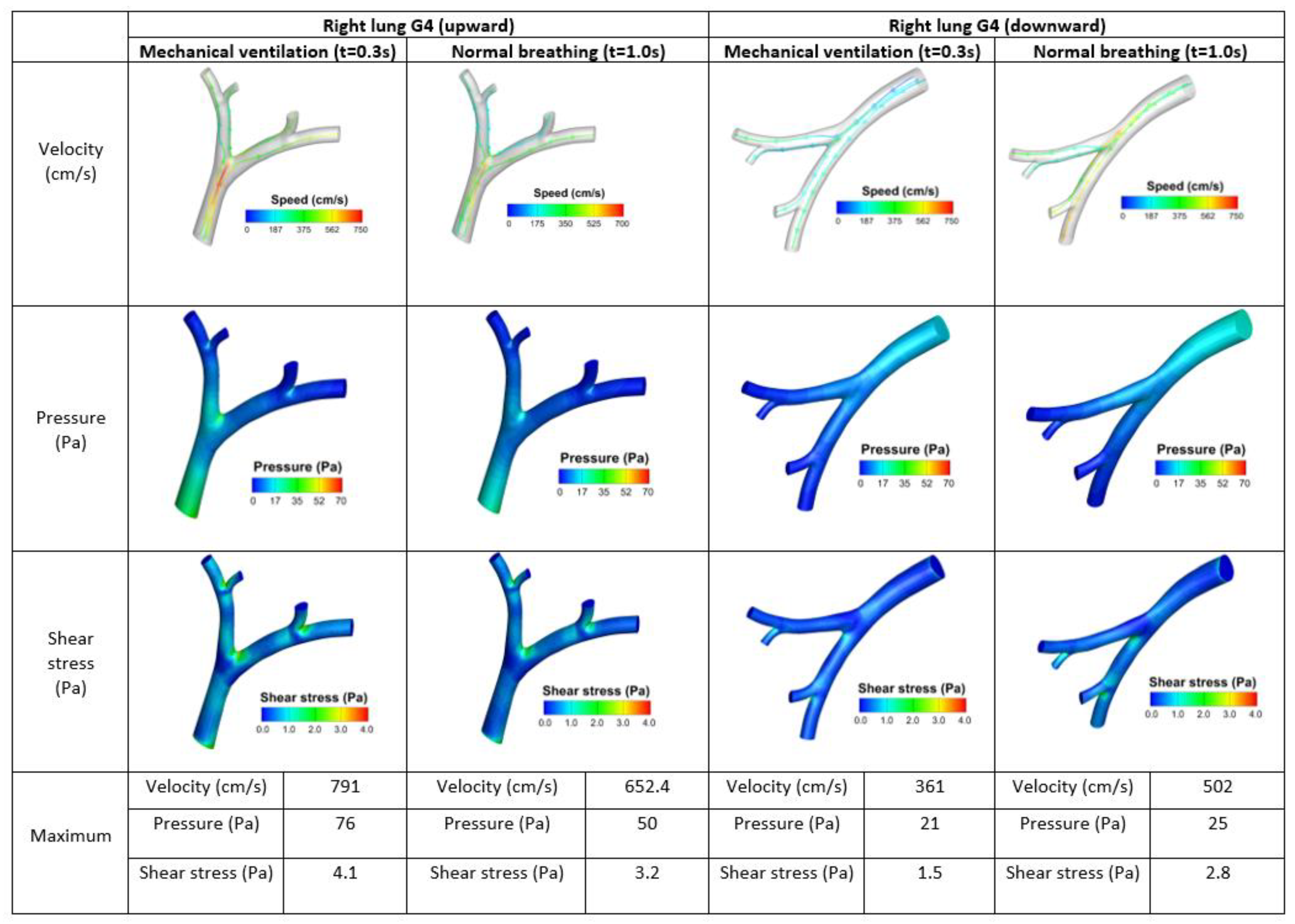
3.1. Streamline of Velocity, Contour of Pressure, and Contour Wall Shear Stress
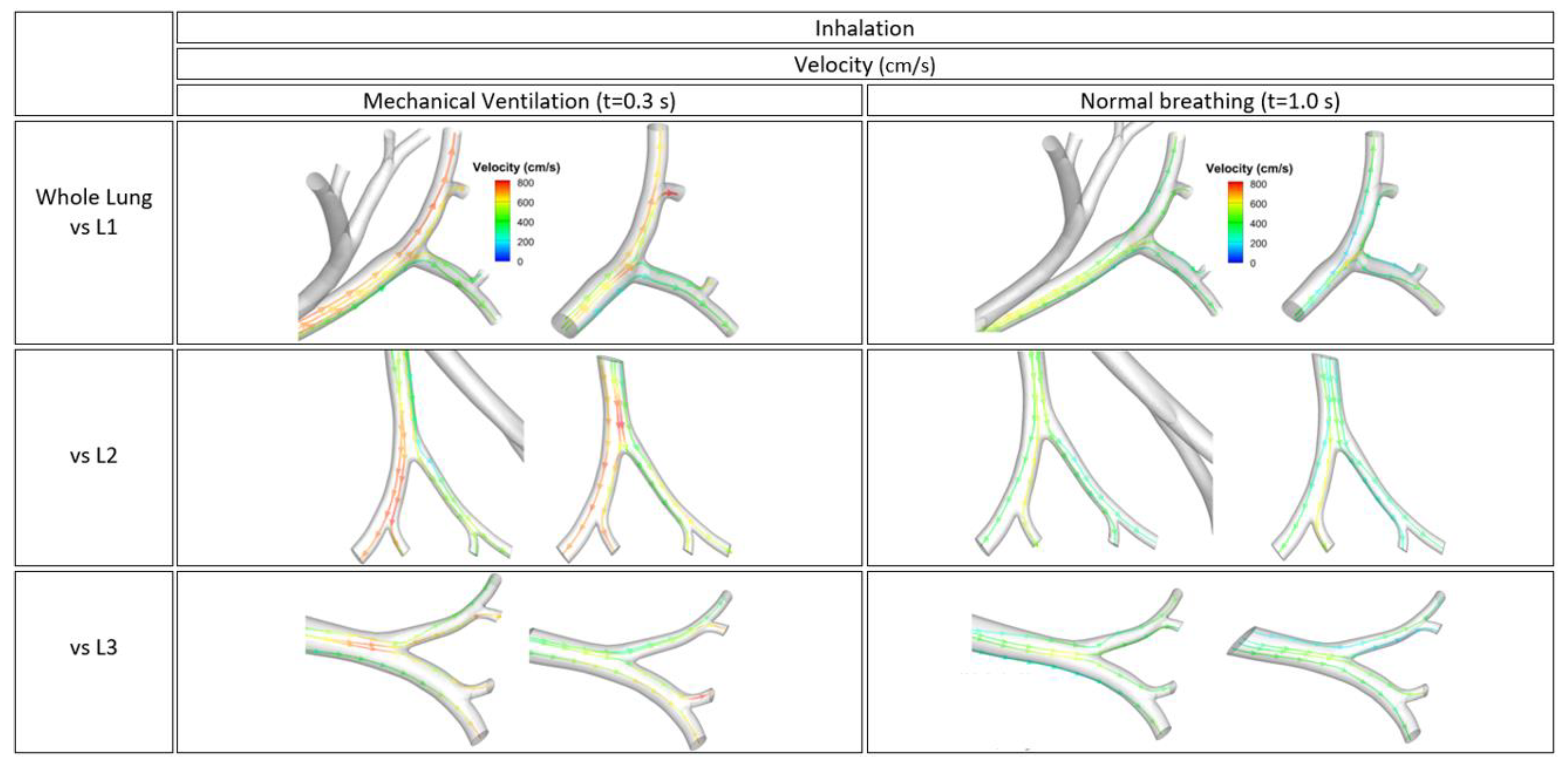
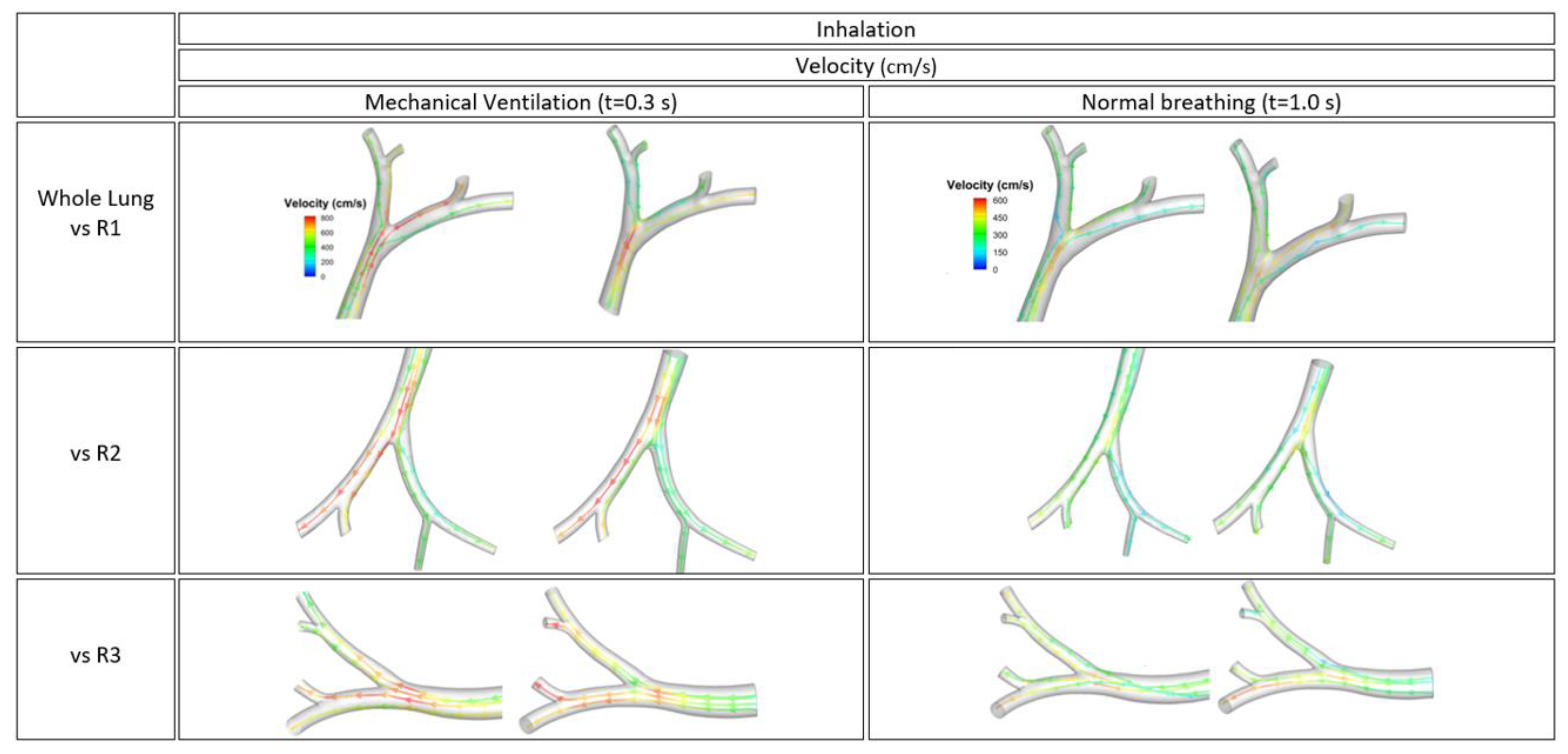
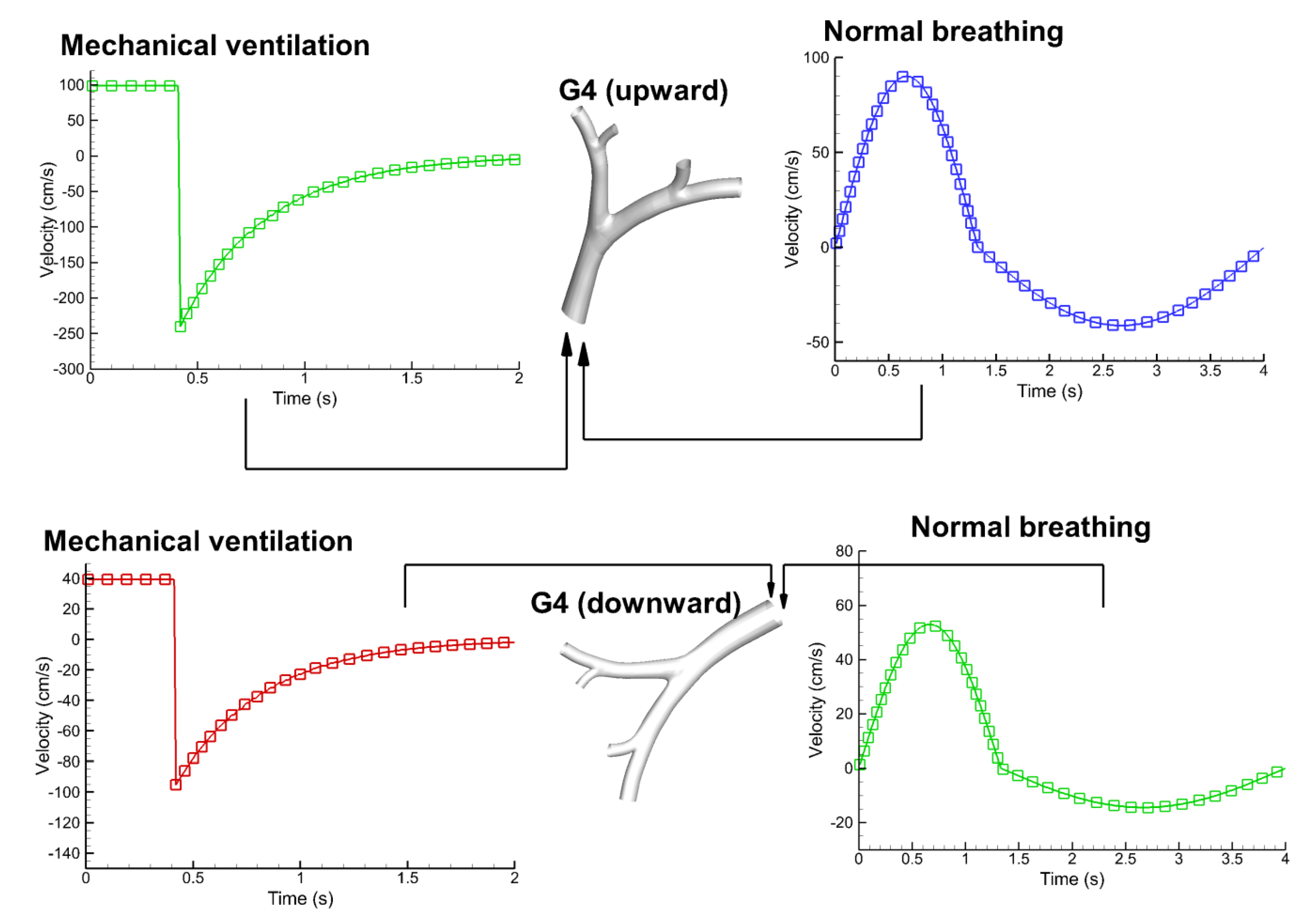
3.2. Velocity Field
3.3. Turbulent Vortices
3.4. Flow Characteristics of Local Bifurcations
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kim, J.; Xi, J. Dynamic growth and deposition of hygroscopic aerosols in the nasal airway of a 5-year-old child. Int. J. Numer. Meth. Eng. 2013, 29, 17–39. [Google Scholar] [CrossRef] [PubMed]
- Si, X.; Xi, J.; Kim, J.; Zhou, Y.; Zhong, H. Modeling of release position and ventilation effects on olfactory aerosol drug delivery. Respir. Physiol. Neurobiol. 2013, 186, 22–32. [Google Scholar] [CrossRef] [PubMed]
- Xi, J.; Berlinski, A.; Zhou, Y.; Greenberg, B.; Ou, X. Breathing resistance and ultrafine particle deposition in nasal-laryngeal airways of a newborn, an infant, a child, and an adult. Ann. Biomed. Eng. 2012, 40, 2579–2595. [Google Scholar] [CrossRef] [PubMed]
- Zhao, K.; Scherer, P.W.; Hajiloo, S.A.; Dalton, P. Effect of anatomy on human nasal air flow and odorant transport patterns: Implications for olfaction. Chem. Senses 2004, 29, 365–379. [Google Scholar] [CrossRef]
- Heyder, J.; Gebhart, J.; Rudolf, G.; Schiller, C.F.; Stahlhofen, W. Deposition of particles in the human respiratory tract in the size range of 0.005–15 μm. J. Aerosol Sci. 1986, 17, 811–825. [Google Scholar] [CrossRef]
- Jaques, P.A.; Kim, C. Measurement of total lung deposition of inhaled ultrafine particles in healthy men and women. Inhal. Toxicol. 2000, 12, 715–731. [Google Scholar]
- Kim, C.; Jaques, P. Analysis of total respiratory deposition of inhaled ultrafine particles in adult subjects at various breathing patterns. Aerosol Sci. Technol. 2004, 38, 525–540. [Google Scholar] [CrossRef] [Green Version]
- Proetz, A. Air currents in the upper respiratory tract and their clinical importance. Ann. Otol. Rhinol. Laryngol. 1951, 60, 439–467. [Google Scholar] [CrossRef]
- Schroter, R.C.; Sudlow, M. Flow patterns in models of the human bronchial airways. Respir. Physiol. 1969, 7, 341–355. [Google Scholar] [CrossRef]
- Morawska, L.; Hofmann, W.; Hitchins-Loveday, J.; Swanson, C.; Mengersen, K. Experimental study of the deposition of combustion aerosols in the human respiratory tract. J. Aerosol Sci. 2005, 36, 939–957. [Google Scholar] [CrossRef] [Green Version]
- Stahlhofen, W.; Rudolf, G.; James, A.C. Intercomparison of experimental regional aerosol deposition data. J. Aerosol Med. 1989, 2, 285–308. [Google Scholar] [CrossRef]
- Chang, H.; El, O. A model study of flow dynamics in human central airways. Respir. Physiol. 1982, 49, 75–95. [Google Scholar] [CrossRef]
- Horsfield, K.; Gladys, D.; Olson, D.; Finlay, G.; Cumming, G. Models of the human bronchial tree. J. Appl. Physiol. 1971, 31, 207–217. [Google Scholar] [CrossRef]
- Van, C.; Hirsch, C.; Paiva, M. Anatomically based three-dimensional model of airways to simulate flow and particle transport using computational fluid dynamics. J. Appl. Physiol. 2005, 98, 970–980. [Google Scholar]
- Heenan, A.F.; Matida, E.; Pollard, A.; Finlay, W.H. Experimental measurements and computational modeling of the flow field in an idealized human oropharynx. Exp. Fluids 2003, 35, 70–84. [Google Scholar] [CrossRef]
- Xi, J.; Kim, J.; Si, X. Transport and absorption of anesthetic vapors in a mouth-lung model extending to G9 bronchioles. Int. J. Anesthesiol. Res. 2013, 1, 6–19. [Google Scholar] [CrossRef]
- Isabey, D.; Chang, H. Steady and unsteady pressure-flow relationships in central airways. J. Appl. Physiol. 1981, 51, 1338–1348. [Google Scholar] [CrossRef]
- Yeh, H.; Schum, G. Models of human lung airways and their application to inhaled particle deposition. J. Math. Biol. 1980, 42, 461–480. [Google Scholar] [CrossRef]
- Elad, D.; Wolf, M.; Keck, T. Air-conditioning in the human nasal cavity. Respir. Physiol. Neurobiol. 2008, 163, 121–127. [Google Scholar] [CrossRef]
- Hörschler, I.; Meinke, M.; Schröder, W. Numerical simulation of the flow field in a model of the nasal cavity. J. Comput. Fluids 2003, 32, 39–45. [Google Scholar] [CrossRef]
- Hammersley, J.; Olson, D. Physical models of the smaller pulmonary airways. J. Appl. Physiol. 1992, 72, 2402–2414. [Google Scholar] [CrossRef]
- Wilcox, D. Turbulence Modeling for CFD, 2nd ed.; DCW Industries: La Canada, CA, USA, 1998. [Google Scholar]
- Xi, J.; Longest, P. Evaluation of a drift flux model for simulating submicrometer aerosol dynamics in human upper tracheobronchial airways. Ann. Biomed. Engin. 2007, 36, 1714–1734. [Google Scholar] [CrossRef]
- Keyhani, K.; Scherer, P.; Mozell, M. Numerical simulation of airflow in the human nasal cavity. J. Biomed. Engin. 1995, 117, 429–441. [Google Scholar] [CrossRef]
- Keyhani, K.; Scherer, P.; Mozell, M. A numerical model of nasal odorant transport for the analysis of human olfaction. J. Theor Biol. 1997, 186, 279–301. [Google Scholar] [CrossRef]
- Lindemann, J.; Leiacker, R.; Rettinger, G.; Keck, T. Nasal mucosal temperature during respiration. Clin. Otolaryngol. 2002, 27, 135–139. [Google Scholar] [CrossRef]
- Lindemann, J.; Keck, T.; Wiesmiller, K.; Sander, B.; Brambs, H.; Rettinger, G.; Pless, D. A numerical simulation of intranasal air temperature during inspiration. Laryngoscope 2004, 114, 1037–1041. [Google Scholar] [CrossRef]
- Lindemann, J.; Keck, T.; Wiesmiller, K.; Rettinger, G.; Brambs, H.; Pless, D. Numerical simulation of intranasal air flow and temperature after resection of the turbinates. Rhinology 2005, 43, 24–28. [Google Scholar]
- Heistracher, T.; Hofmann, W. Physiologically realistic models of bronchial airway bifurcations. J. Aerosol Sci. 1995, 26, 497–509. [Google Scholar] [CrossRef]
- Cohen, B.; Sussman, R.; Lippmann, M. Ultrafine particle deposition in a human tracheobronchial cast. Aerosol Sci. Technol. 1990, 12, 1082–1093. [Google Scholar] [CrossRef]
- Jeong, J.; Hussain, F. On the identification of a vortex. J. Fluid Mech. 1995, 285, 69–94. [Google Scholar] [CrossRef]
- Lee, J.; Yang, N.; Kim, S.; Chung, S. Unsteady flow characteristics through a human nasal airway. Respir. Physiol. Neurobiol. 2010, 172, 136–146. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Matida, A.; Gu, J.; Johnson, M. Numerical simulation of aerosol deposition in a 3-D human nasal cavity using RANS, RANS/EIM, and LES. J. Aerosol Sci. 2007, 38, 683–700. [Google Scholar] [CrossRef]
- Perzl, M.; Schultz, H.; Parezke, H.; Englmeier, K.; Heyder, J. Reconstruction of the lung geometry for the simulation of aerosol transport. J. Aerosol Med. 1996, 9, 409–418. [Google Scholar] [CrossRef]
- Vial, L.; Perchet, D.; Fodil, R.; Caillibotte, G.; Fetita, C.; Preteux, F. Airflow modeling of steady inspiration in two realistic proximal airway trees reconstructed from human thoracic tomodensitometric images. Comput. Methods Biomech. Biomed. Eng. 2005, 8, 267–277. [Google Scholar] [CrossRef]
- Xi, J.; Longest, P.; Martonen, T. Effects of the laryngeal jet on nano-and microparticle transport and deposition in an approximate model of the upper tracheobronchial airways. J. Appl. Physiol. 2008, 104, 1761–1777. [Google Scholar] [CrossRef]
- Kleinstreuer, C.; Zhang, Z. Airflow and particle transport in the human respiratory system. J. Fluid Mech. 2010, 42, 301–334. [Google Scholar] [CrossRef]
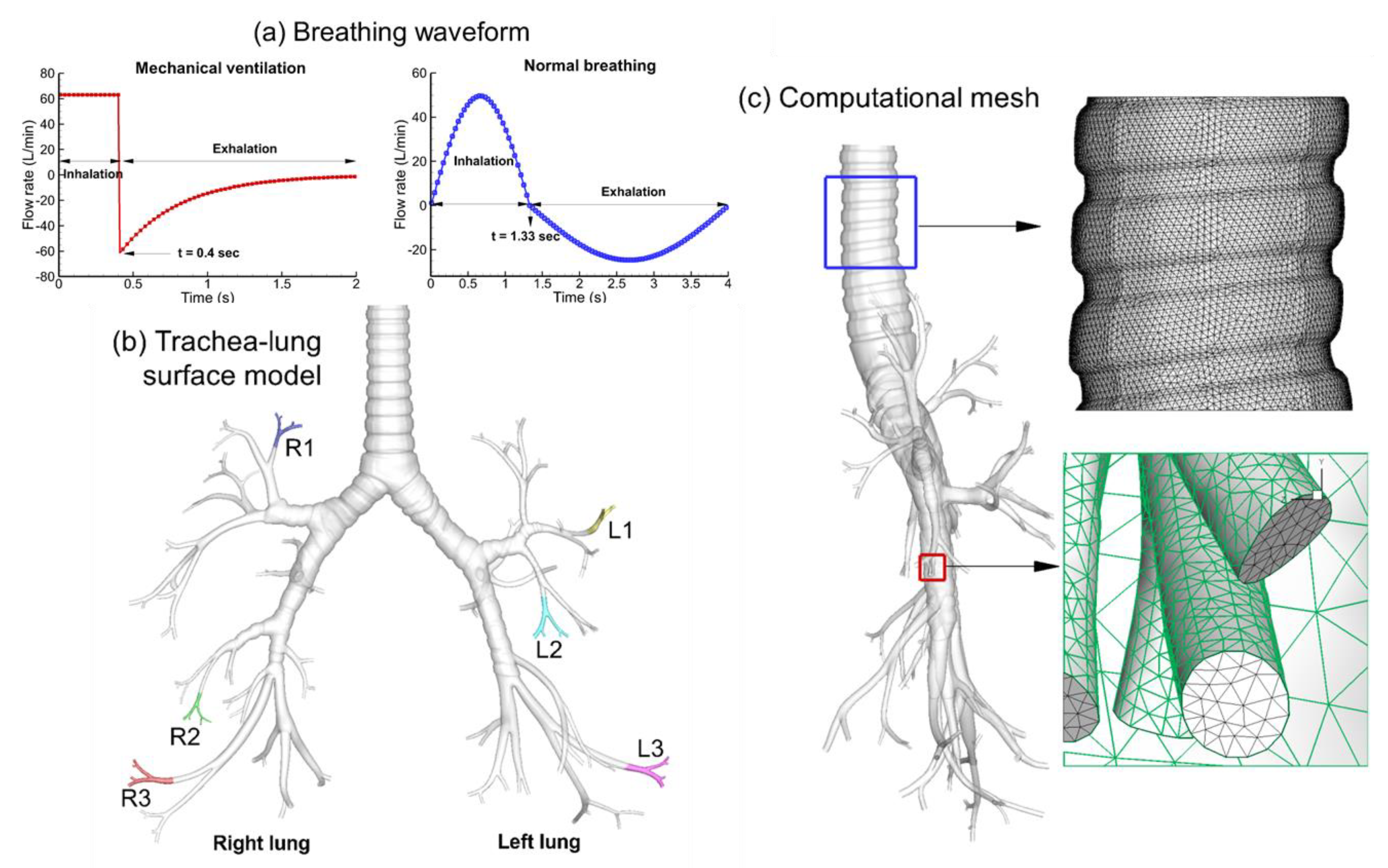
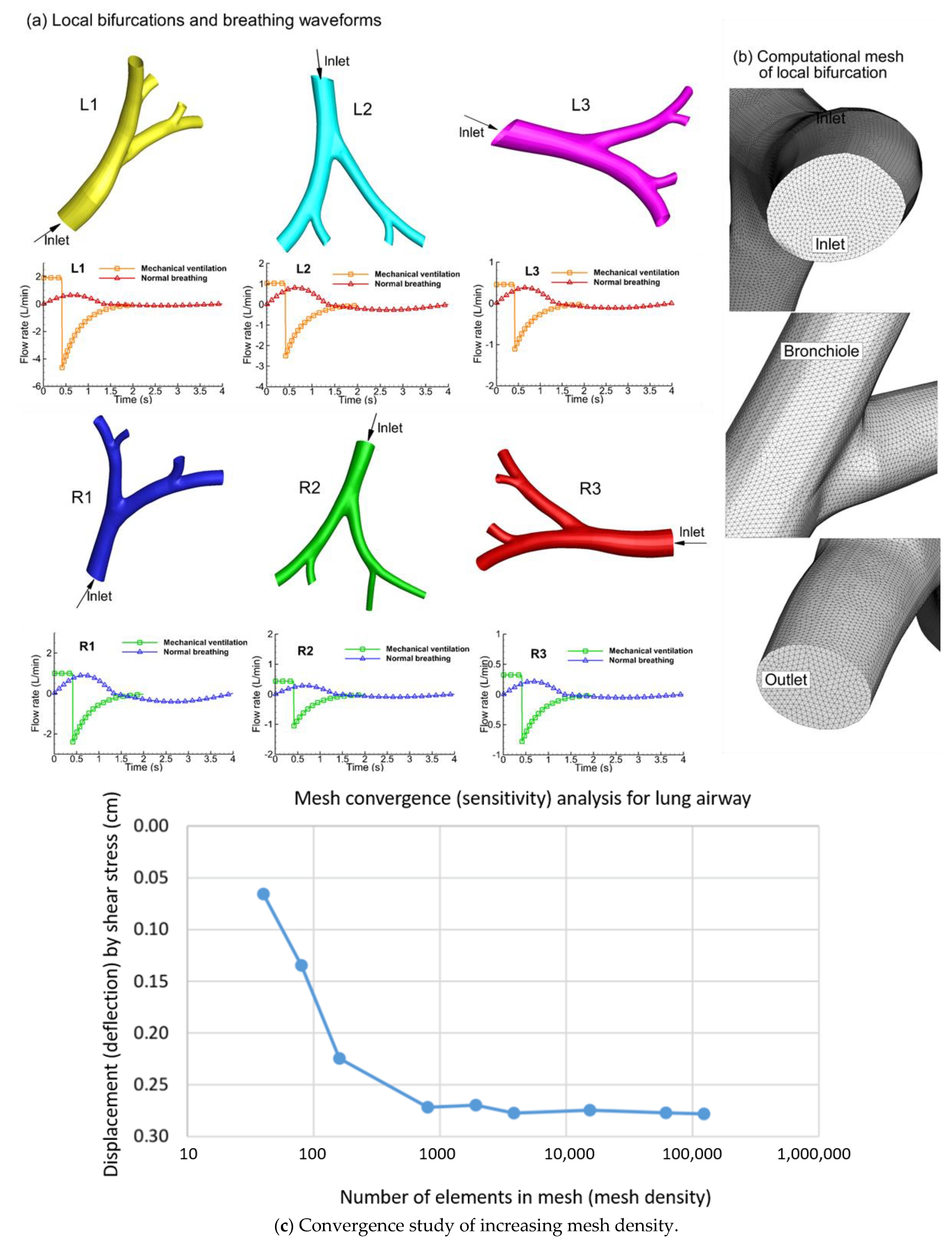






| Tracheobronchial Whole Lung (G9) | ||
|---|---|---|
| Tidal volume (mL) | Ventilation | 420 |
| Normal | 700 | |
| Breathing frequency (Hz) | Ventilation | 0.5 |
| Normal | 0.25 | |
| I:E ratio | Ventilation | 1:4 |
| Normal | 1:2 | |
| Inhalation (s) | Ventilation | 0.4 |
| Normal | 1.33 | |
| Mechanical Ventilation(Inhalation, t = 0.3 s) | Pressure Ratio, Pn(α) | Shear Stress Ratio, S(β) | ||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| P1 | P2 | P3 | P4 | P5 | P6 | P7 | P8 | P9 | S1 | S2 | S3 | S4 | S5 | S6 | S7 | S8 | S9 | |
| Whole lung vs. L1 | 0.96 | 1.06 | 1.03 | 1 | 1.03 | 0.92 | 1 | 1.09 | 1.04 | 1.03 | 1.02 | 0.88 | 1.05 | 0.94 | 0.96 | 1.02 | 0.95 | 1.02 |
| Whole lung vs. L2 | 1.05 | 0.98 | 1.07 | 1 | 0.99 | 0.93 | 0.97 | 0.97 | 1.02 | 1.02 | 0.98 | 0.97 | 0.96 | 0.99 | 1.02 | 1.04 | 1.01 | 1.02 |
| Whole lung vs. L3 | 1.01 | 1.02 | 1.04 | 1.01 | 1.11 | 1.03 | 0.99 | 1.02 | 1.02 | 1.02 | 1.06 | 0.94 | 1.01 | 1.01 | 1.05 | 1.03 | 1.05 | 1.01 |
| Normal Breathing (inhalation, t = 0.9 s) | Pressure ratio, P(α) | Shear stress ratio, S(β) | ||||||||||||||||
| P1 | P2 | P3 | P4 | P5 | P6 | P7 | P8 | P9 | S1 | S2 | S3 | S4 | S5 | S6 | S7 | S8 | S9 | |
| Whole lung vs. R1 | 1.01 | 0.97 | 1.02 | 1.11 | 0.99 | 1.08 | 0.99 | 0.99 | 1.01 | 0.97 | 1.02 | 1.05 | 1.01 | 1.03 | 1 | 0.98 | 1.02 | 1.02 |
| Whole lung vs. R2 | 1.01 | 1.01 | 0.99 | 1 | 1.04 | 1.01 | 0.99 | 1.01 | 1.02 | 1.04 | 1 | 0.98 | 0.99 | 0.93 | 1.01 | 0.99 | 0.98 | 0.95 |
| Whole lung vs. R3 | 0.99 | 1 | 0.98 | 0.99 | 0.88 | 0.98 | 1 | 0.98 | 1.02 | 1.13 | 0.96 | 0.99 | 0.97 | 1.03 | 1.04 | 1.04 | 1.05 | 1.08 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, J.; Pidaparti, R.M. Computational Analysis of Lung and Isolated Airway Bifurcations under Mechanical Ventilation and Normal Breathing. Fluids 2021, 6, 388. https://doi.org/10.3390/fluids6110388
Kim J, Pidaparti RM. Computational Analysis of Lung and Isolated Airway Bifurcations under Mechanical Ventilation and Normal Breathing. Fluids. 2021; 6(11):388. https://doi.org/10.3390/fluids6110388
Chicago/Turabian StyleKim, Jongwon, and Ramana M. Pidaparti. 2021. "Computational Analysis of Lung and Isolated Airway Bifurcations under Mechanical Ventilation and Normal Breathing" Fluids 6, no. 11: 388. https://doi.org/10.3390/fluids6110388
APA StyleKim, J., & Pidaparti, R. M. (2021). Computational Analysis of Lung and Isolated Airway Bifurcations under Mechanical Ventilation and Normal Breathing. Fluids, 6(11), 388. https://doi.org/10.3390/fluids6110388






