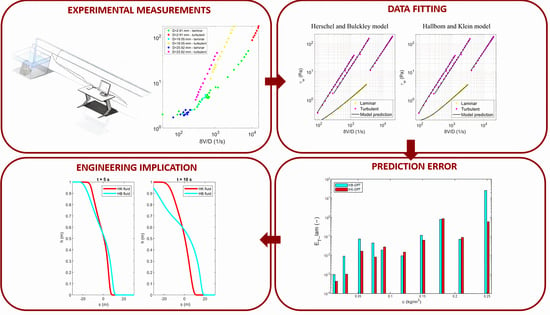
Rheological Characterization of Non-Newtonian Mixtures by Pressure Pipe Tests
Abstract
:1. Introduction
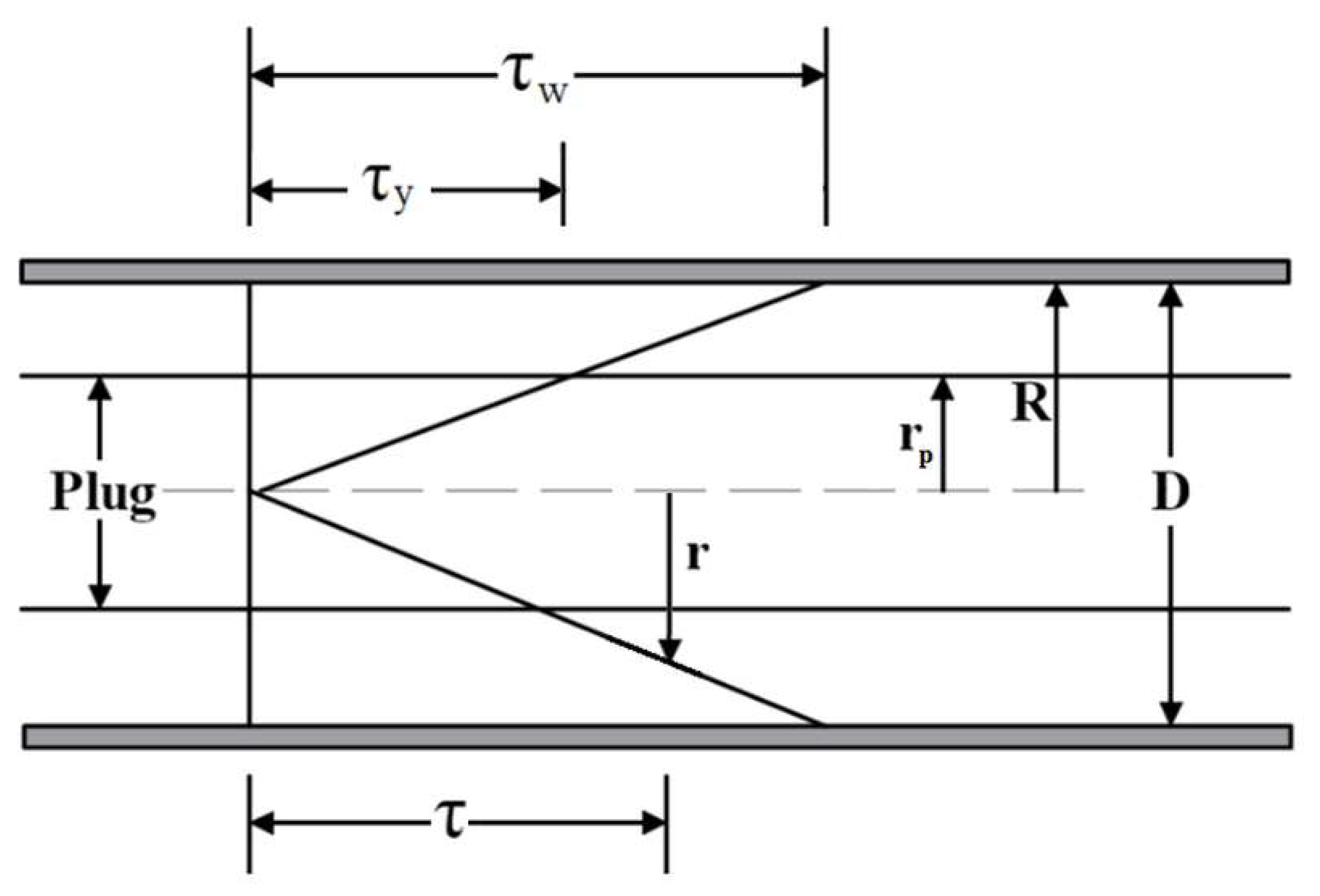
2. Friction Flow Model in Laminar Flow Conditions
2.1. HB Fluid
2.2. HK Fluid
3. Friction Flow Model in Turbulent Flow Conditions
4. Experiments
5. Optimization Process
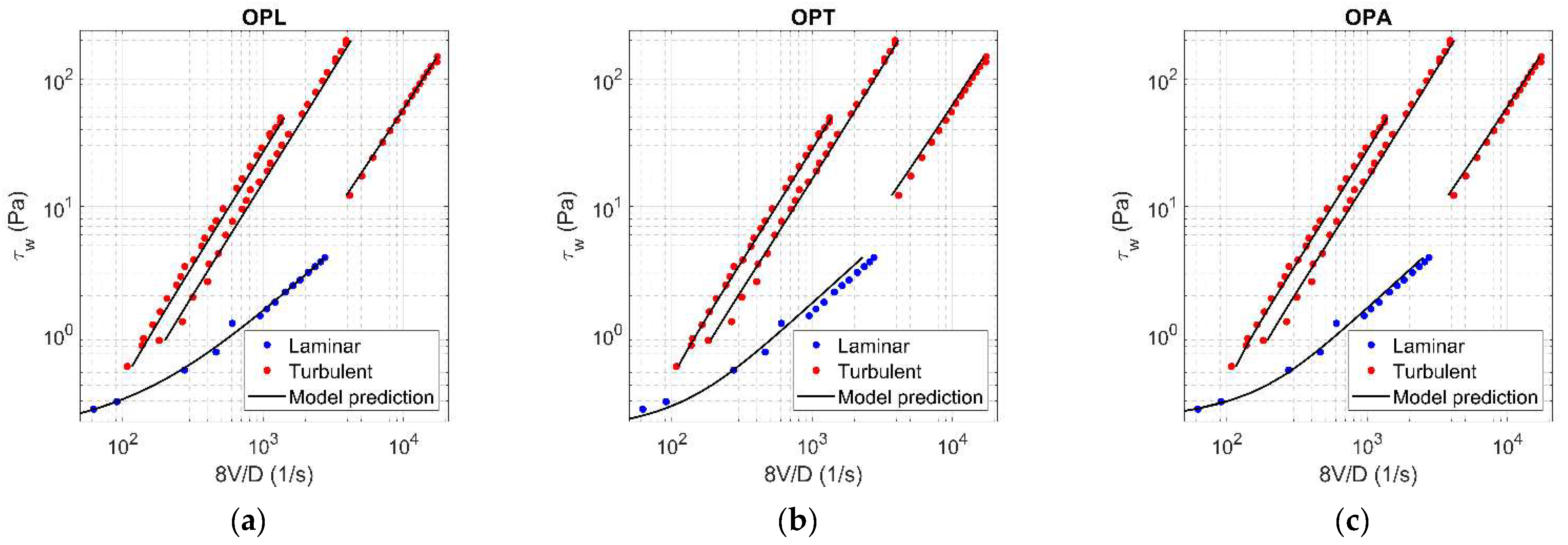
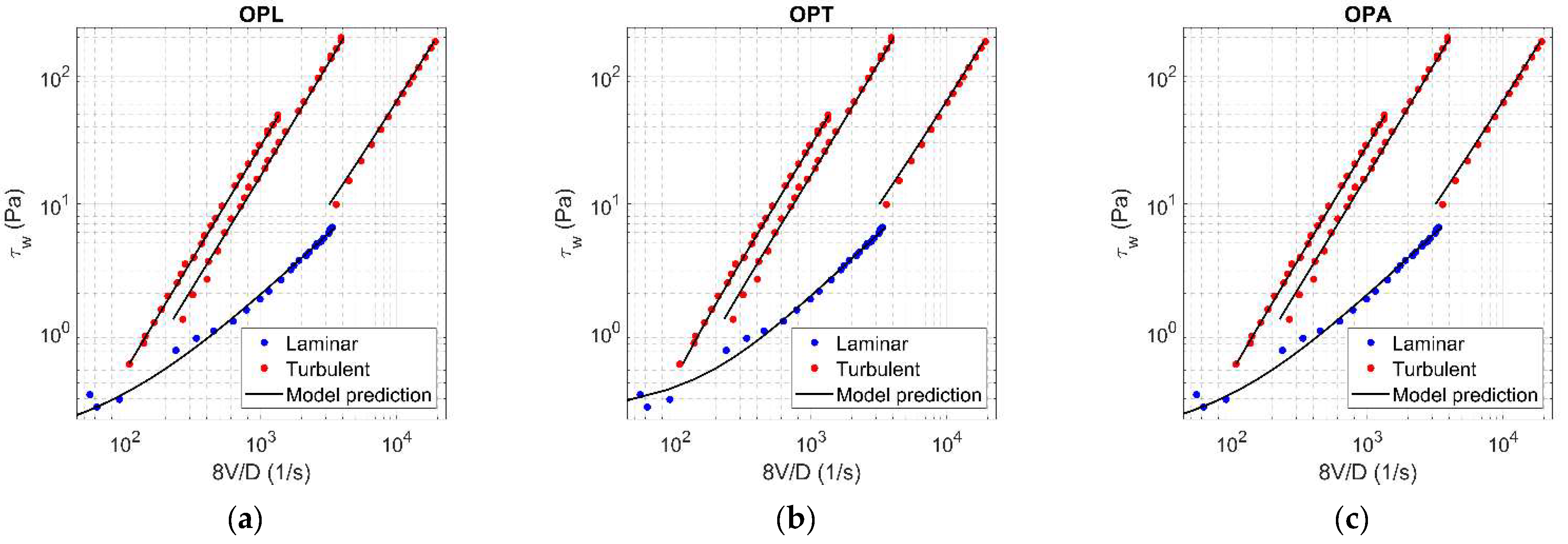
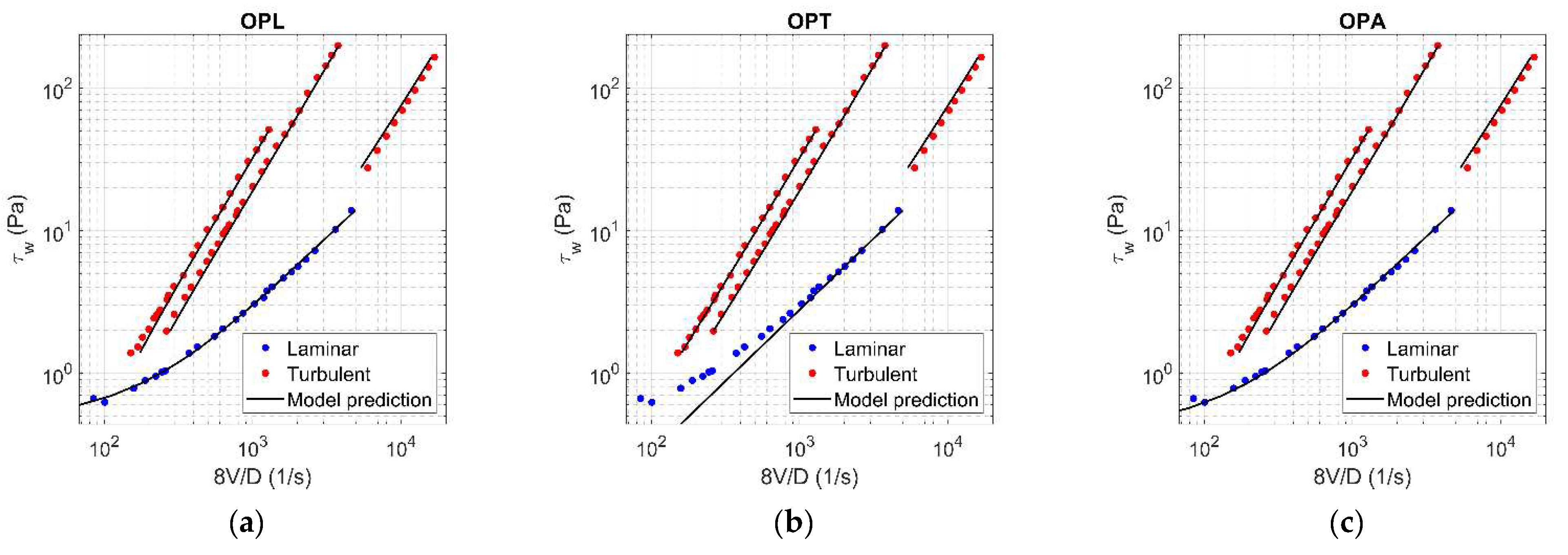
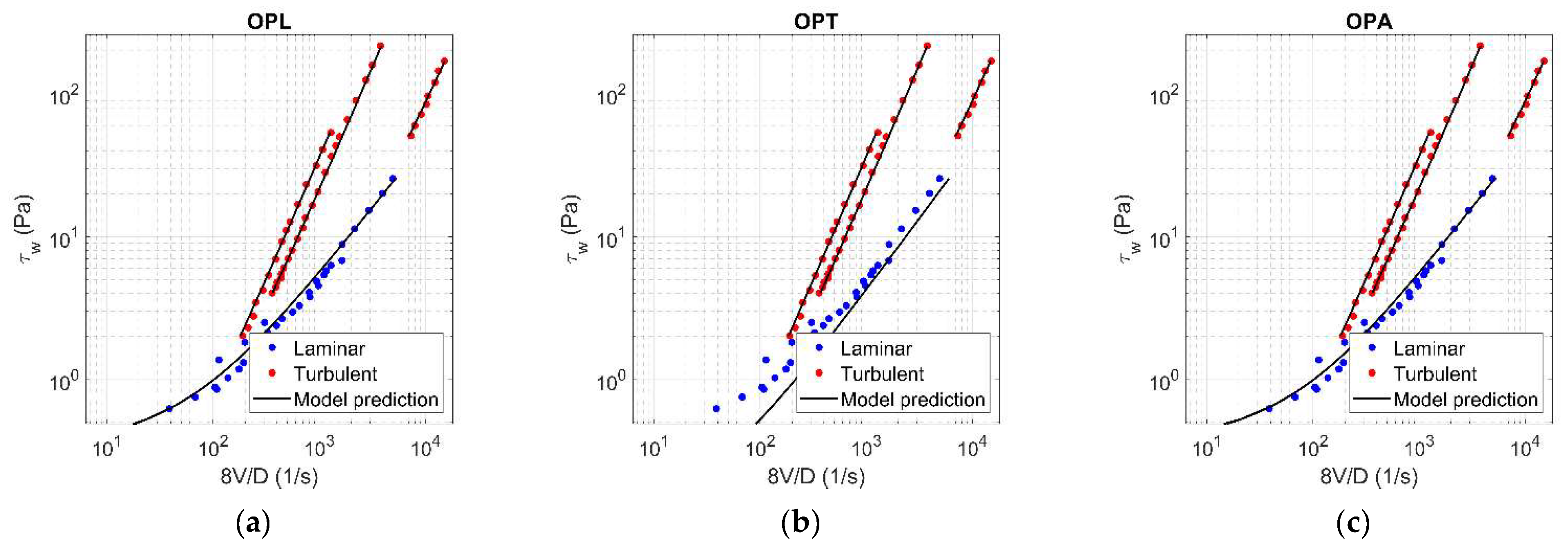
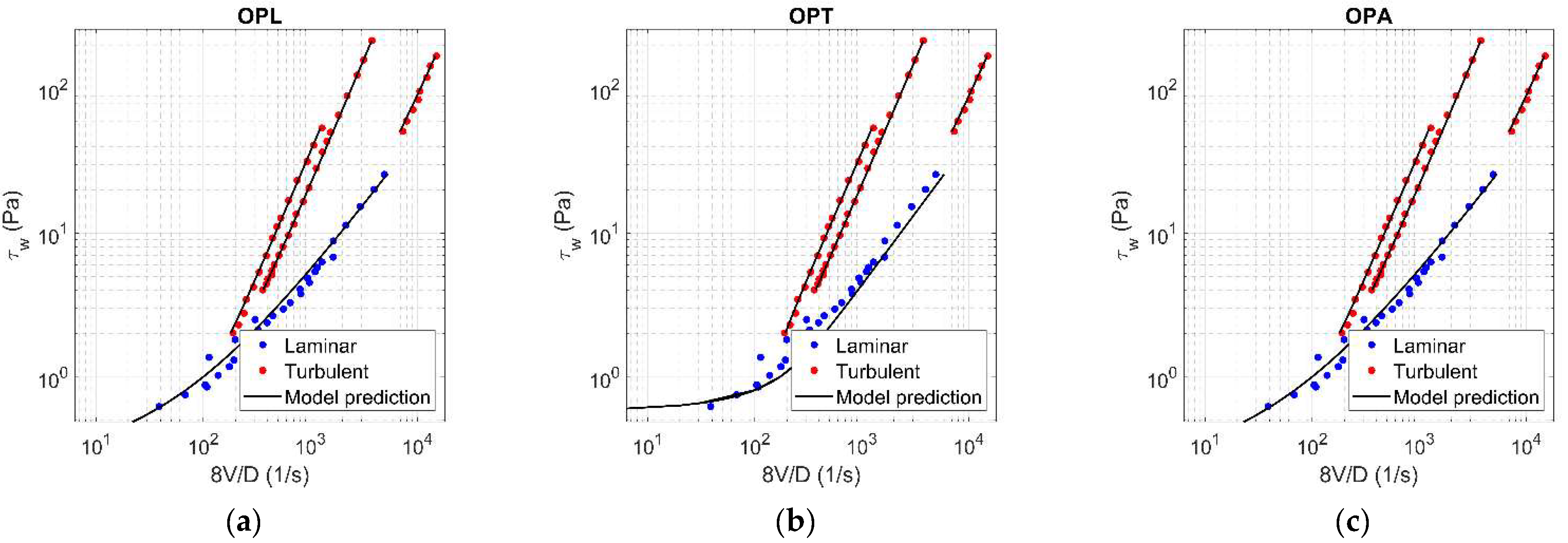
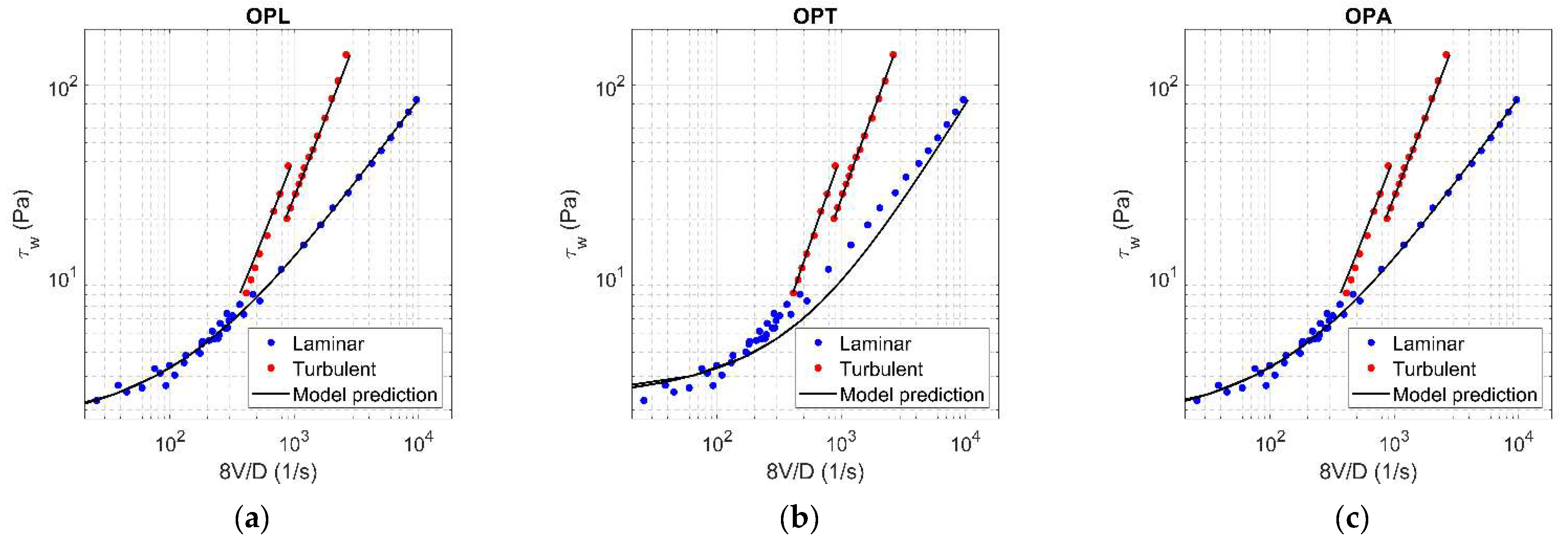
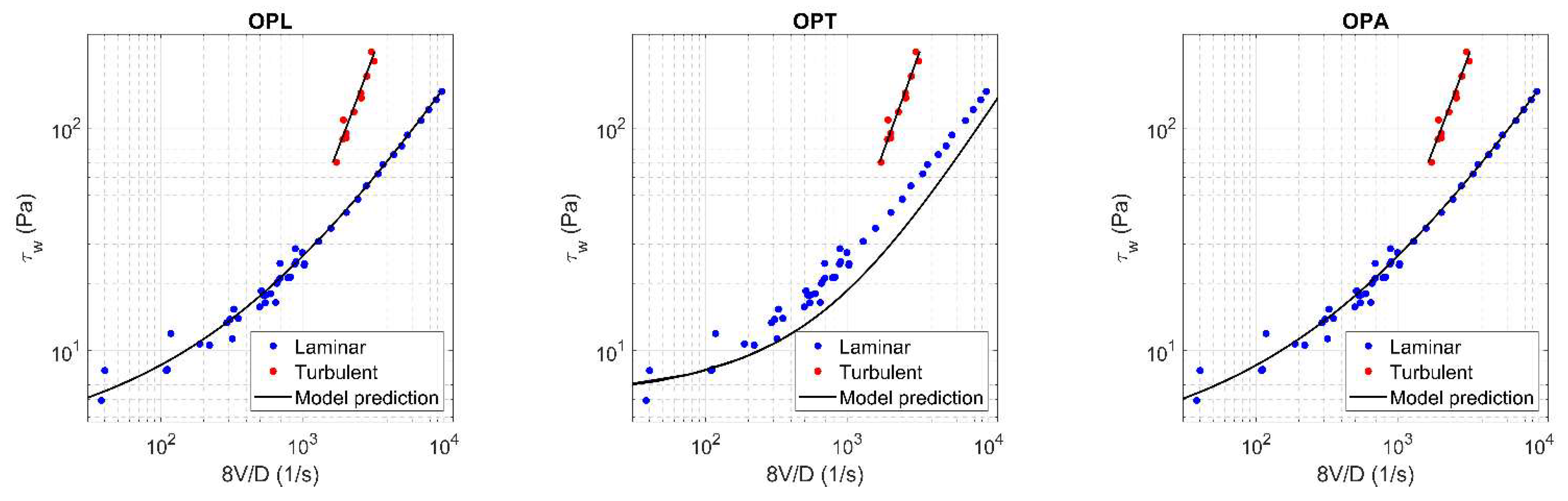
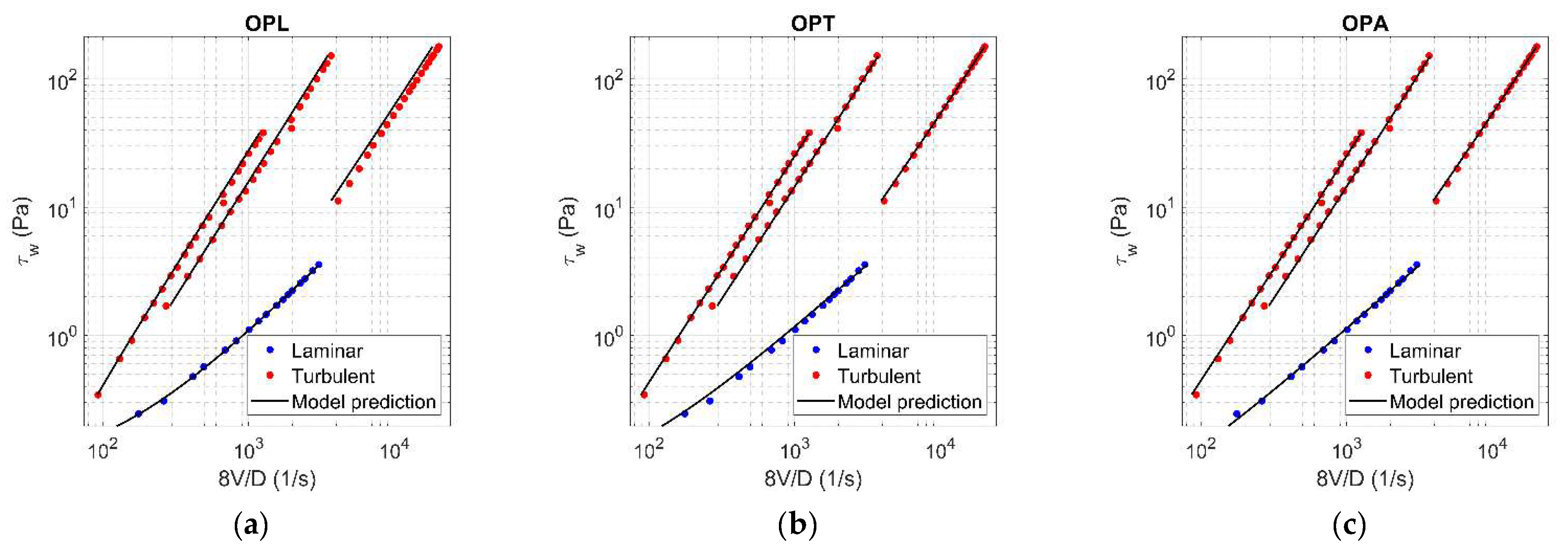
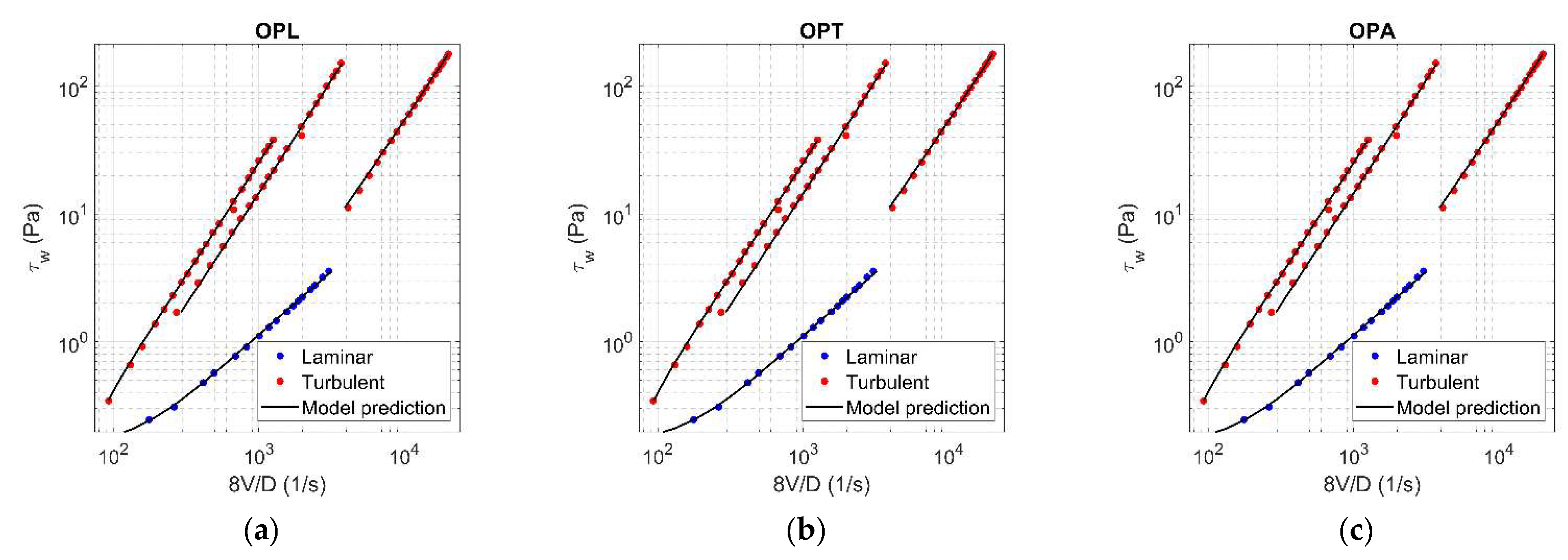
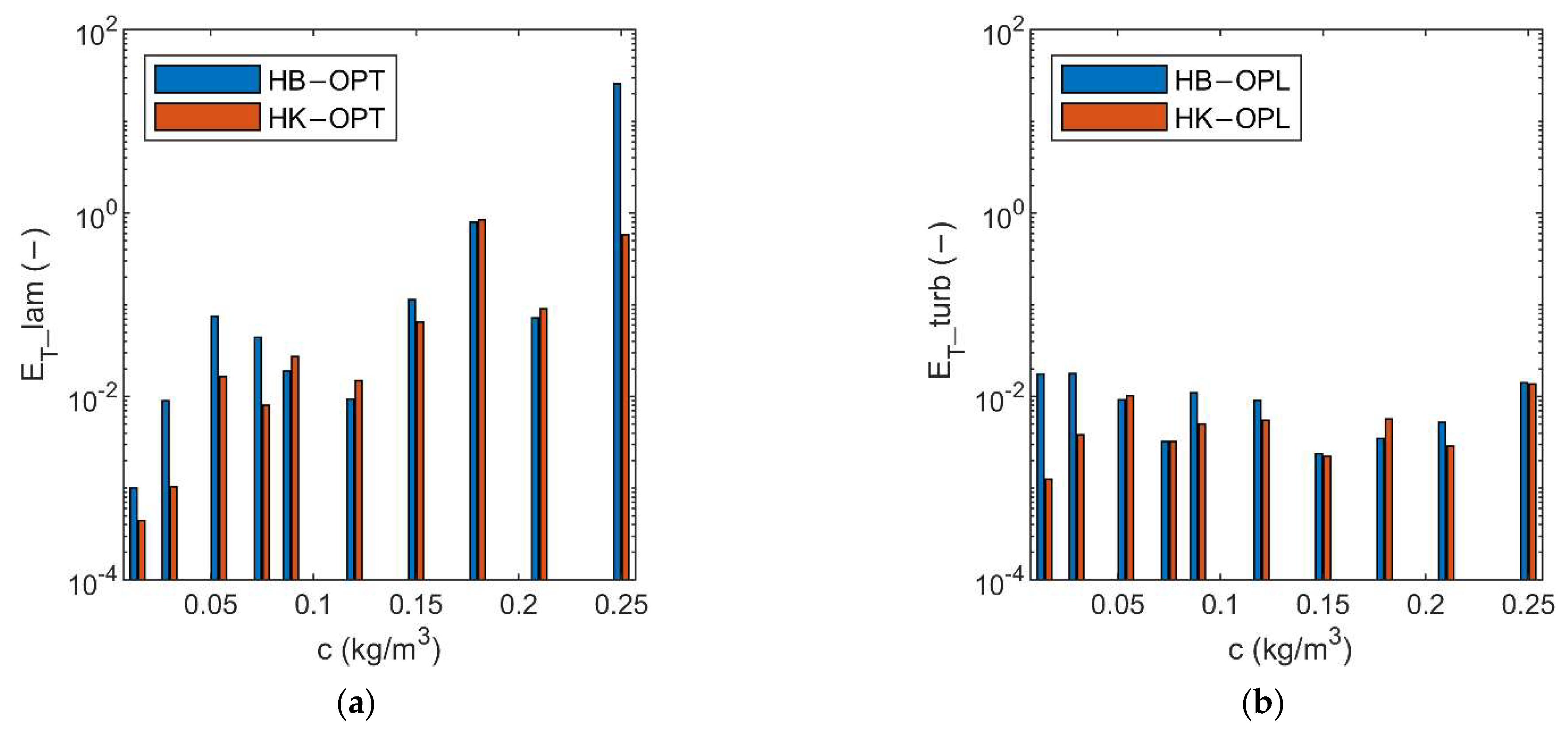
5.1. Results of Optimization on Laminar (OPL) and Turbulent (OPT) Data
5.2. Results of Optimization on all Data (OPA)
5.3. Final Considerations on the Optimization
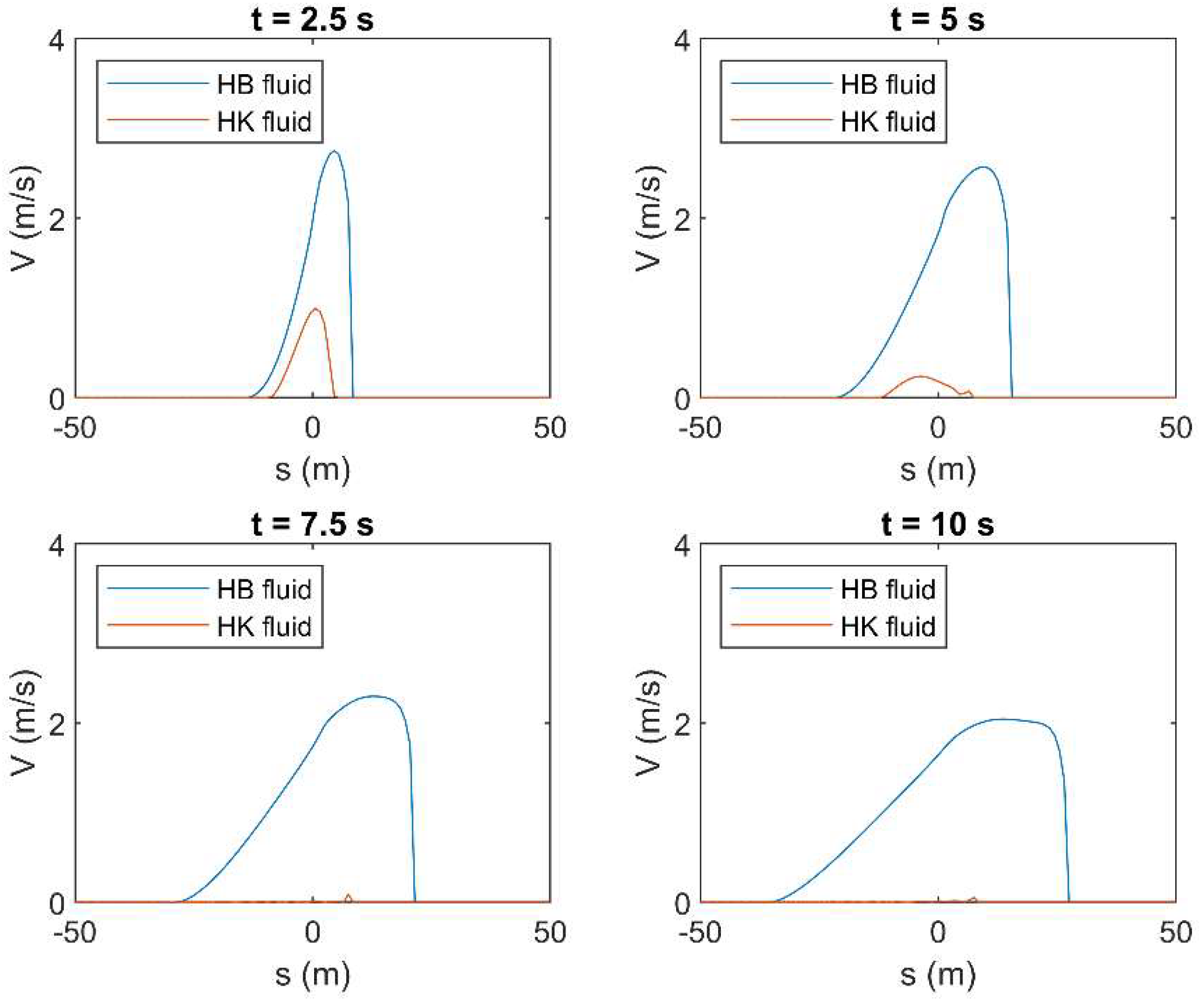
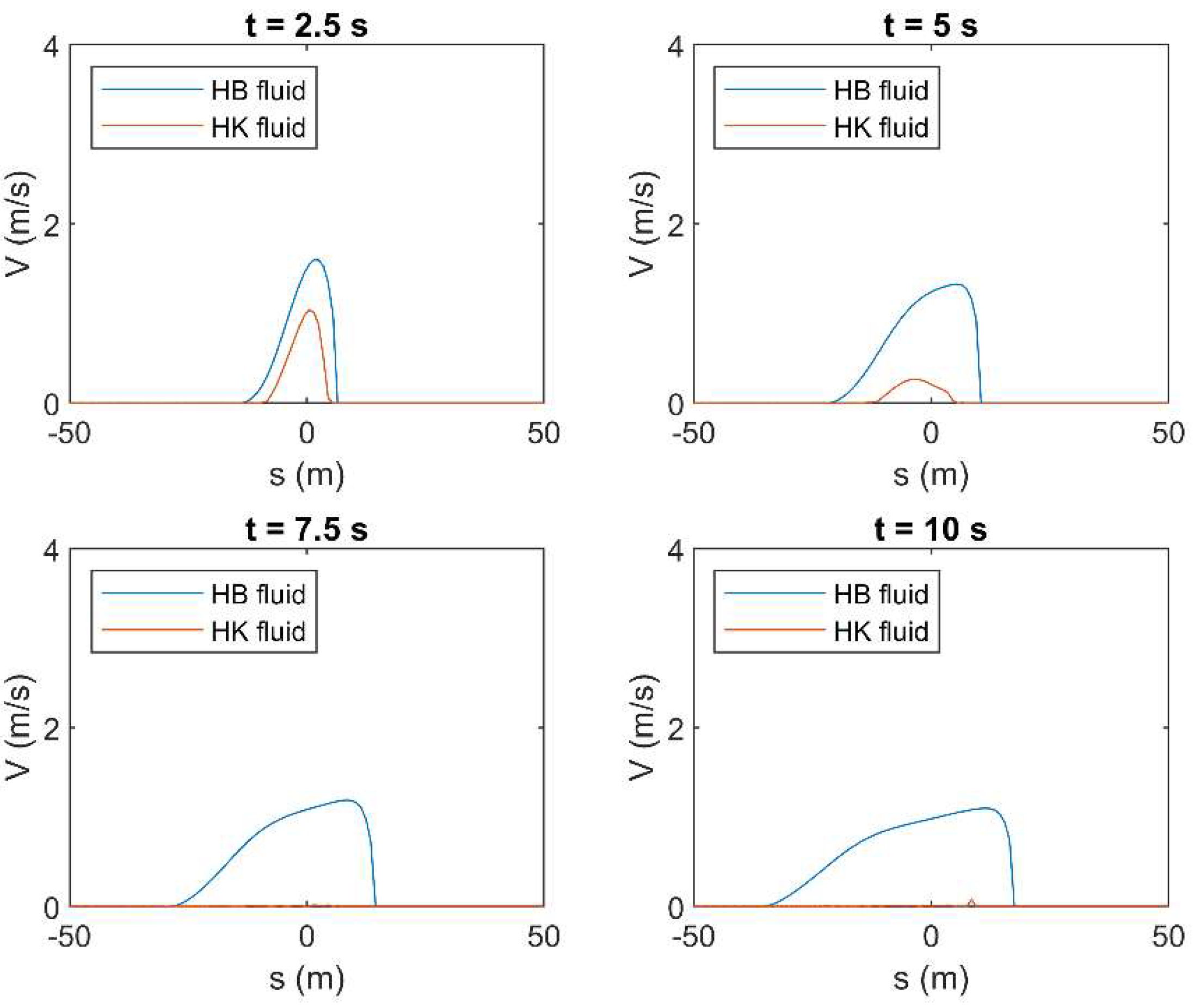
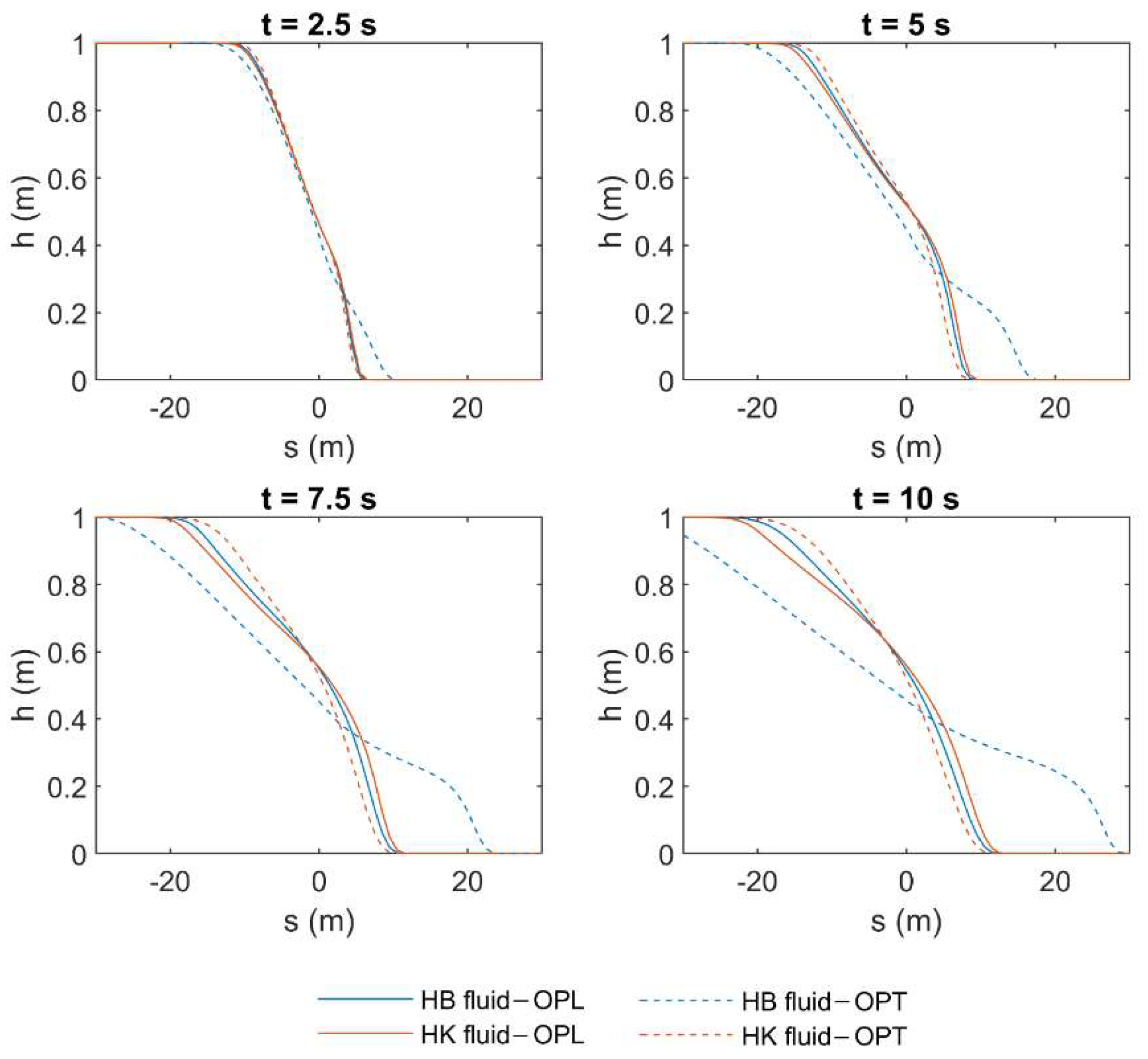
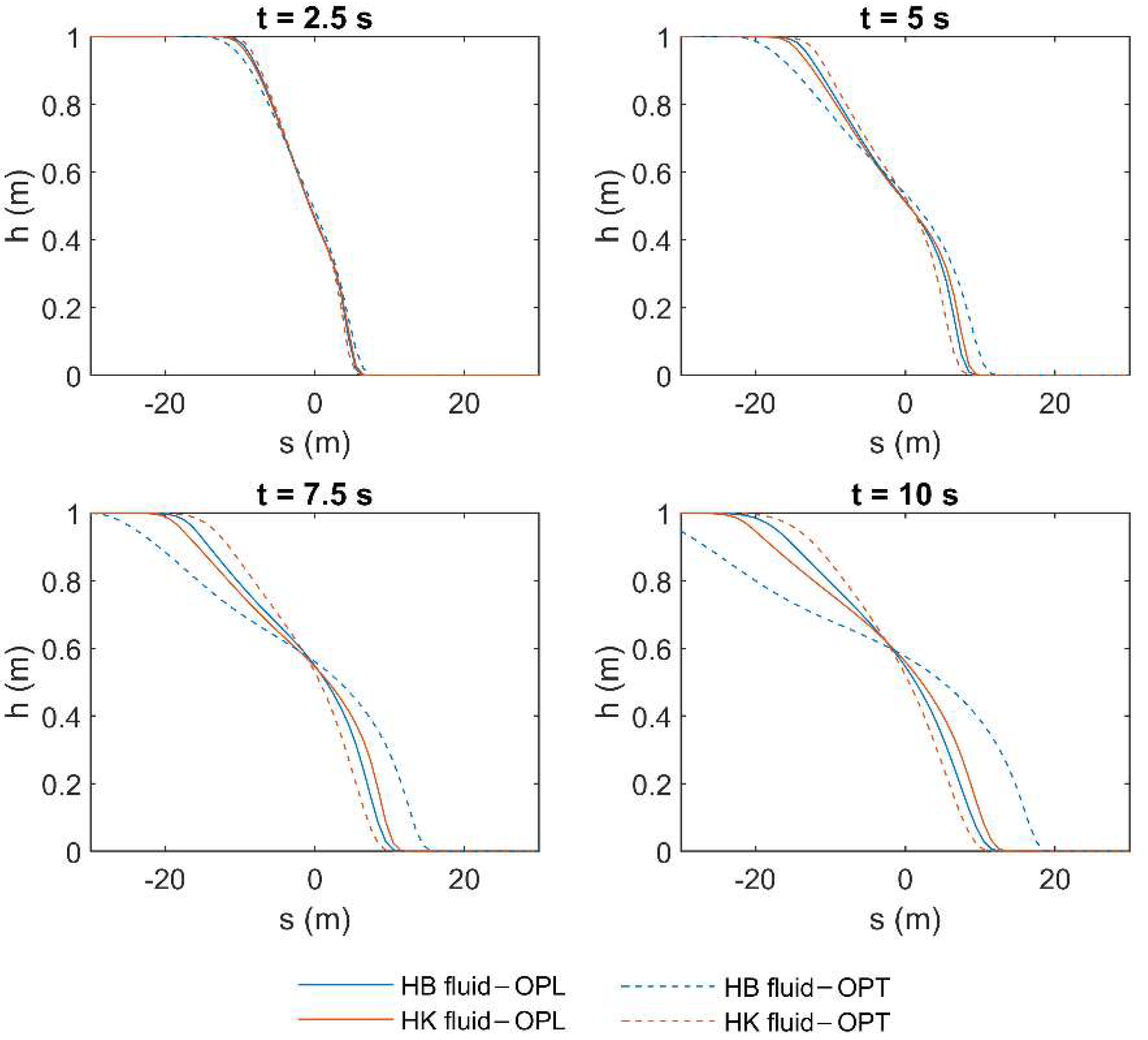
6. Engineering Implication: A Simple Dam-Break Example
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
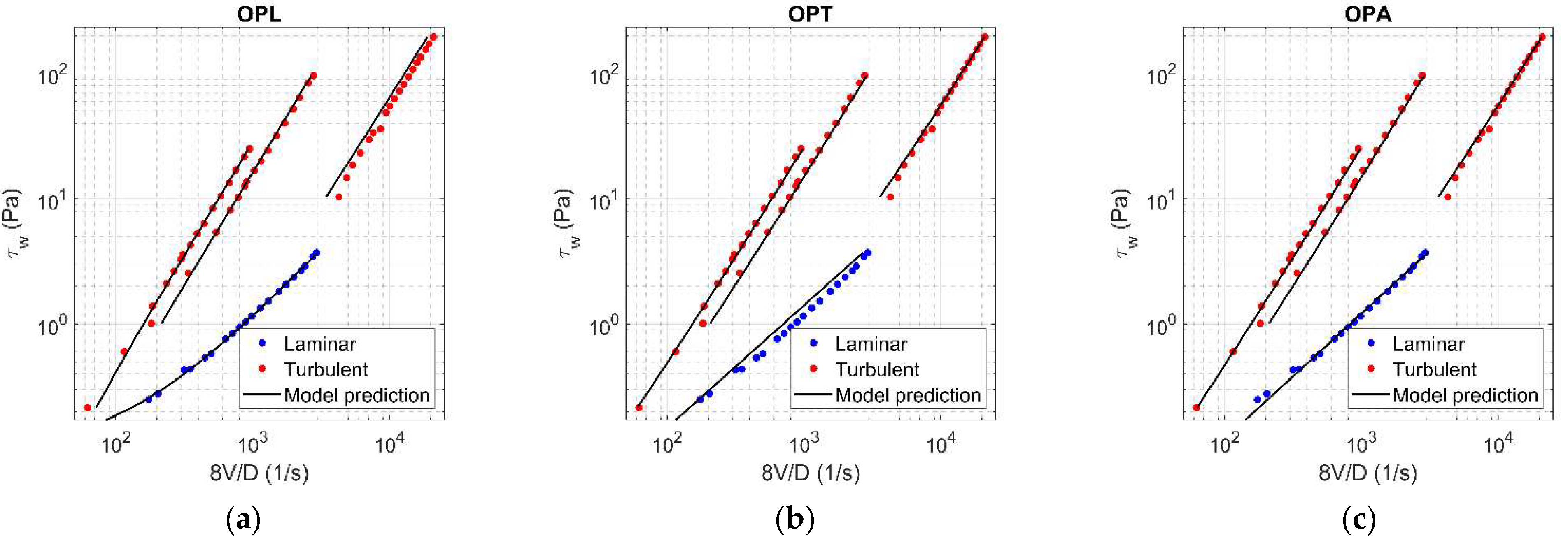
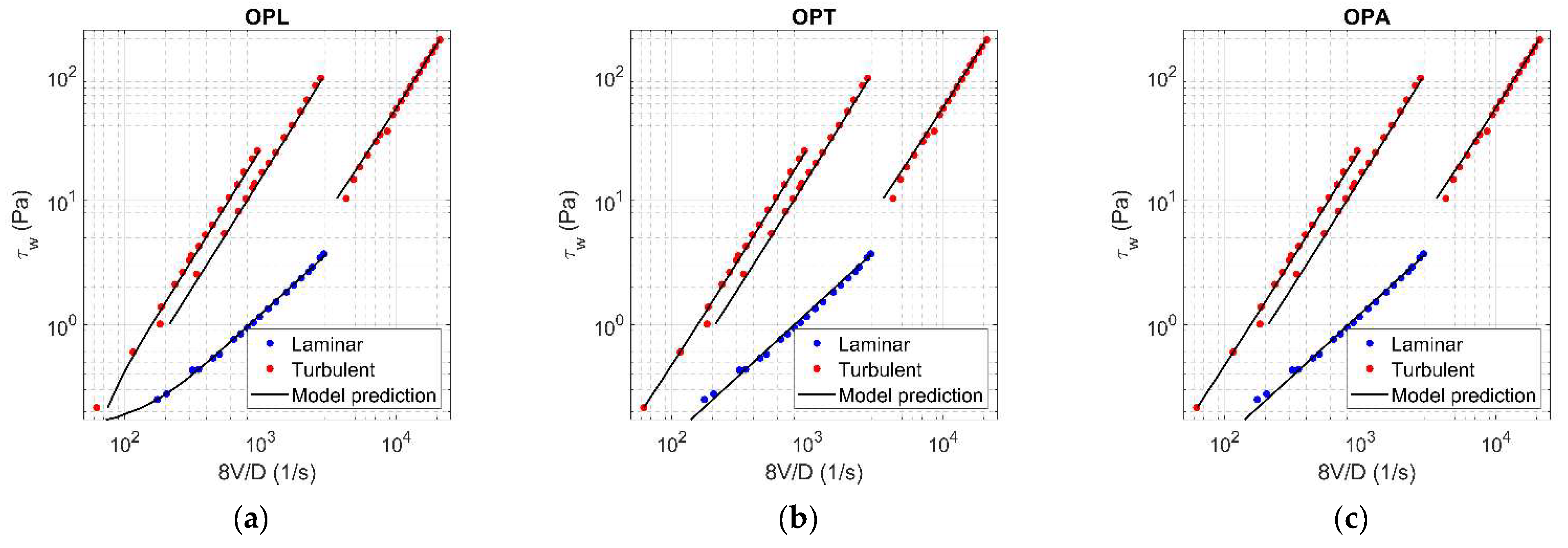
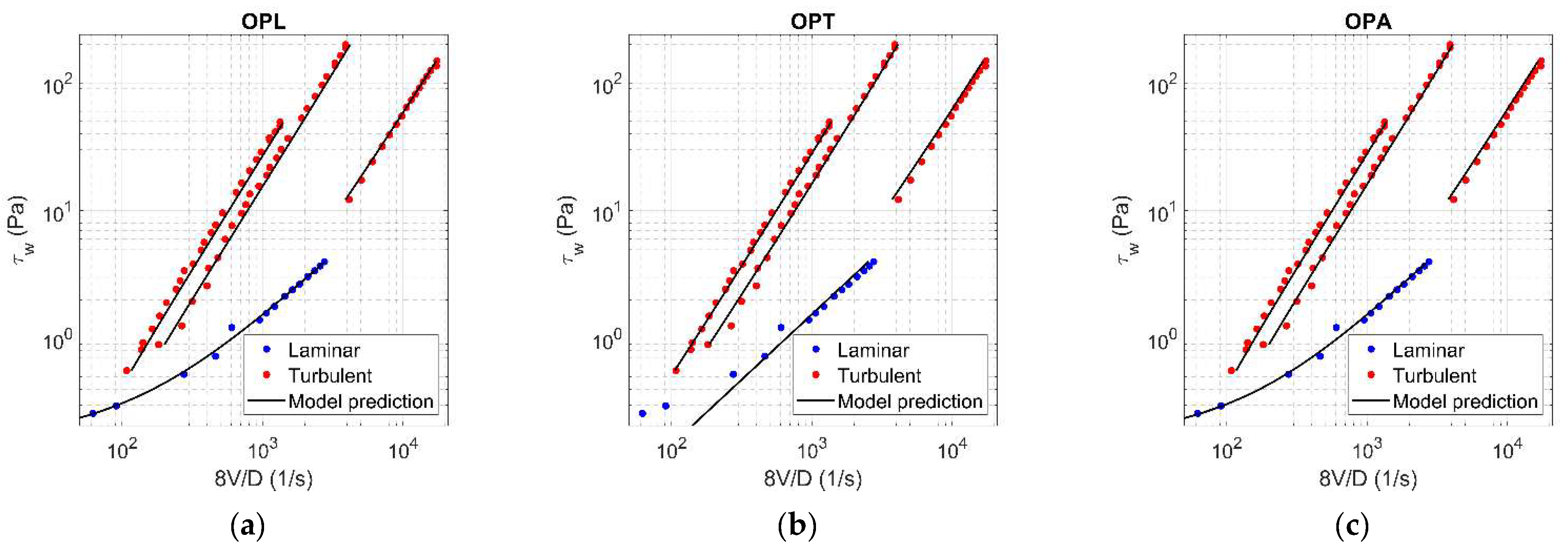








Appendix B


References
- Kazemian, S.; Prasad, A.; Huat, B.B.K. Review of newtonian and non-Newtonian fluids behaviour in the context of grouts. In Proceedings of the 7th International Symposium on Geotechnical Aspects of Underground Construction in Soft Ground, Rome, Italy, 15–19 May 2011. [Google Scholar]
- Amoo, L.M.; Layi Fagbenle, R. Overview of non-Newtonian boundary layer flows and heat transfer. In Applications of Heat, Mass and Fluid Boundary Layers; Woodland Publishing: Salt Lake City, UT, USA, 2020; ISBN 9780128179499. [Google Scholar]
- Herschel, W.H.; Bulkley, R. Konsistenzmessungen von Gummi-Benzollösungen. Kolloid Z. 1926, 39, 291–300. [Google Scholar] [CrossRef]
- Bingham, E.C. An investigation of the laws of plastic flow. US Bur. Stand. Bull. 1916, 13, 309–353. [Google Scholar] [CrossRef]
- Batchelor, G.K. An Introduction to Fluid Dynamics; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Coussot, P.; Nguyen, Q.D.; Huynh, H.T.; Bonn, D. Avalanche behavior in yield stress fluids. Phys. Rev. Lett. 2002, 88, 175501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ancey, C.; Cochard, S. The dam-break problem for Herschel-Bulkley viscoplastic fluids down steep flumes. J. Nonnewton. Fluid Mech. 2009, 158, 18–35. [Google Scholar] [CrossRef]
- Hallbom, D.; Klein, B. A physical model for yield plastic fluids. Part. Sci. Technol. 2009, 27, 1–15. [Google Scholar] [CrossRef]
- Hallbom, D.J.; Klein, B. Flow array for nickel laterite slurry. In Proceedings of the International Laterite Nickel Symposium, Charlotte, NC, USA, 14–18 March 2004. [Google Scholar]
- Magnon, E.; Cayeux, E. Precise method to estimate the herschel-bulkley parameters from pipe rheometer measurements. Fluids 2021, 6, 157. [Google Scholar] [CrossRef]
- Rooki, R.; Ardejani, F.D.; Moradzadeh, A.; Mirzaei, H.; Kelessidis, V.; Maglione, R.; Norouzi, M. Optimal determination of rheological parameters for herschel-bulkley drilling fluids using genetic algorithms (GAs). Korea Aust. Rheol. J. 2012, 24, 163–170. [Google Scholar] [CrossRef]
- Kozicki, W.; Kuang, P.Q. Prediction of lower/upper limiting viscosities. Can. J. Chem. Eng. 1993, 71, 329–331. [Google Scholar] [CrossRef]
- Frigaard, I.A.; Paso, K.G.; de Souza Mendes, P.R. Bingham’s model in the oil and gas industry. Rheol. Acta 2017, 56, 259–282. [Google Scholar] [CrossRef] [Green Version]
- Shelukhin, V.V.; Ružička, M. On Cosserat-Bingham fluids. ZAMM Z. Angew. Math. Mech. 2013, 93, 57–72. [Google Scholar] [CrossRef]
- Casson, N. A Flow Equation for Pigment-Oil Suspensions of the Printing Ink Type. In Proceedings of the Conference on Rheology of Disperse System Organized by the British Society of Rheology, Swansea, UK, 19–20 September 1957. [Google Scholar]
- Chaturani, P.; Samy, R.P. Pulsatile flow of Casson’s fluid through stenosed arteries with applications to blood flow. Biorheology 1986, 23, 499–511. [Google Scholar] [CrossRef]
- Choudhari, R.; Gudekote, M.; Vaidya, H.; Prasad, K.V. Peristaltic flow of Herschel-Bulkley fluid in an elastic tube with slip at porous walls. J. Adv. Res. Fluid Mech. Therm. Sci. 2018, 52, 63–75. [Google Scholar]
- Chilton, R.; Thompson, S.; Stainsby, R. The design of sewage sludge pumping systems. J. Hydraul. Res. 1996, 34, 395–408. [Google Scholar] [CrossRef]
- Steffe, J.F. Rheological Methods in Food Process Engineering, 2nd ed.; Freeman Press: East Lansing, MI, USA, 1996. [Google Scholar]
- Mullineux, G. Non-linear least squares fitting of coefficients in the Herschel-Bulkley model. Appl. Math. Model. 2008, 32, 2538–2551. [Google Scholar] [CrossRef]
- Chilton, R.A.; Stainsby, R. Pressure Loss Equations for Laminar and Turbulent Non-Newtonian Pipe Flow. J. Hydraul. Eng. 1998, 124, 522–529. [Google Scholar] [CrossRef]
- van den Heever, E.M.; Sutherland, A.P.N.; Haldenwang, R. Influence of the Rheological Model Used in Pipe-Flow Prediction Techniques for Homogeneous Non-Newtonian Fluids. J. Hydraul. Eng. 2014, 140, 4014059. [Google Scholar] [CrossRef]
- Molki, A.; Khezzar, L.; Goharzadeh, A. Measurement of fluid velocity development in laminar pipe flow using laser Doppler velocimetry. Eur. J. Phys. 2013, 34, 1127. [Google Scholar] [CrossRef]
- Gul, S.; Erge, O.; van Oort, E. Frictional pressure losses of Non-Newtonian fluids in helical pipes: Applications for automated rheology measurements. J. Nat. Gas Sci. Eng. 2020, 73, 103042. [Google Scholar] [CrossRef]
- Simão, M.; Besharat, M.; Carravetta, A.; Ramos, H.M. Flow velocity distribution towards flowmeter accuracy: CFD, UDV, and field tests. Water 2018, 10, 1807. [Google Scholar] [CrossRef] [Green Version]
- Sarno, L.; Tai, Y.C.; Carravetta, A.; Martino, R.; Nicolina Papa, M.; Kuo, C.Y. Challenges and improvements in applying a particle image velocimetry (PIV) approach to granular flows. J. Phys. Conf. Ser. 2019, 1249, 012011. [Google Scholar] [CrossRef]
- Rabinowitsch, B. Über die Viskosität und Elastizität von Solen. Z. Phys. Chem. 1929, 145, 1–26. [Google Scholar] [CrossRef]
- Mooney, M. Explicit Formulas for Slip and Fluidity. J. Rheol. 1931, 2, 210–222. [Google Scholar] [CrossRef]
- Dodge, D.W.; Metzner, A.B. Turbulent flow of non-newtonian systems. AIChE J. 1959, 5, 189–204. [Google Scholar] [CrossRef]
- Metzner, A.B.; Reed, J.C. Flow of non-newtonian fluids—Correlation of the laminar, transition, and turbulent-flow regions. AIChE J. 1955, 1, 434–440. [Google Scholar] [CrossRef]
- Metzner, A.B. Non-Newtonian Fluid Flow Relationships between Recent Pressure-Drop Correlations. Ind. Eng. Chem. 1957, 49, 1429–1432. [Google Scholar] [CrossRef]
- Lazarus, J.H.; Slatter, P.T. A method for the rheological characterisation of tube viscometer data. J. Pipelines 1988, 7, 165–176. [Google Scholar]
- Wilson, K.C.; Thomas, A.D. A new analysis of the turbulent flow of non-newtonian fluids. Can. J. Chem. Eng. 1985, 63, 539–546. [Google Scholar] [CrossRef]
- Thomas, A.D.; Wilson, K.C. New Analysis of Non-Newtonian Turbulent Flow—Yield-Power-Law Fluids. Can. J. Chem. Eng. 1987, 65, 335–338. [Google Scholar] [CrossRef]
- Carravetta, A.; Conte, M.C.; Fecarotta, O.; Martino, R. Performance of Slurry Flow Models in Pressure Pipe Tests. J. Hydraul. Eng. 2016, 142, 6015020. [Google Scholar] [CrossRef]
- Çengel, Y.A.; Cimbala, J.M. Solutions Manual for Fluid Mechanics: Fundamentals and Applications; McGraw-Hill: New York, NY, USA, 2006. [Google Scholar]
- Bergman, T.L.; Lavine, A.S.; Incropera, F.P.; DeWitt, D.P. Fundamentals of Heat and Mass Transfer, 7th ed.; John Wiley & Sons: New York, NY, USA, 2011. [Google Scholar]
- Carravetta, A.; Fecarotta, O.; Martino, R.; Sabatino, C. Assessment of Rheological Characteristics of a Natural Bingham-Plastic Mixture in Turbulent Pipe Flow. J. Hydraul. Eng. 2010, 136, 820–825. [Google Scholar] [CrossRef] [Green Version]
- Bowen, L.R. How to Handle Slurries. Chem. Eng. 1961, 68, 119–122. [Google Scholar]
- MATLAB Global Optimization Toolbox; TheMathWorks: Natick, MA, USA, 2016.
- Sarno, L.; Carravetta, A.; Martino, R.; Tai, Y.-C. Pressure Coefficient in Dam-Break Flows of Dry Granular Matter. J. Hydraul. Eng. 2013, 139, 1126–1133. [Google Scholar] [CrossRef]
- Sarno, L.; Wang, Y.; Tai, Y.-C.; Martino, R.; Carravetta, A. Asymptotic analysis of the eigenstructure of the two-layer model and a new family of criteria for evaluating the model hyperbolicity. Adv. Water. Resour. 2021, 154, 103966. [Google Scholar] [CrossRef]
- Sarno, L.; Carravetta, A.; Tai, Y.-C.; Martino, R.; Papa, M.N.; Kuo, C.-Y. Measuring the velocity fields of granular flows—Employment of a multi-pass two-dimensional particle image velocimetry (2D-PIV) approach. Adv. Powder Technol. 2018, 29, 3107–3123. [Google Scholar] [CrossRef]
- Sarno, L.; Carravetta, A.; Martino, R.; Tai, Y.-C. A two-layer depth-averaged approach to describe the regime stratification in collapses of dry granular columns. Phys. Fluids 2014, 26, 103303. [Google Scholar] [CrossRef]
- Harten, A.; Lax, P.D.; Leer, B. van On Upstream Differencing and Godunov-Type Schemes for Hyperbolic Conservation Laws. SIAM Rev. 1983, 25, 35–61. [Google Scholar] [CrossRef]
- Aliparast, M. Two-dimensional finite volume method for dam-break flow simulation. Int. J. Sediment. Res. 2009, 24, 99–107. [Google Scholar] [CrossRef]
- Di Cristo, C.; Greco, M.; Iervolino, M.; Vacca, A. Interaction of a dam-break wave with an obstacle over an erodible floodplain. J. Hydroinf. 2020, 22, 5–19. [Google Scholar] [CrossRef]




| 0.0144 | 0.03 | 0.054 | 0.075 | 0.089 | 0.12 | 0.15 | 0.18 | 0.21 | 0.25 | ||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 2.91 | 45 | 48 | 35 | 36 | 52 | 35 | 36 | 36 | 27 | 20 | |
| 18.05 | 22 | 16 | 27 | 27 | 22 | 27 | 30 | 47 | 23 | 21 | |
| 25.82 | 22 | 16 | 27 | 27 | 21 | 27 | 30 | 47 | 23 | 21 | |
| 0.0144 | 0.03 | 0.054 | 0.075 | 0.089 | 0.12 | 0.15 | 0.18 | 0.21 | 0.25 | |
|---|---|---|---|---|---|---|---|---|---|---|
(Pa) | 0.0723 | 0.0790 | 0.1363 | 0.1178 | 0.0799 | 0.3514 | 0.2934 | 0.7756 | 1.4962 | 4.3776 |
(Pa sn) | 0.0005 | 0.0005 | 0.0013 | 0.0016 | 0.0035 | 0.0016 | 0.0076 | 0.0108 | 0.0230 | 0.0631 |
(−) | 1.1110 | 1.1162 | 1.0117 | 1.0144 | 0.9272 | 1.0685 | 0.9445 | 0.9297 | 0.8859 | 0.8343 |
(−) | 0.0001 | 0.0002 | 0.0014 | 0.0020 | 0.0025 | 0.0004 | 0.0099 | 0.0103 | 0.0032 | 0.0305 |
(−) | 0.0176 | 0.0178 | 0.0092 | 0.0032 | 0.0110 | 0.0091 | 0.0024 | 0.0035 | 0.0052 | 0.0141 |
(−) | 0.0139 | 0.0128 | 0.0078 | 0.0029 | 0.0073 | 0.0066 | 0.0054 | 0.0073 | 0.0038 | 0.0273 |
| 0.0144 | 0.03 | 0.054 | 0.075 | 0.089 | 0.12 | 0.15 | 0.18 | 0.21 | 0.25 | |
|---|---|---|---|---|---|---|---|---|---|---|
(Pa) | 0.1214 | 0.1238 | 0.1449 | 0.1043 | 0.0799 | 0.3952 | 0.2048 | 0.5558 | 1.1935 | 3.2606 |
(Pa s) | 0.0011 | 0.0012 | 0.0014 | 0.0018 | 0.0019 | 0.0028 | 0.0046 | 0.0054 | 0.0076 | 0.0115 |
(−) | 2.9963 | 2.9270 | 1.1023 | 0.9446 | 0.7195 | 1.3753 | 0.6729 | 0.6644 | 0.6325 | 0.5485 |
(−) | 0.0003 | 0.0005 | 0.0014 | 0.0020 | 0.0016 | 0.0006 | 0.0093 | 0.0101 | 0.0031 | 0.0313 |
(−) | 0.0012 | 0.0038 | 0.0102 | 0.0032 | 0.0050 | 0.0055 | 0.0022 | 0.0057 | 0.0029 | 0.0137 |
(−) | 0.0010 | 0.0029 | 0.0086 | 0.0029 | 0.0035 | 0.0041 | 0.0051 | 0.0082 | 0.0030 | 0.0279 |
| 0.0144 | 0.03 | 0.054 | 0.075 | 0.089 | 0.12 | 0.15 | 0.18 | 0.21 | 0.25 | |
|---|---|---|---|---|---|---|---|---|---|---|
(Pa) | 0.0447 | 0.0000 | 0.0000 | 0.0466 | 0.0002 | 0.0000 | 0.0886 | 0.0000 | 2.2122 | 0.7080 |
(Pa sn) | 0.0011 | 0.0016 | 0.0013 | 0.0012 | 0.0038 | 0.0045 | 0.0039 | 0.0051 | 0.0041 | 0.0014 |
(−) | 0.9999 | 0.9765 | 1.0229 | 1.0342 | 0.9429 | 0.9556 | 1.0100 | 0.9898 | 1.0702 | 1.1793 |
(−) | 0.0010 | 0.0090 | 0.0753 | 0.0443 | 0.0190 | 0.0094 | 0.1145 | 0.7941 | 0.0717 | 25.8229 |
(−) | 0.0013 | 0.0034 | 0.0062 | 0.0031 | 0.0035 | 0.0041 | 0.0018 | 0.0027 | 0.0007 | 0.0114 |
(−) | 0.0012 | 0.0050 | 0.0187 | 0.0139 | 0.0103 | 0.0057 | 0.0478 | 0.4478 | 0.0480 | 20.7528 |
| 0.0144 | 0.03 | 0.054 | 0.075 | 0.089 | 0.12 | 0.15 | 0.18 | 0.21 | 0.25 | |
|---|---|---|---|---|---|---|---|---|---|---|
(Pa) | 0.1301 | 0.0021 | 0.1590 | 0.2121 | 0.0003 | 0.0006 | 0.5830 | 0.5937 | 2.2167 | 5.8847 |
(Pa s) | 0.0011 | 0.0012 | 0.0017 | 0.0018 | 0.0020 | 0.0028 | 0.0043 | 0.0046 | 0.0079 | 0.0112 |
(−) | 2.9798 | 2.2878 | 2.1208 | 1.5329 | 2.3011 | 2.3045 | 2.9981 | 2.9992 | 1.3203 | 1.0378 |
(−) | 0.0004 | 0.0010 | 0.0165 | 0.0080 | 0.0274 | 0.0148 | 0.0647 | 0.8515 | 0.0906 | 0.5847 |
(−) | 0.0012 | 0.0035 | 0.0062 | 0.0031 | 0.0039 | 0.0044 | 0.0017 | 0.0027 | 0.0010 | 0.0116 |
(−) | 0.0011 | 0.0028 | 0.0081 | 0.0044 | 0.0142 | 0.0074 | 0.0274 | 0.4801 | 0.0607 | 0.4721 |
| 0.0144 | 0.03 | 0.054 | 0.075 | 0.089 | 0.12 | 0.15 | 0.18 | 0.21 | 0.25 | |
|---|---|---|---|---|---|---|---|---|---|---|
(Pa) | 0.0185 | 0.0010 | 0.1412 | 0.1159 | 0.0799 | 0.2095 | 0.3189 | 0.7517 | 1.5972 | 0.0000 |
(Pa sn) | 0.0011 | 0.0012 | 0.0010 | 0.0017 | 0.0025 | 0.0030 | 0.0068 | 0.0117 | 0.0195 | 0.7293 |
(−) | 1.0003 | 1.0037 | 1.0486 | 1.0072 | 0.9755 | 0.9932 | 0.9599 | 0.9189 | 0.9059 | 1.7004 |
(−) | 0.0004 | 0.0007 | 0.0017 | 0.0020 | 0.0037 | 0.0016 | 0.0100 | 0.0104 | 0.0034 | 1.3400 |
(−) | 0.0013 | 0.0035 | 0.0067 | 0.0031 | 0.0042 | 0.0050 | 0.0021 | 0.0031 | 0.0041 | 0.0244 |
(−) | 0.0011 | 0.0027 | 0.0058 | 0.0028 | 0.0040 | 0.0040 | 0.0053 | 0.0072 | 0.0036 | 1.0816 |
| c | 0.0144 | 0.03 | 0.054 | 0.075 | 0.089 | 0.12 | 0.15 | 0.18 | 0.21 | 0.25 |
|---|---|---|---|---|---|---|---|---|---|---|
(Pa) | 0.1264 | 0.0083 | 0.2003 | 0.1247 | 0.0799 | 0.3433 | 0.1843 | 0.3754 | 1.2771 | 3.1178 |
(Pa s) | 0.0011 | 0.0012 | 0.0016 | 0.0018 | 0.0019 | 0.0028 | 0.0044 | 0.0049 | 0.0077 | 0.0111 |
(−) | 2.9976 | 1.6770 | 2.3525 | 1.0991 | 0.7639 | 1.3487 | 0.6325 | 0.5407 | 0.6607 | 0.5305 |
(−) | 0.0003 | 0.0007 | 0.0046 | 0.0021 | 0.0018 | 0.0009 | 0.0093 | 0.0106 | 0.0032 | 0.0314 |
(−) | 0.0012 | 0.0035 | 0.0068 | 0.0032 | 0.0046 | 0.0053 | 0.0021 | 0.0038 | 0.0027 | 0.0130 |
(−) | 0.0010 | 0.0027 | 0.0064 | 0.0029 | 0.0034 | 0.0040 | 0.0051 | 0.0076 | 0.0030 | 0.0278 |
(Pa) | (Pa) | (Pa) | (Pa) | |
|---|---|---|---|---|
| 2.5 | 0.65 | 0.71 | 0.20 | 0.85 |
| 5.0 | 0.99 | 0.92 | 0.29 | 1.09 |
| 7.5 | 1.10 | 1.07 | 0.35 | 0.68 |
| 10 | 0.88 | 0.85 | 0.42 | 0.97 |
(Pa) | (Pa) | (Pa) | (Pa) | |
|---|---|---|---|---|
| 2.5 | 0.61 | 0.66 | 0.42 | 0.86 |
| 5.0 | 1.04 | 0.99 | 0.50 | 1.13 |
| 7.5 | 0.90 | 0.95 | 0.54 | 0.77 |
| 10 | 0.60 | 0.93 | 0.56 | 0.70 |
(Pa) | (Pa) | (Pa) | (Pa) | |
|---|---|---|---|---|
| 2.5 | 5.69 | 5.30 | 1.51 | 6.50 |
| 5.0 | 5.12 | 4.67 | 1.32 | 6.14 |
| 7.5 | 4.57 | 4.02 | 1.20 | 6.19 |
| 10 | 4.51 | 3.42 | 1.31 | 6.03 |
(Pa) | (Pa) | (Pa) | (Pa) | |
|---|---|---|---|---|
| 2.5 | 5.25 | 5.01 | 4.76 | 6.29 |
| 5.0 | 4.83 | 4.33 | 3.27 | 6.05 |
| 7.5 | 4.56 | 3.91 | 2.61 | 5.90 |
| 10 | 4.92 | 3.44 | 2.21 | 6.10 |
(°) | (°) | (°) | (°) | |
|---|---|---|---|---|
| 2.5 | 7.7 | 7.3 | 2.3 | 7.7 |
| 5.0 | 6.4 | 6.6 | 1.8 | 6.3 |
| 7.5 | 4.6 | 5.2 | 1.9 | 4.1 |
| 10 | 3.5 | 3.9 | 1.9 | 3.4 |
(°) | (°) | (°) | (°) | |
|---|---|---|---|---|
| 2.5 | 7.3 | 6.6 | 5.5 | 7.9 |
| 5.0 | 6 | 6.4 | 4.3 | 6.1 |
| 7.5 | 4.7 | 5.4 | 4.0 | 4.1 |
| 10 | 3.5 | 3.7 | 3.6 | 3.4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Carravetta, A.; Fecarotta, O.; Martino, R.; Morani, M.C. Rheological Characterization of Non-Newtonian Mixtures by Pressure Pipe Tests. Fluids 2021, 6, 419. https://doi.org/10.3390/fluids6110419
Carravetta A, Fecarotta O, Martino R, Morani MC. Rheological Characterization of Non-Newtonian Mixtures by Pressure Pipe Tests. Fluids. 2021; 6(11):419. https://doi.org/10.3390/fluids6110419
Chicago/Turabian StyleCarravetta, Armando, Oreste Fecarotta, Riccardo Martino, and Maria Cristina Morani. 2021. "Rheological Characterization of Non-Newtonian Mixtures by Pressure Pipe Tests" Fluids 6, no. 11: 419. https://doi.org/10.3390/fluids6110419
APA StyleCarravetta, A., Fecarotta, O., Martino, R., & Morani, M. C. (2021). Rheological Characterization of Non-Newtonian Mixtures by Pressure Pipe Tests. Fluids, 6(11), 419. https://doi.org/10.3390/fluids6110419