Teaching and Learning Floating and Sinking: Didactic Transformation in a Density-Based Approach
Abstract
:1. Introduction
2. Alternative Ideas and Difficulties in Explaining FS Phenomena
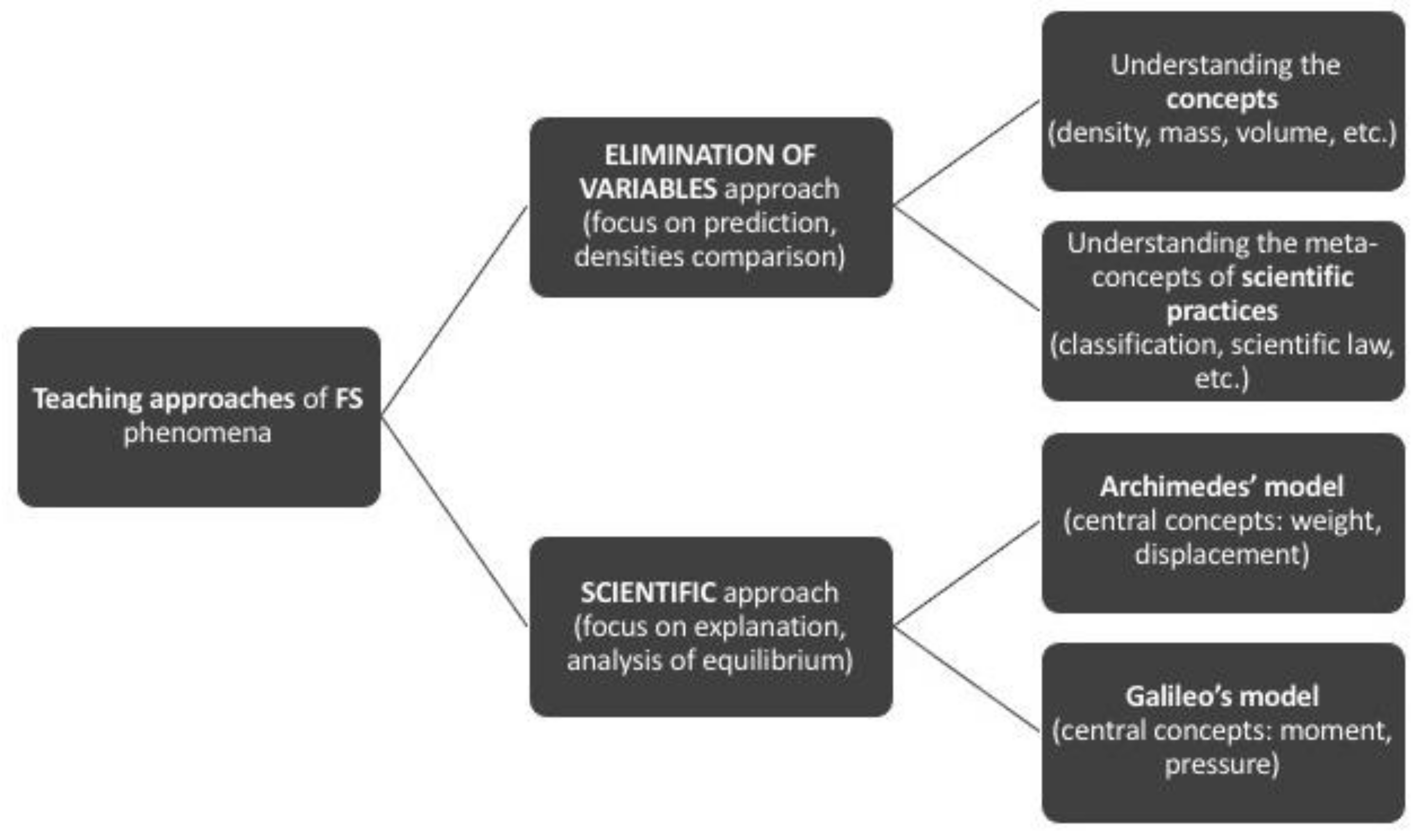
3. Floating and Sinking Teaching Approaches
4. Description of the TLS: Emphasis on the Content of FS and Its Didactic Transformation
4.1. The Design Principles of the TLS
- 1.
- The didactic transformation of content [11] concerning FS phenomena, that is, firstly, the decision to adopt a density-based approach to negotiate FS phenomena, and, secondly, the decision to introduce the concept of density in a qualitative way. Both decisions were driven by the difficulties that primary school students confront when prompted to negotiate FS phenomena.
- 2.
- The participatory design and developmental character [47] of the TLS includes teachers, together with researchers, in its designing, developing and evaluating processes. Teachers discussed the nature of the TLS activities with the researchers, how they understood the activities, the possible difficulties that students might face, and consequently the possible changes and/or specific teaching methods that would be suggested. Thus, the research was adapted to the particular needs of the school and the students.
- 3.
- The TLS’s iterative process [8], which provided the opportunity to both researchers and teachers to evaluate together the initial implementation of the TLS and to propose improvement modifications [19]. Thus, the iterative evolution of the TLS contributed to the final version of the TLS and its didactic transformation.
- 4.
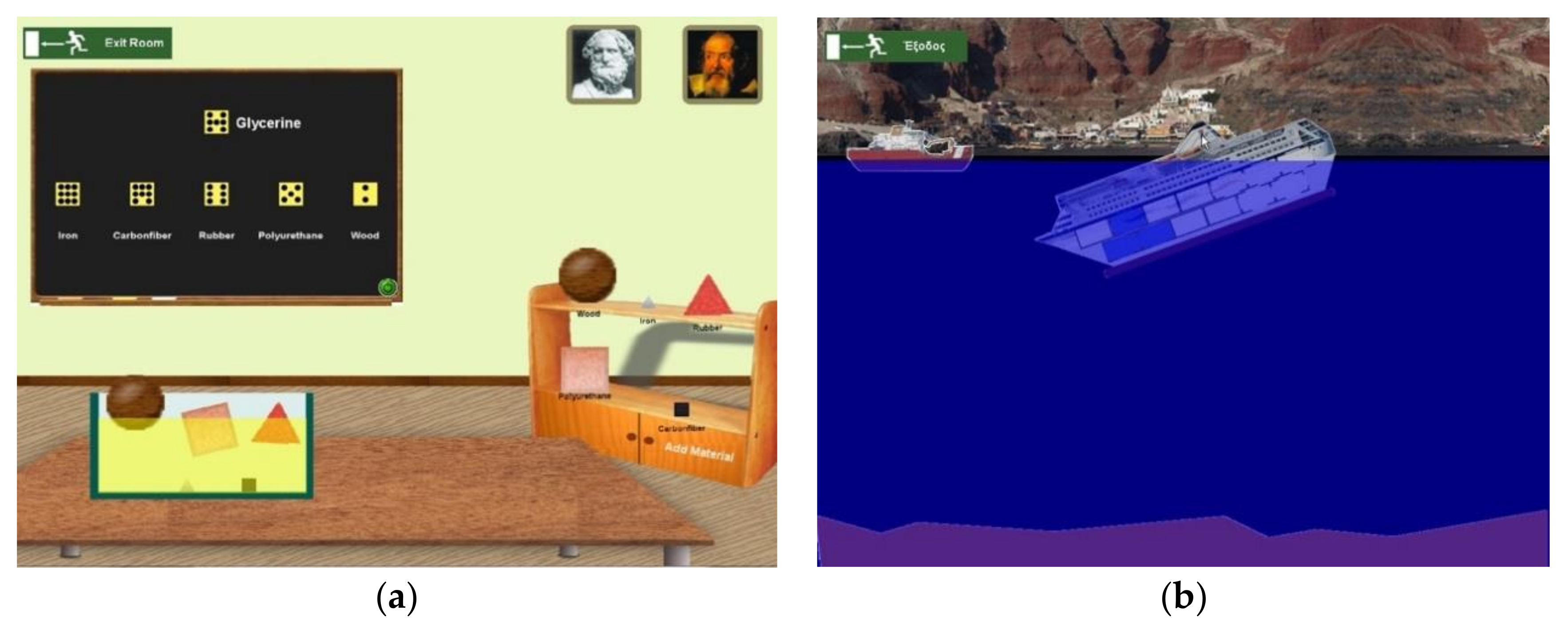
- The technological problem scenario, to provide a supportive context for learning [48]. The scenario was based on salvaging the Sea Diamond shipwreck. Discussion is initiated with an everyday problem (in our case, technological), which poses questions. These are answered through scientific knowledge that is eventually applied to solve the initial real-life problem. The combination of technological and scientific knowledge in teaching promotes active learning, improves students’ performance and attitudes towards science, enhances positive interaction between teachers and students, and provides students with opportunities to participate in authentic exploratory processes, which are usually carried out by scientists [49,50].
- 5.
- The Inquiry-Based Science Education (IBSE) approach, by emphasizing the need to use scientific practices: (a) as a means of teaching and learning, e.g., investigating the variables that possibly influence the FS phenomena in groups, and (b) as an educational end, whose aim is to understand specific aspects of scientific practices [46,51,52]. Within this framework, learning is perceived as active and student-centered, due to pupils’ increased interest and autonomy [53], and the intention is for them to gain ample practice in scientific reading and writing [54].
- 6.
- The use of digital tools, such as a simulation that was developed from scratch for the TLS [17]. Looking at existing educational software on FS phenomena, the proposals were inappropriate for our task, mainly because it was difficult to implement the inquiry-based activities and also because they tended to have a mathematical approach to the introduction of the concept of density. Therefore, a specially designed software package that followed the design principles of the TLS was designed from scratch. Furthermore, a simulated website on a local network was designed and developed for students to investigate information about materials, with the aim of becoming accustomed to scientific reading and writing skills.
4.2. The Didactic Transformation of the TLS
- 1.
- Only static FS phenomena can be interpreted: It was decided not to study buoyant force as an alternative explanatory model of FS phenomena because we considered the analysis of equilibrium approach between buoyant and gravity forces, which is implied in the buoyant force model (see Section 3) is particularly difficult for primary school and some junior high school students. Additionally, the concept of displaced liquid, which is not necessary for the density-based model, was omitted as it might distract students from the expected learning outcomes of the TLS. This means that several aspects of FS phenomena regarding objects in fluids that are not at rest but in motion cannot be explained. For instance, the motion of an object that is initially sunk in the water and then is released when the density of the object is smaller than that of the water can only be explained if an analysis of equilibrium model is used.
- 2.
- Only a qualitative estimation of material density can be determined: We are aware that the qualitative “dots-in-a-box” representation does not accurately match the actual value of material density. It provides an approximate estimation of density that also enables an approximate estimation of the inequality of the relationships between the densities (larger-smaller). For example, the relationship between the density of oil and rubber, of course, is not equal to the ratio of one to two (1:2) (Figure 2); the diagram depicts only that the density of rubber is larger than that of oil. However, when it is necessary to determine the density of a composite object consisting of two materials of different densities, the only information the qualitative “dots-in-a-box” representation can give us is that the density of the composite object ranges between the densities of these two materials. In contrast, if we use the mathematical ratio of the concept of density, then we can precisely calculate the average density of the composite object. Another limitation of the qualitative introduction of density as a property of materials is that it cannot predict nor explain changes in density (especially of gases) under temperature and pressure fluctuations. Such changes could be explained microscopically, at an older age though, using the particle model of matter.
4.3. The FS Content of the TLS
5. Selected Results
5.1. Method
5.2. Results
6. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
- Task 1On a big ship, among other objects, you can find an anchor. Does it float or sink if we drop it into the sea? Justify your answer.The anchor: floats sinks I do not knowBecause: ………………………………………………………………………………………………………
- Task 2
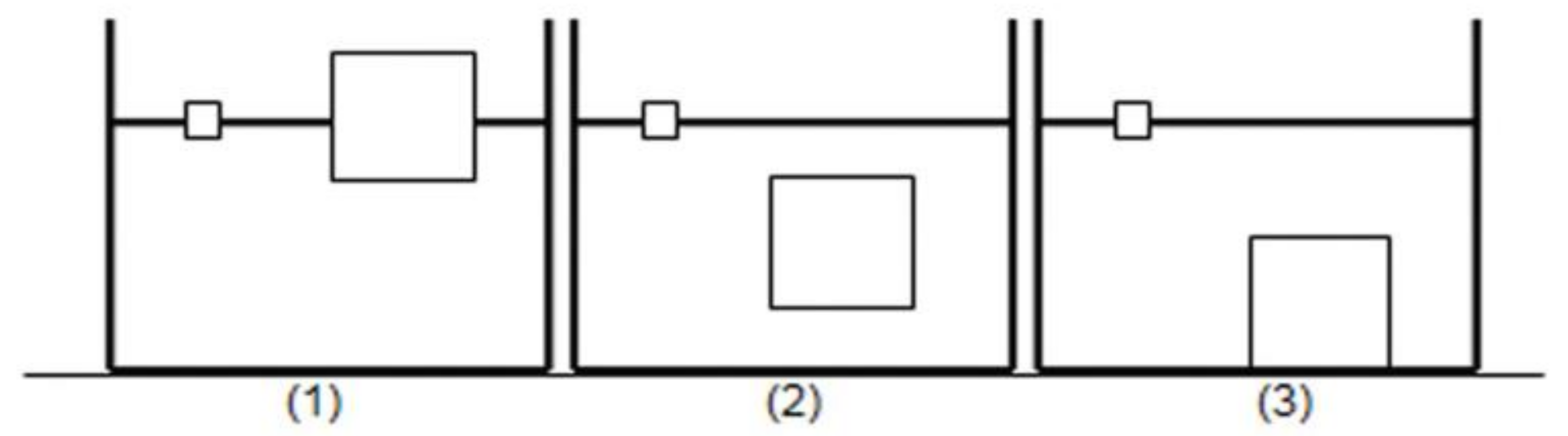
Costas drops a small piece of a particular material into a container filled with water, and he observes that it floats. Afterwards, Irene drops a bigger piece of the same material into the same container. In your opinion, at which point will the big piece stop moving? Circle which number: 1, 2 or 3 in the diagram you think represents the final position of the two bodies that Costas and Irene dropped into the container. Justify your choice.
- Task 3
You are given two objects A and B, and a container which contains a liquid. The densities of the two objects and that of the liquid are given with the “dots-in-a-box” representation, as you can see in the gray box. If you drop objects A and Β into the container with the liquid, what will their final position be? Draw objects A and Β in their final position in the liquid. Justify your answer:
……………………………………………………………………………………………………………………………………………………………………………………………………………… - Task 4
The densities of the two objects and that of the liquid are given with the “dots-in-a-box” representation, as you can see in the gray box. We drop objects A and B into the liquid. Circle which number: 1, 2 or 3 in the diagrams best represents the final positions of the two objects after we have dropped them into the liquid.
Justify your choice:
……………………………………………………………………………………………………………………………………………………………………………………………………………… - Task 5
We drop the two Objects (A) and (B) in the liquid (C). Object (A) floats in the liquid, whereas Object (B) sinks in the liquid. Decide if the following sentence is correct or incorrect:
Object (A) has a greater density than Object (B).It is right It is wrong I don’t knowJustify your choice:……………………………………………………………………………………………………………………………………………………………………………………………………………… - Task 6
While Georgia, Petroula, and Sophia were playing with some toys, one of the toys accidentally fell in the container with the liquid that you can see in the picture. The girls noticed that the toy did not float up towards the surface of the liquid, nor did it sink to the bottom of the container. They wondered what the density of the toy could be, but they disagreed in their opinions:
Georgia says that this object has a greater density than the liquid.Petroula believes that the object has a lower density than the liquid.Sophia says that the object has the same density as the liquid.Which of the girls do you agree with? With:Georgia Petroula Sophia I don’t knowJustify your choice:………………………………………………………………………………………………………………………………………………………………………………………………………………
References
- Joung, Y.J. Children′s typically-perceived-situations of floating and sinking. Int. J. Sci. Educ. 2009, 31, 101–127. [Google Scholar] [CrossRef]
- Psillos, D.; Kariotoglou, P. Teaching Fluids: Intended knowledge and students′ actual conceptual evolution. Int. J. Sci. Educ. 1999, 21, 17–38. [Google Scholar] [CrossRef]
- Kariotoglou, P.; Psillos, D. Teaching and Learning Pressure and Fluids. Fluids 2019, 4, 194. [Google Scholar] [CrossRef] [Green Version]
- Shen, J.; Liu, O.L.; Chang, H.Y. Assessing students′ deep conceptual understanding in physical sciences: An example on sinking and floating. Int. J. Sci. Math. Educ. 2017, 15, 57–70. [Google Scholar] [CrossRef]
- Yin, Y.; Tomita, K.M.; Shavelson, R.J. Diagnosing and dealing with student misconceptions about “Sinking and Floating”. Sci. Scope 2008, 31, 34–39. [Google Scholar]
- Ruiz-Primo, M.A.; Furtak, E.M. Exploring Teachers′ Informal Formative Assessment Practices and Students′ Understanding in the Context of Scientific Inquiry. J. Res. Sci. Teach. 2008, 44, 57–84. [Google Scholar] [CrossRef]
- Méheut, M.; Psillos, D. Teaching-Learning Sequences: Aims and tools for science education research. Int. J. Sci. Educ. 2004, 26, 515–535. [Google Scholar] [CrossRef]
- Psillos, D.; Kariotoglou, P. Iterative Design of Teaching-Learning Sequences: Introducing the Science of Materials in European Schools; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Tiberghien, A.; Vince, J.; Gaidioz, P. Design-based Research: Case of a teaching sequence on mechanics. Int. J. Sci. Educ. 2009, 31, 2275–2314. [Google Scholar] [CrossRef] [Green Version]
- Viennot, L. Physics education research and inquiry-based teaching: A question of didactical consistency. In Designing Theory-Based Teaching-Learning Sequences for Science Education: Proceedings of the Symposium in Honour of Piet Lijnse at the Time of his Retirement as Professor of Physics Didactics at Utrecht University; Kortland, K., Klaassen, K., Eds.; Utrecht University: Utrecht, The Netherlands, 2010. [Google Scholar]
- Chevallard, Y.; Bosch, M. Encyclopedia of Mathematics Education; Lerman, S., Ed.; Springer: Dordrecht, The Netherlands, 2014. [Google Scholar]
- Duit, R. Science education research internationally: Conceptions, research methods, domains of research. Eurasia J. Math. Sci. Technol. Educ. 2007, 3, 3–15. [Google Scholar] [CrossRef]
- Duit, R.; Gropengießer, H.; Kattmann, U.; Komorek, M.; Parchmann, I. The Model of Educational Reconstruction—A Framework for improving teaching and learning science. In Science Education Research and Practice in Europe: Retrospective and Prospective; Jorde, D., Dillon, J., Eds.; Sense Publishers: Rotterdam, Netherlands, 2012; pp. 13–37. [Google Scholar]
- Chinn, C.; Brewer, W. The Role of Anomalous Data in Knowledge Acquisition: A Theoretical Framework and Implications for Science Instruction. Rev. Educ. Res. 1993, 63, 1–49. [Google Scholar] [CrossRef]
- Snir, J. Sink or Float—What do the Experts think? The Historical Development of Explanations for Floatation. Sci. Educ. 1991, 75, 595–609. [Google Scholar] [CrossRef]
- Macbeth, D. On an actual apparatus for conceptual change. Sci. Educ. 2000, 84, 228–264. [Google Scholar] [CrossRef]
- Spyrtou, A.; Zoupidis, A.; Kariotoglou, P. The design and development of an ICT- Enhanced Module concerning density as a property of materials applied in floating-sinking phenomena. Ιn Physics Curriculum Design, Development and Validation; Constantinou, C.P., Papadouris, N., Eds.; University of Cyprus: Nicosia, Cyprus, 2008; pp. 391–407. Available online: http://lsg.ucy.ac.cy/girep2008/papers/THE%20DESIGN%20AND%20DEVELOPMENT%20OF%20AN%20ICT-ENHANCED.pdf (accessed on 20 March 2021).
- Zoupidis, A.; Pnevmatikos, D.; Spyrtou, A.; Kariotoglou, P. The impact of procedural and epistemological knowledge on conceptual understanding: The case of density and floating–sinking phenomena. Instr. Sci. 2016, 44, 315–334. [Google Scholar] [CrossRef]
- Zoupidis, A.; Spyrtou, A.; Malandrakis, G.; Kariotoglou, P. The evolutionary refinement process of a Teaching Learning Sequence for introducing inquiry aspects and density as materials′ property in floating/sinking phenomena. In Iterative Design of Teaching-Learning Sequences; Psillos, D., Kariotoglou, P., Eds.; Springer: Dordrecht, The Netherlands, 2016; pp. 167–199. [Google Scholar]
- Zoupidis, A.; Spyrtou, A.; Pnevmatikos, D.; Kariotoglou, P. Explicitly Linking Simulated with Real Experiments for Conceptual Understanding of Floating/Sinking Phenomena. Themes eLearning 2018, 11, 35–52. [Google Scholar]
- Spyrtou, A.; Lavonen, J.; Zoupidis, A.; Loukomies, A.; Pnevmatikos, D.; Juuti, K.; Kariotoglou, P. Transferring a Teaching Learning Sequence Between Two Different Educational Contexts: The Case of Greece and Finland. Int. J. Sci. Math. Educ. 2018, 16, 443–463. [Google Scholar] [CrossRef] [Green Version]
- Zoupidis, A. Teaching and Learning through the Use of Scientific and Technological Models: The Case of Floating and Sinking Phenomena. Ph.D. Thesis, University of Western Macedonia, School of Education, Florina, Greece, 2012, unpublished work. [Google Scholar]
- Hjalmarson, M.; Lesh, R. Engineering and design research: Intersections for education research and design. In Handbook of Design Research Methods in Education; Baek, J.Y., Lesh, R.A., Eds.; Routledge: Abingdon-on-Thames, UK, 2008; pp. 96–110. [Google Scholar]
- Kelly, A.E.; Lesh, R.; Baek, J. Handbook of Design Research Methods in Education: Innovations in Science, Technology, Mathematics and Engineering Learning and Teaching; Routledge: Abingdon-on-Thames, UK, 2008. [Google Scholar]
- Biddulph, F.; Osborne, R. Pupil′s ideas about floating and sinking. Res. Sci. Educ. 1984, 14, 114–124. [Google Scholar] [CrossRef]
- Smith, C.; Snir, J.; Grosslight, L. Using Conceptual Models to Facilitate Conceptual Change: The Case of Weight-Density Differentiation. Cogn. Instr. 1992, 9, 221–283. [Google Scholar] [CrossRef]
- Kawasaki, K.; Herrenkohl, L.; Yeary, S. Theory Building and modeling in a sinking and floating unit: A case study of third and fourth grade students’ developing epistemologies of science. Int. J. Sci. Educ. 2004, 26, 1299–1324. [Google Scholar] [CrossRef]
- Havu-Nuutinen, S. Examining young children′s conceptual change process in floating and sinking from a social constructivist prospective. Int. J. Sci. Educ. 2005, 27, 259–279. [Google Scholar] [CrossRef]
- Yin, Y.; Tomita, M.K.; Shavelson, R.J. Using Formal Embedded Formative Assessments Aligned with a Short-Term Learning Progression to Promote Conceptual Change and Achievement in Science. Int. J. Sci. Educ. 2014, 36, 531–552. [Google Scholar] [CrossRef]
- Fassoulopoulos, G.; Kariotoglou, P.; Koumaras, P. Consistent and Inconsistent Pupil′s Reasoning about Intensive Quantities, The Case of Density and Pressure. Res. Sci. Educ. 2003, 33, 71–87. Available online: http://www.ingentaconnect.com/content/klu/rise/ (accessed on 20 March 2021). [CrossRef]
- Perkins, D.N.; Grotzer, T.A. Dimensions of causal understanding: The role of complex causal models in students’ understanding of science. Stud. Sci. Educ. 2005, 41, 117–166. [Google Scholar] [CrossRef]
- Hardy, I.; Jonen, A.; Moeller, K.; Stern, E. Effects of Instructional Support Within Constructivist Learning Environments for Elementary School Students′ Understanding of “Floating and Sinking”. J. Educ. Psychol. 2006, 98, 307–326. [Google Scholar] [CrossRef] [Green Version]
- Wiser, M.; Smith, C. Learning and teaching about matter in grades K-8: When should the atomic-molecular theory be introduced? In International Handbook of Research on Conceptual Change; Vosniadou, S., Ed.; Routledge: Abingdon-on-Thames, UK, 2008; pp. 205–239. [Google Scholar]
- Rowell, J.; Dawson, C. Teaching about Floating and Sinking: An Attempt to Link Cognitive Psychology with Classroom Practice. Sci. Educ. 1977, 61, 245–253. [Google Scholar] [CrossRef]
- Smith, C.; Maclin, D.; Grosslight, L.; Davis, H. Teaching for understanding: A study of students′ preinstruction theories of matter and a comparison of the effectiveness of two approaches to teaching students about matter and density. Cogn. Instr. 1997, 15, 317–393. [Google Scholar]
- Xu, L.; Clarke, D. Student difficulties in learning density: A Distributed Cognition Perspective. Res. Sci. Educ. 2011, 42, 769–789. [Google Scholar] [CrossRef]
- Vosniadou, S.; Vamvakoussi, X.; Skopeliti, I. The framework theory approach to the problem of conceptual change. In International Handbook of Research on Conceptual Change; Vosniadou, S., Ed.; Routledge: Abingdon-on-Thames, UK, 2008; pp. 205–239. [Google Scholar]
- Radovanović, J.; Sliško, J.; Stepanović Ilić, I. Active learning of buoyancy: An effective way to change students’ alternative conceptions about floating and sinking. J. Phys. Conf. Ser. 2019, 1286, 012011. [Google Scholar] [CrossRef]
- Bonera, G. Distinguished papers series: Signor galileo galilei′s scales. Int. J. Sci. Educ. 1996, 18, 881–887. [Google Scholar] [CrossRef]
- Galili, I. Weight versus gravitational force: Historical and educational perspectives. Int. J. Sci. Educ. 2001, 23, 1073–1093. [Google Scholar] [CrossRef]
- Bormashenko, E. Surface tension supported floating of heavy objects: Why elongated bodies float better? J. Colloid Interface Sci. 2016, 463, 8–12. [Google Scholar] [CrossRef] [Green Version]
- Inhelder, B.; Piaget, J. The Growth of Logical Thinking from Childhood to Adolescent; Psychology Press: London, UK, 1958. [Google Scholar]
- Hewson, M. The Acquisition of Scientific Knowledge: Analysis and Representation of Student Conceptions Concerning Density. Sci. Educ. 1986, 70, 159–170. [Google Scholar] [CrossRef]
- Strauss, S.; Globerson, T.; Mintz, R. The influence of training for the atomistic of the development of the density concept among gifted and non gifted children. J. Appl. Dev. Psychol. 1983, 4, 125–147. [Google Scholar] [CrossRef]
- National Research Council. A framework for K-12 Science Education: Practices, Crosscutting Concepts, and Core Ideas; The National Academies Press: Washington, DC, USA, 2012. [Google Scholar]
- NGSS Lead States. Next Generation Science Standards: For States, by States; The National Academies Press: Washington, DC, USA, 2013. [Google Scholar]
- Couso, D. Participatory approaches to curriculum design from a design research perspective. In Iterative Design of Teaching-Learning Sequences; Psillos, D., Kariotoglou, P., Eds.; Springer: Dordrecht, The Netherlands, 2016; pp. 47–71. [Google Scholar]
- Krajcik, J. Supporting Science Learning in Context: Project-Based Learning. In Portable Technologies: Science Learning in Context; Tinker, R., Krajcik, J., Eds.; Kluwer Academic/Plenum Publishers: New York, NY, USA, 2001; p. 92. [Google Scholar]
- Benett, J.; Lubben, F.; Hogarth, S. Bringing science to life: A synthesis of the research evidence on the effects of context-based and STS approaches to science teaching. Sci. Educ. 2007, 91, 347–370. [Google Scholar] [CrossRef]
- Waight, N.; Abd-El-Khalick, F. The impact of technology on the enactment of ‘‘inquiry’’ in a technology enthusiast′s sixth grade science classroom. J. Res. Sci. Teach. 2007, 44, 154–182. [Google Scholar] [CrossRef]
- Abd-El-Khalick, F.; BouJaoude, S.; Duschl, R.A.; Hofstein, A.; Lederman, N.G.; Mamlok, R.; Niaz, M.; Treagust, D.; Tuan, H. Inquiry in science education: International perspectives. Sci. Educ. 2004, 88, 397–419. [Google Scholar] [CrossRef]
- Bybee, R.W. Scientific inquiry and science teaching. In Scientific Inquiry and Nature of Science; Flick, L.B., Lederman, N.G., Eds.; Springer: Dordrecht, The Netherlands, 2006; pp. 1–14. [Google Scholar]
- Taber, K. Progressing Science Education. Constructing the Scientific Research Programme into the Contingent Nature of Learning; Springer: New York, NY, USA, 2009; Volume 45, p. 310. [Google Scholar]
- Chamberlain, K.; Crane, C. Reading, Writing & Inquiry in the Science Classroom; Corwin Press: Thousand Oaks, CA, USA, 2009. [Google Scholar]
- Boudreaux, A.; Shaffer, P.; Heron, P.; McDermott, L. Student understanding of control of variables: Deciding whether or not a variable influences the behavior of a system. Am. J. Phys. 2008, 76, 163–170. [Google Scholar] [CrossRef]
- Treagust, D.F.; Chittleborough, G.; Mamiala, L.T. Students′ understanding of the role of scientific models in learning science. Int. J. Sci. Educ. 2002, 24, 357–368. [Google Scholar] [CrossRef]
- White, R.; Gunstone, R. Probing Understanding, 1st ed.; Routledge: Abingdon-on-Thames, UK, 1992. [Google Scholar] [CrossRef]


| Unit | Main Aim | Content | Activities |
|---|---|---|---|
| 1st | Familiarization phase through technological problem. | Technological problem of lifting shipwreck. Distinction of variables that possibly influence FS. Difference between solid and hollow objects. | Groups and classroom discussion. POE activities. Demonstration of experiments. |
| 2nd | Test variables that influence FS. | FS of solid objects influenced both by the material of object and liquid. FS not influenced by other variables, e.g., weight of object. | Groups and classroom discussion. POE activities with gradual increase in openness. |
| 3rd | Introduction of “dots-in-a-box” representation of density. Use of causal relational FS rule for solid objects in water. | “Dots-in-a-box” representation describes “heavier-lighter” relationship between different materials. Compare “dots-in-a-box” representations for solid objects and water to decide objects’ FS in water. | Groups and classroom discussion. |
| 4th | Generalization of causal relational FS rule. “Dots-in-a-box” renamed “density”. | Density of a two-material composite object lies between the densities of these two materials. Compare composite object’s and liquid’s densities to decide object’s FS in a liquid. | Groups and classroom discussion. POE activities. |
| 5th | Lifting shipwreck. | Implementing generalized causal relational FS rule within the technological framework. | Groups and classroom discussion. |
| Pre | Post | Delayed Post | ||||
|---|---|---|---|---|---|---|
| M | SD | M | SD | M | SD | |
| Task 1 | 1.10 | 0.664 | 1.78 | 0.936 | 1.68 | 0.879 |
| Task 2 | 0.78 | 0.613 | 1.39 | 0.862 | 1.37 | 0.859 |
| Task 3 | - | - | 0.63 | 0.488 | 0.56 | 0.502 |
| Task 4 | - | - | 0.73 | 0.449 | 0.54 | 0.505 |
| Task 5 | - | - | 0.44 | 0.502 | 0.44 | 0.502 |
| Task 6 | - | - | 0.51 | 0.506 | 0.46 | 0.505 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zoupidis, A.; Spyrtou, A.; Pnevmatikos, D.; Kariotoglou, P. Teaching and Learning Floating and Sinking: Didactic Transformation in a Density-Based Approach. Fluids 2021, 6, 158. https://doi.org/10.3390/fluids6040158
Zoupidis A, Spyrtou A, Pnevmatikos D, Kariotoglou P. Teaching and Learning Floating and Sinking: Didactic Transformation in a Density-Based Approach. Fluids. 2021; 6(4):158. https://doi.org/10.3390/fluids6040158
Chicago/Turabian StyleZoupidis, Anastasios, Anna Spyrtou, Dimitrios Pnevmatikos, and Petros Kariotoglou. 2021. "Teaching and Learning Floating and Sinking: Didactic Transformation in a Density-Based Approach" Fluids 6, no. 4: 158. https://doi.org/10.3390/fluids6040158
APA StyleZoupidis, A., Spyrtou, A., Pnevmatikos, D., & Kariotoglou, P. (2021). Teaching and Learning Floating and Sinking: Didactic Transformation in a Density-Based Approach. Fluids, 6(4), 158. https://doi.org/10.3390/fluids6040158