Approximate Analytical Models of Shock-Wave Structure at Steady Mach Reflection
Abstract
:1. Introduction
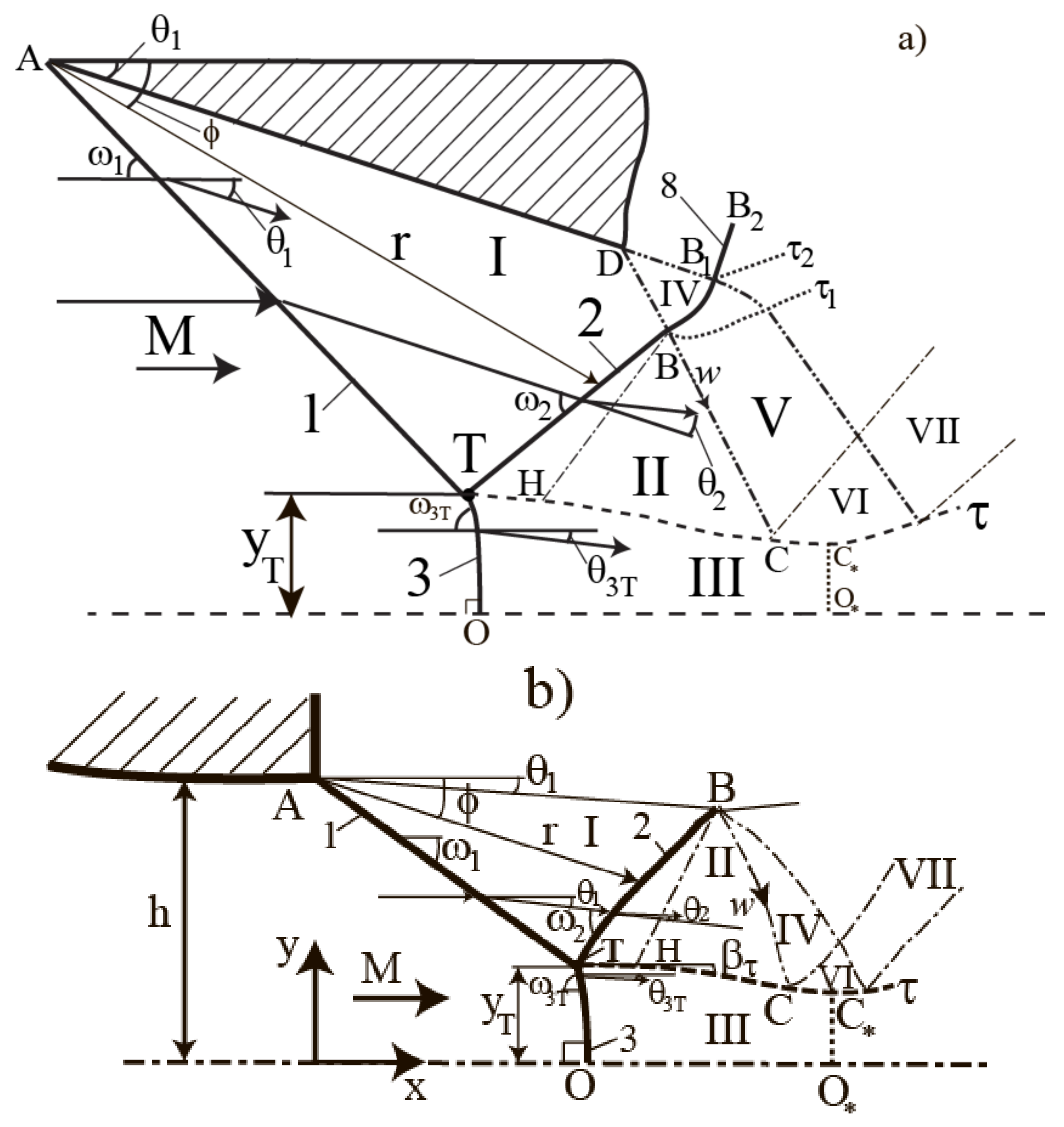
2. Model and Methods
2.1. Triple Point Problem
2.2. “Virtual Nozzle” below the Slipstream
2.3. Approximation of Flow Downstream the Reflected Shock
- -
- the angle of inclination of the slipstream to the horizontal plane is permanently equal to (5);
- -
- the inclination angle of the reflected shock is equal to ;
- -
- the angle of inclination of the first incident characteristic can be taken as (in point B), or , or any value averaged between above-mentioned ones. Here, is the Mach angle, and is the flow Mach number that should be in the critical point above the slipstream . According to static pressure equality on the slipstream, that Mach number can be calculated from the relation .
- -
- the contact discontinuity is really the curvilinear one. Its slope angle is variable, and it is rather small initially (at the triple point). Therefore, an error in the slipstream slope angle estimation influences the estimation of length and width of the subsonic pocket crucially. Let us imagine, for example, a variation of the slipstream slope angle from to in point . If we consider it constant, we mistake the length and width of the subsonic pocket several times. Therefore, neglecting slope angle variation leads to very large underestimation of the Mach stem size;
- -
- the turn of the slipstream influenced by the incident expansion wave IV or V occurs not instantaneously but along the finite sector . As we neglect the length of the sector , it also leads to sufficient discrepancies.
2.4. Reflected Shock Curvature
2.5. Interaction of the Reflected Shock with the Rear Expansion Fan
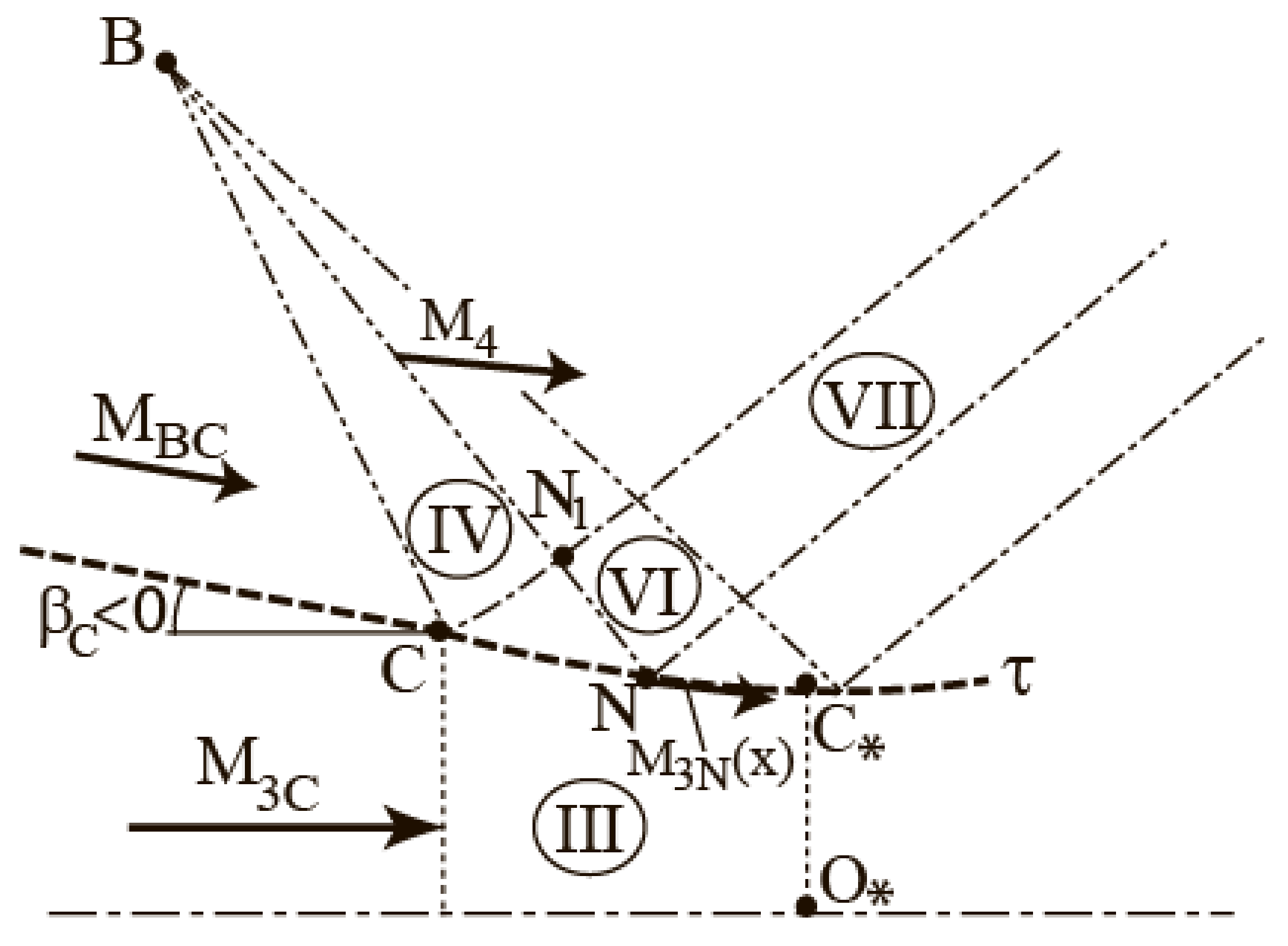
2.6. Expansion Wave Incidence and Reflection from the Slipstream
2.7. General Algorithm
- Values of , , and are given. The problem of Mach reflection of the shock 1 is decided using the triple-shock theory (1–8). In this way, the strengths and of the reflected shock and the Mach one in the vicinity of the triple point, as well as Mach numbers and downstream of those shocks, and the initial inclination angle of the slipstream are to be determined.
- Some initial estimation value of (Mach stem height at the first iteration) is to be appointed (pre-estimated).
- The shape of the reflected shock at sector can be determined by Equations (18) and (19). Those equations are to be integrated until the overexpanded jet boundary or the point of shock intersection with the first characteristic of the tail expansion fan (i.e., till the point B). The flow Mach number and flow direction angle after the shock in point B are to be calculated.
- Equations (13)–(16), which determine the shape of the slipstream at its sector (as well as flow features on both its sides), are to be integrated. Equation (17), which determine the shape of the first incident characteristic (and flow features along it), are to be decided simultaneously until point of the intersection of the incident characteristic with the slipstream.
- Only in the problem of the flow between two wedges, we need to decide the problem of the oblique shock interaction with the expansion fan. Applying the methods elaborated by Li and Ben-Dor [63] or by Meshkov and Omelchenko [64], we restore the shape of the interacting shock and flow parameters in the refracted expansion wave V.
- Flow characteristics at the first border characteristic of the incident expansion fan are to be averaged. Equations (20)–(25) determine the shape of the slipstream at its sector . We solve this equation until one of two following conditions would not be fulfilled: full horizontal turn of the slipstream () or increase in the flow velocity of its lower side to the critical value (). In the first case, the current Mach stem height is considered as too large; at second case, it is considered as too small.
- With the above-mentioned conclusion about the value of , we correct the proposing Mach stem height and return to point 3. The result of the last iteration (when achieving enough accuracy) is considered the final one.
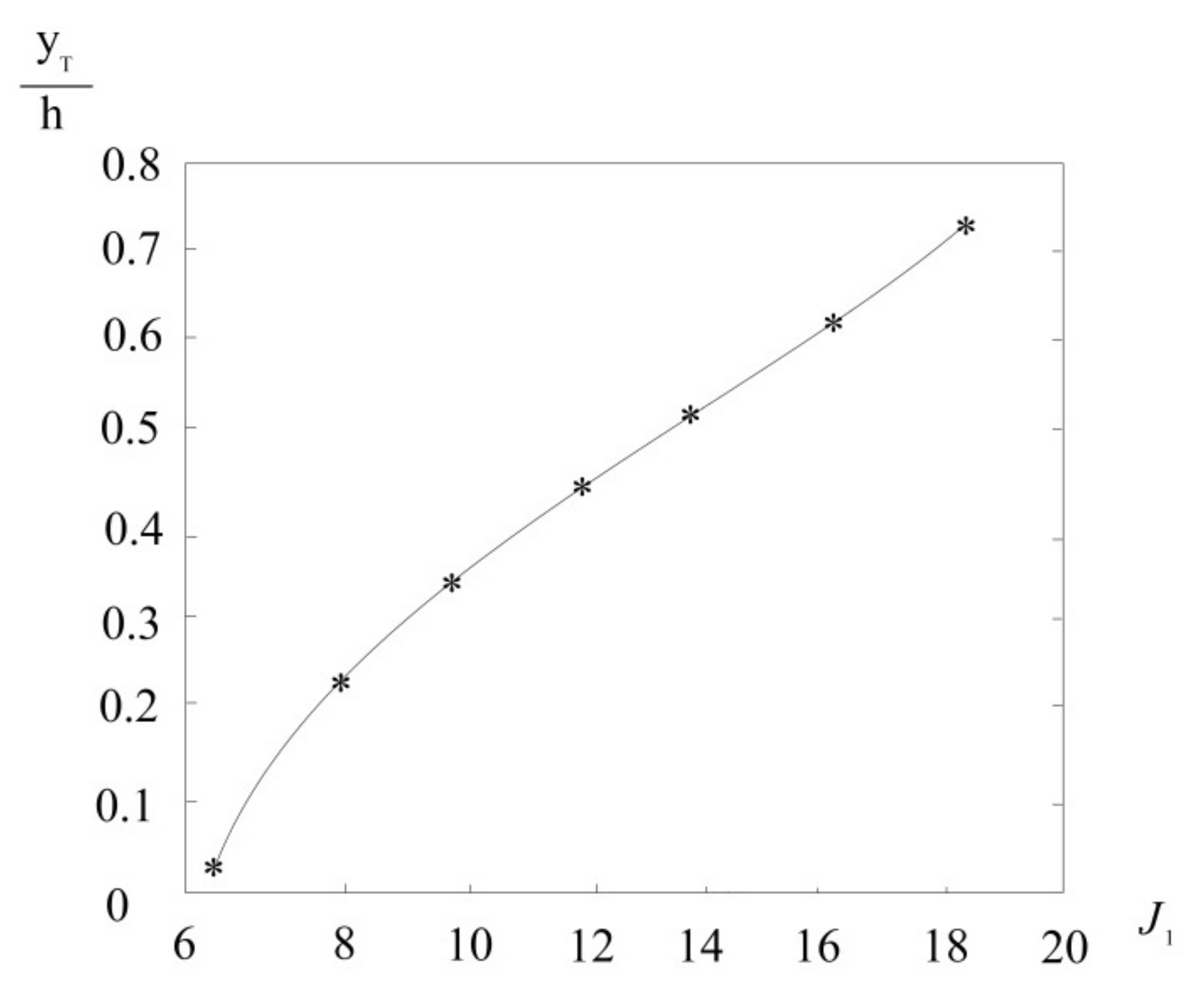
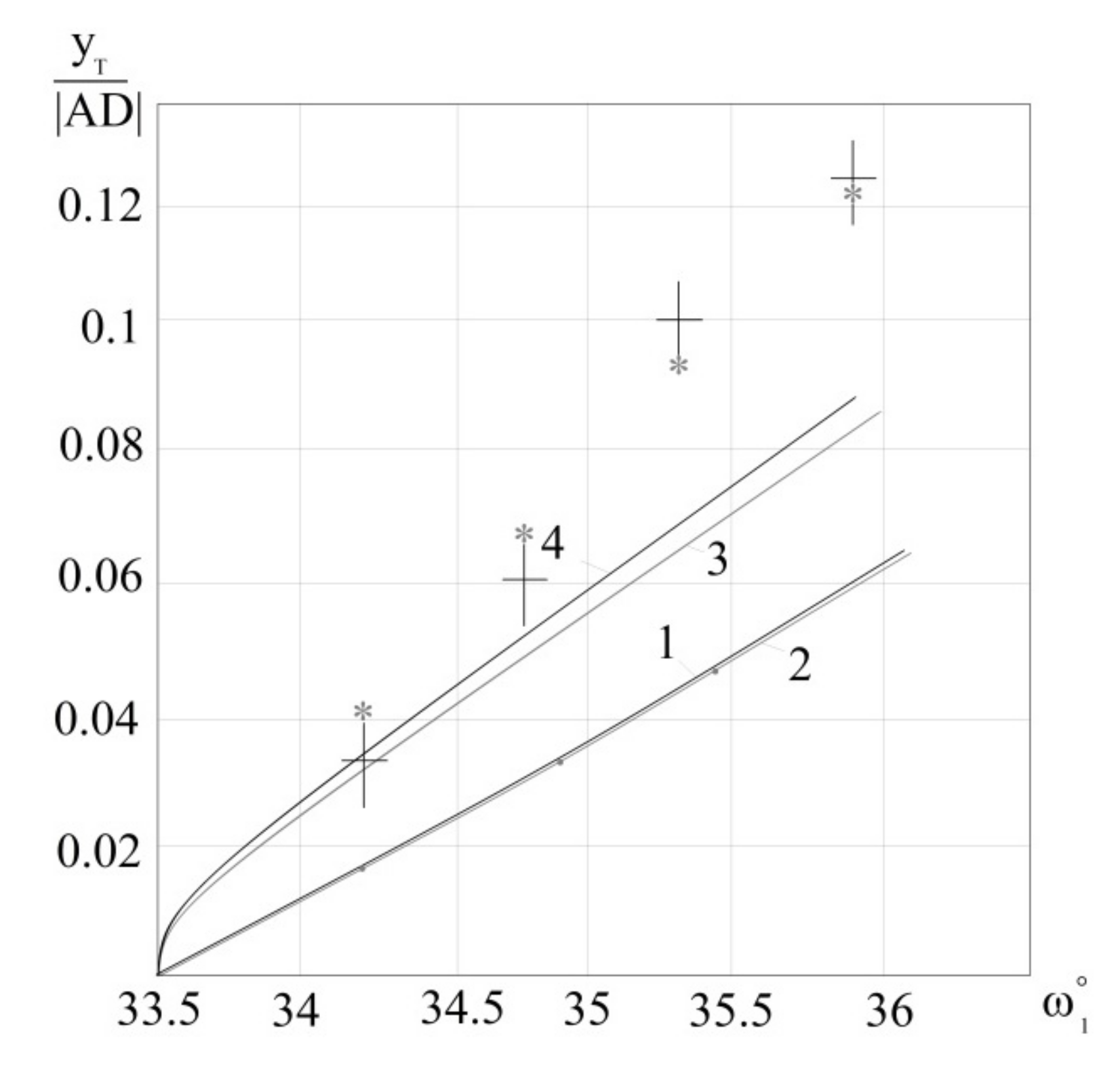
3. Results
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Mach, E. Über den verlauf von Funkenwellen in der Ebene und im Raume. Sitzungsbr. Akad. Wiss. Wien. 1878, 78, 819–838. (In German) [Google Scholar]
- Reichenbach, H. Contribution of Ernst Mach to fluid mechanics. Annu. Rev. Fluid Mech. 1983, 15, 1–28. [Google Scholar] [CrossRef] [Green Version]
- Pack, D.C. The reflection and diffraction of shock waves. J. Fluid Mech. 1964, 18, 549–576. [Google Scholar] [CrossRef]
- Griffith, W.C. Shock waves. J. Fluid Mech. 1981, 106, 81–101. [Google Scholar] [CrossRef]
- Hornung, H.G. Regular and Mach reflection of shock waves. Annu. Rev. Fluid Mech. 1986, 18, 33–58. [Google Scholar] [CrossRef]
- Ben-Dor, G. Shock Wave Reflection Phenomena; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 2007; 342p. [Google Scholar]
- Von Neumann, J. Oblique Reflection of Shock Waves; Collecttion Works; Pergamon Press: London, UK, 1963; Volume 6, pp. 238–299. [Google Scholar]
- Courant, R.; Friedrichs, K.O. Supersonic Flow and Shock Waves; Springer: New York, NY, USA, 1976; 464p. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Course of Theoretical Physics; Fluid Mechanics; Pergamon Press: Oxford, UK, 1987; Volume 6, 552p. [Google Scholar]
- Zel’dovich, Y.B.; Raizer, Y.P. Physics of Shock Waves and High-Temperature Hydrodynamic Phenomena; Academic Press: New York, NY, USA, 1966; Volume I, 464p. [Google Scholar]
- Melnikov, D.A. Reflections of shock waves from the axis of symmetry. J. USSR Acad. Science. Mech. Mech. Eng. 1962, 3, 24–30. (In Russian) [Google Scholar]
- Hornung, H.G.; Oertel, H.; Sandeman, R.J. Transition to Mach reflexion of shock waves in steady and pseudosteady flow with and without relaxation. J. Fluid Mech. 1979, 90, 541–560. [Google Scholar] [CrossRef] [Green Version]
- Hornung, H.G.; Robinson, M.I. Transition from regular to Mach reflection of shock waves. Part 2: The steady-flow criterion. J. Fluid Mech. 1982, 123, 155–164. [Google Scholar] [CrossRef]
- Chpoun, A.; Ben-Dor, G. Numerical confirmation of the hysteresis phenomenon in the regular to Mach reflection transition in steady flows. Shock Waves 1995, 5, 199–204. [Google Scholar] [CrossRef]
- Ivanov, M.S.; Gimelshein, S.F.; Beylich, A.E. Hysteresis effect in stationary reflection of shock waves. Phys. Fluids 1995, 7, 685–687. [Google Scholar] [CrossRef]
- Shimshi, E.; Ben-Dor, G.; Levy, A. Viscous simulation of shock-reflection hysteresis in overexpanded planar nozzles. J. Fluid Mech. 2009, 635, 189–206. [Google Scholar] [CrossRef]
- Adrianov, A.L.; Starykh, A.L.; Uskov, V.N. Interference of Stationary Gasodynamic Discontinuities; Nauka: Novosibirsk, Russia, 1995; 180p. (In Russian) [Google Scholar]
- Uskov, V.N.; Chernyshov, M.V. Special and extreme triple shock-wave configurations. J. Appl. Mech. Tech. Phys. 2006, 47, 492–504. [Google Scholar] [CrossRef]
- Chernyshov, M.V. Extreme Triple Configurations with Negative Slope Angle of the Reflected Shock. Russ. Aeronaut. 2019, 62, 259–266. [Google Scholar] [CrossRef]
- Shoev, G.V.; Ivanov, M.S. Numerical study of shock wave interaction in steady flows of a viscous heat-conducting gas with a low ratio of specific heats. Thermophys. Aeromech. 2016, 23, 343–364. [Google Scholar] [CrossRef]
- Gvozdeva, L.G.; Silnikov, M.V.; Gavrenkov, S.A. Triple shock configurations with negative angle of reflection. Acta Astronaut. 2015, 116, 36–42. [Google Scholar] [CrossRef]
- Gavrenkov, S.A.; Gvozdeva, L.G. Numerical investigation of the onset of instability of triple shock configurations in steady supersonic gas flows. Tech. Phys. Lett. 2012, 38, 587–589. [Google Scholar] [CrossRef]
- Chernyshov, M.V.; Kapralova, A.S.; Savelova, K.E. Ambiguity of solution for triple configurations of stationary shocks with negative reflection angle. Acta Astronaut. 2021, 179, 382–390. [Google Scholar] [CrossRef]
- Tao, Y.; Liu, W.; Fan, X.; Xiong, B.; Yu, J.; Sun, M. A study of the asymmetric shock reflection configurations in steady flows. J. Fluid Mech. 2017, 825, 1–15. [Google Scholar] [CrossRef]
- Lin, J.; Bai, C.-Y.; Wu, Z.-N. Study of asymmetrical shock wave reflection in steady supersonic flow. J. Fluid Mech. 2019, 864, 848–875. [Google Scholar] [CrossRef]
- Roy, S.; Gopalapillai, R. An analytical model for asymmetric Mach reflection configuration in steady flows. J. Fluid Mech. 2019, 863, 242–268. [Google Scholar] [CrossRef]
- Gounko, Y.P. Patterns of steady axisymmetric supersonic compression flows with a Mach disk. Shock Waves 2017, 27, 495–506. [Google Scholar] [CrossRef] [Green Version]
- Choe, S.-G. A method for predicting Mach stem height in steady flows. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2021. Available online: https://doi.org/10.1177/09544100211001518 (accessed on 27 March 2021). (in “OnlineFirst” now). [CrossRef]
- Bulat, P.V.; Volkov, K.N. Detonation jet engine. Part II–Construction features. Int. J. Environ. Sci. Educ. 2016, 11, 5020–5033. [Google Scholar]
- Ivanov, M.S.; Kudrjavtsev, A.N.; Trotsjuk, A.V.; Fomin, V.M. Method of Organization of Detonation Combustion Chamber of Supersonic Ramjet. Engine. Patent RU 2285143 C2, 10 December 2004. (In Russian). [Google Scholar]
- Chernyshov, M.V.; Murzina, K.E.; Matveev, S.A.; Yakovlev, V.V. Shock-wave structures of prospective combined ramjet engine. IOP Conf. Ser. Mater. Sci. Eng. 2019, 618, 012068. [Google Scholar] [CrossRef]
- Savelova, K.E.; Alekseeva, M.M.; Matveev, S.A.; Chernyshov, M.V. Shock-wave structures of prospective combined jet engine. J. Phys. Conf. Ser. 2021, 1959, 012043. [Google Scholar] [CrossRef]
- Prakash, A.; Parsons, N.; Wang, X.; Zhong, X. High-order shock-fitting methods for direct numerical simulation of hypersonic flow with chemical and thermal nonequilibrium. J. Comput. Phys. 2011, 230, 8474–8507. [Google Scholar] [CrossRef]
- Pepe, R.; Bonfiglioli, A.; D’Angola, A.; Colonna, G.; Paciorri, R. An unstructured shock-fitting solver for hypersonic plasma flows in chemical non-equilibrium. Comput. Phys. Commun. 2015, 196, 179–193. [Google Scholar] [CrossRef]
- Li, H.; Ben-Dor, G.; Grönig, H. Analytical Study of the Oblique Reflection of Detonation Waves. AIAA J. 1997, 35, 1712–1720. [Google Scholar] [CrossRef]
- Medvedev, A.E. Reflection of an oblique shock wave in a reacting gas with a finite relaxation-zone length. J. Appl. Mech. Tech. Phys. 2001, 42, 211–218. [Google Scholar] [CrossRef]
- Godunov, S.K.; Zabrodin, A.V.; Ivanov, M.Y.; Kraiko, A.N.; Prokopov, G.P. Numerical Solution of Multidimensional Problems of Gas Dynamics; Nauka: Moscow, Russia, 1976; 400p. (In Russian) [Google Scholar]
- Kozhemyakin, A.O.; Omel’chenko, A.V.; Uskov, V.N. Oblique interaction of supersonic flows. Fluid Dyn. 1999, 34, 551–557. [Google Scholar]
- Chernyshov, M.V.; Tolpegin, O.A. Optimal regular reflection of oblique shocks. Acta Astronaut. 2019, 163, 225–231. [Google Scholar] [CrossRef]
- Henderson, L.F. On the confluence of three shock waves in a perfect gas. Aeronaut. Q. 1964, 15, 181–197. [Google Scholar] [CrossRef]
- Hekiri, H.; Emanuel, G. Shock wave triple point morphology. Shock Waves 2011, 21, 511–521. [Google Scholar] [CrossRef]
- Hekiri, H.; Emanuel, G. Structure and morphology of a triple point. Phys. Fluids 2015, 27, 056102. [Google Scholar] [CrossRef]
- Azevedo, D.J.; Liu, C.S. Engineering approach to the prediction of shock patterns in bounded high-speed flows. AIAA J. 1993, 31, 83–90. [Google Scholar] [CrossRef]
- Medvedev, A.E.; Fomin, V.M. Approximate analytical calculation of the Mach configuration of steady shock waves in a plane constricting channel. J. Appl. Mech. Tech. Phys. 1998, 39, 369–374. [Google Scholar] [CrossRef]
- Omel’chenko, A.V.; Uskov, V.N.; Chernyshev, M.V. An Approximate Analytical Model of Flow in the First Barrel of an Overexpanded Jet. Tech. Phys. Lett. 2003, 29, 243–245. [Google Scholar] [CrossRef]
- Ben-Dor, G.; Takayama, K. The phenomena of shock wave reflection—A review of unsolved problems and future research needs. Shock Waves 1992, 2, 211–223. [Google Scholar] [CrossRef]
- Chanetz, B.; Chernyshev, M.V.; Durand, A.; Ivanov, M.S.; Khotyanovsky, D.V.; Kudryavtsev, A.N.; Omelchenko, A.V.; Uskov, V.N. Analytical, Numerical, and Experimental Investigation of Shock Wave Reflection Transition Induced by Variation of Distance between Wedges, Proceedings of West East High Speed Flow Fields 2002 Conference, Marseille, France, 22–26 April 2002; CIMNE: Barcelona, Spain, 2003; pp. 274–281. [Google Scholar]
- Hadjadj, A.; Kudryavtsev, A.N.; Ivanov, M.S. Numerical Investigation of Shock-Reflection Phenomena in Overexpanded Supersonic Jets. AIAA J. 2004, 42, 570–577. [Google Scholar] [CrossRef]
- Avduevsky, V.S.; Ashratov, E.A.; Pirumov, U.G. Supersonic Non-Isobaric Gas Jets; Mashinostroenie: Moscow, Russia, 1985; 248p. (In Russian) [Google Scholar]
- Sedov, L.I.; Cherny, G.G. On averaging of non-uniform gas streams in channels. Theor. Hydromech. 1954, 12, 27–45. (In Russian) [Google Scholar]
- Yuan, T.; Xiaoqiang, F.; Yilong, Z. Flow visualization for the evolution of the slipstream in steady shock reflection. J. Vis. 2014, 18, 21–24. [Google Scholar]
- Grib, A.A.; Ryabinin, A.G. Approximate integration of the equations of steady supersonic gas flow. Dokl. Akad. Nauk SSSR 1955, 100, 425–428. (in Russian). [Google Scholar]
- Chernyshov, M.V. Interaction of Elements of Shock-Wave Systems between Themselves and with Various Surfaces. Ph.D. Thesis, Saint Petersburg State University, Saint Petersburg, Russia, 2002; 173p. (In Russian). [Google Scholar]
- Thomas, T.Y. First approximation of pressure distribution on curved profiles at supersonic speeds. Proc. Natl. Acad. Sci. USA 1949, 35, 617–627. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Meyer, R.E. On supersonic flow behind a curved shock. Q. Appl. Math. 1957, 14, 433–436. [Google Scholar] [CrossRef] [Green Version]
- Cherny, G.G. Gas Flows with Large Supersonic Velocity; FIZMATGIZ: Moscow, Russia, 1959; 220p. (In Russian) [Google Scholar]
- Bai, C.-Y.; Wu, Z.-N. Size and shape of shock waves and slipline for Mach reflection in steady flow. J. Fluid Mech. 2017, 818, 116–140. [Google Scholar] [CrossRef]
- Silnikov, M.V.; Chernyshov, M.V. The interaction of Prandtl-Meyer wave and quasi-one-dimensional flow region. Acta Astronaut. 2015, 109, 248–253. [Google Scholar] [CrossRef]
- Alekseeva, M.M.; Matveev, S.A.; Savelova, K.E.; Chernyshov, M.V. Interaction of Steady Gasodynamic Discontinuities and Waves on Supersonic Gas Jet Flows from Rocket Engines. AIP Conf. Proc. 2021, 2318, 060004. [Google Scholar]
- Averenkova, G.I.; Ashratov, E.A.; Volkonskaya, T.G. Supersonic Jets of Ideal Gas; Part 2; Moscow State University: Moscow, Russia, 1971; 170p. (In Russian) [Google Scholar]
- Li, H.; Ben-Dor, G. A parametric study of Mach reflection in steady flows. J. Fluid Mech. 1997, 341, 101–125. [Google Scholar] [CrossRef]
- Schotz, M.; Levy, A.; Ben-Dor, G.; Igra, O. Analytical prediction of the wave configuration size in steady Mach reflection. Shock Waves 1997, 7, 363–372. [Google Scholar] [CrossRef]
- Li, H.; Ben-Dor, G. Oblique Shock–Expansion Fan Interaction–Analytical Solution. AIAA J. 1996, 43, 418–421. [Google Scholar] [CrossRef]
- Meshkov, V.R.; Omelchenko, A.V.; Uskov, V.N. The interaction of the stationary shock with the expansion wave of opposite direction. Vestn. St. Petersburg Univ. Math. 2002, 2, 99–106. (in Russian). [Google Scholar]
- Silnikov, M.V.; Chernyshov, M.V.; Uskov, V.N. Analytical solutions for Prandtl-Meyer wave–oblique shock overtaking interaction. Acta Astronaut. 2014, 99, 175–183. [Google Scholar] [CrossRef]
- Silnikov, M.V.; Chernyshov, M.V. Supersonic flow gradients at an overexpanded nozzle lip. Shock Waves 2018, 28, 765–784. [Google Scholar] [CrossRef]
- Omel’chenko, A.V. Relation between derivatives across a strong discontinuity. Comput. Math. Math. Phys. 2002, 42, 1200–1211. [Google Scholar]

| ω1,° | 31 | 35 | 39 | 43 | 47 | 51 | 55 | 59 |
|---|---|---|---|---|---|---|---|---|
| , proposed method | 0.046 | 0.243 | 0.363 | 0.455 | 0.532 | 0.602 | 0.673 | 0.753 |
| , method of characteristics | 0.046 | 0.245 | 0.364 | 0.457 | 0.536 | 0.607 | 0.677 | 0.756 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chernyshov, M.V.; Savelova, K.E.; Kapralova, A.S. Approximate Analytical Models of Shock-Wave Structure at Steady Mach Reflection. Fluids 2021, 6, 305. https://doi.org/10.3390/fluids6090305
Chernyshov MV, Savelova KE, Kapralova AS. Approximate Analytical Models of Shock-Wave Structure at Steady Mach Reflection. Fluids. 2021; 6(9):305. https://doi.org/10.3390/fluids6090305
Chicago/Turabian StyleChernyshov, Mikhail V., Karina E. Savelova, and Anna S. Kapralova. 2021. "Approximate Analytical Models of Shock-Wave Structure at Steady Mach Reflection" Fluids 6, no. 9: 305. https://doi.org/10.3390/fluids6090305






