Modulation of Giant Magnetoimpedance Effect in Co-Based Amorphous Wires by Carbon-Based Nanocoatings
Abstract
1. Introduction
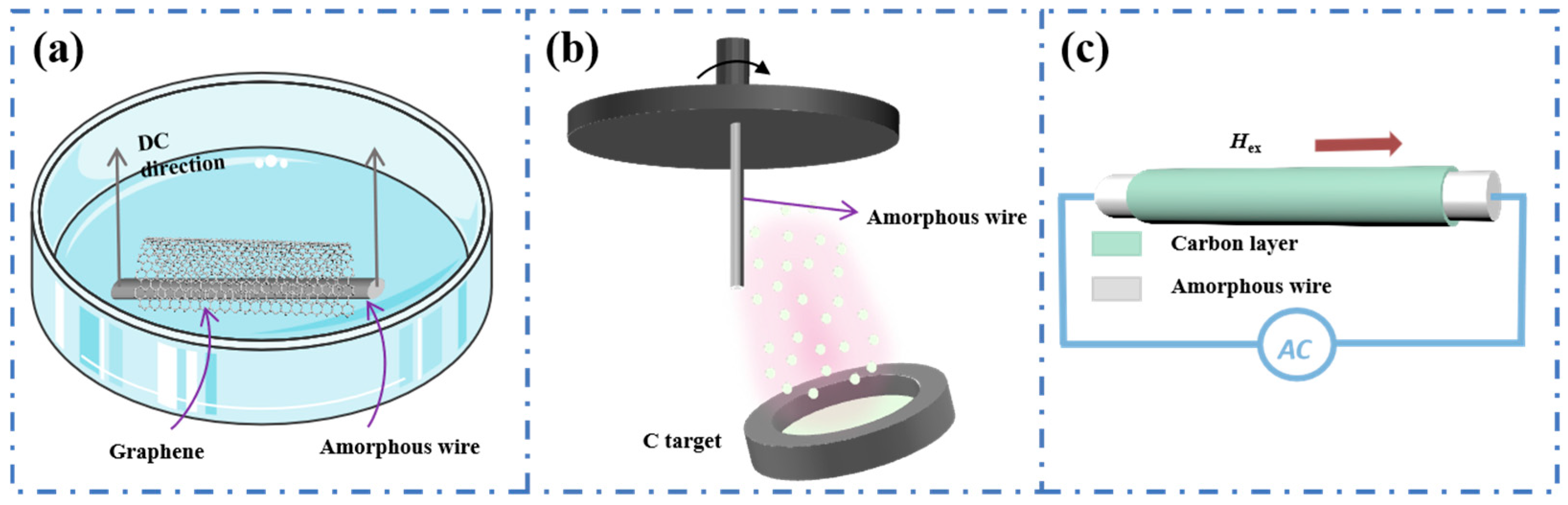
2. Materials and Methods
3. Results
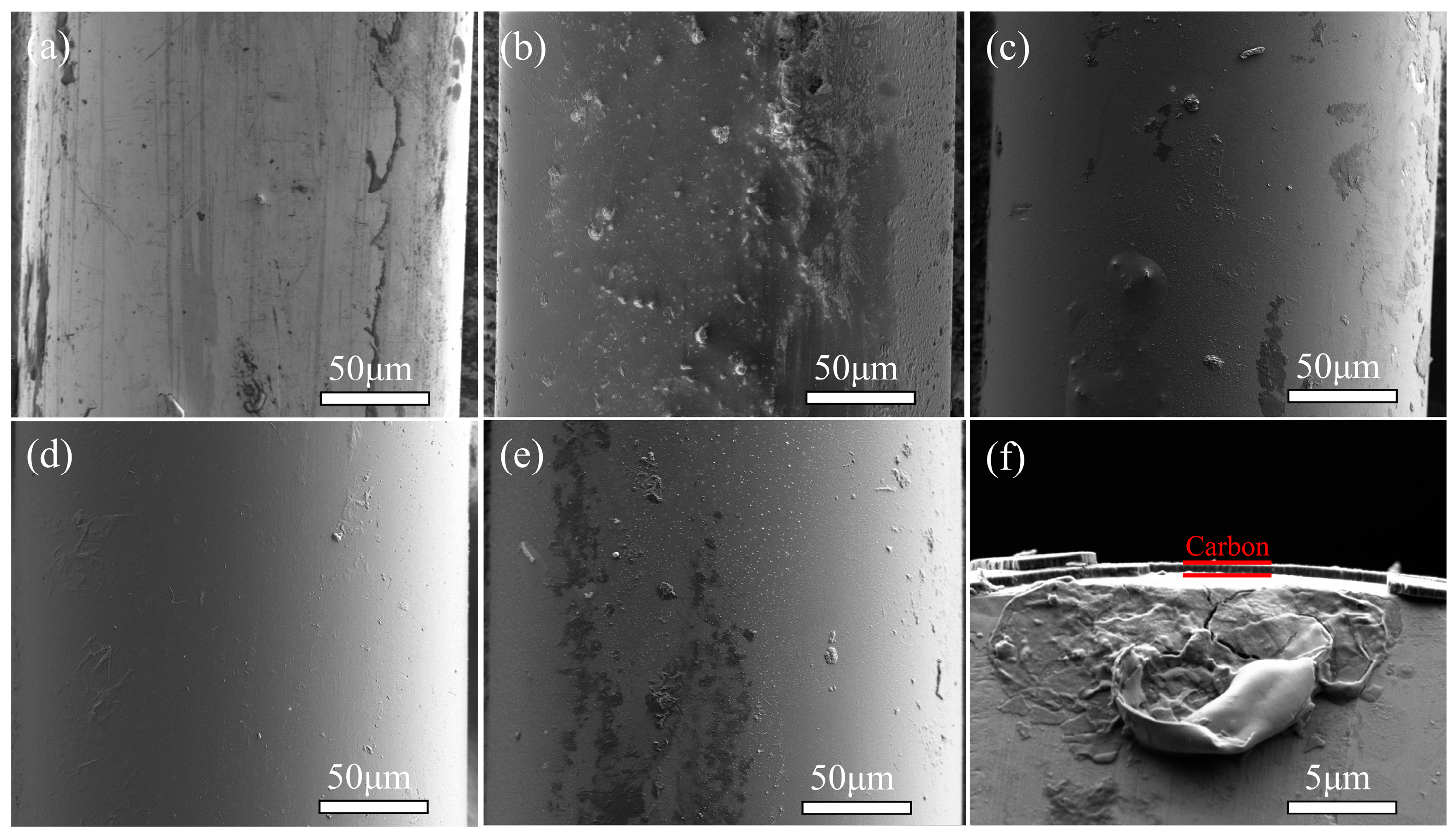
3.1. Characterization of Co-AWs
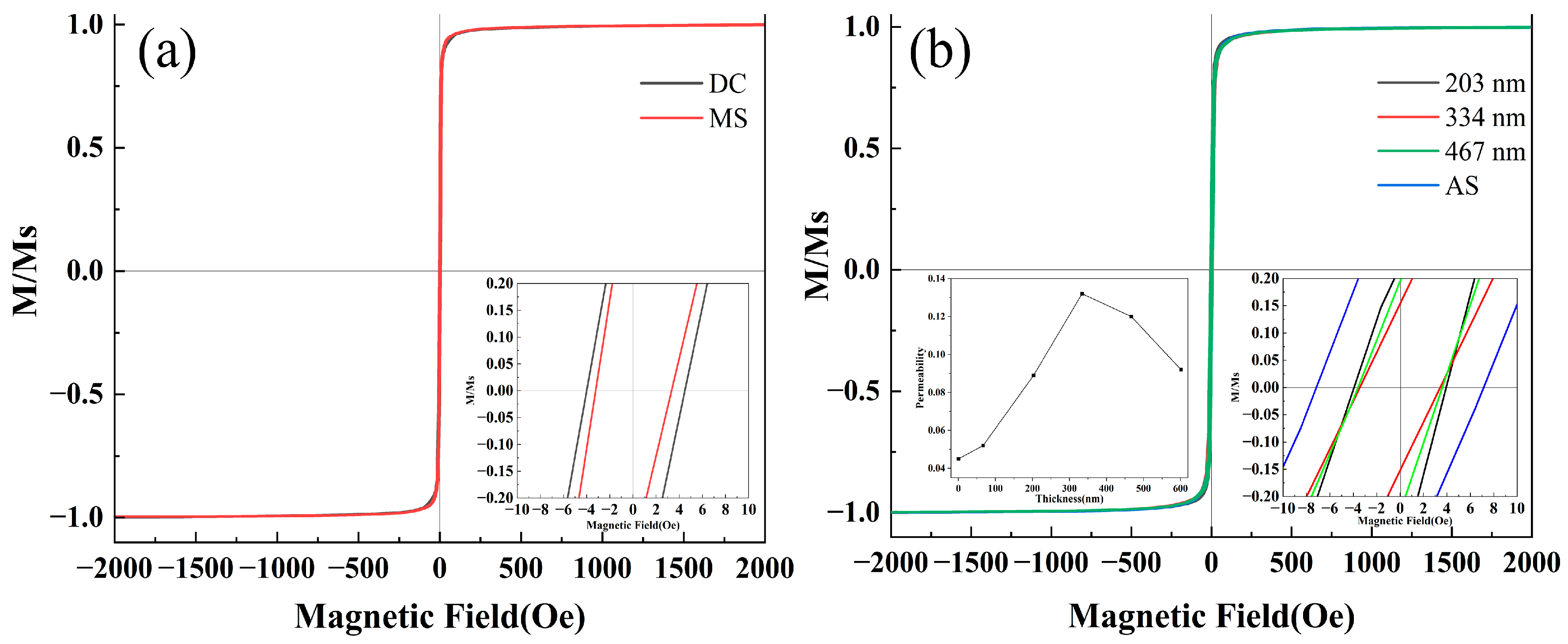
3.2. GMI Behaviors Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Panina, L.V.; Mohri, K. Magneto-impedance effect in amorphous wires. Appl. Phys. Lett. 1994, 65, 1189–1191. [Google Scholar]
- Zhukov, A. (Ed.) Novel Functional Magnetic Materials: Fundamentals and Applications; Springer International Publishing: Cham, Switzerland, 2016. [Google Scholar]
- Phan, M.-H.; Peng, H.-X. Giant magnetoimpedance materials: Fundamentals and applications. Prog. Mater. Sci. 2008, 53, 323–420. [Google Scholar]
- Heiden, C.; Rogalla, H. Barri-Iausen Jump Field Distribution of Iron Whiskers. J. Magn. Magn. Mater. 1982, 26, 275–277. [Google Scholar]
- Zhukova, V.; Zhukov, A.; Blanco, J.M.; Gonzalez, J.; Ponomarev, B.K. Switching field fluctuations in a glass-coated Fe-rich amorphous microwire. J. Magn. Magn. Mater. 2002, 249, 131–135. [Google Scholar]
- Mohri, K.; Humphrey, F.B.; Kawashima, K.; Kimura, K.; Mizutani, M. Large Barkhausen and Matteucci effects in FeCoSiB, FeCrSiB, and FeNiSiB amorphous wires. IEEE Trans. Magn. 1990, 26, 1789–1791. [Google Scholar]
- Vazquez, M.; Chen, D.-X. The magnetization reversal process in amorphous wires. IEEE Trans. Magn. 1995, 31, 1229–1238. [Google Scholar]
- Corte-Leon, P.; Zhukova, V.; Ipatov, M.; Blanco, J.M.; Gonzalez, J.; Churyukanova, M.; Baraibar, J.M.; Taskaev, S.; Zhukov, A. Stress dependence of the magnetic properties of glass-coated amorphous microwires. J. Alloys Compd. 2019, 789, 201–208. [Google Scholar]
- Valenzuela, R.; Freijo, J.J.; Salcedo, A.; Vázquez, M.; Hernando, A. A miniature dc current sensor based on magnetoimpedance. J. Appl. Phys. 1997, 81, 4301–4303. [Google Scholar]
- Cobeño, A.F.; Zhukov, A.; Blanco, J.M.; Larin, V.; Gonzalez, J. Magnetoelastic sensor based on GMI of amorphous microwire. Sens. Actuators A Phys. 2001, 91, 95–98. [Google Scholar]
- Alimohammadi, S.; Williams, P.I.; Meydan, T. A Curvature Sensor Utilizing the Matteucci Effect in Amorphous Wire. Sensors 2023, 23, 1243. [Google Scholar] [CrossRef]
- Šmelko, M.; Draganová, K.; Lipovský, P.; Semrád, K.; Blišťanová, M.; Kašper, P. Non-Destructive Testing of Aircraft Structures Using Microwire-Based Tensile Stress Sensor. Appl. Sci. 2020, 10, 8218. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhang, Q.; Li, X.; Pan, H.; Wang, J.; Zhao, Z. Detection of AFP with an ultra-sensitive giant magnetoimpedance biosensor. Sens. Actuators B Chem. 2019, 293, 53–58. [Google Scholar] [CrossRef]
- Zhu, Z.; Estevez, D.; Feng, T.; Chen, Y.; Li, Y.; Wei, H.; Wang, Y.; Wang, Y.; Zhao, L.; Jawed, S.A.; et al. A Novel Induction-Type Pressure Sensor based on Magneto-Stress Impedance and Magnetoelastic Coupling Effect for Monitoring Hand Rehabilitation. Small 2024, 20, 2400797. [Google Scholar] [CrossRef]
- Semrád, K.; Draganová, K. Non-Destructive Testing of Pipe Conveyor Belts Using Glass-Coated Magnetic Microwires. Sustainability 2022, 14, 8536. [Google Scholar] [CrossRef]
- Yang, H.; Li, S.; Wu, Y.; Bao, X.; Xiang, Z.; Xie, Y.; Pan, L.; Chen, J.; Liu, Y.; Li, R. Advances in Flexible Magnetosensitive Materials and Devices for Wearable Electronics. Adv. Mater. 2024, 36, 2311996. [Google Scholar] [CrossRef]
- Zhukova, V.; Churyukanova, M.; Kaloshkin, S.; Corte-Leon, P.; Ipatov, M.; Zhukov, A. Magnetostriction of amorphous Co-based and Fe-Ni-based magnetic microwires: Effect of stresses and annealing. J. Alloys Compd. 2023, 954, 170122. [Google Scholar] [CrossRef]
- Mu, C.; Hu, L.; Zhu, F.; Cui, Z.; He, A.; Dong, Y.; Man, Q.; Ma, H.; Li, J. Effects of drawing and tension stress annealing on the structure and magnetic properties of Co-based amorphous wire. J. Magn. Magn. Mater. 2024, 589, 171569. [Google Scholar] [CrossRef]
- Gonzalez, Á.; Corte-León, P.; Zhukova, V.; García-Gómez, A.; Ipatov, M.; González, J.M.; María Blanco, J.; Zhukov, A. Heat and current annealing effects on magnetic properties of Fe-rich glass-coated amorphous microwires with different radius. In Proceedings of the 2023 IEEE International Magnetic Conference—Short Papers (INTERMAG Short Papers), Sendai, Japan, 15–19 May 2023; IEEE: New York, NY, USA, 2023; pp. 1–2. [Google Scholar]
- Liu, R.; Cao, G.; Liu, J.; Li, Z.; Zhang, Y.; Liu, Z. Comparative study on GMI properties of Co-based microwires improved by alcohol and liquid nitrogen medium-current annealing. Mater. Res. Express 2021, 8, 065202. [Google Scholar] [CrossRef]
- Jiang, S.D.; Eggers, T.; Thiabgoh, O.; Xing, D.W.; Fang, W.B.; Sun, J.F.; Srikanth, H.; Phan, M.H. Enhancement of Giant Magneto-Impedance in Series Co-Rich Microwires for Low-Field Sensing Applications. J. Electron. Mater. 2018, 47, 2667–2672. [Google Scholar] [CrossRef]
- Zhu, Z.; Peng, M.; Wang, Y.; Qin, F. Ultra-large giant magnetoimpedance effect by a 2D square spiral amorphous microwire. J. Magn. Magn. Mater. 2022, 550, 169090. [Google Scholar] [CrossRef]
- Zhang, S.-L.; Chai, Y.-S.; Fang, D.-Q.; Wang, L.-C.; Xing, D.-W.; Sun, J.-F. Giant magneto-impedance effect of two paralleled amorphous microwires. Rare Met. 2016, 35, 344–348. [Google Scholar]
- Jiang, S.D.; Eggers, T.; Thiabgoh, O.; Xing, D.W.; Fei, W.D.; Shen, H.X.; Liu, J.S.; Zhang, J.R.; Fang, W.B.; Sun, J.F.; et al. Relating surface roughness and magnetic domain structure to giant magneto-impedance of Co-rich melt-extracted microwires. Sci. Rep. 2017, 7, 46253. [Google Scholar]
- Zhukov, A.; Ipatov, M.; Churyukanova, M.; Talaat, A.; Blanco, J.M.; Zhukova, V. Trends in optimization of giant magnetoimpedance effect in amorphous and nanocrystalline materials. J. Alloys Compd. 2017, 727, 887–901. [Google Scholar]
- Zhang, Y.; Huang, C.; Duan, Z.; Liu, Q.; Wang, J.; Shi, W. Enhanced magnetoimpedance effect of Fe75.5Cu1Nb3Si13.5B7 ribbon covered by in-situ growth vertical graphene sheets. Mater. Lett. 2018, 222, 131–134. [Google Scholar]
- Chen, Y.; Zou, J.; Shu, X.; Song, Y.; Zhao, Z. Enhanced giant magneto-impedance effects in sandwich FINEMET/rGO/FeCo composite ribbons. Appl. Surf. Sci. 2021, 545, 149021. [Google Scholar]
- Guo, Y.-B.; Wang, D.; Wang, Z.-M.; Ma, L.; Zhao, Z.-J. Asymmetric magnetoimpedance effect and dipolar interactions of FINEMET/SiO2/FePd composite ribbons. Chin. Phys. B 2023, 32, 070703. [Google Scholar]
- Zhang, Q.; Mao, Q.; Ruan, J.; Wang, Q.; Yang, X.; Zhao, Z. Giant magneto-impedance effect of magnetron sputtered Ni80Fe20/SiO2/Cu composite wires. J. Magn. Magn. Mater. 2008, 320, 2319–2321. [Google Scholar] [CrossRef]
- Xie, L.; Li, X.; Zou, J.T.; Pan, H.L.; Xie, W.H.; Zhao, Z.J. Optimized giant magneto-impedance effect in electroless-deposited NiFeP/Cu composite wires. Surf. Coat. Technol. 2018, 334, 158–163. [Google Scholar]
- Mardani, R.; Shahmirzaee, H.; Ershadifar, H.; Vahdani, M.R. Electrodeposition of Ni32Fe48Mo20 and Ni52Fe33W15 alloy film on Cu microwire from ionic liquid containing plating bath. Surf. Coat. Technol. 2017, 324, 281–287. [Google Scholar]
- Salary, M.M.; Jafar-Zanjani, S.; Mosallaei, H. Time-varying metamaterials based on graphene-wrapped microwires: Modeling and potential applications. Phys. Rev. B 2018, 97, 115421. [Google Scholar]
- Tandon, P.; Sahu, R.; Mishra, A.C.; Singh, K.; Srikanti, K.; Gopalan, R. Magnetoimpedance effect in electrodeposited NiFe/Cu wire using trisodium citrate additive in plating bath. J. Magn. Magn. Mater. 2023, 570, 170490. [Google Scholar]
- Tandon, P.; Sahu, R.; Mishra, A.C. Giant magnetoimpedance effect in electrodeposited CoNiFe/Cu composite wire: Experimental study and analytical modelling. Phys. B Condens. Matter. 2022, 642, 414131. [Google Scholar]
- Xiang, S.; Zou, J.; Li, X.; Xie, W.; Zhao, Z.J. Magnetic properties and giant magneto-impedance effect in electroless-deposited CoP/Cu composite wires. Mater. Res. Express 2019, 6, 066101. [Google Scholar]
- Tandon, P.; Mishra, A.C. The effect of magnetic field orientation on the magnetoimpedance of electroplated NiFeCo/Cu wire. J. Mater. Sci. Mater. Electron. 2022, 33, 18311–18326. [Google Scholar]
- Shi, L.; Ruan, J.; Zhang, J.; Zhao, Z.; Gao, H.B.; Hartmann, U. Enhancement of giant magneto-impedance effect in Ni80Fe20/SiO2/Cu composite wires. Phys. B Condens. Matter. 2009, 404, 3766–3770. [Google Scholar]
- Mbayachi, V.B.; Ndayiragije, E.; Sammani, T.; Taj, S.; Mbuta, E.R.; Khan, A.U. Graphene synthesis, characterization and its applications: A review. Results Chem. 2021, 3, 100163. [Google Scholar]
- Razaq, A.; Bibi, F.; Zheng, X.; Papadakis, R.; Jafri, S.H.M.; Li, H. Review on Graphene-, Graphene Oxide-, Reduced Graphene Oxide-Based Flexible Composites: From Fabrication to Applications. Materials 2022, 15, 1012. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Fal′ko, V.I.; Colombo, L.; Gellert, P.R.; Schwab, M.G.; Kim, K. A roadmap for graphene. Nature 2012, 490, 192–200. [Google Scholar]
- Okhay, O.; Goncalves, G. Carbon and Related Composites for Sensors and Energy Storage: Synthesis, Properties, and Application. C 2024, 10, 101. [Google Scholar] [CrossRef]
- Giri, P.; Gill, I.; Swensgard, M.; Kaiser, A.; Rust, A.; Stuparyk, B.; Fisher, A.; Williams, J.; Renoit, K.; Kreeb, E.; et al. Pristine and Coated Carbon Nanotube Sheets—Characterization and Potential Applications. C 2024, 10, 17. [Google Scholar] [CrossRef]
- Golubeva, E.V.; Stepanova, E.A.; Balymov, K.G.; Volchkov, S.O.; Kurlyandskaya, G.V. Magnetic Properties and the Giant Magnetoimpedance of Amorphous Co-Based Wires with a Carbon Coating. Phys. Met. Metallogr. 2018, 119, 324–331. [Google Scholar] [CrossRef]
- Estevez, D.; Zhao, Y.; Wang, Y.; Qin, F.; Peng, H.-X. Optimizing magnetoimpedance of amorphous microwires by nanocarbon-induced magnetic anisotropy. J. Magn. Magn. Mater. 2020, 502, 166527. [Google Scholar] [CrossRef]
- Zou, J.; Chen, Y.; Shu, X.; Li, X.; Song, Y.; Zhao, Z. Proper pH value enhances giant magneto-impedance effect of FINEMET/rGO composite ribbons by electroless plating. Mater. Sci. Eng. B 2021, 265, 115004. [Google Scholar] [CrossRef]
- Corte-León, P.; Zhukova, V.; Ipatov, M.; Blanco, J.M.; Gonzalez, J.; Dominguez, L.; Churyukanova, M.; Zhukov, A. High frequency giant magnetoimpedance effect of a stress-annealed Fe-rich glass-coated microwire. J. Alloys Compd. 2019, 802, 112–117. [Google Scholar] [CrossRef]
- Vázquez, M.; Hernando, A. A soft magnetic wire for sensor applications. J. Phys. D Appl. Phys. 1996, 29, 939–949. [Google Scholar] [CrossRef]
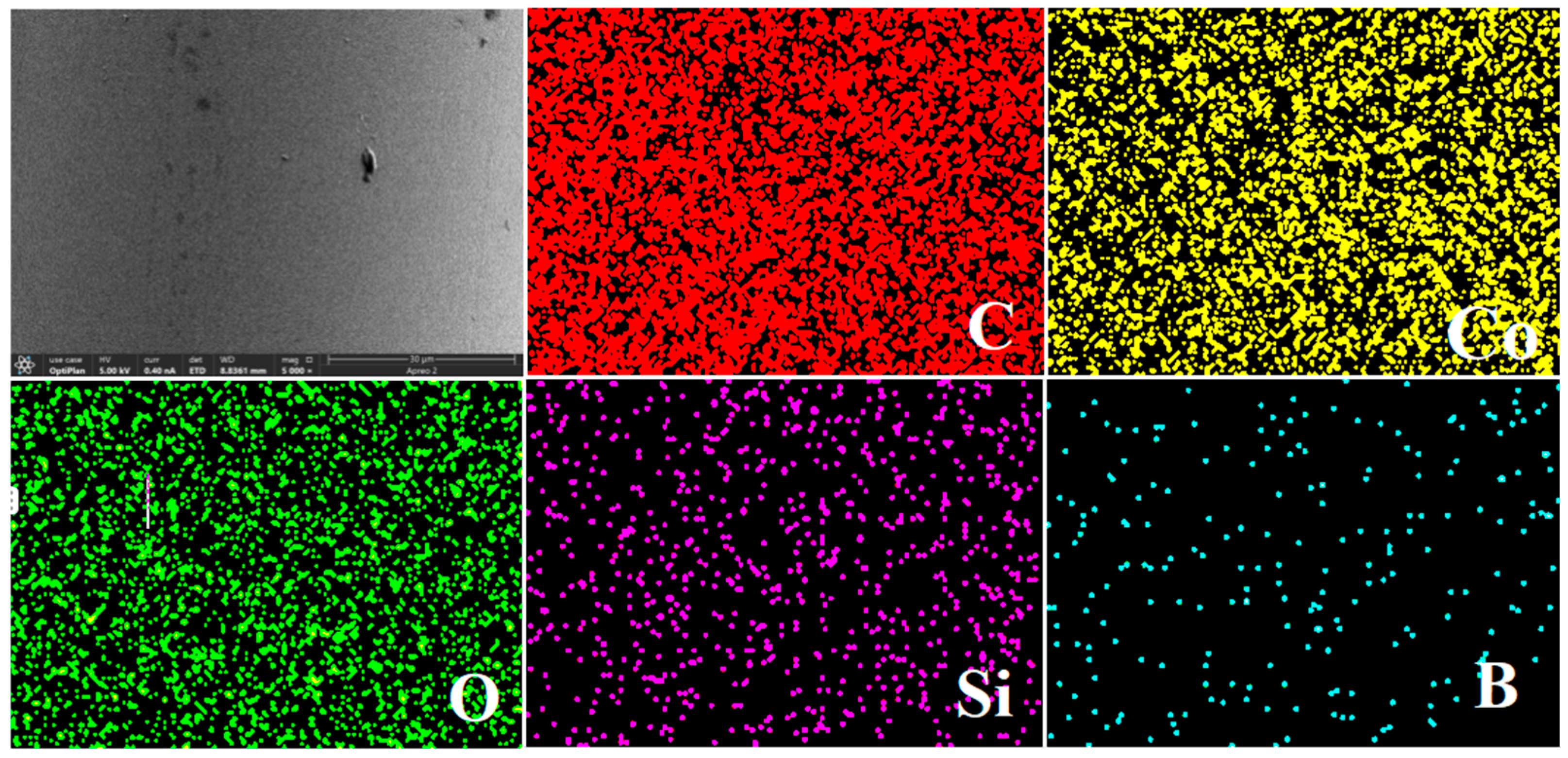
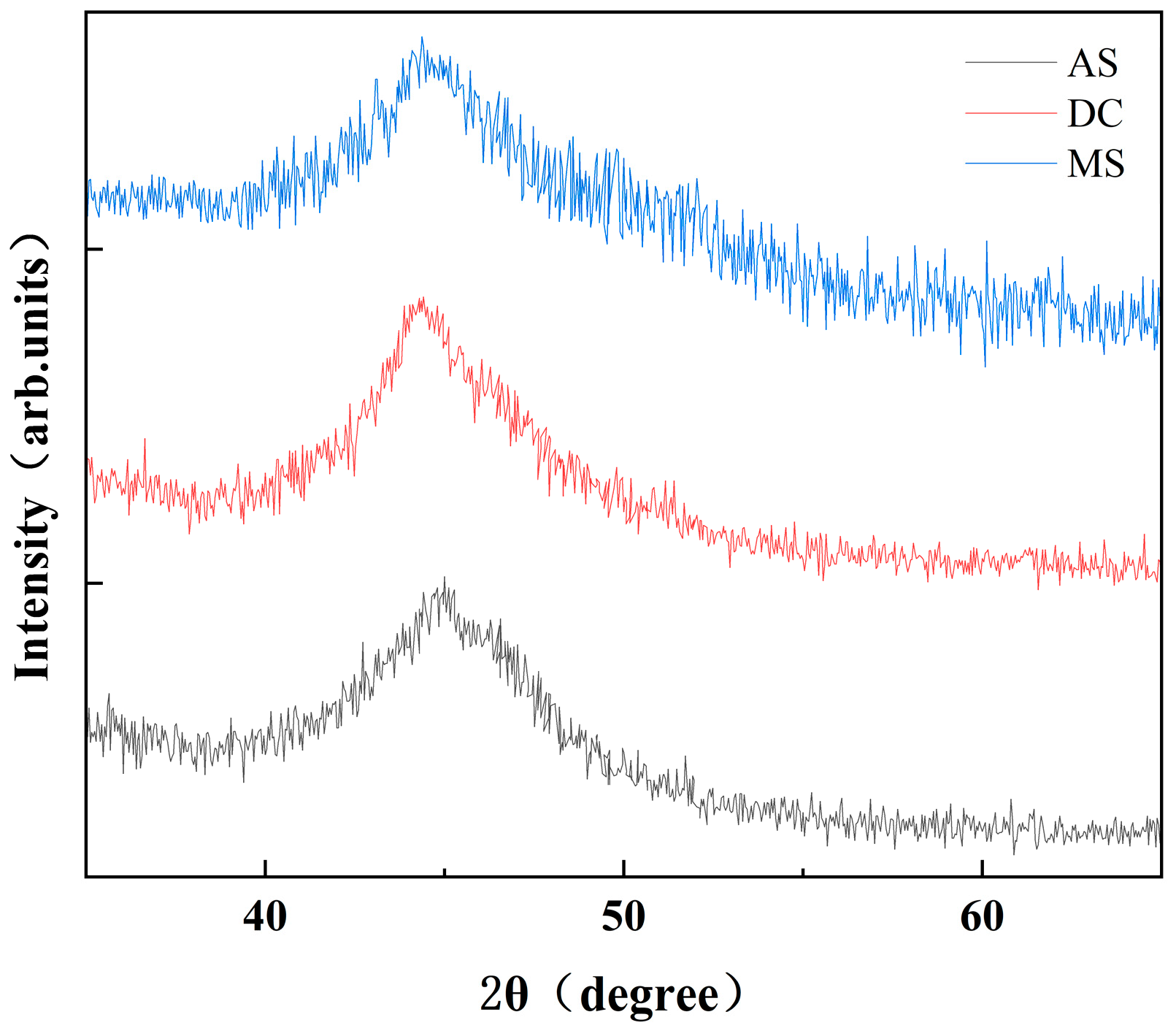
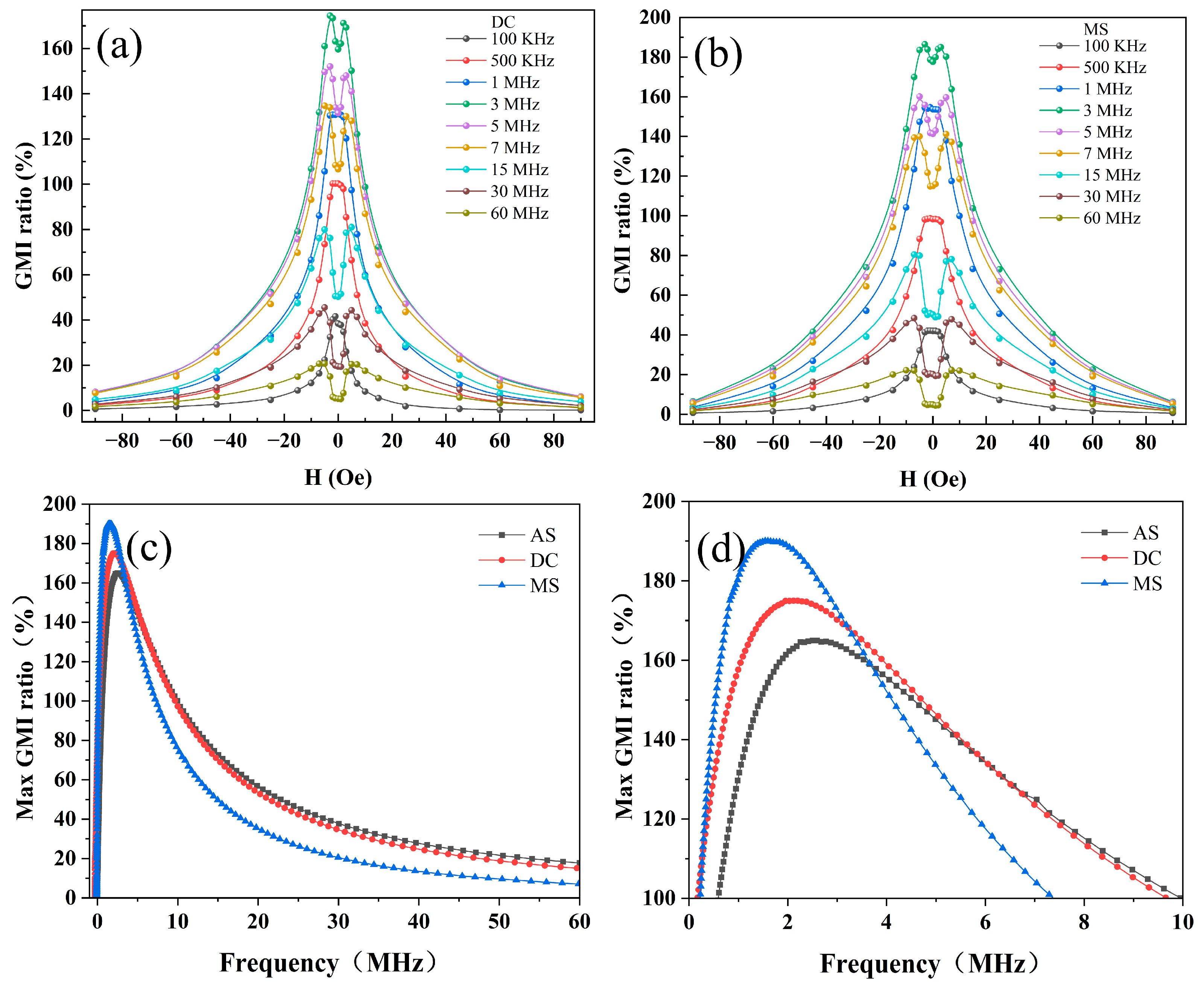
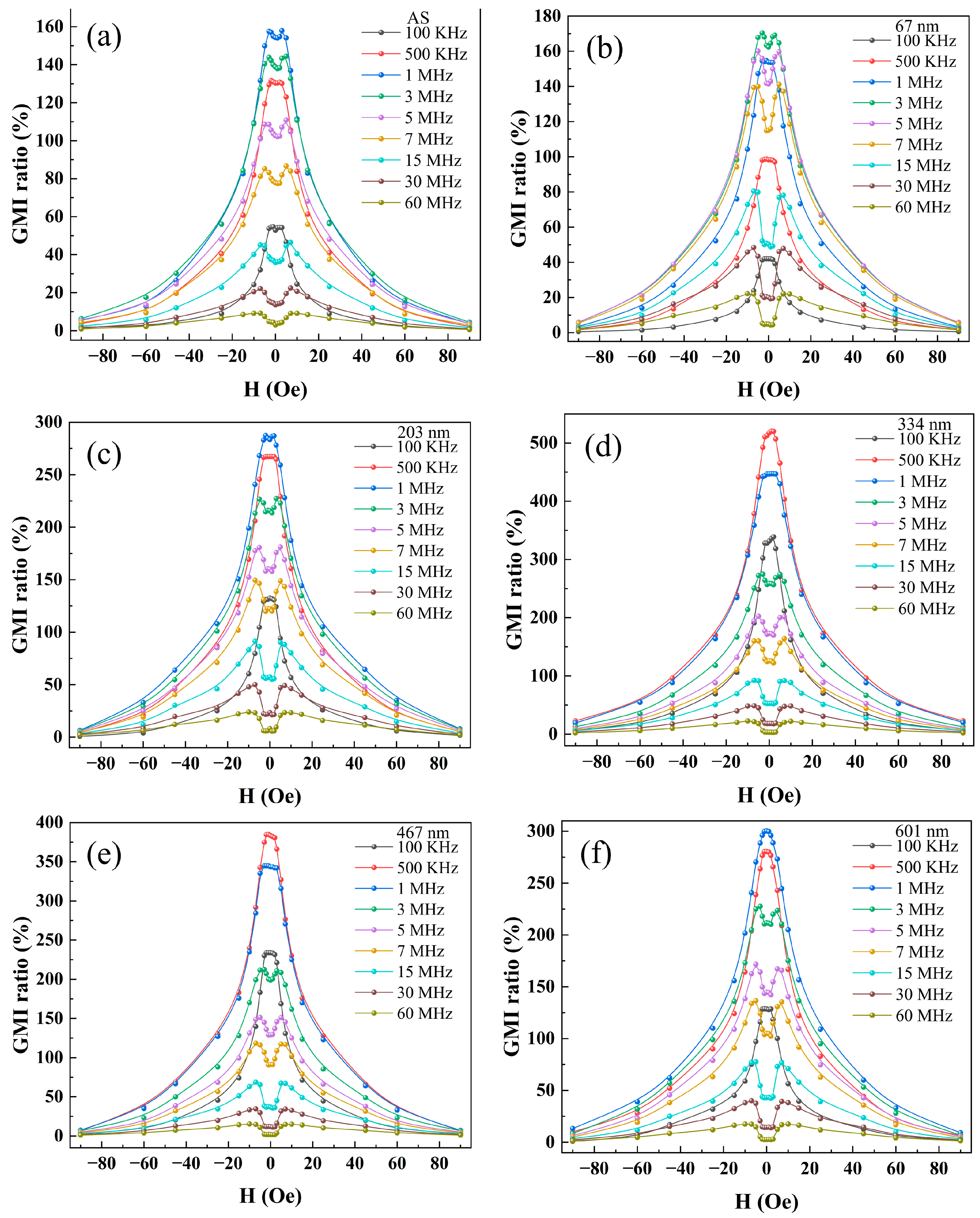


| Sample | AS | DC | 203 nm | 334 nm | 467 nm |
|---|---|---|---|---|---|
| Atomic % | 4.8 | 14.0 | 26.2 | 30.2 | 45.5 |
| Weight | 1.2 | 4.4 | 8.9 | 11.2 | 21.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Z.; Huang, J.; Chen, J.; Lei, C. Modulation of Giant Magnetoimpedance Effect in Co-Based Amorphous Wires by Carbon-Based Nanocoatings. C 2025, 11, 26. https://doi.org/10.3390/c11020026
Yang Z, Huang J, Chen J, Lei C. Modulation of Giant Magnetoimpedance Effect in Co-Based Amorphous Wires by Carbon-Based Nanocoatings. C. 2025; 11(2):26. https://doi.org/10.3390/c11020026
Chicago/Turabian StyleYang, Zhen, Jiabao Huang, Jingyuan Chen, and Chong Lei. 2025. "Modulation of Giant Magnetoimpedance Effect in Co-Based Amorphous Wires by Carbon-Based Nanocoatings" C 11, no. 2: 26. https://doi.org/10.3390/c11020026
APA StyleYang, Z., Huang, J., Chen, J., & Lei, C. (2025). Modulation of Giant Magnetoimpedance Effect in Co-Based Amorphous Wires by Carbon-Based Nanocoatings. C, 11(2), 26. https://doi.org/10.3390/c11020026







