Electrochemical Change Induced by Spherical Indentation in Lithium-Ion Batteries
Abstract
:1. Introduction
2. Methodology
2.1. Mechanical Analysis
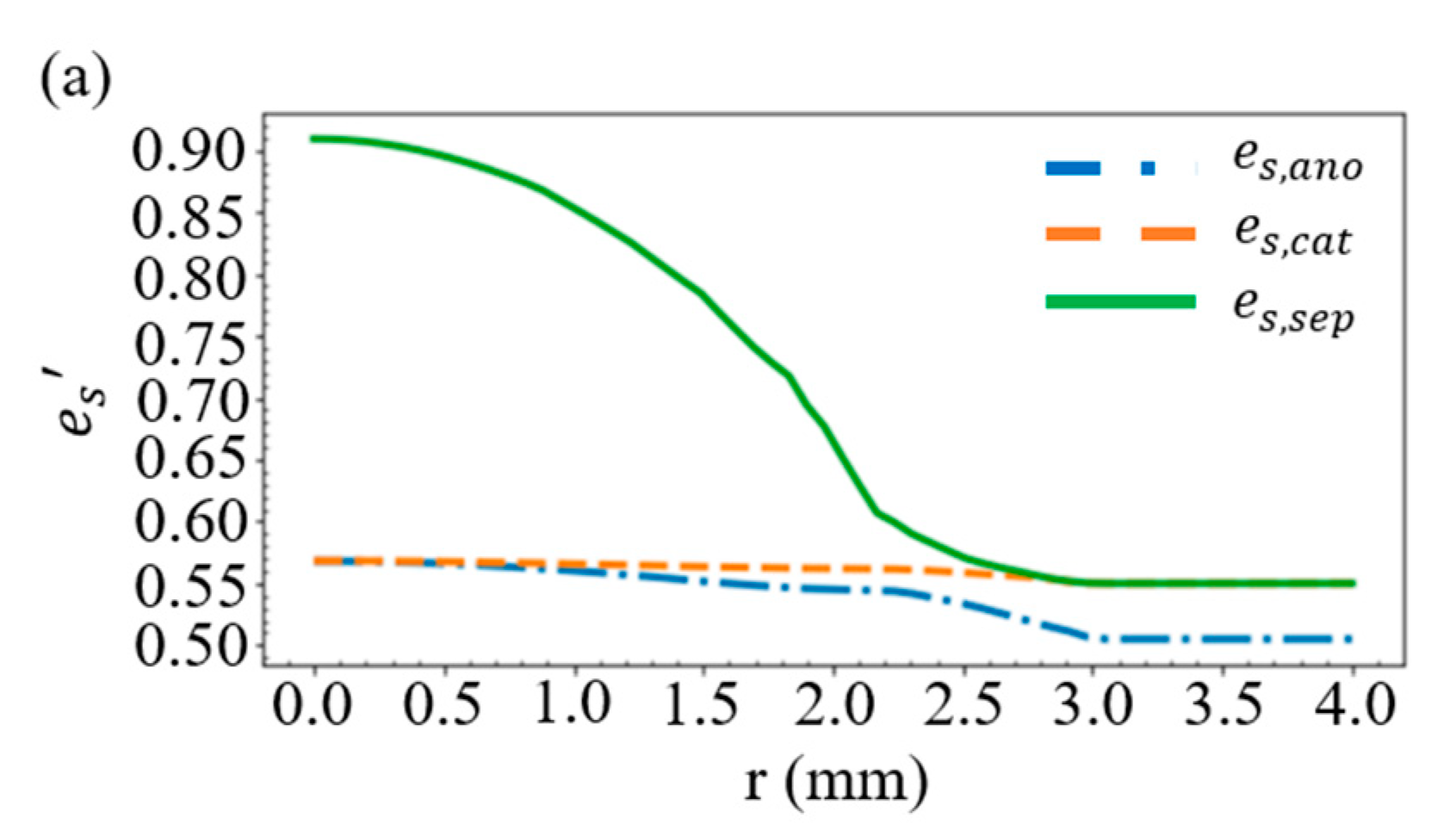
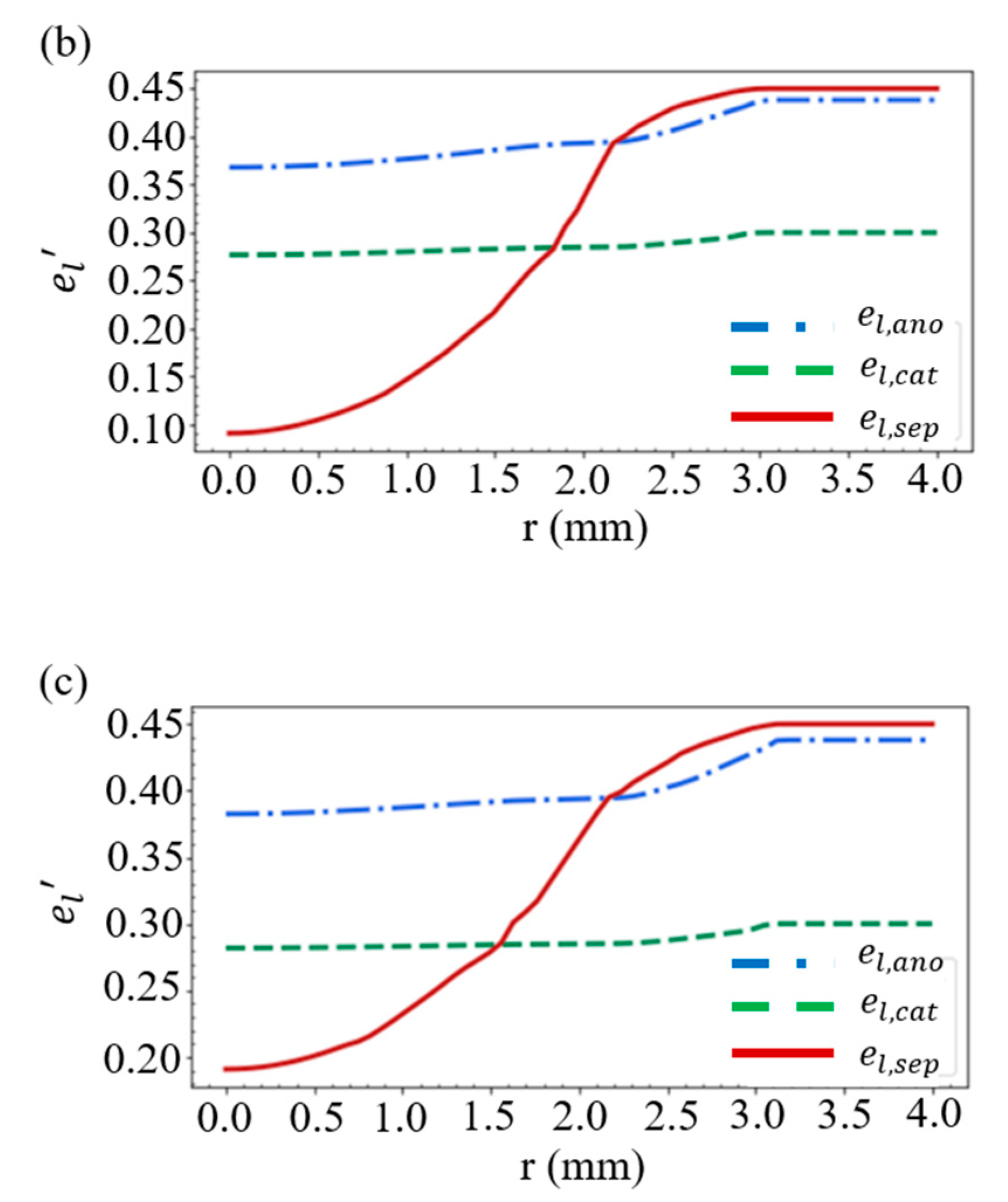
2.2. Strain-Induced Change in Porosity
2.3. Electrochemical Simulation
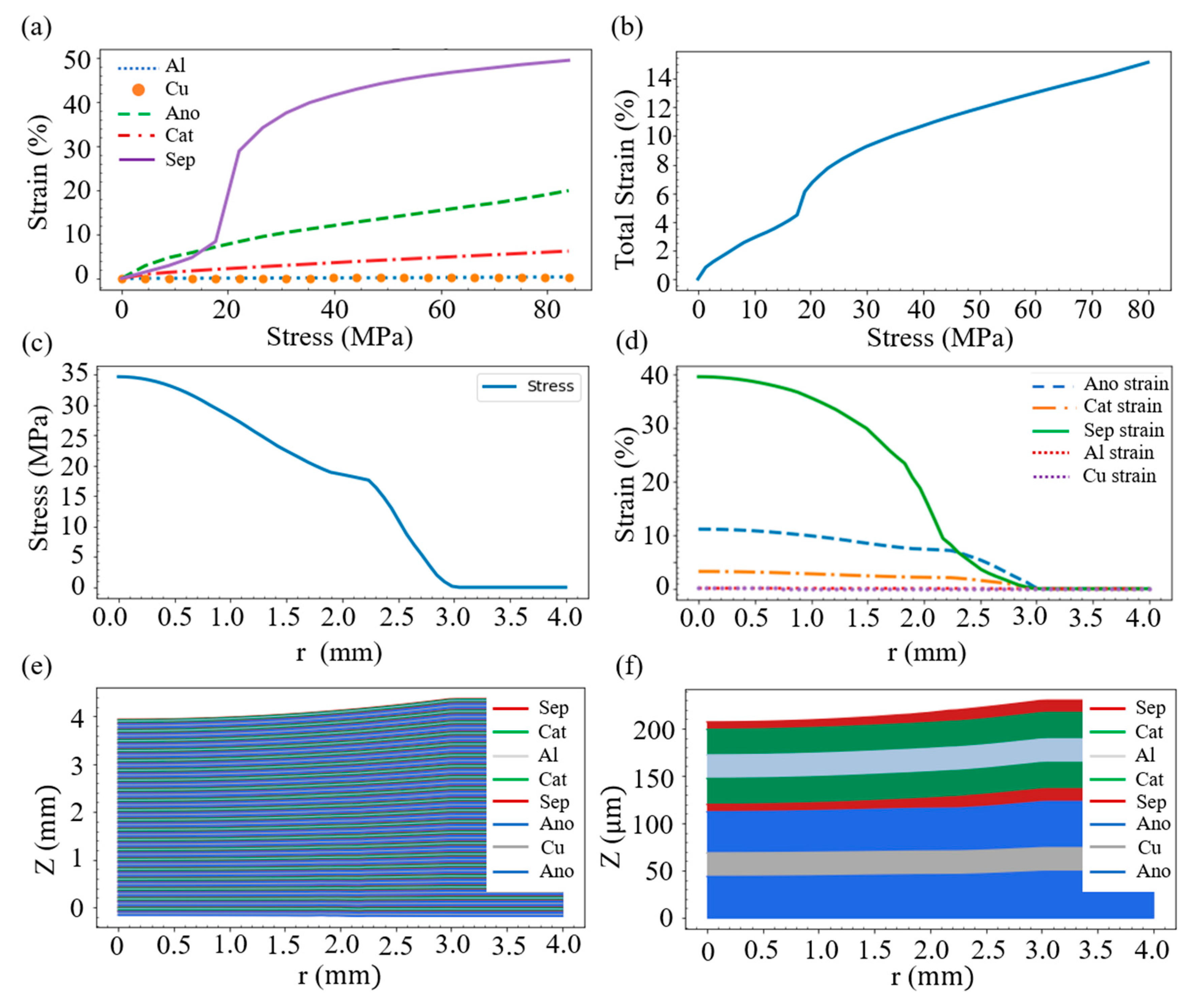
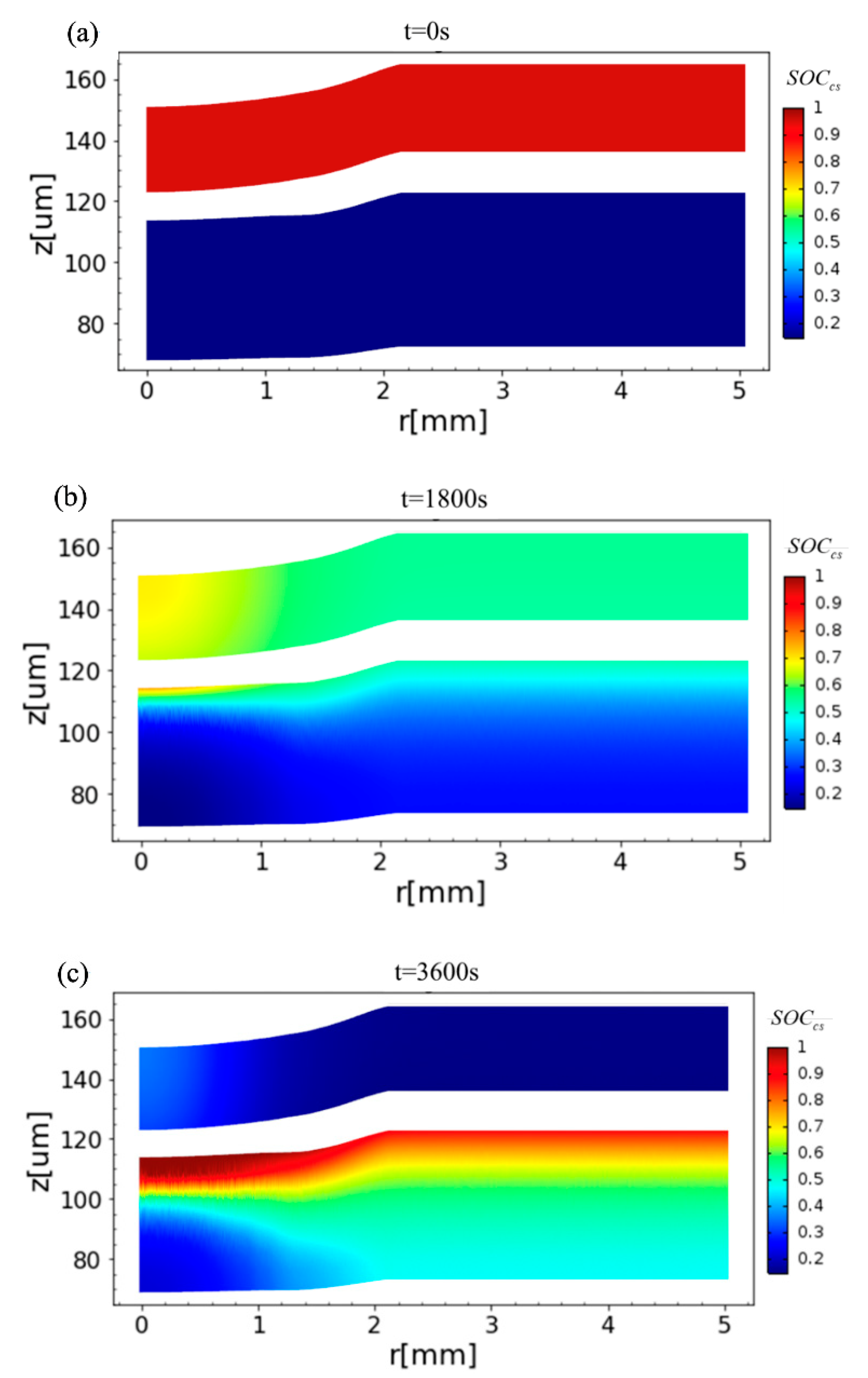
3. Results
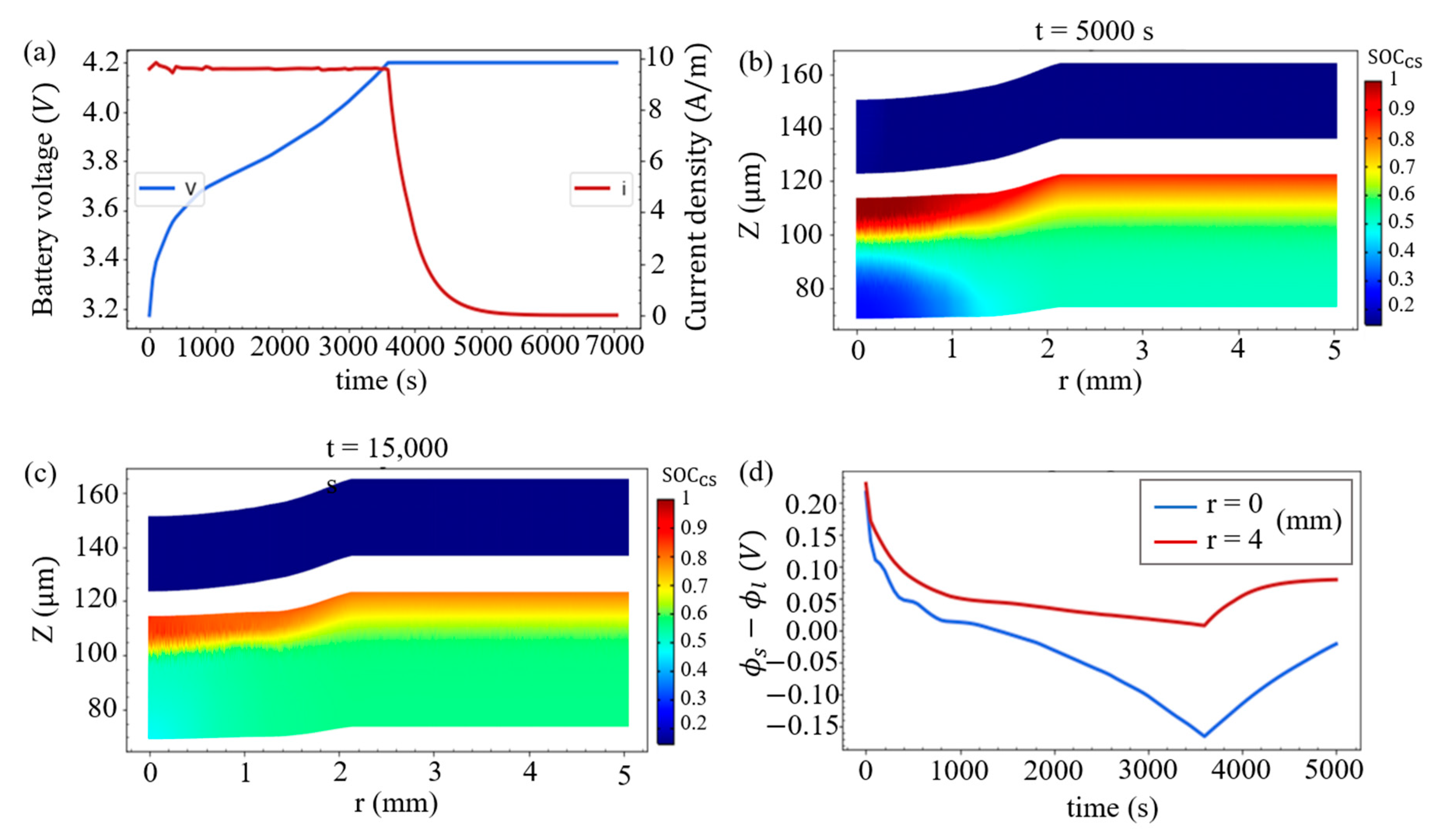
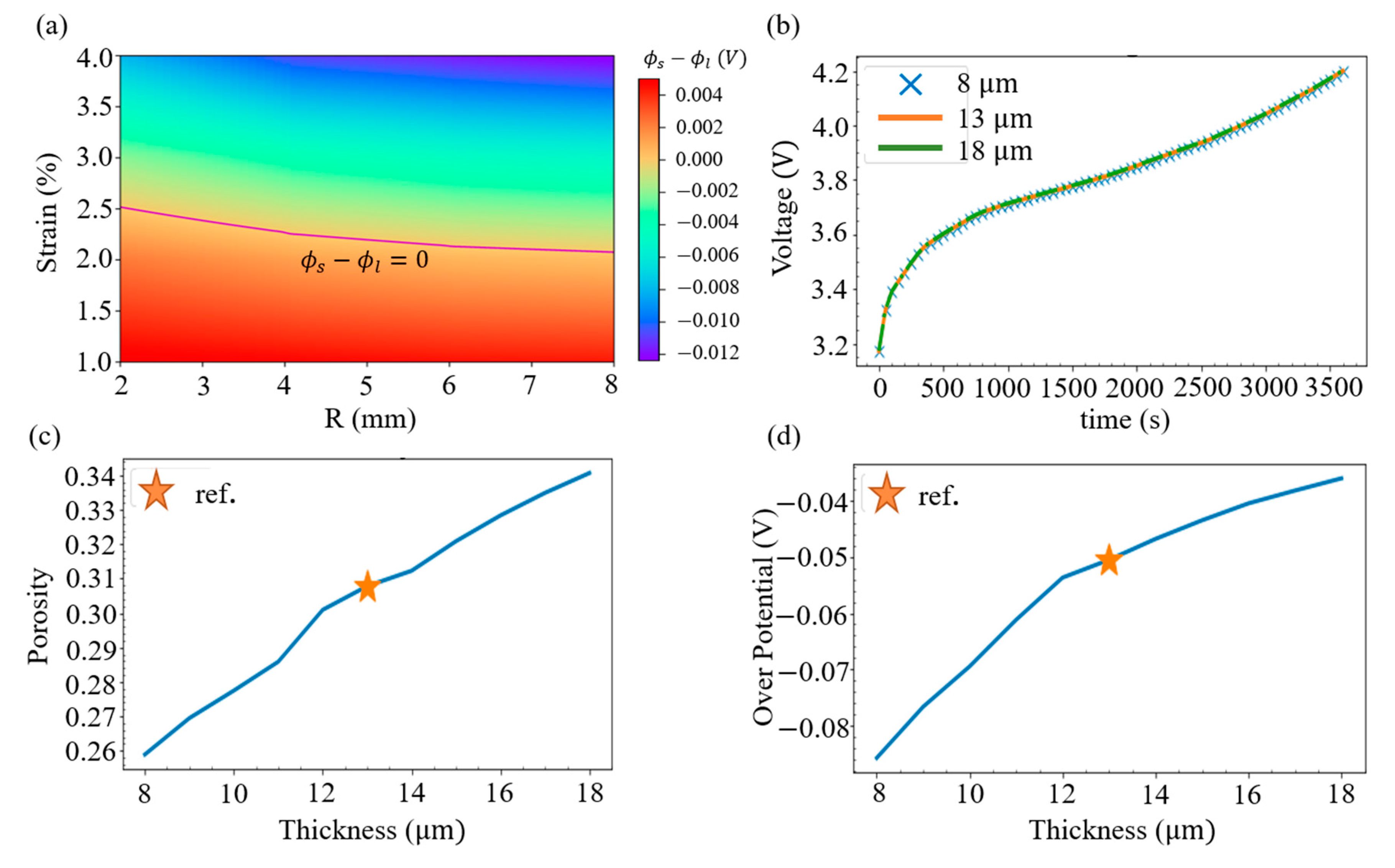
3.1. Battery Strain of 8%
3.2. Charge with CCCV
3.3. Deformation and the SR Overpotential
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| H | thickness of the battery before deformation |
| W | thickness of the battery after deformation |
| hi | thickness of the layer i |
| ε | strain of the layer i |
| εV,i | volumetric strain of the layer i |
| d | distance of the sphere pressed into the battery |
| r | radius of the sphere indentation |
| es | volume fraction of solid |
| el | volume fraction of fluid |
| Ds | diffusion coefficient of lithium in electrode |
| Dl | diffusion coefficient of fluid in electrolyte |
| κs | conductivity of solid phase |
| κl | conductivity of fluid phase |
| F | faraday’s constant |
| i0,1 | exchange current density |
| Rg | gas constant |
| T | temperature |
| ϕs | solid phase potential |
| ϕl | electrolyte phase potential |
| j1 | reaction flux |
| j2 | reduction flux of lithium |
| ndep | molar number of deposition per unit volume |
| as | specific interfacial area |
| η1 | surface overpotential |
| η2 | side-reaction overpotential |
| Cs | concentration of lithium in electrode |
| SOCcs | local state of charge |
| U | open circuit potential |
| Rf | SEI film resistance |
| cl | concentration of lithium ions in electrolyte |
| t+ | transference number |
| k1 | reaction rate constant |
References
- Fantham, T.L.; Gladwin, D.T. An overview of safety for laboratory testing of lithium-ion batteries. Energy Rep. 2021, 7, 2–8. [Google Scholar]
- Williard, N.; He, W.; Hendricks, C.; Pecht, M. Lessons learned from the 787 Dreamliner issue on lithium-ion battery reliability. Energies 2013, 6, 4682–4689. [Google Scholar]
- Hendricks, C.; Williard, N.; Mathew, S.; Pecht, M. A failure modes, mechanisms, and effects analysis (FMMEA) of lithium-ion batteries. J. Power Sources 2015, 297, 113–120. [Google Scholar]
- Zhu, J.; Wierzbicki, T.; Li, W. A review of safety-focused mechanical modeling of commercial lithium-ion batteries. J. Power Sources 2018, 378, 153–168. [Google Scholar]
- Li, Z.; Huang, J.; Liaw, B.Y.; Metzler, V.; Zhang, J. A review of lithium deposition in lithium-ion and lithium metal secondary batteries. J. Power Sources 2014, 254, 168–182. [Google Scholar]
- Loveridge, M.J.; Remy, G.; Kourra, N.; Genieser, R.; Barai, A.; Lain, M.J.; Guo, Y.; Amor-Segan, M.; Williams, M.A.; Amietszajew, T.; et al. Looking deeper into the Galaxy (Note 7). Batteries 2018, 4, 3. [Google Scholar]
- Zhang, C.; Santhanagopalan, S.; Sprague, M.A.; Pesaran, A.A. Coupled mechanical-electrical-thermal modeling for short-circuit prediction in a lithium-ion cell under mechanical abuse. J. Power Sources 2015, 290, 102–113. [Google Scholar]
- Wu, H.; Zhuo, D.; Kong, D.; Cui, Y. Improving battery safety by early detection of internal shorting with a bifunctional separator. Nat. Commun. 2014, 5, 5193. [Google Scholar]
- Yang, X.G.; Leng, Y.; Zhang, G.; Ge, S.; Wang, C.Y. Modeling of lithium plating induced aging of lithium-ion batteries: Transition from linear to nonlinear aging. J. Power Sources 2017, 360, 28–40. [Google Scholar]
- Jana, A.; Ely, D.R.; García, R.E. Dendrite –separator interactions in lithium-based batteries. J. Power Sources 2015, 275, 912–921. [Google Scholar]
- Cannarella, J.; Arnold, C.B. The effects of defects on localized plating in lithium-ion batteries. J. Electrochem. Soc. 2015, 162, A1365–A1373. [Google Scholar]
- Bach, T.C.; Schuster, S.F.; Fleder, E.; Müller, J.; Brand, M.J.; Lorrmann, H.; Jossen, A.; Sextl, G. Nonlinear aging of cylindrical lithium-ion cells linked to heterogeneous compression. J. Energy Storage 2016, 5, 212–223. [Google Scholar]
- Lin, X.; Khosravinia, K.; Hu, X.; Li, J.; Lu, W. Lithium plating mechanism, detection, and mitigation in lithium-ion batteries. Prog. Energy Combust. Sci. 2021, 87, 100953. [Google Scholar]
- Sun, T.; Shen, T.; Zheng, Y.; Ren, D.; Zhu, W.; Li, J.; Wang, Y.; Rui, X.; Wang, S.; Wang, L.; et al. Modeling the inhomogenerous lithium plating in lithium-ion batteries induced by non-uniform temperature distribution. Electrochim. Acta 2022, 425, 140701. [Google Scholar]
- von Lüders, C.; Keil, J.; Webersberger, M.; Jossen, A. Modeling of lithium plating and lithium stripping in lithium-ion batteries. J. Power Sources 2019, 414, 41–47. [Google Scholar]
- Yang, X.G.; Ge, S.; Liu, T.; Leng, Y.; Wang, C.Y. A look into the voltage plateau signal for detection and quantification of lithium plating in lithium-ion cells. J. Power Sources 2018, 395, 251–261. [Google Scholar]
- Tang, M.; Albertus, P.; Newman, J. Two-Dimensional Modeling of Lithium Deposition during Cell Charging. J. Electrochem. Soc. 2009, 156, A390–A399. [Google Scholar]
- Verbrugge, M.W.; Koch, B.J. Microelectrode investigation of ultrahigh-rate lithium deposition and stripping. J. Electroanal. Chem. 1994, 367, 123–129. [Google Scholar]
- Perkins, R.D.; Randall, A.V.; Zhang, X.; Plett, G.L. Controls oriented reduced order modeling of lithium deposition on overcharge. J. Power Sources 2012, 209, 318–325. [Google Scholar]
- Sahraei, E.; Hill, R.; Wierzbicki, T. Calibration and finite element simulation of pouch lithium-ion batteries for mechanical integrity. J. Power Sources 2012, 201, 307–321. [Google Scholar]
- Sahraei, E.; Bosco, E.; Dixon, B.; Lai, B. Microscale failure mechanisms leading to internal short circuit in Li-ion batteries under complex loading scenarios. J. Power Sources 2016, 319, 56–65. [Google Scholar]
- Cannarella, J.; Liua, X.; Leng, C.Z.; Sinko, P.D.; Gor, G.Y.; Arnold, C.B. Mechanical properties of a battery separator under compression and tension. J. Electrochem. Soc. 2014, 161, F3117–F3122. [Google Scholar]
- Zhu, J.; Li, W.; Xia, Y.; Sahraei, E. Testing and modeling the mechanical properties of the granular materials of graphite anode. J. Electrochem. Soc. 2018, 165, A1160–A1168. [Google Scholar]
- Yin, H.; Ma, S.; Li, H.; Wen, G.; Santhanagopalan, S.; Zhang, C. Modeling strategy for progressive failure prediction in lithium-ion batteries under mechanical abuse. eTransportation 2021, 7, 100098. [Google Scholar]
- Li, H.; Zhou, D.; Zhang, M.; Liu, B.; Zhang, C. Multi-field interpretation of internal short circuit and thermal runaway behavior for lithium-ion batteries under mechanical abuse. Energy 2022, 263, 126027. [Google Scholar] [CrossRef]
- Zhou, D.; Li, H.; Li, Z.; Zhang, C. Toward the performance evolution of lithium-ion battery upon impact loading. Electrochim. Acta 2022, 432, 141192. [Google Scholar]
- Li, H.; Liu, B.; Zhou, D.; Zhang, C. Coupled mechanical-electrochemical-thermal study on the short-circuit mechanism of lithium-ion batteries under mechanical abuse. J. Electrochem. Soc. 2020, 167, 120501. [Google Scholar]
- Li, H.; Zhou, D.; Du, C.; Zhang, C. Parametric study on the safety behavior of mechanically induced short circuit for lithium-ion pouch batteries. J. Electrochem. Energy Convers. Storage 2021, 18, 020904. [Google Scholar]
- Bernardi, D.; Pawlikowski, E.; Newman, J. A general energy balance for battery systems. J. Electrochem. Soc. 1985, 132, 5. [Google Scholar]
- Doyle, M.; Fuller, T.F.; Newman, J. Modeling of galvanostatic charge and discharge of the lithium/polymer/insertion cell. J. Electrochem. Soc. 1993, 140, 1526. [Google Scholar]
- Doyle, M.; Newman, J.; Gozdz, A.S.; Schmutz, C.N.; Tarascon, J.M. Comparison of modeling predictions with experimental data from plastic lithium ion cells. J. Electrochem. Soc. 1996, 143, 1890–1903. [Google Scholar]
- Chen, K.C.; Lan, J.Y. Micromorphic modeling of granular materials. Int. J. Solids Struct. 2009, 46, 1554–1563. [Google Scholar]
- Lee, C. Two-phase modelling of submarine granular flows with shear-induced volume change and pore-pressure feedback. J. Fluid Mech. 2021, 907, A31. [Google Scholar]



| Model | Feature | Outcome | Ref. |
|---|---|---|---|
| αa,2 = 0.5, αc,2 = 0.5 | Same charge transfer coefficients. | Overestimation of Li metal oxidation. | [11] |
| αa,2 = 0.33, αc,2 = 0.67 | Transfer coefficient is measured. | Interfacial resistance is attributable to degradation. | [18] |
| Tafel | Li metal will not re-oxidize to lithium ions. | Li metal generated continuously. | [9] |
| Modified Butler–Volmer | Reduced-order model with plating equations is presented. | Li metal deposition when the overpotential is less than zero. | [19] |
| Descriptions | Equations |
|---|---|
| Interfacial reaction kinetics | |
| Charge conservation in the solid phase | |
| Solid phase diffusion | |
| Charge conservation in the electrolyte phase | |
| Mass transfer in the electrolyte phase |
| Parameters | Positive | Separator | Negative |
|---|---|---|---|
| Volume fraction of electrolyte el | 0.3 | 0.45 | 0.438 |
| Volume fraction of active material es | 0.55 | 0.55 | 0.505 |
| Bruggeman exponent γ | 1.5 | 2.3 | 4.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, H.-C.; Chen, K.-C.; Chen, C.-H. Electrochemical Change Induced by Spherical Indentation in Lithium-Ion Batteries. Batteries 2022, 8, 268. https://doi.org/10.3390/batteries8120268
Lin H-C, Chen K-C, Chen C-H. Electrochemical Change Induced by Spherical Indentation in Lithium-Ion Batteries. Batteries. 2022; 8(12):268. https://doi.org/10.3390/batteries8120268
Chicago/Turabian StyleLin, Huan-Cheng, Kuo-Ching Chen, and Chih-Hung Chen. 2022. "Electrochemical Change Induced by Spherical Indentation in Lithium-Ion Batteries" Batteries 8, no. 12: 268. https://doi.org/10.3390/batteries8120268
APA StyleLin, H.-C., Chen, K.-C., & Chen, C.-H. (2022). Electrochemical Change Induced by Spherical Indentation in Lithium-Ion Batteries. Batteries, 8(12), 268. https://doi.org/10.3390/batteries8120268





