Thermal Management of Lithium-Ion Batteries Based on Honeycomb-Structured Liquid Cooling and Phase Change Materials
Abstract
1. Introduction
2. Thermal Characteristic Experiments of Lithium-Ion Battery
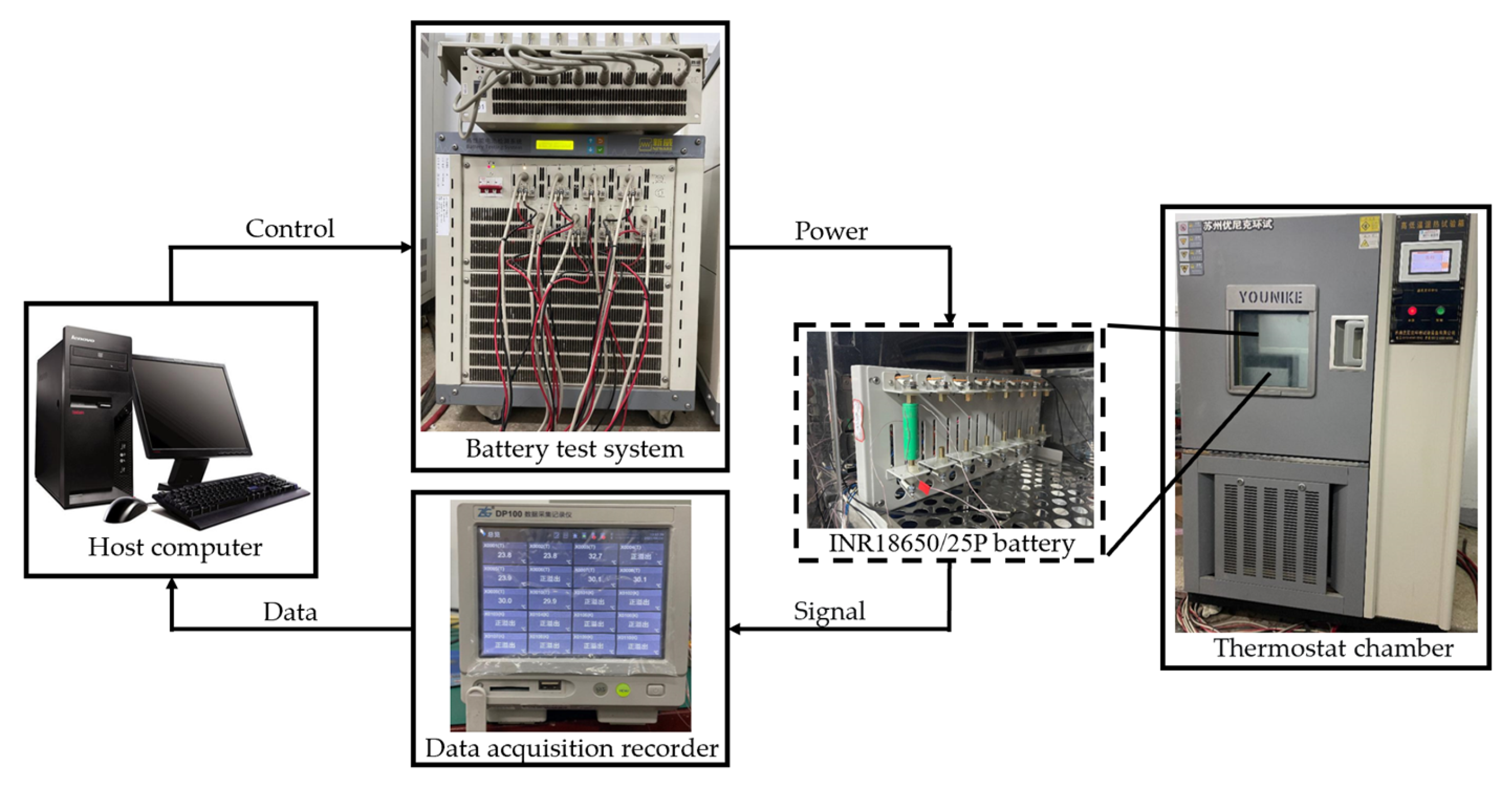
2.1. Experimental Setup
2.2. Internal Resistance Characteristic Test
- The fully discharged battery is placed in a thermostatic chamber with the temperature set at 23.6 °C and then charged completely in a standard charging mode of constant-current and constant-voltage (CCCV). After charging, the battery stands for one hour to keep its temperature consistent with the ambient temperature.
- Discharge the battery with a pulse current of 1C for 10 s, stop the current and stand for 40 s, and then charge the battery with a pulse current of 0.75C for 10 s; stop the loading current and stand for 40 s again. The change in the open-circuit voltage of the battery is collected and recorded during this process.
- Discharge the battery at a constant current of 1C until its state of charge (SOC) = 0.9, and stand for one hour to restore the battery temperature to the ambient temperature;
- Repeat Steps 2 and 3, so that when the SOC of the battery is at 0.1, 0.2, 0.3, 0.4, 0.5, 0.6, 0.7, 0.8, 0.9 and 1, the corresponding voltage abrupt changes can be recorded.
- Change the discharge current in Step 2 to other discharge rates of 2C, 3C and 5C, and repeat steps 2~4 to measure the total discharge internal resistance under different discharge rates and SOC.
2.3. Temperature Rise Characteristic Test
- The fully discharged battery is placed in a thermostatic chamber at 23.6 °C for one hour to make its temperature stable and consistent with the ambient temperature.
- Charge the battery with a constant current of 1.6C rate until the voltage reaches the charging cut-off voltage of 4.2 V, and then charge with a constant voltage of 4.2 V until the current achieves the charging cut-off current of 0.1 A.
- Place the fully charged battery in the thermostat for one hour again so that the battery temperature keeps in line with the ambient temperature of 23.6 °C.
- Discharge the battery at 1C constant current until the voltage reaches the discharge cut-off voltage of 2.5 V. Meanwhile, the temperature change in the battery at each thermocouple is monitored and recorded in real time.
- Repeat steps 1~4 and adjust the intensity of constant current in step 4 to 1C, 2C, 3C and 5C, respectively, until all experiments are completed.
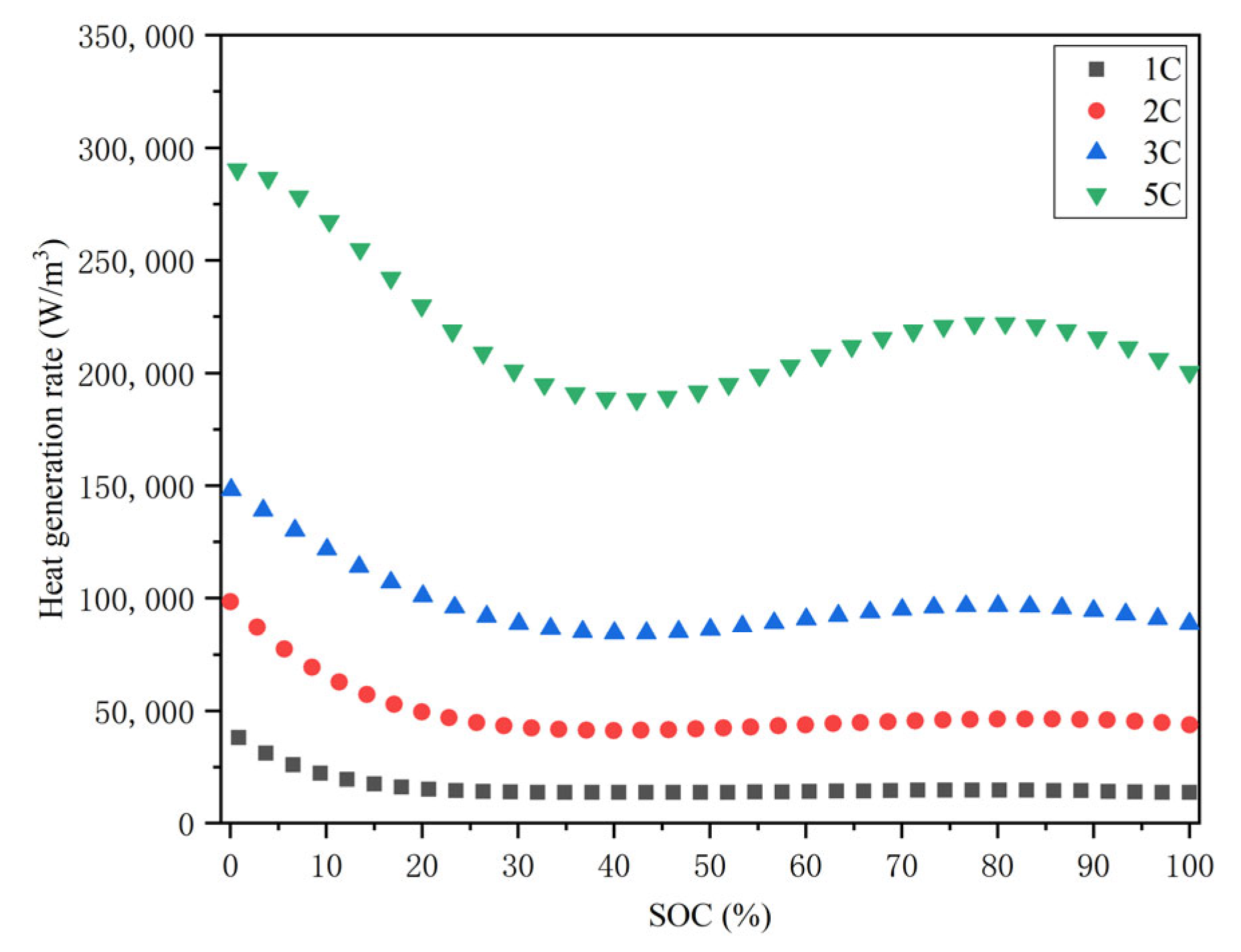
2.4. Heat Generation Rate of Single Battery
3. Numerical Model
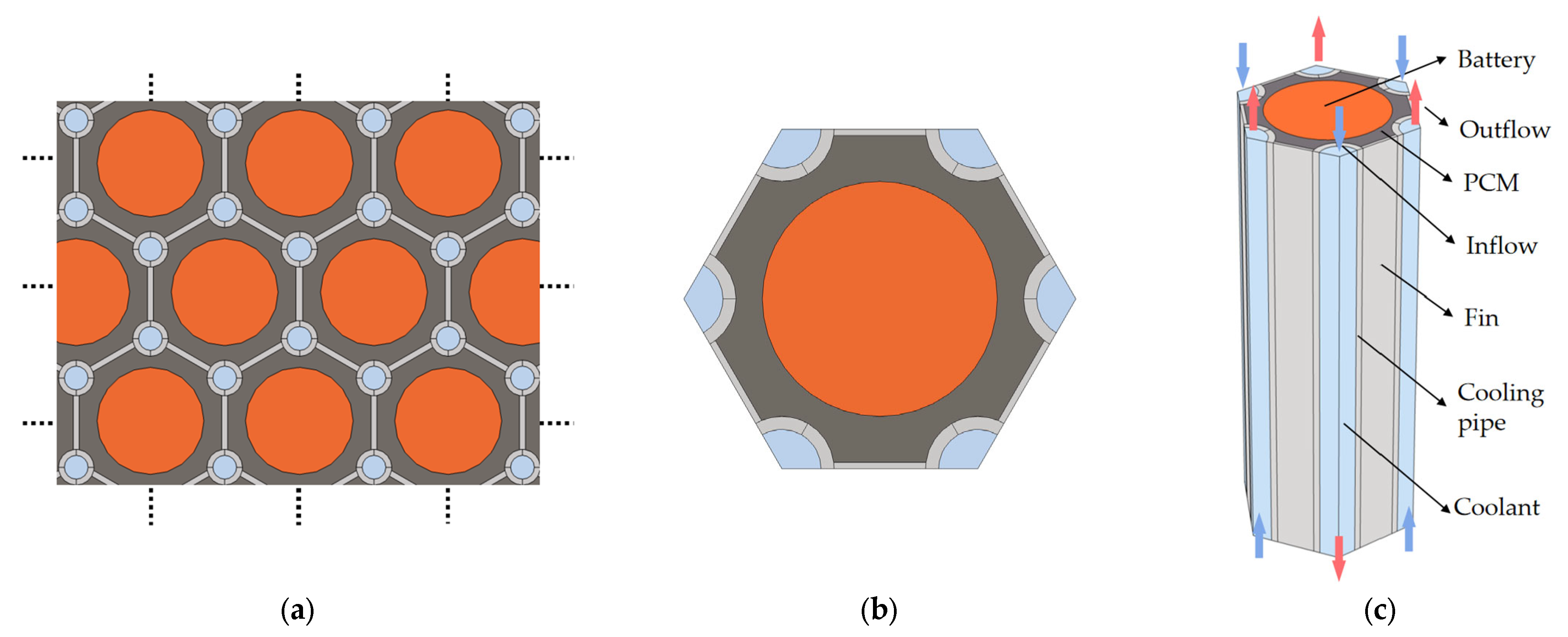
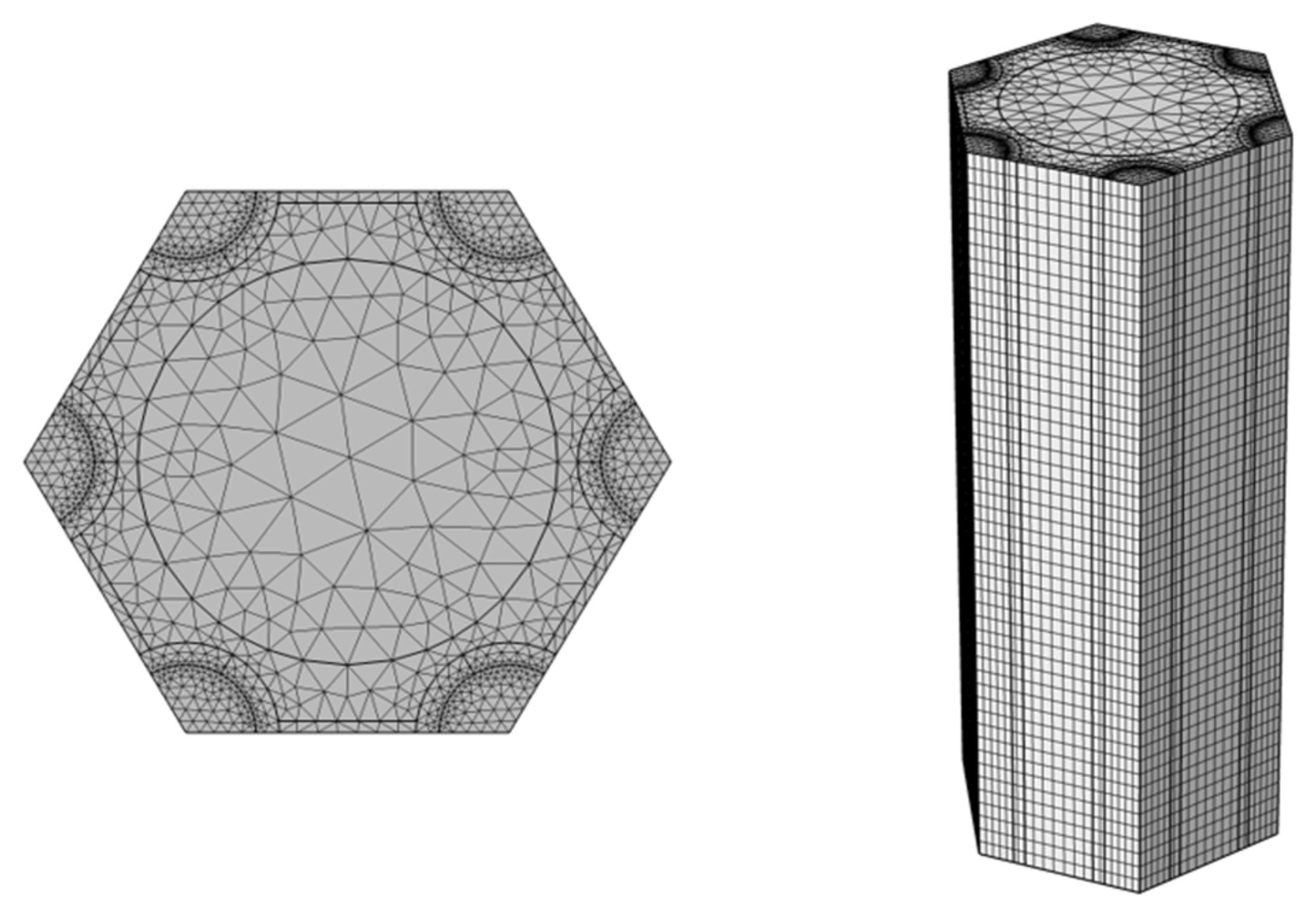
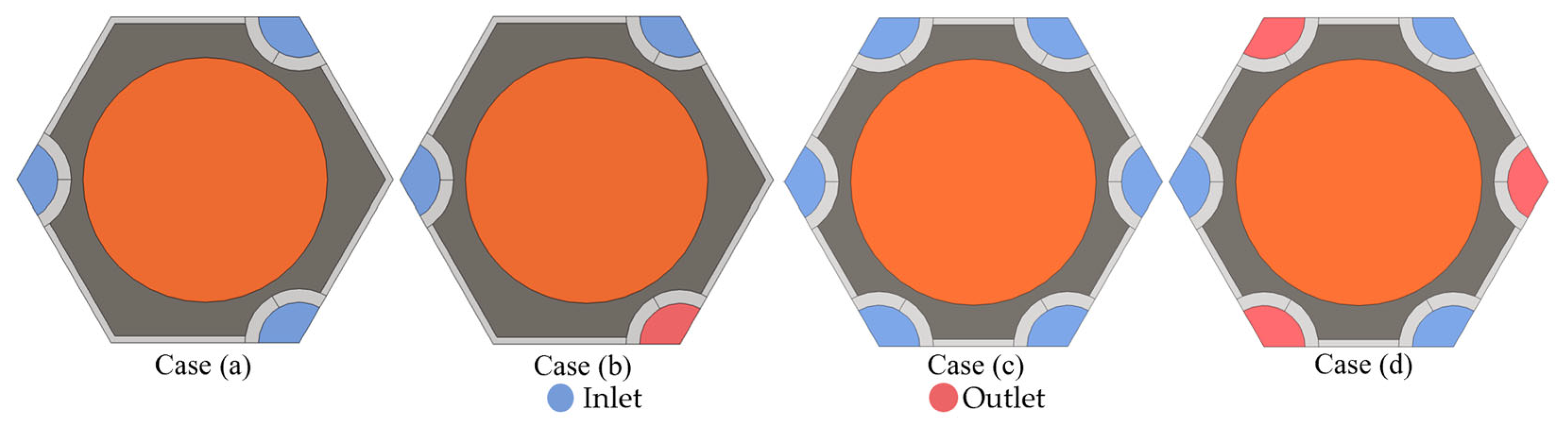
3.1. Geometric Model
3.2. Mathematical Model
3.2.1. Lithium-Ion Battery Model
3.2.2. Composite Phase Change Material Model
3.2.3. Liquid Cooling Model
3.3. Boundary Conditions
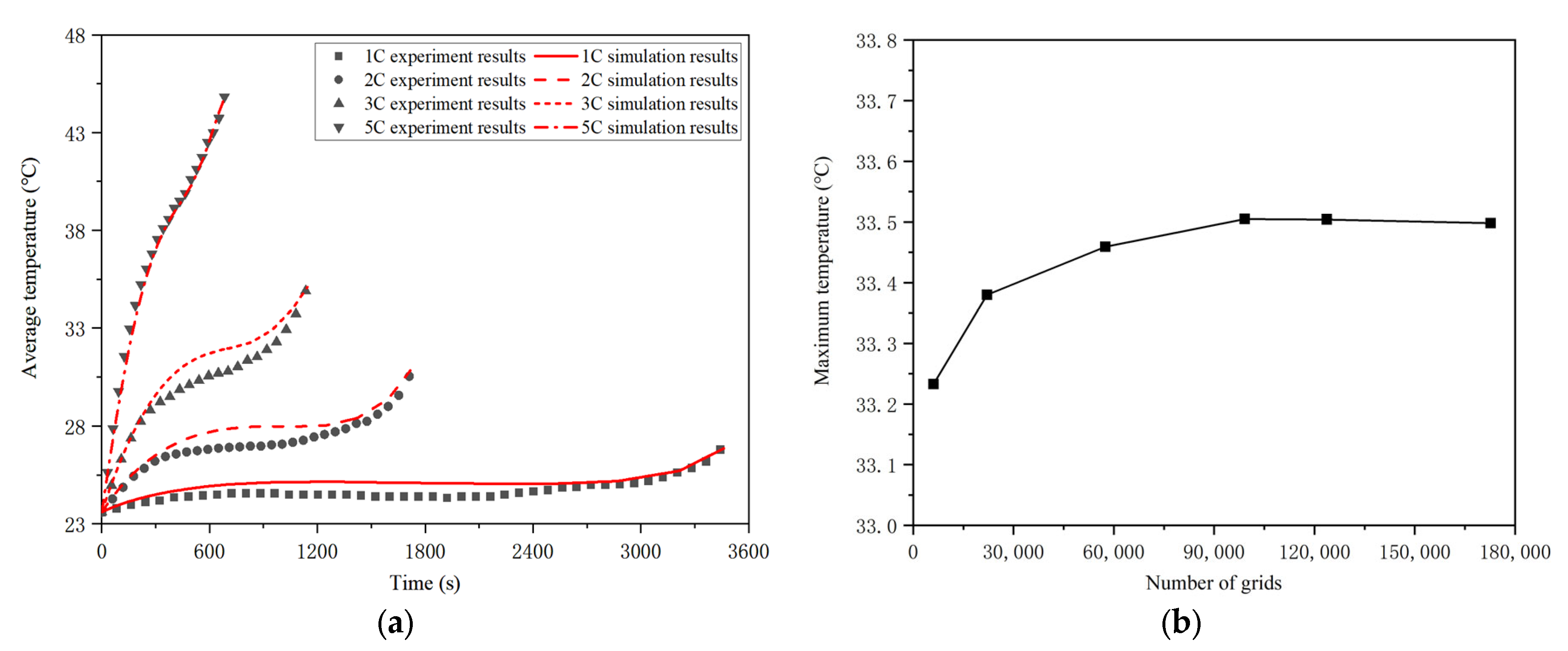
3.4. Model Verification
4. Results and Discussion
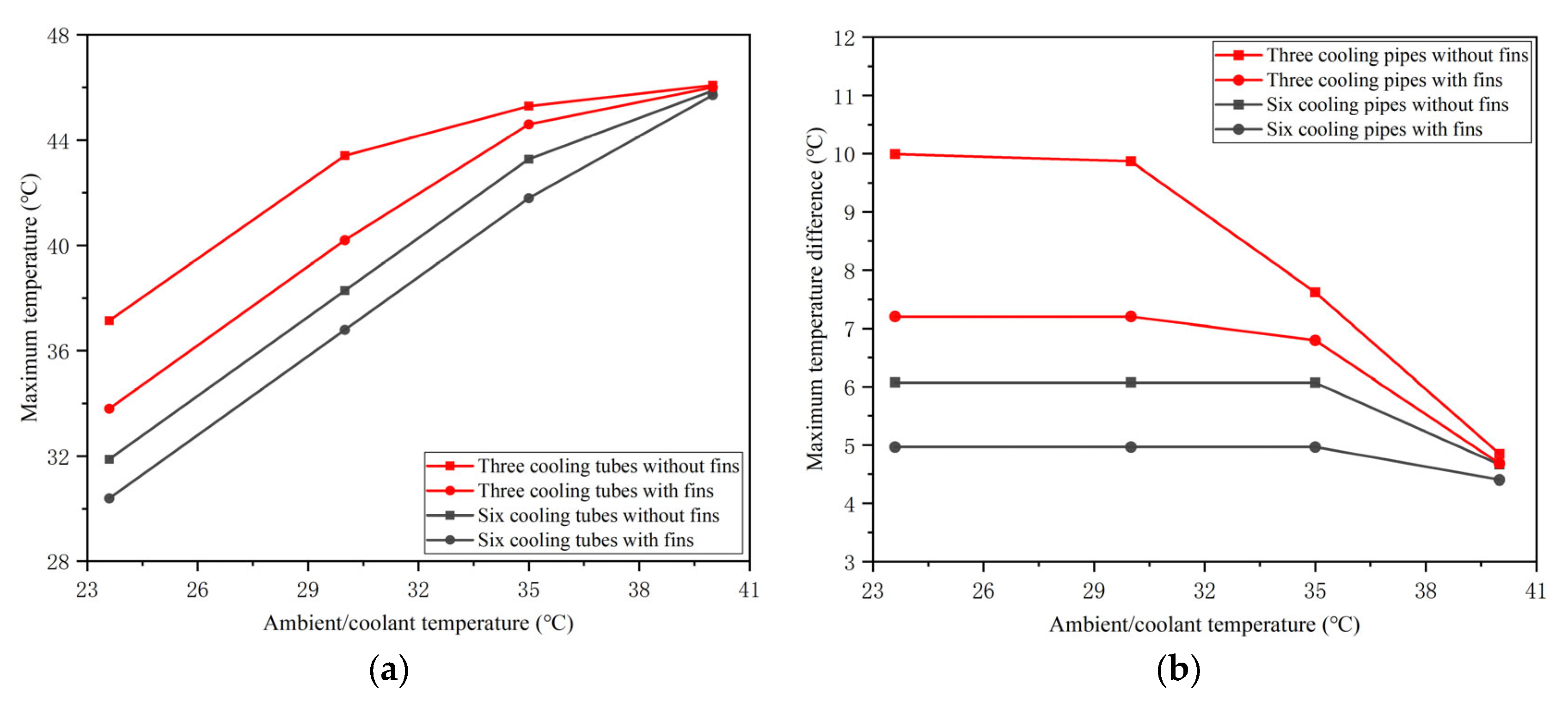
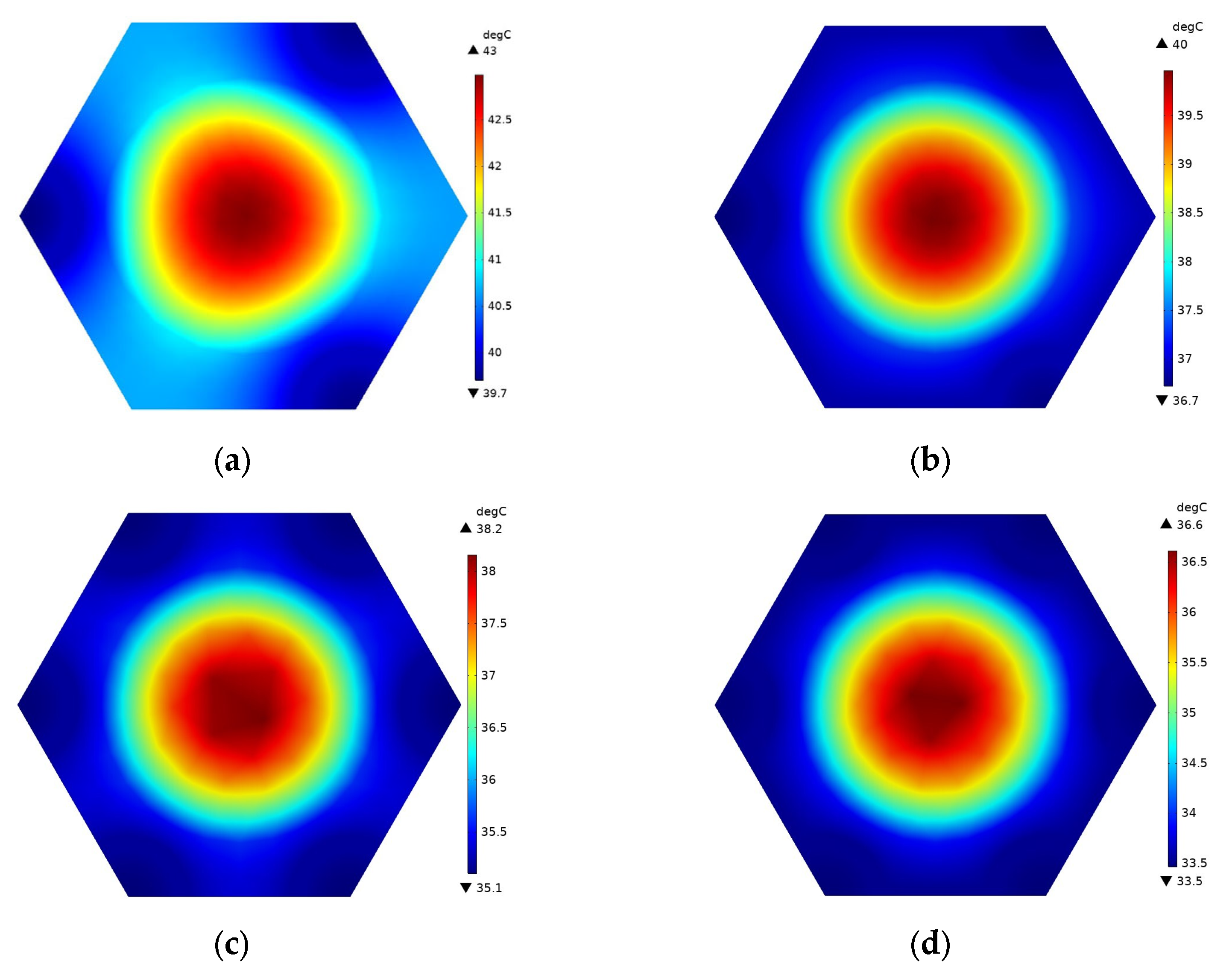
4.1. Effects of the Honeycomb Structure
4.2. Effects of the Coolant Flow Directions
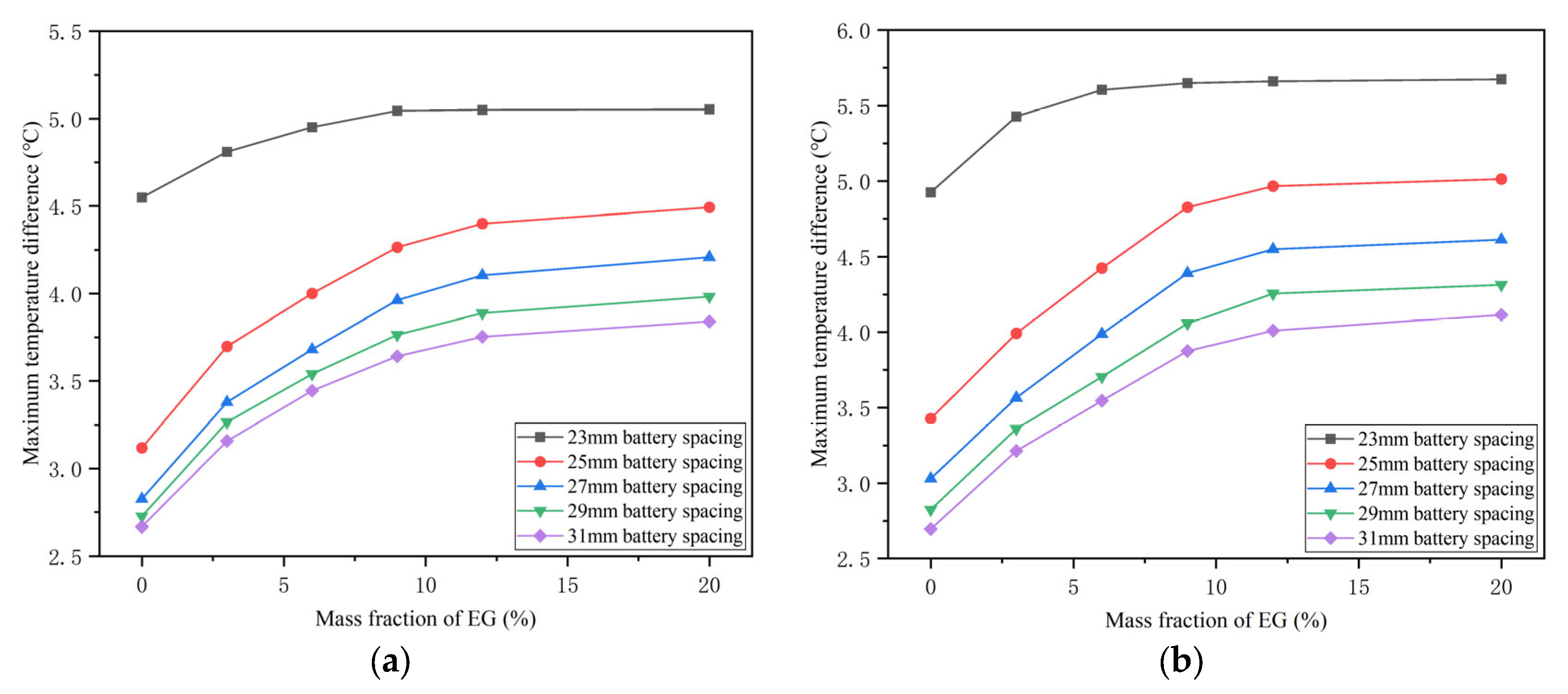
4.3. Effects of the Mass Fraction of EG
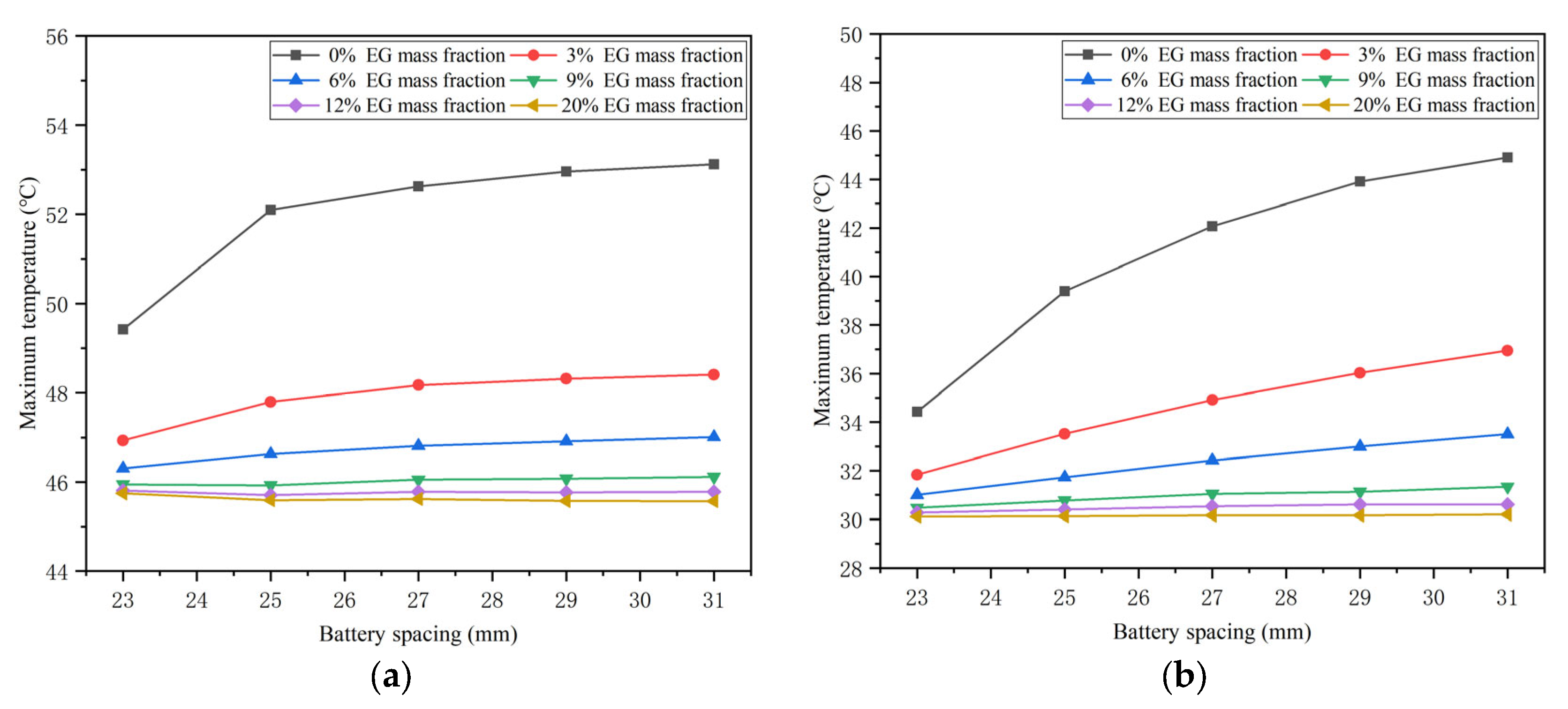
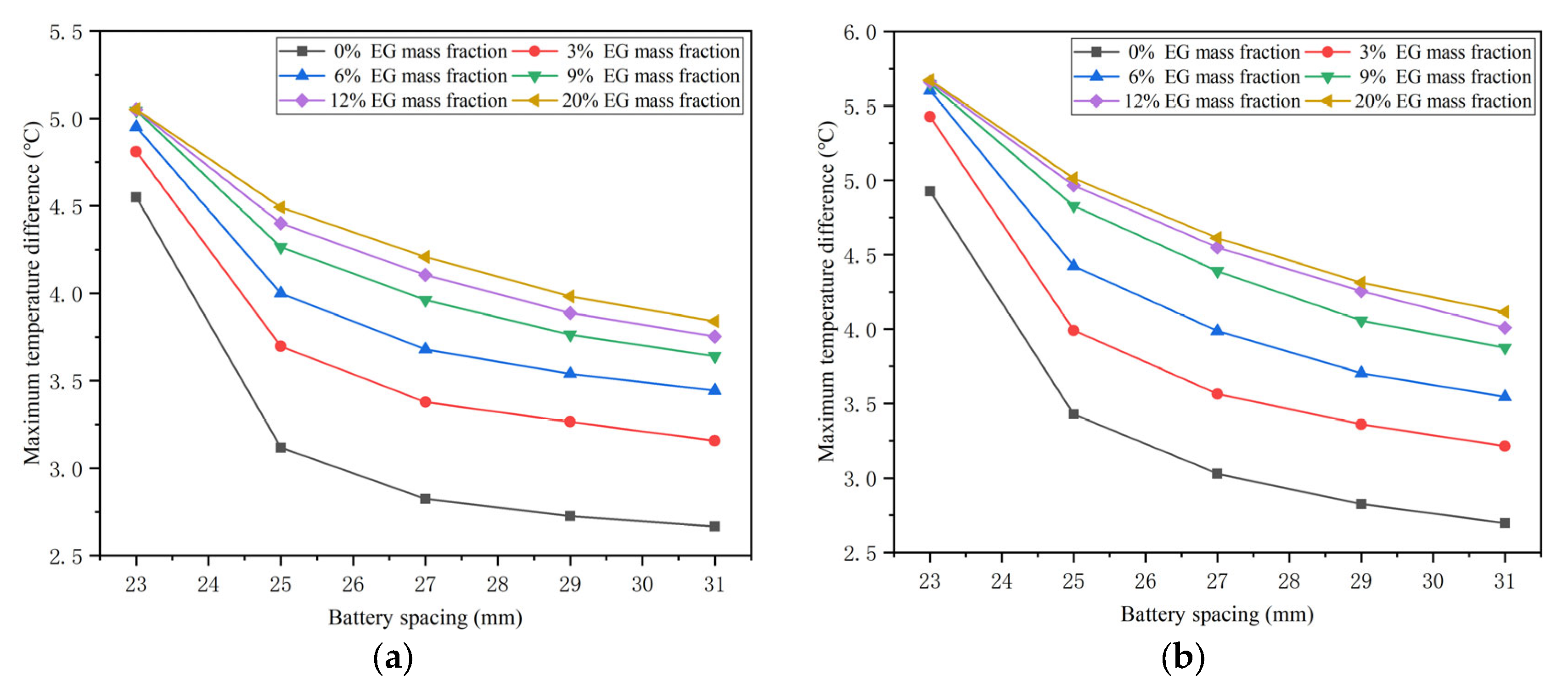
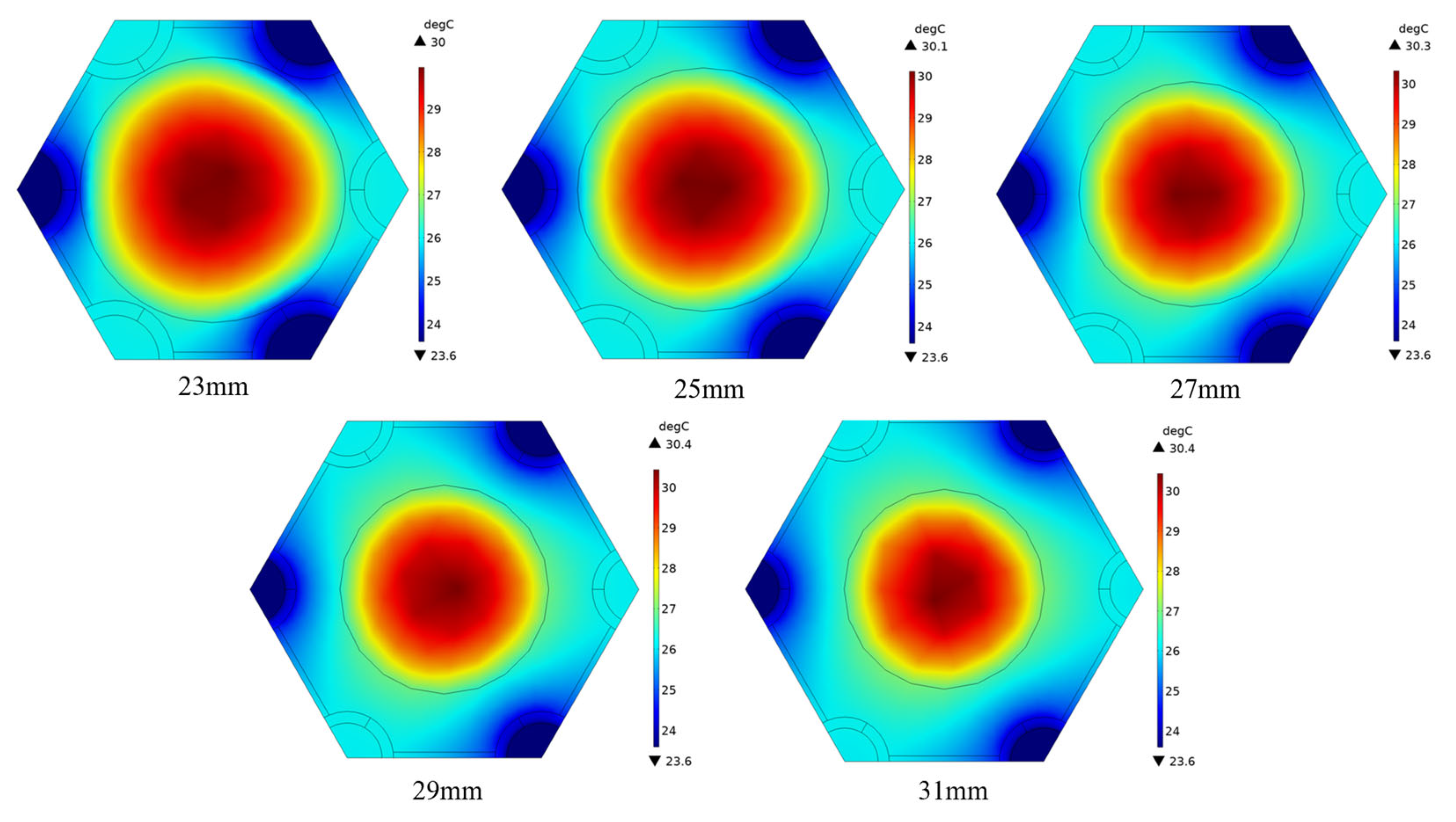
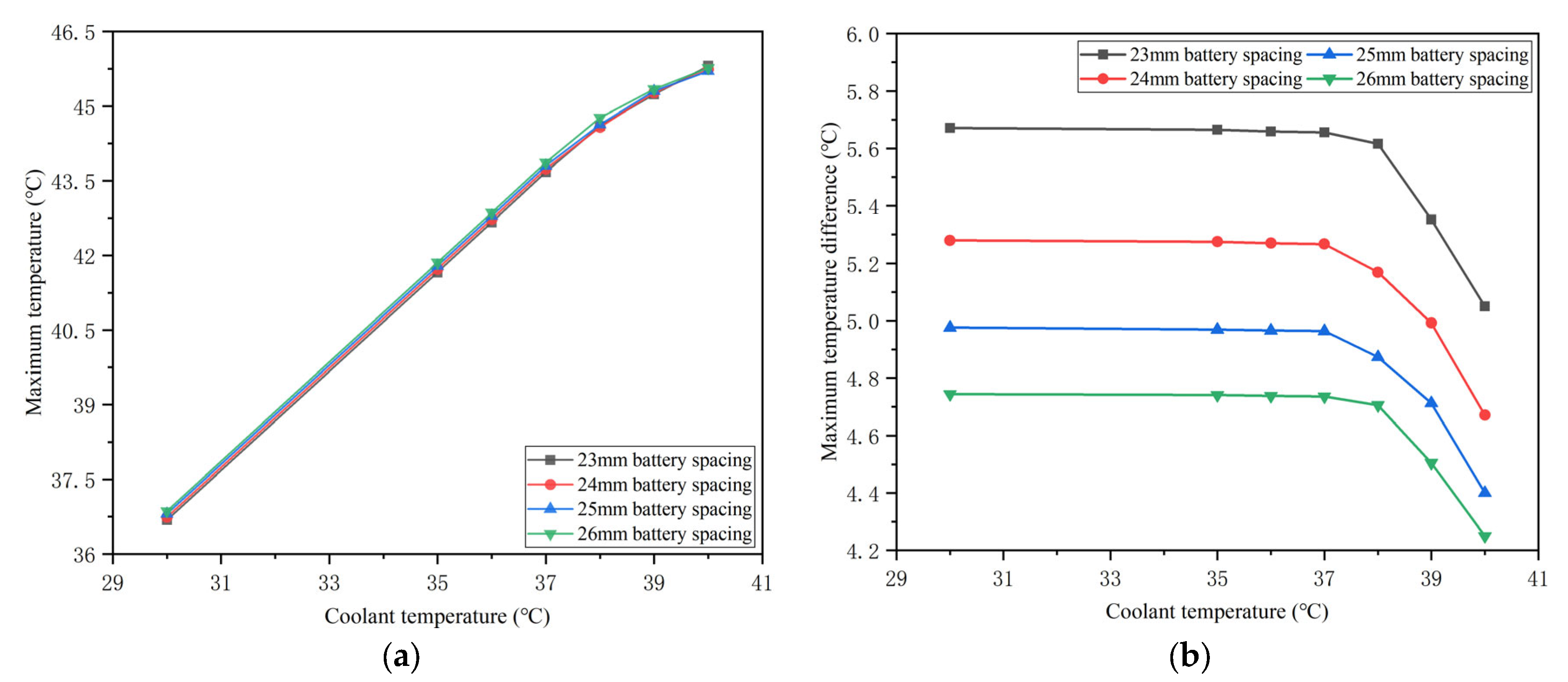
4.4. Effects of the Battery Spacing
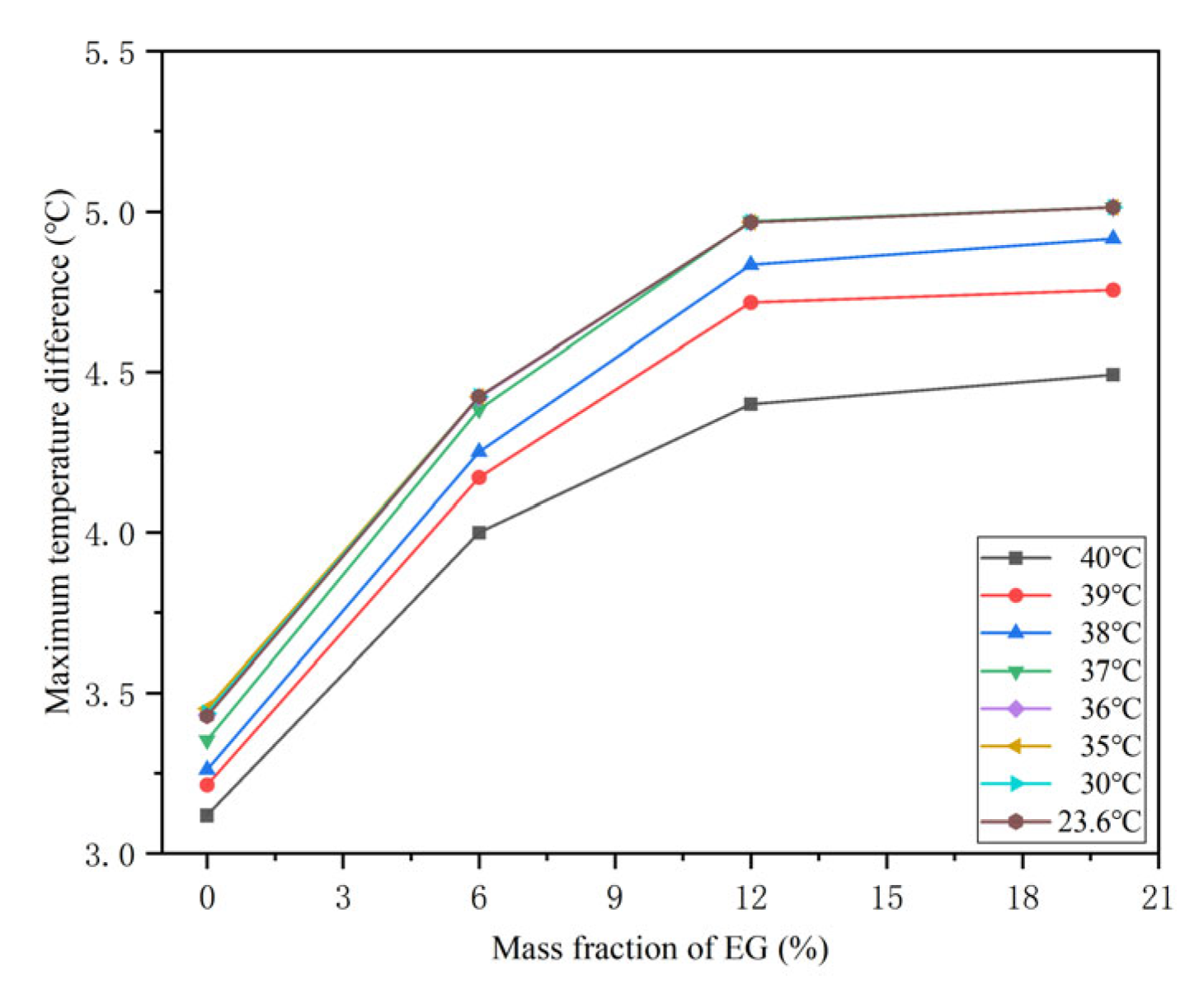
4.5. Effects of the Coolant Temperature
5. Conclusions
- The heat source expression of the battery can be obtained by combining the internal resistance and temperature rise experiment results with the Bernardi heat generation equation. According to the experiment results, the higher the discharge rate, the lower the total internal resistance and the higher the temperature rise at the same ambient temperature. In addition, with the decrease in the remaining energy, the total internal resistance of the battery is unchanged at first, and then it increases at the same ambient temperature and discharge rate.
- The proposed honeycomb structure composed of six cooling tubes and fins using the counter-flow coolant is the optimal basic scheme, which can significantly improve the thermal performance of the battery. In the environment of 40 °C, the maximum temperature and temperature difference in the battery can reach 45.71 °C and 4.4 °C, respectively. Moreover, the maximum battery temperature in this scheme will be further improved and can meet the uniformity requirements by decreasing the ambient temperature.
- The battery spacing and the mass fraction of expanded graphite (EG) jointly affect the thermal performance of the battery at different temperatures. As the spacing between batteries increases, the corresponding maximum temperature difference in the battery will decrease. However, the effect of battery spacing on the maximum temperature is determined by the mass fraction of EG. At a high EG mass fraction, battery spacing has little impact on the maximum temperature. While the EG mass fraction is low, the maximum temperature increases as the battery spacing rises. In addition, increasing the mass fraction of EG can lower the maximum temperature of the battery and increase the maximum temperature difference.
- The BTMS based on the honeycomb structure is relatively compact, and PCM plays the leading role of heat absorption at high temperatures, while liquid cooling has a significant effect at low temperatures. Meanwhile, precooling the coolant at high ambient temperatures can not only significantly reduce the battery temperature but also restore the heat storage capacity of PCM. Under the conditions of 40 °C ambient temperature and 5C discharge rate, the maximum battery temperature in the optimal BTMS cooled by 35 °C coolant is 41.79 °C, which is 4.39 °C lower than that of the corresponding pure PCM-based BTMS. Furthermore, when the temperature difference between the coolant and the environment is within 5 °C, the maximum temperature difference during the whole discharge process meets the uniformity requirements.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Landini, S.; Leworthy, J.; O’Donovan, T.S. A Review of Phase Change Materials for the Thermal Management and Isothermalisation of Lithium-Ion Cells. J. Storage Mater. 2019, 25, 100887. [Google Scholar] [CrossRef]
- Min, H.; Zhang, Z.; Sun, W.; Min, Z.; Yu, Y.; Wang, B. A thermal management system control strategy for electric vehicles under low-temperature driving conditions considering battery lifetime. Appl. Therm. Eng. 2020, 181, 115944. [Google Scholar] [CrossRef]
- Yang, X.; Deng, G.; Cai, Z.; Li, H.; Zeng, J.; Yang, H. Experimental study on novel composite phase change materials with room-temperature flexibility and high-temperature shape stability in a battery thermal management system. Int. J. Heat Mass Transfer 2023, 206, 123953. [Google Scholar] [CrossRef]
- Shelkea, A.V.; Buston, J.E.H.; Gill, J.; Howard, D.; Williams, R.C.E.; Read, E.; Abaza, A.; Cooper, B.; Richards, P.; Wen, J.X. Combined numerical and experimental studies of 21,700 lithium-ion battery thermal runaway induced by different thermal abuse. Int. J. Heat Mass Transfer 2022, 194, 123099. [Google Scholar] [CrossRef]
- Kang, D.; Lee, P.-Y.; Yoo, K.; Kim, J. Internal thermal network model-based inner temperature distribution of high-power lithium-ion battery packs with different shapes for thermal management. J. Storage Mater. 2020, 27, 101017. [Google Scholar] [CrossRef]
- Liu, H.; Wei, Z.; He, W.; Zhao, J. Thermal issues about Li-ion batteries and recent progress in battery thermal management systems: A review. Energy Convers. Manag. 2017, 150, 304–330. [Google Scholar] [CrossRef]
- Rao, Z.; Qian, Z.; Kuang, Y.; Li, Y. Thermal performance of liquid cooling based thermal management system for cylindrical lithium-ion battery module with variable contact surface. Appl. Therm. Eng. 2017, 123, 1514–1522. [Google Scholar] [CrossRef]
- Landini, S.; O’Donovan, T.S. Experimental investigation of lithium-ion cells ageing under isothermal conditions for optimal lifetime performance. J. Storage Mater. 2022, 48, 103680. [Google Scholar] [CrossRef]
- Lisbona, D.; Snee, T. A review of hazards associated with primary lithium and lithium-ion batteries. Process Saf. Environ. Prot. 2011, 89, 434–442. [Google Scholar] [CrossRef]
- Wang, Q.; Jiang, B.; Li, B.; Yan, Y. A critical review of thermal management models and solutions of lithium-ion batteries for the development of pure electric vehicles. Renew. Sustain. Energy Rev. 2016, 64, 106–128. [Google Scholar] [CrossRef]
- Akinlabi, A.A.H.; Solyali, D. Configuration, design, and optimization of air-cooled battery thermal management system for electric vehicles: A review. Renew. Sustain. Energy Rev. 2020, 125, 109815. [Google Scholar] [CrossRef]
- Weragoda, D.M.; Tian, G.; Burkitbayev, A.; Lo, K.-H.; Zhang, T. A comprehensive review on heat pipe based battery thermal management systems. Appl. Therm. Eng. 2023, 224, 120070. [Google Scholar] [CrossRef]
- Panchal, S.; Dincer, I.; Agelin-Chaab, M.; Fraser, R.; Fowler, M. Experimental and theoretical investigation of temperature distributions in a prismatic lithium-ion battery. Int. J. Therm. Sci. 2016, 99, 204–212. [Google Scholar] [CrossRef]
- Gungor, S.; Cetkin, E.; Lorente, S. Canopy-to-canopy liquid cooling for the thermal management of lithium-ion batteries, a constructal approach. Int. J. Heat Mass Transfer 2022, 182, 121918. [Google Scholar] [CrossRef]
- Tong, W.; Somasundaram, K.; Birgersson, E.; Mujumdar, A.S.; Yap, C. Numerical investigation of water cooling for a lithium-ion bipolar battery pack. Int. J. Therm. Sci. 2015, 94, 259–269. [Google Scholar] [CrossRef]
- Yin, B.; Zuo, S.; Xu, Y.; Chen, S. Performance of liquid cooling battery thermal management system in vibration environment. J. Storage Mater. 2022, 53, 105232. [Google Scholar] [CrossRef]
- Lai, Y.; Wu, W.; Chen, K.; Wang, S.; Xin, C. A compact and lightweight liquid-cooled thermal management solution for cylindrical lithium-ion power battery pack. Int. J. Heat Mass Transfer 2019, 144, 118581. [Google Scholar] [CrossRef]
- Liu, Z.; Wang, H.; Yang, C.; Zhao, J. Simulation study of lithium-ion battery thermal management system based on a variable flow velocity method with liquid metal. Appl. Therm. Eng. 2020, 179, 115578. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, Z.; Min, H.; Li, H.; Li, Q. Performance investigation of a passive battery thermal management system applied with phase change material. J. Storage Mater. 2021, 35, 102279. [Google Scholar] [CrossRef]
- Lazrak, A.; Fourmigué, J.-F.; Robin, J.-F. An innovative practical battery thermal management system based on phase change materials: Numerical and experimental investigations. Appl. Therm. Eng. 2018, 128, 20–32. [Google Scholar] [CrossRef]
- Luo, J.; Zou, D.; Wang, Y.; Wang, S.; Huang, L. Battery thermal management systems (BTMs) based on phase change material (PCM): A comprehensive review. Chem. Eng. J 2022, 430, 132741. [Google Scholar] [CrossRef]
- He, J.; Yang, X.; Zhang, G. A phase change material with enhanced thermal conductivity and secondary heat dissipation capability by introducing a binary thermal conductive skeleton for battery thermal management. Appl. Therm. Eng. 2019, 148, 984–991. [Google Scholar] [CrossRef]
- Jiang, G.; Huang, J.; Fu, Y.; Cao, M.; Liu, M. Thermal optimization of composite phase change material/expanded graphite for Li-ion battery thermal management. Appl. Therm. Eng. 2016, 108, 1119–1125. [Google Scholar] [CrossRef]
- Mauro, G.M.; Iasiello, M.; Bianco, N.; Chiu, W.K.S.; Naso, V. Mono- and Multi-Objective CFD Optimization of Graded Foam-Filled Channels. Materials 2022, 15, 968. [Google Scholar] [CrossRef] [PubMed]
- Verma, A.; Rakshit, D. Performance analysis of PCM-fin combination for heat abatement of Li-ion battery pack in electric vehicles at high ambient temperature. Therm. Sci. Eng. Prog 2022, 32, 101314. [Google Scholar] [CrossRef]
- Arshad, A.; Jabbal, M.; Sardari, P.T.; Bashir, M.A.; Faraji, H.; Yan, Y. Transient simulation of finned heat sinks embedded with PCM for electronics cooling. Therm. Sci. Eng. Prog 2020, 18, 100520. [Google Scholar] [CrossRef]
- Bianco, N.; Busiello, S.; Iasiello, M.; Mauro, G.M. Finned heat sinks with phase change materials and metal foams: Pareto optimization to address cost and operation time. Appl. Therm. Eng. 2021, 197, 117436. [Google Scholar] [CrossRef]
- Lebrouhi, B.E.; Lamrani, B.; Ouassaid, M.; Abd-Lefdil, M.; Maaroufi, M.; Kousksou, T. Low-cost numerical lumped modelling of lithium-ion battery pack with phase change material and liquid cooling thermal management system. J. Storage Mater. 2022, 54, 105293. [Google Scholar] [CrossRef]
- Fan, Y.; Wang, Z.; Xiong, X.; Zhu, J.; Gao, Q.; Wang, H.; Wu, H. Novel concept design of low energy hybrid battery thermal management system using PCM and multistage Tesla valve liquid cooling. Appl. Therm. Eng. 2023, 220, 119680. [Google Scholar] [CrossRef]
- Xin, Q.; Xiao, J.; Yang, T.; Zhang, H.; Long, X. Thermal management of lithium-ion batteries under high ambient temperature and rapid discharging using composite PCM and liquid cooling. Appl. Therm. Eng. 2022, 210, 118230. [Google Scholar] [CrossRef]
- Weng, J.; Xiao, C.; Yang, X.; Ouyang, D.; Chen, M.; Zhang, G.; Lee Waiming, E.; Kit Yuen, R.K.; Wang, J. An energy-saving battery thermal management strategy coupling tubular phase-change-material with dynamic liquid cooling under different ambient temperatures. Renew. Energ. 2022, 195, 918–930. [Google Scholar] [CrossRef]
- Peng, P.; Wang, Y.; Jiang, F. Numerical study of PCM thermal behavior of a novel PCM-heat pipe combined system for Li-ion battery thermal management. Appl. Therm. Eng. 2022, 209, 118293. [Google Scholar] [CrossRef]
- Qi, X.; Sajadi, S.M.; Mahmoud, M.Z.; Li, Z.; Shamseldin, M.A.; Aybar, H.Ş. Study of circular, horizontal and vertical elliptical enclosures filled with phase change material in thermal management of lithium-ion batteries in an air-cooled system. J. Storage Mater. 2022, 53, 105041. [Google Scholar] [CrossRef]
- Yang, W.; Zhou, F.; Liu, Y.; Xu, S.; Chen, X. Thermal performance of honeycomb-like battery thermal management system with bionic liquid mini-channel and phase change materials for cylindrical lithium-ion battery. Appl. Therm. Eng. 2021, 188, 116649. [Google Scholar] [CrossRef]
- Zhao, D.; Lei, Z.; An, C. Research on battery thermal management system based on liquid cooling plate with honeycomb-like flow channel. Appl. Therm. Eng. 2023, 218, 119324. [Google Scholar] [CrossRef]
- Wang, S.; Fernandez, C.; Yu, C.; Fan, Y.; Cao, W.; Stroe, D.-I. A novel charged state prediction method of the lithium ion battery packs based on the composite equivalent modeling and improved splice Kalman filtering algorithm. J. Power Sources 2020, 471, 228450. [Google Scholar] [CrossRef]
- Landini, S.; O’Donovan, T.S. Novel experimental approach for the characterisation of Lithium-Ion cells performance in isothermal conditions. Energy 2021, 214, 118965. [Google Scholar] [CrossRef]
- Newman, J.; Bernardi, D.; Pawlikowski, E. A General Energy-Balance for Battery Systems. J. Electrochem. Soc. 1985, 132, 5. [Google Scholar]
- Liu, F.; Wang, J.; Liu, Y.; Wang, F.; Yang, N.; Liu, X.; Liu, H.; Li, W.; Liu, H.; Huang, B. Performance analysis of phase change material in battery thermal management with biomimetic honeycomb fin. Appl. Therm. Eng. 2021, 196, 117296. [Google Scholar] [CrossRef]
- Weng, J.; He, Y.; Ouyang, D.; Yang, X.; Chen, M.; Cui, S.; Zhang, G.; Yuen, R.K.K.; Wang, J. Honeycomb-inspired design of a thermal management module and its mitigation effect on thermal runaway propagation. Appl. Therm. Eng. 2021, 195, 117147. [Google Scholar] [CrossRef]
- Lu, J. The Research and Simulation Analysis of Heat Dissipation with Liquid Cooling for the Power Battery Pack of Pure Electric Vehicles. Master’ Thesis, University of Electronic Science and Technology of China, Chengdu, China, 2018. [Google Scholar]
- Ling, Z.; Chen, J.; Xu, T.; Fang, X.; Gao, X.; Zhang, Z. Thermal conductivity of an organic phase change material/expanded graphite composite across the phase change temperature range and a novel thermal conductivity model. Energy Convers. Manag. 2015, 102, 202–208. [Google Scholar] [CrossRef]







| Key Indicators | Data |
|---|---|
| Nominal capacity | 2500 mAh |
| Nominal voltage | 3.6 V |
| Standard charging mode | 1.25 A constant current charge to 4.20 V, constant voltage charge to 100 mA |
| Charge/Discharge cut-off voltage | 4.2 V/2.5 V |
| Maximum charge/discharge current | 4 A/30 A |
| Battery height | 65.00 ± 0.15 mm |
| Battery diameter | 18.35 ± 0.10 mm |
| Battery weight | 48 g |
| Experimental Instruments | Accuracy |
|---|---|
| Thermostat chamber | 0.5% |
| Data acquisition recorder | 0.05% |
| T-type thermocouples | 1% |
| Battery test system | 0.2% |
| Discharge Rate | |||||||
|---|---|---|---|---|---|---|---|
| 1C | 13,752.01 | −0.491 | 0.0117 | −2.047 × 10−5 | 1.403 × 10−8 | −4.368 × 10−12 | 5.202 × 10−16 |
| 2C | 43,722.65 | 20.752 | −0.0496 | 3.731 × 10−5 | −1.376 × 10−8 | −2.123 × 10−12 | 5.01 × 10−15 |
| 3C | 88,659.77 | 60.361 | −0.0496 | −3.859 × 10−4 | 5.395 × 10−7 | −5.914 × 10−11 | −7.776 × 10−14 |
| 5C | 200,078.4 | 280.647 | −0.5359 | −3.14 × 10−3 | 3.359 × 10−6 | 1.409 × 10−8 | −1.651 × 10−11 |
| Materials | Density | Specific Heat Capacity | Thermal Conductivity | Dynamic Viscosity |
|---|---|---|---|---|
| kg/m3 | J/(kg·K) | W/(m·K) | Pa·s | |
| Battery | 2755.9 | 1129.95 | Radial 1.6 | - |
| Axial 27 | ||||
| Aluminum | 2719 | 871 | 238 | - |
| Water | 998 | 4180 | 0.599 | 1.01 × 10−3 |
| CPCM | Specific Heat Capacity | Thermal Conductivity | Latent Heat | Melting Temperature | Density |
|---|---|---|---|---|---|
| J/(kg·K) | W/(m·K) | kJ/kg | K | kg/m3 | |
| PA/0 wt%EG | 2000 | 0.2 | 275 | 314–317 | 800 |
| PA/3 wt%EG | 1963 | 0.58 | 266.8 | 314–317 | 825 |
| PA/6 wt%EG | 1926 | 1.23 | 258.5 | 314–317 | 832 |
| PA/9 wt%EG | 1889 | 3.15 | 250.3 | 314–317 | 845 |
| PA/12 wt%EG | 1852 | 5.74 | 242 | 314–317 | 897 |
| PA/20 wt%EG | 1754 | 10.6 | 220 | 314–317 | 913 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, T.; Su, S.; Xin, Q.; Zeng, J.; Zhang, H.; Zeng, X.; Xiao, J. Thermal Management of Lithium-Ion Batteries Based on Honeycomb-Structured Liquid Cooling and Phase Change Materials. Batteries 2023, 9, 287. https://doi.org/10.3390/batteries9060287
Yang T, Su S, Xin Q, Zeng J, Zhang H, Zeng X, Xiao J. Thermal Management of Lithium-Ion Batteries Based on Honeycomb-Structured Liquid Cooling and Phase Change Materials. Batteries. 2023; 9(6):287. https://doi.org/10.3390/batteries9060287
Chicago/Turabian StyleYang, Tianqi, Shenglin Su, Qianqian Xin, Juan Zeng, Hengyun Zhang, Xianyou Zeng, and Jinsheng Xiao. 2023. "Thermal Management of Lithium-Ion Batteries Based on Honeycomb-Structured Liquid Cooling and Phase Change Materials" Batteries 9, no. 6: 287. https://doi.org/10.3390/batteries9060287
APA StyleYang, T., Su, S., Xin, Q., Zeng, J., Zhang, H., Zeng, X., & Xiao, J. (2023). Thermal Management of Lithium-Ion Batteries Based on Honeycomb-Structured Liquid Cooling and Phase Change Materials. Batteries, 9(6), 287. https://doi.org/10.3390/batteries9060287








