Brochosome-Inspired Metal-Containing Particles as Biomimetic Building Blocks for Nanoplasmonics: Conceptual Generalizations
Abstract
:1. Introduction
2. Materials and Methods
3. Results
3.1. Classification
3.1.1. Structural Replicas
3.1.2. Conceptual Generalizations
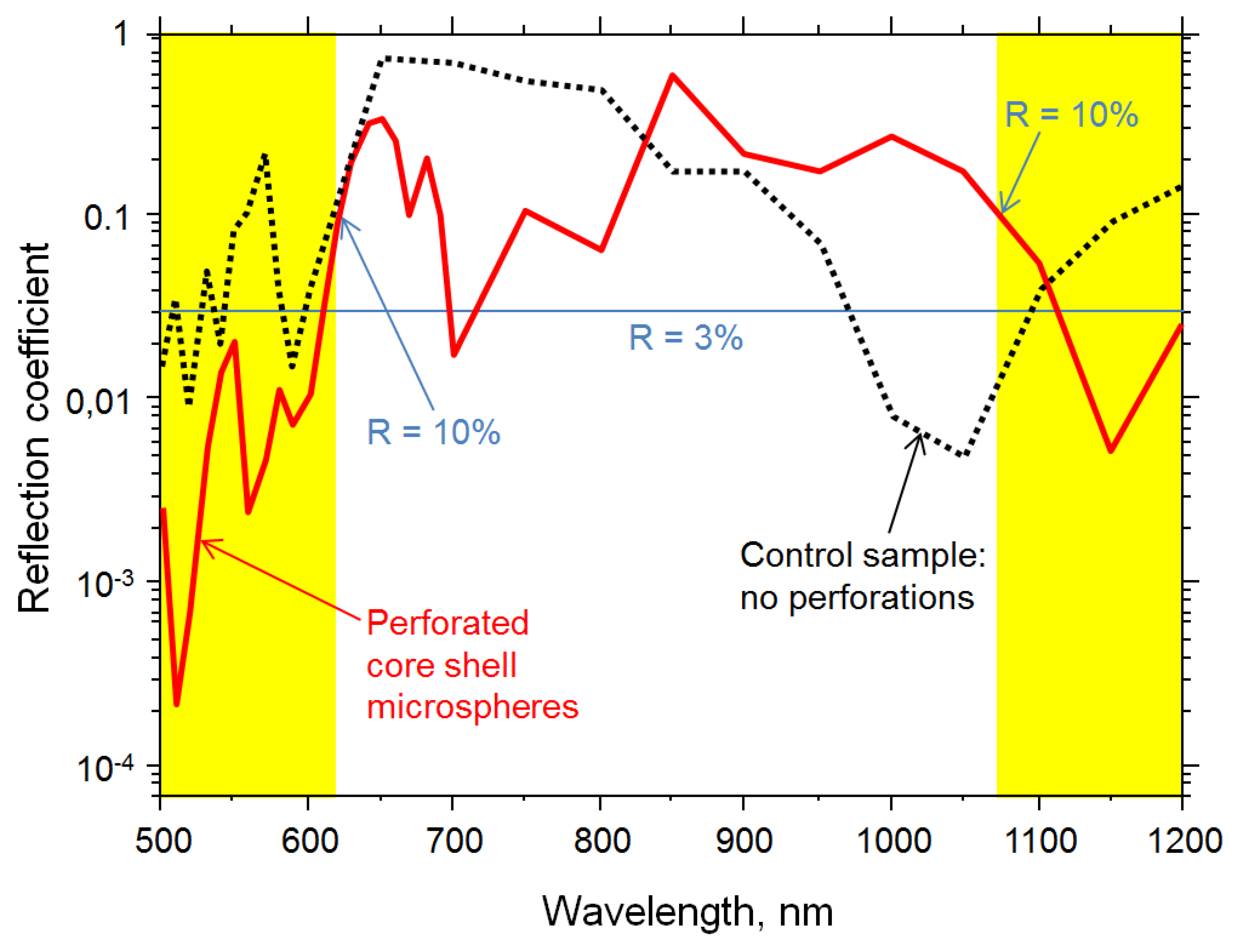
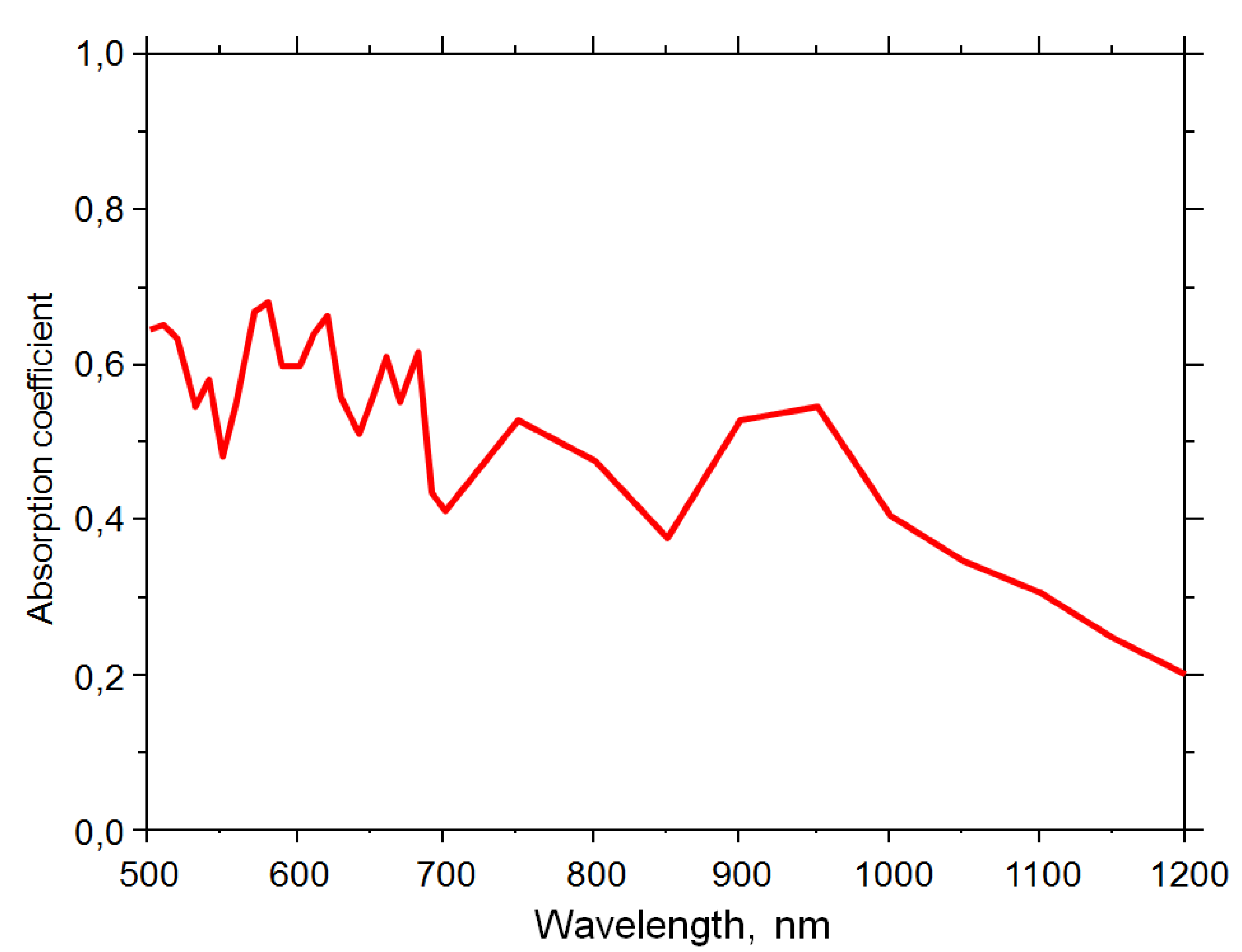
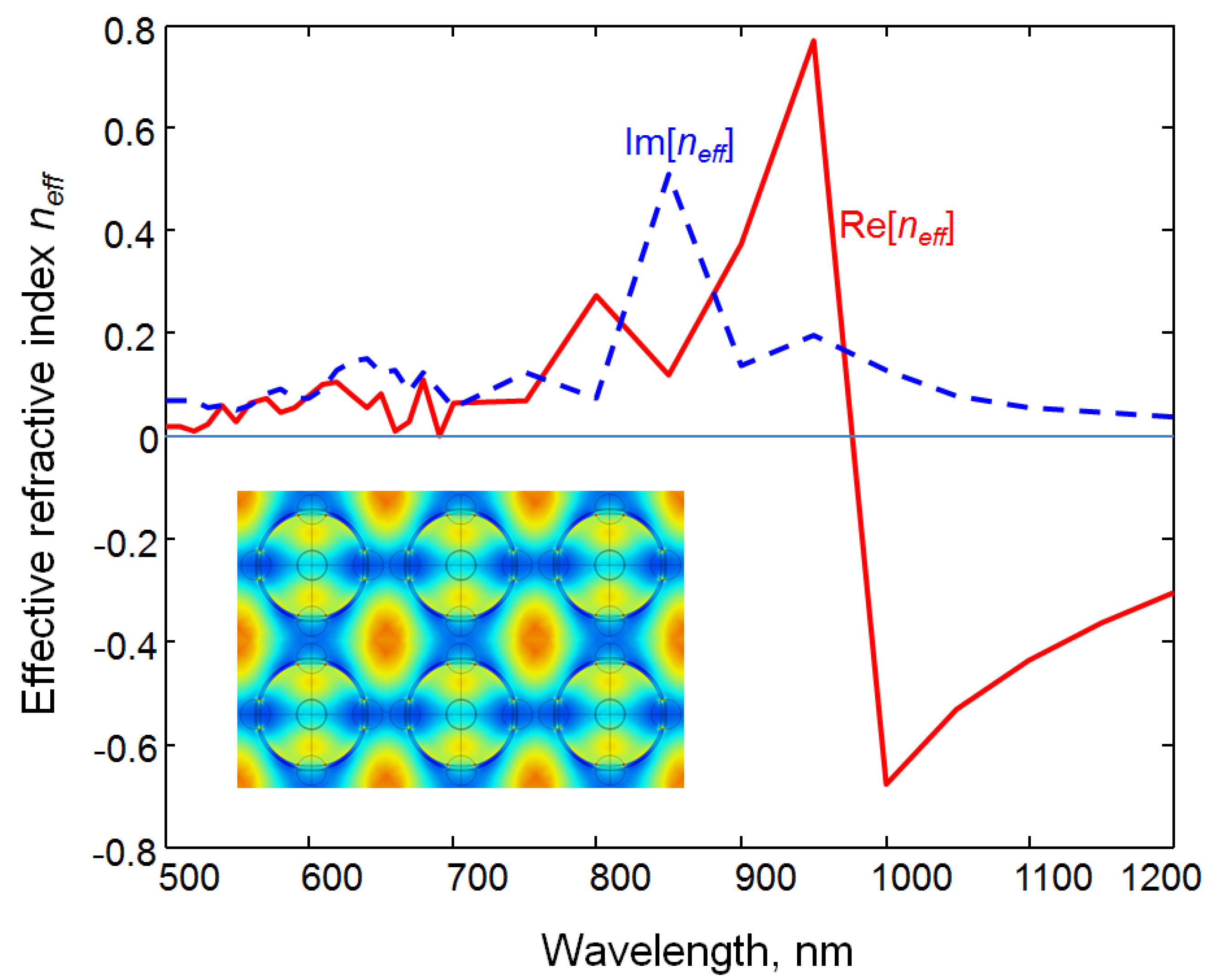
3.2. Simulations
4. Discussion and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Maier, S.A. Plasmonics: Fundamentals and Applications; Springer: New York, NY, USA, 2007. [Google Scholar] [CrossRef]
- Barnes, W.L.; Dereux, A.; Ebbesen, T.W. Surface plasmon subwavelength optics. Nature 2003, 424, 824–830. [Google Scholar] [CrossRef]
- Ozbay, E. Plasmonics: Merging Photonics and Electronics at Nanoscale Dimensions. Science 2006, 311, 189–193. [Google Scholar] [CrossRef]
- Schuller, J.A.; Barnard, E.S.; Cai, W.; Jun, Y.C.; White, J.S.; Brongersma, M.L. Plasmonics for extreme light concentration and manipulation. Nat. Mater. 2010, 9, 193–204. [Google Scholar] [CrossRef]
- Gramotnev, D.K.; Bozhevolnyi, S.I. Plasmonics beyond the diffraction limit. Nat. Photonics 2010, 4, 83–91. [Google Scholar] [CrossRef]
- Atwater, H.A.; Polman, A. Plasmonics for improved photovoltaic devices. Nat. Mater. 2010, 9, 205–213. [Google Scholar] [CrossRef]
- Andress, W.F.; Yoon, H.; Yeung, K.Y.M.; Qin, L.; West, K.; Pfeiffer, L.; Ham, D. Ultra-Subwavelength Two-Dimensional Plasmonic Circuits. Nano Lett. 2012, 12, 2272–2277. [Google Scholar] [CrossRef] [PubMed]
- Lim, D.K.; Jeon, K.S.; Kim, H.M.; Nam, J.M.; Suh, Y.D. Nanogap-engineerable raman-active nanodumbbells for single-molecule detection. Nat. Mater. 2010, 9, 60–67. [Google Scholar] [CrossRef] [PubMed]
- Choi, M.; Lee, S.H.; Kim, Y.; Kang, S.B.; Shin, J.; Kwak, M.H.; Kang, K.Y.; Lee, Y.H.; Park, N.; Min, B. A terahertz metamaterial with unnaturally high refractive index. Nature 2011, 470, 369–373. [Google Scholar] [CrossRef]
- Schwartz, B.T.; Piestun, R. Total external reflection from metamaterials with ultralow refractive index. J. Opt. Soc. Am. B 2003, 20, 2448–2453. [Google Scholar] [CrossRef]
- Smith, D.R.; Pendry, J.B.; Wiltshire, M.C.K. Metamaterials and Negative Refractive Index. Science 2004, 305, 788–792. [Google Scholar] [CrossRef] [Green Version]
- Cai, W.; Shalaev, V. Optical Metamaterials: Fundamentals and Applications; Springer: Dordrecht, The Netherlands, 2009. [Google Scholar] [CrossRef] [Green Version]
- Garrrett, N.L.; Vukusic, P.; Ogrin, F.; Sirotkin, E.; Winlove, C.P.; Moger, J. Spectroscopy on the wing: Naturally inspired SERS substrates for biochemical analysis. J. Biophotonics 2009, 2, 157–166. [Google Scholar] [CrossRef]
- Jakšić, Z.; Pantelić, D.; Sarajlić, M.; Savić-Šević, S.; Matović, J.; Jelenković, B.; Vasiljević-Radović, D.; Ćurčić, S.; Vuković, S.; Pavlović, V.; et al. Butterfly scales as bionic templates for complex ordered nanophotonic materials: A pathway to biomimetic plasmonics. Opt. Mater. 2013, 35, 1869–1875. [Google Scholar] [CrossRef]
- Black, K.C.L.; Sileika, T.S.; Yi, J.; Zhang, R.; Rivera, J.G.; Messersmith, P.B. Bacterial Killing by Light-Triggered Release of Silver from Biomimetic Metal Nanorods. Small 2014, 10, 169–178. [Google Scholar] [CrossRef]
- Chen, J.-D.; Zhou, L.; Ou, Q.-D.; Li, Y.-Q.; Shen, S.; Lee, S.-T.; Tang, J.-X. Enhanced Light Harvesting in Organic Solar Cells Featuring a Biomimetic Active Layer and a Self-Cleaning Antireflective Coating. Adv. Energy Mater. 2014, 4, 1301777. [Google Scholar] [CrossRef]
- Leem, J.W.; Kim, S.; Park, C.; Kim, E.; Yu, J.S. Strong Photocurrent Enhancements in Plasmonic Organic Photovoltaics by Biomimetic Nanoarchitectures with Efficient Light Harvesting. ACS Appl. Mater. Interfaces 2015, 7, 6706–6715. [Google Scholar] [CrossRef] [PubMed]
- Yan, R.; Chen, M.; Zhou, H.; Liu, T.; Tang, X.; Zhang, K.; Zhu, H.; Ye, J.; Zhang, D.; Fan, T. Bio-inspired Plasmonic Nanoarchitectured Hybrid System Towards Enhanced Far Red-to-Near Infrared Solar Photocatalysis. Sci. Rep. 2016, 6, 20001. [Google Scholar] [CrossRef] [Green Version]
- Hu, Y.; Cheng, H.; Zhao, X.; Wu, J.; Muhammad, F.; Lin, S.; He, J.; Zhou, L.; Zhang, C.; Deng, Y.; et al. Surface-Enhanced Raman Scattering Active Gold Nanoparticles with Enzyme-Mimicking Activities for Measuring Glucose and Lactate in Living Tissues. ACS Nano 2017, 11, 5558–5566. [Google Scholar] [CrossRef] [PubMed]
- Aranaz, I.; Castro, C.; Heras, A.; Acosta, N. On the Ability of Low Molecular Weight Chitosan Enzymatically Depolymerized to Produce and Stabilize Silver Nanoparticles. Biomimetics 2018, 3, 21. [Google Scholar] [CrossRef] [Green Version]
- Nishino, T.; Tanigawa, H.; Sekiguchi, A. Antifouling Effect on Biomimetic Metamaterial Surfaces. J. Photopolym. Sci. Technol. 2018, 31, 129–132. [Google Scholar] [CrossRef] [Green Version]
- Jakšić, Z.; Jakšić, O. Biomimetic Nanomembranes: An Overview. Biomimetics 2020, 5, 24. [Google Scholar] [CrossRef] [PubMed]
- Saylan, Y.; Erdem, Ö.; Inci, F.; Denizli, A. Advances in Biomimetic Systems for Molecular Recognition and Biosensing. Biomimetics 2020, 5, 20. [Google Scholar] [CrossRef]
- Gorshkova, Y.; Barbinta-Patrascu, M.-E.; Bokuchava, G.; Badea, N.; Ungureanu, C.; Lazea-Stoyanova, A.; Răileanu, M.; Bacalum, M.; Turchenko, V.; Zhigunov, A.; et al. Biological Performances of Plasmonic Biohybrids Based on Phyto-Silver/Silver Chloride Nanoparticles. Nanomaterials 2021, 11, 1811. [Google Scholar] [CrossRef]
- Guglielmelli, A.; Rosa, P.; Contardi, M.; Prato, M.; Mangino, G.; Miglietta, S.; Petrozza, V.; Pani, R.; Calogero, A.; Athanassiou, A.; et al. Biomimetic keratin gold nanoparticle-mediated in vitro photothermal therapy on glioblastoma multiforme. Nanomedicine 2021, 16, 121–138. [Google Scholar] [CrossRef] [PubMed]
- Hao, Z.; Li, Y.; Liu, X.; Jiang, T.; He, Y.; Zhang, X.; Cong, C.; Wang, D.; Liu, Z.; Gao, D. Enhancing biocatalysis of a MXene-based biomimetic plasmonic assembly for targeted cancer treatments in NIR-II biowindow. Chem. Eng. J. 2021, 425, 130639. [Google Scholar] [CrossRef]
- Rakitov, R.A. Secretion of brochosomes during the ontogenesis of a leafhopper, Oncometopia orbona (F.) (Insecta, Homoptera, Cicadellidae). Tissue Cell 2000, 32, 28–39. [Google Scholar] [CrossRef]
- Rakitov, R.A.; Gorb, S.N. Brochosomes protect leafhoppers (Insecta, Hemiptera, Cicadellidae) from sticky exudates. J. Roy. Soc. Interf. 2013, 10, 20130445. [Google Scholar] [CrossRef]
- Rakitov, R.A. The covering formed by brochosomes on the cuticle of leafhoppers (Homoptera, Cicadellidae). Entomol. Rev. 1995, 74, 90–103. [Google Scholar]
- Rakitov, R.A. Powdering of egg nests with brochosomes and related sexual dimorphism in leafhoppers (Hemiptera: Cicadellidae). Zool. J. Linn. Soc. 2004, 140, 353–381. [Google Scholar] [CrossRef] [Green Version]
- Rakitov, R.; Gorb, S.N. Brochosomal coats turn leafhopper (Insecta, Hemiptera, Cicadellidae) integument to superhydrophobic state. Proc. R. Soc. B Biol. Sci. 2013, 280, 20122391. [Google Scholar] [CrossRef] [Green Version]
- Rakitov, R.; Moysa, A.A.; Kopylov, A.T.; Moshkovskii, S.A.; Peters, R.S.; Meusemann, K.; Misof, B.; Dietrich, C.H.; Johnson, K.P.; Podsiadlowski, L.; et al. Brochosomins and other novel proteins from brochosomes of leafhoppers (Insecta, Hemiptera, Cicadellidae). Insect Biochem. Mol. Biol. 2018, 94, 10–17. [Google Scholar] [CrossRef] [PubMed]
- Yang, S.; Sun, N.; Stogin, B.B.; Wang, J.; Huang, Y.; Wong, T.-S. Ultra-antireflective synthetic brochosomes. Nat. Comm. 2017, 8, 1285. [Google Scholar] [CrossRef]
- Sukamanchi, R.; Mathew, D.; Kumar, K.S. Durable Superhydrophobic Particles Mimicking Leafhopper Surface: Superoleophilicity and Very Low Surface Energy. ACS Sustain. Chem. Eng. 2017, 5, 252–260. [Google Scholar] [CrossRef]
- Borja, L. Synthesis replicates camouflaging-brochosome particle structure. MRS Bull. 2018, 43, 10. [Google Scholar] [CrossRef] [Green Version]
- Ding, Q.; Kang, Y.; Li, W.; Sun, G.; Liu, H.; Li, M.; Ye, Z.; Zhou, M.; Zhou, J.; Yang, S. Bioinspired Brochosomes as Broadband and Omnidirectional Surface-Enhanced Raman Scattering Substrates. J. Phys. Chem. Lett. 2019, 10, 6484–6491. [Google Scholar] [CrossRef] [PubMed]
- Lei, C.-W.; Chen, R.-Y.; Yang, H. Leafhopper Wing-Inspired Broadband Omnidirectional Antireflective Embroidered Ball-Like Structure Arrays Using a Nonlithography-Based Methodology. Langmuir 2020, 36, 5296–5302. [Google Scholar] [CrossRef]
- Shih, M.-S.; Chen, H.-Y.; Li, P.-C.; Yang, H. Broadband omnidirectional antireflection coatings inspired by embroidered ball-like structures on leafhoppers. Appl. Surf. Sci. 2020, 532, 147397. [Google Scholar] [CrossRef]
- Li, P.-C.; Chen, H.-Y.; Chiang, K.-T.; Yang, H. Reversible embroidered ball-like antireflective structure arrays inspired by leafhopper wings. J. Colloid Interface Sci. 2021, 599, 119–129. [Google Scholar] [CrossRef]
- Rakić, A.D.; Djurišić, A.B.; Elazar, J.M.; Majewski, M.L. Optical properties of metallic films for vertical-cavity optoelectronic devices. Appl. Opt. 1998, 37, 5271–5283. [Google Scholar] [CrossRef] [PubMed]
- Boltasseva, A.; Atwater, H.A. Low-Loss Plasmonic Metamaterials. Science 2011, 331, 290–291. [Google Scholar] [CrossRef] [PubMed]
- Smith, D.R.; Schultz, S.; Markoš, P.; Soukoulis, C.M. Determination of effective permittivity and permeability of metamaterials from reflection and transmission coefficients. Phys. Rev. B 2002, 65, 195104. [Google Scholar] [CrossRef] [Green Version]
- Ebbesen, T.W.; Lezec, H.J.; Ghaemi, H.F.; Thio, T.; Wolff, P.A. Extraordinary optical transmission through sub-wavelength hole arrays. Nature 1998, 391, 667–669. [Google Scholar] [CrossRef]
- Garcia-Vidal, F.J.; Martin-Moreno, L.; Ebbesen, T.W.; Kuipers, L. Light passing through subwavelength apertures. Rev. Mod. Phys. 2010, 82, 729–787. [Google Scholar] [CrossRef] [Green Version]
- Bonod, N.; Enoch, S.; Li, L.; Popov, E.; Nevière, M. Resonant optical transmission through thin metallic films with and without holes. Opt. Express 2003, 11, 482–490. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Oulton, R.F.; Sorger, V.J.; Zentgraf, T.; Ma, R.-M.; Gladden, C.; Dai, L.; Bartal, G.; Zhang, X. Plasmon lasers at deep subwavelength scale. Nature 2009, 461, 629–632. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jakšić, Z.; Smiljanić, M.M.; Vasiljević-Radović, D.; Obradov, M.; Radulović, K.; Tanasković, D.; Krstajić, P.M. Field localization control in aperture-based plasmonics by Boolean superposition of primitive forms at deep subwavelength scale. Opt. Quant. Electron. 2016, 48, 225. [Google Scholar] [CrossRef]
- Li, J.-F.; Zhang, Y.-J.; Ding, S.-Y.; Panneerselvam, R.; Tian, Z.-Q. Core–Shell Nanoparticle-Enhanced Raman Spectroscopy. Chem. Rev. 2017, 117, 5002–5069. [Google Scholar] [CrossRef] [PubMed]
- Aurenhammer, F. Voronoi diagrams—A survey of a fundamental geometric data structure. ACM Comput. Surv. 1991, 23, 345–405. [Google Scholar] [CrossRef]
- Horák, M.; Křápek, V.; Hrtoň, M.; Konečná, A.; Ligmajer, F.; Stöger-Pollach, M.; Šamořil, T.; Paták, A.; Édes, Z.; Metelka, O.; et al. Limits of Babinet’s principle for solid and hollow plasmonic antennas. Sci. Rep. 2019, 9, 4004. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Anderson, O.R. Radiolaria; Springer: New York, NY, USA, 1983. [Google Scholar] [CrossRef]
- Sanchez, C.; Arribart, H.; Giraud Guille, M.M. Biomimetism and bioinspiration as tools for the design of innovative materials and systems. Nat. Mater. 2005, 4, 277–288. [Google Scholar] [CrossRef]
- Walter, E.C.; Zach, M.P.; Favier, F.; Murray, B.J.; Inazu, K.; Hemminger, J.C.; Penner, R.M. Metal Nanowire Arrays by Electrodeposition. ChemPhysChem 2003, 4, 131–138. [Google Scholar] [CrossRef]
- Maier, S.A.; Atwater, H.A. Plasmonics: Localization and guiding of electromagnetic energy in metal/dielectric structures. J. Appl. Phys. 2005, 98, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Burda, C.; Chen, X.; Narayanan, R.; El-Sayed, M.A. Chemistry and Properties of Nanocrystals of Different Shapes. Chem. Rev. 2005, 105, 1025–1102. [Google Scholar] [CrossRef] [PubMed]
- Turing, A.M. The chemical basis of morphogenesis. Bull. Math. Biol. 1990, 52, 153–197. [Google Scholar] [CrossRef]
- Segalman, R.A. Patterning with block copolymer thin films. Mater. Sci. Eng. R Rep. 2005, 48, 191–226. [Google Scholar] [CrossRef] [Green Version]
- Albert, J.N.L.; Epps, T.H. Self-assembly of block copolymer thin films. Mater. Today 2010, 13, 24–33. [Google Scholar] [CrossRef]
- Jakšić, Z.; Obradov, M.; Jakšić, O.; Tanasković, D. An extended family of perforated submicrometer hollow or core-shell plasmonic particles as antireflective synthetic brochosomes. Prepr. Res. Sq. 2021, 1–14. [Google Scholar] [CrossRef]
- Feng, T.; Liu, F.; Tam, W.Y.; Li, J. Effective parameters retrieval for complex metamaterials with low symmetries. EPL 2013, 102, 18003. [Google Scholar] [CrossRef]
- Lalanne, P.; Lemercier-Lalanne, D. On the effective medium theory of subwavelength periodic structures. J. Mod. Opt. 1996, 43, 2063–2085. [Google Scholar] [CrossRef]
- Chebykin, A.V.; Orlov, A.A.; Vozianova, A.V.; Maslovski, S.I.; Kivshar, Y.S.; Belov, P.A. Nonlocal effective medium model for multilayered metal-dielectric metamaterials. Phys. Rev. B 2011, 84, 115438. [Google Scholar] [CrossRef] [Green Version]
- Brown, J. Artificial dielectrics. In Progress in Dielectrics; Birks, J.B., Ed.; Wiley: Hoboken, NJ, USA, 1960; Volume 2, pp. 193–225. [Google Scholar] [CrossRef] [Green Version]
- Mendis, R.; Nagai, M.; Wang, Y.; Karl, N.; Mittleman, D.M. Terahertz Artificial Dielectric Lens. Sci. Rep. 2016, 6, 23023. [Google Scholar] [CrossRef]
- Hua, C.; Cheng, Z.; Ma, Y.; He, H.; Xu, G.; Liu, Y.; Yang, S.; Han, G. Enhanced Electrochromic Tungsten Oxide by Bio-Inspired Brochosomes. J. Electrochem. Soc. 2021, 168, 042503. [Google Scholar] [CrossRef]
- Pan, Q.; Zhang, H.; Yang, Y.; Cheng, C. 3D Brochosomes-Like TiO2/WO3/BiVO4 Arrays as Photoanode for Photoelectrochemical Hydrogen Production. Small 2019, 15, 1900924. [Google Scholar] [CrossRef] [PubMed]

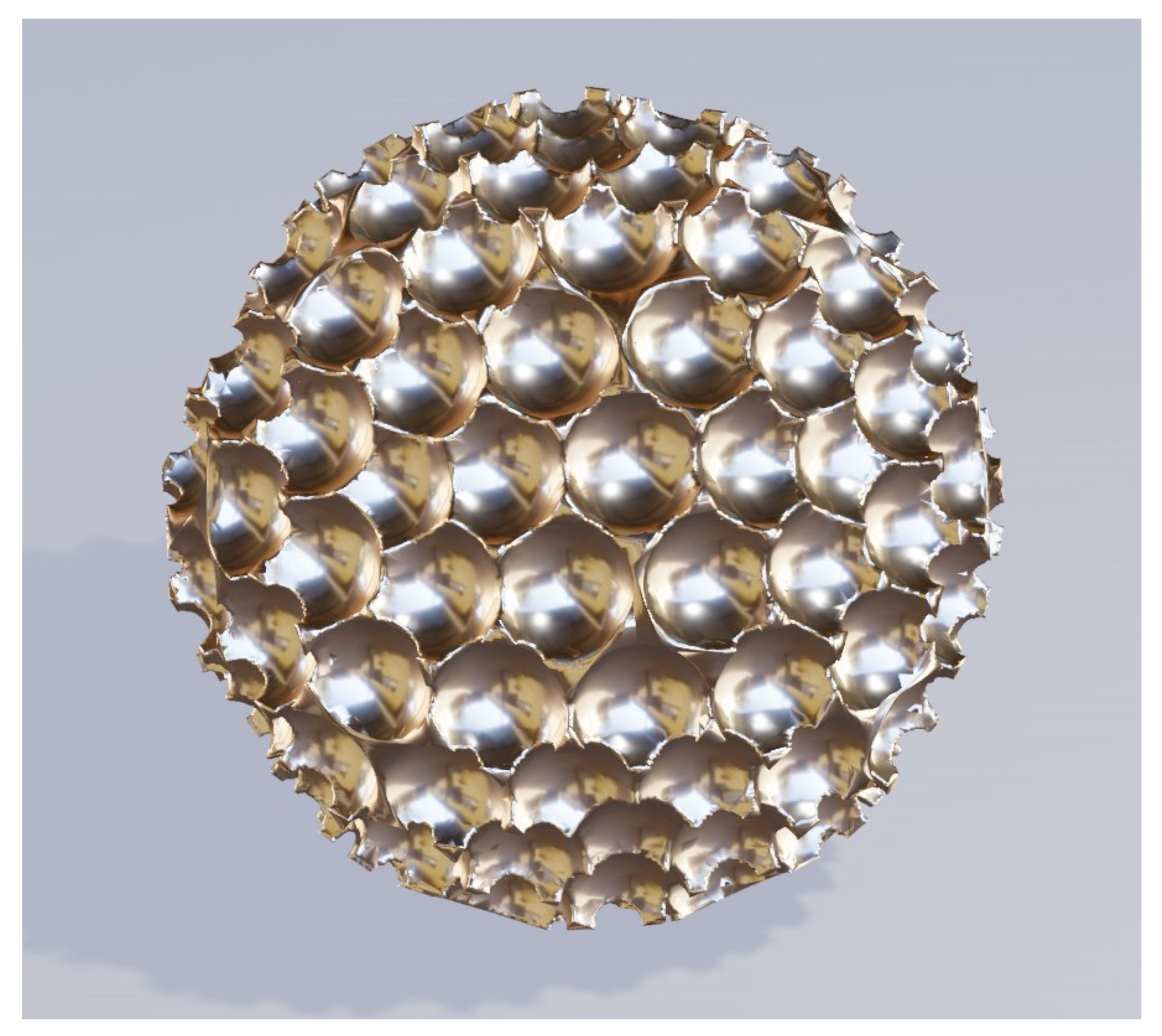


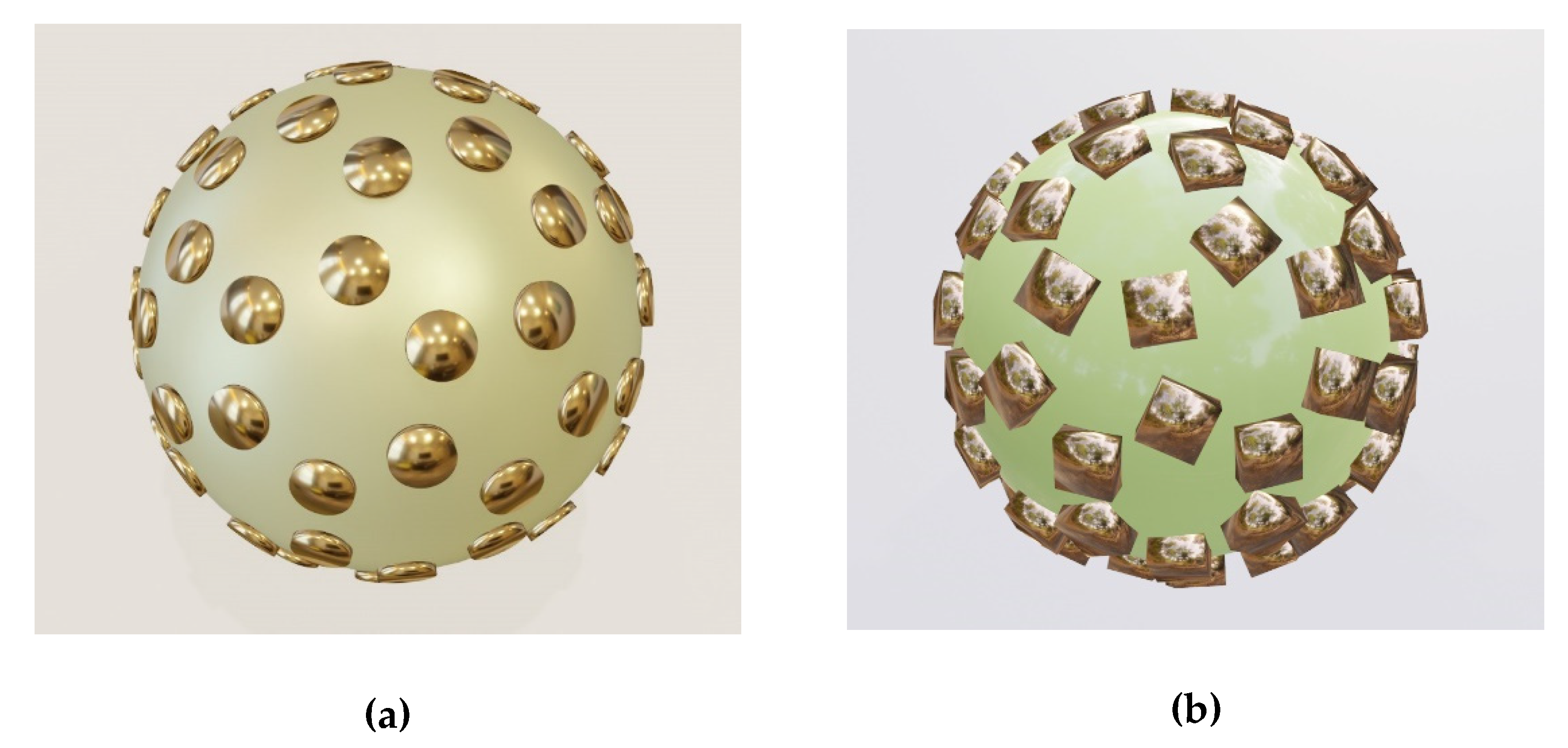
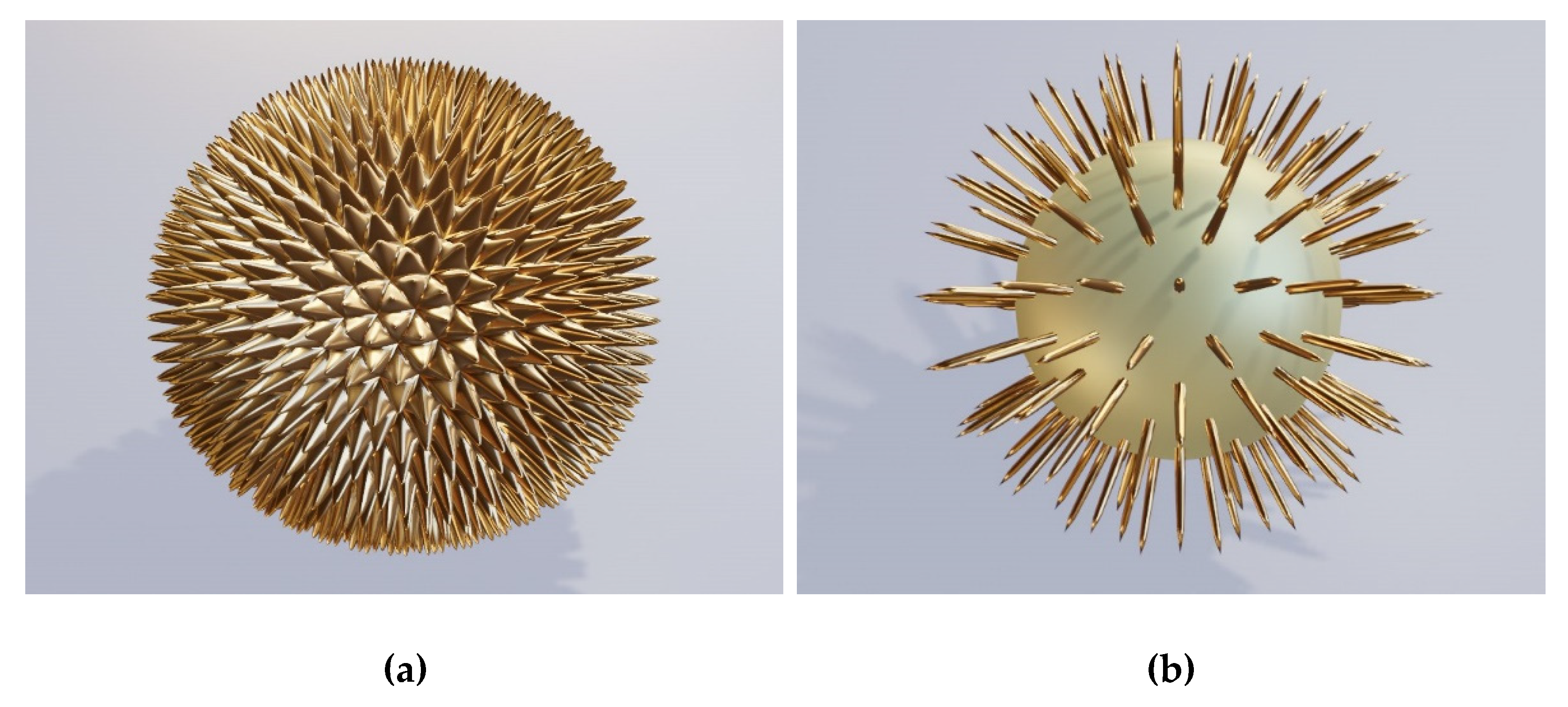
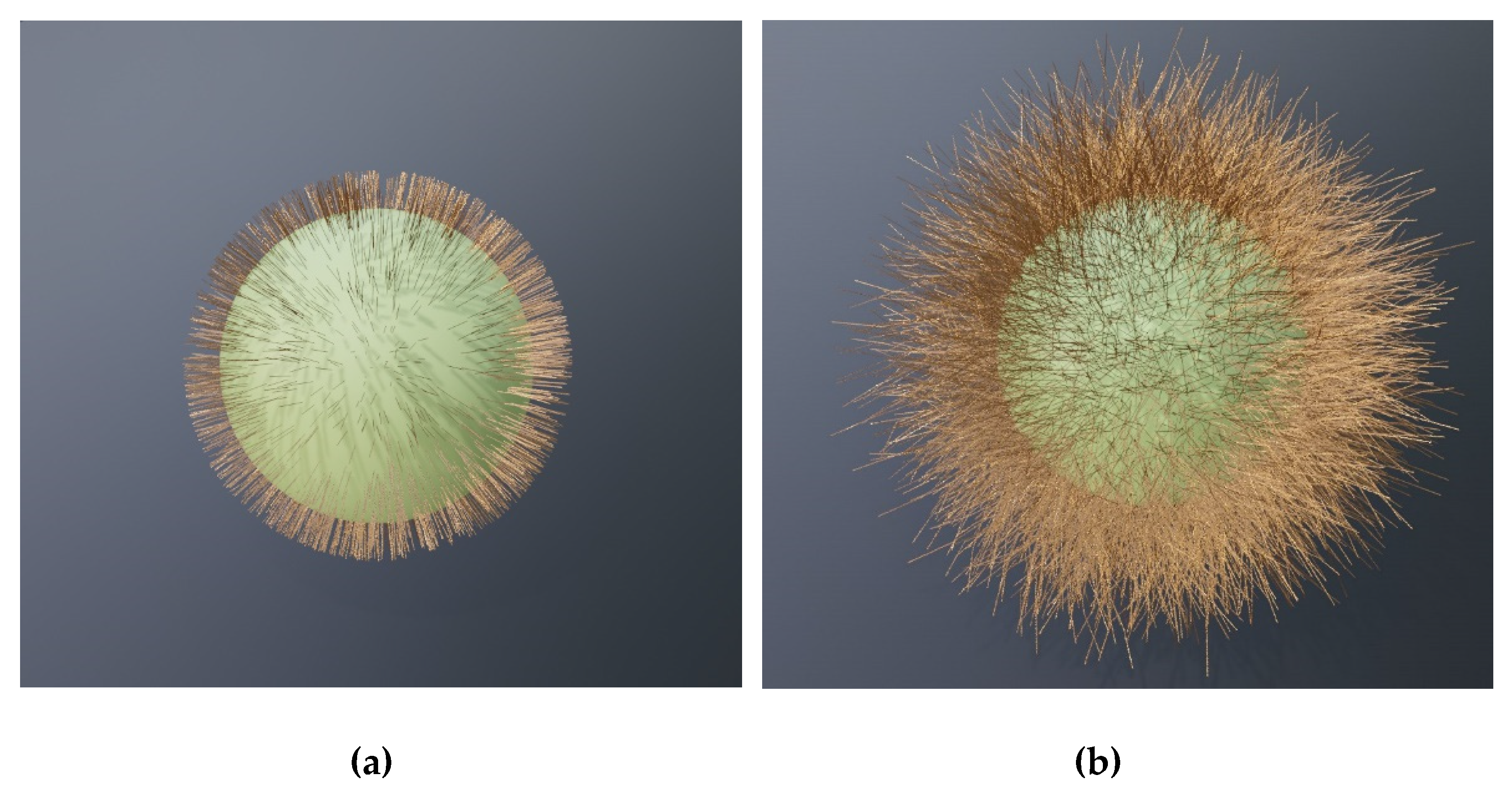
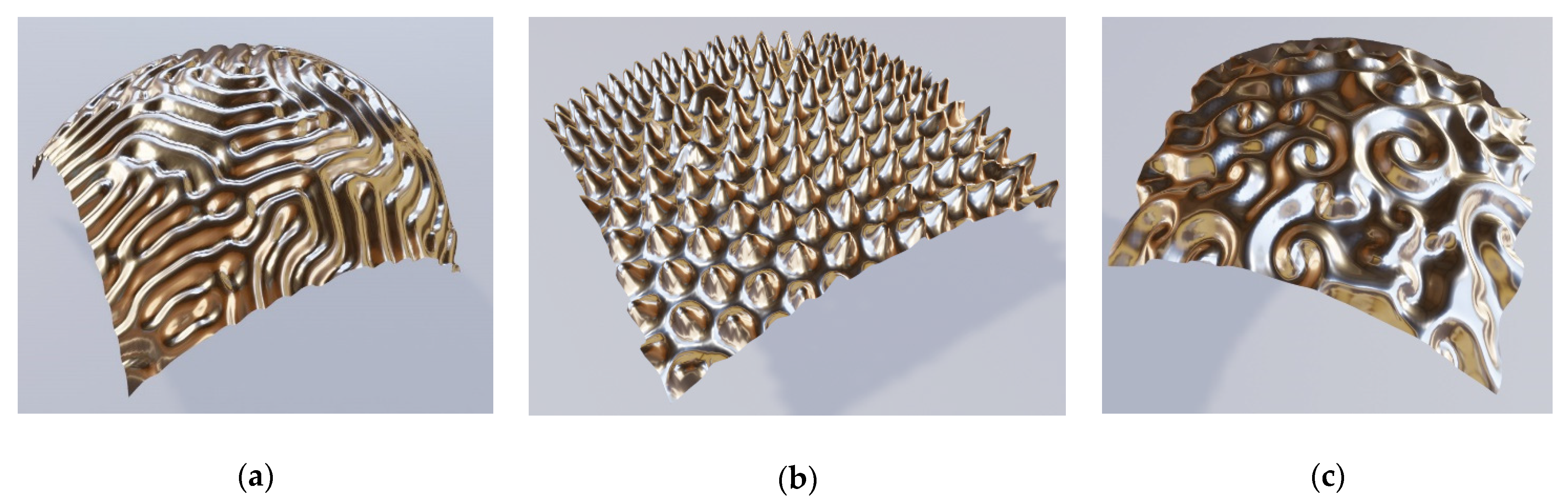



| Surface Features | Variations of Surface Features | Comment |
|---|---|---|
| Spatial layout of apertures and protrusions |
| Distribution of surface features (holes, cylinders, nanowires, etc.) |
| Apertures: shapes |
| Subtractive processing of shell surface (perforation) |
| Apertures: sizes |
| Subtractive processing of shell surface (perforation) |
| Protrusions: shapes |
| Additive processing of shell surface (Babinet inverse of the surface holes) |
| Protrusions: sizes |
| Additive processing of shell surface (Babinet inverse of the surface holes) |
| Surface reliefs |
| Additive or subtractive processing of shell surface in order to obtain variations of the heights of its various points (different distances from the center of the sphere) |
| Combined forms |
| Superposition of subtractive and/or additive forms |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jakšić, Z.; Obradov, M.; Jakšić, O. Brochosome-Inspired Metal-Containing Particles as Biomimetic Building Blocks for Nanoplasmonics: Conceptual Generalizations. Biomimetics 2021, 6, 69. https://doi.org/10.3390/biomimetics6040069
Jakšić Z, Obradov M, Jakšić O. Brochosome-Inspired Metal-Containing Particles as Biomimetic Building Blocks for Nanoplasmonics: Conceptual Generalizations. Biomimetics. 2021; 6(4):69. https://doi.org/10.3390/biomimetics6040069
Chicago/Turabian StyleJakšić, Zoran, Marko Obradov, and Olga Jakšić. 2021. "Brochosome-Inspired Metal-Containing Particles as Biomimetic Building Blocks for Nanoplasmonics: Conceptual Generalizations" Biomimetics 6, no. 4: 69. https://doi.org/10.3390/biomimetics6040069
APA StyleJakšić, Z., Obradov, M., & Jakšić, O. (2021). Brochosome-Inspired Metal-Containing Particles as Biomimetic Building Blocks for Nanoplasmonics: Conceptual Generalizations. Biomimetics, 6(4), 69. https://doi.org/10.3390/biomimetics6040069









