Research on Six-Wheel Distributed Unmanned Vehicle Path Tracking Strategy Based on Hierarchical Control
Abstract
:1. Introduction
- (1)
- Most researchers have only focused on the improvement of accuracy performance during UGV path tracking control, but ignored the problem of stability during vehicle driving.
- (2)
- The application of the MPC algorithm for path tracking control causes the problems of long adjustment time and weak anti-interference capability because its calculation is complex and time-consuming, and these problems have not been better solved.
- (3)
- The state quantities considered by most researchers based on the MPC algorithm are mainly the position and heading angle of the vehicle, which fail to consider and constrain the fluctuation of slip rate and excessive transverse moment caused by the complex road surface, resulting in the instability phenomenon of UGV.
- (4)
- At present, most scholars mainly study the traditional front-wheel steering or four-wheel independent drive unmanned vehicle—addressing the accuracy and stability issues during path tracking of distributed unmanned ground vehicles (DUGV) with six-wheel independent steering and four-wheel independent steering is not common.
2. Mathematical Model
2.1. Upper Layer Kinematic Model
2.2. Lower Kinematic Model
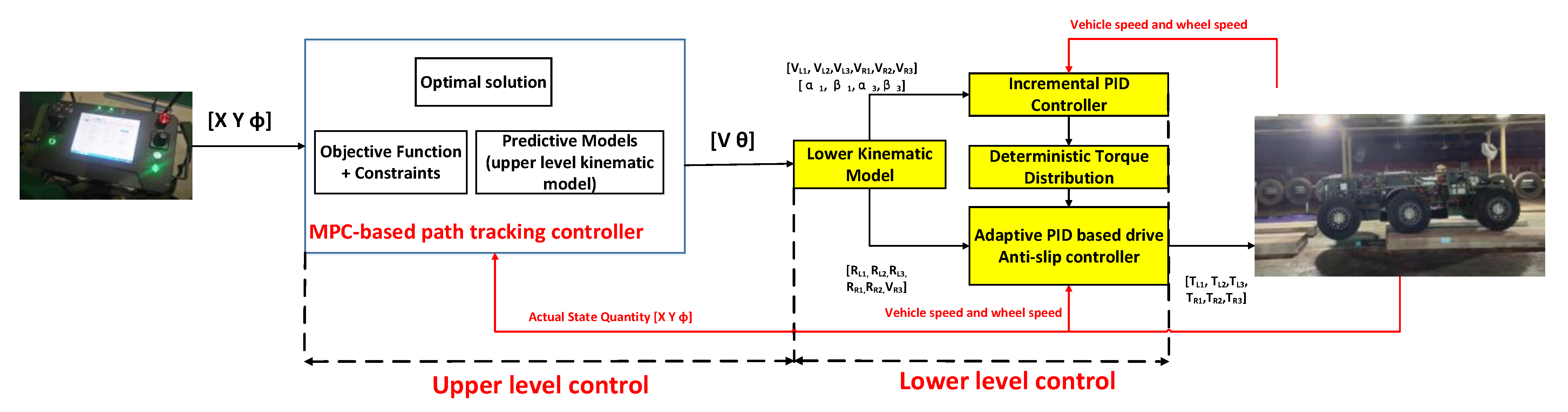
3. Control Design
3.1. Coordinated Control Strategies for Upper Level Design
3.1.1. Six-Wheeled Distributed Unmanned Vehicle Prediction Equation
3.1.2. QP Optimization
3.2. Coordinated Control Strategies for Lower Level Design
3.2.1. Distribution Strategy Based on Deterministic Moments
3.2.2. Adaptive PID-Based Drive Anti-Slip Control
4. Simulation Results
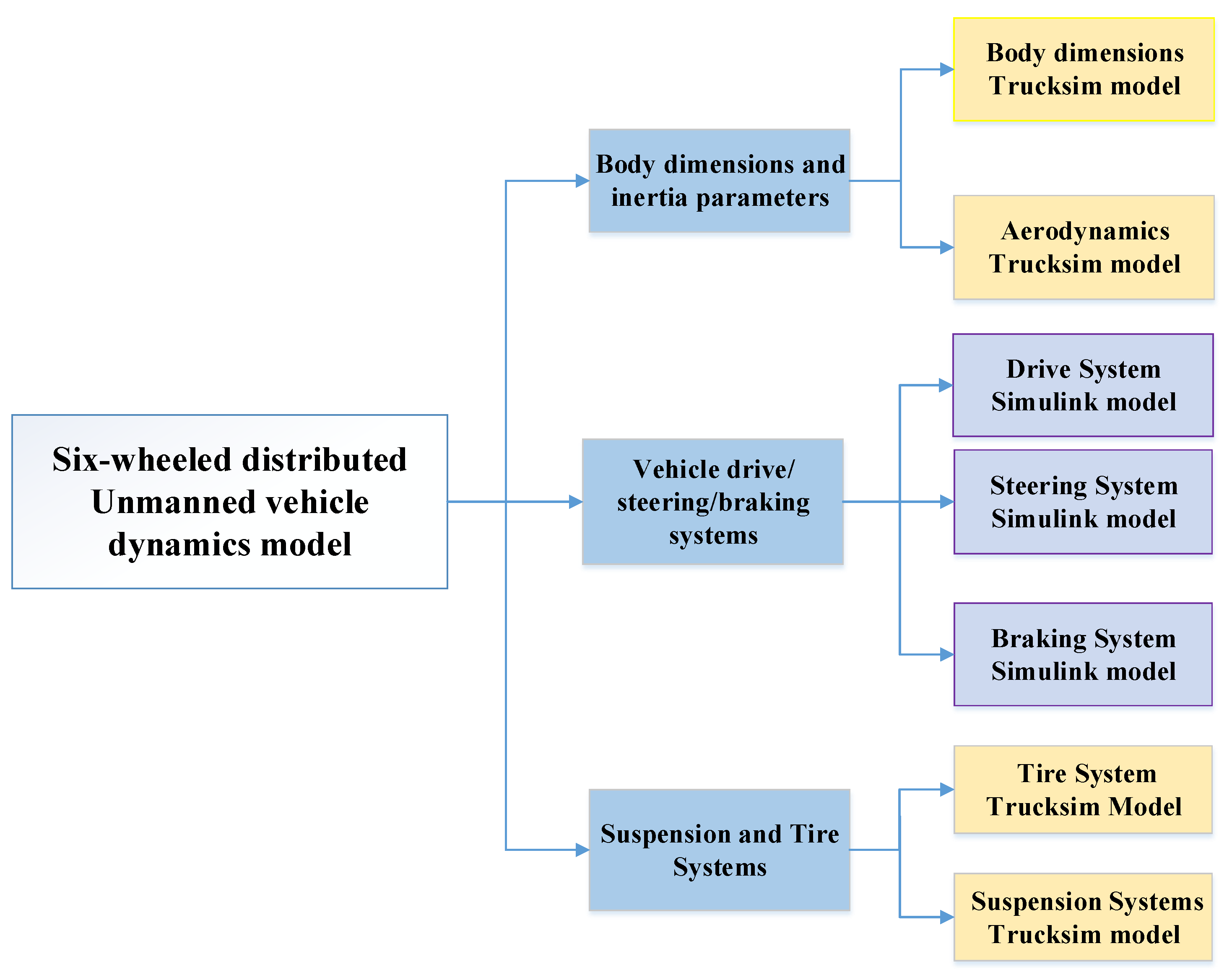
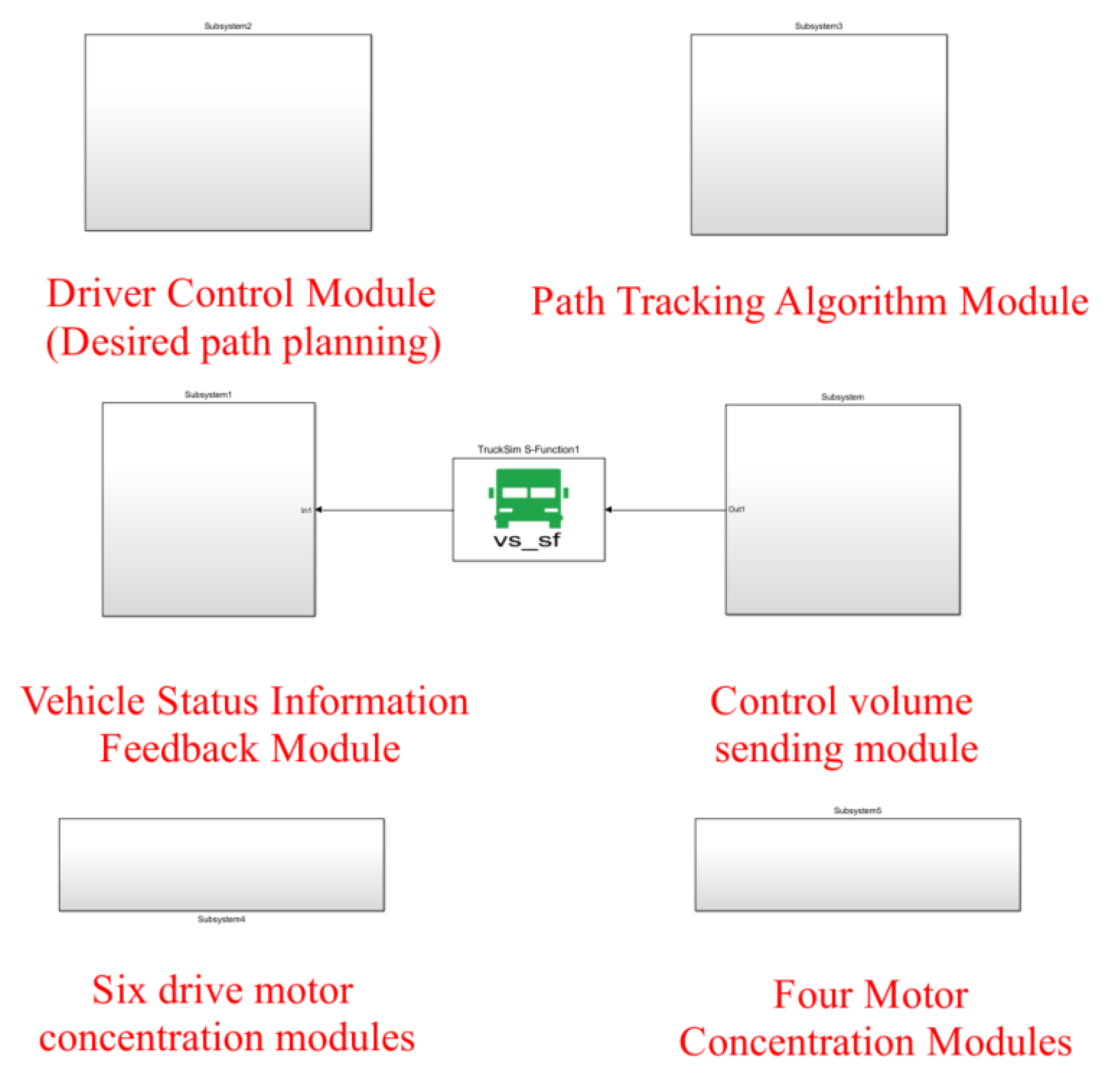
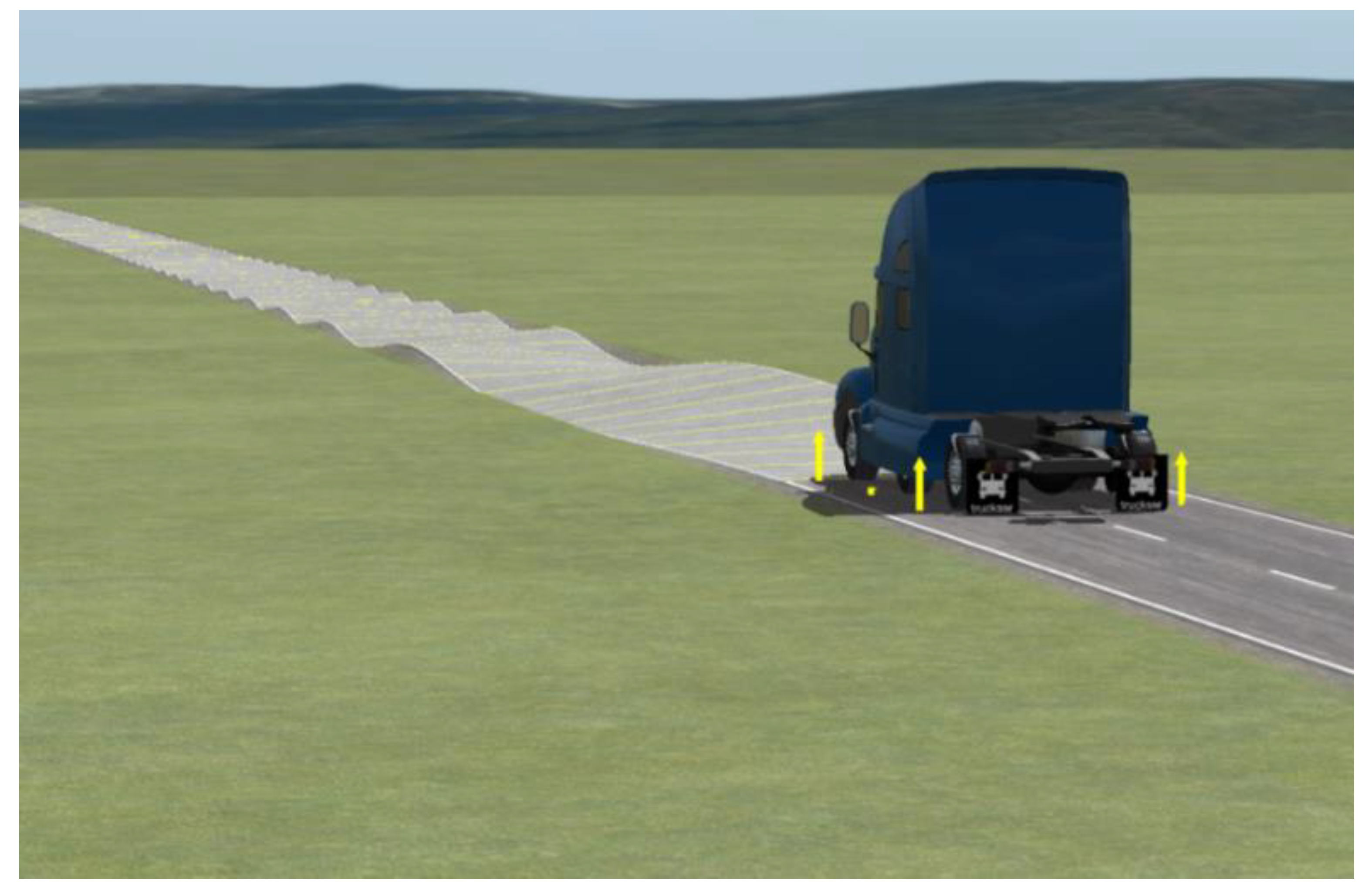
4.1. Distributed Unmanned Vehicle Simulation Platform Based on Trucksim/Simulink
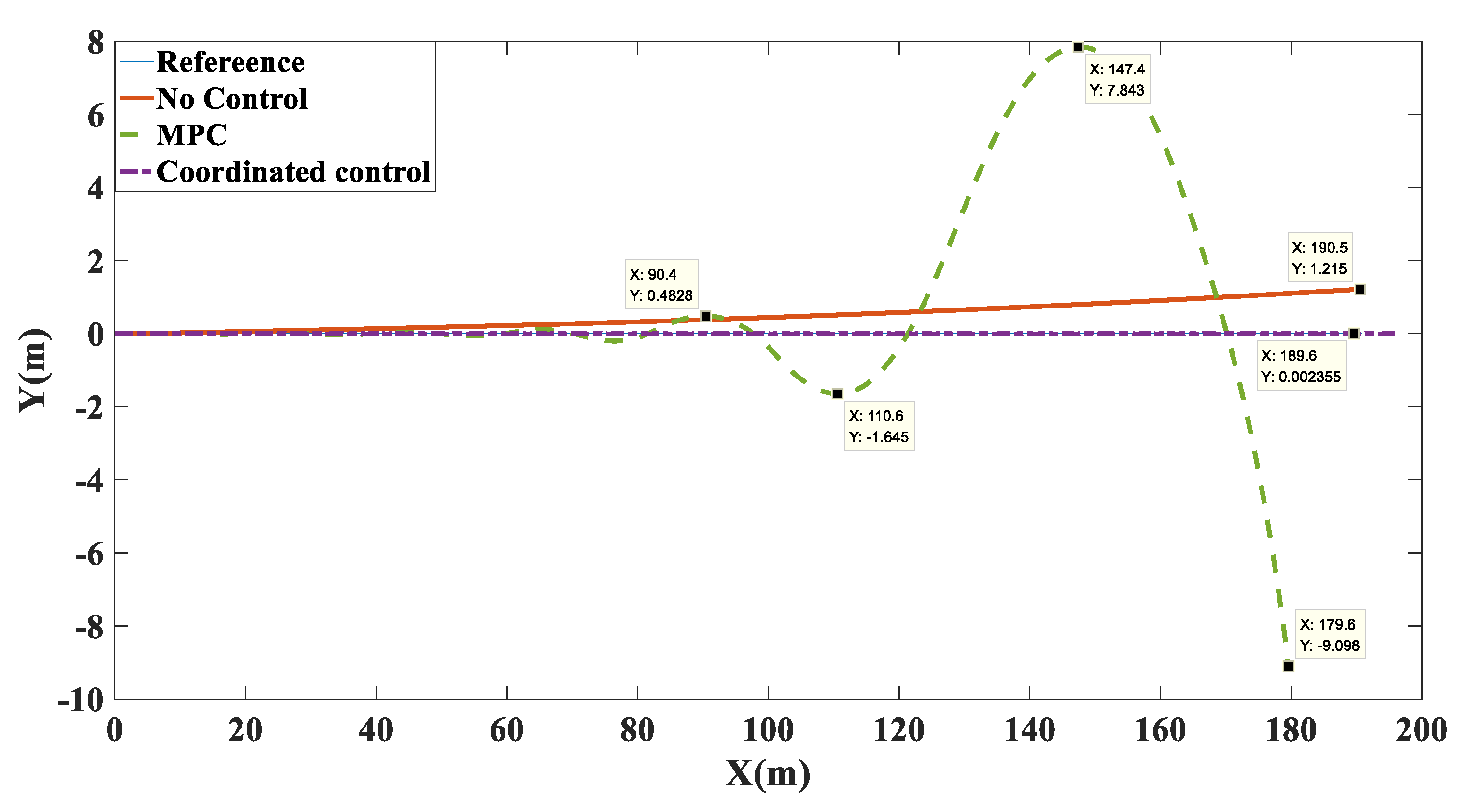
4.2. Split Mu Straight Path Following Experiment
4.3. Sine Sweep Straight Path Following Experiment
5. Conclusions
- (1)
- Based on the physical structure of distributed unmanned vehicles with six-wheel independent drive and four-wheel independent steering, we designed a hierarchical kinematic model of unmanned vehicle path tracking based on the six-wheel Ackermann theory.
- (2)
- Based on HC theory, a coordinated control strategy for path tracking and stability of distributed unmanned vehicles was designed. The strategy is divided into two levels of control. In the upper level of control, the upper kinematic model is used as the prediction model of MPC, and the solution problem of future control increments is converted into the optimal solution problem of quadratic programming by setting the optimal objective function and constraints. The lower level of control is to map the optimal control quantities obtained from the upper level to the six-wheel speed control quantities and the four-wheel turning angle control quantities through the lower-level kinematics, and design the six-wheel torque distribution rules based on deterministic torque and stability-based slip rate control for executing the control demand calculated by the upper-level controller to prevent the unmanned vehicle from producing sideslip and to precisely generate the demand transverse moment to ensure the stability of the unmanned vehicle driving.
- (3)
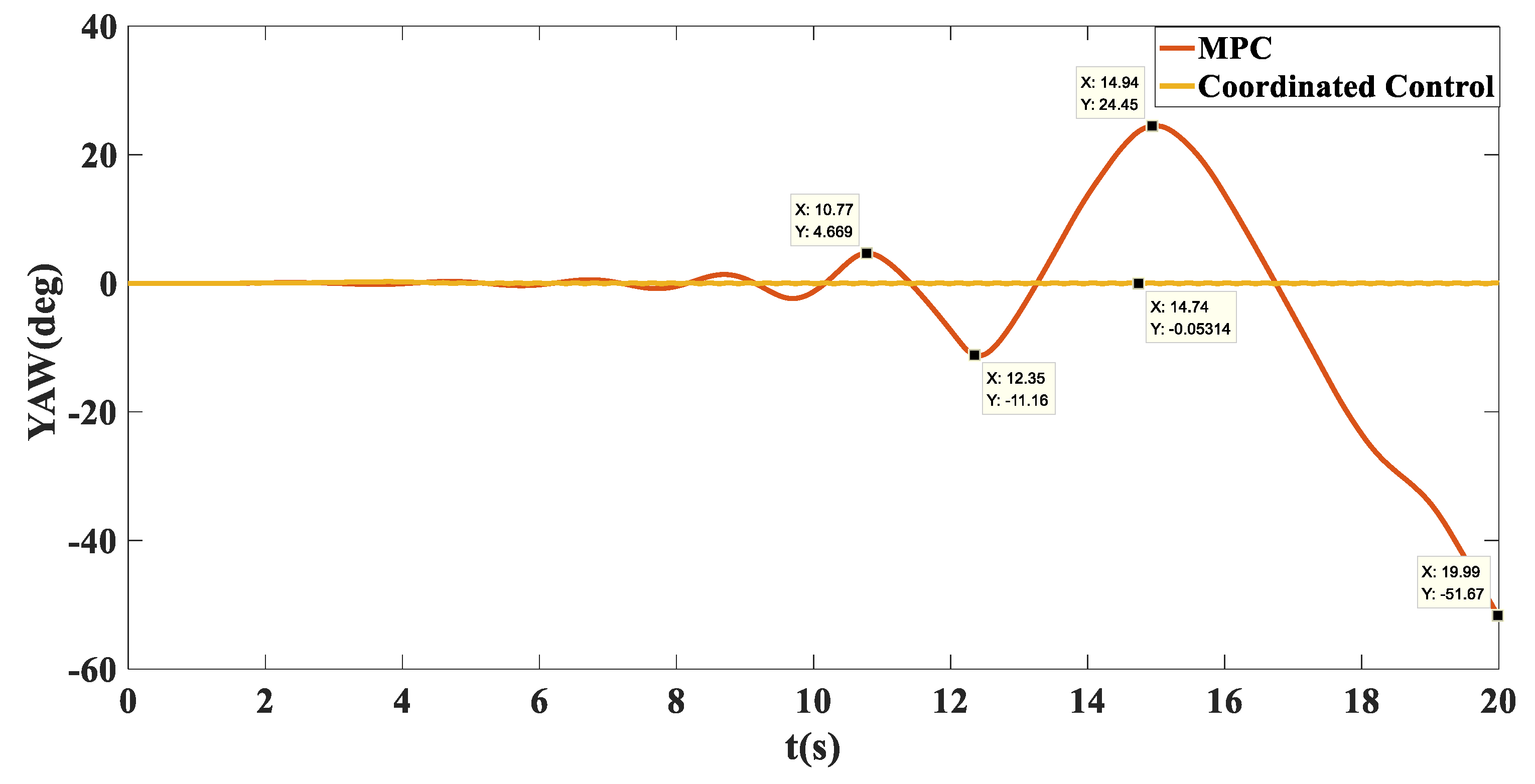
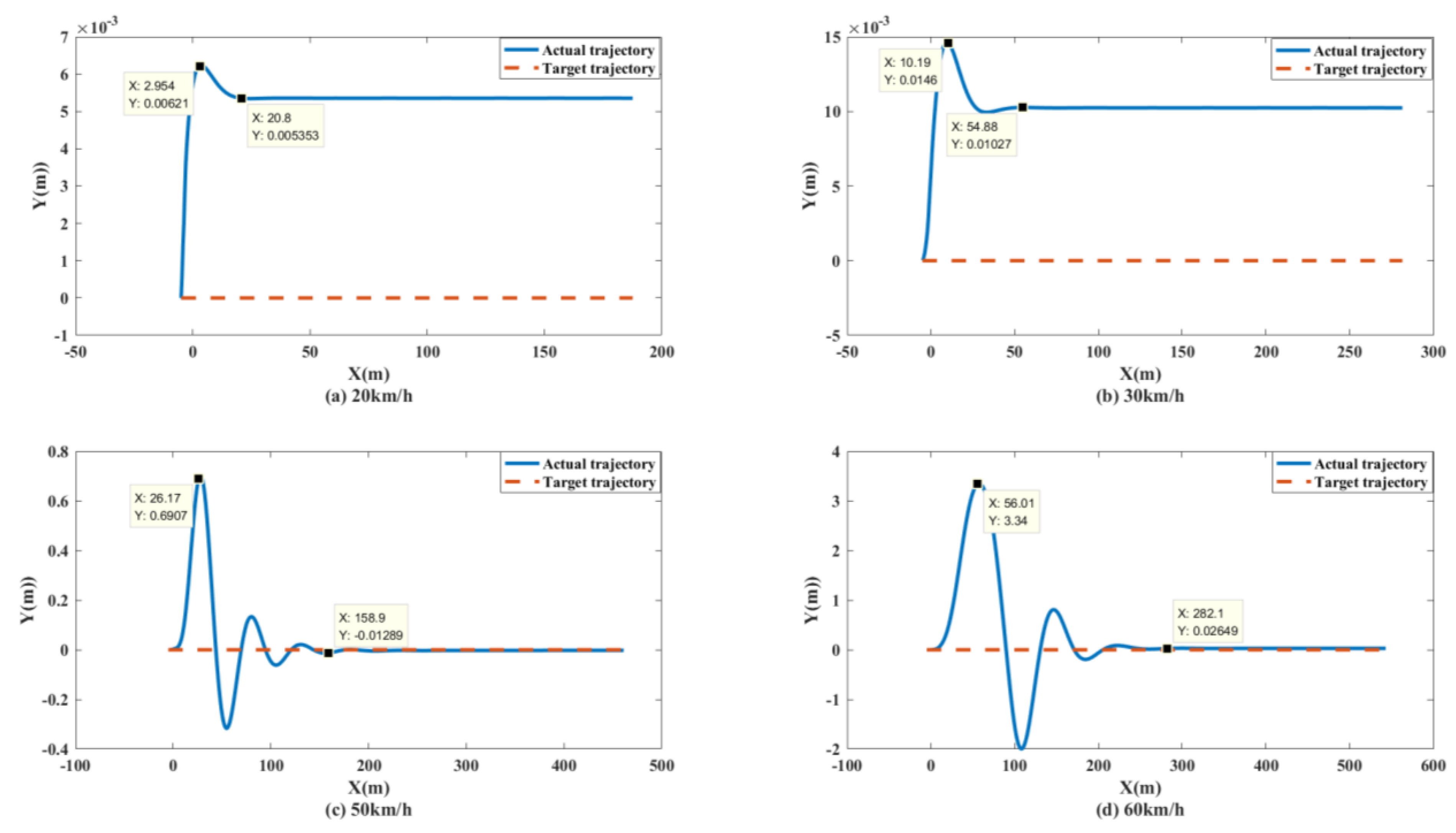
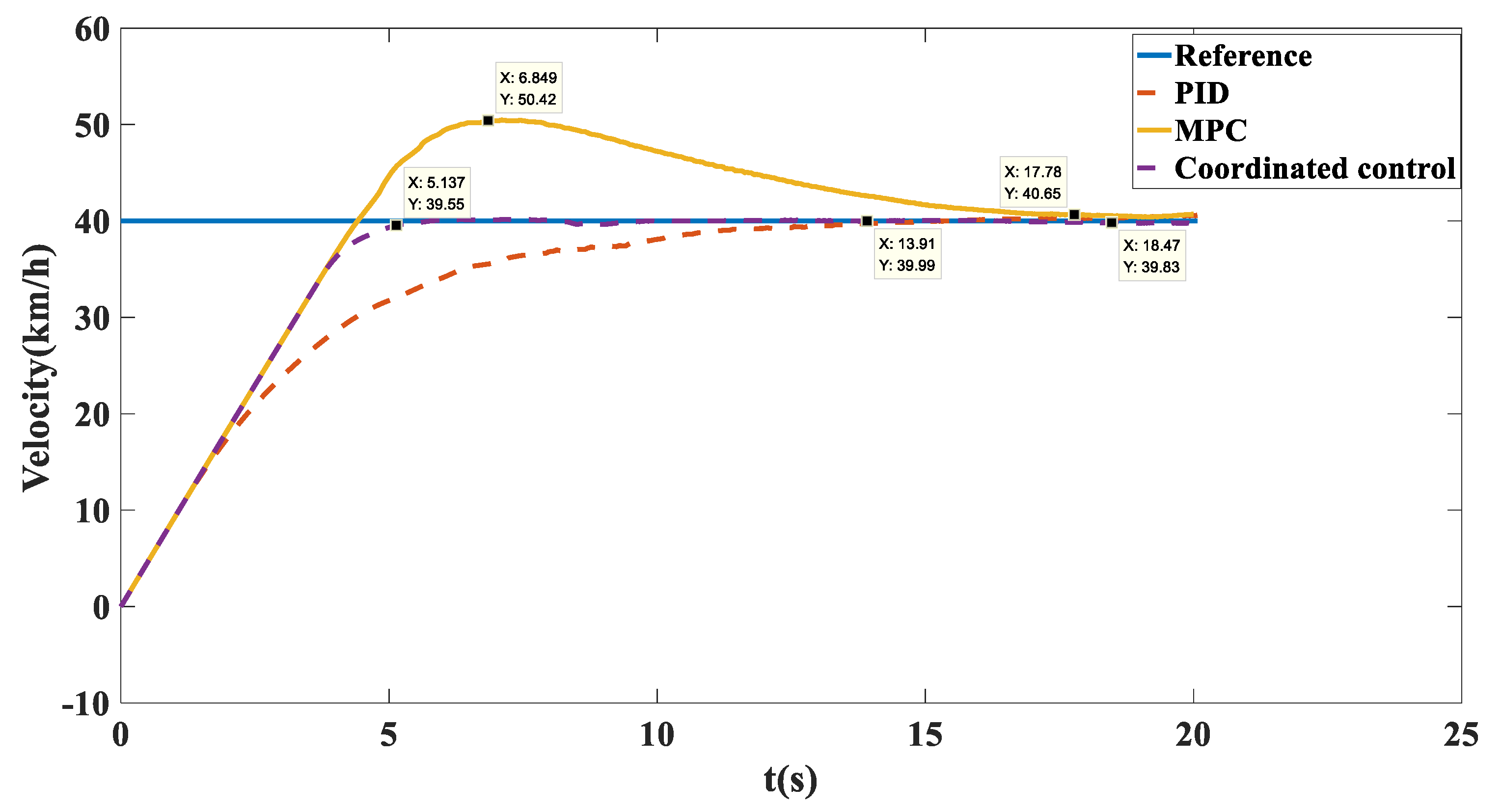
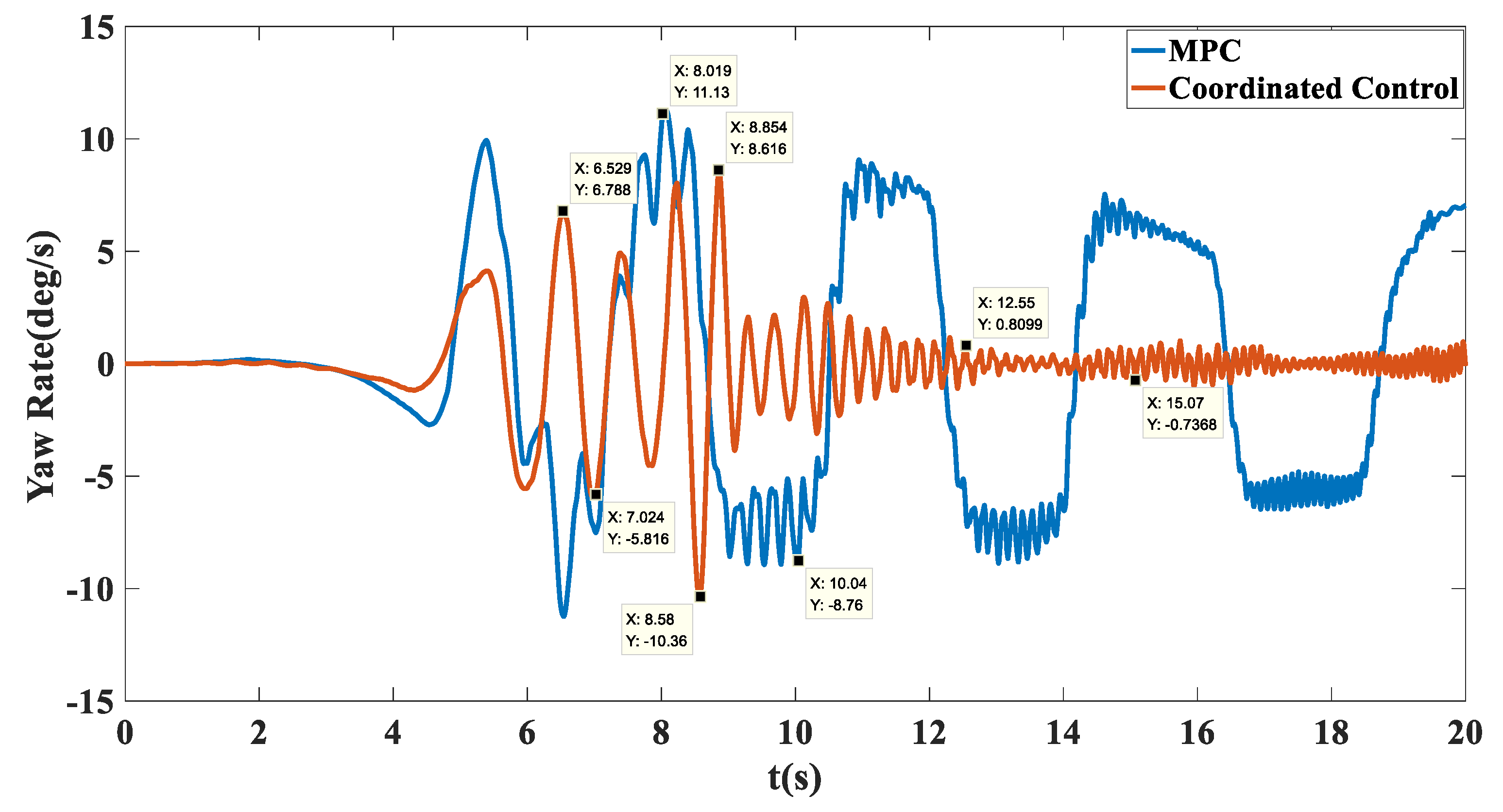
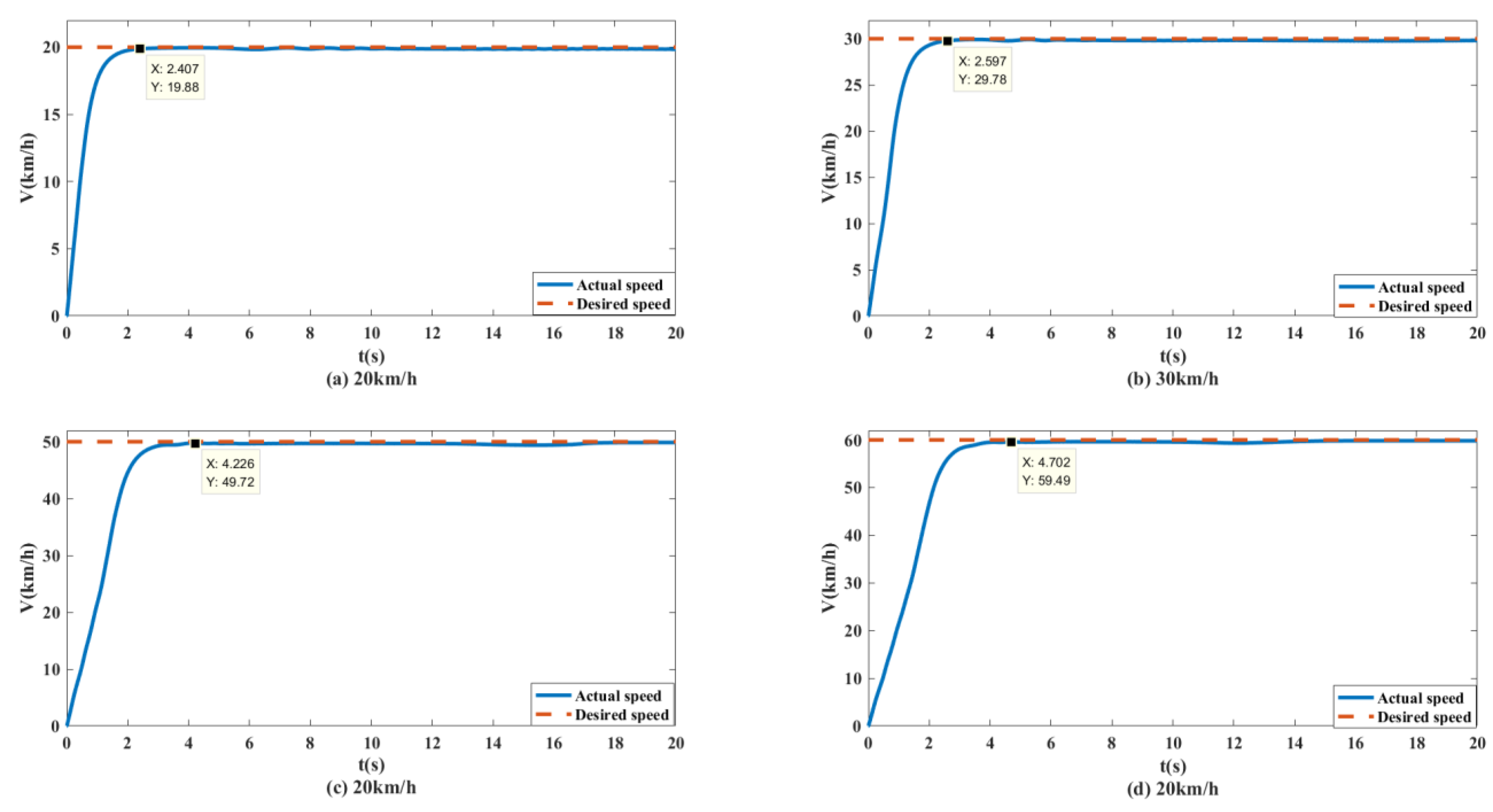
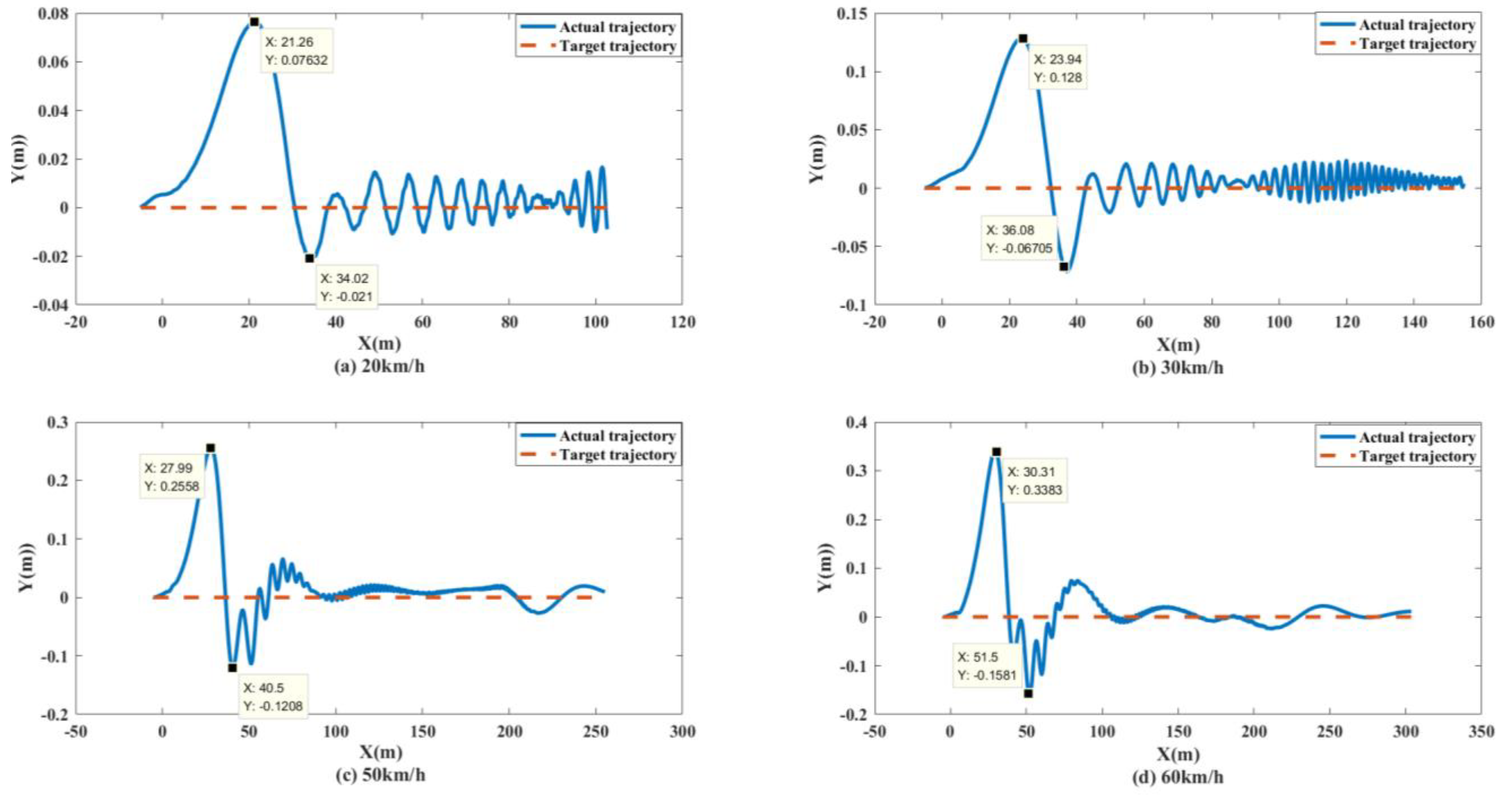
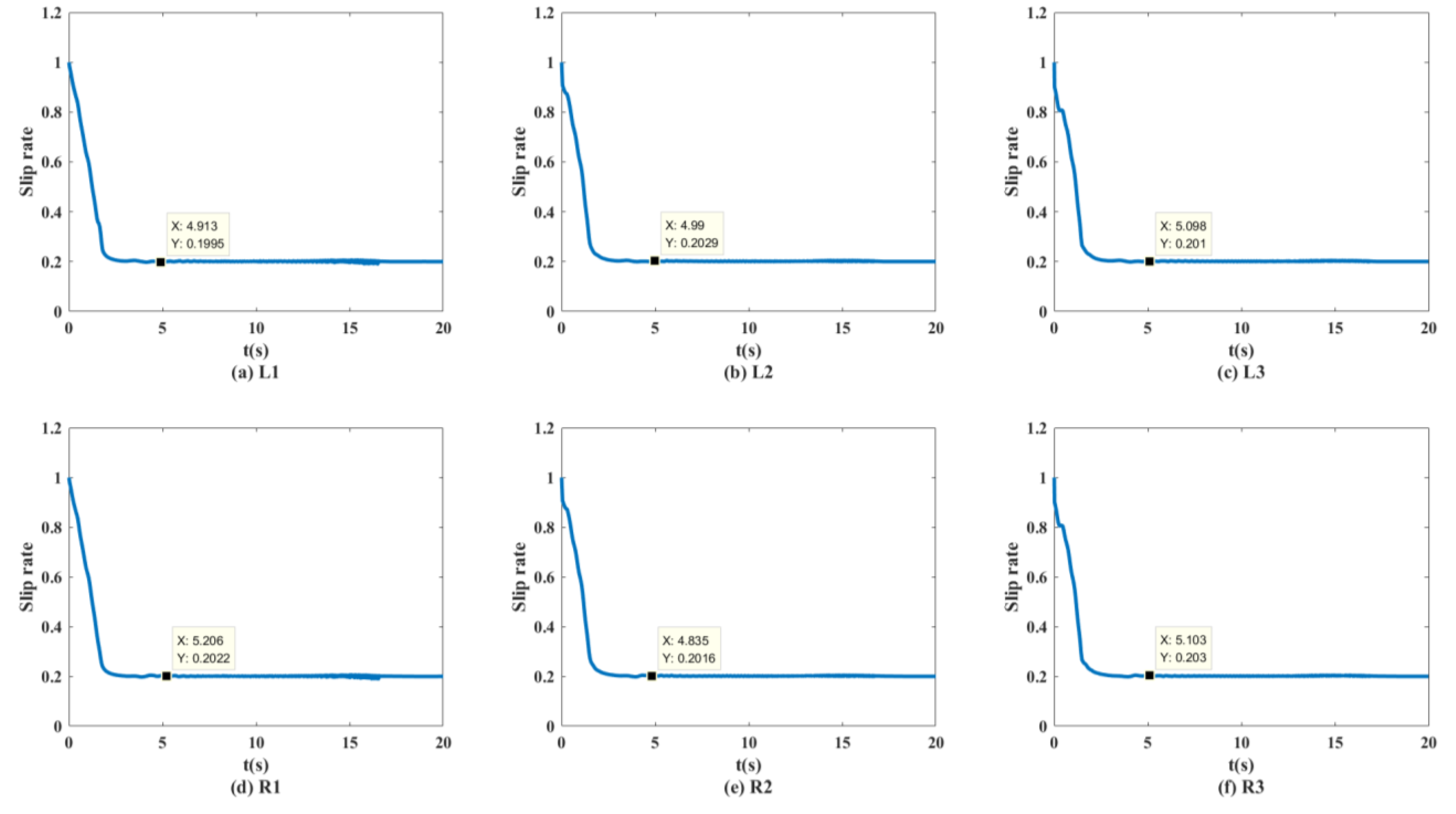
- An unmanned vehicle simulation platform based on Trucksim/Simulink with six-wheel independent drive and four-wheel independent steering was established, and the path tracking tests of 20 km/h, 30 km/h, 40 km/h, 50 km/h and 60 km/h were carried out on this platform on the Split mu straight road and the Sine sweep straight road. The simulation results show that the coordinated control has better response characteristics than MPC, which can output the deterministic moment and control the wheel slip rate at 20%, improving the accuracy and stability of the unmanned vehicle path tracking. Therefore, the coordinated control strategy can achieve stable and accurate path tracking under various working conditions.
- (4)
- In the coordinated control algorithm designed in this paper, there is a coupling relationship between speed tracking and path tracking. In future research, the joint control of the speed and path of the unmanned vehicle will be studied. In addition, the parameters of the vehicle model and control system in this paper are taken as fixed parameters. When the vehicle is under different working conditions, these state parameters have errors with the actual values. The future research direction is to further apply the adaptive control technology to the research of this paper, so that it can have the ability of online update and dynamic change.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, W.; Xu, H.; Xu, X. Enhancing the passing ability of unmanned vehicles using a variable wheelbase driving system. IEEE Access 2019, 7, 115871–115885. [Google Scholar] [CrossRef]
- Wang, W.; Xu, X.; Xu, H. Enhancing lateral dynamic performance of all-terrain vehicles using variable wheelbase chassis. Adv. Mech. Eng. 2020, 12, 1–19. [Google Scholar] [CrossRef]
- Zhu, J.; Wang, Z.; Zhang, L.; Dorrell, D. Braking/steering coordination control for in-wheel motor drive electric vehicles based on nonlinear model predictive control. Mech. Mach. Theory 2019, 142, 103586–103603. [Google Scholar] [CrossRef]
- Chen, L.; Zhang, J.; Li, Y.; Yuan, Y. Directional-stability-aware brake blending control synthesis for over-actuated electric vehicles during straight-line deceleration, Mechatronics 2016, 38, 121–131. Mechatronics 2016, 38, 121–131. [Google Scholar] [CrossRef]
- Zhou, F.; Qin, Y.; You, Y.; ZOU, T.; Li, G.; Zhang, Z.; Chang, Y. Distributed electric car path following layered coordination control method research. Mech. Sci. Technol. 2022, 22, 1–10. [Google Scholar]
- Liu, M.; You, Y.; Zhou, F. Simulation research on Path Tracking Control of Fully Electric Drive Distributed Unmanned Vehicle. Intell. Comput. Appl. 2022, 12, 61–79. [Google Scholar]
- Hossain, T.; Habibullah, H.; Islam, R. Steering and Speed Control System Design for Autonomous Vehicles by Developing an Optimal Hybrid Controller to Track Reference Trajectory. Machines 2022, 10, 420. [Google Scholar] [CrossRef]
- Elsisi, M.; Mahmoud, K.; Lehtonen, M.; Darwish, M. An improved neural network algorithm to efficiently track various trajectories of robot manipulator arms. IEEE Access 2021, 9, 11911–11920. [Google Scholar] [CrossRef]
- Zhang, H.; Yang, X.; Liang, J.; Xu, X.; Sun, X. GPS Path Tracking Control of Military Unmanned Vehicle Based on Preview Variable Universe Fuzzy Sliding Mode Control. Machines 2021, 9, 304. [Google Scholar] [CrossRef]
- Hou, Z.G.; Zou, A.M.; Long, C.; Min, T. Adaptive control of an electrically driven nonholonomic mobile robot via backstepping and fuzzy approach. IEEE Trans. Control Syst. Technol. 2009, 17, 803–815. [Google Scholar] [CrossRef]
- You, Y.; Yang, Z.; Zou, T.; Sui, Y.; Xu, C.; Zhang, C.; Xu, H.; Zhang, Z.; Han, J. A New Trajectory Tracking Control Method for Fully Electrically Driven Quadruped Robot. Machines 2022, 10, 292. [Google Scholar] [CrossRef]
- Huang, S.; Lin, Y. Simulation-based performance evaluation of model predictive control for building energy systems. Appl. Energy 2021, 281, 116027. [Google Scholar] [CrossRef]
- Guo, H.; Cao, D.; Chen, H.; Sun, Z.; Hu, Y. Model predictive path following control for autonomous cars considering a measurable disturbance: Implementation, testing, and verification. Mech. Syst. Signal Process. 2019, 118, 41–60. [Google Scholar] [CrossRef]
- Jiang, Y.; Gong, J.; Xiong, G. Research on Longitudinal and Horizontal Cooperative Planning Algorithm for Unmanned Vehicle Based on Differential Motion Constraint. Acta Autom. Sin. 2013, 39, 2012–2020. [Google Scholar] [CrossRef]
- Zhao, X.; Chen, H. Research on Lateral Control Method for Intelligent Vehicle Path Tracking. Automot. Eng. 2011, 33, 382–387. [Google Scholar]
- Zhang, C.; Gao, G.; Zhao, C.; Li, L.; Li, C.; Chen, X. Research on 4WS Agricultural Machine Path Tracking Algorithm Based on Fuzzy Control Pure Tracking Model. Machines 2022, 10, 597. [Google Scholar] [CrossRef]
- You, Z. Research on Trajectory Tracking Control of Unmanned Vehicle Based on MPC Algorithm; Jilin University: Changchun, China, 2018. [Google Scholar]
- Hoang, G.; Kim, H.K.; Kim, S.B. Path tracking controller of quadruped robot for obstacle avoidance using potential functions method. Int. J. Sci. Eng. 2013, 4, 1–5. [Google Scholar] [CrossRef]
- Dini, N.; Majd, V. An MPC-based two-dimensional push recovery of a quadruped robot in trotting gait using its reduced virtual model. Mech. Mach. Theory 2019, 146, 1–25. [Google Scholar] [CrossRef]
- Gong, J.; Xu, W.; Jiang, Y. Multiconstrained Model Predictive Control for Autonomous Ground Vehicle Trajectory Tracking. J. Beijing Inst. Technol. 2015, 24, 441–448. [Google Scholar] [CrossRef]
- Sun, Y. Research on Trajectory Tracking Control of Unmanned Vehicle Based on Model Predictive Control; Beijing Institute of Technology: Beijing, China, 2015. [Google Scholar]
- Zhao, T.; Xing, X.; Feng, W. Coordinated control for path following of two-wheel independently actuated autonomous ground vehicle. Intell. Transp. Syst. Iet 2019, 13, 628–635. [Google Scholar] [CrossRef]
- Wang, G.; Liu, L.; Meng, Y.; Gu, Q.; Bai, G. Integrated path tracking control of steering and braking based on holistic MPC. IFAC-Pap. 2021, 54, 45–50. [Google Scholar] [CrossRef]
- Jiang, Y.; Xu, X.; Zhang, L.; Zou, T. Model free predictive path tracking control of variable-configuration unmanned ground vehicle. ISA Trans. 2022, 38, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Jiang, Y.; Xu, X.; Zhang, L. Heading tracking of 6wid/4wis unmanned ground vehicles with variable wheelbase based on model free adaptive control. Mech. Syst. Signal Process. 2021, 159, 107715–107725. [Google Scholar] [CrossRef]
- Kang, J.; Kim, W.; Lee, J.; Yi, K. Design, implementation, and test of skid steering-based autonomous driving controller for a robotic vehicle with articulated suspension. J. Mech. Sci. Technol. 2010, 24, 793–800. [Google Scholar] [CrossRef]
- Xu, X.; Lu, S.; Chen, L.; Cai, Y.; Li, Y. Distributed drive based on differential and independent to coordinate unmanned vehicle trajectory tracking. J. Automob. Eng. 2018, 40, 475–481. [Google Scholar]
- Min, W.; Park, J.; Lee, B.; Man, H. The performance of independent wheels steering vehicle(4WS) applied Ackerman geometry. In Proceedings of the International Conference on Control, Seoul, Republic of Korea, 14–17 October 2008; pp. 197–202. [Google Scholar] [CrossRef]
- Economou, J.; Luk, P.; Tsourdos, A.; White, B. Hybrid modelling of an all-electric front-wheel Ackerman steered vehicle. In Proceedings of the Vehicular Technology Conference VTC 2003-Fall, Orlando, FL, USA, 6–9 October 2003; Volume 5, pp. 3294–3298. [Google Scholar] [CrossRef]
- Wang, Y.; Gao, J.; Li, K.; Chen, H. Integrated design of control allocation and triple-step control for over-actuated electric ground vehicles with actuator faults. J. Frankl. Inst. 2019, 357, 3150–3167. [Google Scholar] [CrossRef]
- Jiang, Y.; Meng, H.; Chen, G.; Yang, C.; Xu, X.; Zhang, L.; Xu, H. Differential-steering based path tracking control and energy-saving torque distribution strategy of 6wid unmanned ground vehicle. Energy 2022, 254, 1–15. [Google Scholar] [CrossRef]
- Yu, C.; Li, Z.; Tian, S. Straight Running Stability Control Based on Optimal Torque Distribution for a Four in-wheel Motor Drive Electric Vehicle. Energy Procedia 2017, 105, 2825–2830. [Google Scholar] [CrossRef]
- Wang, J.; Nan, J.; Xu, X.; Gao, Z.; Qiao, M. Control Strategy and Simulation of Four-wheel-hub Vehicles Torque Distribution on Bisectional Road. Energy Procedia 2017, 105, 2666–2671. [Google Scholar] [CrossRef]
- Xu, T.; Ji, X.; Liu, Y. Differential drive-based yaw stabilization using MPC for distributed-drive articulated heavy vehicle. IEEE Access 2020, 8, 104052–104062. [Google Scholar] [CrossRef]
- Shi, Y.; He, X.; Zou, W.; Yu, B.; Yuan, L.; Li, M.; Pan, G.; Ba, K. Multi-Objective Optimal Torque Control with Simultaneous Motion and Force Tracking for Hydraulic Quadruped Robots. Machines 2022, 10, 170. [Google Scholar] [CrossRef]
- Huang, Y.; Wei, Q.; Ma, H.; An, H. Motion planning for a bounding quadruped robot using ilqg based MPC. J. Phys. Conf. Ser. 2021, 1905, 012016. [Google Scholar] [CrossRef]
- Li, J.; Wang, J.; Wang, S. Neural Approximation-based Model Predictive Tracking Control of Non-holonomic Wheel-legged Robots. Int. J. Control. Autom. Systems 2021, 19, 372–381. [Google Scholar] [CrossRef]
- Xu, Y.; Yan, J.; Qian, H.; Lin, T. Design and Control of Intelligent Omnidirectional Hybrid Electric Vehicle; China Machine Press: Beijing, China, 2017; pp. 35–41. [Google Scholar]
- Liu, J.; Li, H.; Liu, Z. Discussion on Solving extreme Points by Lagrange Multiplier method. Res. Adv. Math. 2017, 20, 13–17. [Google Scholar] [CrossRef]
- Wang, Z.; Ding, X.; Zhang, L. Review on key technologies of anti-skid control for electric vehicle driven by four-wheel hub motor. J. Mech. Eng. 2019, 55, 99–120. [Google Scholar]
- Chen, L.; Liao, Z.; Zhang, Z. Anti-skid control of multi-wheel wheel motor driven vehicle based on road surface adaptive. Acta Armendarii. 2021, 10, 2278–2290. [Google Scholar]
- Feng, Y.; Yang, J.; Ji, Z.; Zhang, W. Control strategy of electric vehicle drive based on Optimal slip rate. Trans. Chin. Soc. Agric. Eng. 2015, 31, 119–125. [Google Scholar] [CrossRef]
- Hu, B.; Ying, H. Review of Research and Development of Fuzzy PID Control Technology and Some Important Problems it Faces. Acta Autom. Sin. 2001, 27, 567–584. [Google Scholar] [CrossRef]
- Zhang, Q.; Qu, S. Fuzzy PID Implementation of Vehicle Adaptive Cruise Control System. Automot. Eng. 2012, 307, 569–572. [Google Scholar] [CrossRef]











| Parameters | Values | Units |
|---|---|---|
| Sprung mass | 2900 | kg |
| Gravitational acceleration | 9.8 | m/s2 |
| The horizontal distance between the center of gravity and the front axle | 2 | m |
| The horizontal distance between the front axle and the middle axle | 2.2 | m |
| The horizontal distance between the rear axle and middle axle | 2.2 | m |
| Wheelbase | 2200 | mm |
| Height of center of gravity | 1.25 | m |
| Tire diameter | 0.996 | m |
| Tire width | 0.309 | m |
| Power of in-wheel motor | 65 | kW |
| Maximum off-road speed | 45 | (km/h) |
| Sampling period | 5 | ms |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zou, T.; You, Y.; Meng, H.; Chang, Y. Research on Six-Wheel Distributed Unmanned Vehicle Path Tracking Strategy Based on Hierarchical Control. Biomimetics 2022, 7, 238. https://doi.org/10.3390/biomimetics7040238
Zou T, You Y, Meng H, Chang Y. Research on Six-Wheel Distributed Unmanned Vehicle Path Tracking Strategy Based on Hierarchical Control. Biomimetics. 2022; 7(4):238. https://doi.org/10.3390/biomimetics7040238
Chicago/Turabian StyleZou, Teng’an, Yulong You, Hao Meng, and Yukang Chang. 2022. "Research on Six-Wheel Distributed Unmanned Vehicle Path Tracking Strategy Based on Hierarchical Control" Biomimetics 7, no. 4: 238. https://doi.org/10.3390/biomimetics7040238
APA StyleZou, T., You, Y., Meng, H., & Chang, Y. (2022). Research on Six-Wheel Distributed Unmanned Vehicle Path Tracking Strategy Based on Hierarchical Control. Biomimetics, 7(4), 238. https://doi.org/10.3390/biomimetics7040238





