A Novel Digital Design Approach for Metal Additive Manufacturing to Address Local Thermal Effects
Abstract
:1. Introduction
2. Design Approach Overview
2.1. Digital Design Approach for Metal AM That Addresses Thermal Effects
2.2. Design Optimization and Analysis Steps
2.3. Process Simulation Step
2.4. Lattice Introduction Step
2.5. Lattice Optimization Step
3. Results
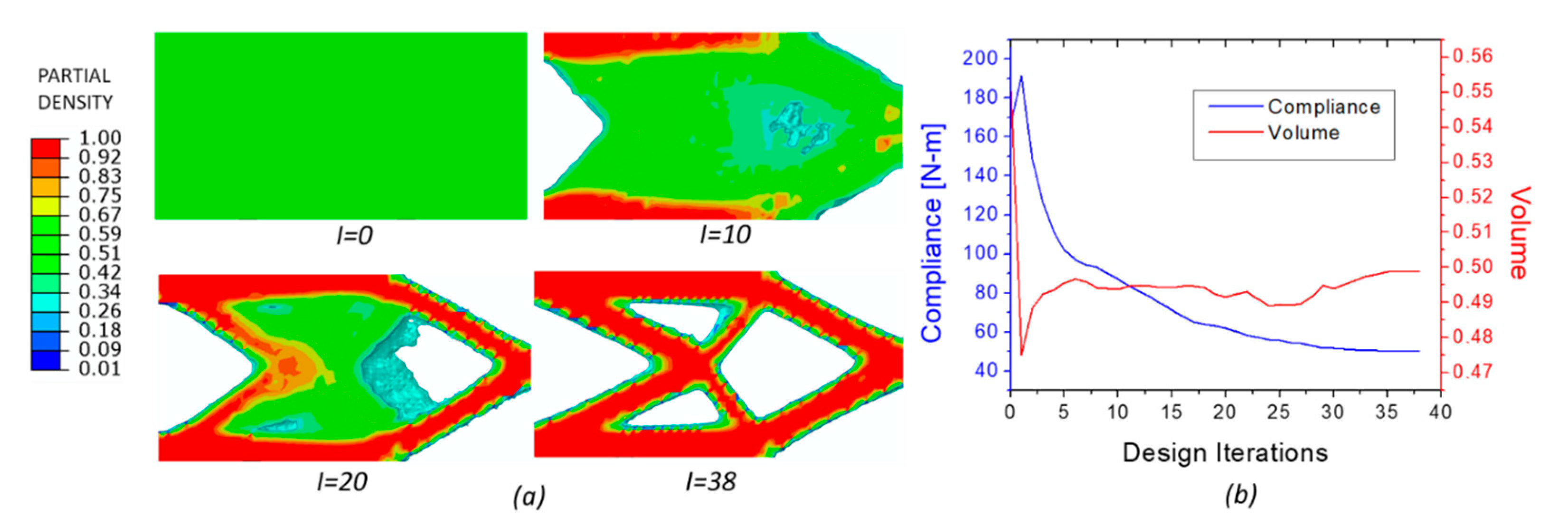
3.1. Topology Optimization
3.2. Process Simulations
3.3. Lattice Introduction
3.4. Lattice Optimization
3.5. Effect of Lattice on Hotspot
4. Discussion
4.1. Topology Optimization and CAD Generation
4.2. Lattice Optimization
4.3. Process Simulations
4.4. Advantages and Limitations of Proposed Methodology
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Yan, R.; Luo, D.; Huang, H.; Li, R.; Yu, N.; Liu, C.; Hu, M.; Rong, Q. Electron beam melting in the fabrication of three-dimensional mesh titanium mandibular prosthesis scaffold. Sci. Rep. 2018, 8, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Zadpoor, A.A.; Malda, J. Additive Manufacturing of Biomaterials, Tissues, and Organs. Ann. Biomed. Eng. 2016, 45, 1–11. [Google Scholar] [CrossRef]
- Orme, M.; Madera, I.; Gschweitl, M.; Ferrari, M. Topology Optimization for Additive Manufacturing as an Enabler for Light Weight Flight Hardware. Designs 2018, 2, 51. [Google Scholar] [CrossRef] [Green Version]
- Chadha, C.; Crowe, K.A.; Carmen, C.L.; Patterson, A.E. Exploring an AM-Enabled Combination-of-Functions Approach for Modular Product Design. Designs 2018, 2, 37. [Google Scholar] [CrossRef] [Green Version]
- Singamneni, S.; Lv, Y.; Hewitt, A.; Chalk, R.; Thomas, W.; Jordison, D. Additive Manufacturing for the Aircraft Industry: A Review. J. Aeronaut. Aerosp. Eng. 2019, 8, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Wieding, J.; Jonitz, A.; Bader, R. The Effect of Structural Design on Mechanical Properties and Cellular Response of Additive Manufactured Titanium Scaffolds. Materials 2012, 5, 1336–1347. [Google Scholar] [CrossRef]
- Bose, S.; Robertson, S.F.; Bandyopadhyay, A. Surface modification of biomaterials and biomedical devices using additive manufacturing. Acta Biomater. 2018, 66, 6–22. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Carroll, B.E.; Palmer, T.A.; Beese, A.M. Anisotropic tensile behavior of Ti-6Al-4V components fabricated with directed energy deposition additive manufacturing. Acta Mater. 2015, 87, 309–320. [Google Scholar] [CrossRef]
- Evans, A.; Hutchinson, J.; Ashby, M. Multifunctionality of cellular metal systems. Prog. Mater. Sci. 1998, 43, 171–221. [Google Scholar] [CrossRef]
- Wauthle, R.; Vrancken, B.; Beynaerts, B.; Jorissen, K.; Schrooten, J.; Kruth, J.-P.; Van Humbeeck, J. Effects of build orientation and heat treatment on the microstructure and mechanical properties of selective laser melted Ti6Al4V lattice structures. Addit. Manuf. 2015, 5, 77–84. [Google Scholar] [CrossRef]
- DebRoy, T.; Wei, H.L.; Zuback, J.S.; Mukherjee, T.; Elmer, J.W.; Milewski, J.O.; Beese, A.M.; Wilson-Heid, A.; De, A.; Zhang, W. Additive manufacturing of metallic components–Process, structure and properties. Prog. Mater. Sci. 2018, 92, 112–224. [Google Scholar] [CrossRef]
- Murr, L.; Gaytan, S.M.; Ramirez, D.A.; Martinez, E.; Hernandez, J.; Amato, K.N.; Shindo, P.W.; Medina, F.R.; Wicker, R.B. Metal Fabrication by Additive Manufacturing Using Laser and Electron Beam Melting Technologies. J. Mater. Sci. Technol. 2012, 28, 1–14. [Google Scholar] [CrossRef]
- Calignano, F. Investigation of the accuracy and roughness in the laser powder bed fusion process. Virtual Phys. Prototyp. 2018, 13, 97–104. [Google Scholar] [CrossRef]
- Saboori, A.; Aversa, A.; Marchese, G.; Biamino, S.; Lombardi, M.; Fino, P. Microstructure and Mechanical Properties of AISI 316L Produced by Directed Energy Deposition-Based Additive Manufacturing: A Review. Appl. Sci. 2020, 10, 3310. [Google Scholar] [CrossRef]
- Beese, A.M.; Carroll, B.E. Review of Mechanical Properties of Ti-6Al-4V Made by Laser-Based Additive Manufacturing Using Powder Feedstock. JOM 2015, 68, 724–734. [Google Scholar] [CrossRef]
- Gaynor, A.T.; Guest, J.K. Topology optimization considering overhang constraints: Eliminating sacrificial support material in additive manufacturing through design. Struct. Multidiscip. Optim. 2016, 54, 1157–1172. [Google Scholar] [CrossRef]
- Langelaar, M. An additive manufacturing filter for topology optimization of print-ready designs. Struct. Multidiscip. Optim. 2016, 55, 871–883. [Google Scholar] [CrossRef] [Green Version]
- Wang, C.; Qian, X. Simultaneous optimization of build orientation and topology for additive manufacturing. Addit. Manuf. 2020, 34. [Google Scholar] [CrossRef]
- Han, Y.; Xu, B.; Zhao, L.; Xie, Y.M. Topology optimization of continuum structures under hybrid additive-subtractive manufacturing constraints. Struct. Multidiscip. Optim. 2019, 60, 2571–2595. [Google Scholar] [CrossRef]
- Manogharan, G.; Wysk, R.; Harrysson, O.; Aman, R. AIMS-A Metal Additive-hybrid Manufacturing System: System Architecture and Attributes. Procedia Manuf. 2015, 1, 273–286. [Google Scholar] [CrossRef] [Green Version]
- Panesar, A.; Abdi, M.; Hickman, D.; Ashcroft, I. Strategies for functionally graded lattice structures derived using topology optimisation for Additive Manufacturing. Addit. Manuf. 2018, 19, 81–94. [Google Scholar] [CrossRef]
- Echeta, I.; Feng, X.; Dutton, B.; Leach, R.; Piano, S. Review of defects in lattice structures manufactured by powder bed fusion. Int. J. Adv. Manuf. Technol. 2019, 106, 2649–2668. [Google Scholar] [CrossRef] [Green Version]
- Pasini, D.; Guest, J.K. Imperfect architected materials: Mechanics and topology optimization. MRS Bull. 2019, 44, 766–772. [Google Scholar] [CrossRef]
- Miki, T.; Yamada, T. Topology optimization for considering distortion in additive manufacturing. Struct. Multidiscip. Optim. 2020. [Google Scholar] [CrossRef]
- Liu, J.; Gaynor, A.T.; Chen, S.; Kang, Z.; Suresh, K.; Takezawa, A.; Li, L.; Kato, J.; Tang, J.; Wang, C.C.L.; et al. Current and future trends in topology optimization for additive manufacturing. Struct. Multidiscip. Optim. 2018, 57, 2457–2483. [Google Scholar] [CrossRef] [Green Version]
- Li, C.; Liu, Z.; Fang, X.; Guo, Y.B. Residual Stress in Metal Additive Manufacturing. Procedia CIRP 2018, 71, 348–353. [Google Scholar] [CrossRef]
- Simson, T.; Emmel, A.; Dwars, A.; Böhm, J. Residual stress measurements on AISI 316L samples manufactured by selective laser melting. Addit. Manuf. 2017, 17, 183–189. [Google Scholar] [CrossRef]
- Baufeld, B.; Biest, O.; Gault, R. Additive manufacturing of Ti-6Al-4V components by shaped metal deposition: Microstructure and mechanical properties. Mater. Des. 2010, 31, S106–S111. [Google Scholar] [CrossRef]
- Shao, J.; Yu, G.; He, X.; Li, S.; Chen, R.; Zhao, Y. Grain size evolution under different cooling rate in laser additive manufacturing of superalloy. Opt. Laser Technol. 2019, 119. [Google Scholar] [CrossRef] [Green Version]
- Gockel, J.; Beuth, J.; Taminger, K. Integrated control of solidification microstructure and melt pool dimensions in electron beam wire feed additive manufacturing of Ti-6Al-4V. Addit. Manuf. 2014, 1, 119–126. [Google Scholar] [CrossRef]
- Monkova, K.; Monka, P. Qualitative parameters of complex part produced by additive approach. In Proceedings of the 2017 8th International Conference on Mechanical and Aerospace Engineering (ICMAE), Prague, Czech Republic, 22–25 July 2017; Institute of Electrical and Electronics Engineers (IEEE): Prague, Czech Republic, 2017; pp. 691–694. [Google Scholar]
- Gouge, M.; Denlinger, E.; Irwin, J.; Li, C.; Michaleris, P. Experimental validation of thermo-mechanical part-scale modeling for laser powder bed fusion processes. Addit. Manuf. 2019, 29, 100771. [Google Scholar] [CrossRef]
- Cheng, L.; To, A.C. Part-scale build orientation optimization for minimizing residual stress and support volume for metal additive manufacturing: Theory and experimental validation. Comput. Des. 2019, 113, 1–23. [Google Scholar] [CrossRef]
- Choo, H.; Sham, K.-L.; Bohling, J.; Ngo, A.; Xiao, X.; Ren, Y.; Depond, P.; Matthews, M.J.; Garlea, E. Effect of laser power on defect, texture, and microstructure of a laser powder bed fusion processed 316L stainless steel. Mater. Des. 2019, 164, 107534. [Google Scholar] [CrossRef]
- Zhang, B.; Meng, W.J.; Shamsaei, N.; Phan, N.; Shamsaei, N. Effect of heat treatments on pore morphology and microstructure of laser additive manufactured parts. Mater. Des. Process. Commun. 2019, 1, e29. [Google Scholar] [CrossRef] [Green Version]
- Bobbio, L.D.; Qin, S.; Dunbar, A.; Michaleris, P.; Beese, A.M. Characterization of the strength of support structures used in powder bed fusion additive manufacturing of Ti-6Al-4V. Addit. Manuf. 2017, 14, 60–68. [Google Scholar] [CrossRef]
- Ranjan, R.; Yang, Y.; Ayas, C.; Langelaar, M.; Van Keulen, F. Controlling local overheating in topology optimization for additive manufacturing. In Proceedings of the Euspen Special Interest Group Meeting: Additive Manufacturing, Leuven, Belgium, 10–11 October 2017. [Google Scholar]
- Son, K.N.; Weibel, J.A.; Kumaresan, V.; Garimella, S.V. Design of multifunctional lattice-frame materials for compact heat exchangers. Int. J. Heat Mass Transf. 2017, 115, 619–629. [Google Scholar] [CrossRef] [Green Version]
- Cheng, L.; Liu, J.; Liang, X.; To, A.C. Coupling lattice structure topology optimization with design-dependent feature evolution for additive manufactured heat conduction design. Comput. Methods Appl. Mech. Eng. 2018, 332, 408–439. [Google Scholar] [CrossRef]
- Lynch, M.E.; Mordasky, M.; Cheng, L.; To, A. Design, testing, and mechanical behavior of additively manufactured casing with optimized lattice structure. Addit. Manuf. 2018, 22, 462–471. [Google Scholar] [CrossRef]
- Sidambe, A.T. Biocompatibility of Advanced Manufactured Titanium Implants-A Review. Materials 2014, 7, 8168–8188. [Google Scholar] [CrossRef] [Green Version]
- Najafi, A.R.; Safdari, M.; Tortorelli, D.A.; Geubelle, P. Shape optimization using a NURBS-based interface-enriched generalized FEM. Int. J. Numer. Methods Eng. 2017, 111, 927–954. [Google Scholar] [CrossRef]
- Zuo, Z.H.; Xie, Y. A simple and compact Python code for complex 3D topology optimization. Adv. Eng. Softw. 2015, 85, 1–11. [Google Scholar] [CrossRef]
- Cheng, B.; Shrestha, S.; Chou, Y.K. Stress and Deformation Evaluations of Scanning Strategy Effect in Selective Laser Melting. Processing 2016, 12. [Google Scholar] [CrossRef]
- Dunbar, A.J.; Denlinger, E.R.; Gouge, M.F.; Michaleris, P. Experimental validation of finite element modeling for laser powder bed fusion deformation. Addit. Manuf. 2016, 12, 108–120. [Google Scholar] [CrossRef]
- Denlinger, E.R.; Gouge, M.; Irwin, J.; Michaleris, P. Thermomechanical model development and in situ experimental validation of the Laser Powder-Bed Fusion process. Addit. Manuf. 2017, 16, 73–80. [Google Scholar] [CrossRef]
- Brown, W.F.; Mindlin, H.; Ho, C.Y. Aerospace Structural Metals Handbook; CINDAS/Purdue University: West Lafayette, IN, USA, 1995. [Google Scholar]
- Xiao, H. 11.5.2.1 Thermal Evaporation. In Introduction to Semiconductor Manufacturing Technology; Society of Photo-Optical Instrumentation Engineers (SPIE): Bellingham, WA, USA, 2012; p. 485. [Google Scholar]
- Zhang, G.; Chen, J.; Zheng, M.; Yan, Z.; Lu, X.; Lin, X.; Huang, W. Element Vaporization of Ti-6Al-4V Alloy during Selective Laser Melting. Metals 2020, 10, 435. [Google Scholar] [CrossRef] [Green Version]
- Rai, R.; Elmer, J.W.; Palmer, T.A.; Debroy, T. Heat transfer and fluid flow during keyhole mode laser welding of tantalum, Ti-6Al-4V, 304L stainless steel and vanadium. J. Phys. D Appl. Phys. 2007, 40, 5753–5766. [Google Scholar] [CrossRef] [Green Version]
- Hooper, P. Melt pool temperature and cooling rates in laser powder bed fusion. Addit. Manuf. 2018, 22, 548–559. [Google Scholar] [CrossRef]
- Cheng, L.; Liu, J.; To, A.C. Concurrent lattice infill with feature evolution optimization for additive manufactured heat conduction design. Struct. Multidiscip. Optim. 2018, 58, 511–535. [Google Scholar] [CrossRef]









| Parameters | Value |
|---|---|
| SLM Printer model | EOS M 290 |
| Laser Power (Watts) | 250 |
| Heat source absorption efficiency (%) | 40 |
| Laser beam diameter (mm) | 0.15 |
| Travel speed (mm/s) | 1000 |
| Layer thickness (mm) | 0.04 |
| Hatch spacing (mm) | 0.15 |
| Interlayer rotation angle | 67° |
| Lattice Parameters | Value |
|---|---|
| Unit cell | X/W/Star |
| Unit cell dimension (mm) | 15 × 10 × 10 |
| Min. beam radius (mm) | 1 |
| Max. beam radius (mm) | 4 |
| Parameters | Topology Optimized Beam | TO Beam with X Lattice | TO Beam with W Lattice | TO Beam with Star Lattice |
|---|---|---|---|---|
| Part Volume (cm3) | 307.75 | 267.73 | 272.21 | 269.85 |
| Support Volume (cm3) | 8.16 | 8.95 | 8.94 | 8.96 |
| Build time (hhh:mm:ss) | 84:21:59 | 76:30:55 | 77:24:51 | 77:47:56 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
I. Perumal, V.; R. Najafi, A.; Kontsos, A. A Novel Digital Design Approach for Metal Additive Manufacturing to Address Local Thermal Effects. Designs 2020, 4, 41. https://doi.org/10.3390/designs4040041
I. Perumal V, R. Najafi A, Kontsos A. A Novel Digital Design Approach for Metal Additive Manufacturing to Address Local Thermal Effects. Designs. 2020; 4(4):41. https://doi.org/10.3390/designs4040041
Chicago/Turabian StyleI. Perumal, Vignesh, Ahmad R. Najafi, and Antonios Kontsos. 2020. "A Novel Digital Design Approach for Metal Additive Manufacturing to Address Local Thermal Effects" Designs 4, no. 4: 41. https://doi.org/10.3390/designs4040041
APA StyleI. Perumal, V., R. Najafi, A., & Kontsos, A. (2020). A Novel Digital Design Approach for Metal Additive Manufacturing to Address Local Thermal Effects. Designs, 4(4), 41. https://doi.org/10.3390/designs4040041







