Trihedral Lattice Towers Optimization with a Limitation on the Resonant Vortex Excitation Occurrence
Abstract
:1. Introduction
2. Materials and Methods
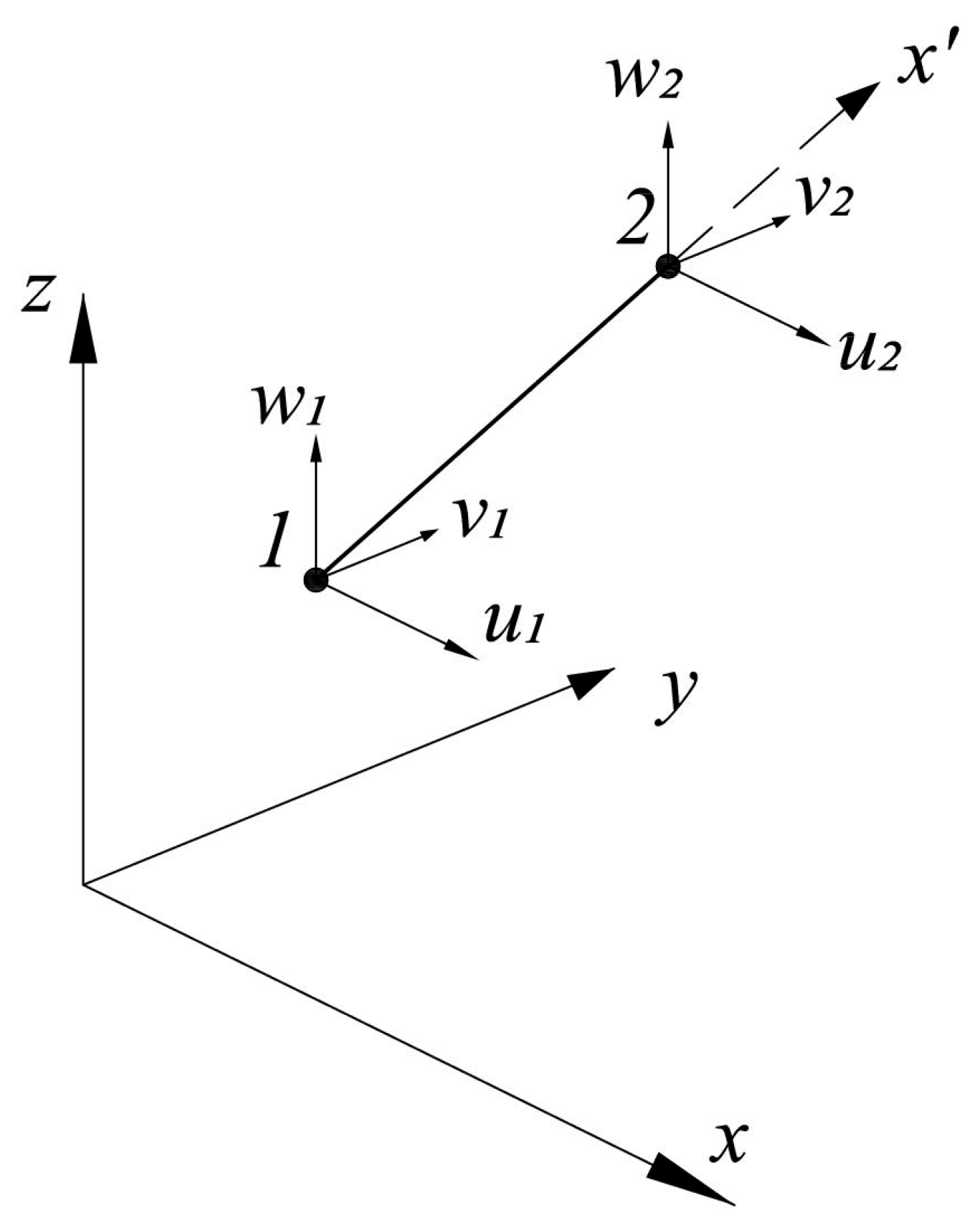
2.1. Formulation of the Problem
- is the coefficient taking into account the change in the thickness of the ice along the height. It is adopted according to Table 12.3 of SR 20.13330.2016;
- is the coefficient that takes into account the change in the thickness of the ice depending on the diameter of the elements of the circular cross-section. It is determined according to Table 12.4 of SR 20.13330.2016;
- is the coefficient that takes into account the ratio of the surface area of the element subject to icing to the total surface area of the element. is taken equal to 0.6;
- is the ice density taken equal to 900 kg/m3;
- is the acceleration of gravity, m/s2.
- (1)
- for structures in which the first natural frequency , Hz, is greater than the limit value of natural frequency (according to Table 11.5 of SR 20.13330.2016):
- (2)
- for structures with :
- (3)
- For structures, in which the second natural frequency is less than the limiting one, the dynamic calculation is made taking into account the s first vibration modes. The number s is determined from the condition
- r is the total number of nodes;
- q is the number of degrees of freedom in the node;
- is the nodal load from the average component of the wind pressure in the node k in the direction of the l-th degree of freedom.
- 1.
- Structural elements must satisfy the conditions of strength and stability , where N is the axial force in the element, A is the cross-sectional area of the element, φ is the coefficient of buckling, γc is the coefficient of working conditions, Ry is the design strength of steel.
- 2.
- The slenderness of the elements should not exceed the limit values . Ultimate slenderness is determined in accordance with SR 16.13330.2017. For compressed chord elements:
- 3.
- Resonant vortex excitation should not occur in the structure. In accordance with SR 20.13330.2016, resonant vortex excitation in the i-th eigenmode does not occur if
- 4.
- Variable parameters must lie in the range ()
- 5.
- Parameters and must be integers.
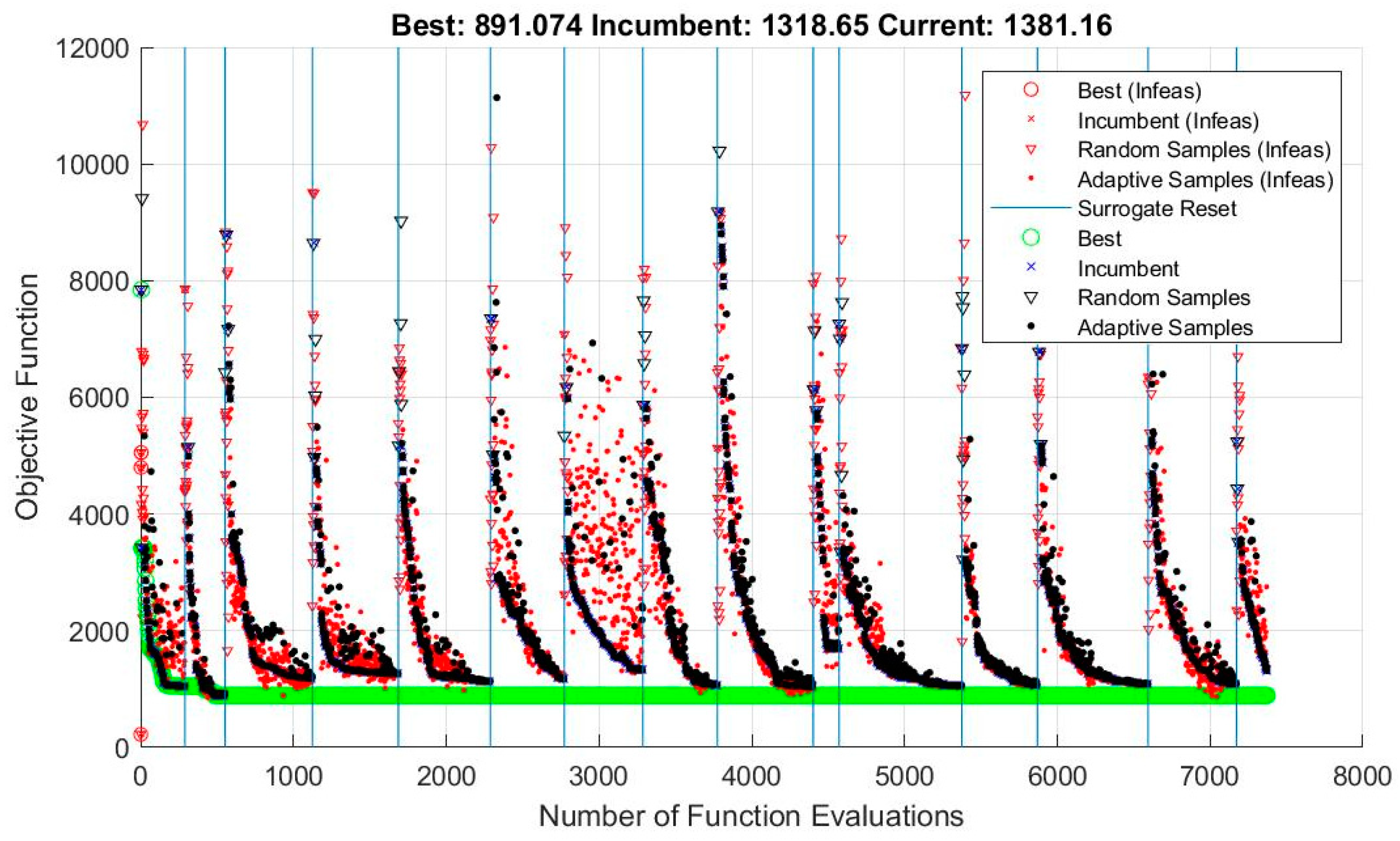
2.2. Technique for Solving the Optimization Problem
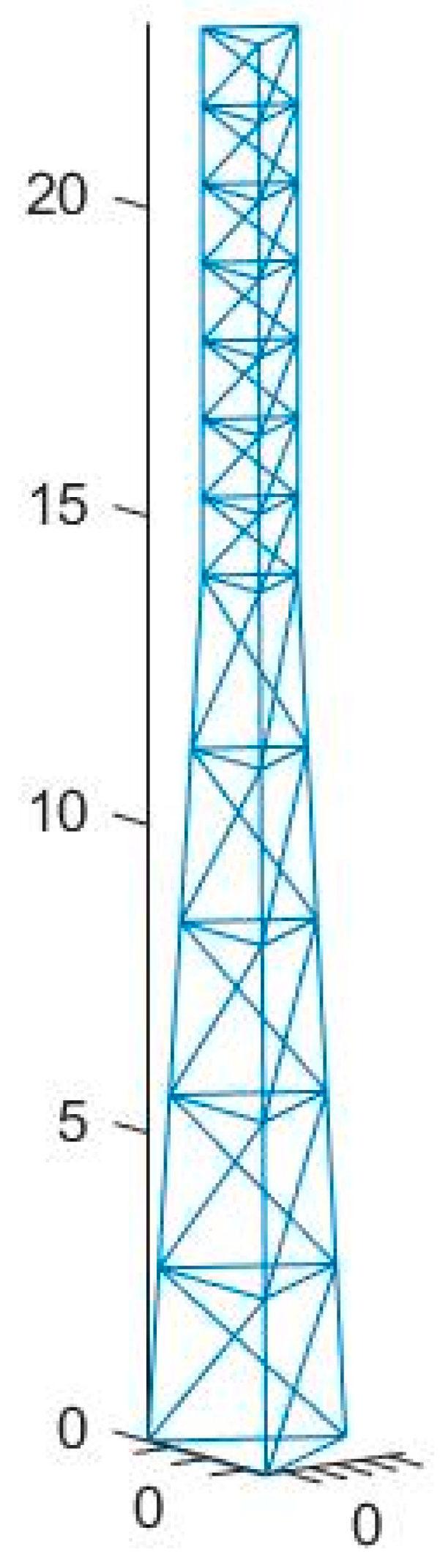
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shu, Q.; Huang, Z.; Yuan, G.; Ma, W.; Ye, S.; Zhou, J. Impact of wind loads on the resistance capacity of the transmission tower subjected to ground surface deformations. Thin-Walled Struct. 2018, 131, 619–630. [Google Scholar] [CrossRef]
- Yuan, G.; Yang, B.; Huang, Z.; Tan, X. Experimental study on the stability of the transmission tower with hybrid slab foundation. Eng. Struct. 2018, 162, 151–165. [Google Scholar] [CrossRef] [Green Version]
- Xie, Q.; Zhang, J. Experimental study on failure modes and retrofitting method of latticed transmission tower. Eng. Struct. 2021, 226, 111365. [Google Scholar] [CrossRef]
- Tian, L.; Pan, H.; Ma, R.; Zhang, L.; Liu, Z. Full-scale test and numerical failure analysis of a latticed steel tubular transmission tower. Eng. Struct. 2020, 208, 109919. [Google Scholar] [CrossRef]
- Singh, V.K.; Gautam, A.K. Study on Evaluation of Angle Connection for Transmission Towers. In International Conference on Advances in Structural Mechanics and Applications; Springer: Cham, Switzerland, 2022; pp. 353–363. [Google Scholar] [CrossRef]
- Axisa, R.; Muscat, M.; Sant, T.; Farrugia, R.N. Structural assessment of a lattice tower for a small, multi-bladed wind turbine. Int. J. Energy Environ. Eng. 2017, 8, 343–358. [Google Scholar] [CrossRef] [Green Version]
- Zwick, D.; Muskulus, M.; Moe, G. Iterative optimization approach for the design of full-height lattice towers for offshore wind turbines. Energy Procedia 2012, 24, 297–304. [Google Scholar] [CrossRef] [Green Version]
- Chew, K.H.; Tai, K.; Ng, E.Y.K.; Muskulus, M. Optimization of offshore wind turbine support structures using an analytical gradient-based method. Energy Procedia 2015, 80, 100–107. [Google Scholar] [CrossRef] [Green Version]
- Das, A. Modelling and analysis of lattice towers for wind turbines. Int. J. Sci. Res. 2015, 4, 999–1003. [Google Scholar]
- Stavridou, N.; Koltsakis, E.; Baniotopoulos, C. Structural analysis and optimal design of steel lattice wind turbine towers. Proc. Inst. Civ. Eng. -Struct. Build. 2019, 172, 564–579. [Google Scholar] [CrossRef]
- Balagopal, R.; Prasad Rao, N.; Rokade, R.P. Simplified model to predict deflection and natural frequency of steel pole structures. J. Inst. Eng. (India) Ser. A. 2018, 99, 595–607. [Google Scholar] [CrossRef]
- Zhou, Q.; Zhao, L.; Zhu, Q.; Zhu, Y. Mean wind loads on equilateral triangular lattice tower under skewed wind loading. J. Wind Eng. Ind. Aerodyn. 2021, 208, 104467. [Google Scholar] [CrossRef]
- Ghugal, Y.M.; Salunkhe, U.S. Analysis and Design of three and four legged 400kV steel transmission line towers: Comparative study. Int. J. Earth Sci. Eng. 2011, 4, 691–694. [Google Scholar]
- Preeti, C.; Mohan, K.J. Analysis of transmission towers with different configurations. Jordan J. Civ. Eng. 2013, 7, 450–460. [Google Scholar]
- Rao, N.P.; Balagopal, R.; Rokade, R.P.; Mohan, S.J. Schifflerised angle sections for triangular-based communication towers. IES J. Part A Civ. Struct. Eng. 2013, 6, 189–198. [Google Scholar] [CrossRef]
- Feng, R.Q.; Liu, F.C.; Xu, W.J.; Ma, M.; Liu, Y. Topology optimization method of lattice structures based on a genetic algorithm. Int. J. Steel Struct. 2016, 16, 743–753. [Google Scholar] [CrossRef]
- Jovašević, S.; Mohammadi, M.R.S.; Rebelo, C.; Pavlović, M.; Veljković, M. New Lattice-Tubular Tower for Onshore WEC–Part 1: Structural Optimization. Procedia Eng. 2017, 199, 3236–3241. [Google Scholar] [CrossRef]
- Fu, J.Y.; Wu, B.G.; Wu, J.R.; Deng, T.; Pi, Y.L.; Xie, Z.N. Wind resistant size optimization of geometrically nonlinear lattice structures using a modified optimality criterion method. Eng. Struct. 2018, 173, 573–588. [Google Scholar] [CrossRef]
- Sivakumar, P.; Rajaraman, A.; Samuel Knight, G.M.; Ramachandramurthy, D.S. Object-Oriented Optimization Approach Using Genetic Algorithms for Lattice Towers. J. Comput. Civ. Eng. 2004, 18, 162–171. [Google Scholar] [CrossRef]
- Nguyen, T.-H.; Vu, A.-T. Weight optimization of steel lattice transmission towers based on Differential Evolution and machine learning classification technique. Frat. Ed Integrità Strutt. 2022, 59, 172–187. [Google Scholar] [CrossRef]
- Chen, J.; Yang, R.; Ma, R.; Li, J. Design optimization of wind turbine tower with lattice-tubular hybrid structure using particle swarm algorithm. Struct. Des. Tall Spec. Build. 2016, 25, 743–758. [Google Scholar] [CrossRef]
- Akhtyamova, L.; Chepurnenko, A.; Rozen, M.; Al-Wali, E. Trihedral lattice towers geometry optimization. E3S Web Conf. 2021, 281, 01024. [Google Scholar] [CrossRef]
- Badertdinov, I.R.; Kuznetsov, I.L.; Kashapov, N.F.; Gilmanshin, I.R.; Sabitov, L.S. Optimal geometrical parameters of trihedral steel support’s cross section. IOP Conf. Ser. Mater. Sci. Eng. 2018, 412, 012005. [Google Scholar] [CrossRef]
- Chepurnenko, A.S.; Sabitov, L.S.; Yazyev, B.M.; Galimyanova, G.R.; Akhtyamova, L.S. Trihedral lattice towers with optimal cross-sectional shape. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1083, 012012. [Google Scholar] [CrossRef]
- Kaveh, A.; Hamedani, K.B.; Hamedani, B.B. Optimal Design of Large-scale Dome Truss Structures with Multiple Frequency Constraints Using Success-history Based Adaptive Differential Evolution Algorithm. Period. Polytech. Civ. Eng. 2022. Available online: https://pp.bme.hu/ci/article/view/21147 (accessed on 2 December 2022).
- Kaveh, A.; Zaerreza, A.; Hosseini, S.M. An enhanced shuffled Shepherd Optimization Algorithm for optimal design of large-scale space structures. Eng. Comput. 2022, 38, 1505–1526. [Google Scholar] [CrossRef]
- Pilarska, D. Two subdivision methods based on the regular octahedron for single-and double-layer spherical geodesic domes. Int. J. Space Struct. 2020, 35, 160–173. [Google Scholar] [CrossRef]
- Pilarska, D.; Maleska, T. Numerical Analysis of Steel Geodesic Dome under Seismic Excitations. Materials 2021, 14, 4493. [Google Scholar] [CrossRef]
- Szafran, J.; Rykaluk, K. A full-scale experiment of a lattice telecommunication tower under breaking load. J. Constr. Steel Res. 2016, 120, 160–175. [Google Scholar] [CrossRef]
- Szafran, J.; Juszczyk, K.; Kamiński, M. Reliability assessment of steel lattice tower subjected to random wind load by the stochastic finite-element method. ASCE-ASME J. Risk Uncertain. Eng. Syst. Part A Civ. Eng. 2020, 6, 04020003. [Google Scholar] [CrossRef]
- Ruscheweyh, H. Vortex excited vibrations. In Wind-Excited Vibrations of Structures; Springer: Vienna, Austria, 1994; pp. 51–84. [Google Scholar]
- Müller, J.; Day, M. Surrogate optimization of computationally expensive black-box problems with hidden constraints. INFORMS J. Comput. 2019, 31, 689–702. [Google Scholar] [CrossRef]
- Gutmann, H.-M. A Radial Basis Function Method for Global Optimization. J. Glob. Optim. 2001, 19, 201–227. [Google Scholar] [CrossRef]
- Mirjalili, S. Genetic algorithm. In Evolutionary Algorithms and Neural Networks; Springer: Cham, Switzerland, 2019; pp. 43–55. [Google Scholar]
- Structural Engineering Software|Liraland Group. Available online: https://www.liraland.com/ (accessed on 2 December 2022).
- Khodadadi, A.; Buelow, P.V. Form exploration and GA-based optimization of lattice towers comparing with Shukhov water tower. In Proceedings of the IASS Annual Symposia. International Association for Shell and Spatial Structures (IASS), Brasilia, Brazil, 15–19 September 2014; No. 16. pp. 1–8. [Google Scholar]
- Akıncıoğlu, S. Taguchi optimization of multiple performance characteristics in the electrical discharge machining of the TiGr2. Facta Univ. Ser. Mech. Eng. 2022, 20, 237–253. [Google Scholar] [CrossRef]
- Wirowski, A. Optimization of fundamental natural frequency of structures using VPL on the example of truss towers. Vib. Phys. Syst. 2020, 31, 2020229-1–2020229-8. [Google Scholar]
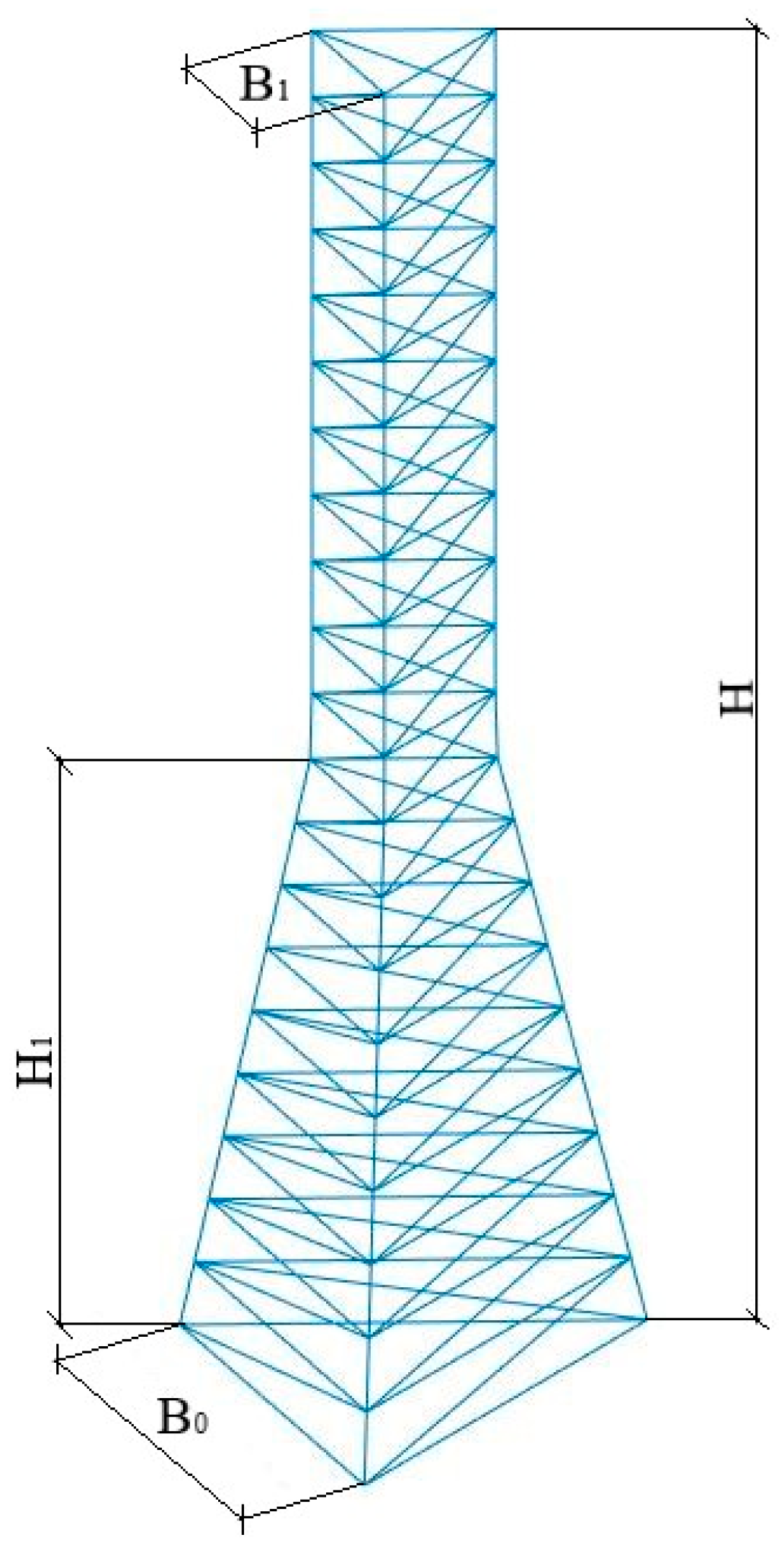

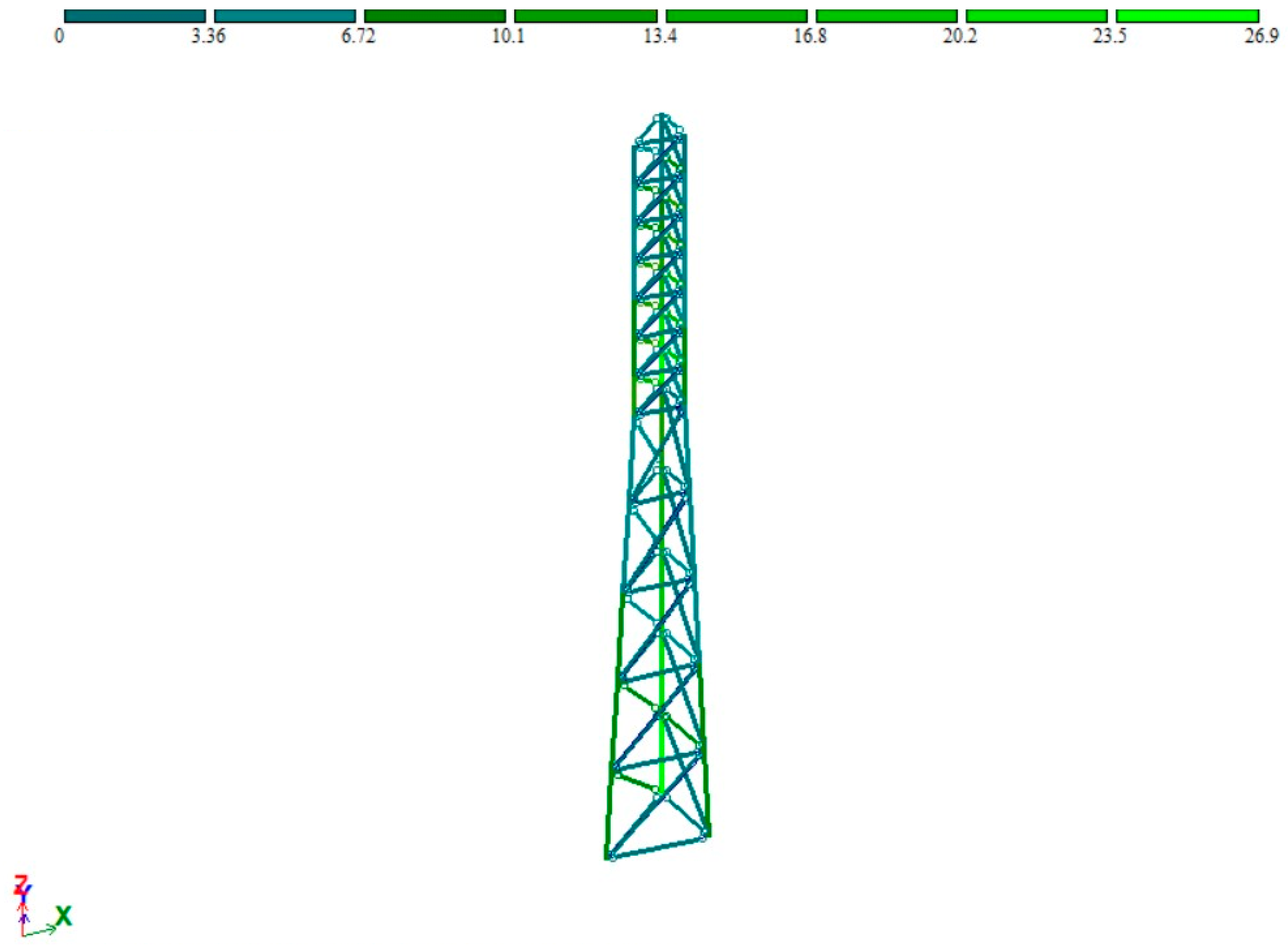
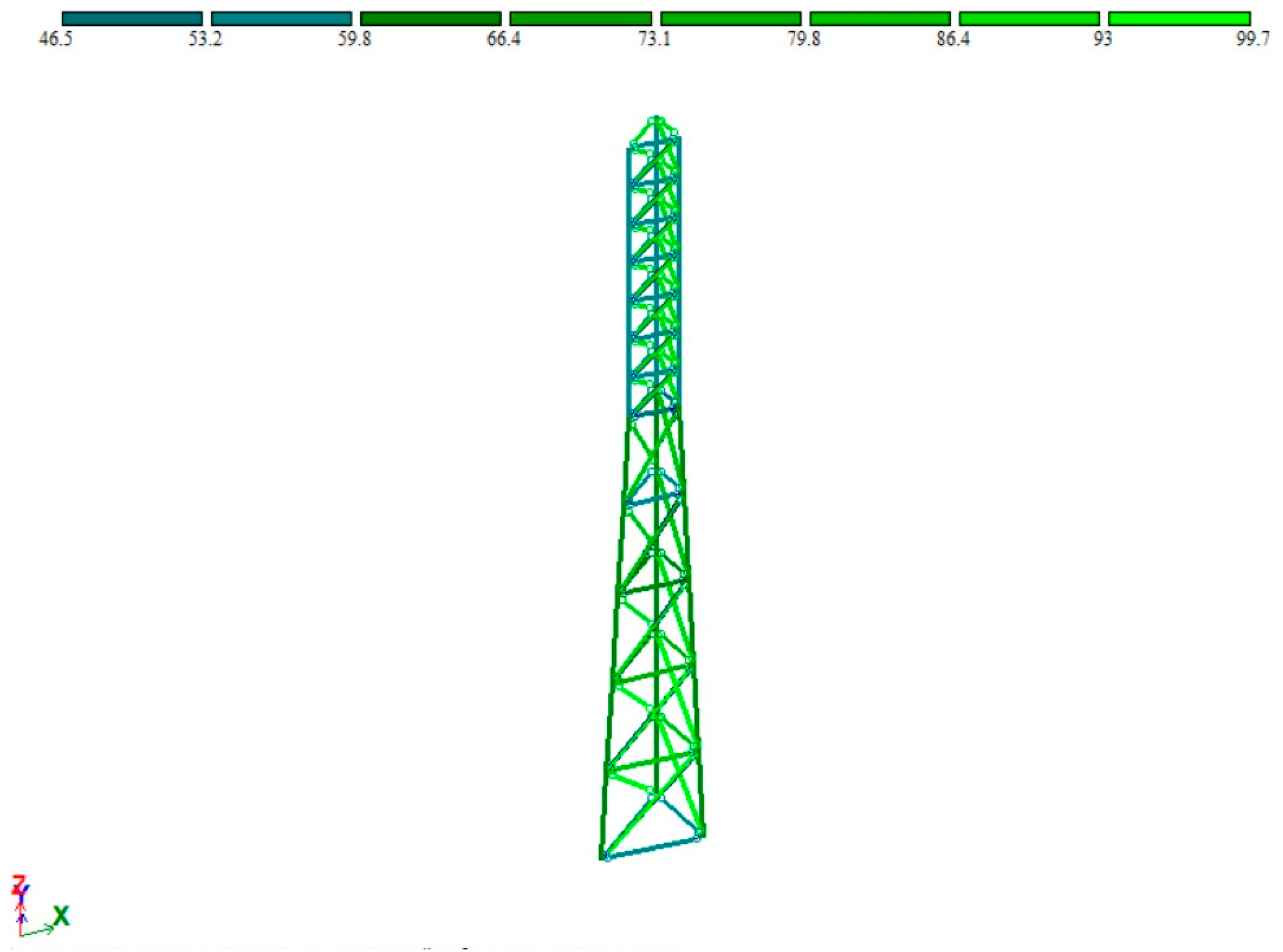
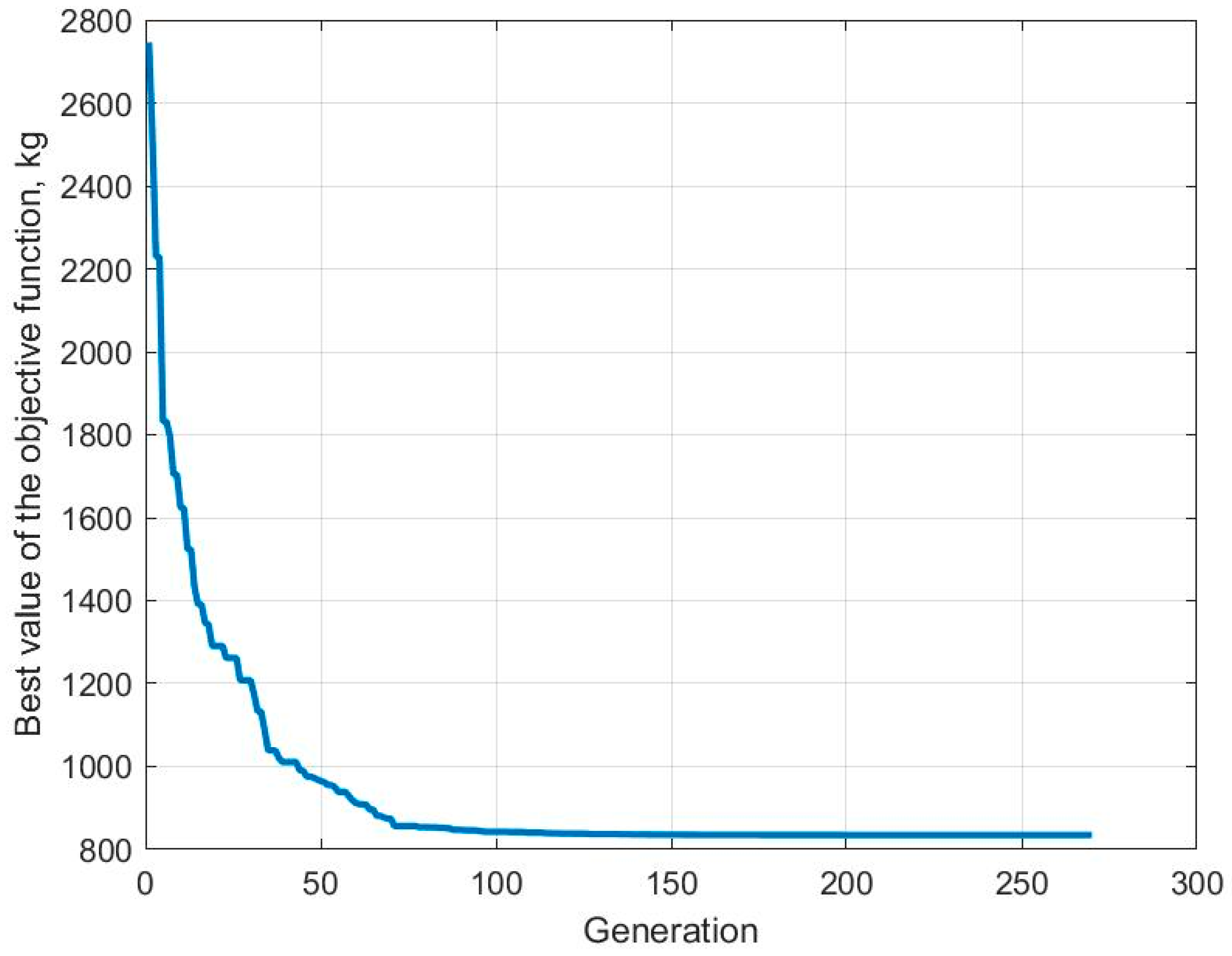
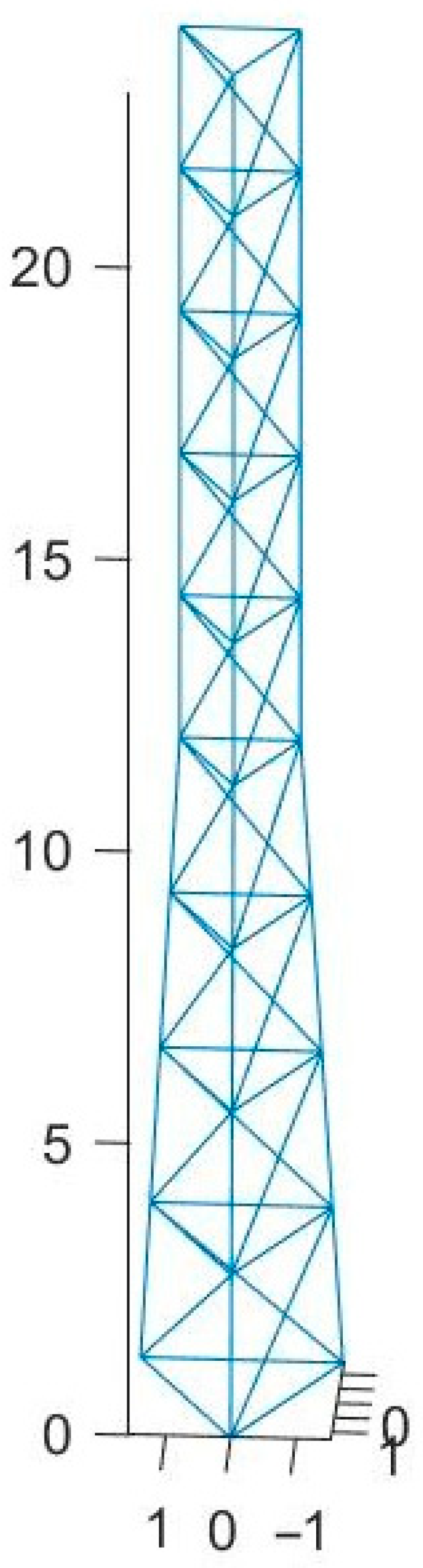

| Parameter | B0, m | B1, m | H1, m | Dp, Dp1, Dr, Dr1, Dh, Dh1, m | ||
|---|---|---|---|---|---|---|
| lb | 1 | 0.84 | 1 | 4 | 4 | 0.025 |
| ub | 5 | 2 | 15 | 10 | 10 | 0.3 |
| B0, m | B1, m | H1, m | n1 | n2 |
|---|---|---|---|---|
| 3.13 | 1.51 | 14.12 | 5 | 7 |
| Dp, mm | Dr, mm | Dh, mm | Dp1, mm | Dr1, mm | Dh1, mm |
| 89 | 68 | 54 | 45 | 38 | 28 |
| tp, mm | tr, mm | th, mm | tp1, mm | tr1, mm | th1, mm |
| 3.5 | 3.5 | 3 | 2.5 | 2.5 | 2.5 |
| B0, m | B1, m | H1, m | n1 | n2 |
|---|---|---|---|---|
| 3.11 | 1.86 | 10.82 | 4 | 5 |
| Dp, mm | Dr, mm | Dh, mm | Dp1, mm | Dr1, mm | Dh1, mm |
| 76 | 68 | 54 | 50 | 54 | 32 |
| tp, mm | tr, mm | th, mm | tp1, mm | tr1, mm | th1, mm |
| 3 | 3 | 3 | 3 | 3 | 2.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chepurnenko, A.; Akhtyamova, L.; Ivashchenko, I.; Akopyan, V. Trihedral Lattice Towers Optimization with a Limitation on the Resonant Vortex Excitation Occurrence. Designs 2023, 7, 10. https://doi.org/10.3390/designs7010010
Chepurnenko A, Akhtyamova L, Ivashchenko I, Akopyan V. Trihedral Lattice Towers Optimization with a Limitation on the Resonant Vortex Excitation Occurrence. Designs. 2023; 7(1):10. https://doi.org/10.3390/designs7010010
Chicago/Turabian StyleChepurnenko, Anton, Leisan Akhtyamova, Irina Ivashchenko, and Vladimir Akopyan. 2023. "Trihedral Lattice Towers Optimization with a Limitation on the Resonant Vortex Excitation Occurrence" Designs 7, no. 1: 10. https://doi.org/10.3390/designs7010010
APA StyleChepurnenko, A., Akhtyamova, L., Ivashchenko, I., & Akopyan, V. (2023). Trihedral Lattice Towers Optimization with a Limitation on the Resonant Vortex Excitation Occurrence. Designs, 7(1), 10. https://doi.org/10.3390/designs7010010






