Performance Evaluation and Dimensional Analysis of Multistage Helicoaxial Pump for Two-Phase Flow
Abstract
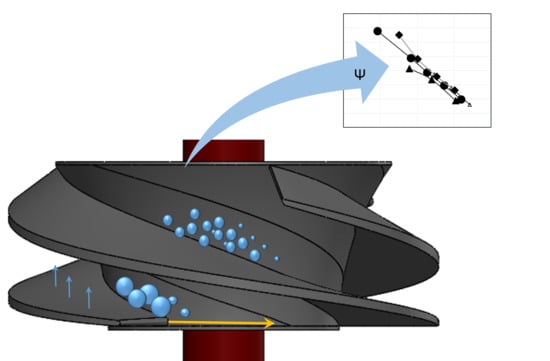
:1. Introduction
2. Experimental Setup and Performance Parameters
3. Results and Discussion
Standard Affinity Laws Using Homogeneous Model Applied to Helico-Axial Pump
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| BEP | Best Efficiency Point |
| BFSL | Best fit straight line |
| CFD | Computational Fluid Dynamics |
| Ds | Impeller outer diameter, m |
| ESP | Electrical Submersible Pump |
| g | Gravitational acceleration, m/s2 |
| GVF, α | Gas Void Fraction |
| N | rotational speed, rpm |
| Ns | Specific speed [US] |
| p | Pressure, bar |
| P | Power, kW |
| Q | Volumetric flow rate, m3/hr |
| H | Pump head |
| η | Efficiency |
| Torque, N.m | |
| μ | Dynamic viscosity, mPa.s |
| Φ | Flow rate coefficient |
| Power input coefficient | |
| Ψ | Head coefficient |
| ρ | Fluid density, kg/m3 |
| ω | Angular speed, rad/s |
Appendix A
| Flow Meter | Model No | Range | Accuracy (of Reading) |
|---|---|---|---|
| Water | Turbines Inc WM0600x6 | 56.8–567.8 m3/h | ±1% |
| Air | Omega-FTB-938 | 13.6–220.9 (Actual m3/h) * | ±1% |
| Water | Omega-FTB-1431 | 3.4–40.9 m3/h | ±1% |
| Air | Omega-FTB-933 | 1.7–17 (Actual m3/h) * | ±1% |
| Transducer Type | Range (Bar) | Accuracy |
|---|---|---|
| Omega-PX 429-500 GI | 0–34.5 | ±0.08% |
| Omega-PX 429-750 GI | 0–51.7 | ±0.08% |
| Omega-PX481A-1000G5V | 0–69 | 0.3% |
| Outcomes | Uncertainty Interval |
|---|---|
| Efficiency | ±1.06% |
| Power input | ±1.04 kW |
| Head Output | ±3 m |
References
- Turpin, J.L.; Lea, J.F.; Bearden, J.L. Gas-Liquid Flow Through Centrifugal Pumps-Correlation of Data. In Proceedings of the Third International Pump Symposiun, Claremore, OK, USA, 1 January 1986; pp. 13–20. [Google Scholar]
- Cirilo, R. Air-Water Flow Through Electrical Submersible Pumps; The University of Tulsa: Tulsa, OK, USA, 1998. [Google Scholar]
- Romero, M. An Evaluation of An Electric Submersible Pumping System for High GOR Wells; University of Tulsa: Tulsa, OK, USA, 1999. [Google Scholar]
- Pessoa, R. Experimental Investigation of Two-Phase Flow Performance of Electrical Submersible Pump Stages; Society of Petroleum Engineers: New Orleans, LA, USA, 2000. [Google Scholar]
- Duran, J.; Prado, M. ESP Stages Air-Water Two-Phase Performance Modeling and Experimental Data; Society of Petroleum Engineers: Richardson, TX, USA, 2003. [Google Scholar]
- Zhou, D.; Sachdeva, R. Simple model of electric submersible pump in gassy well. J. Pet. Sci. Eng. 2010, 70, 204–213. [Google Scholar] [CrossRef]
- Lea, J.F.; Bearden, J.L. Effect of Gaseous Fluids on Submersible Pump Performance. J. Pet. Technol. 1982, 34, 2922–2930. [Google Scholar] [CrossRef]
- Pirouzpanah, S.; Gudigopuram, S.R.; Morrison, G.L. Two-phase flow characterization in a split vane impeller Electrical Submersible Pump. J. Pet. Sci. Eng. 2017, 148, 82–93. [Google Scholar] [CrossRef]
- Gié, P.; Buvat, P.; Bratu, C.; Durando, P. Poseidon Multiphase Pump: Field Tests Results. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 4–7 May 1992; p. 14. [Google Scholar]
- Zhang, J.; Cai, S.; Li, Y.; . Zhu, H.; Zhang, Y. Visualization study of gas–liquid two-phase flow patterns inside a three-stage rotodynamic multiphase pump. Exp. Therm. Fluid Sci. 2016, 70. [Google Scholar] [CrossRef]
- Kuchpil, C.; Souza, E.M.; Cerqueira, M.B.; Coelho, E.J.J.; Carbone, L.C.; Silva, L.T. Barracuda Subsea Helico-Axial Multiphase Pump Project. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 6–9 May 2013. [Google Scholar]
- Charron, Y.; Pagnier, P.; Marchetta, E.; Stihle, S. Multiphase Flow Helico-Axial Turbine: Applications and Performance. In Proceedings of the Abu Dhabi International Conference and Exhibition; Society of Petroleum Engineers: Abu Dhabi, United Arab Emirates, 2004; p. 8. [Google Scholar]
- Hua, G.; Falcone, G.; Catalin, T.; Morrison, G. Comparison of Multiphase Pumping Technologies for Subsea and Downhole Applications. Oil Gas Facil. 2012, 1, 36–46. [Google Scholar] [CrossRef]
- Rojas, M.; Barrios, L.; Harris, G.; Cheah, K.W. Full-Scale Investigation of Gas-Handling Capabilities of High-Flow Helicoaxial ESP Stages for Deepwater Application. In SPE Electric Submersible Pump Symposium; Society of Petroleum Engineers: The Woodlands, TX, USA, 2017; p. 14. [Google Scholar]
- Simpson, A.; Rhys-Davies, J.; Husman, M.; Youri, E. A Tough, Truly Multiphase Downhole Pump for Unconventional Wells. In SPE Electric Submersible Pump Symposium; Society of Petroleum Engineers: The Woodlands, TX, USA, 2017; p. 20. [Google Scholar]
- Shang, S.; Bustamante, N.G. Understanding ESP Performance in Multiphase Flow Condition in SAGD Production Wells. In Abu Dhabi International Petroleum Exhibition Conference; Society of Petroleum Engineers: Abu Dhabi, UAE, 2017; p. 20. [Google Scholar]
- Xu, Y.; Cao, S.; Sano, T.; Wakai, T. Experimental Investigation on Transient Pressure Characteristics in a Helico-Axial Multiphase Pump. Energies 2019, 12, 461. [Google Scholar] [CrossRef]
- Zhang, J.; Tan, L. Energy Performance and Pressure Fluctuation of a Multiphase Pump with Different Gas Volume Fractions. Energies 2018, 11, 1216. [Google Scholar] [CrossRef]
- Suh, J.W.; Kim, J.W.; Choi, Y.S.; Kim, J.H.; Joo, W.G.; Lee, K.Y. Multi-Objective Optimization of the Hydrodynamic Performance of the Second Stage of a Multi-Phase Pump. Energies 2017, 10, 1334. [Google Scholar] [CrossRef]
- Gulich, J.F. Centrifugal Pumps, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Kline, S.J.; McClintock, F.A. Describing the uncertainity in single sample experiments. Mech. Eng. 1953, 3–8. [Google Scholar] [CrossRef]
- Buckingham, E. On Physically Similar Systems; Illustrations of the Use of Dimensional Equations. Phys. Rev. 1914, 4, 345–376. [Google Scholar] [CrossRef]
- Patil, A.; Sundar, S.; Wang, Y.; Solom, M.; Kirkland, K.V.; Morrison, G. Chatacterization of Steam Impulse Turbine for Two-Phase Flow. Int. J. Heat Fluid Flow 2019, 79, 108439. [Google Scholar]
- Morrison, G.; Wenjie, Y.; Rahul, A.; Abhay, P. Development of Modified Affinity Law for Centrifugal Pump to Predict the Effect of Viscosity. J. Energy Res. Technol. 2018, 140, 092005. [Google Scholar] [CrossRef]
- Patil, A.; Morrison, G. Affinity Law Modified to Predict the Pump Head Performance for Different Viscosities Using the Morrison Number. J. Fluids Eng. 2018, 141, 021203. [Google Scholar] [CrossRef]
- Patil, A.; Yin, W.; Agarwal, R.; Delgado, A.; Morrison, G. Extending Classical Friction Loss Modeling to Predict the Viscous Performance of Pumping Devices. J. Fluids Eng. 2019, 141, 101202. [Google Scholar] [CrossRef]












| Rotational Speed (rpm) | Liquid Viscosities (mPa-s) | Gas Volume Fraction (%) |
|---|---|---|
| 3000, 3600 | 1, 5 | 0, 5, 6, 7, 10, 15, 20, 25, 30, 35, 40, 45, 50 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Patil, A.; Gudigopuram, S.; Ayyildiz, B.; Delgado, A.; Morrison, G. Performance Evaluation and Dimensional Analysis of Multistage Helicoaxial Pump for Two-Phase Flow. Int. J. Turbomach. Propuls. Power 2019, 4, 22. https://doi.org/10.3390/ijtpp4030022
Patil A, Gudigopuram S, Ayyildiz B, Delgado A, Morrison G. Performance Evaluation and Dimensional Analysis of Multistage Helicoaxial Pump for Two-Phase Flow. International Journal of Turbomachinery, Propulsion and Power. 2019; 4(3):22. https://doi.org/10.3390/ijtpp4030022
Chicago/Turabian StylePatil, Abhay, Sujan Gudigopuram, Burak Ayyildiz, Adolfo Delgado, and Gerald Morrison. 2019. "Performance Evaluation and Dimensional Analysis of Multistage Helicoaxial Pump for Two-Phase Flow" International Journal of Turbomachinery, Propulsion and Power 4, no. 3: 22. https://doi.org/10.3390/ijtpp4030022
APA StylePatil, A., Gudigopuram, S., Ayyildiz, B., Delgado, A., & Morrison, G. (2019). Performance Evaluation and Dimensional Analysis of Multistage Helicoaxial Pump for Two-Phase Flow. International Journal of Turbomachinery, Propulsion and Power, 4(3), 22. https://doi.org/10.3390/ijtpp4030022