Numerical Investigation of the Performance Impact of Stator Tilting Endwall Designs on a Mixed Flow Turbine
Abstract
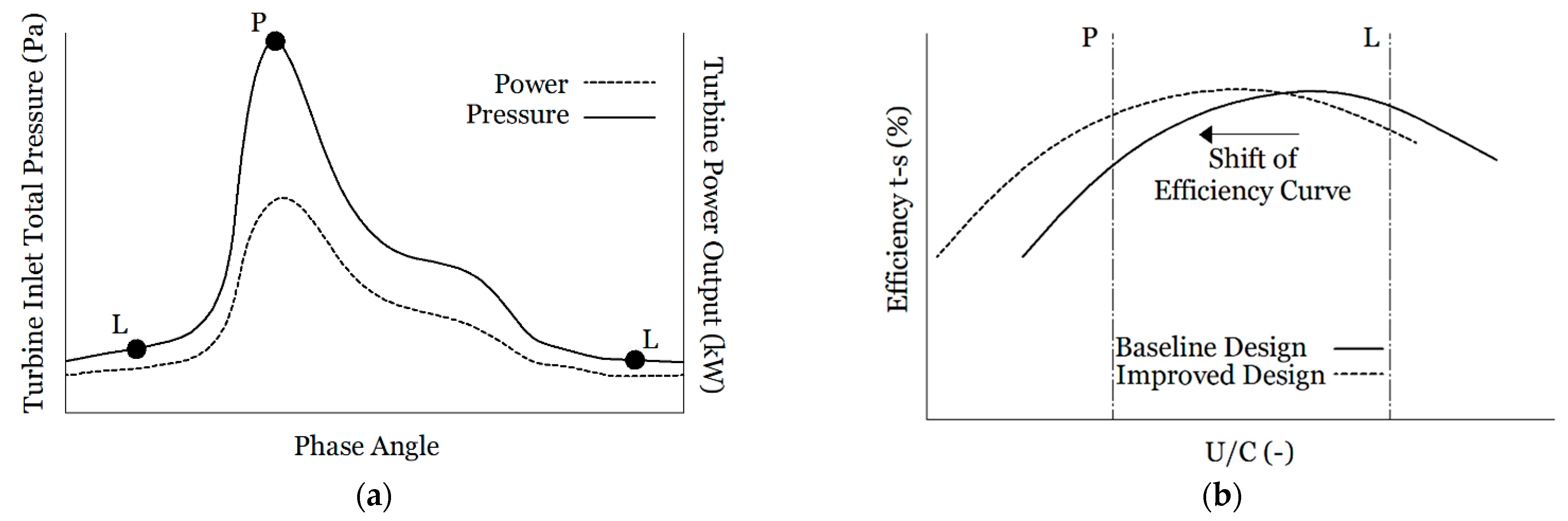
:1. Introduction
2. Materials and Methods
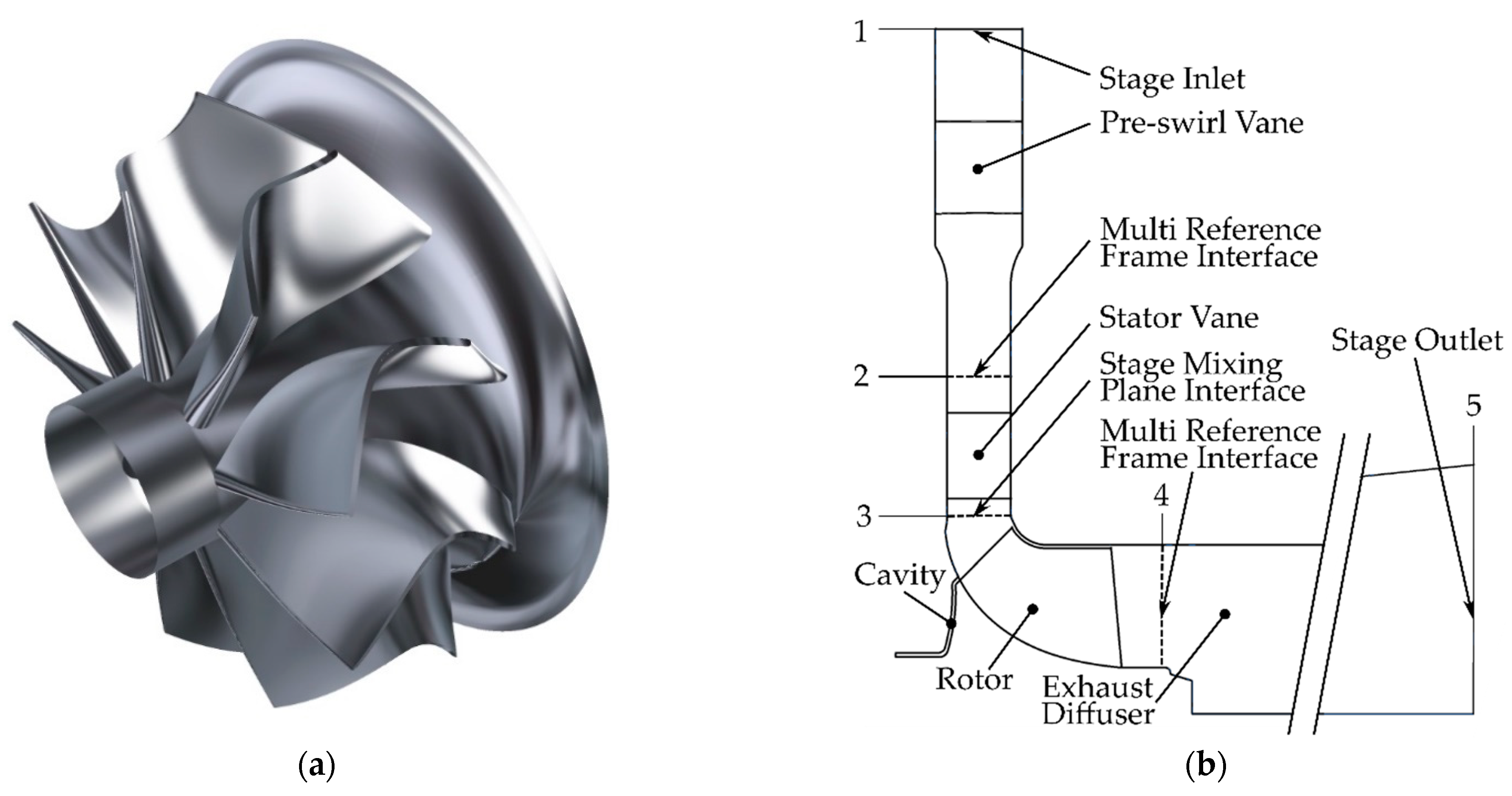
2.1. Baseline Turbine Stage
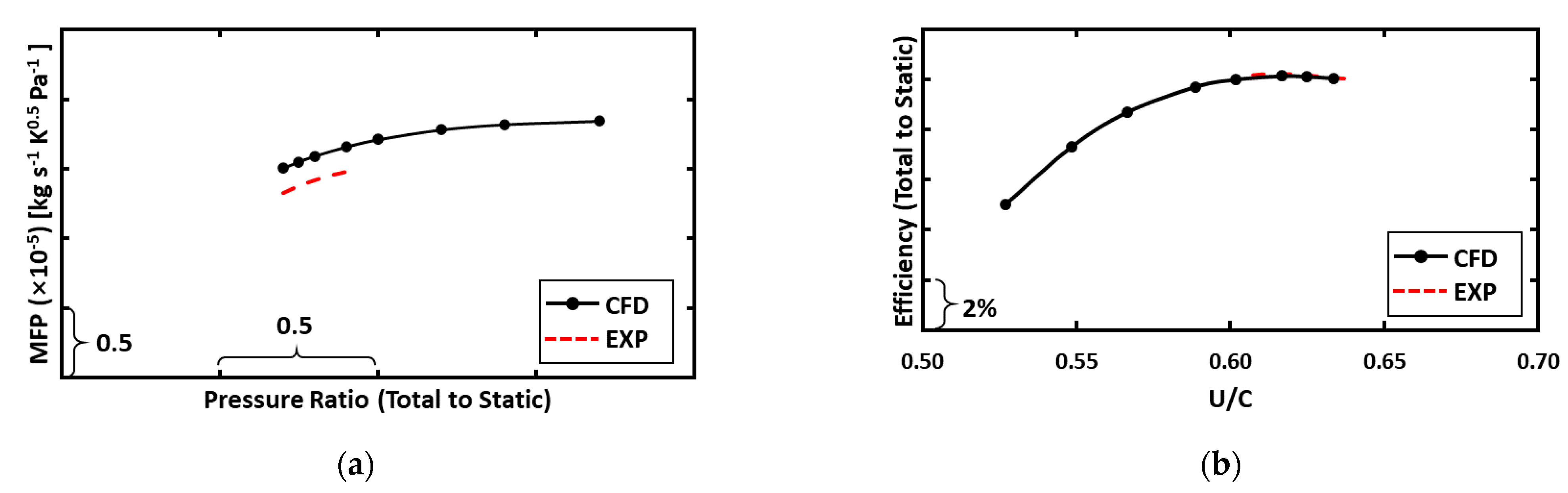
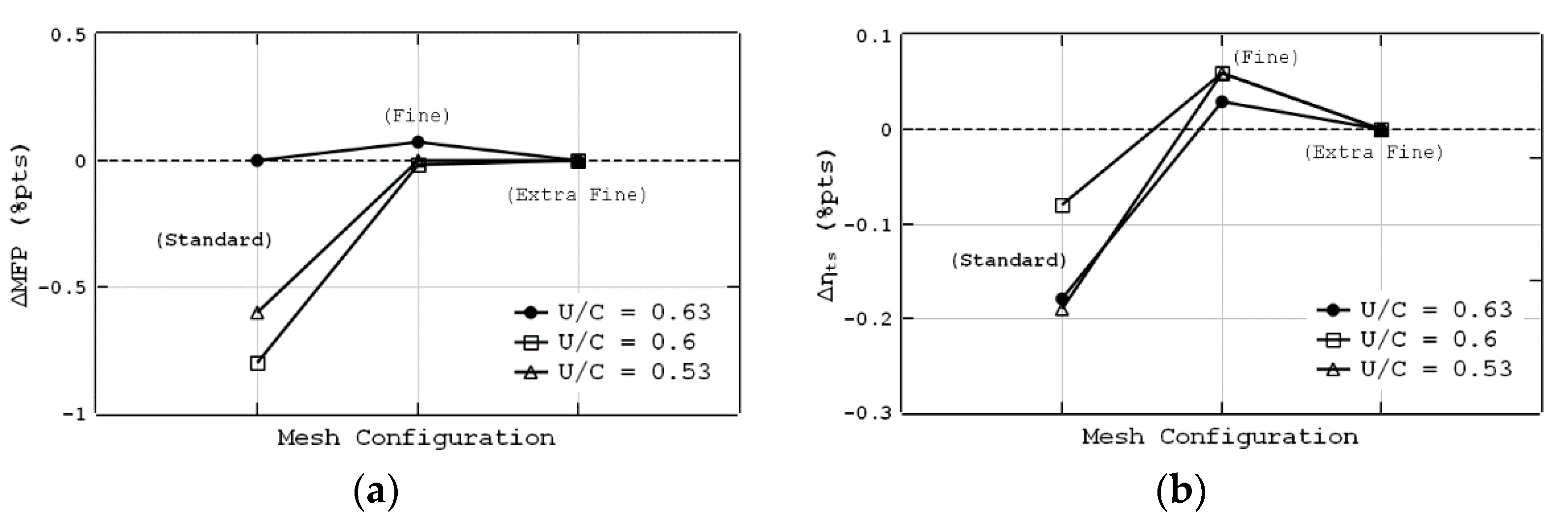
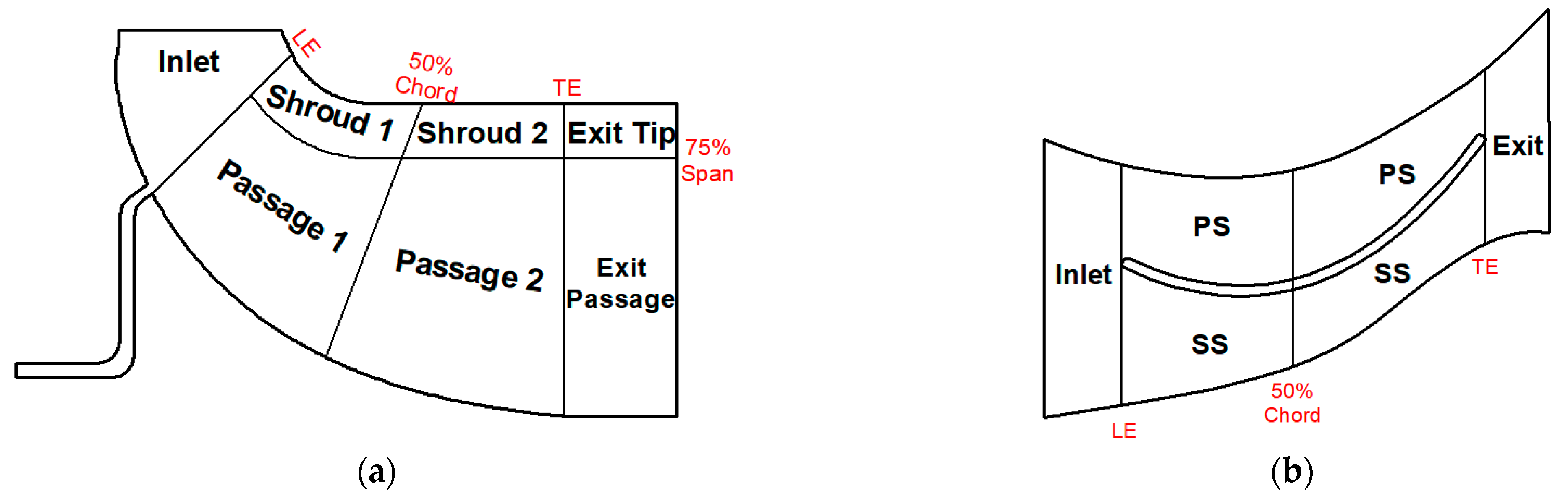
2.2. CFD Modeling Method and Validation
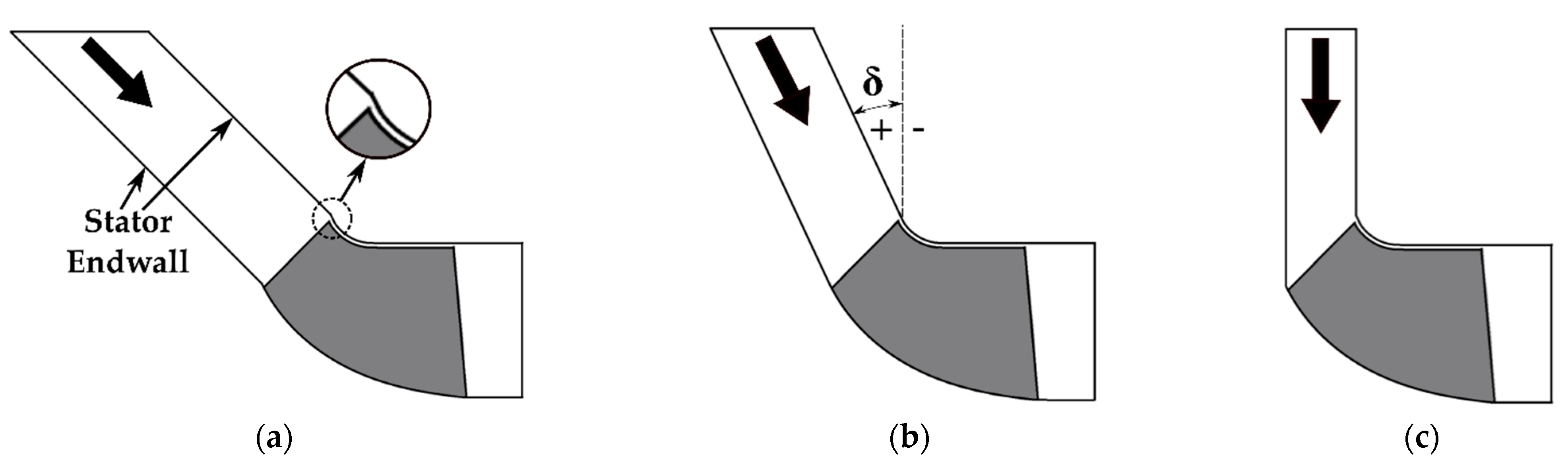
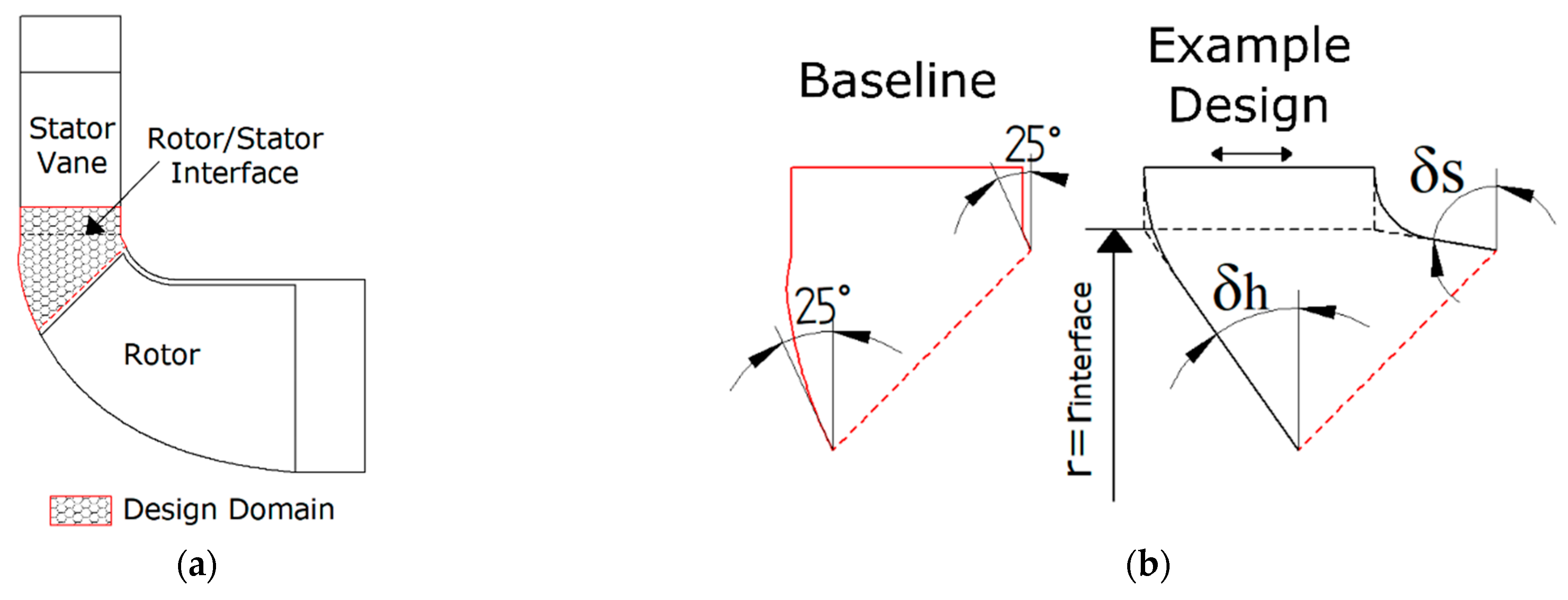
2.3. Design of Stator Tilting Endwall
3. Results and Discussions
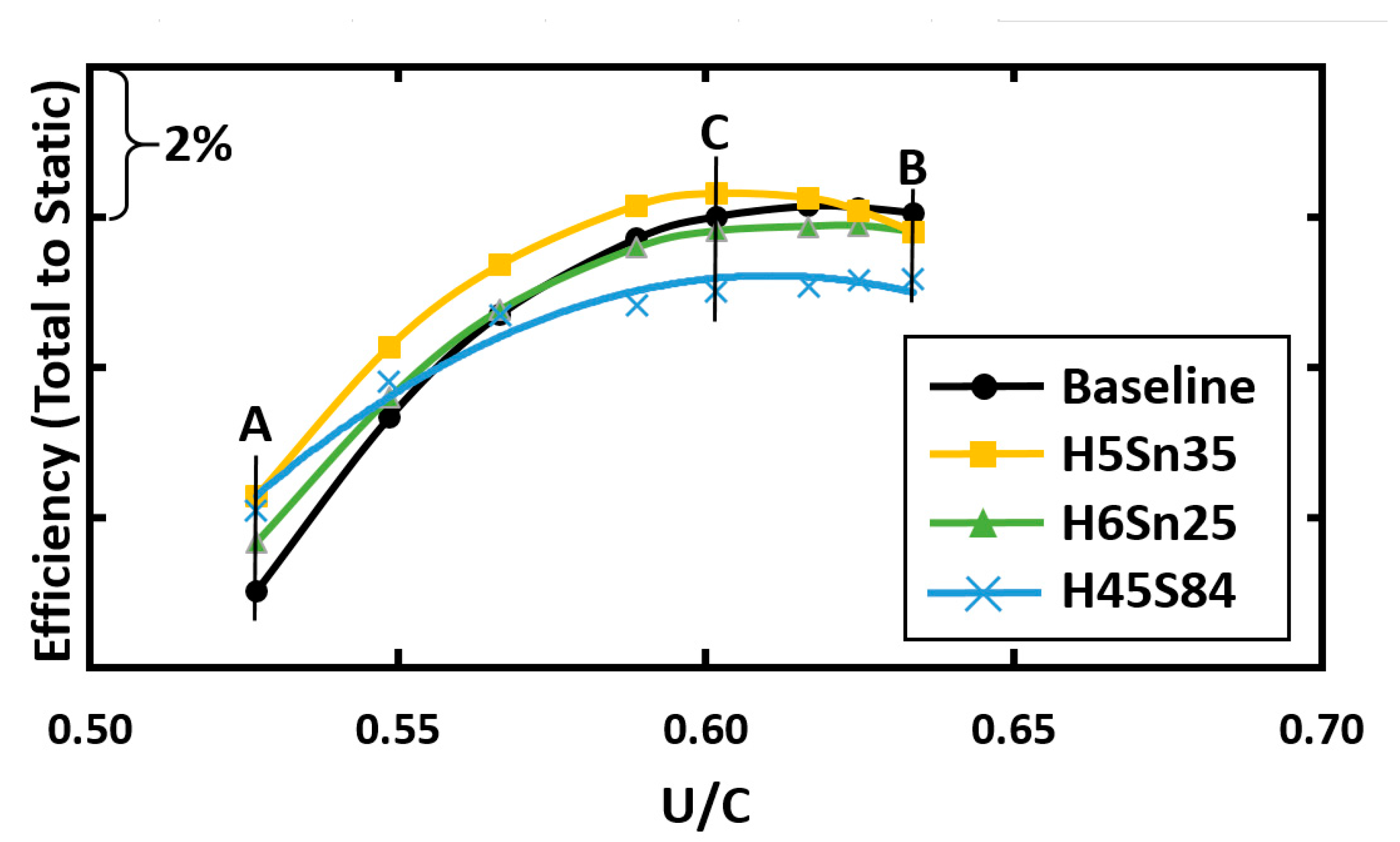
3.1. General Performance
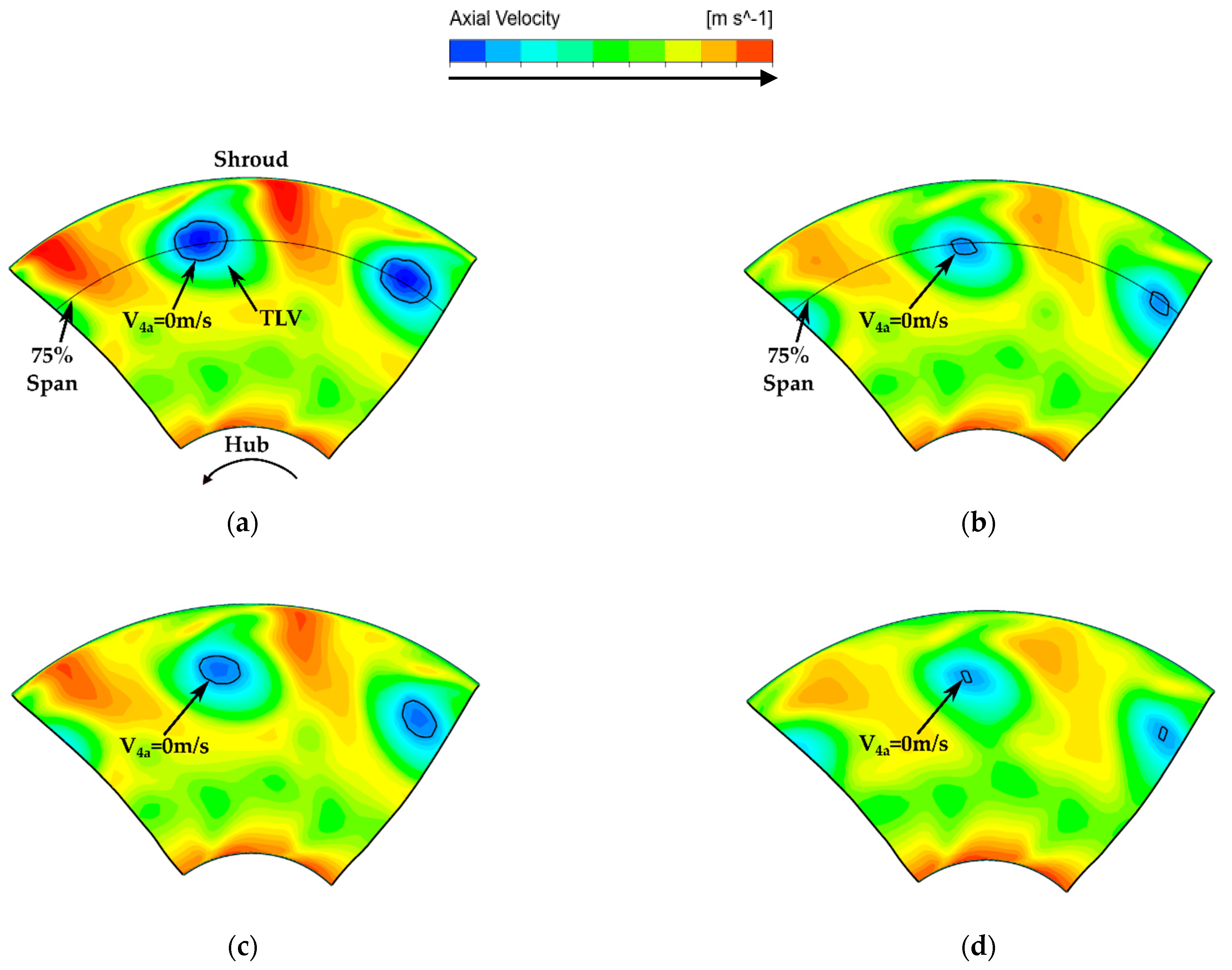
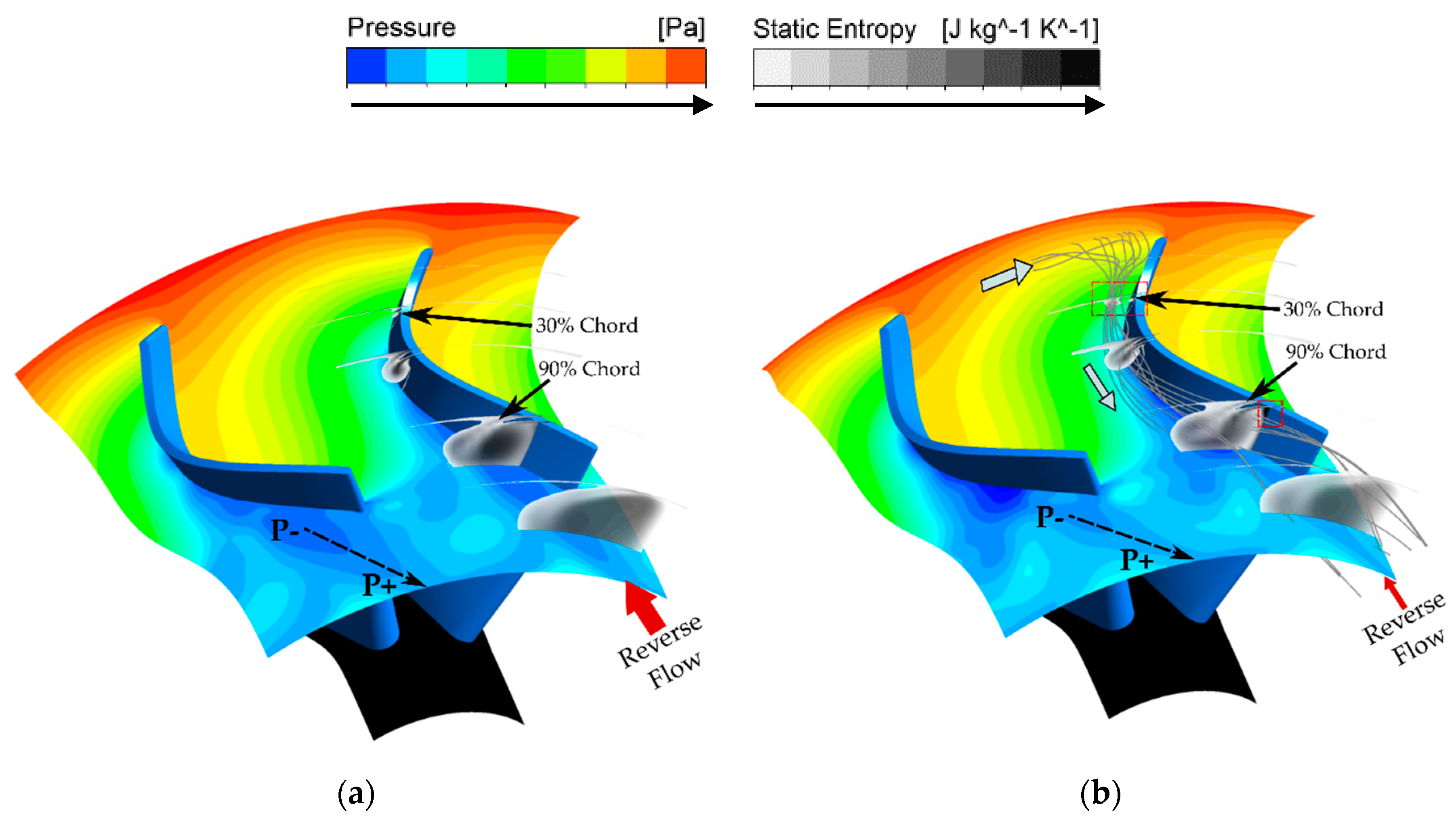
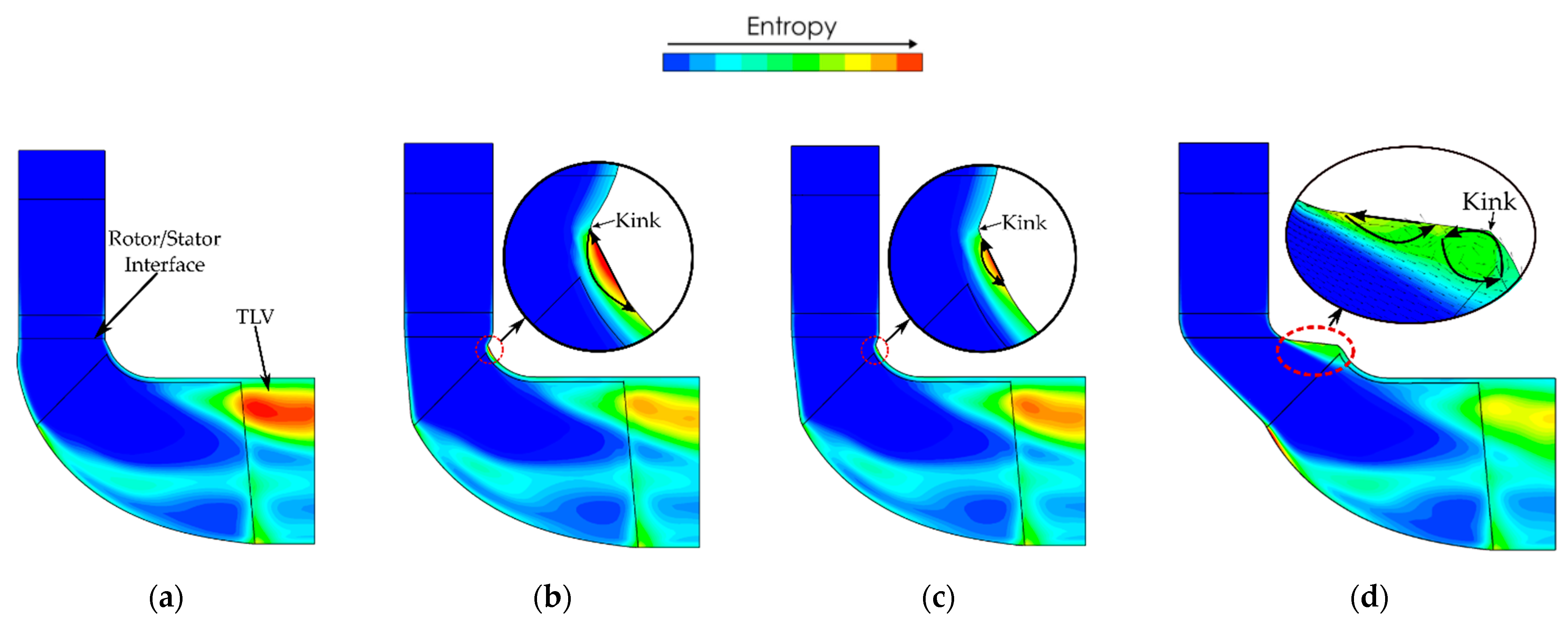
3.2. Analysis at Point A
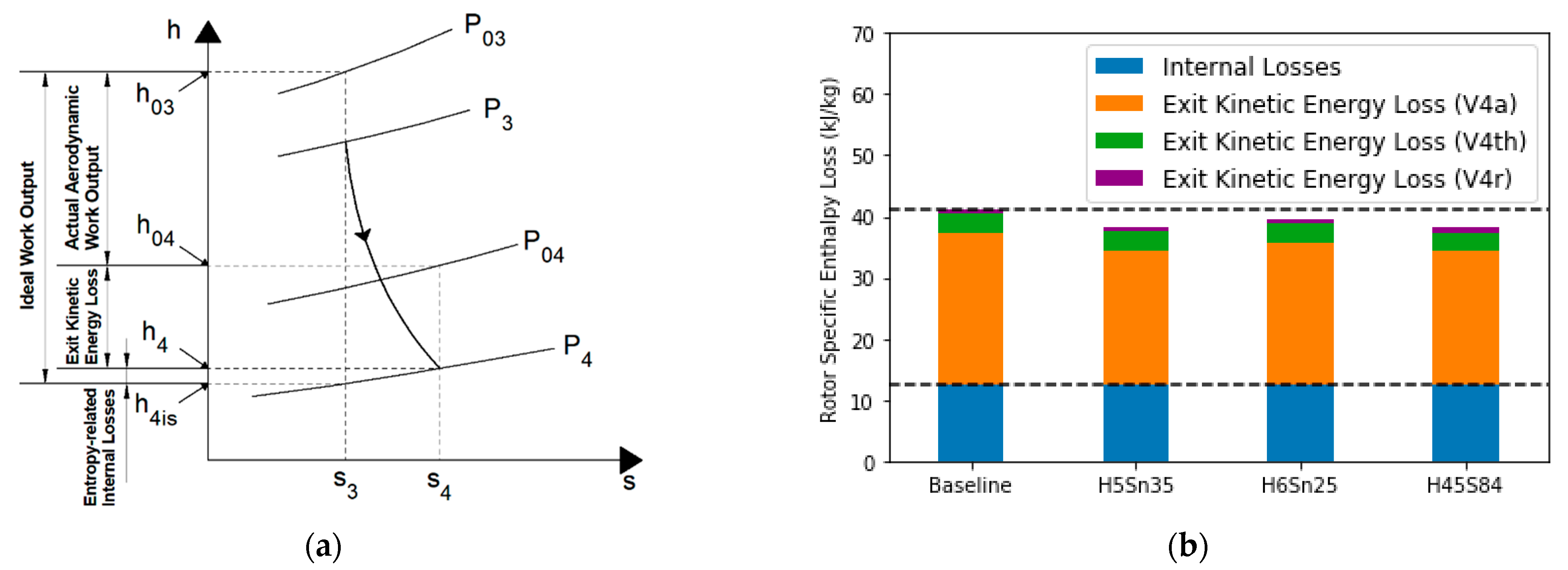
3.2.1. Loss Breakdown
3.2.2. Flow Field Analysis
3.3. Analysis of Points B and C
3.4. Preliminary Results without an Exhaust Diffuser
3.5. Preliminary Results of Unsteady CFD Simulations
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Variables | |
| δ | stator endwall tilting angle |
| ε | diffuser effectiveness |
| η | efficiency |
| ω | rotational speed |
| h | specific enthalpy |
| K | total pressure loss coefficient |
| k | specific heat ratio |
| P | pressure |
| m | mass flow |
| r | radius |
| s | specific entropy |
| T | temperature |
| U | rotor tip speed based on mean radius |
| V | velocity |
| Subscripts | |
| 0 | total state |
| 1 | stage inlet, pre-swirl vane domain inlet |
| 2 | pre-swirl vane domain outlet, stator domain inlet |
| 3 | stator domain outlet, rotor domain inlet |
| 4 | rotor domain outlet, exhaust diffuser domain inlet |
| 5 | stage outlet, exhaust diffuser domain outlet |
| a | axial |
| diffuser | exhaust diffuser domain |
| h | hub |
| in | domain inlet |
| is | isentropic |
| interface | rotor/stator interface |
| out | domain outlet |
| preswirl | pre-swirl vane domain |
| r | reduced |
| rotor | rotor domain |
| s | shroud |
| stage | turbine stage |
| stator | stator domain |
| ts | total to static |
| Abbreviation | |
| CFD | computational fluid dynamics |
| CFV | cavity flow vortex |
| EXP | experimental |
| LE | leading edge |
| MFP | mass flow parameter |
| MFT | mixed flow turbine |
| pts | points |
| PS | pressure side |
| QUB | Queen’s University Belfast |
| RFT | radial flow turbine |
| RMS | root-mean-square |
| SS | suction side |
| SST | shear stress transport |
| TLV | tip leakage vortex |
| TE | trailing edge |
| U/C | blade speed to isentropic jet velocity ratio |
References
- Leonard, T.; Spence, S.; Starke, A.; Filsinger, D. Numerical and experimental investigation of the impact of mixed flow turbine inlet cone angle and inlet blade angle. J. Turbomach. 2019, 141. [Google Scholar] [CrossRef]
- Baines, N.C. Fundamentals of Turbocharging; Concepts NREC: White River Junction, VT, USA, 2005; Volume 1. [Google Scholar]
- Baines, N. Radial-and mixed-flow turbine options for high-boost turbochargers. In Proceedings of the 7th International Conference on Turbochargers and Turbocharging, Savoy Palace, London, 14–15 May 2002; pp. 35–44. [Google Scholar]
- Fredriksson, C.; Baines, N. The mixed flow forward swept turbine for next generation turbocharged downsized automotive engines. In Proceedings of the ASME Turbo Expo 2010: Power for Land, Sea, and Air, Glasgow, UK, 14–18 June 2010; pp. 559–563. [Google Scholar] [CrossRef]
- Chen, H.; Baines, N.C. The aerodynamic loading of radial and mixed-flow turbines. Int. J. Mech. Sci. 1994, 36, 63–79. [Google Scholar] [CrossRef]
- Moustapha, H.; Zelesky, M.F.; Baines, N.C.; Japikse, D. Axial and Radial Turbines; Concepts NREC: White River Junction, VT, USA, 2003; Volume 2. [Google Scholar]
- Kirtley, K.R.; Beach, T.A.; Rogo, C. Aeroloads and secondary flows in a transonic mixed-flow turbine stage. J. Turbomach. 1993, 115, 590–600. [Google Scholar] [CrossRef]
- Chou, C.-C.; Gibbs, C.A. The design and testing of a mixed-flow turbine for turbochargers. SAE Tech. Pap. 1989. [Google Scholar] [CrossRef]
- Rajoo, S.; Martinez-Botas, R. Mixed flow turbine research: A review. J. Turbomach. 2008, 130, 044001–044012. [Google Scholar] [CrossRef]
- Lee, S.P.; Jupp, M.L.; Nickson, A.K. The introduction of a tilted volute design for operation with a mixed flow turbine for turbocharger applications. In Proceedings of the 2016 7th International Conference on Mechanical and Aerospace Engineering (ICMAE), London, UK, 18–20 July 2016; pp. 165–170. [Google Scholar]
- Lee, S.P.; Jupp, M.L.; Nickson, A.K.; Allport, J.M. Analysis of a tilted turbine housing volute design under pulsating inlet conditions. In Proceedings of the ASME Turbo Expo 2017: Turbomachinery Technical Conference and Exposition, Charlotte, NC, USA, 26–30 June 2017. [Google Scholar] [CrossRef]
- Lee, S.P.; Barrans, S.M.; Jupp, M.L.; Nickson, A.K. Investigation into the impact of span-wise flow distribution on the performance of a mixed flow turbine. In Proceedings of the ASME Turbo Expo 2018: Turbomachinery Technical Conference and Exposition, Oslo, Norway, 11–15 June 2018. [Google Scholar] [CrossRef]
- Morrison, R.; Spence, S.; Kim, S.; Filsinger, D.; Leonard, T. Investigation of the effects of flow conditions at rotor inlet on mixed flow turbine performance for automotive applications. In Proceedings of the International Turbocharging Seminar 2016, Tianjin, China, 21–22 September 2016. [Google Scholar]
- Gao, J.; Zheng, Q.; Yue, G. Reduction of tip clearance losses in an unshrouded turbine by rotor casing contouring. J. Propuls. Power 2012, 28, 936–945. [Google Scholar] [CrossRef]
- Dambach, R.; Hodson, H.P.; Huntsman, I. 1998 turbomachinery committee best paper award: An experimental study of tip clearance flow in a radial inflow turbine. J. Turbomach. 1999, 121, 644–650. [Google Scholar] [CrossRef]
- Leonard, T.M.; Spence, S.; Early, J.; Filsinger, D. A numerical study of inlet geometry for a low inertia mixed flow turbocharger turbine. In Proceedings of the ASME Turbo Expo 2014: Turbine Technical Conference and Exposition, Dusseldorf, Germany, 16–20 June 2014. [Google Scholar] [CrossRef]
- Morrison, R.; Spence, S.; Kim, S.I.; Leonard, T.; Starke, A. Evaluating the use of leaned stator vanes to produce a non-uniform flow distribution across the inlet span of a mixed flow turbine rotor. J. Turbomach. 2020, 142. [Google Scholar] [CrossRef]
- Deng, Q.; Niu, J.; Mao, J.; Feng, Z. Experimental and numerical investigation on overall performance of a radial inflow turbine for 100kW mircoturbine. In Proceedings of the ASME Turbo Expo 2007: Power for Land, Sea, and Air, Montreal, QB, Canada, 14–17 May 2007; pp. 919–926. [Google Scholar] [CrossRef]
- Yang, B.; Newton, P.; Martinez-Botas, R.F. Understanding of secondary flows and losses in radial and mixed flow turbines. J. Turbomach. 2020, 142, 1–29. [Google Scholar] [CrossRef]
- Liu, Y.; Yang, C.; Qi, M.; Zhang, H.; Zhao, B. Shock, leakage flow and wake interactions in a radial turbine with variable guide vanes. In Proceedings of the ASME Turbo Expo 2014: Turbine Technical Conference and Exposition, Dusseldorf, Germany, 16–20 June 2014. [Google Scholar]
- Palenschat, T.; Newton, P.; Martinez-Botas, R.F.; Müller, M.; Leweux, J. 3-D computational loss analysis of an asymmetric volute twin-scroll turbocharger. In Proceedings of the ASME Turbo Expo 2017: Turbomachinery Technical Conference and Exposition, Charlotte, NC, USA, 26–30 June 2017. [Google Scholar] [CrossRef]
- Cao, T.; Xu, L.; Yang, M.; Martinez-Botas, R.F. Radial turbine rotor response to pulsating inlet flows. J. Turbomach. 2013, 136. [Google Scholar] [CrossRef]
- Simpson, A.T.; Spence, S.W.T.; Watterson, J.K. Numerical and experimental study of the performance effects of varying vaneless space and vane solidity in radial turbine stators. J. Turbomach. 2013, 135, 031001–031012. [Google Scholar] [CrossRef]
- Japikse, D.; Baines, N.C. Introduction to Turbomachinery; Concepts ETI: White River Junction, VT, USA, 1994. [Google Scholar]
- Zangeneh-Kazemi, M.; Dawes, W.N.; Hawthorne, W.R. Three dimensional flow in radial-inflow turbines. In Proceedings of the ASME 1988 International Gas Turbine and Aeroengine Congress and Exposition, Amsterdam, The Netherlands, 6–9 June 1989. [Google Scholar]
- Palfreyman, D.; Martinez-Botas, R.F. Numerical study of the internal flow field characteristics in mixed flow turbines. In Proceedings of the ASME Turbo Expo 2002: Power for Land, Sea, and Air, Amsterdam, The Netherlands, 3–6 May 2002; pp. 455–472. [Google Scholar] [CrossRef]
- Karamanis, N.; Martinez-Botas, R.F.; Su, C.C. Mixed flow turbines: Inlet and exit flow under steady and pulsating conditions. J. Turbomach. 2000, 123, 359–371. [Google Scholar] [CrossRef]
- Denton, J.D. The 1993 IGTI scholar lecture: Loss mechanisms in turbomachines. J. Turbomach. 1993, 115, 621–656. [Google Scholar] [CrossRef]
- Kock, F.; Herwig, H. Entropy production calculation for turbulent shear flows and their implementation in cfd codes. Int. J. Heat Fluid Flow 2005, 26, 672–680. [Google Scholar] [CrossRef]
- Newton, P. An Experimental and Computational Study of Pulsating Flow within a Double Entry Turbine with Different Nozzle Settings. Ph.D. Thesis, Imperial College London, London, UK, 2014. [Google Scholar]
- Elliott, M.; Spence, S.; Seiler, M.; Geron, M. Performance improvement of a mixed flow turbine using 3D blading. In Proceedings of the ASME Turbo Expo 2020: Turbomachinery Technical Conference and Exposition, Virtual Conference. 21–25 September 2020. [Google Scholar]




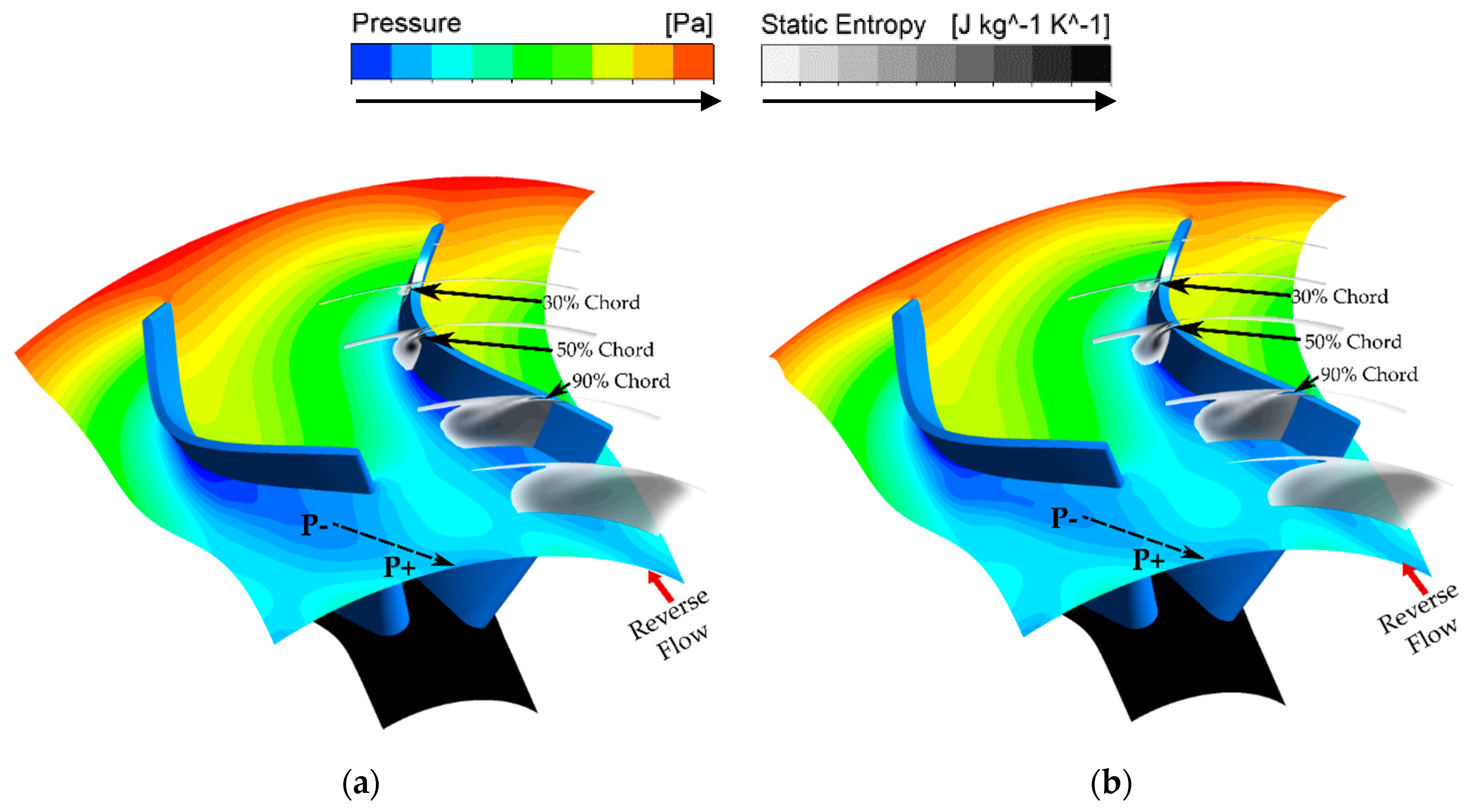
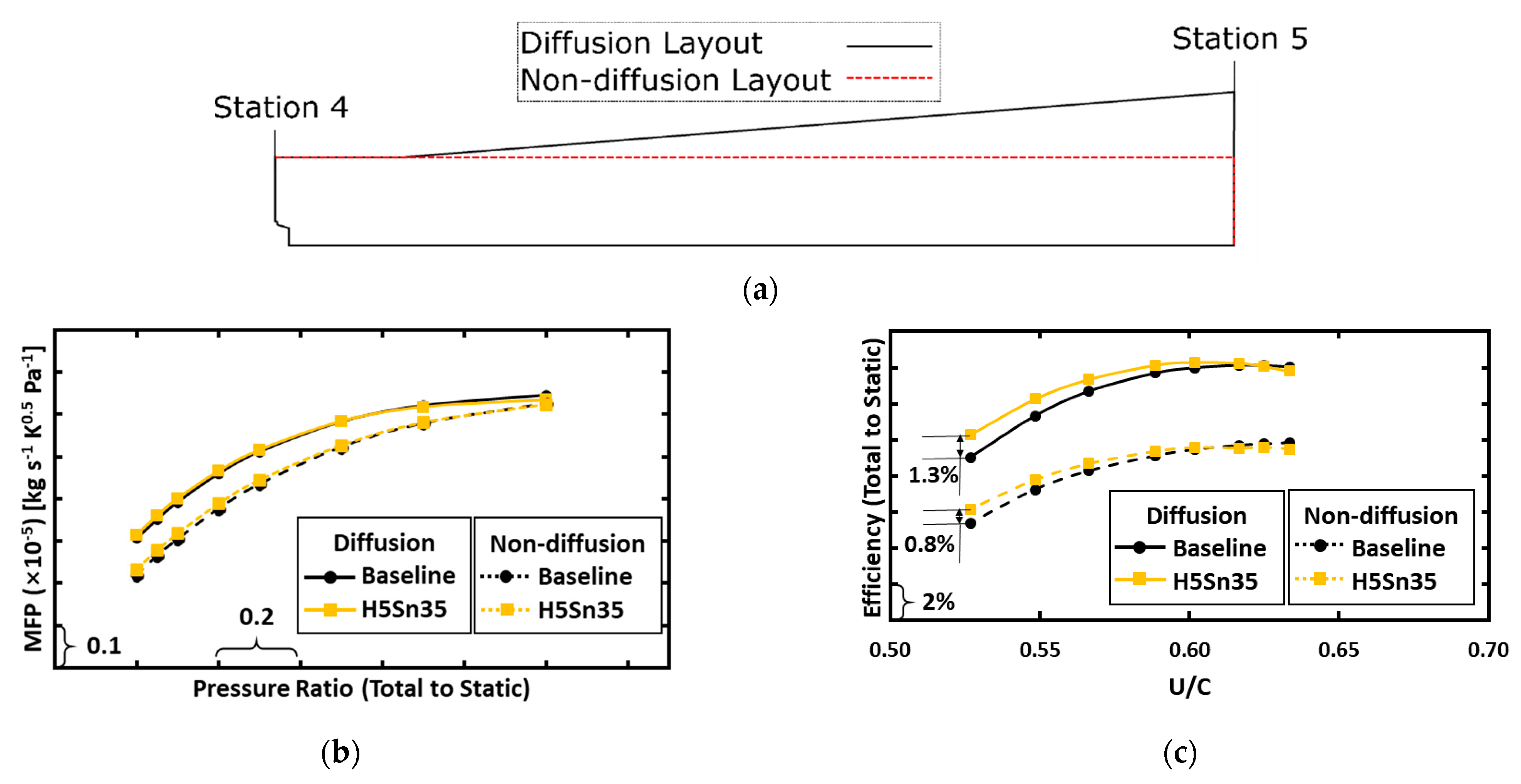

| Mesh Configuration | Pre-Swirl Vane | Stator Vane | Rotor | Cavity | Diffuser |
|---|---|---|---|---|---|
| Standard | 1 | 1 | 1 | 1 | 1 |
| Fine | 1.33 | 1.36 | 1.36 | 1.38 | 1.41 |
| Extra Fine | 1.83 | 1.84 | 1.82 | 1.90 | 1.87 |
| Design | Kpre-swirl | Kstator | Δηts,rotor | Δεdiffuser |
|---|---|---|---|---|
| Baseline | 0.039 | 0.043 | - | - |
| H5Sn35 | 0.039 | 0.042 | +2.0% | +2.2% |
| H6Sn25 | 0.039 | 0.043 | +1.05% | +1.7% |
| H45S84 | 0.041 | 0.047 | +1.96% | +1.8% |
| Design | Kpre-swirl | Kstator | Δηts,rotor | Δhexit kinetic energy (kJ/kg) | Δhinternal (kJ/kg) | Δεdiffuser |
|---|---|---|---|---|---|---|
| Baseline | 0.040 | 0.048 | - | 12.42 | 8.14 | - |
| H5Sn35 | 0.040 | 0.048 | −0.3% | 12.57 | 8.26 | −0.1% |
| Design | Kpre-swirl | Kstator | Δηts,rotor | Δhexit kinetic energy (kJ/kg) | Δhinternal (kJ/kg) | Δεdiffuser |
|---|---|---|---|---|---|---|
| Baseline | 0.040 | 0.047 | - | 14.74 | 9.41 | - |
| H5Sn35 | 0.040 | 0.045 | +0.35% | 14.20 | 9.42 | −0.9% |
| H45S84 | 0.042 | 0.041 | −1.25% | 15.75 | 9.93 | −0.4% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (http://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Gao, Y.; Fridh, J.; Morrison, R.; Ren, P.; Spence, S. Numerical Investigation of the Performance Impact of Stator Tilting Endwall Designs on a Mixed Flow Turbine. Int. J. Turbomach. Propuls. Power 2021, 6, 14. https://doi.org/10.3390/ijtpp6020014
Gao Y, Fridh J, Morrison R, Ren P, Spence S. Numerical Investigation of the Performance Impact of Stator Tilting Endwall Designs on a Mixed Flow Turbine. International Journal of Turbomachinery, Propulsion and Power. 2021; 6(2):14. https://doi.org/10.3390/ijtpp6020014
Chicago/Turabian StyleGao, Yang, Jens Fridh, Richard Morrison, Pangbo Ren, and Stephen Spence. 2021. "Numerical Investigation of the Performance Impact of Stator Tilting Endwall Designs on a Mixed Flow Turbine" International Journal of Turbomachinery, Propulsion and Power 6, no. 2: 14. https://doi.org/10.3390/ijtpp6020014
APA StyleGao, Y., Fridh, J., Morrison, R., Ren, P., & Spence, S. (2021). Numerical Investigation of the Performance Impact of Stator Tilting Endwall Designs on a Mixed Flow Turbine. International Journal of Turbomachinery, Propulsion and Power, 6(2), 14. https://doi.org/10.3390/ijtpp6020014






