State of the Art on Two-Phase Non-Miscible Liquid/Gas Flow Transport Analysis in Radial Centrifugal Pumps Part B: Review of Experimental Investigations
Abstract
:1. Introduction
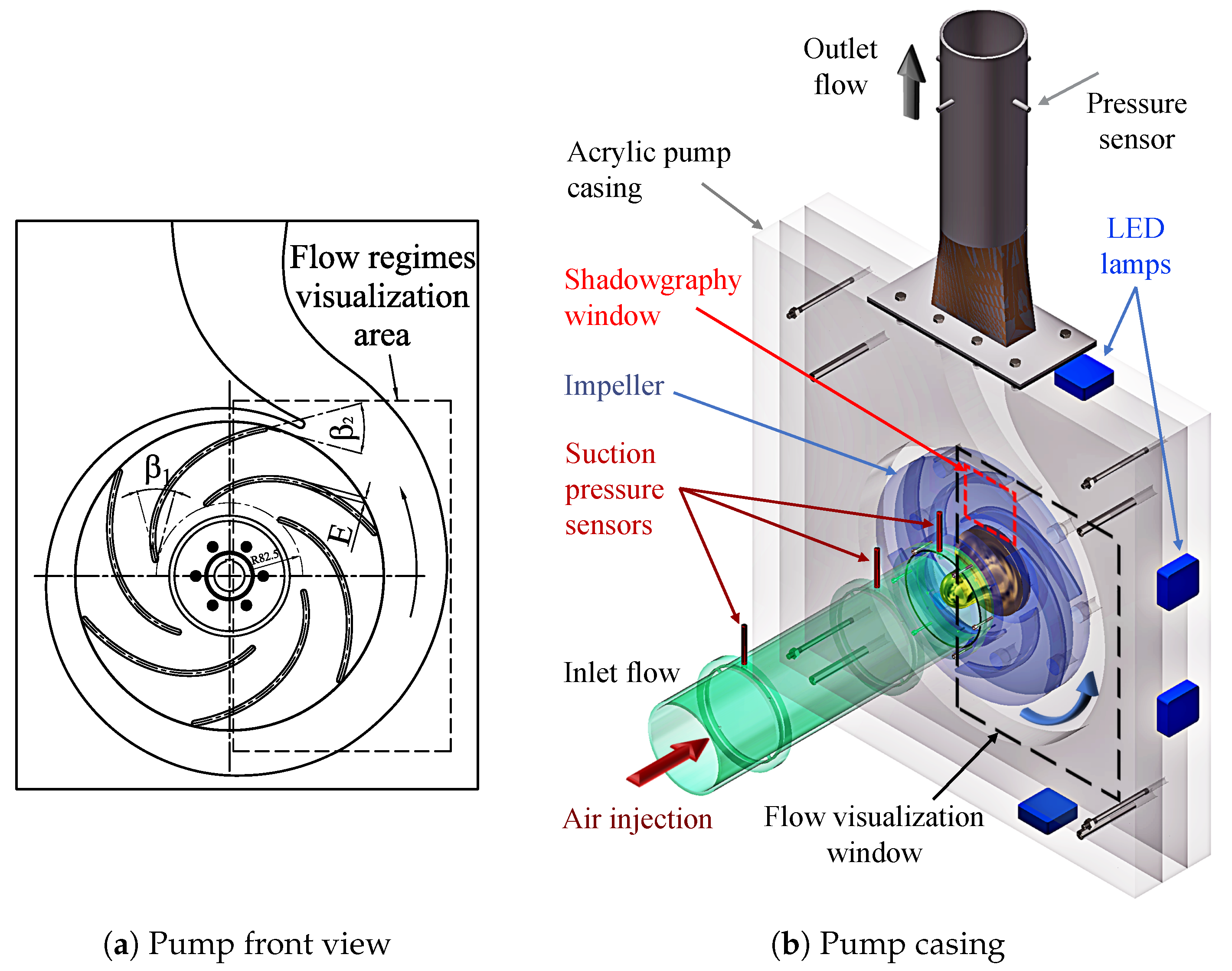
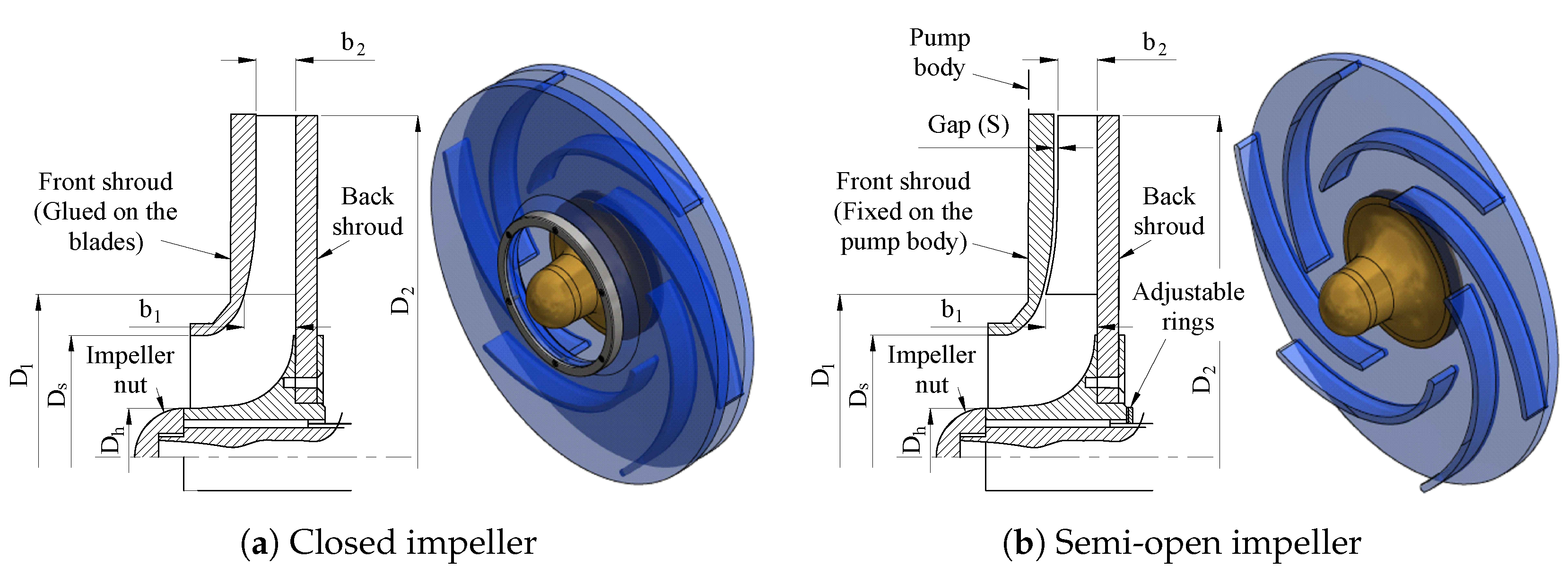
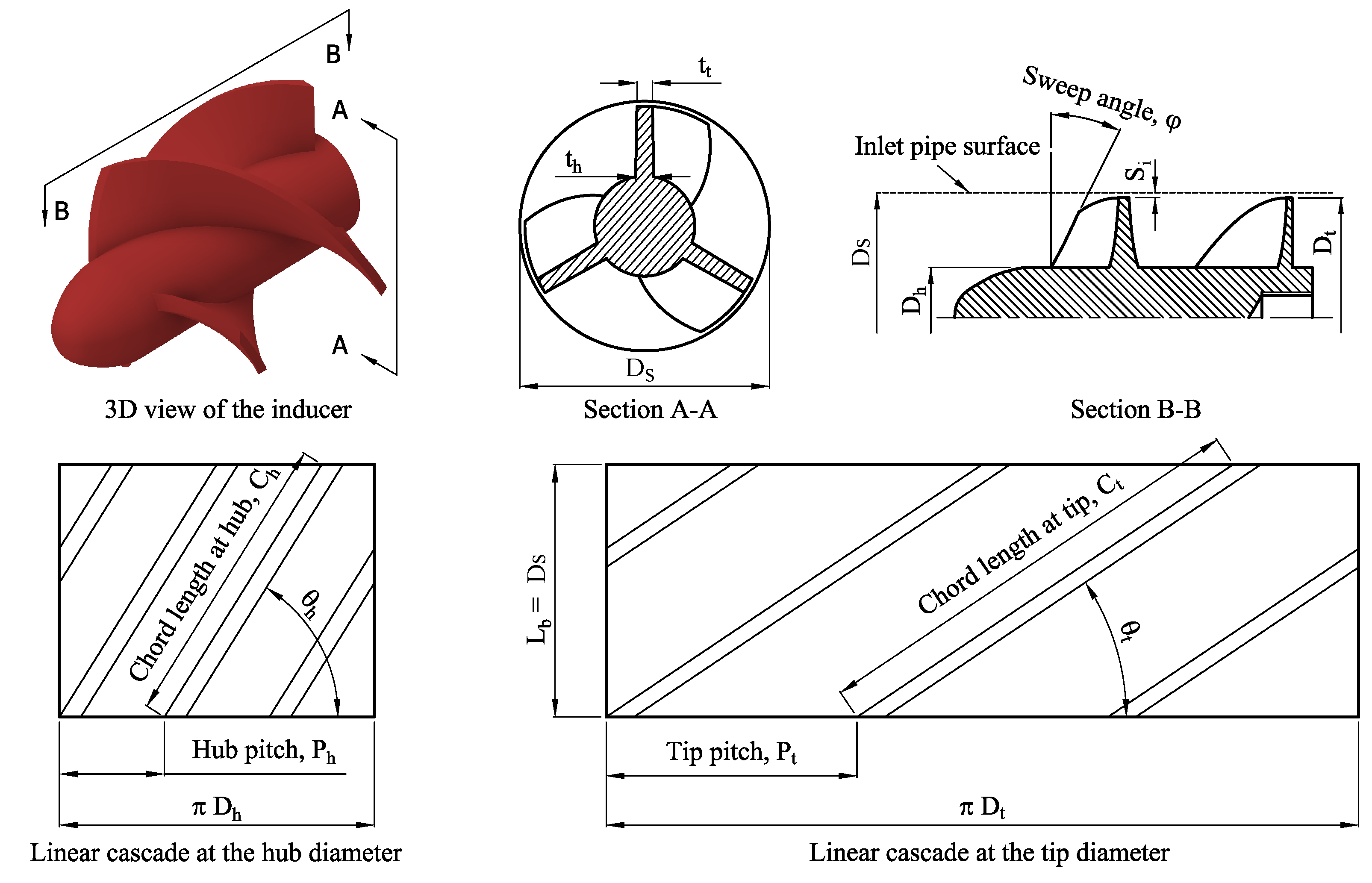
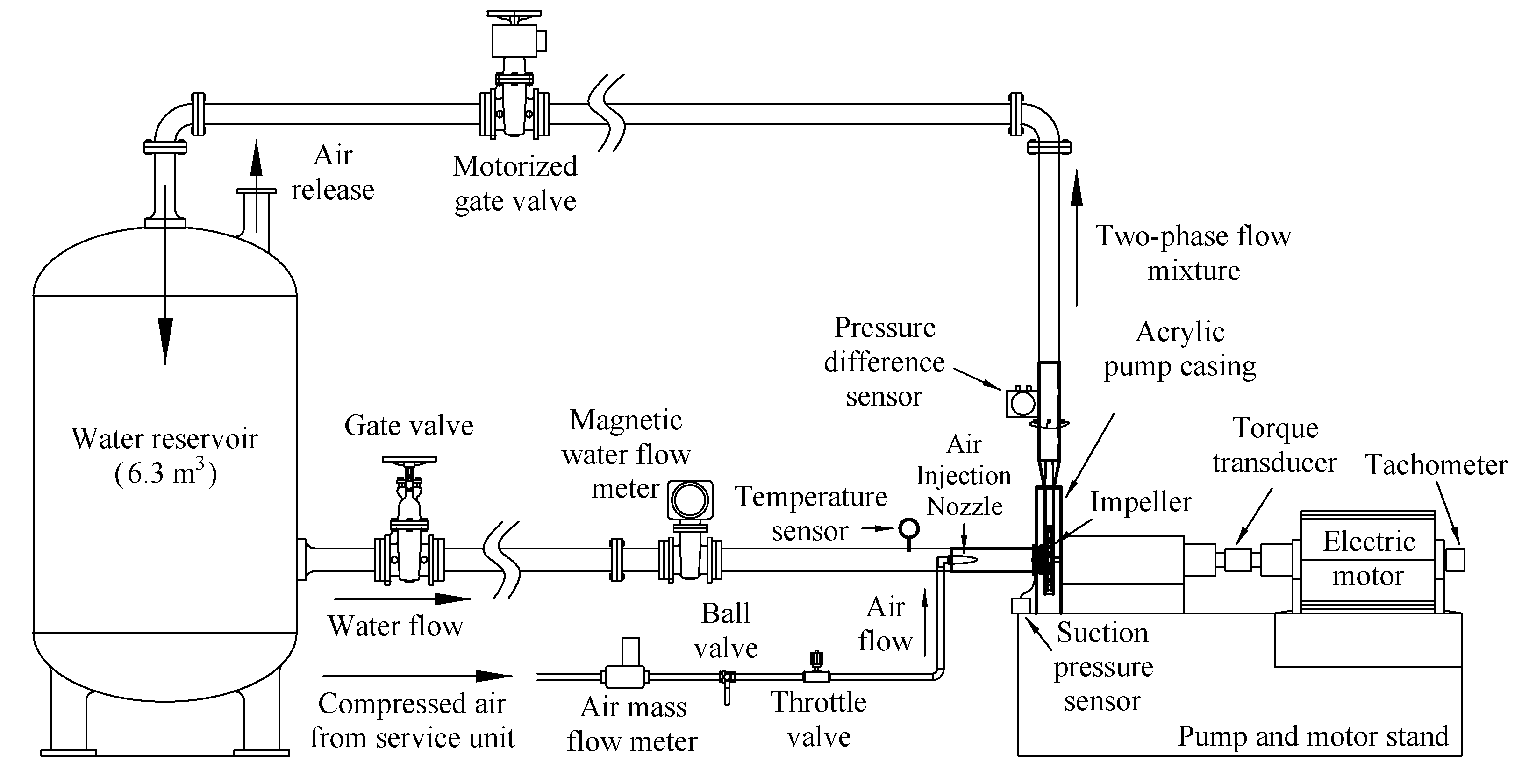
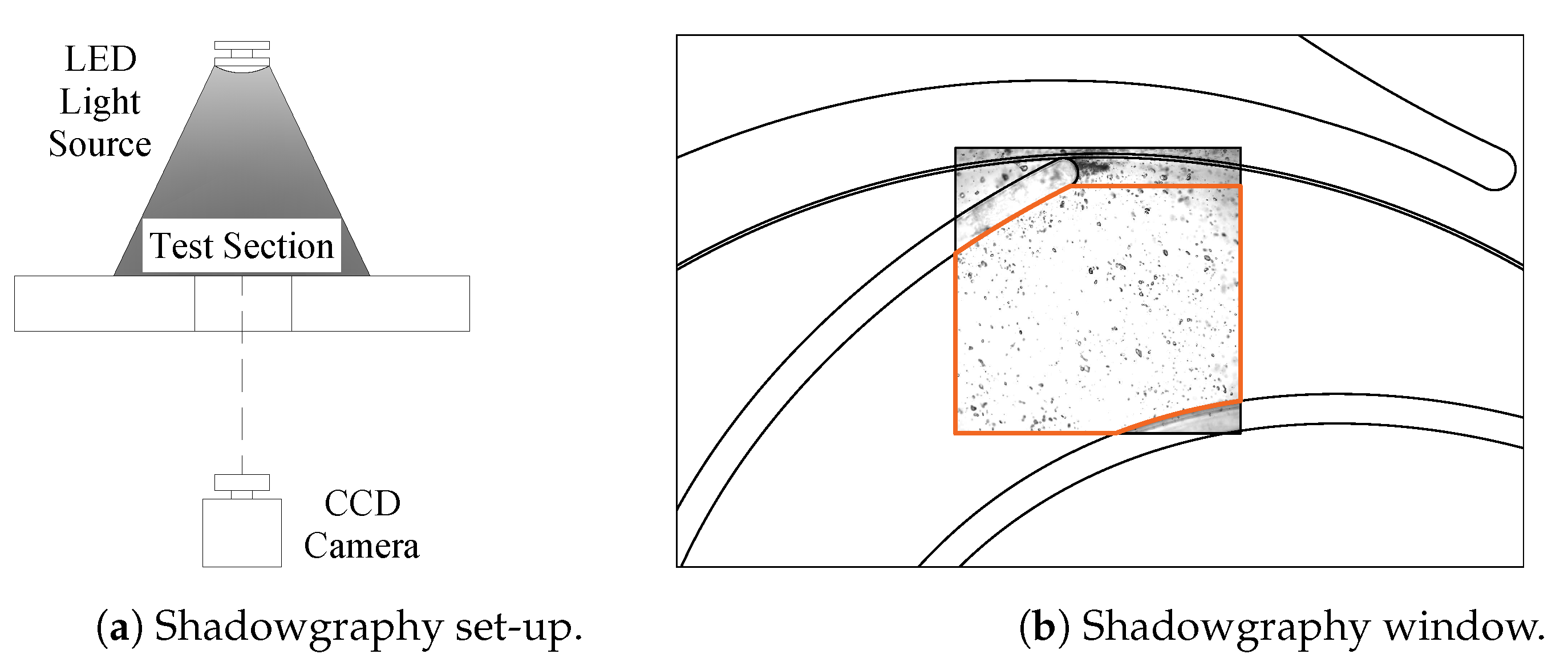
2. Experimental Set-Up
3. Pump Performance Calculations
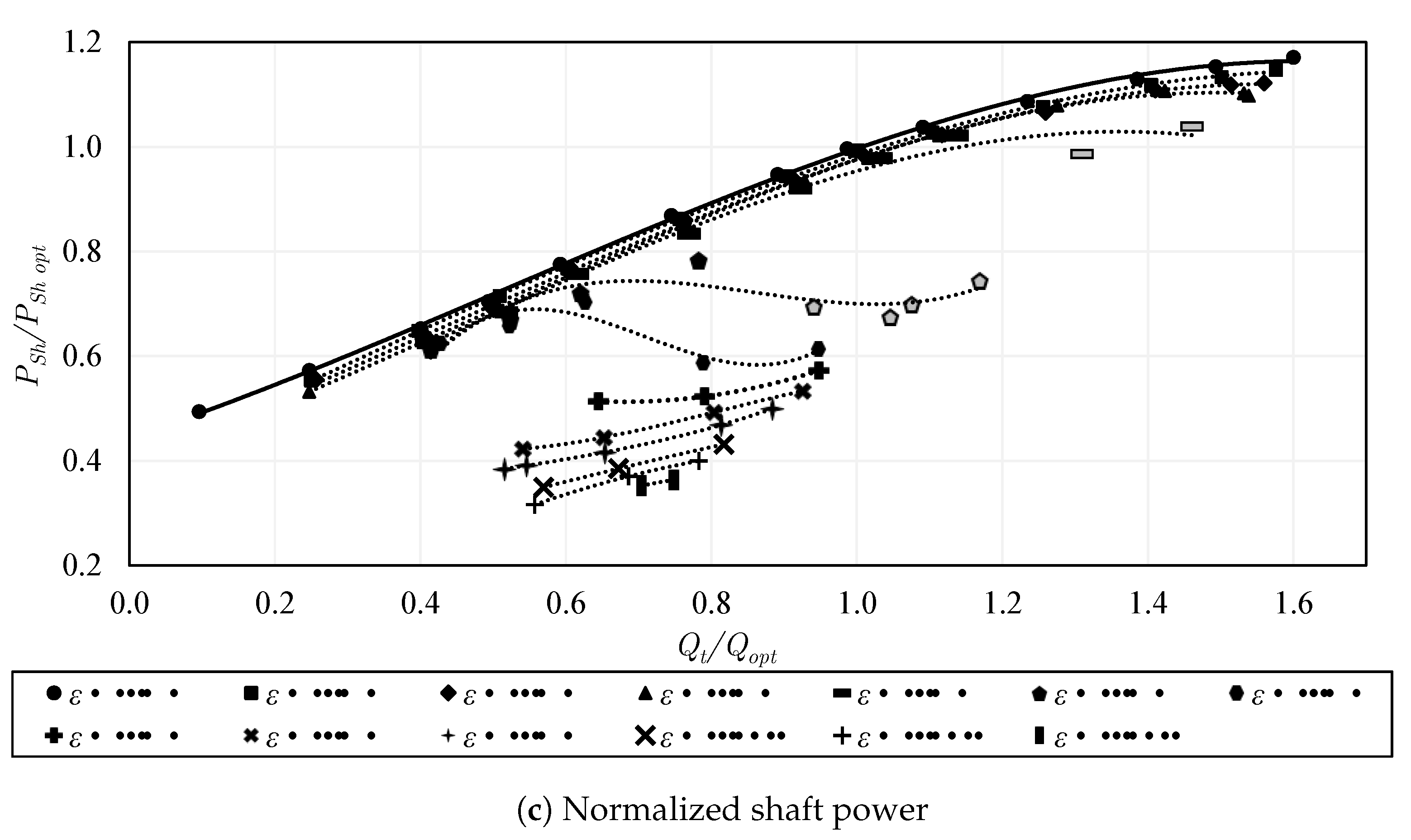
4. Measurements of Two-Phase Pumping Performance
- First measurement approach (Figure 12a).
- Full closing of the motorized gate valve.
- Progressive increasing of the water flow rate by opening the motorized valve.
- Progressive increasing of the air flow rate until reaching the desired air volume fraction and recording the measurement points after attaining a steady-state.
- Second measurement approach (Figure 12b).
- Full opening of the motorized gate valve.
- Progressive increasing of the air flow rate until reaching the desired air volume fraction of the first measurement point.
- Reducing the air flow rate to set the following measurement point.
- Reducing the water flow rate until attaining the desired gas volume fraction and recording the measurement points after attaining a steady-state.
- Third measurement approach (Figure 12c).
- Setting a single-phase water flow and keeping the water gate valve fixed.
- Progressive increasing of the air flow rate and recording the measurement points after attaining a steady-state at each air volume fraction.
- Progressive reducing of the air flow rate and recording the measurement points again after attaining a steady-state at each air volume fraction.
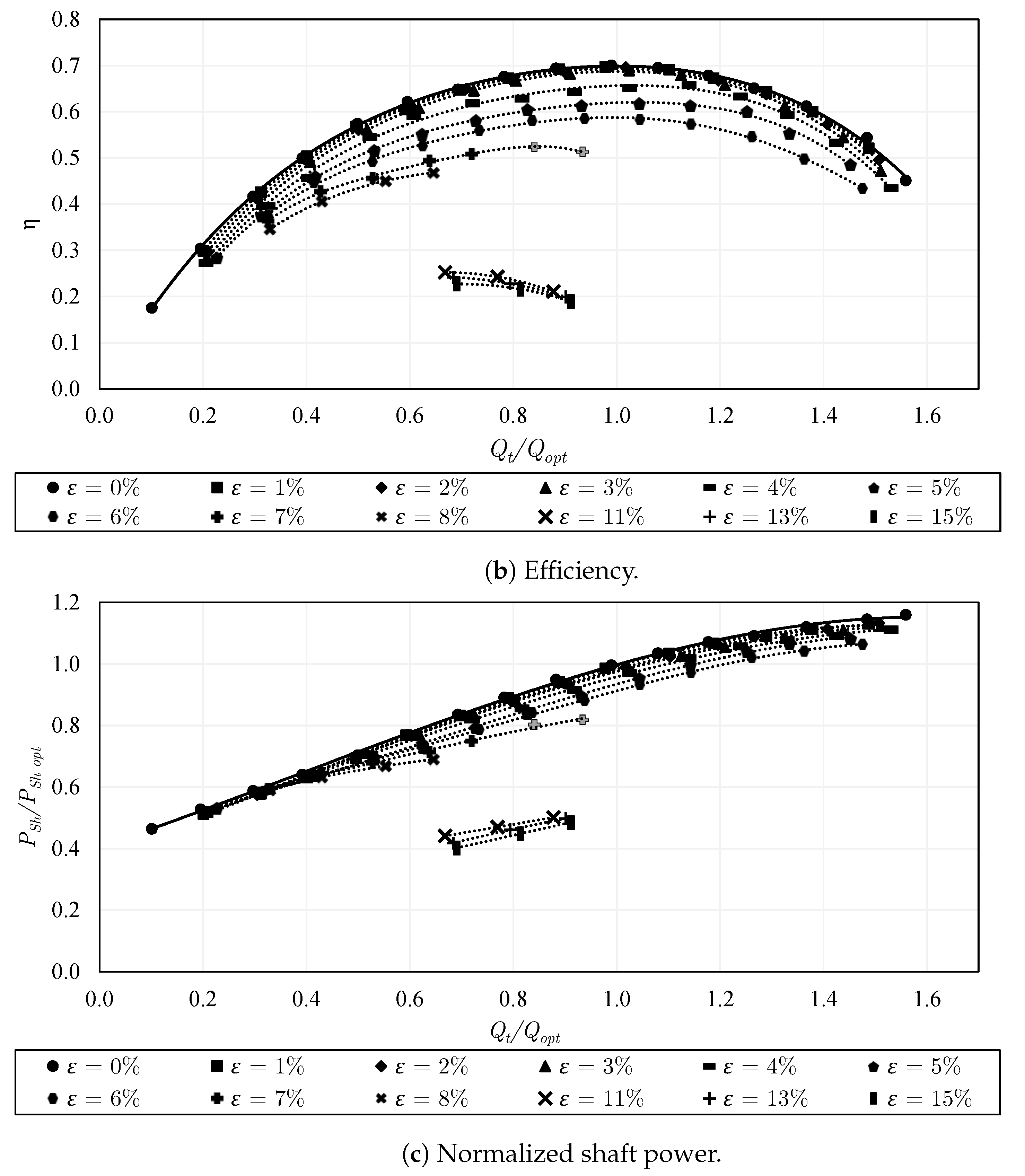
5. Results
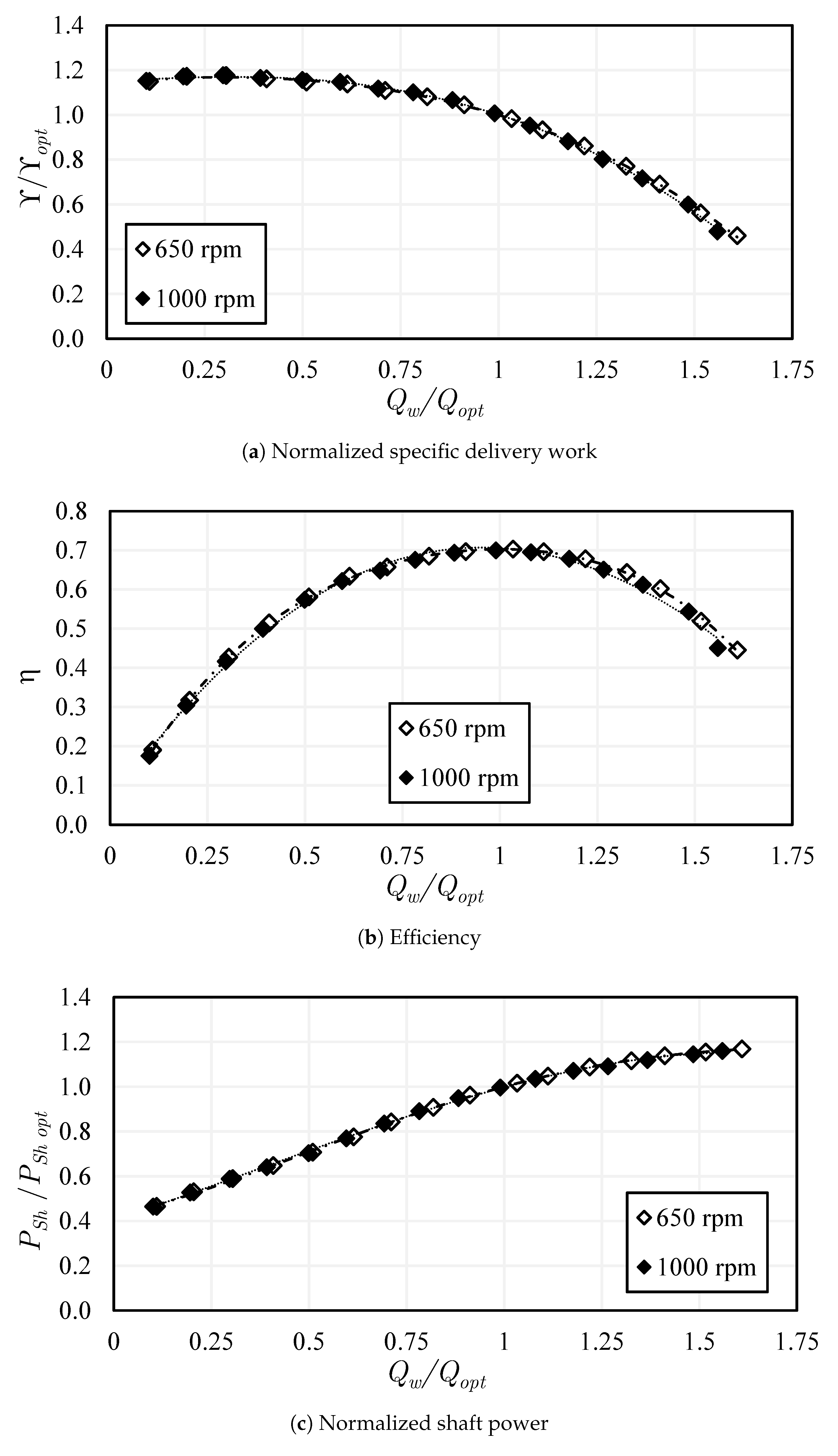
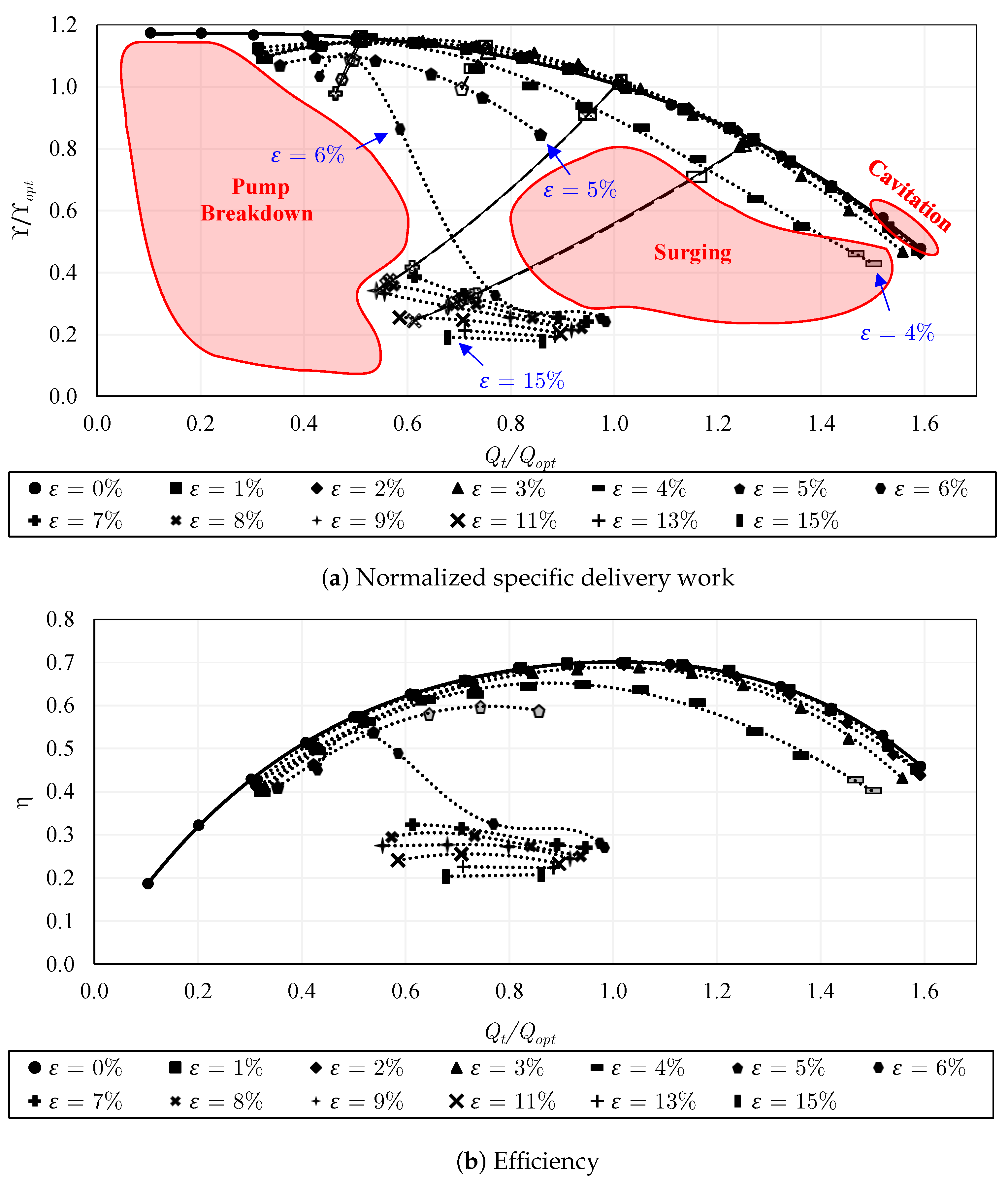
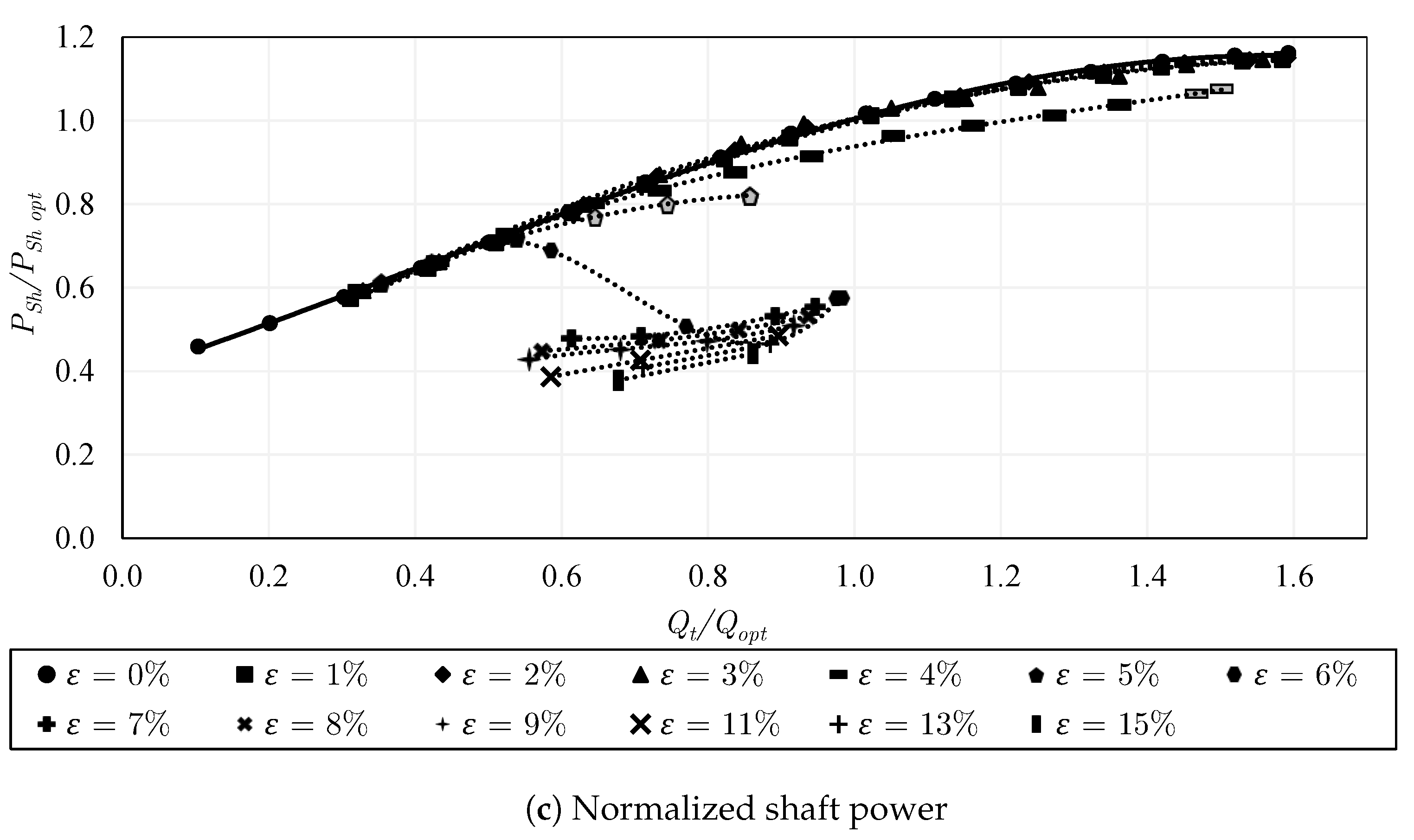
5.1. Performance of Single-Phase Flow
5.2. Pump Configuration 1: Closed Impeller
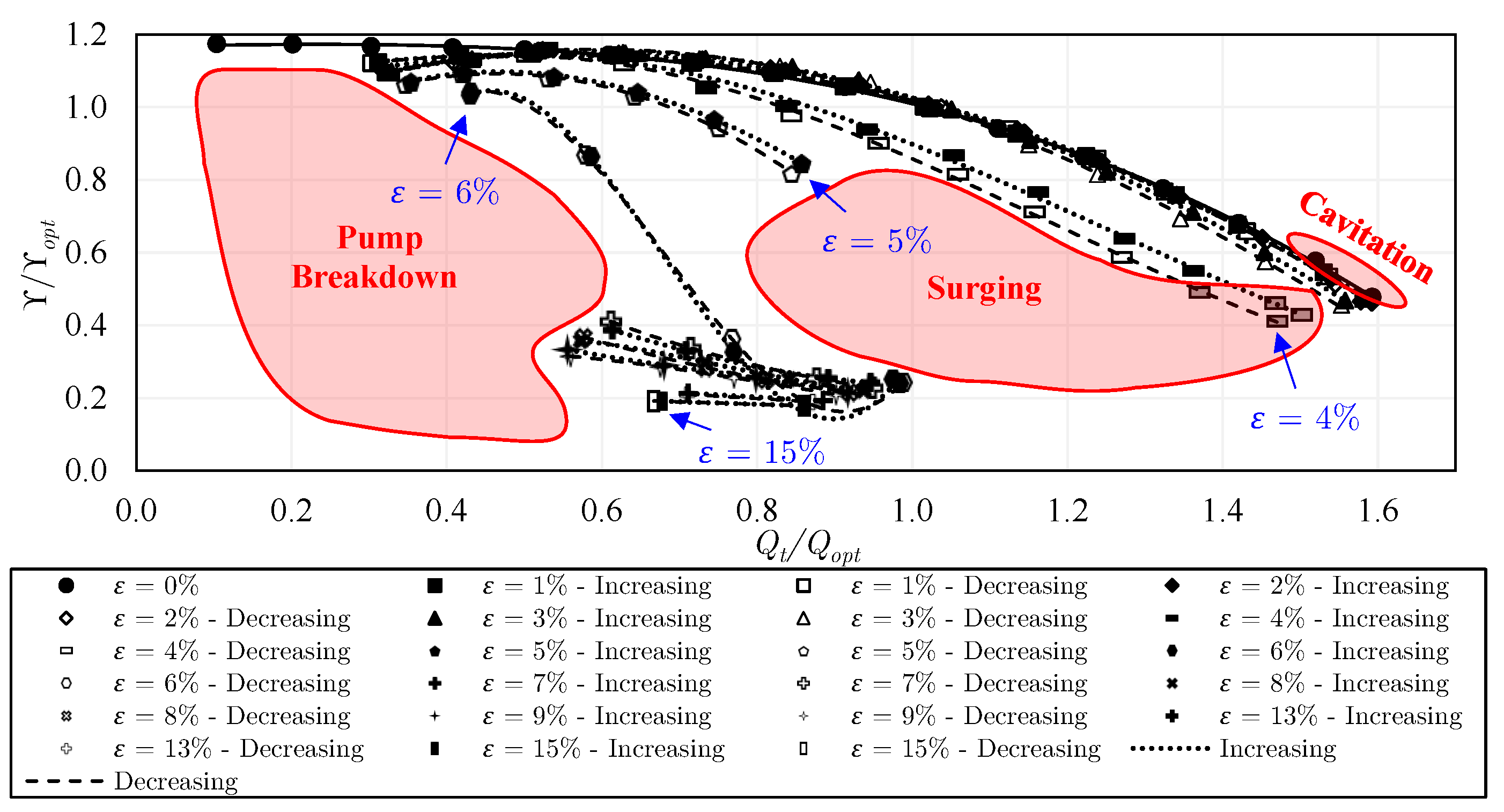
5.3. Pump Configuration 2: Semi-Open Impeller with a Standard Gap
5.4. Pump Configuration 3: Semi-Open Impeller with an Increased Gap
5.5. Pump Configuration 4: Semi-Open Impeller with a Standard Gap and Inducer
5.6. Pump Configuration 5: TTE Semi-Open Impeller with a Standard Gap at 650 Rpm
5.7. Pump Configuration 6: TTE Semi-Open Impeller with a Standard Gap at 1000 Rpm
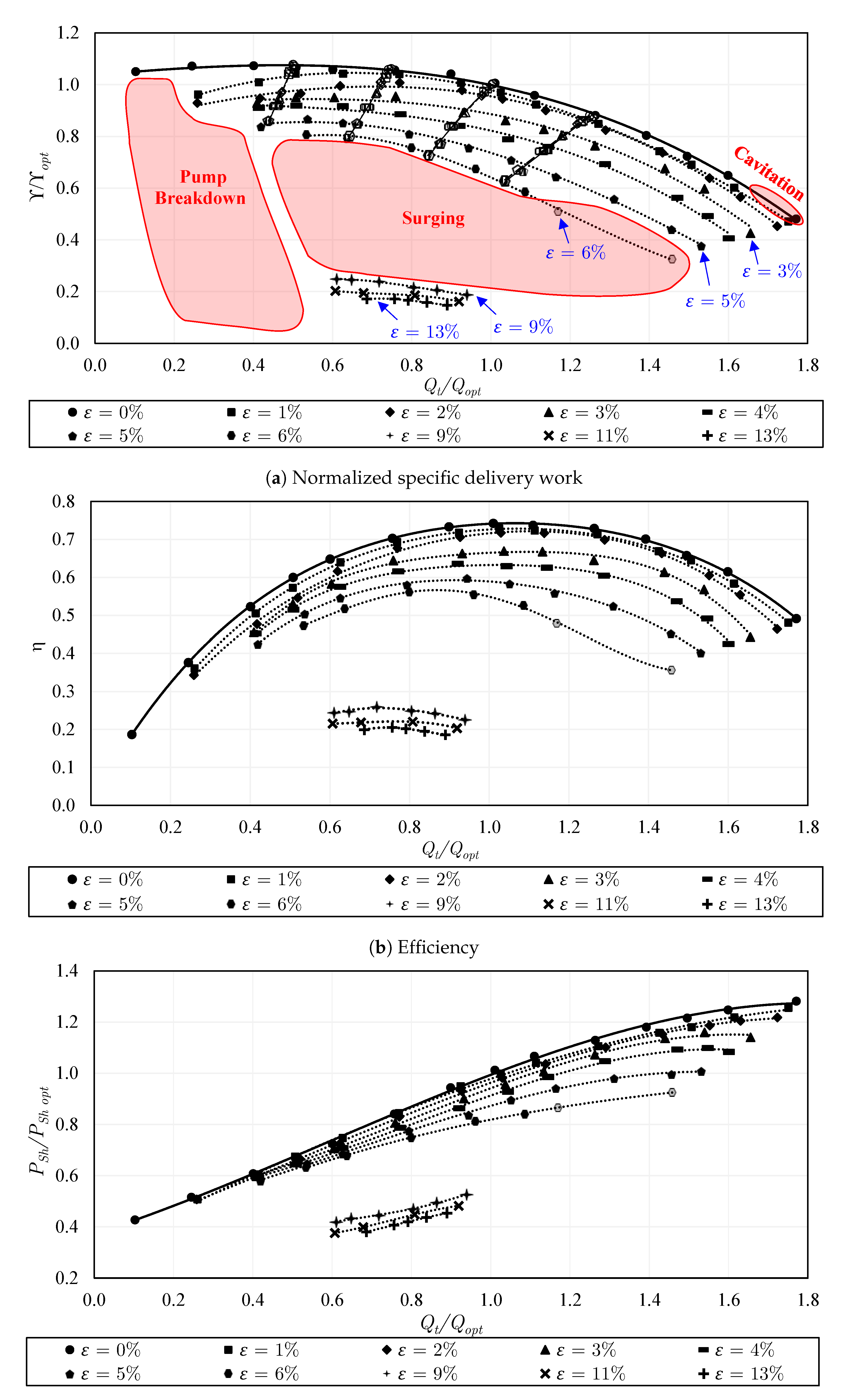
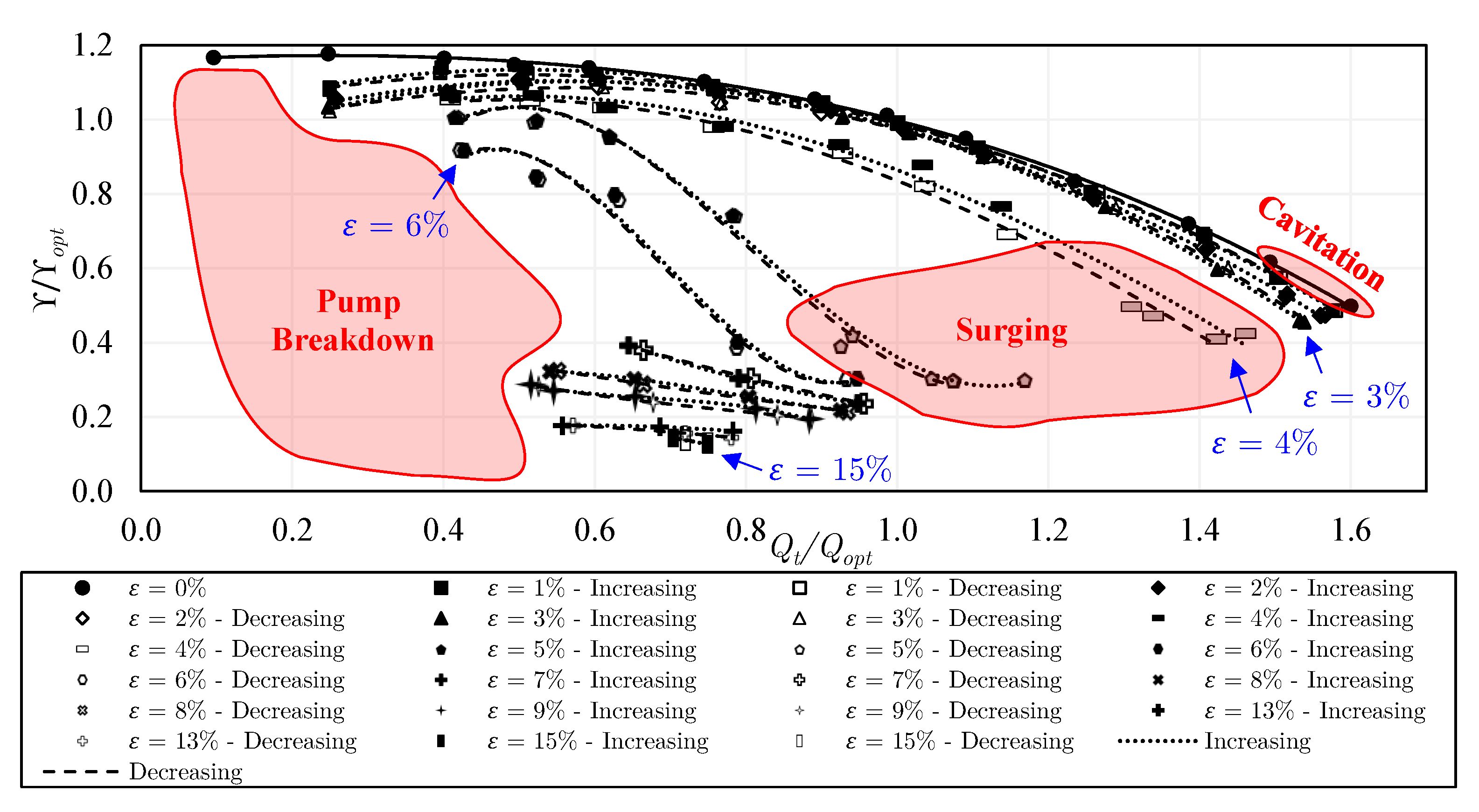
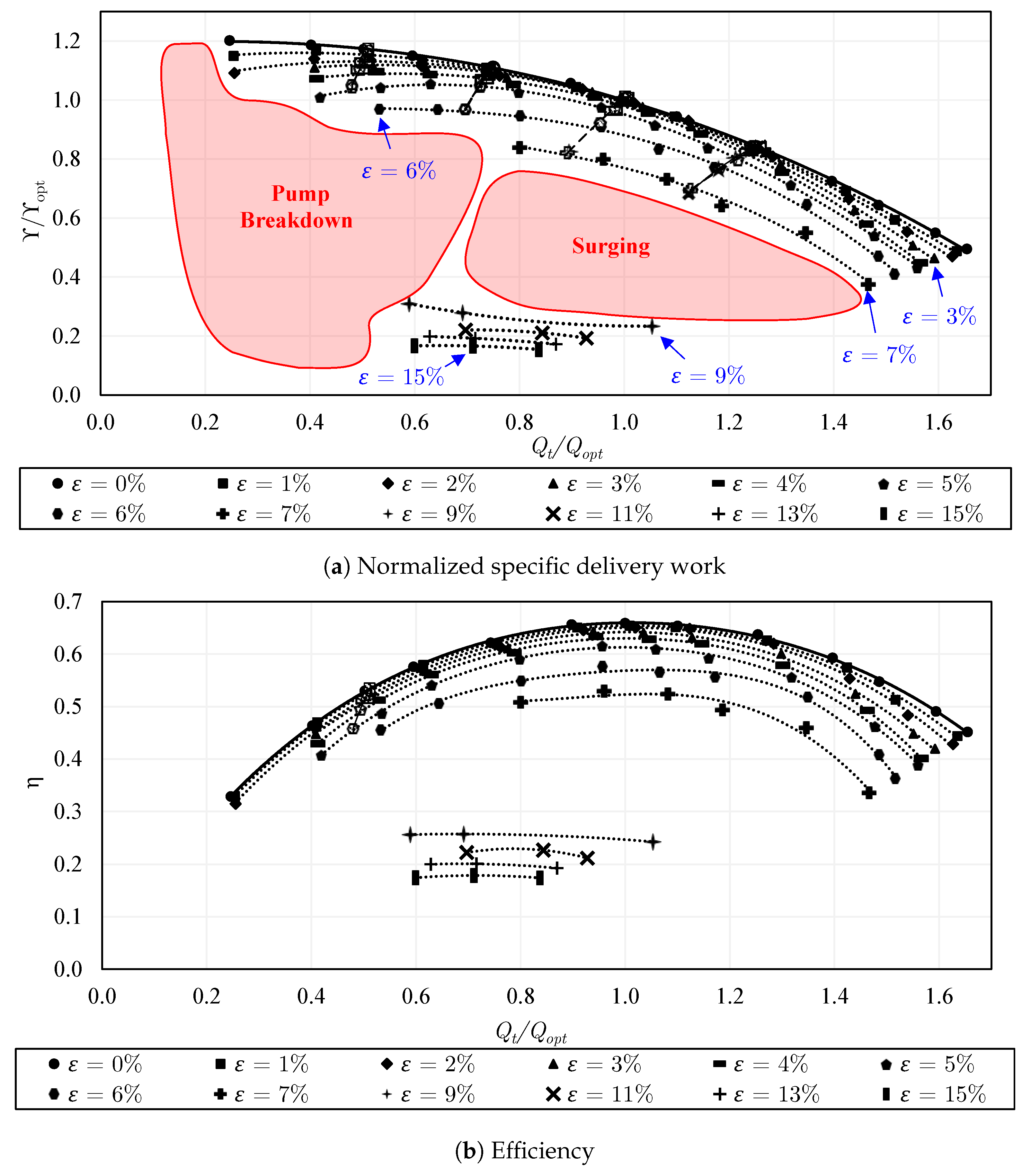
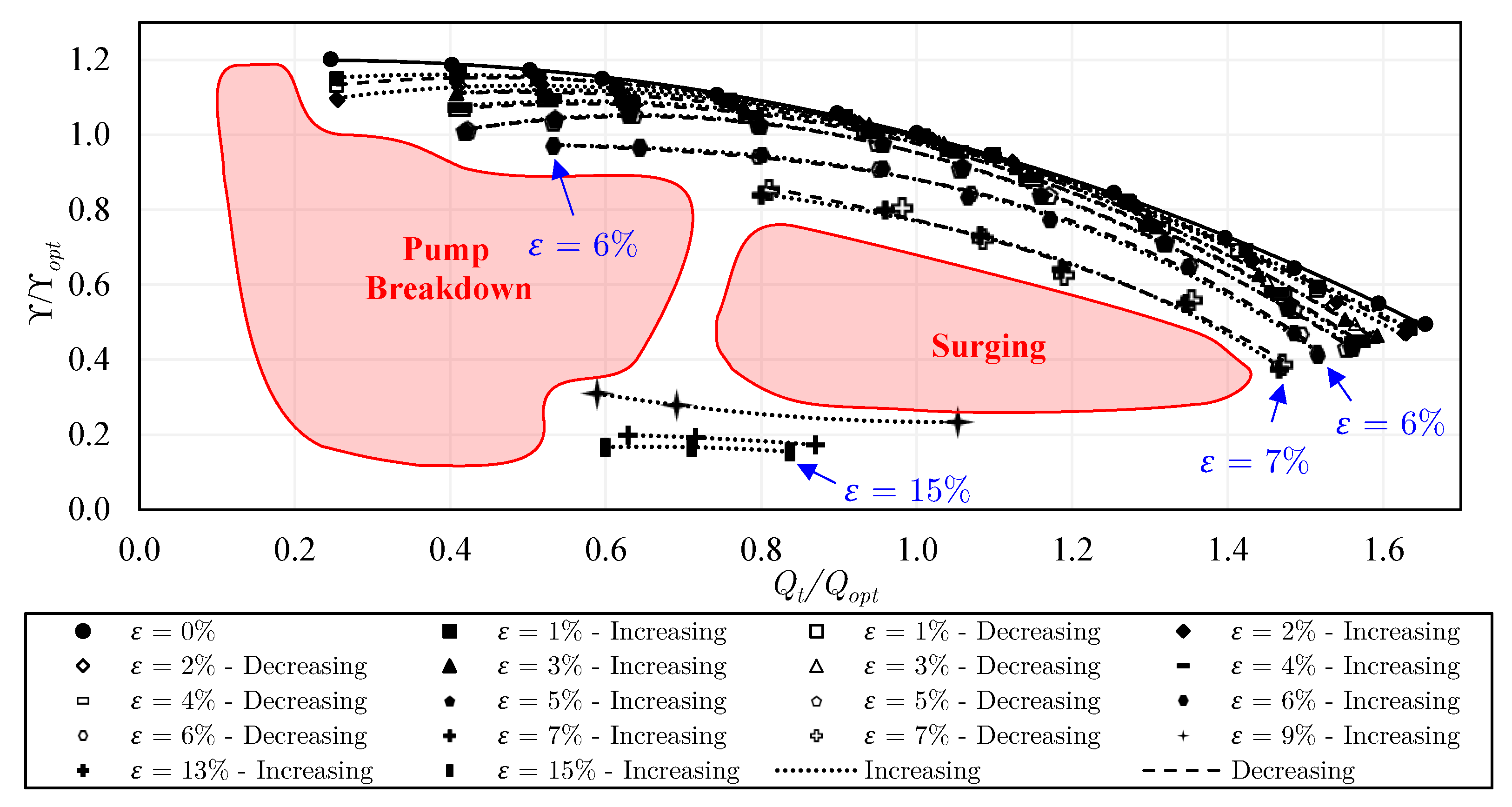
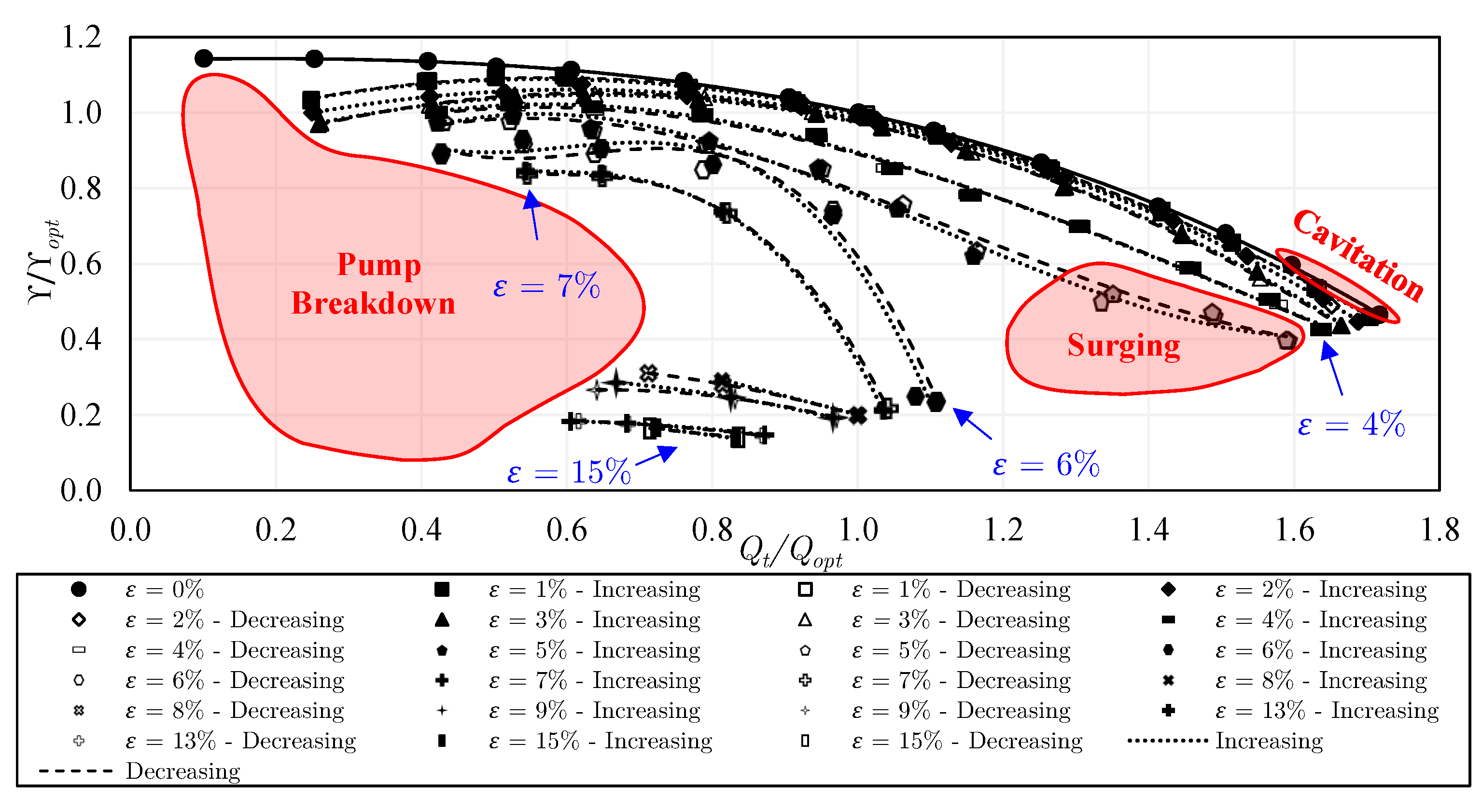
5.8. Performance Degradation
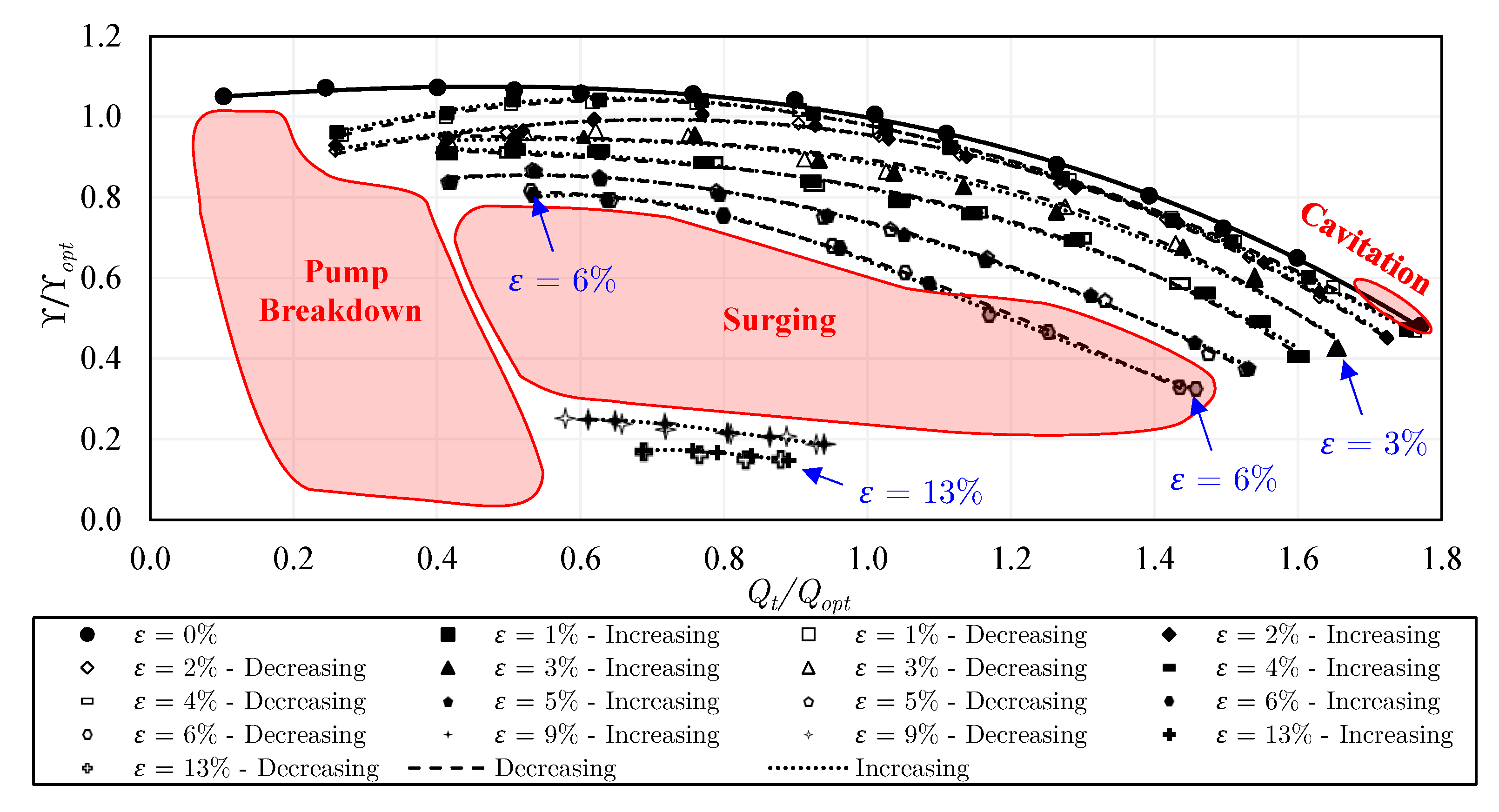
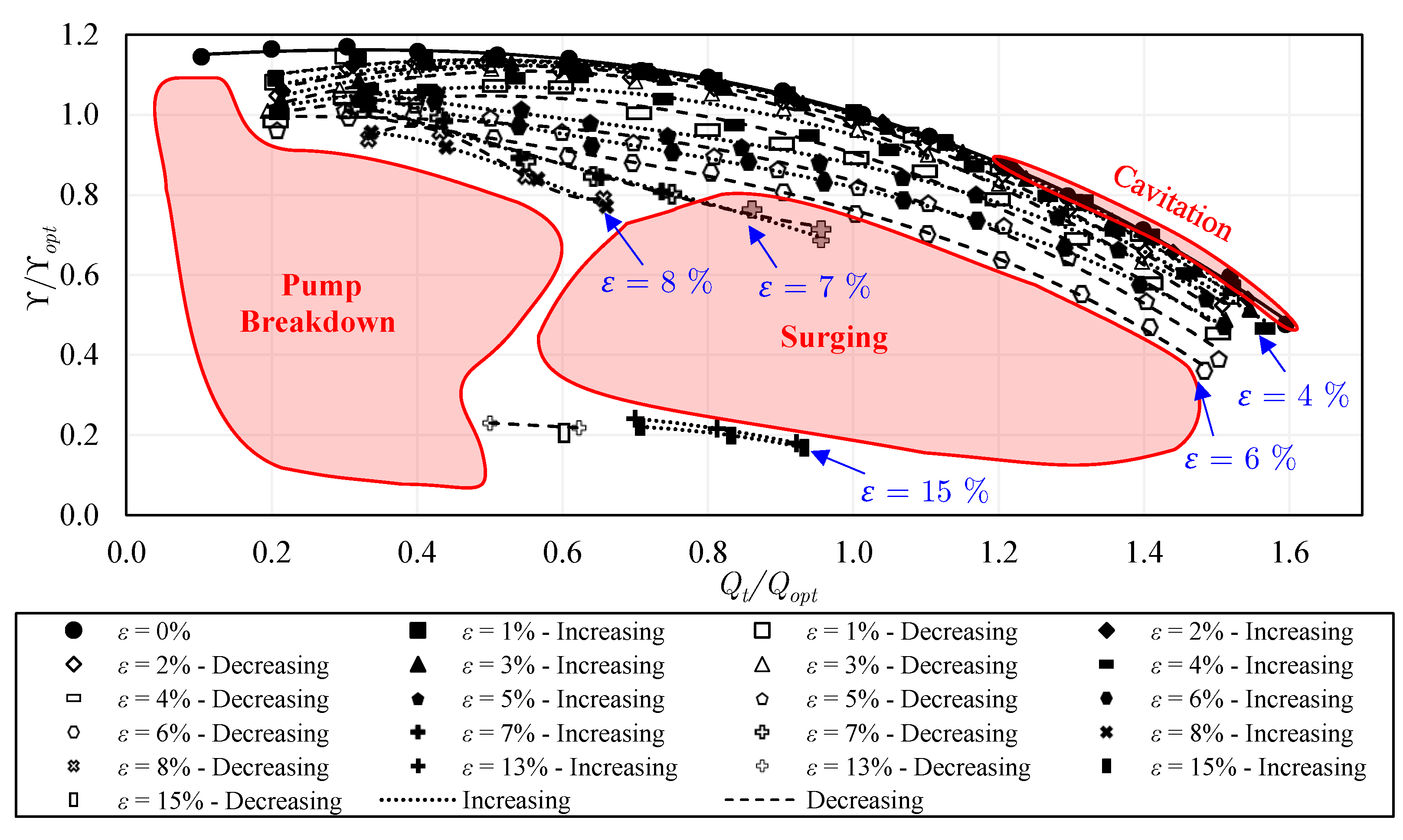
5.9. Intensity of Pump Surging and Instabilities
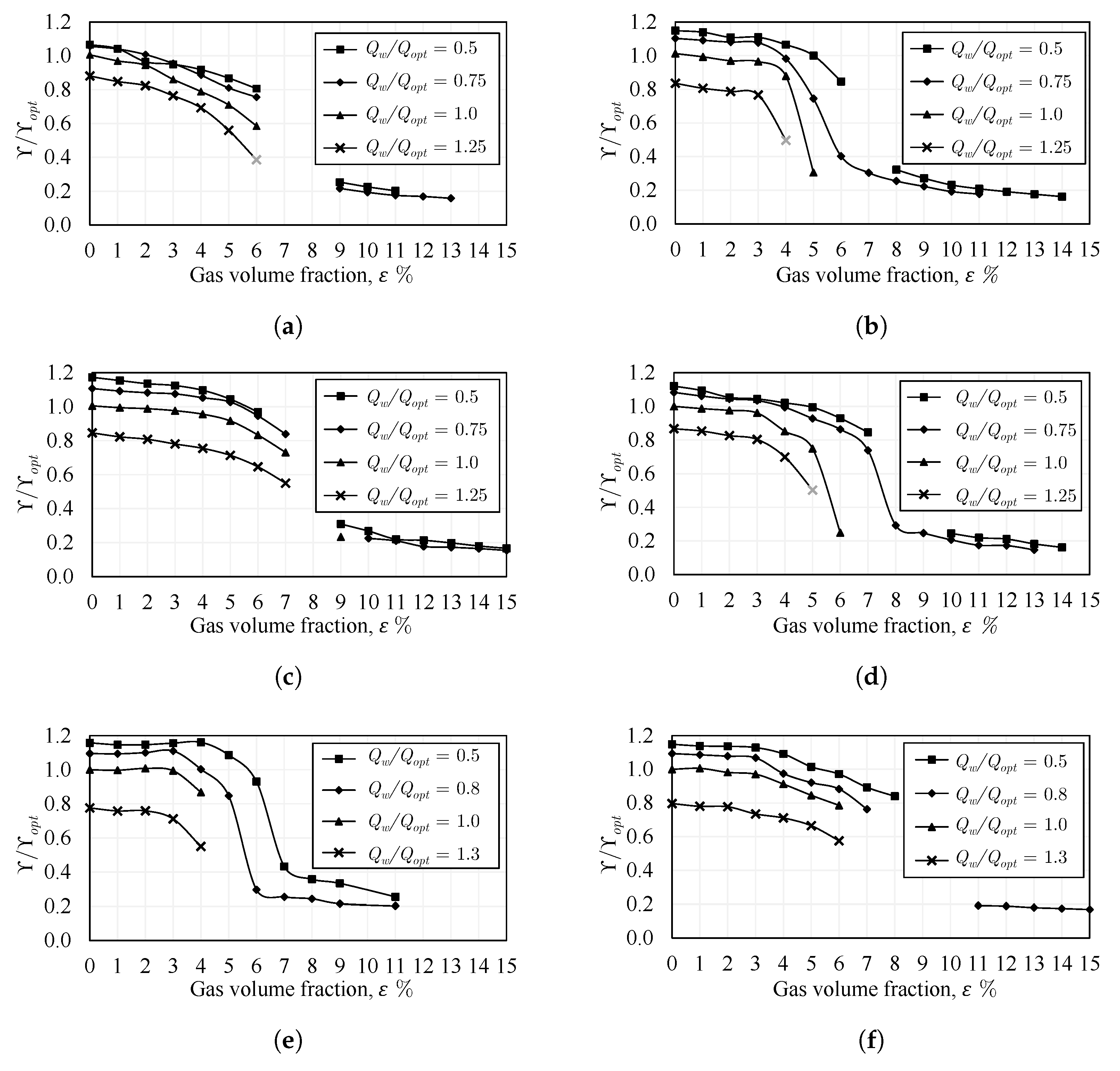
5.10. Performance Curves for Constant Air Flow Rates
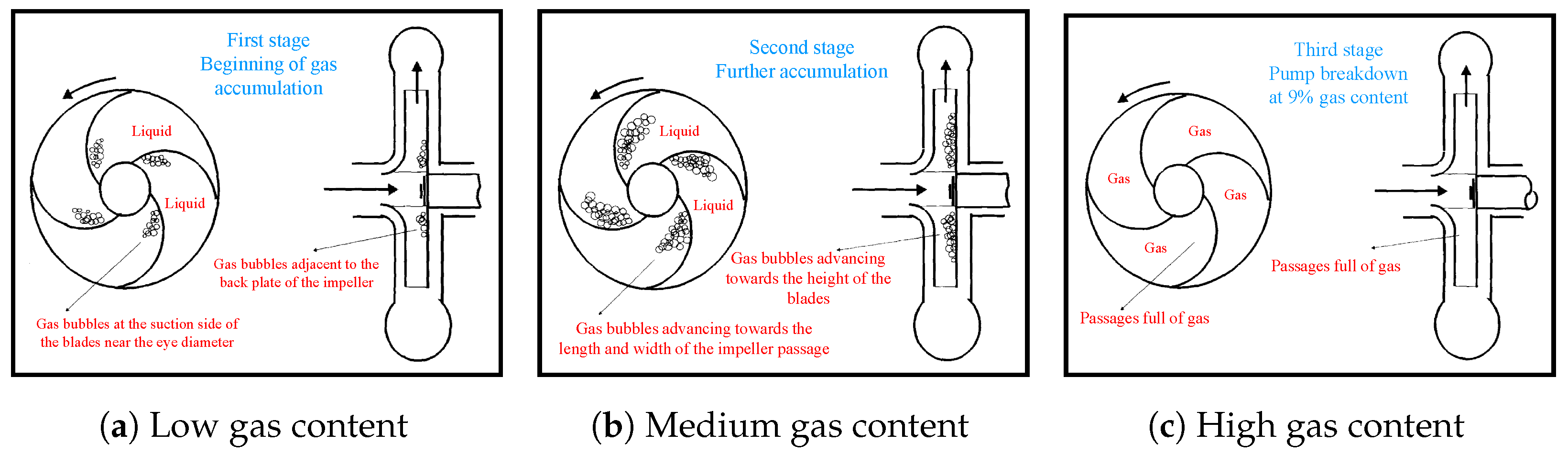
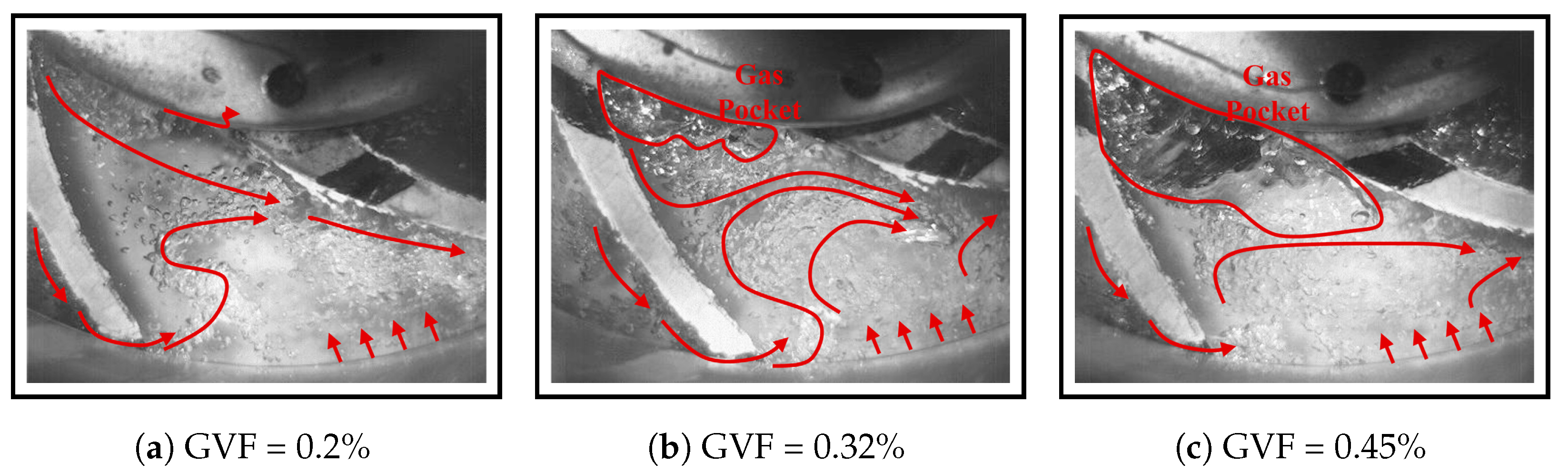
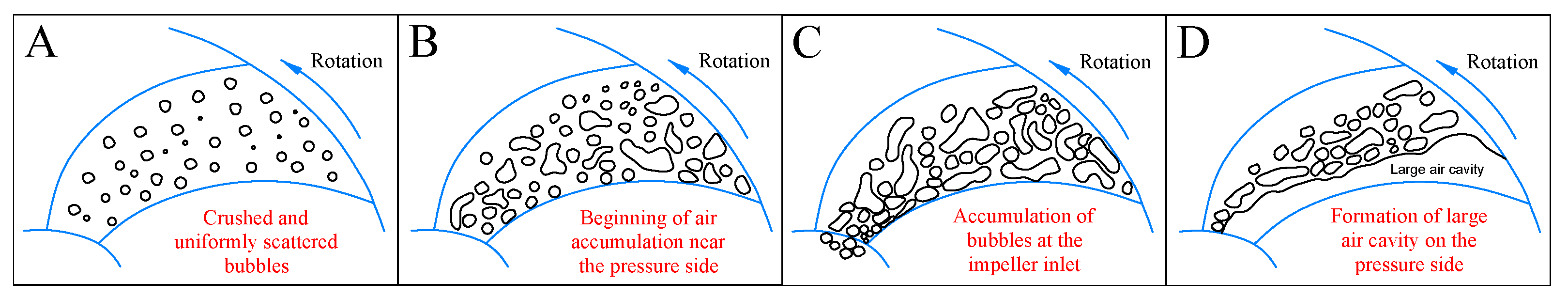
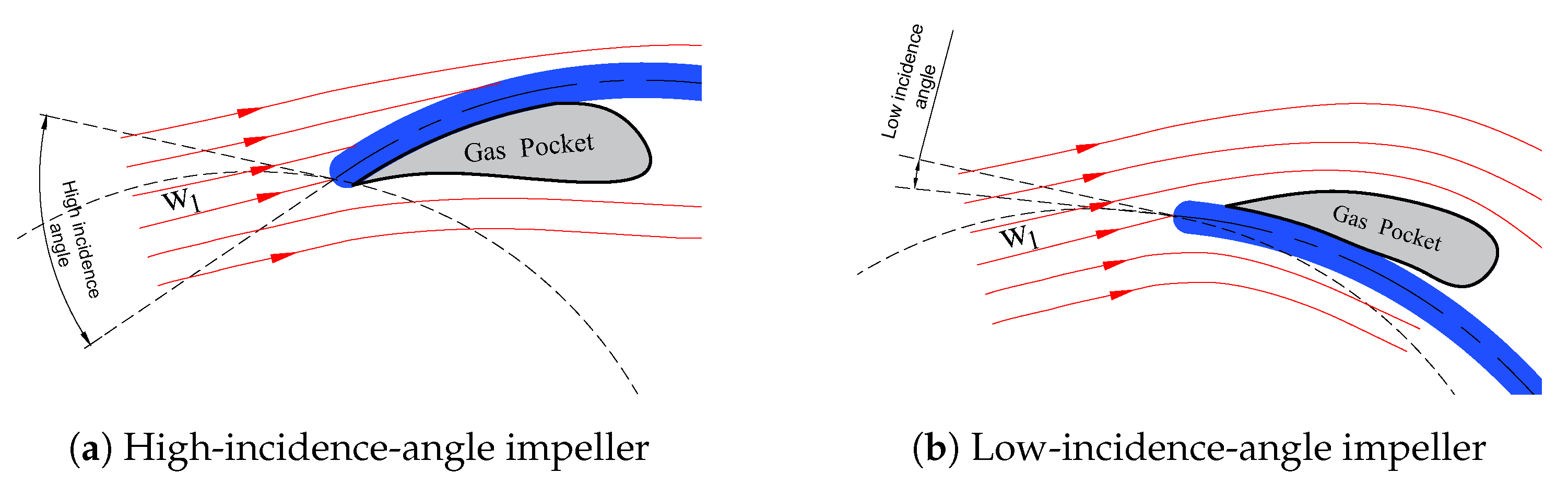
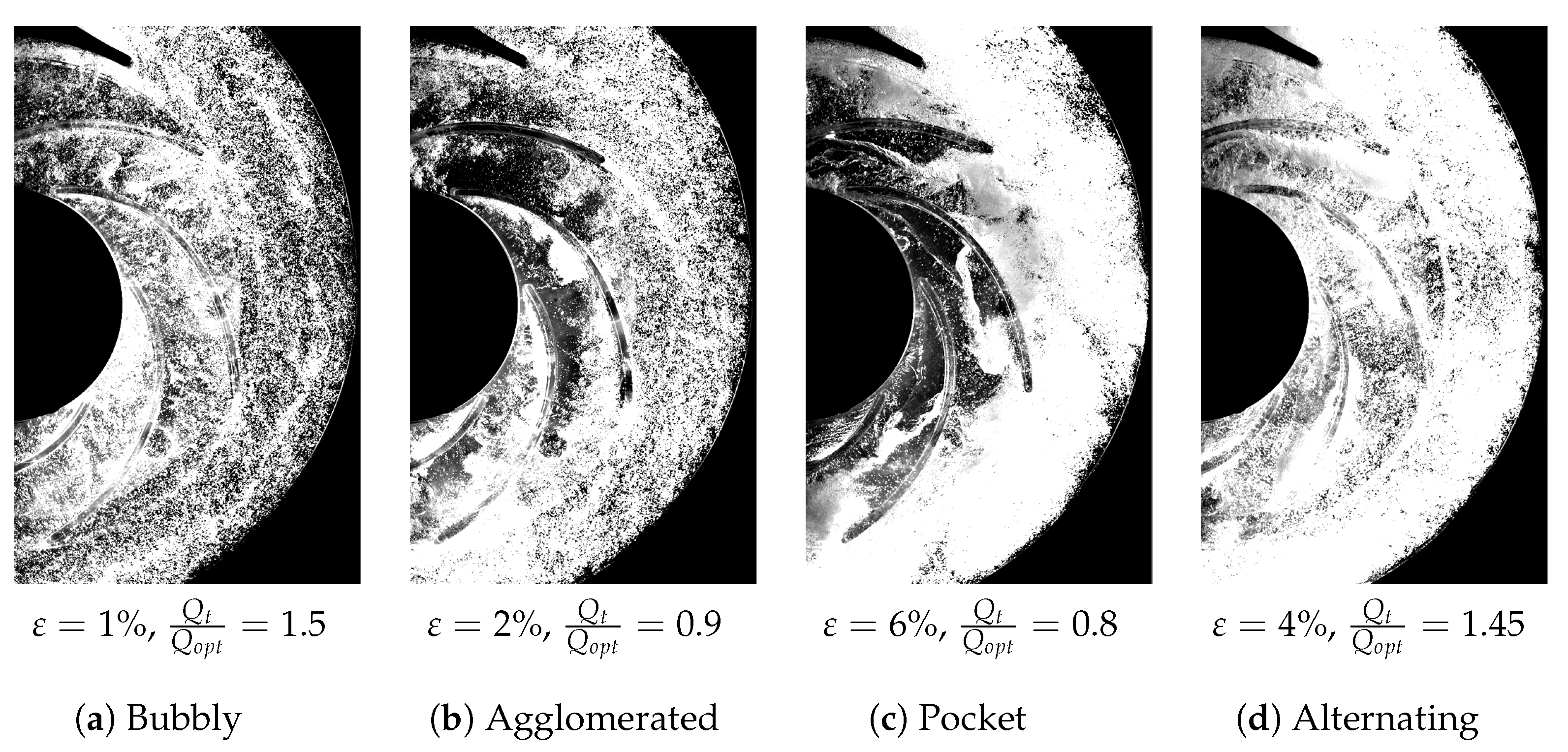
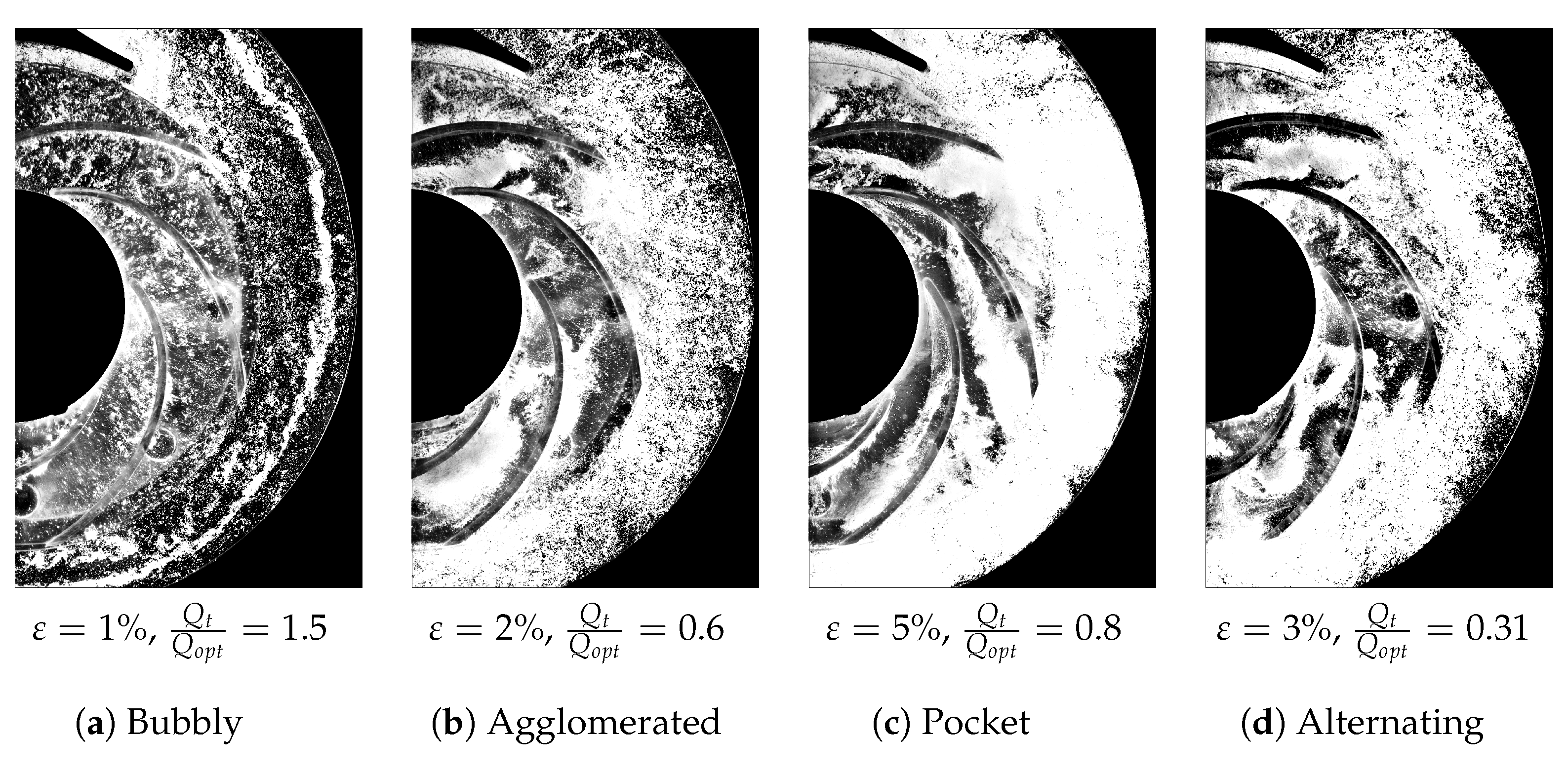
5.11. Visualization of Flow Regimes
- Bubbly regime—Gas bubbles are distributed nearly all over the impeller without considerable agglomerations between them. In the majority of cases, more bubbles appear closer to the suction side of the blades (see, for example, Figure 33a).
- Pocket regime—Large air pockets steadily stand on the suction side of all impeller blades. These pockets are characterized by only a slight variation in size.
- Alternating pocket regime—Large air pockets stand close to the blade inlet and mostly on the suction surface with unstable characteristics (appearing, disappearing, and large fluctuations in size).
- Segregated regime—The air pockets spread over the whole length of the blades until the impeller outlet. This was only found in the closed impeller and it did not take place in the semi-open impeller cases. Sometimes, an asymmetric air ring forms in front of the (closed) impeller, as shown in Figure 31e. A. Poullikkas [11] observed a similar asymmetric gas ring.
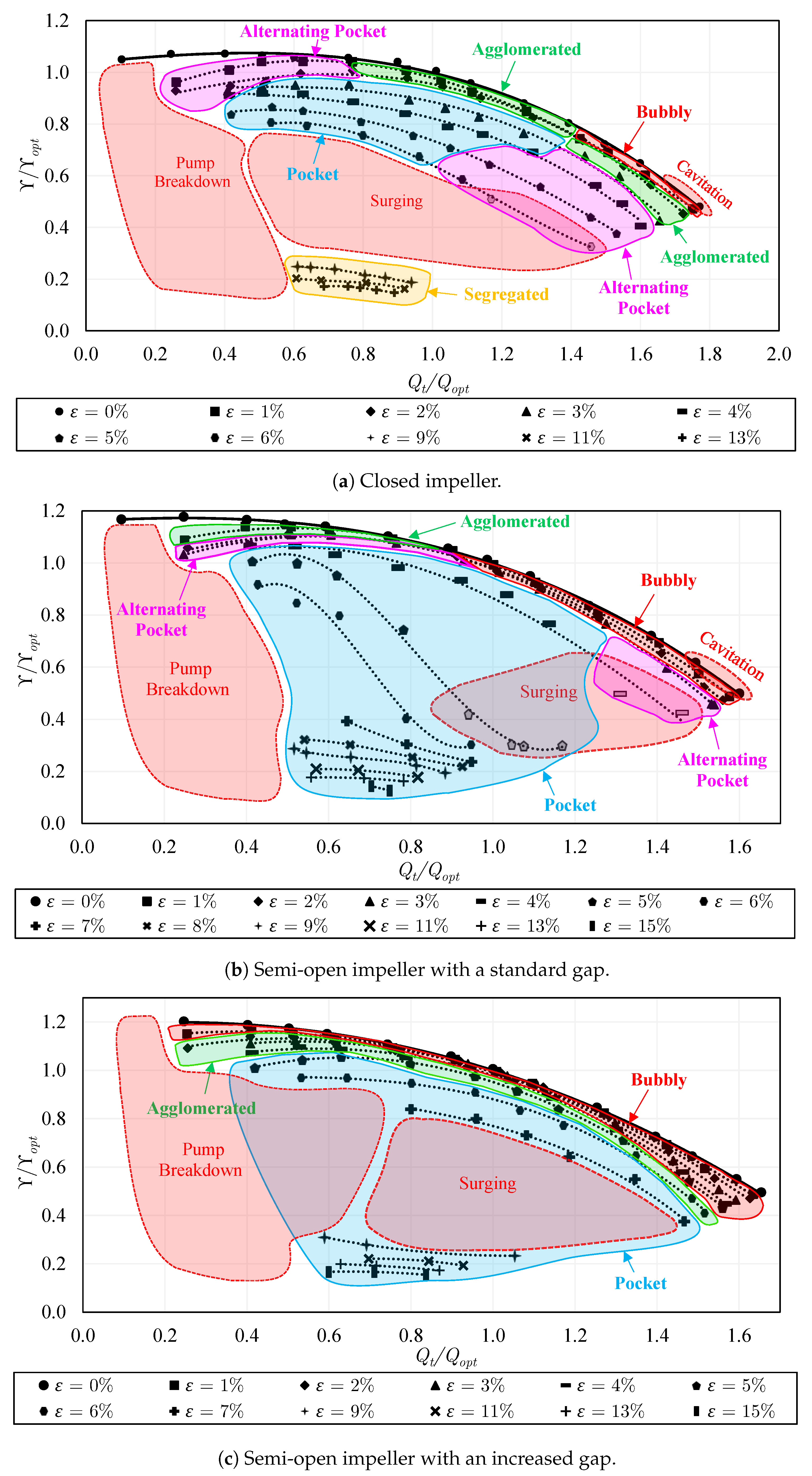
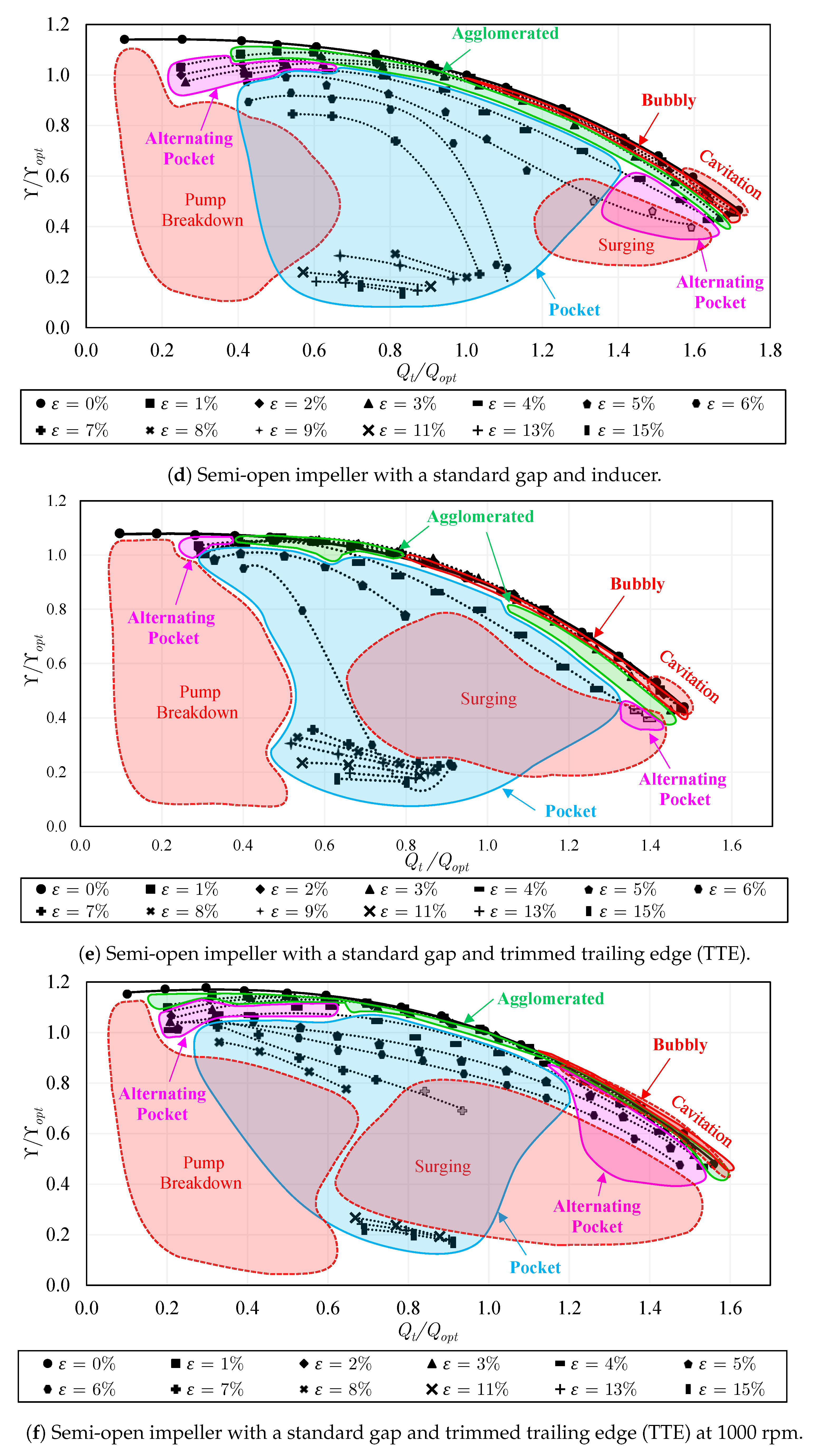
5.12. Flow Regime Maps
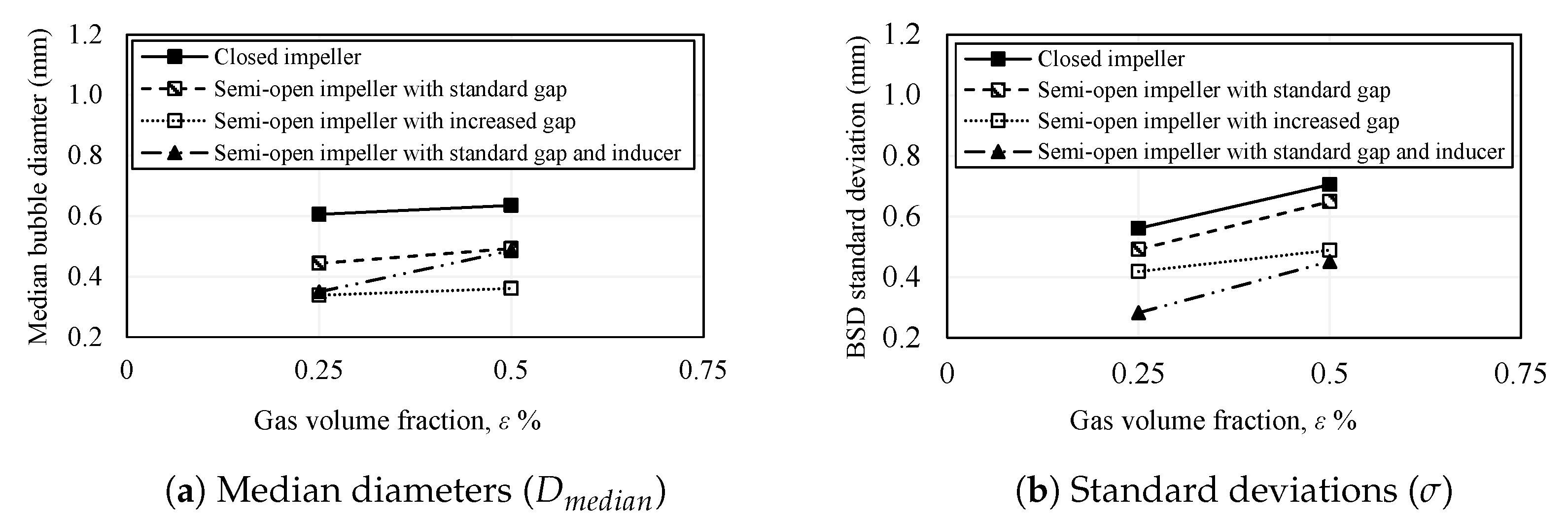
5.13. Bubble Size Distribution
6. Conclusions
- The single-phase flow performance of the closed impeller is slightly higher than that of the semi-open impeller with the standard gap due to the leakage flow. A substantial reduction in the single-phase performance happens in the semi-open impeller when the gap is increased. Quantitatively, a drop of 9% to 40% in specific delivery work takes place from part-load to overload, respectively. On the other hand, the installation of the inducer does not lead to important variations in the single-phase performance. The use of the trimmed trailing edge semi-open impeller causes a drop of about 7 to 28% in the specific delivery work compared to the same impeller with a round trailing edge. The two considered rotational speeds typically show very comparable single-phase performance on normalized scales. Accordingly, for achieving the highest possible single-phase performance, the closed impeller is recommended over the semi-open impeller, the standard gap over the increased gap, and the round trailing edge over the trimmed trailing edge.
- No significant hysteresis occurs in the closed impeller, while, in the semi-open impeller with a standard gap, strong hysteresis is visible between and , due to the former gas accumulations on the blades. However, increasing the tip clearance gap could beneficially prevent hysteresis among different experimental approaches. Likewise, the use of the inducer before the semi-open impeller could strongly decrease the performance hysteresis. When the first two experimental approaches are compared, performance hysteresis takes place within a wider range of gas volume fractions with the trimmed trailing edge semi-open impeller (configuration 5), i.e., = 3–5%, compared to = 4–5% in the round trailing edge semi-open impeller (configuration 2). Nevertheless, the hysteresis is lower in the trimmed trailing edge semi-open impeller compared to that of the round trailing edge semi-open impeller when the first and third approaches are compared. When the rotational speed is increased to 1000 rpm, the performance hysteresis increases remarkably and occurs within a wider range of gas volume fraction, i.e., = 2–6% compared to = 3–5% in the lower rotational speed (650 rpm) when comparing the results of the first and second approaches.
- For two-phase pumping performance, the degradation is much lower in the semi-open impeller with the standard gap compared to that of the closed impeller for . However, for the behavior is reversed, where the performance of the semi-open impeller with a standard gap drops significantly compared to that of the closed impeller. This is mainly apparent at overload flow. Increasing the gap of the semi-open impeller could positively shift the sharp performance drop, allowing very solid pumping until , followed by pump surging. Further, adding the inducer to the semi-open impeller with a standard gap enhances the part-load performance within the range of to and overload performance within to . The two-phase performance and the effective operating range of the round trailing edge semi-open impeller were found to be better than those of the trimmed trailing edge semi-open impeller. Similarly, the overall two-phase performance and the working range of the increased rpm are improved compared to the lower rpm as a result of the enhanced phase mixing, where the abrupt performance reduction is shifted to .
- Regarding the performance curves of constant air flow inlet, the closed impeller exhibits strongly unstable curves compared to most of the semi-open impeller cases. Consequently, the flow instabilities were found to be stronger in the closed impeller. Additionally, pump surging takes place in a wider range of flow conditions in the closed impeller. Increasing the tip clearance gap of the semi-open impeller could strongly decrease the instabilities when compared to those of the semi-open impeller with a standard gap. Similarly, the use of the inducer could diminish the instabilities and reduce the surging region of the semi-open impeller. Comparing the performance curves of the constant air flow inlet, the trimmed trailing edge semi-open impeller shows higher instabilities compared to the round trailing edge semi-open impeller. The intensity of pump surging in the trimmed trailing edge semi-open impeller was found to be lower than that of the round trailing edge semi-open impeller. However, only a low number of data points could be measured and compared under surging conditions. Additionally, the surging region of the trimmed trailing edge semi-open impeller is larger than that of the round trailing edge semi-open impeller. The increase of the rotational speed increases the flow rate and the occurrence of pump surging.
- The transitions between different two-phase flow patterns on the two-phase performance maps explain the location of each flow regime, and the sudden variations and discontinuities of the performance in each pump configuration. Further, the maps reveal the improved gas accumulation resistance of the semi-open impeller with a standard gap compared to the closed impeller. The resistance to gas accumulation increases with increasing the tip clearance gap. Still, the inducer has only a slight impact on the flow regime map of the semi-open impeller. Additionally, gas accumulations take place easier in the trimmed trailing edge impeller due to its lower performance compared to the round trailing edge semi-open impeller.
- The comparisons of sample bubble size distributions showed that the curves of the closed impeller are generally wider with higher median diameters compared to the other pump configurations using semi-open impellers. When the tip clearance gap is increased or the inducer is installed, the bubble size distributions become narrower, with somewhat lower median diameters.
- –
- The closed impeller is suitable only for single-phase flow.
- –
- The semi-open impeller with a standard gap is recommended for gas volume fractions between and .
- –
- Installing the inducer is advised for gas volume fractions between and .
- –
- For gas volume fractions between and , a larger tip clearance gap is preferred.
- –
- The round trailing edge (profiled) is always a better choice compared to the trimmed trailing edge (non-profiled).
- –
- In general, a higher rotational speed is preferred over a lower one, even at the cost of some increased instabilities.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclatures
| Roman characters | ||
| Cross-sectional area of discharge line | () | |
| Cross-sectional area of suction line | () | |
| Blade inlet width | () | |
| Blade outlet width | () | |
| Chord length at inducer blade hub | () | |
| Chord length at inducer blade tip | () | |
| Impeller blade inlet diameter | () | |
| Impeller blade outlet diameter | () | |
| The Sauter mean diameter of bubbles | () | |
| Median bubble diameter | () | |
| Liquid-to-gas density ratio | (-) | |
| Diameter of suction pipe | () | |
| E | Blade thickness | () |
| g | Gravitational acceleration | () |
| H | Pump head | () |
| Mass flow rate of the fluid | () | |
| Air mass flow rate | () | |
| Water mass flow rate | () | |
| Total mass flow rate ( + ) | () | |
| n | Rotational speed | () |
| Specific speed | () | |
| Discharge static pressure | () | |
| Pitch at inducer blade hub | () | |
| Pump useful power | () | |
| Suction static pressure | () | |
| Shaft power | () | |
| Maximum shaft power | () | |
| Shaft power at optimal conditions | () | |
| Pitch at inducer blade tip | () | |
| Q | Volume flow rate | () |
| Air volume flow rate | () | |
| Maximum flow rate | () | |
| Optimal (nominal) flow rate | () | |
| Water volume flow rate | () | |
| Total volume flow rate ( + ) | () | |
| R | Gas constant of air | () |
| Rotational Reynolds number of water | (-) | |
| S | Impeller tip clearance gap | () |
| Inducer tip clearance gap | () | |
| T | Flow temperature | () |
| Suction elevation | () | |
| Delivery elevation | () | |
| Greek characters | ||
| Blade inlet angle | () | |
| Blade outlet angle | () | |
| (Inlet) Gas volume fraction | (%) | |
| Pump efficiency | (%) | |
| Water dynamic viscosity | () | |
| Gas mass fraction | (%) | |
| Angular speed | () | |
| Fluid density | () | |
| Air density | () | |
| Water density | () | |
| Standard deviation of the bubble size distribution | () | |
| Inducer area solidity | (-) | |
| Inducer hub solidity | (-) | |
| Inducer tip solidity | (-) | |
| Torque | () | |
| Specific delivery work | () | |
| Maximum specific delivery work | () | |
| Optimal specific delivery work | () | |
| Subscripts | ||
| 1 | Inlet | |
| 2 | Outlet | |
| a | Air phase | |
| D | Discharge | |
| i | Inducer | |
| Optimal conditions of the pump (conditions at maximum efficiency) | ||
| Maximum values | ||
| S | Suction | |
| Shaft (power) | ||
| t | Total parameters | |
| w | Water phase | |
| Abbreviations | ||
| % FS | Percentage of full scale (Quantification of accuracy) | |
| % RD | Percentage of reading (Quantification of accuracy) | |
| BSD | Bubble size distribution | |
| CFD | Computational fluid dynamics | |
| GVF | Gas volume fraction | |
| HireCT | High-resolution gamma-ray-computed tomography | |
| LED | Light-emitting diodes | |
| NPSH | Net positive suction head (m) |
References
- Ewing, M.E.; Weinandy, J.J.; Christensen, R.N. Observations of Two-Phase Flow Patterns in a Horizontal Circular Channel. Heat Transf. Eng. 1999, 20, 9–14. [Google Scholar]
- Wibisono, Y.; Cornelissen, E.R.; Kemperman, A.J.B.; Van Der Meer, W.G.J.; Nijmeijer, K. Two-phase flow in membrane processes: A technology with a future. J. Membr. Sci. 2014, 453, 566–602. [Google Scholar] [CrossRef]
- Chen, I.Y.; Tseng, C.Y.; Lin, Y.T.; Wang, C.C. Two-phase flow pressure change subject to sudden contraction in small rectangular channels. Int. J. Multiph. Flow 2009, 35, 297–306. [Google Scholar] [CrossRef]
- Sharma, A.; Tyagi, V.; Chen, C.; Buddhi, D. Review on thermal energy storage with phase change materials and applications. Renew. Sustain. Energy Rev. 2009, 13, 318–345. [Google Scholar] [CrossRef]
- Volk, M. Pump Characteristics and Applications; CRC Press: Boca Raton, FL, USA, 2013. [Google Scholar]
- Jones, W.V. Motor Selection Made Easy: Choosing the Right Motor for Centrifugal Pump Applications. IEEE Ind. Appl. Mag. 2013, 19, 36–45. [Google Scholar] [CrossRef]
- Caridad, J.; Asuaje, M.; Kenyery, F.; Tremante, A.; Aguillón, O. Characterization of a centrifugal pump impeller under two-phase flow conditions. J. Pet. Sci. Eng. 2008, 63, 18–22. [Google Scholar] [CrossRef]
- Zhu, Z.; Xie, P.; Ou, G.; Cui, B.; Li, Y. Design and experimental analyses of small-flow high-head centrifugal-vortex pump for gas-liquid two-phase mixture. Chin. J. Chem. Eng. 2008, 16, 528–534. [Google Scholar] [CrossRef]
- Amoresano, A.; Langella, G.; Niola, V.; Quaremba, G. Advanced image analysis of two-phase flow inside a centrifugal pump. Adv. Mech. Eng. 2014, 6, 1–11. [Google Scholar] [CrossRef]
- Chan, A.; Kawaji, M.; Nakamura, H.; Kukita, Y. Experimental study of two-phase pump performance using a full size nuclear reactor pump. Nucl. Eng. Des. 1999, 193, 159–172. [Google Scholar] [CrossRef]
- Poullikkas, A. Effects of two-phase liquid-gas flow on the performance of nuclear reactor cooling pumps. Prog. Nucl. Energy 2003, 42, 3–10. [Google Scholar] [CrossRef]
- Mansour, M.; Kováts, P.; Wunderlich, B.; Thévenin, D. Experimental investigations of a two-phase gas/liquid flow in a diverging horizontal channel. Exp. Therm. Fluid Sci. 2018, 93, 210–217. [Google Scholar] [CrossRef]
- Kopparthy, S.; Mansour, M.; Janiga, G.; Thévenin, D. Numerical investigations of turbulent single-phase and two-phase flows in a diffuser. Int. J. Multiph. Flow 2020, 130, 103333. [Google Scholar] [CrossRef]
- Ahmed, W.H.; Ching, C.Y.; Shoukri, M. Development of two-phase flow downstream of a horizontal sudden expansion. Int. J. Heat Fluid Flow 2008, 29, 194–206. [Google Scholar] [CrossRef]
- Li, W.G. Effects of viscosity of fluids on centrifugal pump performance and flow pattern in the impeller. Int. J. Heat Fluid Flow 2000, 21, 207–212. [Google Scholar] [CrossRef]
- Zhu, J.; Zhu, H.; Zhang, J.; Zhang, H.Q. A numerical study on flow patterns inside an electrical submersible pump (ESP) and comparison with visualization experiments. J. Petrol. Sci. Eng. 2019, 173, 339–350. [Google Scholar] [CrossRef]
- Zhu, J.; Zhang, H.Q. Mechanistic modeling and numerical simulation of in-situ gas void fraction inside ESP impeller. J. Nat. Gas Sci. Eng. 2016, 36, 144–154. [Google Scholar] [CrossRef]
- Zhou, L.; Shi, W.; Cao, W.; Yang, H. CFD investigation and PIV validation of flow field in a compact return diffuser under strong part-load conditions. Sci. China Technol. Sci. 2015, 58, 405–414. [Google Scholar] [CrossRef]
- Si, Q.; Bois, G.; Zhang, K.; Yuan, S. Air-water two-phase flow experimental and numerical analysis in a centrifugal pump. In Proceedings of the 12th European Conference on Turbomachinery Fluid Dynamics and Thermodynamics, ETC12, Stockholm, Sweden, 3–7 April 2017; Volume 12. [Google Scholar]
- Parikh, T.; Mansour, M.; Thévenin, D. Investigations on the effect of tip clearance gap and inducer on the transport of air-water two-phase flow by centrifugal pumps. Chem. Eng. Sci. 2020, 218, 115554. [Google Scholar] [CrossRef]
- Kye, B.; Park, K.; Choi, H.; Lee, M.; Kim, J.H. Flow characteristics in a volute-type centrifugal pump using large eddy simulation. Int. J. Heat Fluid Flow 2018, 72, 52–60. [Google Scholar] [CrossRef]
- Posa, A. LES investigation on the dependence of the flow through a centrifugal pump on the diffuser geometry. Int. J. Heat Fluid Flow 2021, 87, 108750. [Google Scholar] [CrossRef]
- Jiang, Q.; Heng, Y.; Liu, X.; Zhang, W.; Bois, G.; Si, Q. A review of design considerations of centrifugal pump capability for handling inlet gas-liquid two-phase flows. Energies 2019, 12, 1078. [Google Scholar] [CrossRef]
- Campo, A.; Chisely, E.A. Experimental characterization of two-phase flow centrifugal pumps. In Proceedings of the ASME 2010 Power Conference American Society of Mechanical Engineers, Chicago, IL, USA, 13–15 July 2010; pp. 803–816. [Google Scholar]
- Poullikkas, A. Two Phase Flow and Cavitation in Centrifugal Pump: A Theoretical and Experimental Investigation. Ph.D. Thesis, Loughborough University, Loughborough, UK, 1992. [Google Scholar]
- Manzano Ruiz, J.J. Experimental and Theoretical Study of Two-Phase Flow in Centrifugal Pumps. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1980. [Google Scholar]
- Noghrehkar, G.R.; Kawaji, M.; Chan, A.M.C.; Nakamura, H.; Kukita, Y. Investigation of centrifugal pump performance under two-phase flow conditions. J. Fluids Eng. 1995, 117, 129–137. [Google Scholar] [CrossRef]
- Mansour, M.; Wunderlich, B.; Thévenin, D. Experimental study of two-phase air/water flow in a centrifugal pump working with a closed or a semi-open impeller. In Proceedings of the ASME Turbo Expo 2018: Turbomachinery Technical Conference and Exposition, American Society of Mechanical Engineers, Oslo, Norway, 11–15 June 2018; p. V009T27A012. [Google Scholar]
- Mansour, M.; Wunderlich, B.; Thévenin, D. Effect of tip clearance gap and inducer on the transport of two-phase air-water flows by centrifugal pumps. Exp. Therm. Fluid Sci. 2018, 99, 487–509. [Google Scholar] [CrossRef]
- Mansour, M.; Parikh, T.; Engel, S.; Wunderlich, B.; Thévenin, D. Investigation on the influence of an inducer on the transport of single and two-phase air-flows by centrifugal pumps. In Proceedings of the 48th Turbomachinery & 35th Pump Symposia, Houston, TX, USA, 9–12 September 2019. [Google Scholar]
- Mansour, M.; Parikh, T.; Thévenin, D. Influence of the shape of the impeller blade trailing edge on single and two-phase air-water flows in a centrifugal pump. In Proceedings of the 49th Turbomachinery & 36th Pump Symposia, Virtual, Houston, TX, USA, 8–10 December 2020. [Google Scholar]
- Mansour, M.; Kopparthy, S.; Thévenin, D. Investigations on the effect of rotational speed on the transport of air-water two-phase flows by centrifugal pumps. Int. J. Heat Fluid Flow 2022, 94, 108939. [Google Scholar] [CrossRef]
- Pessoa, R.; Prado, M. Two-phase flow performance for electrical submersible pump stages. SPE Prod. Facil. 2003, 18, 13–27. [Google Scholar] [CrossRef]
- He, D.; Zhao, L.; Chang, Z.; Zhang, Z.; Guo, P.; Bai, B. On the performance of a centrifugal pump under bubble inflow: Effect of gas-liquid distribution in the impeller. J. Pet. Sci. Eng. 2021, 203, 108587. [Google Scholar] [CrossRef]
- Zhao, L.; Chang, Z.; Zhang, Z.; Huang, R.; He, D. Visualization of gas-liquid flow pattern in a centrifugal pump impeller and its influence on the pump performance. Meas. Sens. 2021, 13, 100033. [Google Scholar] [CrossRef]
- Gamboa, J.; Prado, M. Review of electrical-submersible-pump surging correlation and models. SPE Prod. Oper. 2011, 26, 314–324. [Google Scholar] [CrossRef]
- Zhu, J.; Guo, X.; Liang, F.; Zhang, H.Q. Experimental study and mechanistic modeling of pressure surging in electrical submersible pump. J. Nat. Gas Sci. Eng. 2017, 45, 625–636. [Google Scholar] [CrossRef]
- Monte Verde, W.; Biazussi, J.L.; Sassim, N.A.; Bannwart, A.C. Experimental study of gas-liquid two-phase flow patterns within centrifugal pumps impellers. Exp. Therm. Fluid Sci. 2017, 85, 37–51. [Google Scholar] [CrossRef]
- Tillack, P. Förderverhalten von Kreiselpumpen bei Viskosen, Gasbeladenen Flüssigkeiten. Ph.D. Thesis, Technische Universität Kaiserslautern, Kaiserslautern, Germany, 1998. [Google Scholar]
- Sauer, M. Einfluss der Zuströmung auf das Förderverhalten von Kreiselpumpen Radialer Bauart bei Flüssigkeits-/Gasförderung. Ph.D. Thesis, Technische Universität Kaiserslautern, Kaiserslautern, Germany, 2003. [Google Scholar]
- Budris, A.R.; Mayleben, P.A. Effects of entrained air, NPSH margin, and suction piping on cavitation in centrifugal pumps. In Proceedings of the 15th International Pump Users Symposium, Houston, TX, USA, 2–5 March 1998. [Google Scholar]
- Si, Q.; Bois, G.; Jiang, Q.; He, W.; Ali, A.; Yuan, S. Investigation on the handling ability of centrifugal pumps under air–water two-phase inflow: Model and experimental validation. Energies 2018, 11, 3048. [Google Scholar] [CrossRef]
- Pumps, S. Sulzer Centrifugal Pump Handbook; Elsevier: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Beltur, R. Experimental Investigation of Two-Phase Flow Performance of ESP Stages. Master’s Thesis, Department of Petroleum Engineering, University of Tulsa, Tulsa, OK, USA, 2003. [Google Scholar]
- Cappellino, C.A.; Roll, D.R.; Wilson, G. Design Considerations and application guidelines for pumping liquids with entrained gas using open impeller centrifugal pumps. In Proceedings of the 9th International Pump Users Symposium, Houston, TX, USA, 3–5 March 1992; pp. 262–264. [Google Scholar]
- Cirilo, R. Air-Water Flow through Electric Submersible Pumps. Ph.D. Thesis, Department of Petroleum Engineering, University of Tulsa, Tulsa, OK, USA, 1998. [Google Scholar]
- Shi, G.; Tao, S.; Liu, X.; Wen, H.; Shu, Z. Effect of gas volume fraction on the gas-phase distribution in the passage and blade surface of the axial flow screw-type oil-gas multiphase pump. Processes 2021, 9, 760. [Google Scholar] [CrossRef]
- Xu, Y.; Cao, S.; Reclari, M.; Wakai, T.; Sano, T. Multiphase performance and internal flow pattern of helico-axial pumps. IOP Conf. Ser. Earth Environ. Sci. 2019, 240, 032029. [Google Scholar] [CrossRef]
- Mansour, M.; Ali, M.H.; Abdel-Maksoud, R.M. Experimental study of expansion and compression effects on the stability of Taylor vortex flow. Fluid Dyn. Res. 2016, 48, 045502. [Google Scholar] [CrossRef]
- Murakami, M.; Minemura, K. Effects of entrained air on the performance of a centrifugal pump: 1st report, performance and flow conditions. Bull. JSME 1974, 17, 1047–1055. [Google Scholar] [CrossRef]
- Minemura, K.; Murakami, M.; Katagiri, H. Characteristics of centrifugal pumps handling air-water mixtures and size of air bubbles in pump impellers. Bull. JSME 1985, 28, 2310–2318. [Google Scholar] [CrossRef]
- Chang, L.; Xu, Q.; Yang, C.; Su, X.; Wang, H.; Guo, L. Experimental study on gas–liquid flow patterns and bubble size in a high-speed rotating impeller of a three-stage centrifugal pump. Exp. Therm. Fluid Sci. 2023, 145, 110896. [Google Scholar] [CrossRef]
- Cubas, J.M.; Stel, H.; Ofuchi, E.M.; Marcelino Neto, M.A.; Morales, R.E. Visualization of two-phase gas-liquid flow in a radial centrifugal pump with a vaned diffuser. J. Pet. Sci. Eng. 2020, 187, 106848. [Google Scholar] [CrossRef]
- Barrios, L.; Prado, M.G. Modeling Two-Phase Flow Inside an Electrical Submersible Pump Stage. J. Energy Resour. Technol. 2011, 133, 042902. [Google Scholar] [CrossRef]
- Zhu, J.; Zhang, H.Q. CFD simulation of ESP performance and bubble size estimation under gassy conditions. In Proceedings of the SPE Annual Technical Conference and Exhibition SPE 2014, Amsterdam, The Netherlands, 27–29 October 2014; p. SPE-170727. [Google Scholar]
- Hundshagen, M.; Rave, K.; Nguyen, B.D.; Popp, S.; Hasse, C.; Mansour, M.; Thévenin, D.; Skoda, R. Two-phase flow simulations of liquid/gas transport in radial centrifugal pumps with special emphasis on the transition from bubbles to adherent gas accumulations. J. Fluids Eng. 2022, 144, 101202. [Google Scholar] [CrossRef]
- Hundshagen, M.; Mansour, M.; Thévenin, D.; Skoda, R. Numerical investigation of two-phase air-water flow in a centrifugal pump with closed or semi-open impeller. In Proceedings of the 13th European Turbomachinery Conference on Turbomachinery Fluid Dynamics and Thermodynamics, ETC13 2019, Lausanne, Switzerland, 8–12 April 2019; Volume 13. [Google Scholar]
- Hundshagen, M.; Mansour, M.; Thévenin, D.; Skoda, R. Experimental investigation and 3D-CFD simulation of centrifugal pumps for gas-laden liquids with closed and semi-open impeller. In Proceedings of the 4th International Rotating Equipment Conference 2019, Wiesbaden, Germany, 24–25 September 2019. [Google Scholar]
- Yan, S.; Sun, S.; Luo, X.; Chen, S.; Li, C.; Feng, J. Numerical investigation on bubble distribution of a multistage centrifugal pump based on a population balance model. Energies 2020, 13, 908. [Google Scholar] [CrossRef]
- Schiavello, B. Two-Phase Flow Rotodynamic Pumps-Experiments and Design Criteria. Pumps Offshore, Course; Number 7133; Worthing ton Simson Ltd.: Newark, UK, 1986. [Google Scholar]
- Gülich, J.F. Centrifugal Pumps; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Trevisan, F.E. Modeling and Visualization of Air and Viscous Liquid in Electrical Submersible Pump. Ph.D. Thesis, Department of Petroleum Engineering, University of Tulsa, Tulsa, OK, USA, 2009. [Google Scholar]
- Trevisan, F.E.; Prado, M. Experimental investigation of the viscous effect on two-phase-flow patterns and hydraulic performance of electrical submersible pumps. J. Can. Pet. Technol. 2011, 50, 45–52. [Google Scholar] [CrossRef]
- Banjar, H.M.; Gamboa, J.; Zhang, H.Q. Experimental study of liquid viscosity effect on two-phase stage performance of electrical submersible pumps. In Proceedings of the SPE Annual Technical Conference and Exhibition. Society of Petroleum Engineers 2013, New Orleans, LA, USA, 30 September–2 October 2013. [Google Scholar]
- Paternost, G.M.; Bannwart, A.C.; Estevam, V. Experimental study of a centrifugal pump handling viscous fluid and two-phase flow. SPE Prod. Oper. 2015, 30, 146–155. [Google Scholar] [CrossRef]
- Stepanoff, A.J. Pumps and Blowers: Two-Phase Flow; J. Wiley & Sons: Hoboken, NJ, USA, 1965. [Google Scholar]
- Turpin, J.L.; Lea, J.F.; Bearden, J.L. Gas-liquid flow through centrifugal pumps—Correlation of data. In Proceedings of the 3rd International Pump Symposium Turbomachinery Laboratories, Houston, TX, USA, 1986; pp. 13–20. [Google Scholar]
- Gamboa, J. Prediction of the Transition in Two-Phase Performance of an Electrical Submersible Pump. Ph.D. Thesis, Department of Petroleum Engineering, University of Tulsa, Tulsa, OK, USA, 2008. [Google Scholar]
- Zhu, J.; Zhu, H.; Zhang, J.; Zhang, H.Q. An experimental study of surfactant effect on gas tolerance in electrical submersible pump (ESP). In Proceedings of the ASME 2017 International Mechanical Engineering Congress Exposition, Tampa, FL, USA, 3–9 November 2017; p. V007T09A038. [Google Scholar]
- Kosmowski, I.; Hergt, P. Förderung gasbeladener Medien mit Hilfe von Normal-und Sonderausführungen von Kreiselpumpen. KSB Technical Report 26, 1990.
- Thum, D. Untersuchung von Homogenisierungseinrichtungen auf das Förderverhalten Radialer Kreiselpumpen bei Gasbeladenen Strömungen. Ph.D. Thesis, Technische Universität Kaiserslautern, Kaiserslautern, Germany, 2007. [Google Scholar]
- Barrios, L. Visualization and Modeling of Multiphase Performance inside an Electrical Submersible Pump. Ph.D. Thesis, Department of Petroleum Engineering, University of Tulsa, Tulsa, OK, USA, 2007. [Google Scholar]
- Barrios, L.; Prado, M.G. Experimental visualization of two-phase flow inside an electrical submersible pump stage. J. Energy Resour. Technol. 2011, 133, 042901. [Google Scholar] [CrossRef]
- Zhang, J.; Cai, S.; Li, Y.; Zhu, H.; Zhang, Y. Visualization study of gas–liquid two-phase flow patterns inside a three-stage rotodynamic multiphase pump. Exp. Therm. Fluid Sci. 2016, 70, 125–138. [Google Scholar] [CrossRef]
- Shao, C.; Li, C.; Zhou, J. Experimental investigation of flow patterns and external performance of a centrifugal pump that transports gas-liquid two-phase mixtures. Int. J. Heat Fluid Flow 2018, 71, 460–469. [Google Scholar] [CrossRef]
- Schäfer, T.; Bieberle, A.; Neumann, M.; Hampel, U. Application of gamma-ray computed tomography for the analysis of gas holdup distributions in centrifugal pumps. Flow Meas. Instrum. 2015, 46, 262–267. [Google Scholar] [CrossRef]
- Neumann, M.; Schäfer, T.; Bieberle, A.; Hampel, U. An experimental study on the gas entrainment in horizontally and vertically installed centrifugal pumps. J. Fluids Eng. 2016, 138, 91301. [Google Scholar] [CrossRef]
- Murakami, M.; Minemura, K.; Takimoto, M. Effects of entrained air on the performance of centrifugal pumps under cavitating conditions. Bull. JSME 1980, 23, 1435–1442. [Google Scholar] [CrossRef]
- Sekoguchi, K.; Takada, S.; Kanemori, Y. Study of air-water two-phase centrifugal pump by means of electric resistivity probe technique for void fraction measurement: 1st report, measurement of void fraction distribution in a radial flow impeller. Bull. JSME 1984, 27, 931–938. [Google Scholar] [CrossRef]
- Sato, S.; Furukawa, A.; Takamatsu, Y. Air-water two-phase flow performance of centrifugal pump impellers with various blade angles. JSME Int. J. Ser. B Fluids Therm. Eng. 1996, 39, 223–229. [Google Scholar] [CrossRef]
- Zhu, J. Experiments, CFD Simulation and Modeling of ESP Performance under Gassy Conditions. Ph.D. Thesis, Department of Petroleum Engineering, University of Tulsa, Tulsa, OK, USA, 2017. [Google Scholar]
- Mansour, M. Transport of Two-Phase Air-Water Flows in Radial Centrifugal pumps. Ph.D. Thesis, University of Magdeburg, Magdeburg, Germany, 2020. [Google Scholar]
- Sterrett, J.D. An Experimental and Analytical Investigation into the Performance of Centrifugal Pumps Operating with Air-Water Mixtures. Ph.D. Thesis, Auburn University, Auburn, AL, USA, 1994. [Google Scholar]
- Serena, A. A Multiphase Pump Experimental Analysis. Ph.D. Thesis, Norwegian University of Science and Technology, Trondheim, Norway, 2016. [Google Scholar]
- Ge, Z.; He, D.; Huang, R.; Zuo, J.; Luo, X. Application of CFD-PBM coupling model for analysis of gas-liquid distribution characteristics in centrifugal pump. J. Pet. Sci. Eng. 2020, 194, 107518. [Google Scholar] [CrossRef]
- Murakami, M.; Minemura, K. Effects of entrained air on the performance of centrifugal pumps: 2nd report, effects of number of blades. Bull. JSME 1974, 17, 1286–1295. [Google Scholar] [CrossRef]
- Merry, H. Effects of two-phase liquid/gas flow on the performance of centrifugal pumps. In Proceedings of the Pumps Compressors Offshore Oil Gas, IMechE Conference C130/76, London, UK, 1976; p. 61. [Google Scholar]
- Furukawa, A.; Kuwano, T.; Okuma, K. Air-water two-phase flow performance of centrifugal pump with tandem circular cascades. Proc. JSME ICFE 1997, 97, 479–484. [Google Scholar]
- Furukawa, A.; Shirasu, S.; Sato, S. Experimental study of gas-liquid two-phase flow pumping action of centrifugal impeller. Proc. ASME Conf. Fed 1995, 226, 89–96. [Google Scholar]
- Mansour, M.; Kopparthy, S.B.; Thévenin, D. Improving air-water two-phase flow pumping in centrifugal pumps using novel grooved front shrouds. Chem. Eng. Res. Des. 2023, 197, 173–191. [Google Scholar] [CrossRef]
- Mansour, M.; Parikh, T.; Engel, S.; Thévenin, D. Numerical investigations of gas-liquid two-phase flow in a pump inducer. J. Fluids Eng. 2019, 142, 021302. [Google Scholar] [CrossRef]
- Mansour, M.; Parikh, T.; Thévenin, D. Influence of blade pitch and number of blades of a pump inducer on single and two-phase flow performance. In Proceedings of the ASME Turbo Expo 2020: Turbomachinery Technical Conference and Exposition. American Society of Mechanical Engineers, London, UK, 21–25 June 2020; Volume 9, p. V009T21A010. [Google Scholar]
- Moffat, R.J. Describing the uncertainties in experimental results. Exp. Therm. Fluid Sci. 1988, 1, 3–17. [Google Scholar] [CrossRef]
- Soave, G. Equilibrium constants from a modified Redlich-Kwong equation of state. Chem. Eng. Sci. 1972, 27, 1197–1203. [Google Scholar] [CrossRef]
- Peng, D.Y.; Robinson, D.B. A new two-constant equation of state. Ind. Eng. Chem. Fund. 1976, 15, 59–64. [Google Scholar] [CrossRef]
- Guo, X.M.; Zhu, Z.C.; Shi, G.P.; Huang, Y. Effects of rotational speeds on the performance of a centrifugal pump with a variable-pitch inducer. J. Hydrodyn. Ser. B 2017, 29, 854–862. [Google Scholar] [CrossRef]
- Moore, J. A Wake and an Eddy in a Rotating, Radial-Flow Passage—Part 1: Experimental Observations. J. Eng. Power 1973, 95, 205–212. [Google Scholar] [CrossRef]
















| Parameter | Symbol | Value |
|---|---|---|
| Impeller outer to inlet diameter ratio | ||
| Blade thickness | E | 6 mm |
| Blade inlet angle | ||
| Blade outlet angle | ||
| Inducer hub to tip diameter ratio | % | |
| Inducer hub solidity | ||
| Inducer tip solidity | ||
| Inducer area solidity | % | |
| Inducer hub blade angle | 58 | |
| Inducer tip blade angle | 34 | |
| Inducer sweep angle | ||
| Inducer hub blade thickness | % | |
| Inducer tip blade thickness | % | |
| Inducer blade axial length | ||
| Standard tip clearance gap to blade outlet width | ||
| Increased tip clearance gap to blade outlet width | ||
| Inducer tip clearance gap to blade outlet width |
| Device | Model | Uncertainty |
|---|---|---|
| Water volume flow meter | Endress+Hauser Promag | RD |
| Air mass flow meter | Bronkhorst F-113AC-HD-55-V | RD plus FS |
| Differential pressure sensor | Deltabar M PMD55 (−3:+3 bar) | FS |
| Suction pressure sensor | Sensotec Z | FS |
| Suction temperature sensor | Pt100 Sensor Probe, Class B | K (max. absolute error) |
| Torque transducer | HBM T1 | FS |
| Analog tachometer | TDP 0.2 LT-4 | RD |
| # | Impeller Type | Tip Clearance Gap | Blade Trailing Edge | With Inducer | Rotational Speed |
|---|---|---|---|---|---|
| 1 | Closed | No gap | Round | No | 650 rpm |
| 2 | Semi-open | Standard gap | Round | No | 650 rpm |
| 3 | Semi-open | Increased gap | Round | No | 650 rpm |
| 4 | Semi-open | Standard gap | Round | Yes | 650 rpm |
| 5 | Semi-open | Standard gap | Trimmed | No | 650 rpm |
| 6 | Semi-open | Standard gap | Trimmed | No | 1000 rpm |
| Parameter | Range | Unit |
|---|---|---|
| Normalized water volume flow rate, | 0.1–1.6 | − |
| Gas volume fraction, | 0–15 | % |
| Suction pressure, | 1.06–1.28 | |
| Suction temperature, T | ||
| Rotational speed, n | ||
| Rotational Reynolds number of water at 650 , Re | 9,181,340 | − |
| Rotational Reynolds number of water at 1000 , Re | 14,125,138 | − |
| Density ratio, | 670–810 | − |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Mansour, M.; Thévenin, D. State of the Art on Two-Phase Non-Miscible Liquid/Gas Flow Transport Analysis in Radial Centrifugal Pumps Part B: Review of Experimental Investigations. Int. J. Turbomach. Propuls. Power 2023, 8, 42. https://doi.org/10.3390/ijtpp8040042
Mansour M, Thévenin D. State of the Art on Two-Phase Non-Miscible Liquid/Gas Flow Transport Analysis in Radial Centrifugal Pumps Part B: Review of Experimental Investigations. International Journal of Turbomachinery, Propulsion and Power. 2023; 8(4):42. https://doi.org/10.3390/ijtpp8040042
Chicago/Turabian StyleMansour, Michael, and Dominique Thévenin. 2023. "State of the Art on Two-Phase Non-Miscible Liquid/Gas Flow Transport Analysis in Radial Centrifugal Pumps Part B: Review of Experimental Investigations" International Journal of Turbomachinery, Propulsion and Power 8, no. 4: 42. https://doi.org/10.3390/ijtpp8040042
APA StyleMansour, M., & Thévenin, D. (2023). State of the Art on Two-Phase Non-Miscible Liquid/Gas Flow Transport Analysis in Radial Centrifugal Pumps Part B: Review of Experimental Investigations. International Journal of Turbomachinery, Propulsion and Power, 8(4), 42. https://doi.org/10.3390/ijtpp8040042







