Rotationally Induced Local Heat Transfer Features in a Two-Pass Cooling Channel: Experimental–Numerical Investigation †
Abstract
:1. Introduction
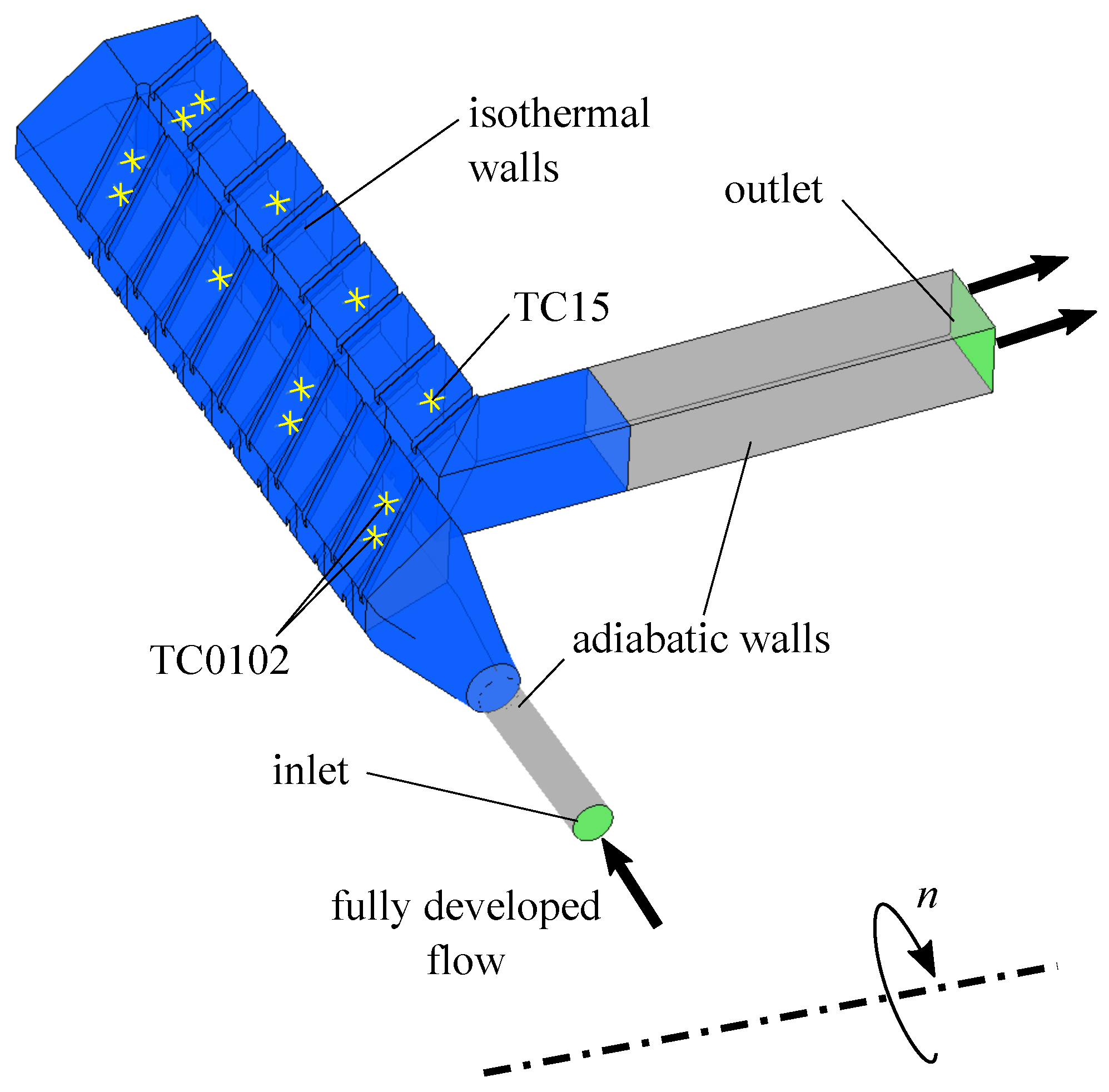
2. Test Model
3. Experimental Setup
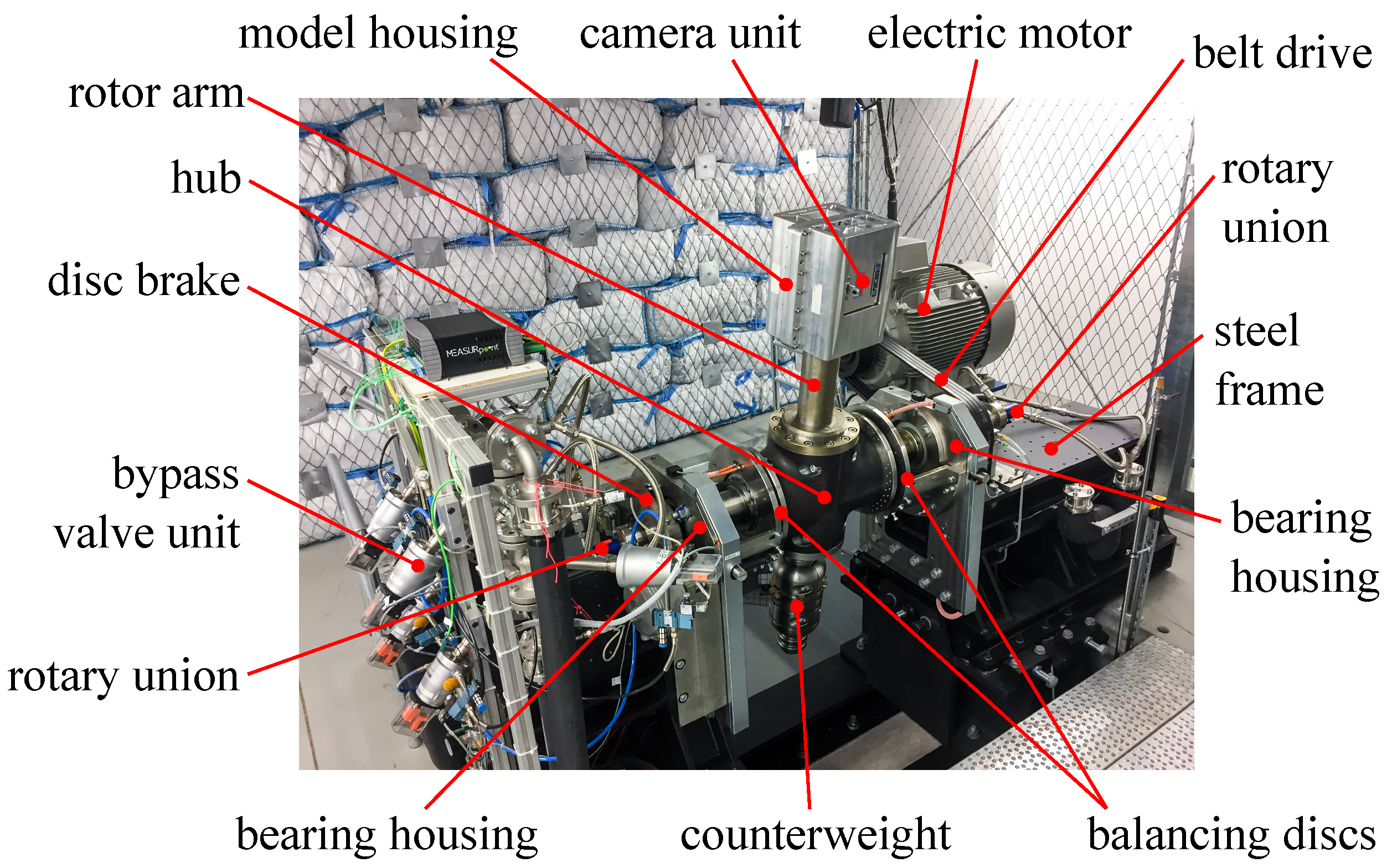
3.1. Rotor
3.2. Rotating Camera Unit
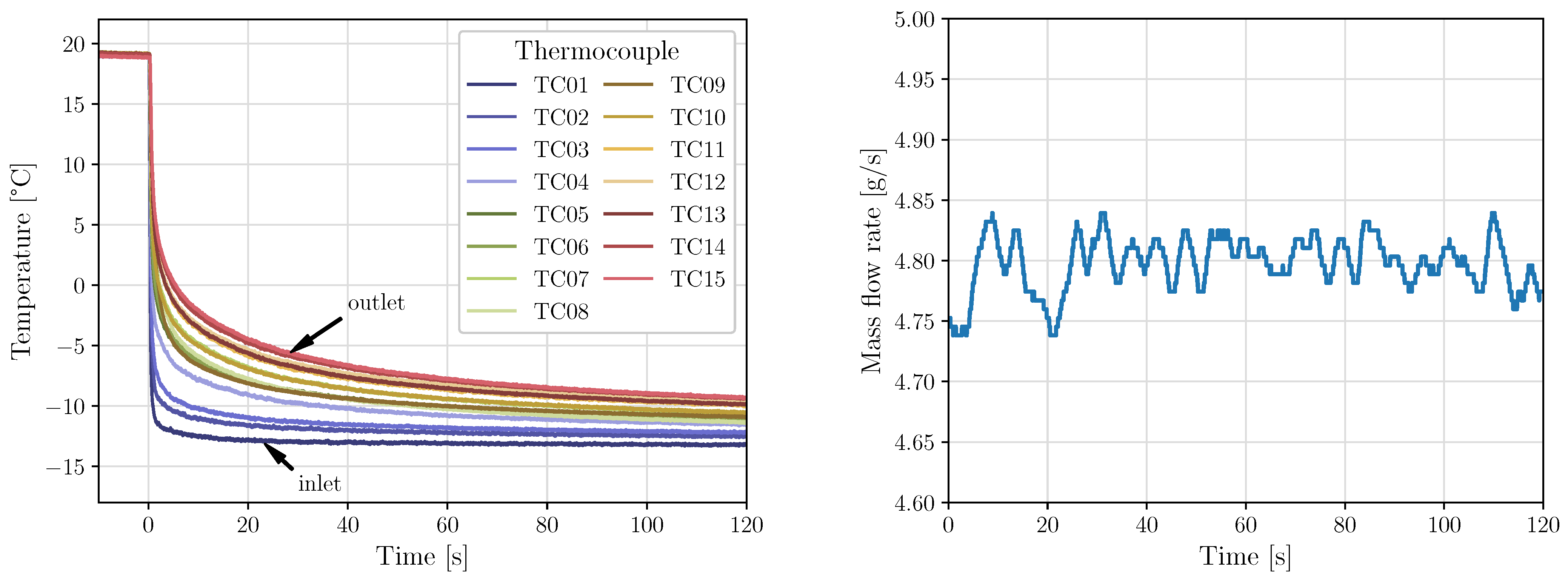
3.3. Fluid Temperature Change
3.4. Wall Temperature Change
4. Experimental Data Acquisition and Synchronization
5. Experimental Data Analysis
6. Dimensionless Quantities
7. Numerical Setup
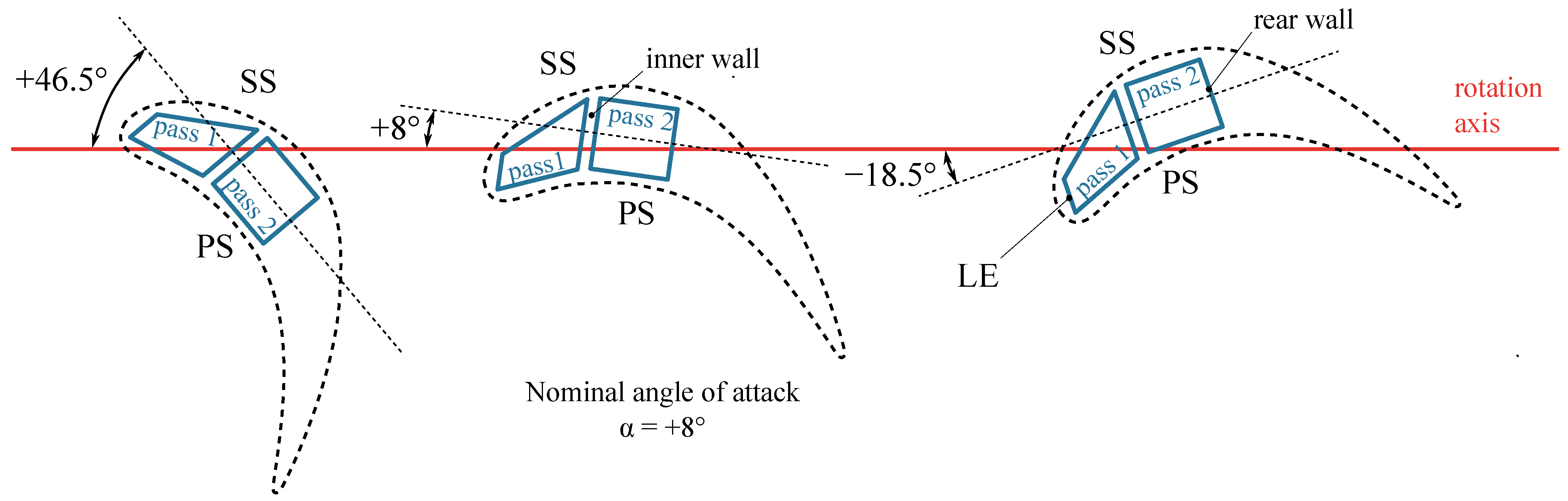
7.1. Angles of Attack
7.2. Boundary Conditions
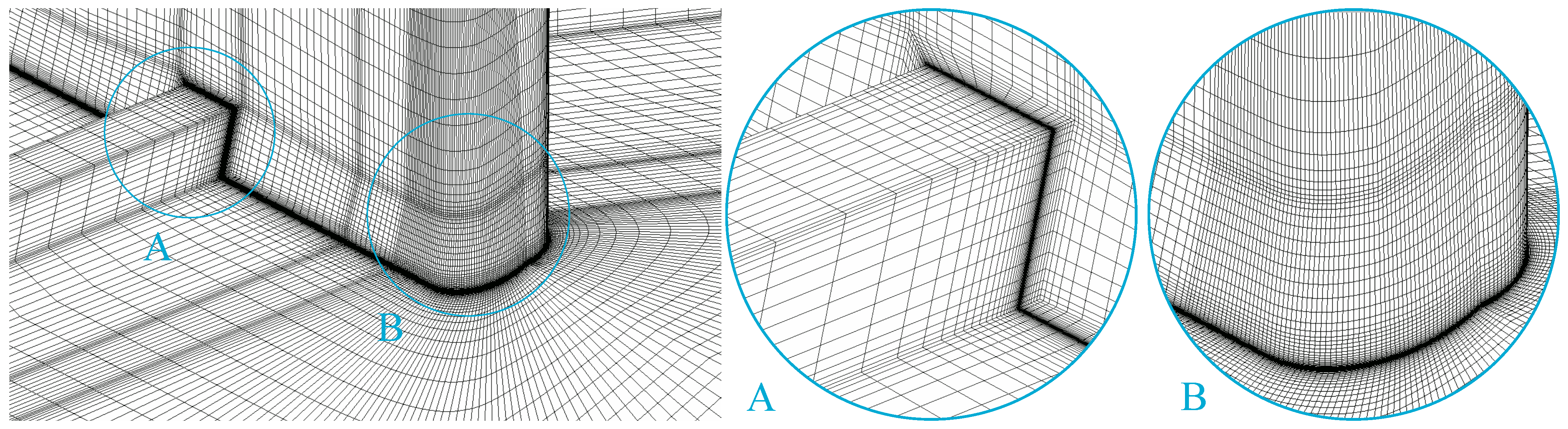
7.3. Computational Grid
8. Numerical Heat Transfer Evaluation
9. Results and Discussion
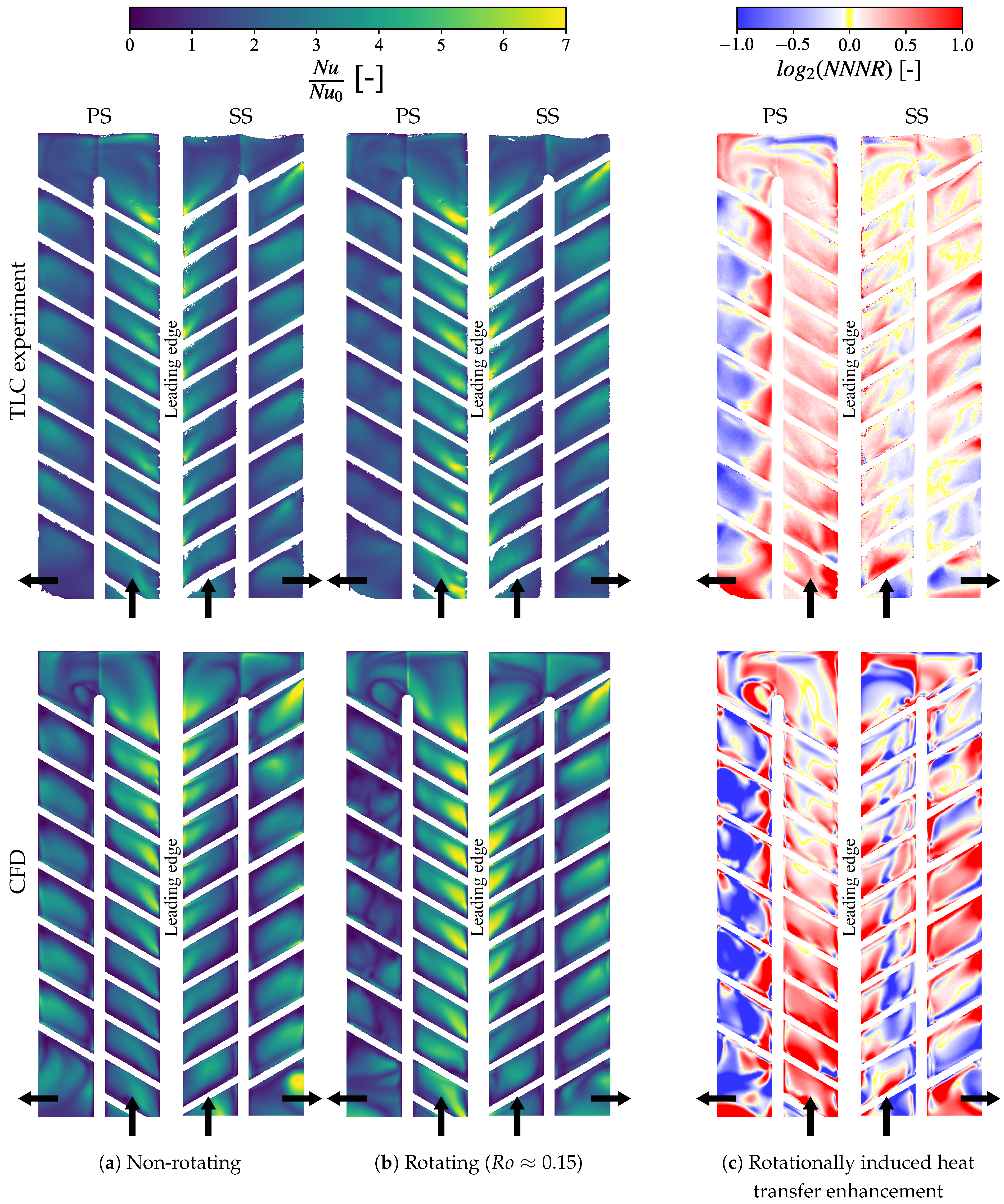
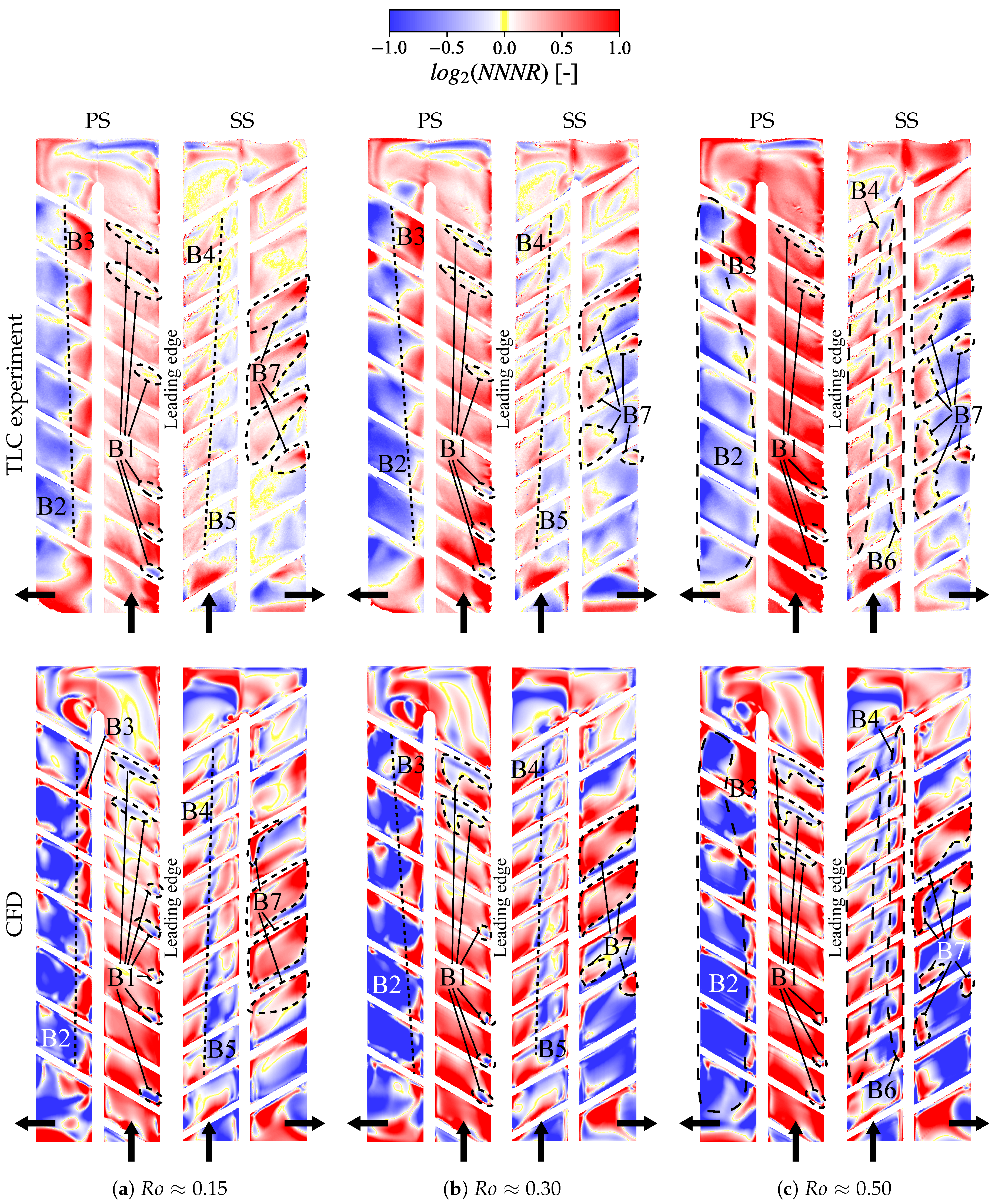
9.1. General Heat Transfer Characteristics
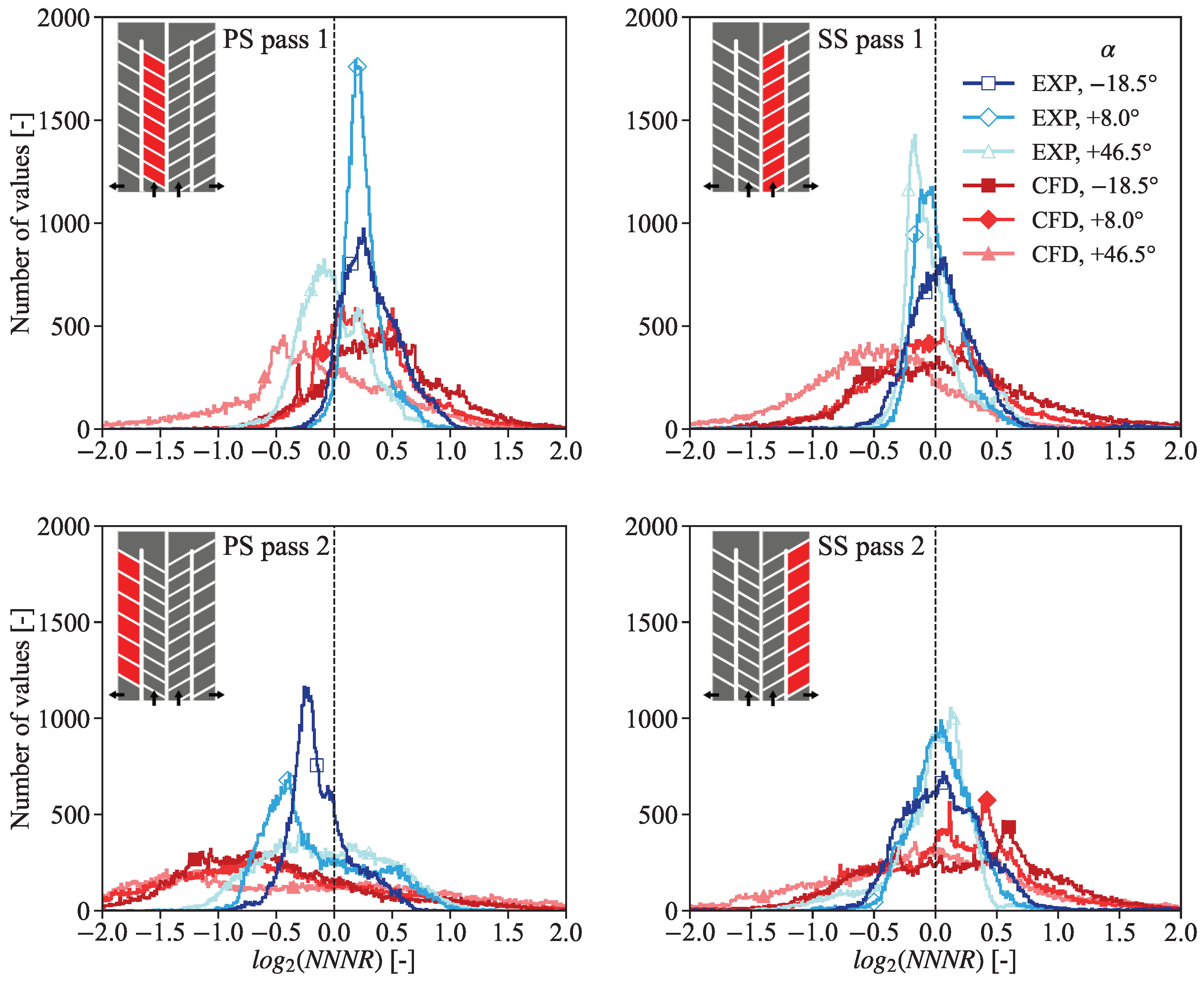
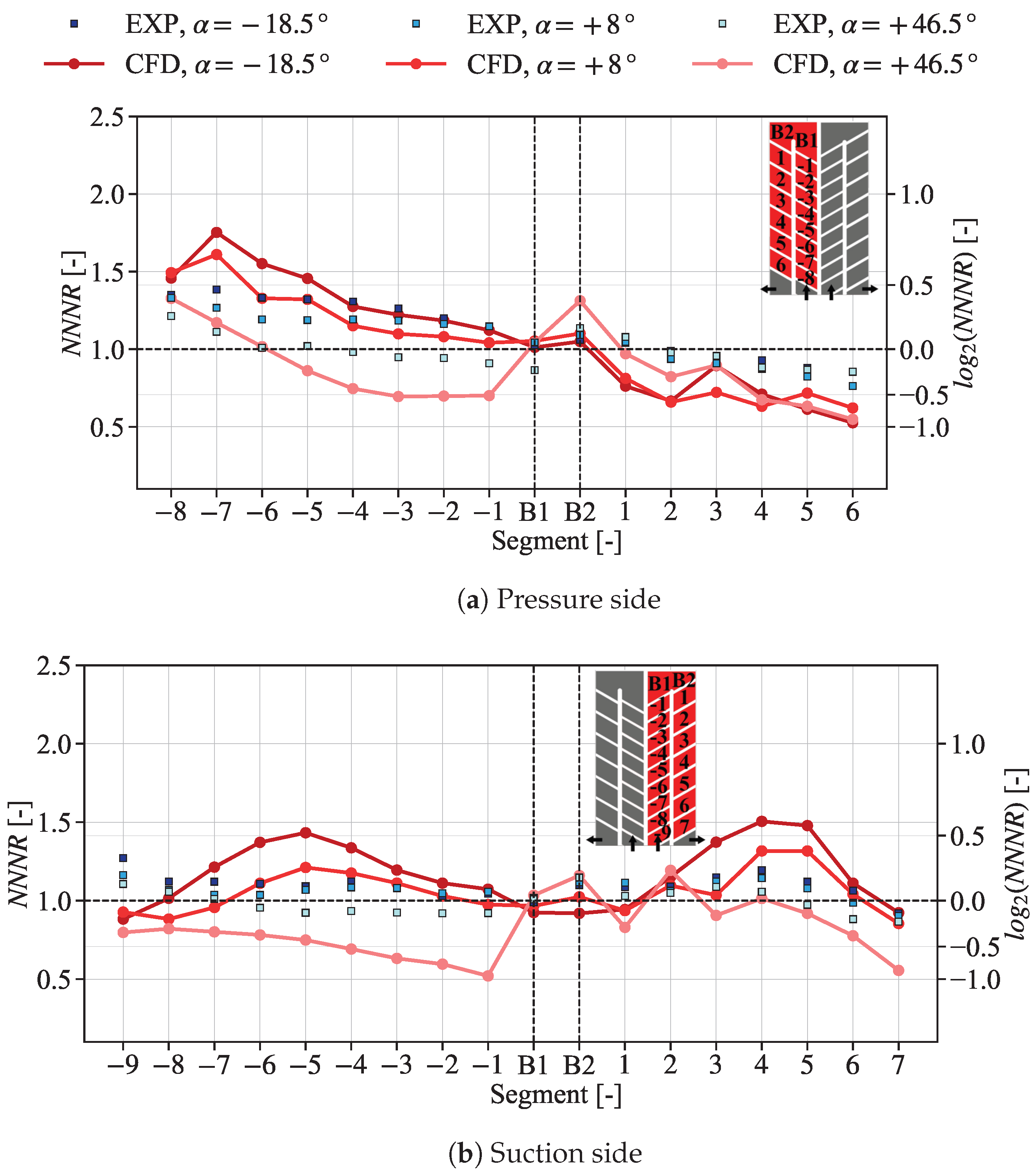
9.2. Numerical Validation at Different Angles of Attack
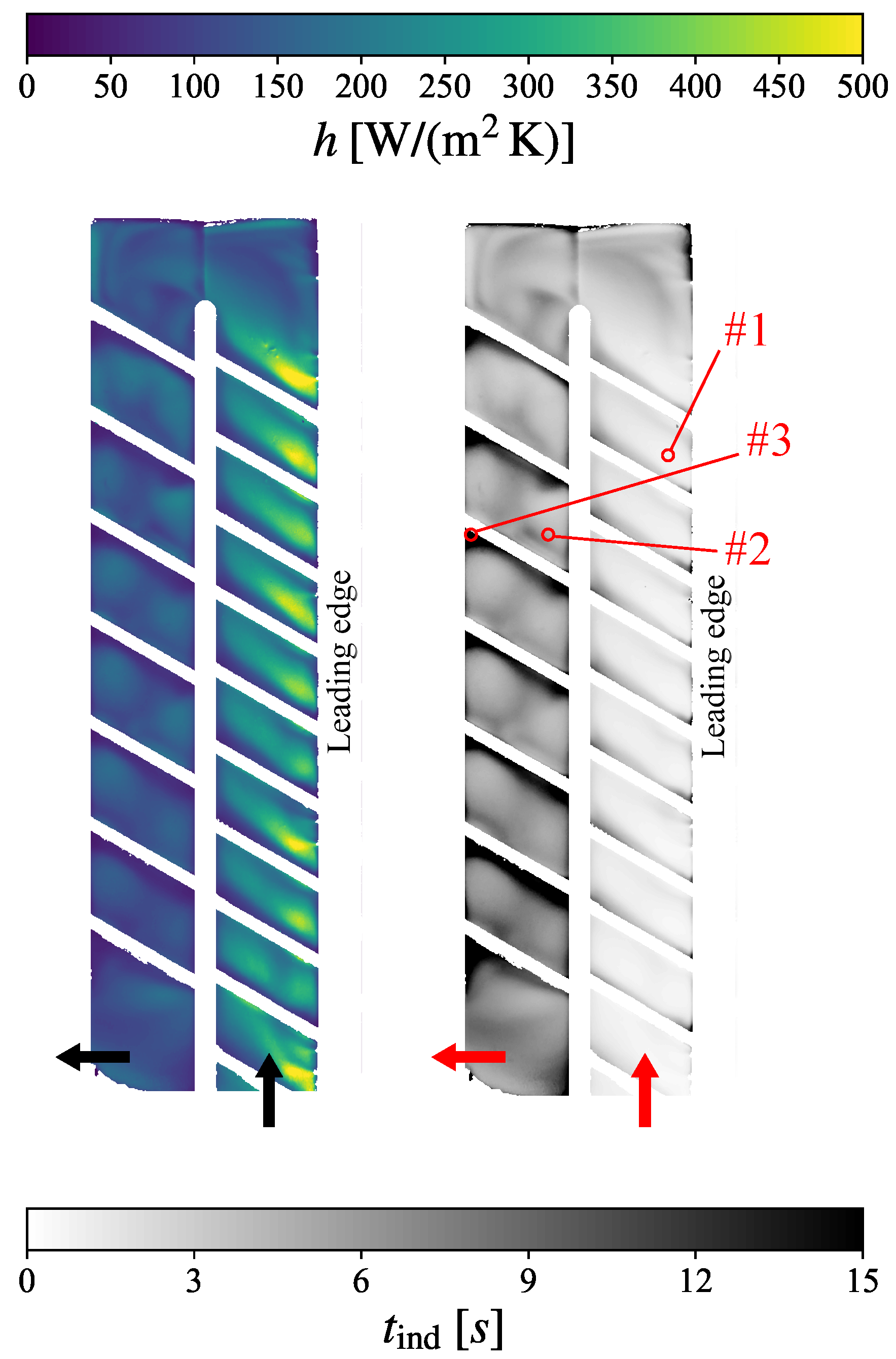
- Experimental uncertainty: In order to assess the uncertainty of a typical TLC experiment, a Monte Carlo analysis was performed on three different representative pixels, which is presented in Appendix A. Results disclosed local deviations up to 16.6% in the heat transfer coefficient (h) and up to 22.2% in the normalized Nusselt number ratio (NNNR).
- Lateral heat conduction effects present in the TLC experiment, as mentioned in the beginning of Section 9.2. The estimation of this experimental error is far from trivial, since there exist no simple analytical solutions for the transient three-dimensional heat conduction equation which can be applied here.
- Mismatch in the buoyancy number () between the transient TLC experiment and the steady-state numerical simulation. In order to fully address the effect of on the heat transfer rates, a computationally expensive transient conjugated CFD simulation would be required. On average, however, the time-averaged for the experiment and numerical simulation are well aligned, as reflected in Table A3.
- Numerical modeling error and discretization error. The latter effect was quantified through a mesh independence study (not reported here) and resulted in uncertainties of up to 6% for the PS and 3% for the SS.
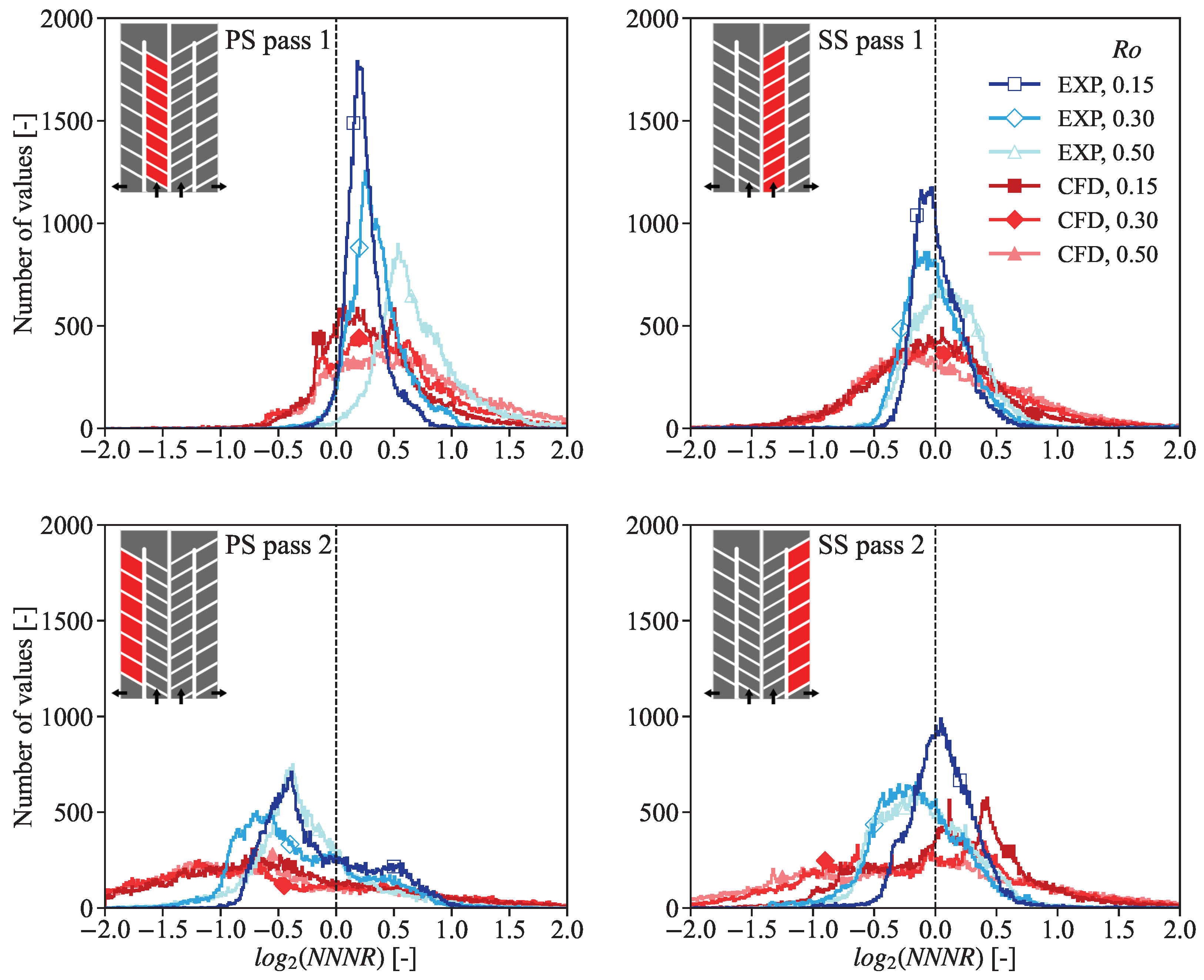
9.3. Rotational Effect
10. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature and Abbreviations
| Roman characters | |
| A | Cross-sectional area of the channel, |
| Specific heat capacity, J/(kgK) | |
| Hydraulic diameter of pass 1, | |
| h | Heat transfer coefficient, W/(m2K) |
| k | Thermal conductivity, W/(mK) |
| Mass flow rate, kg/s | |
| n | Rotational speed, rpm |
| p | Pressure, bar |
| Specific heat flux, / | |
| Mean model radius, | |
| t | Time, |
| T | Temperature, °C |
| Simensionless wall distance of the first cell, – | |
| Greek characters | |
| Angle of attack, ° | |
| Dynamic viscosity, kg/(ms) | |
| Density, / | |
| Time step, | |
| Rotational speed, / | |
| Dimensionless parameters | |
| Buoyancy number, – | |
| NNNR | Normalized Nusselt number ratio, – |
| Nusselt number, – | |
| Nusselt number from Dittus–Boelter correlation, – | |
| Prandtl number, – | |
| Reynolds number, – | |
| Rotation number, – | |
| Subscripts | |
| 0 | Initial |
| f | Fluid |
| ind | Indication |
| in | Inlet, i.e., at thermocouple position TC0102 |
| out | Outlet, i.e., at thermocouple position TC15 |
| ref | Reference |
| ROT | Rotating |
| STAT | Stationary, i.e., non-rotating |
| sync | Synchronization |
| w | Wall |
| The following abbreviations are used in this manuscript: | |
| CFD | Computational fluid dynamics |
| DNS | Direct numerical simulation |
| EXP | Experimental |
| IR | Infrared |
| LES | Large eddy simulation |
| M | Number of trials for Monte Carlo analysis |
| MC | Monte Carlo |
| NUM | Numerical |
| pass 1 | First/inlet passage |
| pass 2 | Second/outlet passage |
| PMMA | Acrylic glass (polymethyl methacrylate) |
| PS | Pressure side |
| RANS | Reynolds-averaged Navier–Stokes |
| SS | Suction side |
| SST | Shear stress transport |
| TC | Thermocouple |
| TLC | Thermochromic liquid crystal |
| URANS | Unsteady Reynolds-averaged Navier–Stokes |
Appendix A. Experimental Uncertainty Analysis
| Nominal Value | Source | Confidence Interval | ||||
|---|---|---|---|---|---|---|
| 0 | Rectangular | Camera frame rate ( 30 fps) | 100% | |||
| Pixel-dependent | Normal | Signal processing | Signal analysis | 95% | ||
| Pixel-dependent | Normal | TC reading and CFD according to [16] | 95% | |||
| 11.1 °C | Normal | TLC calibration | 95% | |||
| Normal | Material properties | 95% | ||||
| Normal | Material properties | 95% | ||||
| Normal | Material properties | 95% | ||||
| # | Pass | tind [s] | h [W/(m2K)] | Uh/h | tind [s] | h [W/(m2K)] | Uh/h | NNNR [−] | UNNNR/NNNR |
|---|---|---|---|---|---|---|---|---|---|
| 1 | pass 1 | +17.1% | 0.76 | 485.7 | +16.6% | 1.30 | +22.2% | ||
| −13.3% | −13.0% | −19.2% | |||||||
| 2 | pass 2 | 10.19 | 86.2 | +6.65% | 4.38 | 154.0 | +6.57% | 1.76 | +9.37% |
| −6.34% | −6.21% | −8.75% | |||||||
| 3 | pass 2 | 20.49 | 52.4 | +7.37% | 24.87 | 43.3 | +6.06% | 0.83 | +9.55% |
| −6.97% | −5.80% | −8.95% | |||||||
Appendix B. Actual Dimensionless Quantities
| TLC Experiment | CFD | |||||||
|---|---|---|---|---|---|---|---|---|
| Parameter | Target Value | Angle: | +46.5° | +8° | −18.5° | +46.5° | +8° | −18.5° |
| 15,000 | 14,915 | 14,910 | 14,821 | 14,832 | 14,890 | 14,913 | ||
| 14,881 | 15,106 | 14,836 | 14,843 | 15,098 | 14,891 | |||
| 14,964 | 14,967 | |||||||
| 14,634 | 14,612 | |||||||
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | ||
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | ||
References
- Han, J.C. Fundamental Gas Turbine Heat Transfer. J. Therm. Sci. Eng. Appl. 2013, 5, 021007. [Google Scholar] [CrossRef]
- Ligrani, P. Heat Transfer Augmentation Technologies for Internal Cooling of Turbine Components of Gas Turbine Engines. Int. J. Rotating Mach. 2013, 2013, 275653. [Google Scholar] [CrossRef]
- Wagner, J.H.; Johnson, B.V.; Hajek, T.J. Heat Transfer in Rotating Passages with Smooth Walls and Radial Outward Flow. J. Turbomach. 1991, 113, 42–51. [Google Scholar] [CrossRef]
- Johnson, B.; Wagner, J.; Steuber, G.; Yeh, F. Heat transfer in rotating serpentine passages with selected model orientation for smooth or skewed trip walls. Int. J. Turbomach. 1994, 116, 738–744. [Google Scholar] [CrossRef]
- Zhou, F.; Lagrone, J.; Acharya, S. Internal Cooling in 4:1 AR Passages at High Rotation Numbers. In Turbo Expo: Power for Land, Sea, and Air; American Society of Mechanical Engineers: New York, NY, USA, 2004; pp. 451–460. [Google Scholar] [CrossRef]
- Chang, S.W.; Yang, T.L.; Wang, W.J. Heat Transfer in a Rotating Twin-Pass Trapezoidal-Sectioned Passage Roughened by Skewed Ribs on Two Opposite Walls. Heat Transf. Eng. 2006, 27, 63–79. [Google Scholar] [CrossRef]
- Huh, M.; Lei, J.; Liu, Y.H.; Han, J.C. High Rotation Number Effects on Heat Transfer in a Rectangular (AR = 2:1) Two-Pass Channel. J. Turbomach. 2010, 133, 021001. [Google Scholar] [CrossRef]
- Wright, L.M.; Yang, S.F.; Wu, H.W.; Han, J.C.; Lee, C.P.; Azad, S.; Um, J. Heat Transfer in a Rotating, Blade-Shaped Serpentine Cooling Passage With Discrete Ribbed Walls at High Reynolds Numbers. J. Heat Transf. 2019, 142, 012002. [Google Scholar] [CrossRef]
- Ekkad, S.V.; Singh, P. Detailed Heat Transfer Measurements for Rotating Turbulent Flows in Gas Turbine Systems. Energies 2021, 14, 39. [Google Scholar] [CrossRef]
- Ekkad, S.V.; Singh, P. Liquid Crystal Thermography in Gas Turbine Heat Transfer: A Review on Measurement Techniques and Recent Investigations. Crystals 2021, 11, 1332. [Google Scholar] [CrossRef]
- Lorenzon, A.; Casarsa, L. Validation of the Transient Liquid Crystal Thermography Technique for Heat Transfer Measurements on a Rotating Cooling Passage. Energies 2020, 13, 4759. [Google Scholar] [CrossRef]
- Waidmann, C.; Poser, R.; Nieland, S.; von Wolfersdorf, J. Design of a Rotating Test Rig for Transient Thermochromic Liquid Crystal Heat Transfer Experiments. In Proceedings of the ISROMAC 2016, International Symposium on Transport Phenomena and Dynamics of Rotating Machinery, Honolulu, HI, USA, 10–15 April 2016; Available online: https://hal.science/hal-01884257v1 (accessed on 20 November 2023).
- Waidmann, C.; Poser, R.; Göhring, M.; von Wolfersdorf, J. First Operation of a Rotating Test Rig for Transient Thermochromic Liquid Crystal Heat Transfer Experiments. In Proceedings of the XXIV Biannual Symposium on Measuring Techniques in Turbomachinery Transonic and Supersonic Flow in Cascades and Turbomachines, Prague, Czech Republic, 30–31 August 2018; Available online: https://www.meastechturbo.com/paper-archives/item/344-first-operation-of-a-rotating-test-rig-for-transient-thermochromic-liquid-crystal-heat-transfer-experiments (accessed on 20 November 2023).
- Waidmann, C. Heat Transfer Measurements in Rotating Turbine Blade Cooling Channel Configurations Using the Transient Thermochromic Liquid Crystal Technique. Ph.D. Thesis, University of Stuttgart, Stuttgart, Germany, 2020. Available online: https://elib.uni-stuttgart.de/handle/11682/11344 (accessed on 20 November 2023).
- Waidmann, C.; Poser, R.; Gutiérrez de Arcos, D.; Göhring, M.; von Wolfersdorf, J.; Semmler, K.; Jäppelt, B. Experimental Investigation of Local Heat Transfer in a Rotating Two-Pass Cooling Channel Using the Transient Thermochromic Liquid Crystal (TLC) Technique. In Proceedings of the ASME Turbo Expo 2022: Turbomachinery Technical Conference and Exposition, Rotterdam, The Netherlands, 13–17 June 2022; Volume 6B: Heat Transfer—General Interest/Additive Manufacturing Impacts on Heat Transfer; Internal Air Systems; Internal Cooling, p. V06BT15A009. Available online: https://asmedigitalcollection.asme.org/GT/proceedings-abstract/GT2022/86045/V06BT15A009/1149022 (accessed on 20 November 2023).
- Gutiérrez de Arcos, D.; Waidmann, C.; Poser, R.; von Wolfersdorf, J.; Jäppelt, B. Comparison of Experimental and Numerical Local Rotational Heat Transfer Effects in a Two-Pass Cooling Channel Configuration. In Proceedings of the ASME Turbo Expo 2022: Turbomachinery Technical Conference and Exposition, Rotterdam, The Netherlands, 13–17 June 2022; Volume 6B: Heat Transfer—General Interest/Additive Manufacturing Impacts on Heat Transfer; Internal Air Systems; Internal Cooling. p. V06BT15A008. Available online: https://asmedigitalcollection.asme.org/GT/proceedings-abstract/GT2022/86045/V06BT15A008/1148975 (accessed on 20 November 2023).
- Gutiérrez de Arcos, D.; Waidmann, C.; Poser, R.; von Wolfersdorf, J.; Jäppelt, B.; Semmler, K. Effect of protrusions and leading edge ribs on the local heat transfer characteristics in a two-pass cooling channel under rotation. In Proceedings of the GPPS Technical Conference, Chania, Greece, 4–6 September 2024; Available online: https://www.researchgate.net/publication/384361224_Effect_of_protrusions_and_leading_edge_ribs_on_the_local_heat_transfer_characteristics_in_a_two-pass_cooling_channel_under_rotation (accessed on 10 December 2023).
- Pagnacco, F.; Furlani, L.; Armellini, A.; Casarsa, L.; Davis, A. Rotating Heat Transfer Measurements on Realistic Multi-pass Geometry. Energy Procedia 2016, 101, 758–765. [Google Scholar] [CrossRef]
- Pearce, R.; Ireland, P.; He, L.; McGilvray, M.; Romero, E. Computational and Experimental Study of Heat Transfer in Rotating Ribbed Radial Turbine Cooling Passages. In Turbo Expo: Power for Land, Sea, and Air; American Society of Mechanical Engineers: New York, NY, USA, 2015; Volume 5A: Heat Transfer, p. V05AT11A026. Available online: https://asmedigitalcollection.asme.org/GT/proceedings-abstract/GT2015/56710/V05AT11A026/237166 (accessed on 20 November 2023).
- Massini, D.; Burberi, E.; Carcasci, C.; Cocchi, L.; Facchini, B.; Armellini, A.; Casarsa, L.; Furlani, L. Effect of rotation on a gas turbine blade internal cooling system: Experimental investigation. J. Eng. Gas Turbines Power 2017, 139, 101902. [Google Scholar] [CrossRef]
- Burberi, E.; Massini, D.; Cocchi, L.; Mazzei, L.; Andreini, A.; Facchini, B. Effect of rotation on a gas turbine blade internal cooling system: Numerical investigation. J. Turbomach. 2017, 139, 031005. [Google Scholar] [CrossRef]
- Duchaine, F.; Gicquel, L.; Grosnickel, T.; Koupper, C. Large-Eddy simulation of the flow developing in static and rotating ribbed channels. J. Turbomach. 2020, 142, 041003. [Google Scholar] [CrossRef]
- Singh, P.; Sarja, A.; Ekkad, S.V. Experimental and Numerical Study of Chord-Wise Eight-Passage Serpentine Cooling Design for Eliminating the Coriolis Force Adverse Effect on Heat Transfer. J. Therm. Sci. Eng. Appl. 2021, 13, 011026. [Google Scholar] [CrossRef]
- Murata, A.; Mochizuki, S. Effect of centrifugal buoyancy on turbulent heat transfer in an orthogonally rotating square duct with transverse or angled rib turbulators. Int. J. Heat Mass Transf. 2001, 44, 2739–2750. [Google Scholar] [CrossRef]
- Abdel-Wahab, S.; Tafti, D.K. Large eddy simulation of flow and heat transfer in a 90 deg ribbed duct with rotation: Effect of Coriolis and centrifugal buoyancy forces. J. Turbomach. 2004, 126, 627–636. [Google Scholar] [CrossRef]
- Sewall, E.A.; Tafti, D.K. Large Eddy Simulation of Flow and Heat Transfer in the Developing Flow Region of a Rotating Gas Turbine Blade Internal Cooling Duct With Coriolis and Buoyancy Forces. J. Turbomach. 2007, 130, 011005. [Google Scholar] [CrossRef]
- Saravani, M.S.; DiPasquale, N.J.; Beyhaghi, S.; Amano, R.S. Heat transfer in internal cooling channels of gas turbine blades: Buoyancy and density ratio effects. J. Energy Resour. Technol. 2019, 141, 112001. [Google Scholar] [CrossRef]
- Göhring, M.; Krille, T.; Feile, J.; von Wolfersdorf, J. Heat Transfer Predictions in Smooth and Ribbed Two-Pass Cooling Channels under Stationary and Rotating Conditions. In Proceedings of the 16th International Symposium on Transport Phenomena and Dynamics of Rotating Machinery, Honolulu, HI, USA, 10–15 April 2016; Available online: https://hal.science/hal-01884254 (accessed on 20 November 2023).
- Göhring, M.; Hartmann, C.; von Wolfersdorf, J. Numerical Investigation of Transient Heat Transfer Experiments Under Rotation. Turbo Expo: Power for Land, Sea, and Air; American Society of Mechanical Engineers: New York, NY, USA, 2018; Volume 5A: Heat Transfer, p. V05AT11A011. Available online: https://asmedigitalcollection.asme.org/GT/proceedings-abstract/GT2018/51081/V05AT11A011/272426 (accessed on 20 November 2023).
- Göhring, M. Numerical Investigation of Internal Two-Pass Gas Turbine Cooling Channels Under the Influence of Rotation. Ph.D. Thesis, University of Stuttgart, Stuttgart, Germany, 2020. Available online: https://elib.uni-stuttgart.de/handle/11682/11076 (accessed on 20 November 2023).
- Steurer, A.; Poser, R.; von Wolfersdorf, J.; Retzko, S. Application of the Transient Heat Transfer Measurement Technique Using Thermochromic Liquid Crystals in a Network Configuration with Intersecting Circular Passages. J. Turbomach. 2019, 141, 051010. [Google Scholar] [CrossRef]
- Jenkins, S.C.; Shevchuk, I.V.; von Wolfersdorf, J.; Weigand, B. Transient Thermal Field Measurements in a High Aspect Ratio Channel Related to Transient Thermochromic Liquid Crystal Experiments. J. Turbomach. 2012, 134, 031002. [Google Scholar] [CrossRef]
- Waidmann, C.; Poser, R.; von Wolfersdorf, J.; Fois, M.; Semmler, K. Investigations of heat transfer and pressure loss in an engine-similar two-pass internal blade cooling configuration. In Proceedings of the 10th European Conference on Turbomachinery Fluid dynamics & Thermodynamics, European Turnomachinery Society, Lappeenranta, Finland, 15–19 April 2013; Available online: https://www.euroturbo.eu/publications/proceedings-papers/etc2013-168 (accessed on 20 November 2023).
- Ireland, P.T.; Jones, T.V. Liquid crystal measurements of heat transfer and surface shear stress. Meas. Sci. Technol. 2000, 11, 969–986. [Google Scholar] [CrossRef]
- Poser, R.; von Wolfersdorf, J.; Semmler, K. Transient Heat Transfer Experiments in Complex Passages. In Proceedings of the HT2005 ASME Summer Heat Transfer Conference, San Francisco, CA, USA, 17–22 July 2005; Available online: https://asmedigitalcollection.asme.org/HT/proceedings-abstract/HT2005/47314/797/313393 (accessed on 20 November 2023).
- Poser, R.; von Wolfersdorf, J.; Lutum, E. Advanced evaluation of transient heat transfer experiments using thermochromic liquid crystals. Proc. Inst. Mech. Eng. Part J. Power Energy 2007, 221, 793–801. Available online: https://journals.sagepub.com/doi/10.1243/09576509JPE464 (accessed on 20 November 2023). [CrossRef]
- Poser, R.; Ferguson, J.R.; von Wolfersdorf, J. Temporal Signal Preprocessing and Evaluation of Thermochromic Liquid Crystal Indications in Transient Heat Transfer Experiments. In Proceedings of the 8th European Conference on Turbomachinery Fluid Dynamics and Thermodynamics, Graz, Austria, 23–27 March 2009; pp. 785–795. [Google Scholar]
- Poser, R.; von Wolfersdorf, J. Transient Liquid Crystal Thermography in Complex Internal Cooling Systems; VKI Lecture Series—Internal Cooling in Turbomachinery; von Karman Institute for Fluid Dynamics: Brussels, Belgium, 2010. [Google Scholar]
- Taler, J.; Duda, P. Solving Direct and Inverse Heat Conduction Problems; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Myers, G. Analytical Methods in Conduction Heat Transfer, 2nd ed.; Genium Publishing Corp.: New York, NY, USA, 1998. [Google Scholar]
- White, F.M.; Majdalani, J. Preliminary Concepts. In Viscous Fluid Flow; McGraw-Hill: New York, NY, USA, 2006; Volume 3, Chapter 1. [Google Scholar]
- ANSYS Inc. ANSYS CFX Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2021; Release 21.2. [Google Scholar]
- ANSYS Inc. ANSYS ICEM CFD User’s Manual; ANSYS Inc.: Canonsburg, PA, USA, 2016; Release 17.1. [Google Scholar]
- Incropera, F.P.; DeWitt, D.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer, 7th ed.; International Student version ed.; Wiley: Singapore, 2013. [Google Scholar]
- Williams, W.C. If the Dittus and Boelter equation is really the McAdams equation, then should not the McAdams equation really be the Koo equation? Int. J. Heat Mass Transf. 2011, 54, 1682–1683. [Google Scholar] [CrossRef]
- Liou, T.M.; Chen, C.C.; Chen, M.Y. Rotating effect on fluid flow in two smooth ducts connected by a 180-degree bend. J. Fluids Eng. 2003, 125, 138–148. [Google Scholar] [CrossRef]
- Crowder, S.; Delker, C.; Forrest, E.; Martin, N. Introduction to Statistics in Metrology; Springer: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- Forster, M.; Seibold, F.; Krille, T.; Waidmann, C.; Weigand, B.; Poser, R. A Monte Carlo approach to evaluate the local measurement uncertainty in transient heat transfer experiments using liquid crystal thermography. Measurement 2022, 190, 110648. [Google Scholar] [CrossRef]
- Brandrup, J.; Immergut, E.H.; Grulke, E.A.; Abe, A.; Bloch, D.R. Polymer Handbook; Wiley: New York, NY, USA, 1999; Volume 89. [Google Scholar]




| α | ||||
|---|---|---|---|---|
| +46.5° | +8° | −18.5° | ||
| 0 | X | X | X | |
| X | X | X | ||
| X | ||||
| X | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Published by MDPI on behalf of the EUROTURBO. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Gutiérrez de Arcos, D.; Waidmann, C.; Poser, R.; von Wolfersdorf, J.; Göhring, M. Rotationally Induced Local Heat Transfer Features in a Two-Pass Cooling Channel: Experimental–Numerical Investigation. Int. J. Turbomach. Propuls. Power 2024, 9, 34. https://doi.org/10.3390/ijtpp9040034
Gutiérrez de Arcos D, Waidmann C, Poser R, von Wolfersdorf J, Göhring M. Rotationally Induced Local Heat Transfer Features in a Two-Pass Cooling Channel: Experimental–Numerical Investigation. International Journal of Turbomachinery, Propulsion and Power. 2024; 9(4):34. https://doi.org/10.3390/ijtpp9040034
Chicago/Turabian StyleGutiérrez de Arcos, David, Christian Waidmann, Rico Poser, Jens von Wolfersdorf, and Michael Göhring. 2024. "Rotationally Induced Local Heat Transfer Features in a Two-Pass Cooling Channel: Experimental–Numerical Investigation" International Journal of Turbomachinery, Propulsion and Power 9, no. 4: 34. https://doi.org/10.3390/ijtpp9040034
APA StyleGutiérrez de Arcos, D., Waidmann, C., Poser, R., von Wolfersdorf, J., & Göhring, M. (2024). Rotationally Induced Local Heat Transfer Features in a Two-Pass Cooling Channel: Experimental–Numerical Investigation. International Journal of Turbomachinery, Propulsion and Power, 9(4), 34. https://doi.org/10.3390/ijtpp9040034






